Fiber Residual Stress Effects on Modal Gain Equalization of Few-Mode Fiber Amplifier
Abstract
1. Introduction
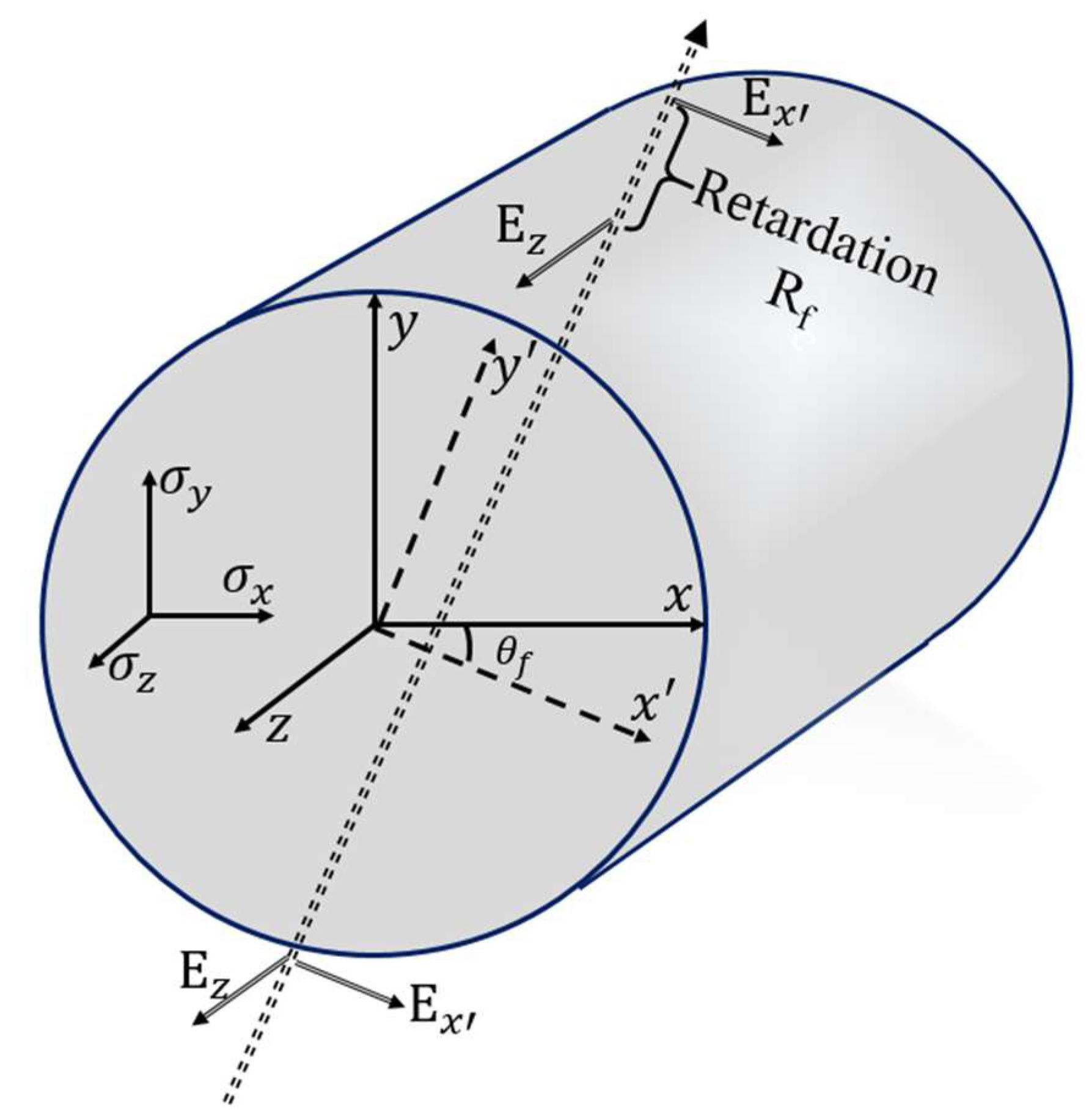
2. Principle
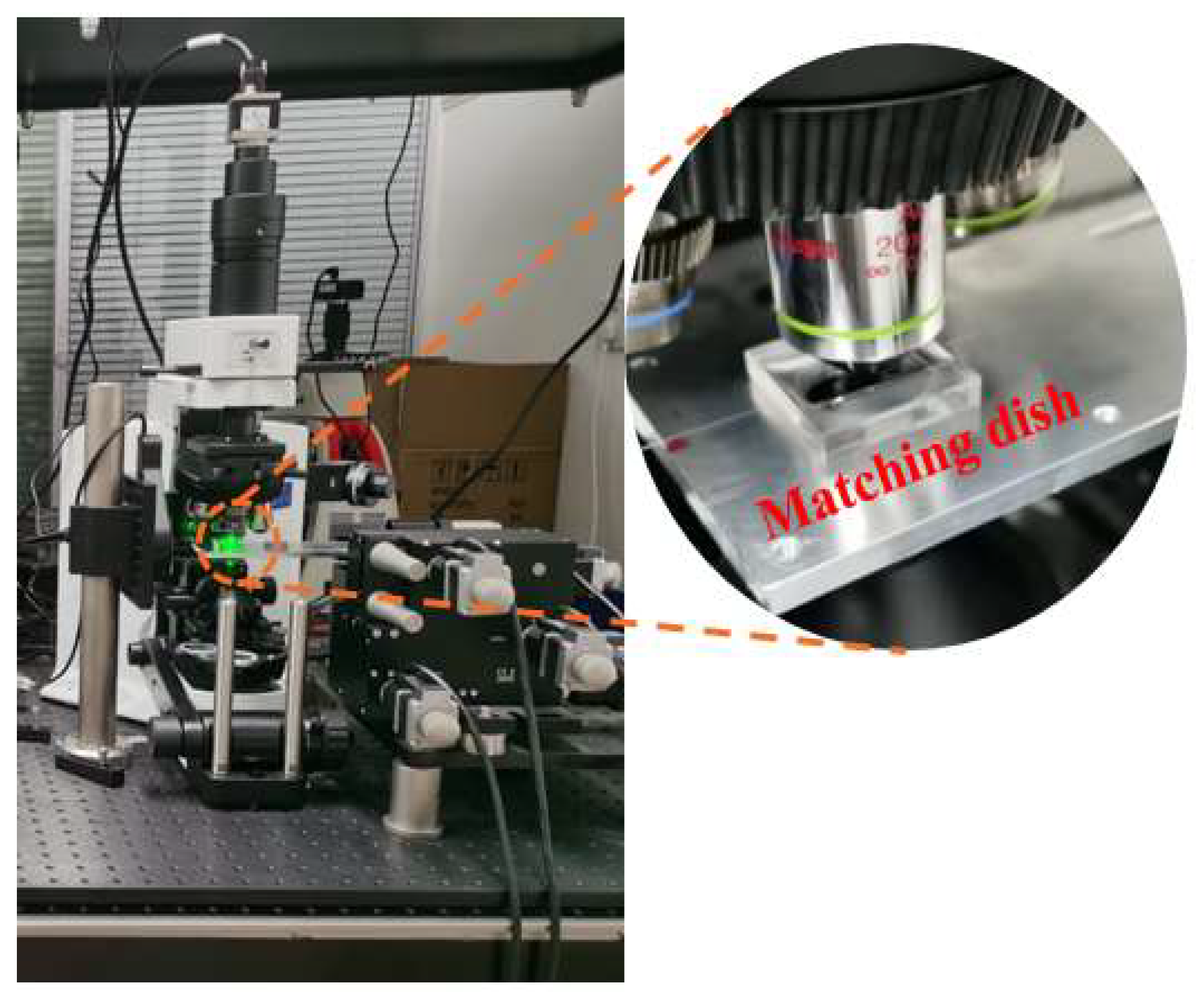
3. Experimental Measurement
4. Result
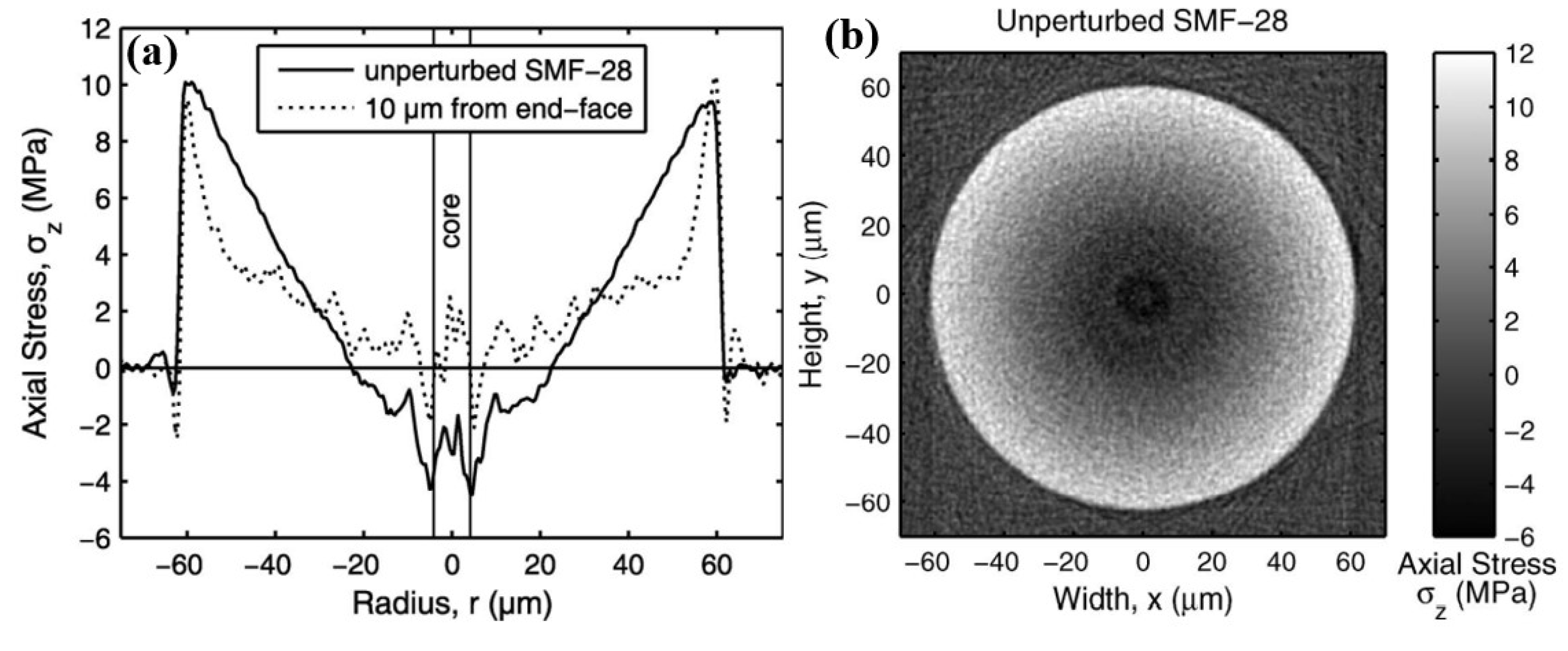
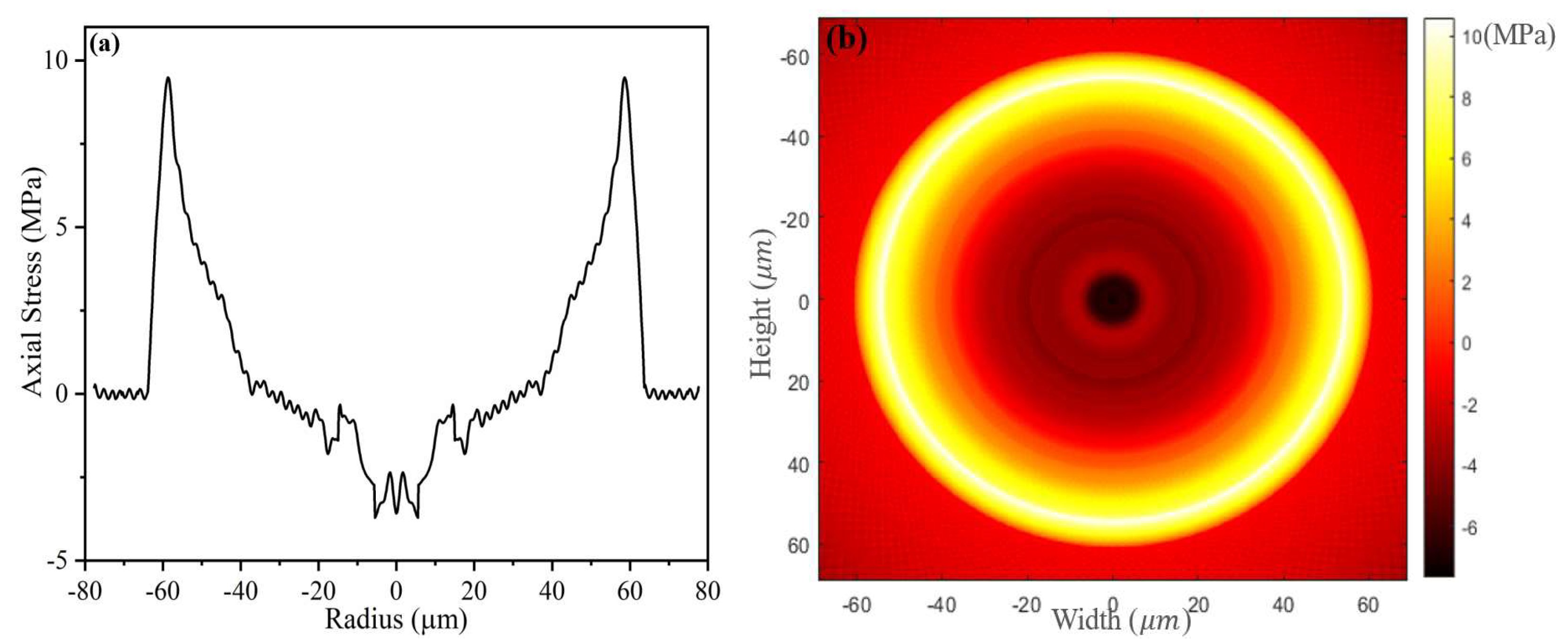
4.1. Passive FMF
4.2. FM-EDF-LAER
4.3. FM-EDF-HAER
5. Discussion
- (1)
- We assumed that there was an ideal FM-EDF-HAER, but with residual stress distribution and RI profile being consistent with those of the passive FMF, and marked it as the ideal fiber. In this case, the calculated modal gains and DMG as a function of wavelength are shown in Figure 8a. In the wavelength range of 1530~1565 nm, the DMG reached the maximum of 0.96 dB at 1565 nm, and the maximum DMG appeared between LP02 and LP01.
- (2)
- The experimental RI profile of the FM-EDF-LAER was analyzed with FMFA theory to calculate the DMG. The corresponding results are shown in Figure 8b. Since the fiber had been doped with a lower EDC, the maximum signal modal gain was 18.35 dB, which was lower than the maximum modal gain (about 20.03 dB) of the ideal fiber. In the C-band range, the DMG reached the maximum of 1.34 dB at 1565 nm, and the maximum DMG appeared between LP02 and LP11.
- (3)
- The experimental RI profile of the FM-EDF-HAER was analyzed with FMFA theory to calculate the DMG, and the corresponding results are shown in Figure 8c. The maximum signal modal gain of this fiber was 20.39 dB. Similarly, as the wavelength continued to increase, the DMG at 1565 nm reached a maximum of 1.67 dB. Moreover, the maximum DMG appeared between LP11 and LP21.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Richardson, D.J.; Fini, J.M.; Nelson, L.E. Space-division multiplexing in optical fibres. Nat. Photonics 2013, 7, 354–362. [Google Scholar] [CrossRef]
- Li, G.; Bai, N.; Zhao, N.; Xia, C. Space-division multiplexing: The next frontier in optical communication. Adv. Opt. Photonics 2014, 6, 413–487. [Google Scholar] [CrossRef]
- Ho, K.-P.; Kahn, J.M. Mode-dependent loss and gain: Statistics and effect on mode-division multiplexing. Opt. Express 2011, 19, 16612–16635. [Google Scholar] [CrossRef] [PubMed]
- Ryf, R.; Randel, S.; Gnauck, A.H.; Bolle, C.; Essiambre, R.J.; Winzer, P.J.; Peckham, D.W.; McCurdy, A.; Lingle, R. Space-division multiplexing over 10 km of three-mode fiber using coherent 6 × 6 MIMO processing. In Proceedings of the Optical Fiber Communication Conference and Exposition and the National Fiber Optic Engineers Conference 2011, Los Angeles, CA, USA, 6–10 March 2011; pp. 1–3. [Google Scholar]
- Jung, Y.; Alam, S.; Li, Z.; Dhar, A.; Giles, D.; Giles, I.P.; Sahu, J.K.; Poletti, F.; Grüner-Nielsen, L.; Richardson, D.J. First demonstration and detailed characterization of a multimode amplifier for space division multiplexed transmission systems. Opt. Express 2011, 19, B952–B957. [Google Scholar] [CrossRef]
- Savović, S.; Djordjevich, A.; Savović, I. Theoretical investigation of bending loss in step-index plastic optical fibers. Opt. Commun. 2020, 475, 126200. [Google Scholar] [CrossRef]
- Ge, D.; Li, J.; Zhu, J.; Shen, L.; Gao, Y.; Yu, J.; Wu, Z.; Li, Z.; Chen, Z.; He, Y. Design of a Weakly-Coupled Ring-Core FMF and Demonstration of 6-mode 10-km IM/DD Transmission. In Proceedings of the Optical Fiber Communications Conference and Exposition (OFC) 2018, San Diego, CA, USA, 11–15 March 2018; pp. 1–3. [Google Scholar]
- Zhao, Q.; Pei, L.; Zheng, J.J.; Wang, J.S.; Xie, Y.H.; Li, J.; Ning, T.G. Demonstration of a ring-core few-mode erbium-doped fiber for mode gain equalization based on layered doping. J. Opt. Soc. Am. B Opt. Phys. 2022, 39, 1972–1978. [Google Scholar] [CrossRef]
- Chang, Y.; Pei, L.; Zheng, J.; Wang, J.; He, Q.; Li, J.; Ning, T.; Zhong, L. Demonstration of an all-fiber cladding-pumped FM-EDFA with low differential modal gain. Opt. Laser Technol. 2022, 155, 108446. [Google Scholar] [CrossRef]
- Li, Z.; Pei, L.; Wang, J.; Zheng, J.; Xu, W.; Ning, T.; Li, J. A uniform ion doping air-hole-assisted FM-EDF for low differential modal gain. Opt. Fiber Technol. 2023, 75, 103196. [Google Scholar] [CrossRef]
- Li, Z.Q.; Pei, L.; Zheng, J.J.; Wang, J.S.; Xu, W.X.; Ning, T.G.; Li, J. A Multi-Layer Erbium-Doped Air-Hole-Assisted Few-Mode Fiber with Ultra-Low Differential Modal Gain. Photonics 2022, 9, 305. [Google Scholar] [CrossRef]
- Hibino, Y.; Hanawa, F.; Horiguchi, M. Drawing-induced residual stress effects on optical characteristics in pure-silica-core single-mode fibers. J. Appl. Phys. 1989, 65, 30–34. [Google Scholar] [CrossRef]
- Raine, K.W.; Feced, R.; Kanellopoulos, S.E.; Handerek, V.A. Measurement of axial stress at high spatial resolution in ultraviolet-exposed fibers. Appl. Opt. 1999, 38, 1086–1095. [Google Scholar] [CrossRef]
- Park, Y.; Paek, U.-C.; Kim, D.Y. Complete determination of the stress tensor of a polarization-maintaining fiber by photoelastic tomography. Opt. Lett. 2002, 27, 1217–1219. [Google Scholar] [CrossRef] [PubMed]
- Montarou, C.C.; Gaylord, T.K.; Bachim, B.L.; Dachevski, A.I.; Agarwal, A. Two-wave-plate compensator method for full-field retardation measurements. Appl. Opt. 2006, 45, 271–280. [Google Scholar] [CrossRef] [PubMed]
- Hutsel, M.R.; Ingle, R.R.; Gaylord, T.K. Technique and Apparatus for Accurate Cross-Sectional Stress Profiling of Optical Fibers. IEEE Trans. Instrum. Meas. 2011, 60, 971–979. [Google Scholar] [CrossRef]
- Feng, T.; Jenkins, M.H.; Yan, F.; Gaylord, T.K. Arc fusion splicing effects in large-mode-area single-mode ytterbium-doped fibers. Appl. Opt. 2013, 52, 7706–7711. [Google Scholar] [CrossRef] [PubMed]
- Feng, T.; Jenkins, M.H.; Yan, F.; Gaylord, T.K. Joint Residual Stress/Refractive Index Characterization of Large-Mode-Area Erbium-Doped Fibers. J. Light. Technol. 2013, 31, 2426–2433. [Google Scholar] [CrossRef]
- Wang, P.; Jenkins, M.H.; Gaylord, T.K. Arc-discharge effects on residual stress and refractive index in single-mode optical fibers. Appl. Opt. 2016, 55, 2451–2456. [Google Scholar] [CrossRef]
- Anuszkiewicz, A.; Bidus, M.; Filipkowski, A.; Pysz, D.; Dlubek, M.; Buczynski, R. Experimental analysis of axial stress distribution in nanostructured core fused silica fibers. Opt. Mater. Express 2019, 9, 4370–4378. [Google Scholar] [CrossRef]
- Hoffman, J.; Khadka, S.; Kumosa, M. Determination of gel point and completion of curing in a single fiber/polymer composite. Compos. Sci. Technol. 2020, 188, 107997. [Google Scholar] [CrossRef]
- Khadka, S.; Kumosa, M.; Hoffman, J. Determination of residual stresses in a single FBG fiber/epoxy composite system. Compos. Sci. Technol. 2022, 218, 109138. [Google Scholar] [CrossRef]
- Hutsel, M.R.; Ingle, R.; Gaylord, T.K. Accurate cross-sectional stress profiling of optical fibers. Appl. Opt. 2009, 48, 4985–4995. [Google Scholar] [CrossRef] [PubMed]
- Chu, P.L.; Whitbread, T. Measurement of stresses in optical fiber and preform. Appl. Opt. 1982, 21, 4241–4245. [Google Scholar] [CrossRef] [PubMed]
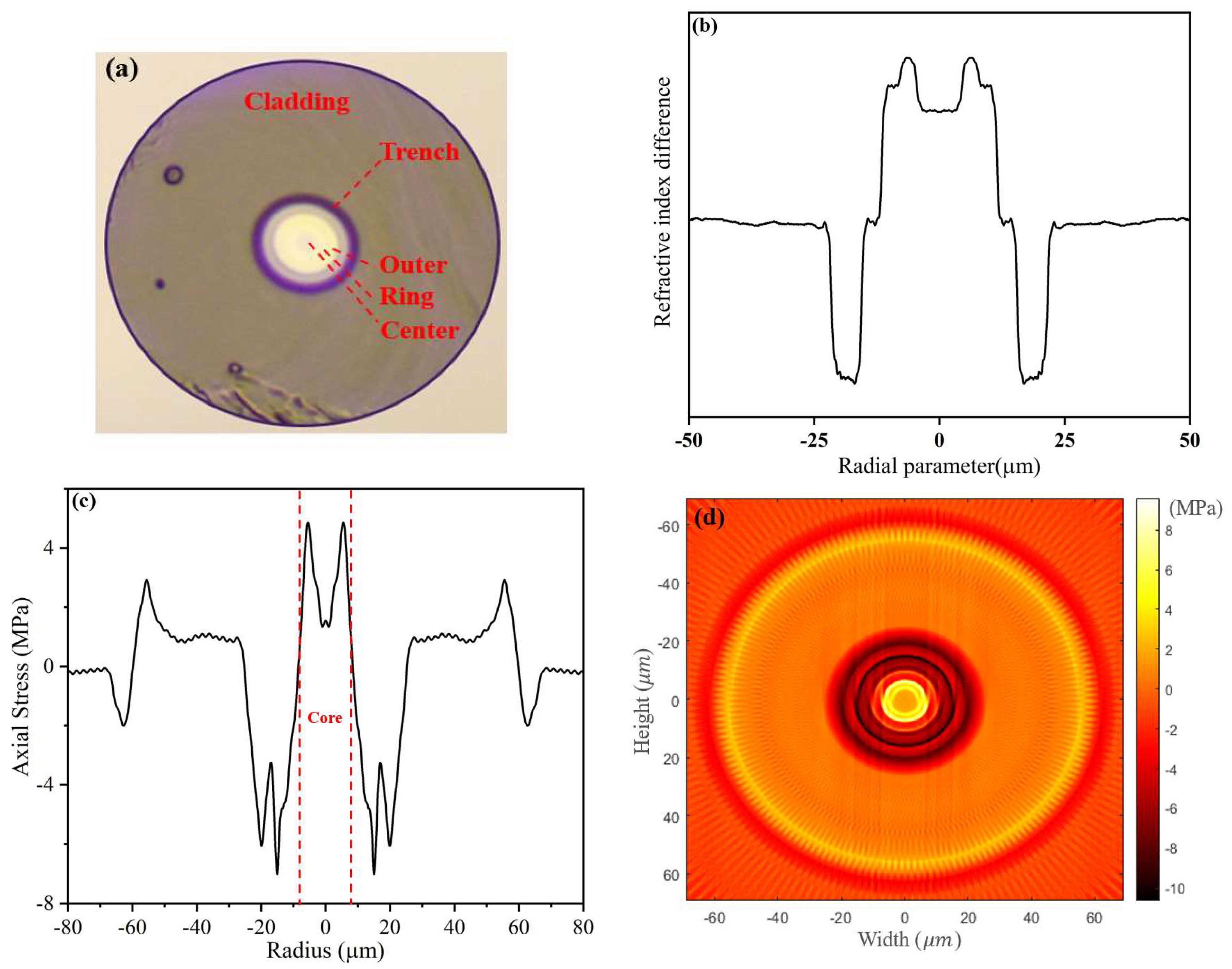
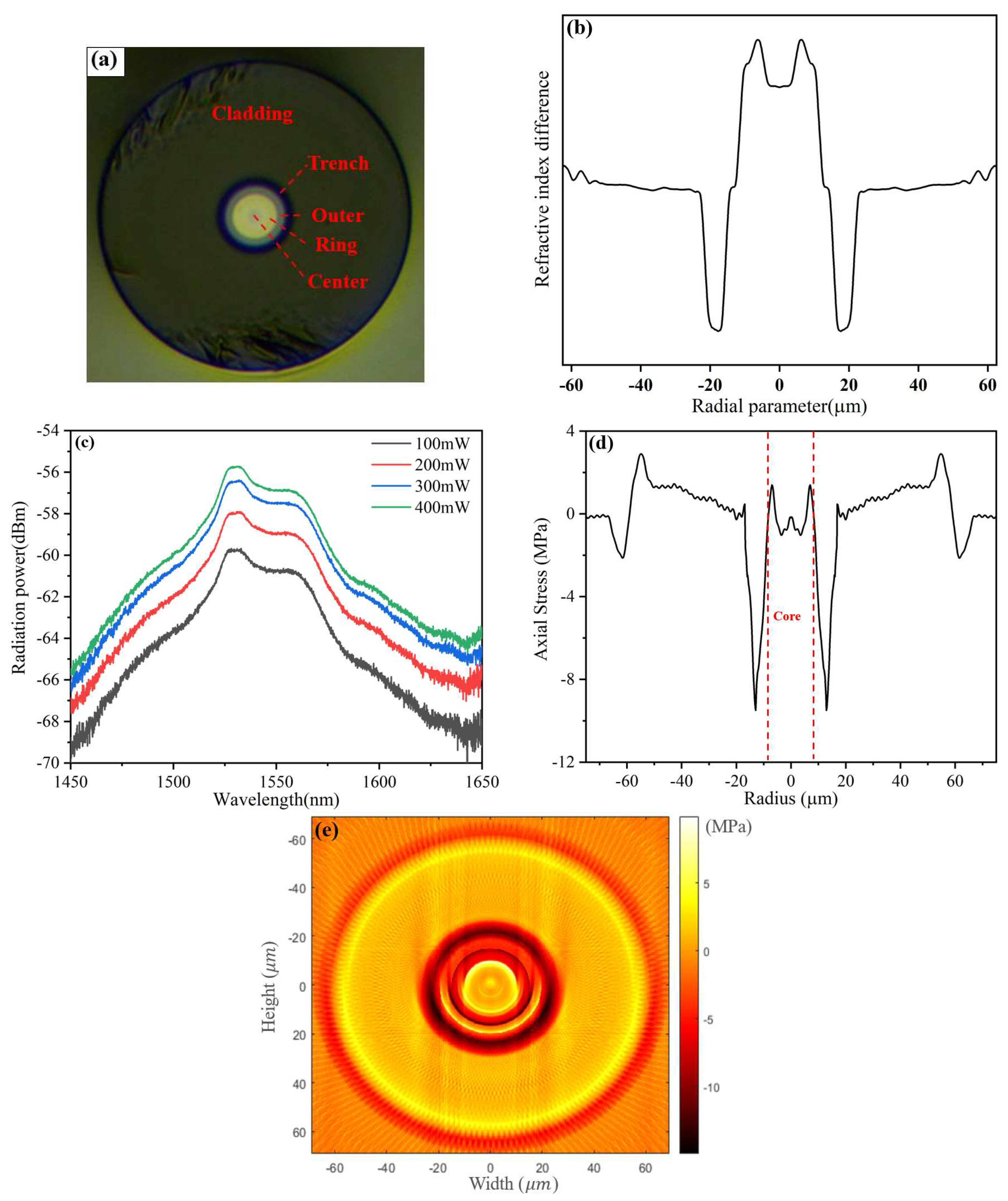
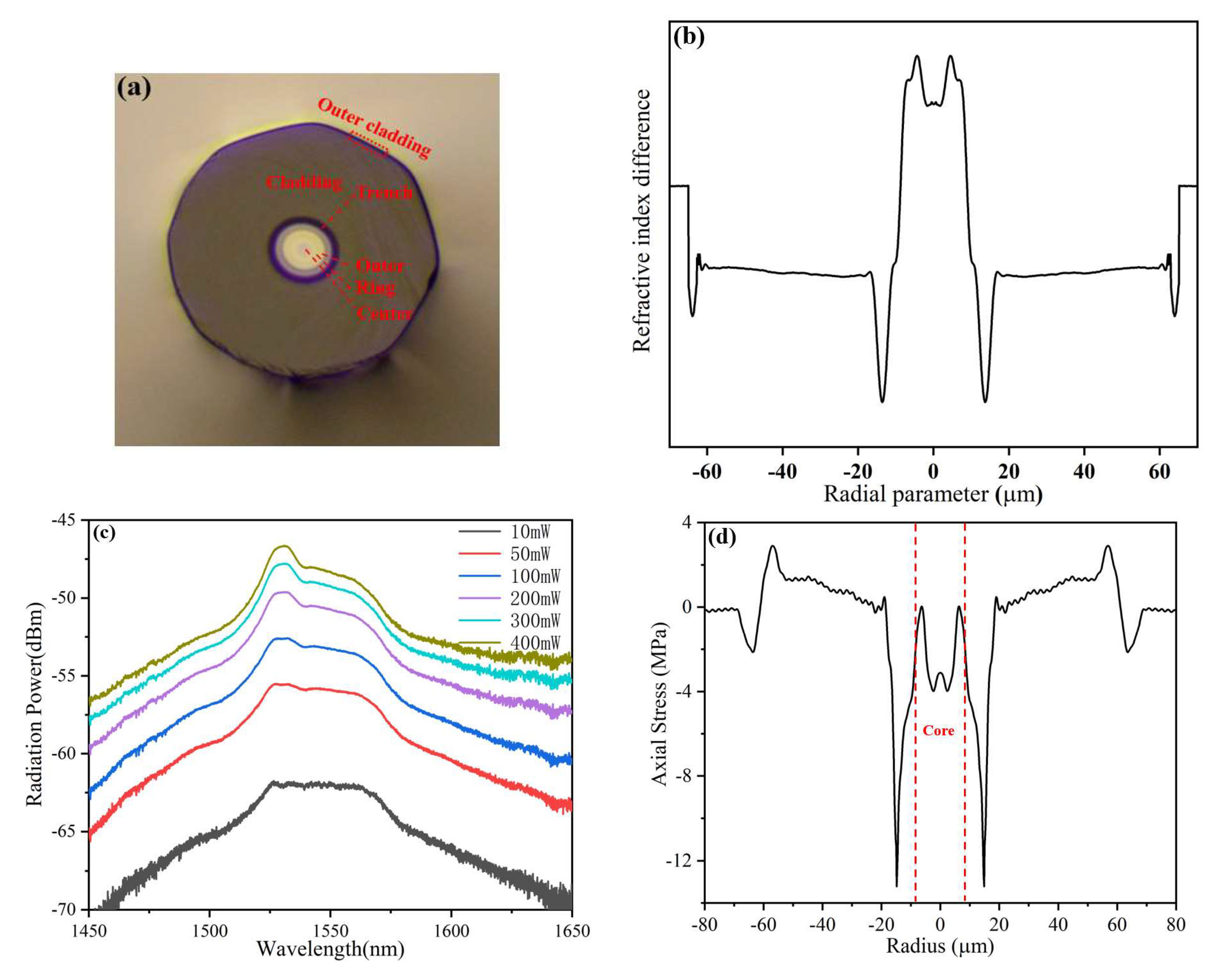
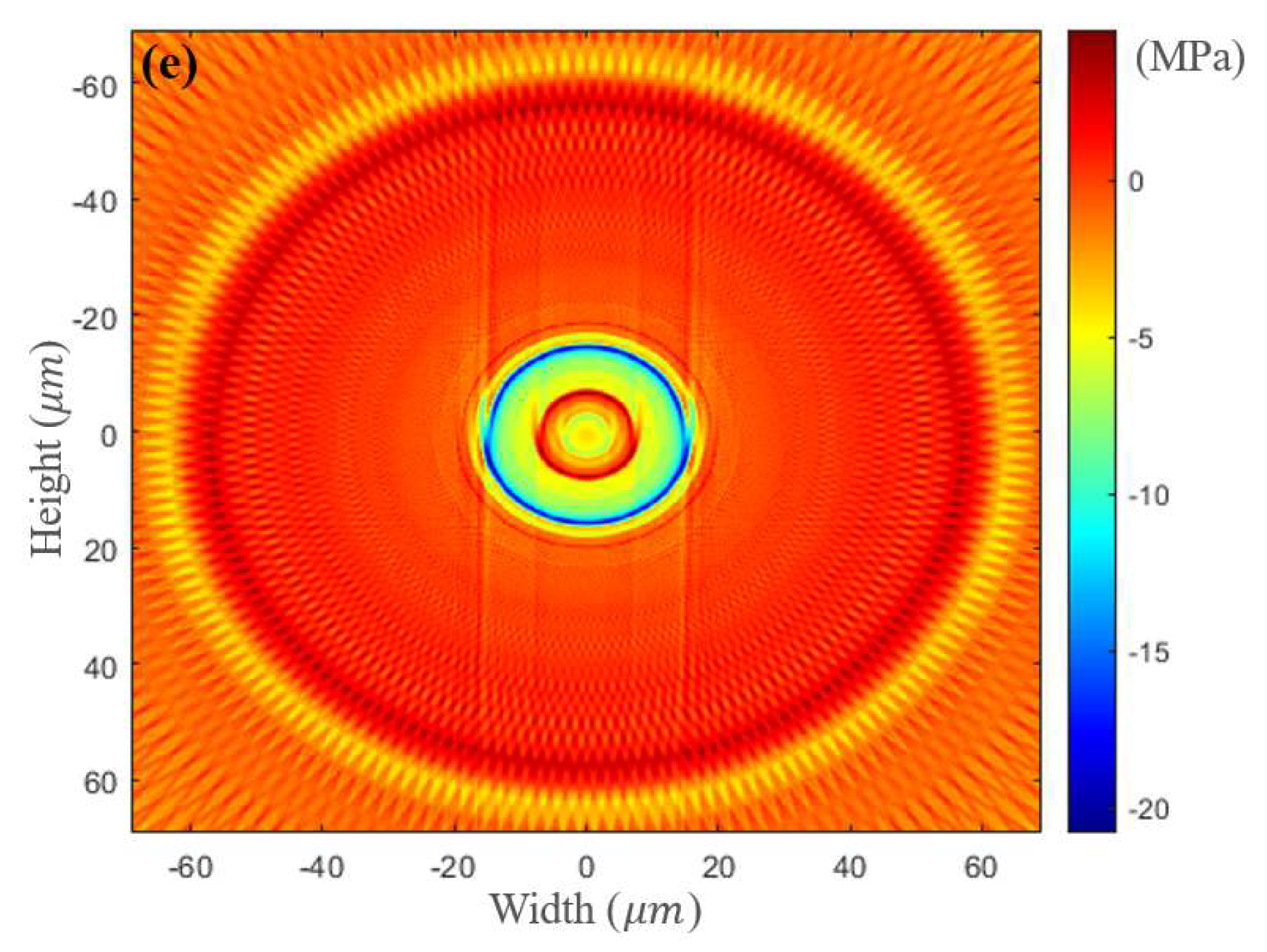

| Fiber | Maximum SEP (dBm) | Peak Residual Stress in Core (MPa) | Property of Residual Stress in Core | RI Change | DMG (dB) |
|---|---|---|---|---|---|
| Ideal fiber | −46.70 | 4.86 | Tensile stress | Broken layer transition | 0.96 |
| FM-EDF-LAER | −55.76 | 1.39 | Partial transformation to compressive stress | Gradually smooth curve layer transition | 1.34 |
| FM-EDF-HAER | −46.70 | 0.01 | Compressive stress | Totally smooth curve layer transition | 1.67 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pei, L.; Chang, Y.; Wang, J.; Zheng, J.; Ning, T.; Li, J.; Bai, B.; Shen, L.; Zhong, L. Fiber Residual Stress Effects on Modal Gain Equalization of Few-Mode Fiber Amplifier. Sensors 2023, 23, 2574. https://doi.org/10.3390/s23052574
Pei L, Chang Y, Wang J, Zheng J, Ning T, Li J, Bai B, Shen L, Zhong L. Fiber Residual Stress Effects on Modal Gain Equalization of Few-Mode Fiber Amplifier. Sensors. 2023; 23(5):2574. https://doi.org/10.3390/s23052574
Chicago/Turabian StylePei, Li, Yanbiao Chang, Jianshuai Wang, Jingjing Zheng, Tigang Ning, Jing Li, Bing Bai, Lei Shen, and Li Zhong. 2023. "Fiber Residual Stress Effects on Modal Gain Equalization of Few-Mode Fiber Amplifier" Sensors 23, no. 5: 2574. https://doi.org/10.3390/s23052574
APA StylePei, L., Chang, Y., Wang, J., Zheng, J., Ning, T., Li, J., Bai, B., Shen, L., & Zhong, L. (2023). Fiber Residual Stress Effects on Modal Gain Equalization of Few-Mode Fiber Amplifier. Sensors, 23(5), 2574. https://doi.org/10.3390/s23052574







