A New Gain-Phase Error Pre-Calibration Method for Uniform Linear Arrays
Abstract
1. Introduction
2. Problem Formulation
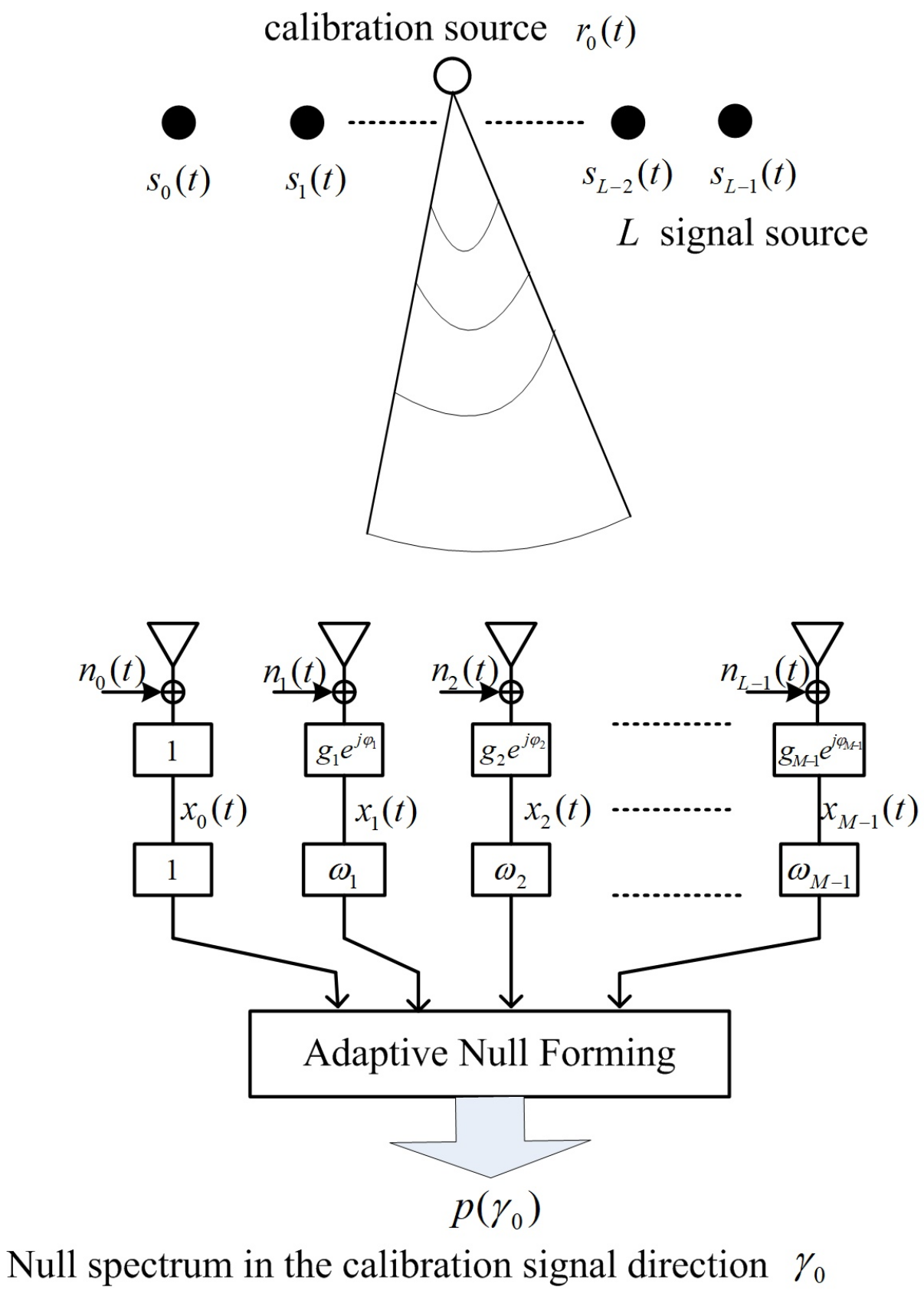
2.1. Signal Model
2.2. Adaptive Antenna Nulling Technique
3. Proposed Method
| Algorithm 1 Proposed gain-phase error estimation method |
|
4. Estimation of the SAUNSV
4.1. EIV Model for the SAUNSV Estimation
4.2. Proposed WTLS Algorithm
| Algorithm 2 Proposed WTLS |
|
4.3. Solution to the WTLS Problem
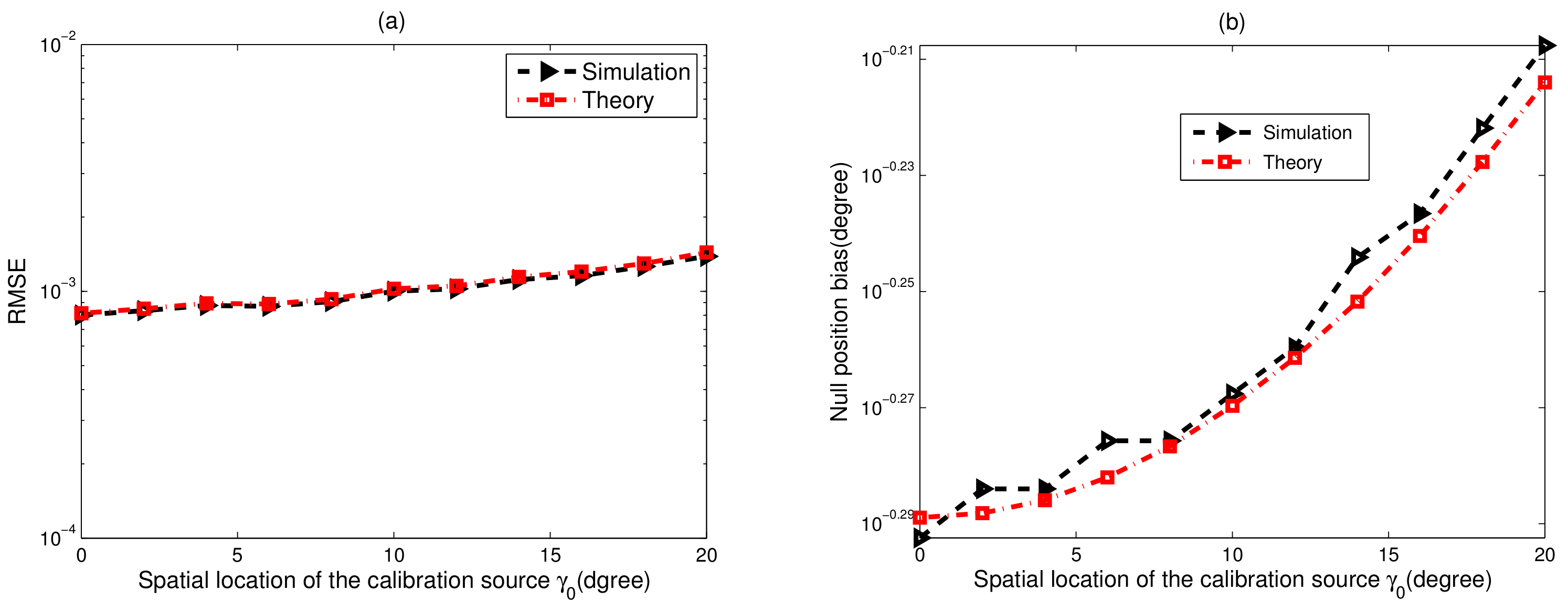
4.4. Spatial Location of the Calibration Source
5. Simulation Results and Discussion
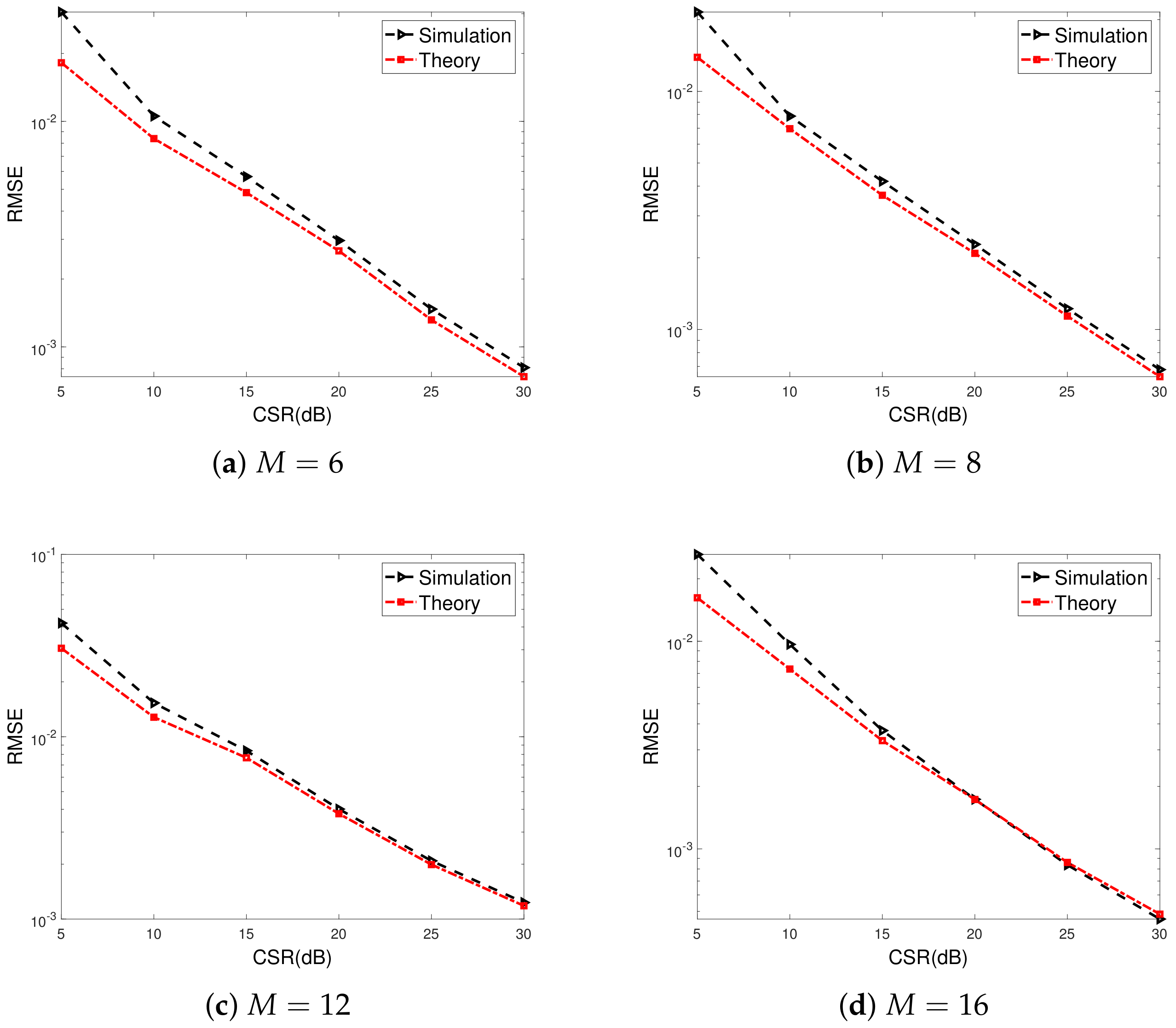
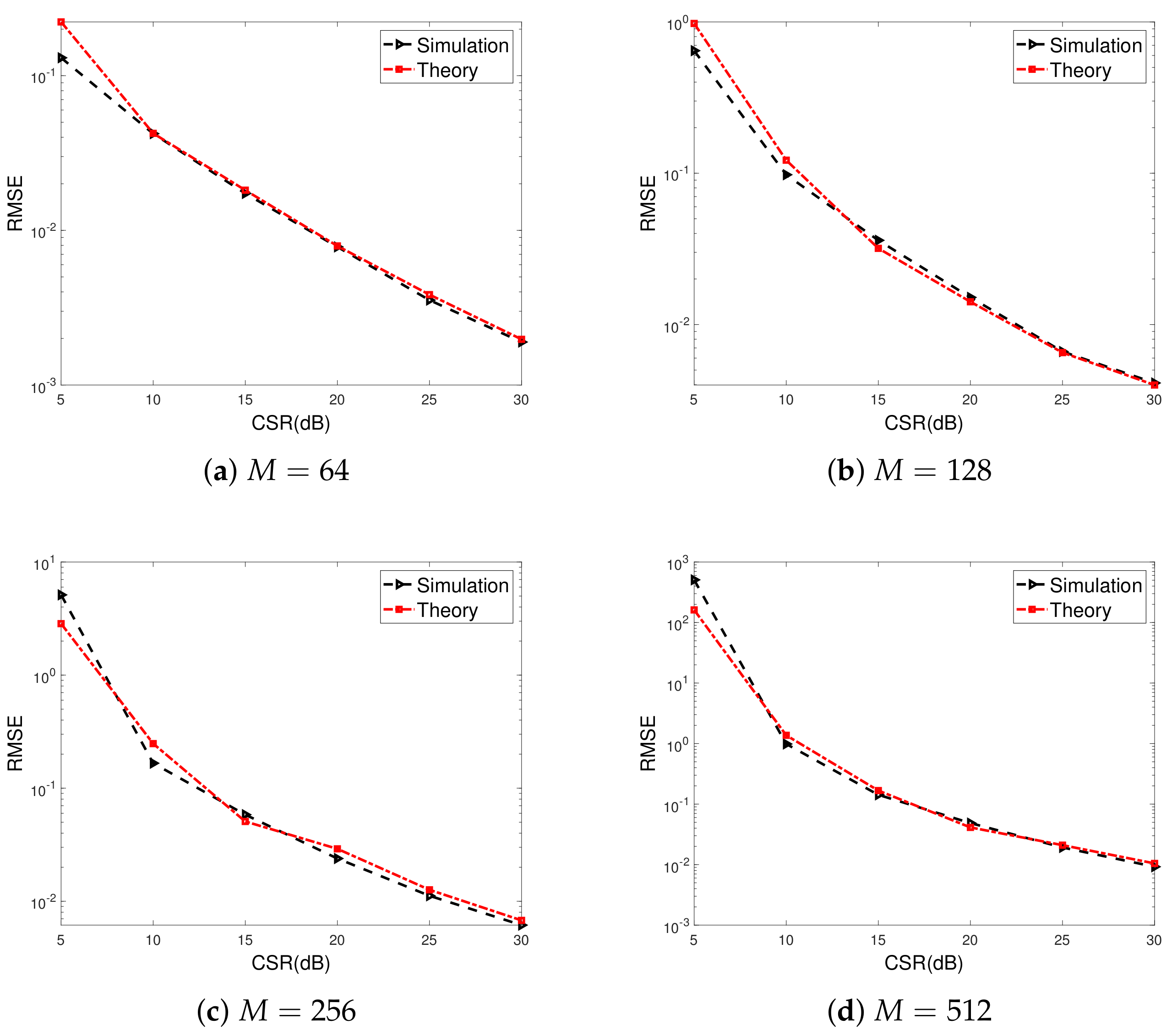
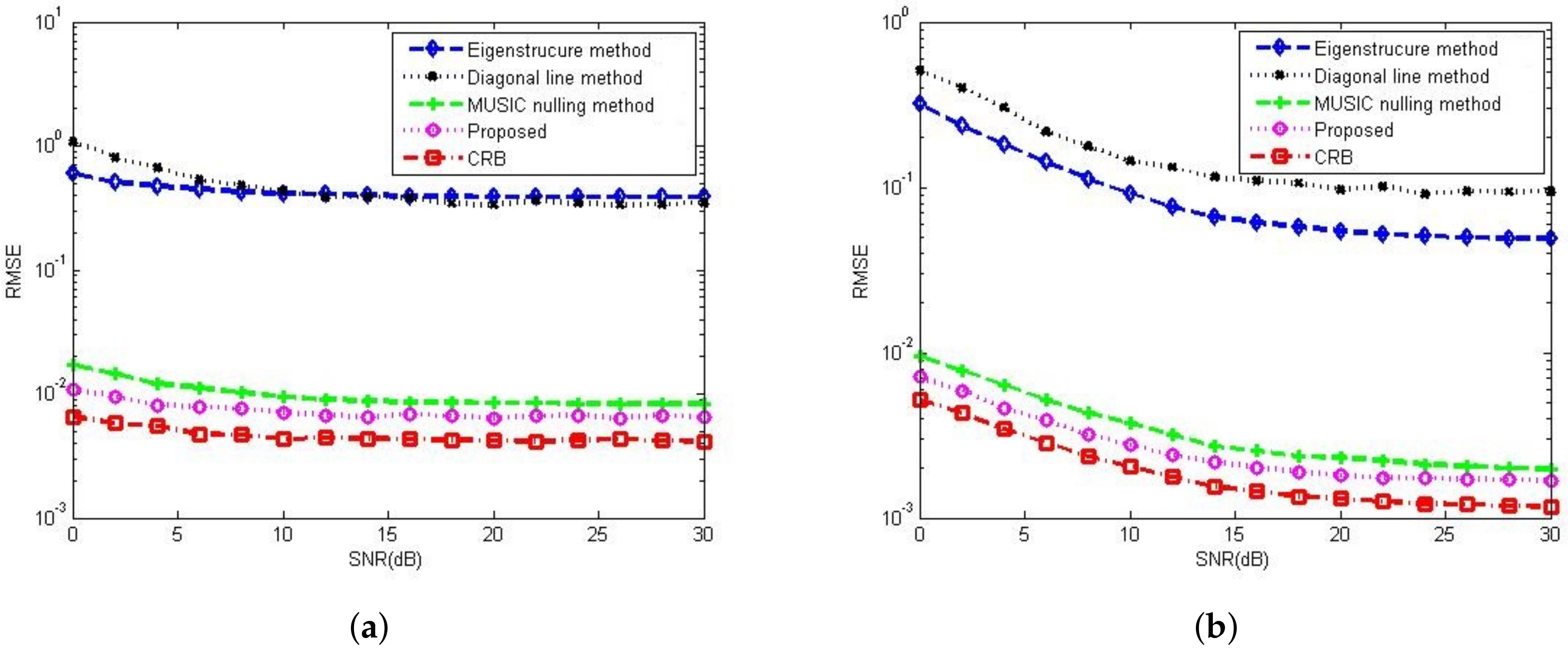
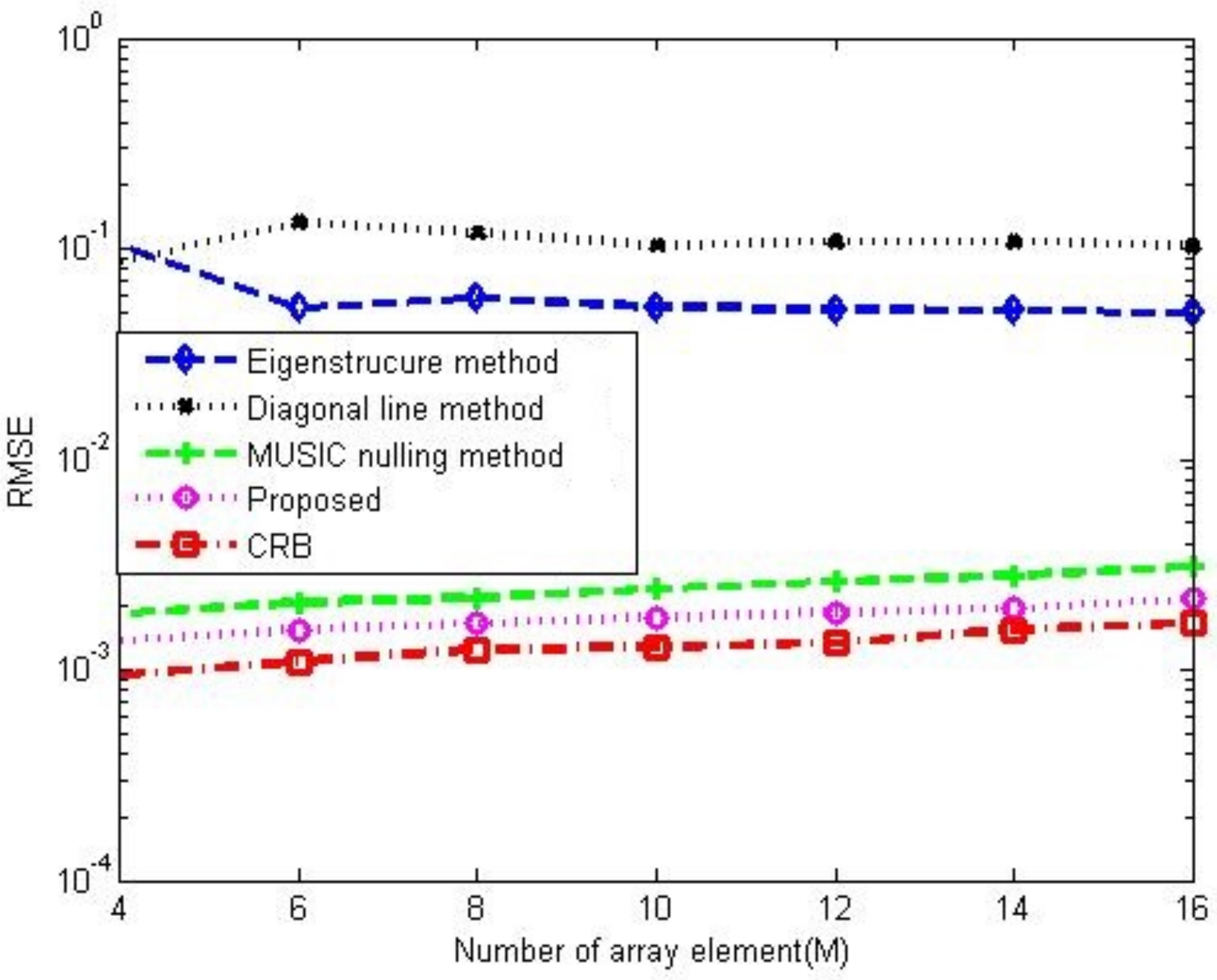
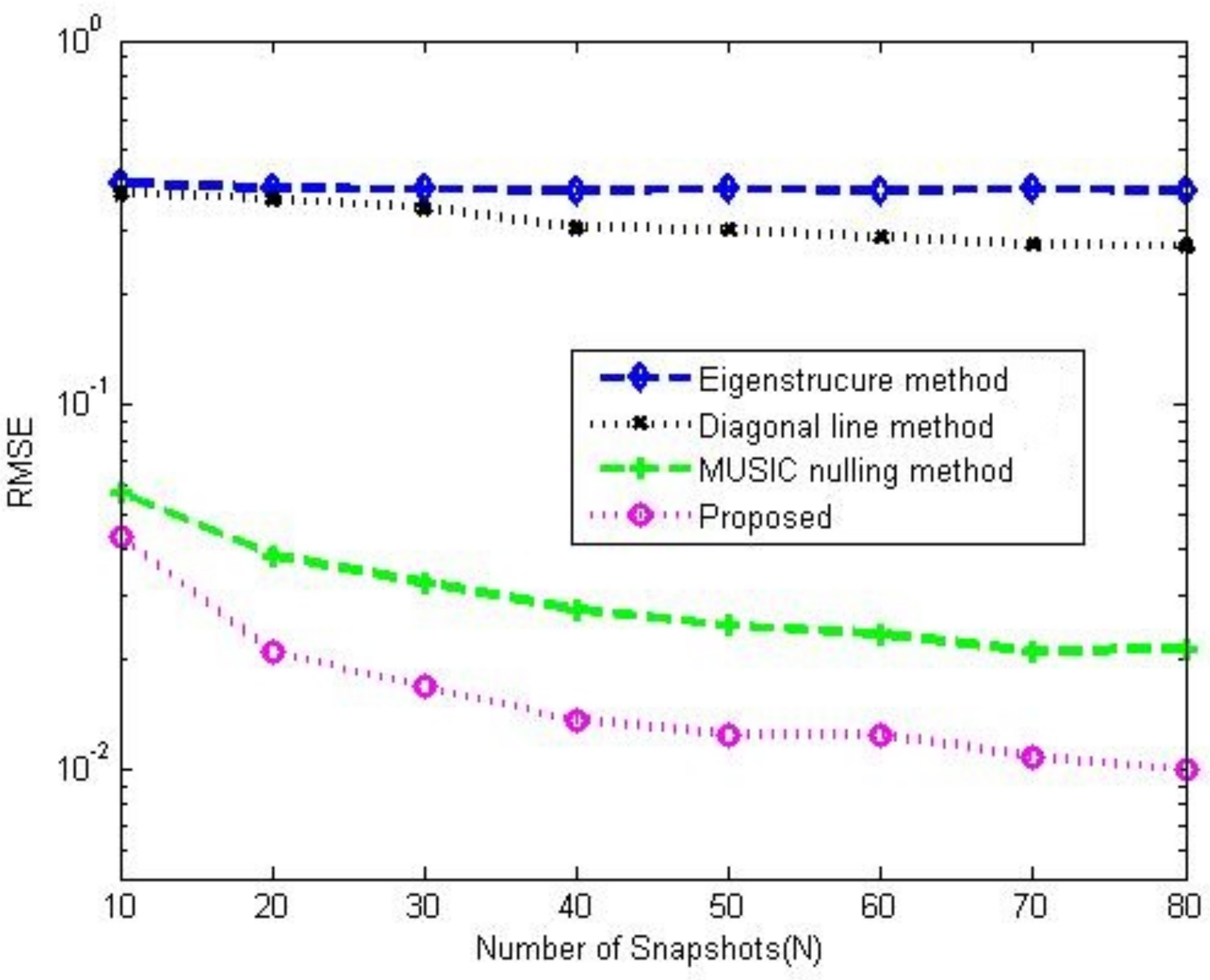
5.1. Gain-Phase Error Estimation Performance
5.2. Comparison to Other Calibration Methods
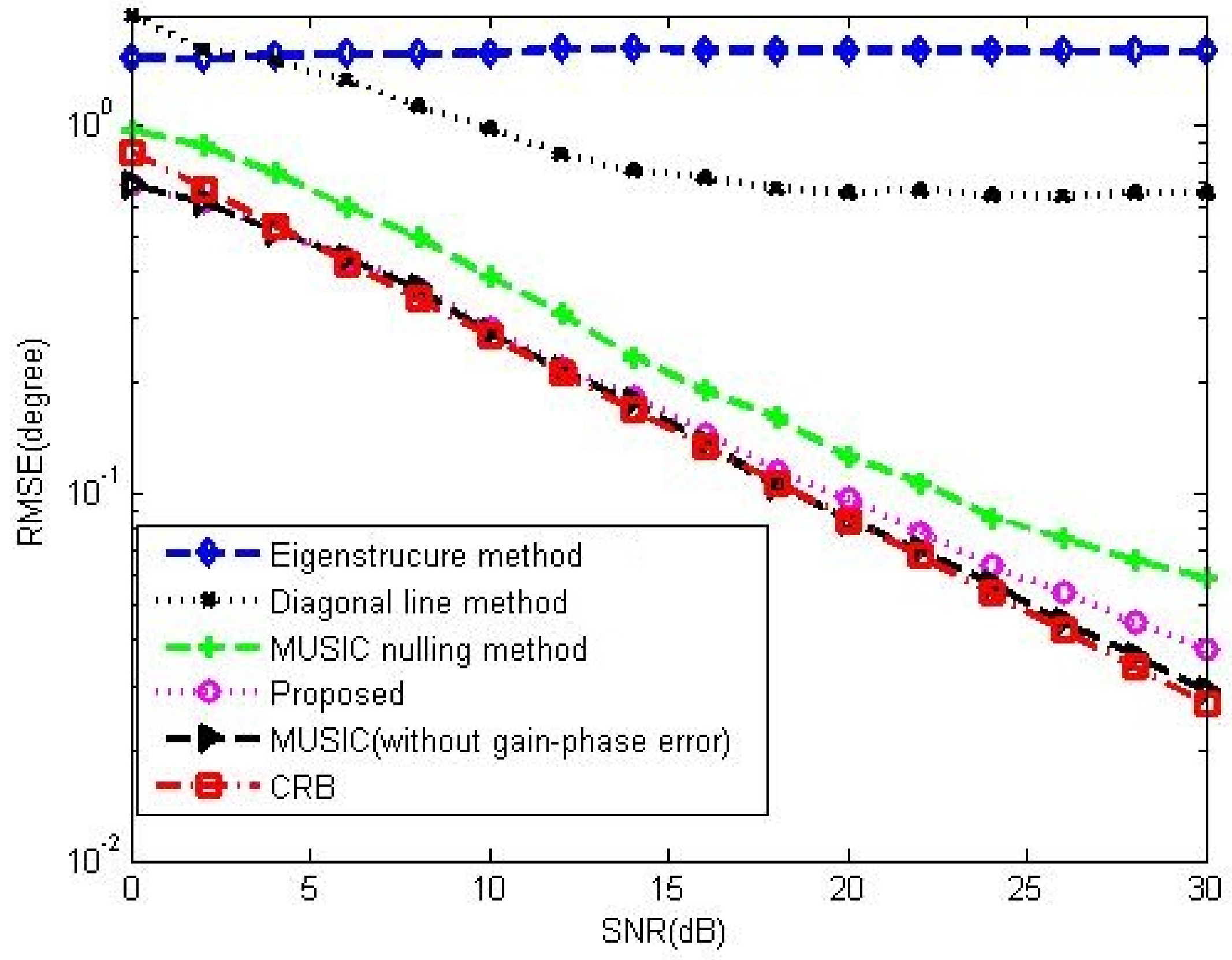
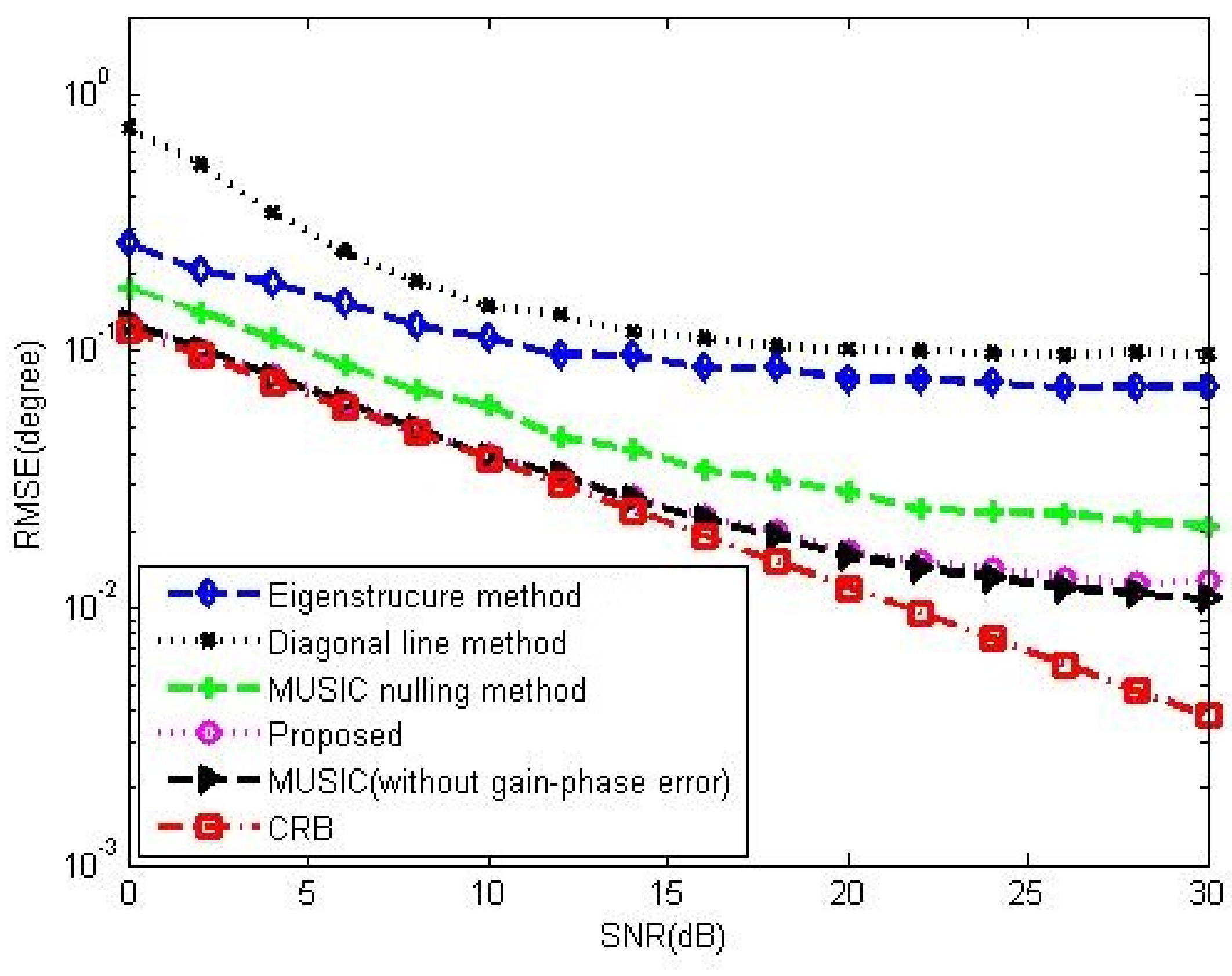
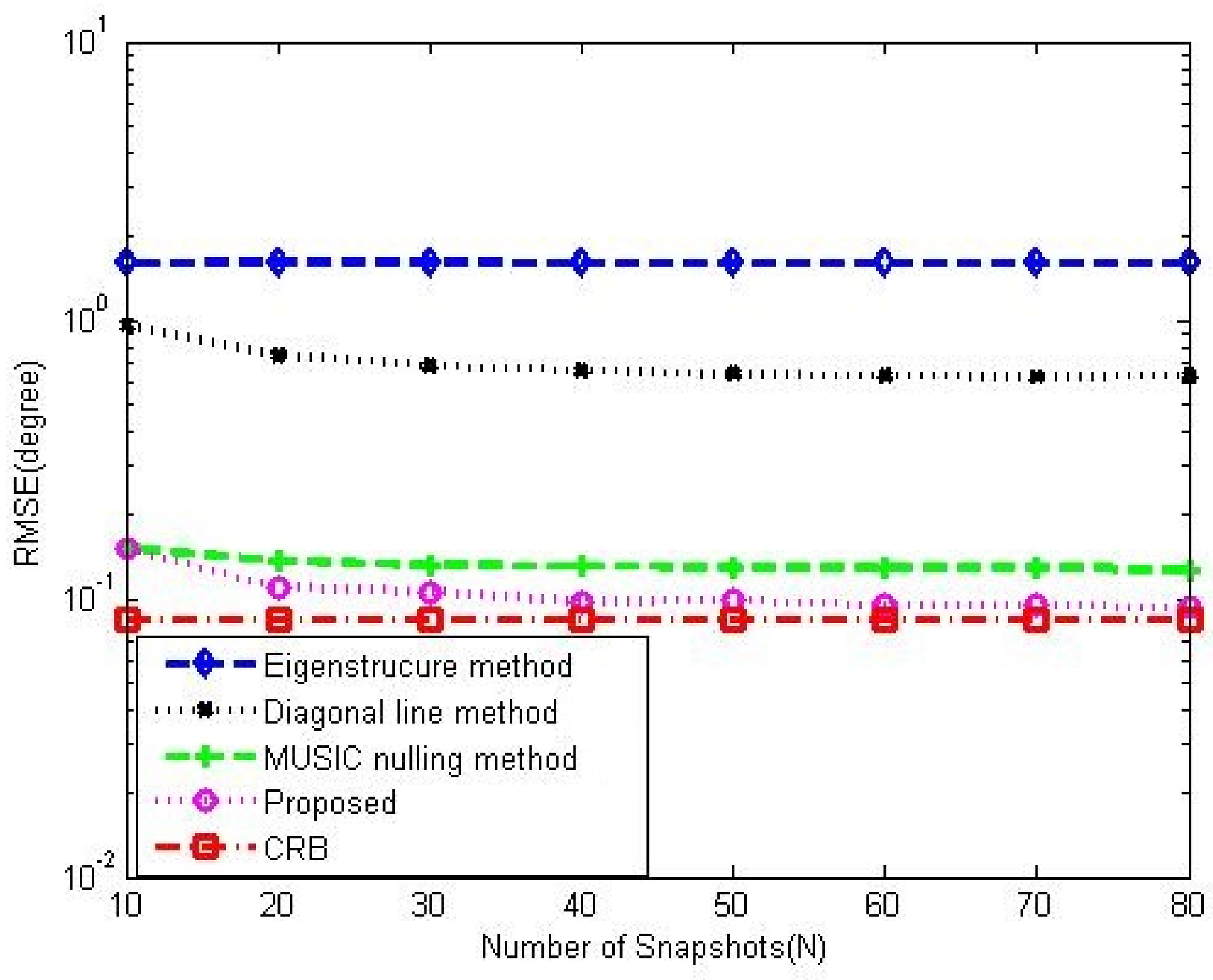
5.3. DOA Estimation Performance
5.4. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| ULAs | Uniform linear arrays |
| DOA | Direction of arrival |
| EIV | Errors-in-variables |
| WTLS | Weighted total least-squares |
| MUSIC | Multiple signal classification |
| ESPRIT | Estimating signal parameters via rotational invariance technique |
| ML | Maximum likelihood |
| CS | Compressed sensing |
| SBL | Sparse Bayesian learning |
| SAUNSV | Sub-array unperturbed null steering vector |
| CSR | Calibration signal-to-source signal ratio |
| RMSE | Root-mean-square error |
| LEA | Linear equispaced arrays |
| SBAC | Sparse Bayesian array calibration |
| CSA | Central symmetric arrays |
| GESPR | Greedy sparse phase retrieval |
| QR-RLS | QR-recursive least squares |
| CLMS | Constrained least mean square |
| CSR | Calibration signal-to-source signal power ratio |
| UMA | Unconditional-model assumption |
| CRB | Cramer–Rao bound |
| PCA | Principal component analysis |
Appendix A
Appendix B
References
- Krim, H.; Viberg, M. Two decades of array signal processing research: The parametric approach. IEEE Signal Process. Mag. 1996, 13, 66–94. [Google Scholar] [CrossRef]
- So, H.C. Source Localization: Algorithms and Analysis, Handbook of Position Location: Theory, Practice and Advances; Chapter 2; Zekavat, S.A., Buehrer, M., Eds.; Wiley-IEEE Press: Hoboken, NJ, USA, 2011. [Google Scholar]
- Schmidt, R.O. Multiple emitter location and signal parameters estimation. IEEE Trans. Antennas Propag. 1986, 34, 267–280. [Google Scholar] [CrossRef]
- Roy, R.; Kailath, T. ESPRIT-estimation of signal parameters via rotational invariance techniques. IEEE Trans. Acoust. Speech Signal Process. 1989, 37, 984–995. [Google Scholar] [CrossRef]
- Qiao, H.; Pal, P. On maximum-likelihood methods for localizing more sources than sensors. IEEE Signal Process. Lett. 2017, 24, 703–706. [Google Scholar] [CrossRef]
- Zhang, X.; Jiang, T.; Li, Y.; Liu, X. An off-grid DOA estimation method using proximal splitting and successive nonconvex sparsity approximation. IEEE Access 2019, 7, 66764–66773. [Google Scholar] [CrossRef]
- Dai, J.; So, H.C. Sparse Bayesian learning approach for outlier-resistant direction-of-arrival estimation. IEEE Trans. Signal Process. 2018, 66, 744–756. [Google Scholar] [CrossRef]
- Liao, B.; Chan, S.C. Direction finding in partly calibrated uniform linear arrays with unknown gains and phases. IEEE Trans. Aerosp. Electron. Syst. 2015, 51, 217–227. [Google Scholar] [CrossRef]
- Xu, J.; Wang, B.; Hu, F. Near-field sources localization in partly calibrated sensor arrays with known gains and phases. IEEE Wireless Commun. Lett. 2019, 8, 89–92. [Google Scholar] [CrossRef]
- Rocca, P.; Hannan, M.A.; Salucci, M.; Massa, A. Single-snapshot DoA estimation in array antennas with mutual coupling through a multiscaling BCS strategy. IEEE Trans. Antennas Propag. 2017, 65, 3203–3213. [Google Scholar] [CrossRef]
- Zhang, X.; Jiang, T.; Li, Y.; Zakharov, Y. A novel block sparse reconstruction method for DOA estimation with unknown mutual coupling. IEEE Comm. Lett. 2019, 23, 1845–1848. [Google Scholar] [CrossRef]
- Wang, W.; Wu, R.; Liang, J.; So, H.C. Phase retrieval approach for DOA estimation with array errors. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 217–227. [Google Scholar] [CrossRef]
- Weiss, A.J.; Friedlander, B. Eigenstructure methods for direction finding with sensor gain and phase uncertainties. Circuits. Syst. Signal Process. 1990, 9, 271–300. [Google Scholar] [CrossRef]
- Friedlander, B.; Weiss, A.J. Performance of direction-finding systems with sensor gain and phase uncertainties. Circuits. Syst. Signal Process. 1993, 12, 3–35. [Google Scholar] [CrossRef]
- Fuhrmann, D.R. Estimation of sensor gain and phase. IEEE Trans. Signal Process. 1994, 42, 77–87. [Google Scholar] [CrossRef] [PubMed]
- Paulraj, P.; Kailath, T. Direction of arrival estimation by eigenstructure methods with unknown sensor gain and phase. In Proceedings of the ICASSP ’85. IEEE International Conference on Acoustics, Speech, and Signal Processing, Tampa, FL, USA, 26–29 April 1985; pp. 640–643. [Google Scholar]
- Li, Y.; Er, M.H. Theoretical analyses of gain and phase calibration with optimal implementation for linear equispaced array. IEEE Trans. Signal Process. 2006, 54, 712–723. [Google Scholar]
- Liu, Z.M.; Zhou, Y.Y. A unified framework and sparse Bayesian perspective for Direction-of-Arrival estimation in the presence of array imperfections. IEEE Trans. Signal Process. 2013, 61, 3786–3798. [Google Scholar] [CrossRef]
- Liu, A.; Liao, G.; Zeng, C.; Yang, Z.; Xu, Q. An eigenstructure method for estimating DOA and sensor gain-phase errors. IEEE Trans. Signal Process. 2011, 59, 5944–5956. [Google Scholar] [CrossRef]
- Cao, S.; Ye, Z.; Xu, D.; Xu, X. A Hadamard product based method for DOA estimation and gain-phase error calibration. IEEE Trans. Aerosp. Electron. Syst. 2013, 49, 1124–1233. [Google Scholar] [CrossRef]
- Xie, W.; Wang, C.; Wen, F.; Liu, J.; Wan, Q. DOA and gain-phase errors estimation for noncircular sources with central symmetric array. EEE Sens. J. 2017, 17, 3068–3078. [Google Scholar] [CrossRef]
- Sklivanitis, G.; Real, G.; Pados, D.A. Robust direction finding in the fluctuating oceans by complex L1-norm principal component analysis. J. Acoust. Soc. Am. 2019, 146, 3016. [Google Scholar] [CrossRef]
- Dubrovinskaya, E.; Kebkal, V.; Kebkal, O.; Kebkal, K.; Casari, P. Underwater Localization via Wideband Direction-of-Arrival Estimation Using Acoustic Arrays of Arbitrary Shape. Sensors 2020, 20, 3862. [Google Scholar] [CrossRef]
- Lo, J.; Marple, S. Eigenstructure metod for array sensor localization. In Proceedings of the ICASSP ’87. IEEE International Conference on Acoustics, Speech, and Signal Processing, Dallas, TX, USA, 6–9 April 1987; pp. 2660–2663. [Google Scholar]
- Ng, B.P.; Lie, J.P.; Er, M.H.; Feng, A. A practical simple geometry and gain/phase calibration technique for antenna array processing. IEEE Trans. Antennas Propag. 2009, 57, 1963–1972. [Google Scholar]
- Rockah, Y.; Schultheiss, P.M. Array shape calibration using sources in unknown locations-part I: Far-Field sources. IEEE Trans. Acoust. Speech Signal Process. 1987, 35, 286–299. [Google Scholar] [CrossRef]
- Ng, B.C.; See, C.M.S. Sensor-array calibration using a maximum-likelihood approach. IEEE Trans. Antennas Propag. 1996, 44, 827–835. [Google Scholar]
- Kim, H.; Haimovich, A.M.; Eldar, Y.C. Non-coherent direction of arrival estimation from magnitude-only measurements. IEEE Signal Process. Lett. 2015, 22, 925–929. [Google Scholar] [CrossRef]
- Tan, Y.; Shi, J.; Wang, Y.; Lian, Q. Non-coherent direction of arrival estimation utilizing linear model approximation. Signal Process. 2019, 157, 261–265. [Google Scholar]
- Shechtman, Y.; Beck, A.; Eldar, Y.C. GESPAR: Efficient phase retrieval of sparse signals. IEEE Trans. Signal Process. 2014, 62, 928–938. [Google Scholar] [CrossRef]
- Applebaum, S.P. Adaptive arrays. IEEE Trans. Antennas Propag. 1976, 24, 585–598. [Google Scholar] [CrossRef]
- Compton, R.T., Jr. The power inversion array: Concept and performance. IEEE Trans. Aerosp. Electron. Syst. 1979, 15, 803–814. [Google Scholar] [CrossRef]
- Leng, S.; Ser, W.; Ko, C.C. Adaptive beamformer derived from a constrained null steering design. Signal Process. 2010, 90, 1530–1541. [Google Scholar] [CrossRef]
- Leng, S.; Ser, W. IIR array processing based fast adaptive null steering algorithm using shift-invariant subarrays. Signal Process. 2012, 92, 2116–2125. [Google Scholar] [CrossRef]
- Schepker, H.; Tran, L.T.T.; Nordholm, S.E.; Doclo, S. Null-steering beamformer for acoustic feedback cancellation in a multi-microphone earpiece optimizing the maximum stable gain. In Proceedings of the 2017 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP), New Orleans, LA, USA, 5–9 March 2017; pp. 341–345. [Google Scholar]
- Schepker, H.; Nordholm, S.E.; Doclo, S. Acoustic Feedback Suppression for Multi-Microphone Hearing Devices Using a Soft-Constrained Null-Steering Beamformer. IEEE/ACM Trans. Audio Speech Lang. Process. 2020, 28, 929–940. [Google Scholar] [CrossRef]
- Schepker, H.; Tran, L.T.T.; Nordholm, S.E.; Doclo, S. Null-Steering Beamformer-Based Feedback Cancellation for Multi-Microphone Hearing Aids with Incoming Signal Preservation. IEEE/ACM Trans. Audio Speech Lang. Process. 2019, 27, 679–691. [Google Scholar] [CrossRef]
- Weiss, A.J.; Friedlander, B. ‘Almost blind’ steering vector estimation using second-order moments. IEEE Trans. Signal Process. 1996, 44, 1024–1027. [Google Scholar] [CrossRef]
- Astely, D.; Swindlehurst, A.L.; Ottersten, B. Spatial signature estimation for uniform linear arrays with unknown receiver gains and phases. IEEE Trans. Signal Process. 1999, 47, 2128–2138. [Google Scholar] [CrossRef]
- Jalal, B.; Yang, X.; Wu, X.; Long, T.; Sarkar, T.K. Efficient direction-of-arrival estimation method based on variable-step-size LMS algorithm. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1576–1580. [Google Scholar] [CrossRef]
- Liu, C.; Zhao, H. Efficient DOA estimation method using bias-compensated adaptive filtering. IEEE Trans. Veh. Technol. 2020, 69, 13087–13097. [Google Scholar] [CrossRef]
- Golub, G.H.; Loan, C.F.V. An analysis of the total least squares problem. SIAM J. Numer. Anal. 1980, 17, 883–893. [Google Scholar] [CrossRef]
- Huffel, S.V.; Vanderwalle, J. The Total Least Square Problem: Computational Aspects and Analysis; SIAM: Philadelphia, PA, USA, 1991. [Google Scholar]
- Stoica, P.; Nehorai, A. Performance study of conditional and unconditional direction-of arrival estimation. IEEE Trans. Acoust. Speech Signal Process. 1990, 38, 1783–1795. [Google Scholar] [CrossRef]
- Hjorungnes, A. Complex-Valued Matrix Derivatives: With Applications in Signal Processing and Communications; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Markovsky, I.; Rastello, M.L.; Premmoli, A.; Kukush, A.; Huffel, S.V. The element-wise weighted total least-squares problem. Comput. Stat. Data Anal. 2006, 50, 181–309. [Google Scholar] [CrossRef]
- Schaffrin, B.; Wieser, A. On weighted total least-squares adjustment for linear regression. J. Geod. 2008, 82, 415–421. [Google Scholar] [CrossRef]
- Zhu, H.; Leus, G.; Giannakis, G.B. Sparsity-cognizant total least-squares for perturbed compressive sampling. IEEE Trans. Signal Process. 2011, 59, 2002–2016. [Google Scholar] [CrossRef]
- Wang, L.; Yu, H. Weighted total least-squares joint adjustment with weight correction factors. Commun. Stat. Simul. Comput. 2018, 48, 2689–2770. [Google Scholar] [CrossRef]
- Wilkinson, J.H. The Algebraic Eigenvalue Problem; Oxford University Press: New York, NY, USA, 1965. [Google Scholar]
- Chen, P.; Chen, Z.; Cao, Z.; Wang, X. A new atomic norm for DOA estimation with gain-phase errors. IEEE Trans. Signal Process. 2020, 68, 4293–4306. [Google Scholar] [CrossRef]
- Stoica, P.; Nehorai, A. Music, Maximum likelihood, and Cramer-Rao bound. IEEE Trans. Acoust Speech Signal Process. 1989, 35, 720–741. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Tang, X.; Zhang, Z. A New Gain-Phase Error Pre-Calibration Method for Uniform Linear Arrays. Sensors 2023, 23, 2544. https://doi.org/10.3390/s23052544
Liu C, Tang X, Zhang Z. A New Gain-Phase Error Pre-Calibration Method for Uniform Linear Arrays. Sensors. 2023; 23(5):2544. https://doi.org/10.3390/s23052544
Chicago/Turabian StyleLiu, Chang, Xiao Tang, and Zhi Zhang. 2023. "A New Gain-Phase Error Pre-Calibration Method for Uniform Linear Arrays" Sensors 23, no. 5: 2544. https://doi.org/10.3390/s23052544
APA StyleLiu, C., Tang, X., & Zhang, Z. (2023). A New Gain-Phase Error Pre-Calibration Method for Uniform Linear Arrays. Sensors, 23(5), 2544. https://doi.org/10.3390/s23052544




