Assessment of GNSS Galileo Contribution to the Modernization of CROPOS’s Services
Abstract
1. Introduction
2. Materials and Methods
2.1. CROPOS
2.2. Galileo Satellite System and Its Applicability for High-Accuracy Positioning
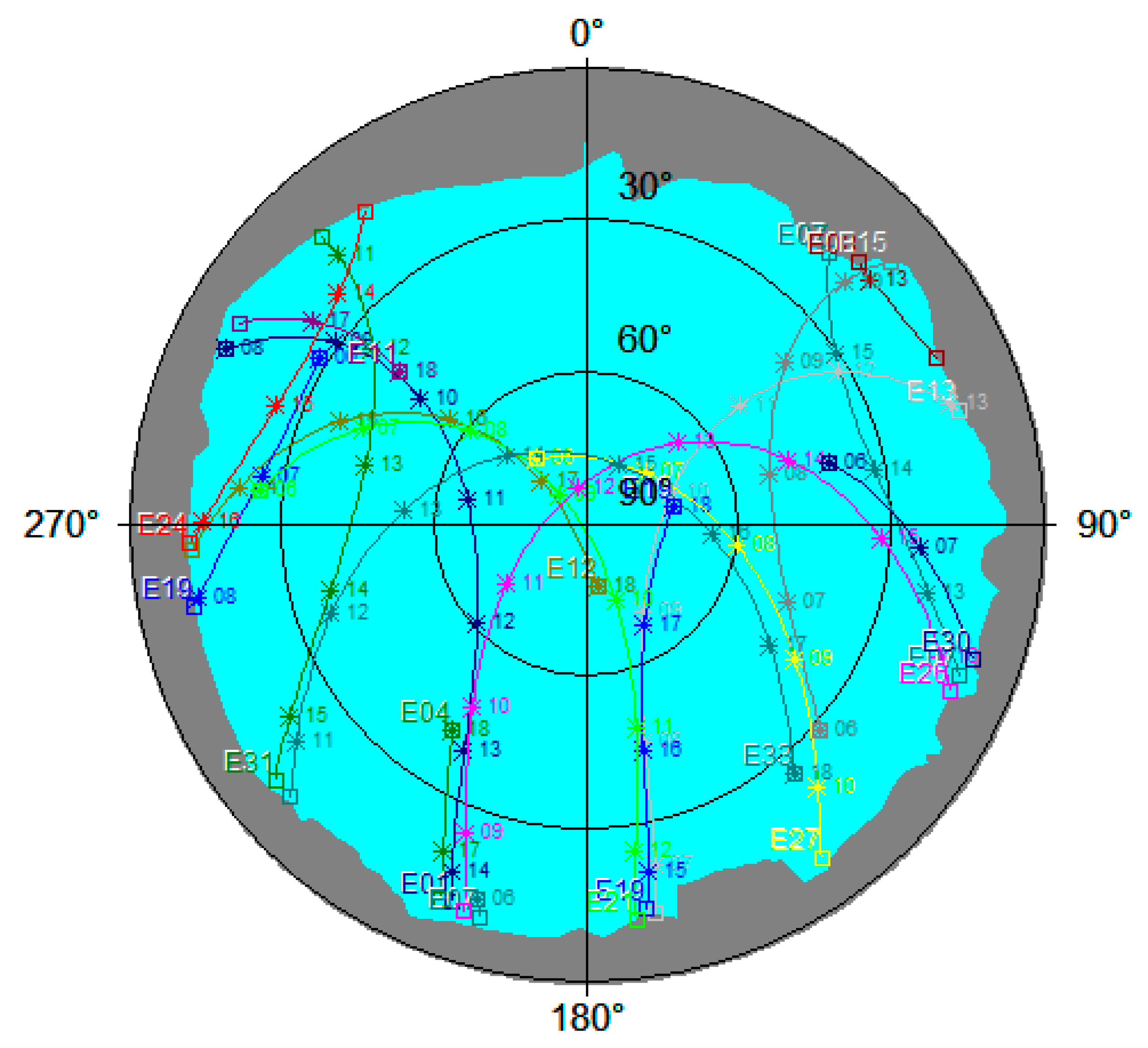
2.3. Mission Planning
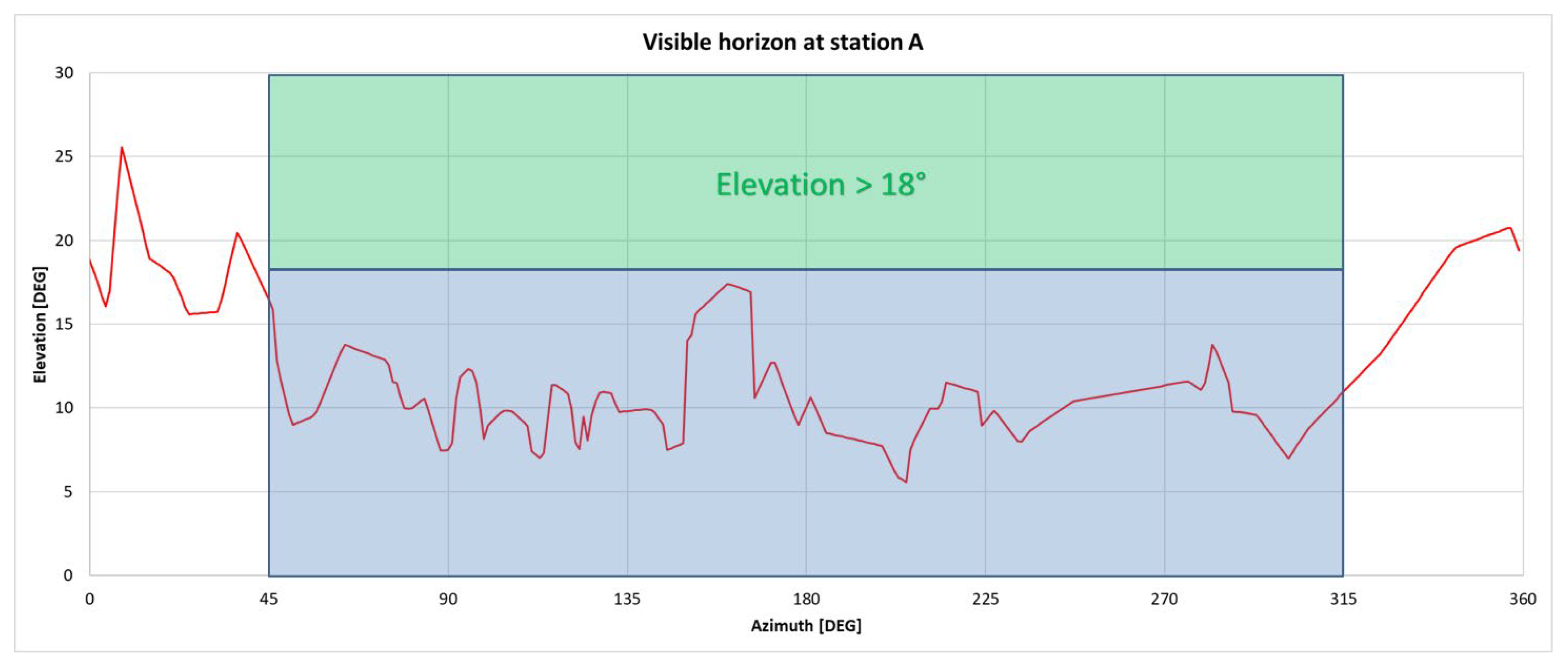
2.4. Preparation and Execution of the Field Activities
- VPPS (GGG): GGG, 10°, 30 s, RTCM 3.2 MSM, PDOP 6
- VPPS (GAL-only): GAL, 10°, 30 s, RTCM 3.2 MSM, PDOP 6
- GPPS (GGGB): GGGB, 10°, logging interval 5 s, duration 22 min.
- VPPS (GGG): 30 s,
- VPPS (GGG): 30 s,
- VPPS (GGG): 30 s,
- VPPS (GAL-only): 30 s,
- VPPS (GAL-only): 30 s,
- VPPS (GAL-only): 30 s,
- GPPS (GGGB): 22 min.
3. Results
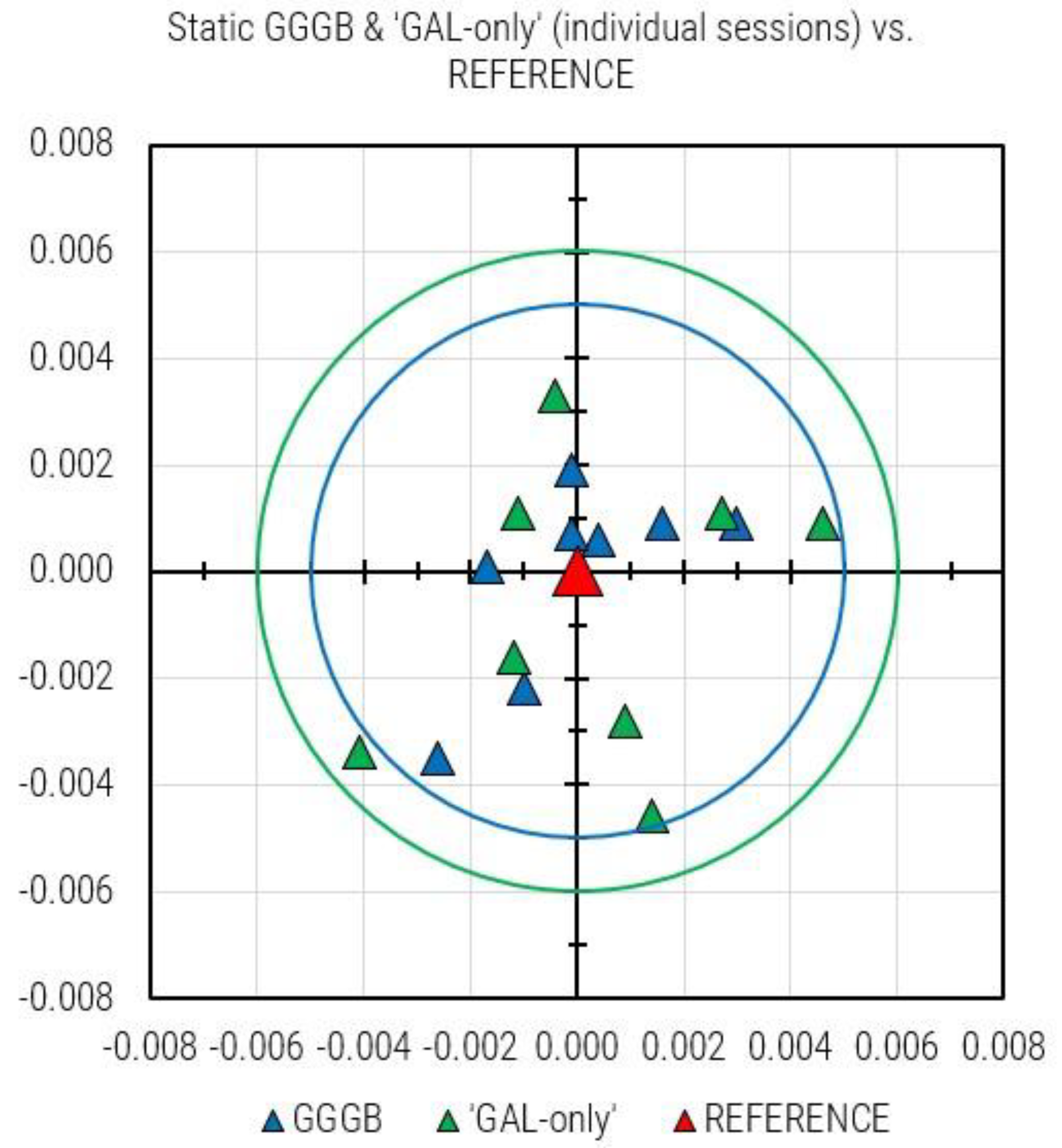
3.1. Static Observations and Processing in Trimble Business Center
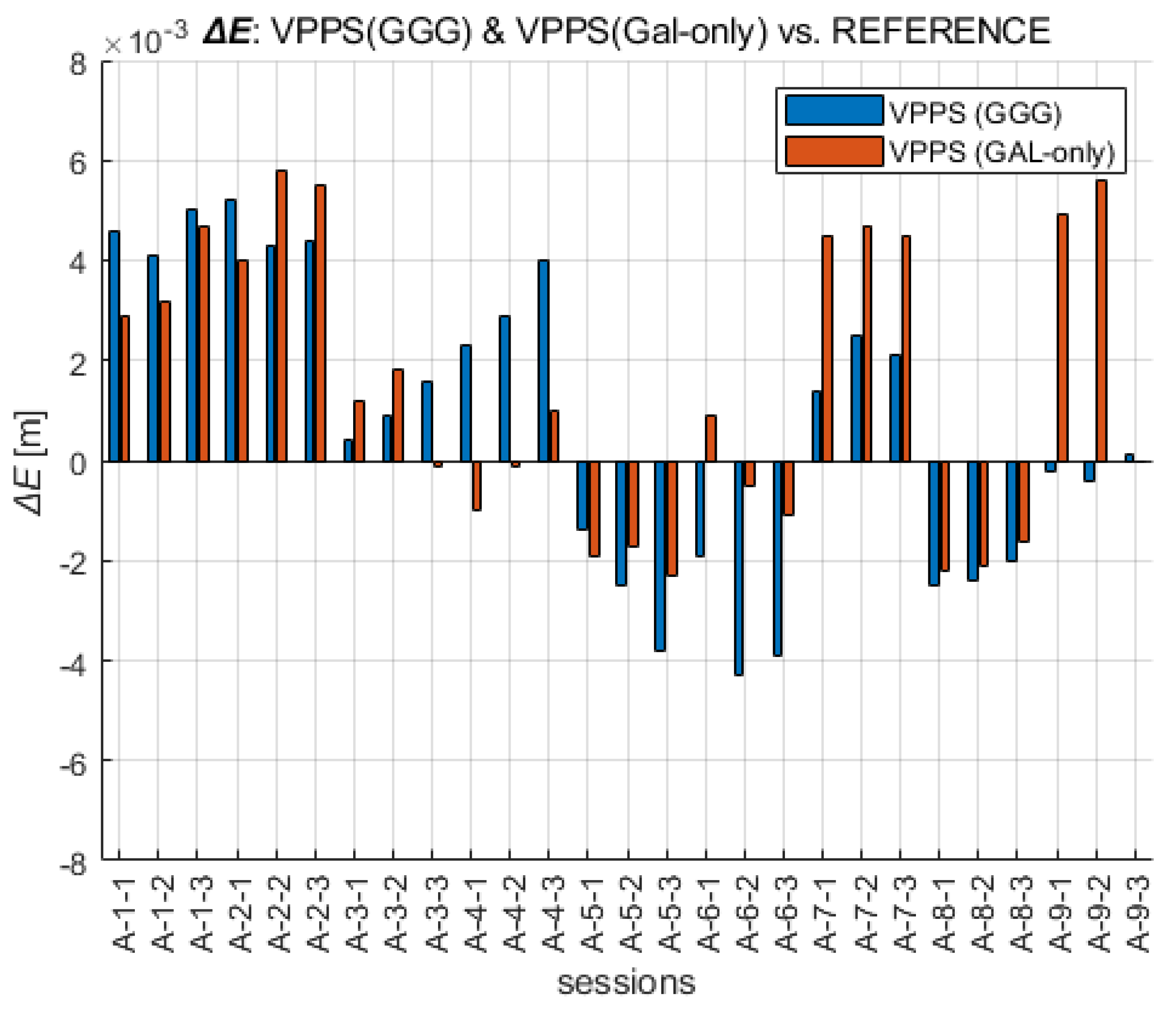
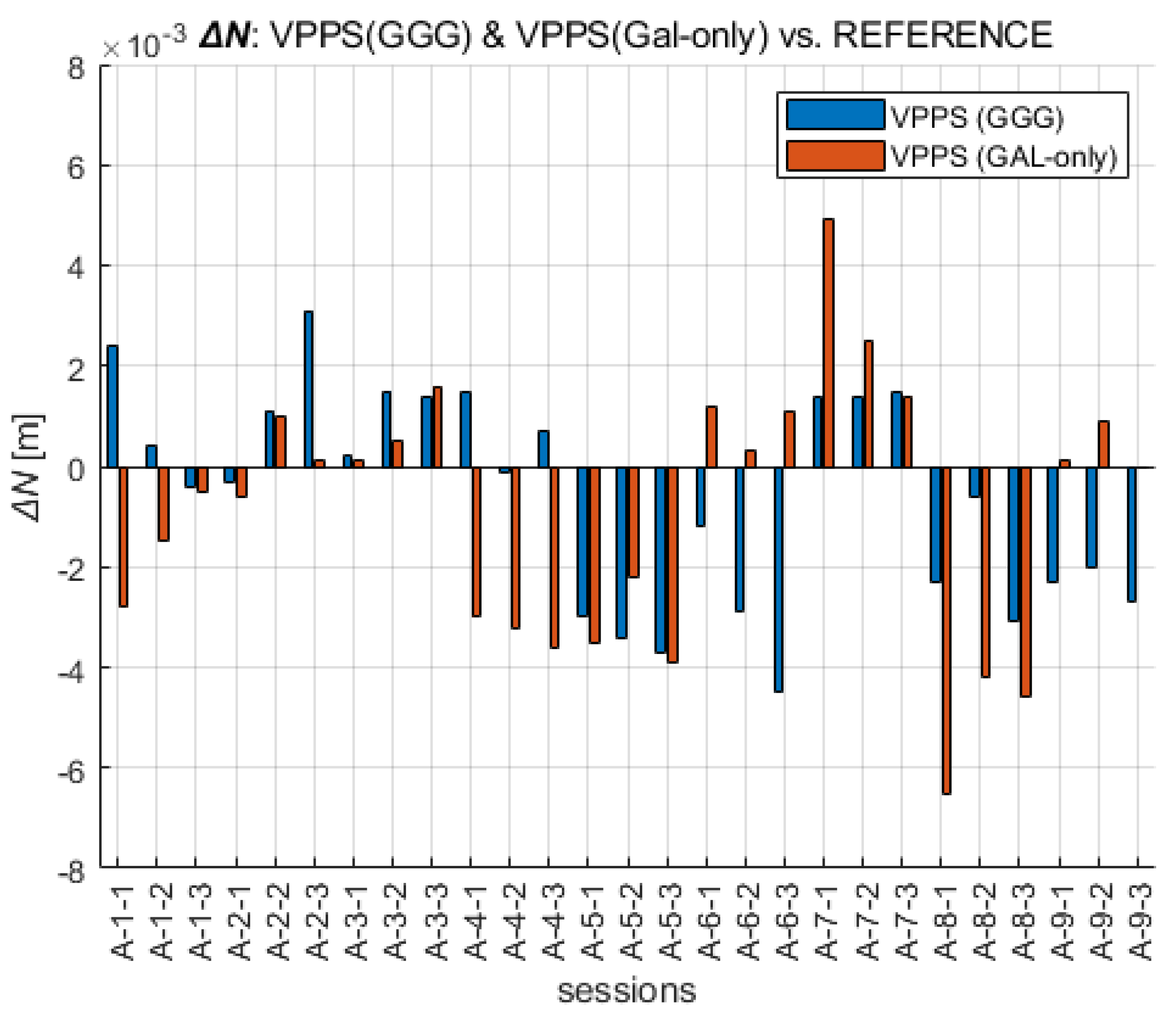
3.2. Kinematic Observations Using VPPS
4. Discussion
4.1. Analysis of the Number of Satellites That Contributed to VPPS Results and Estimated Precision Assessment
4.2. Analysis and Discussion of the VPPS Results
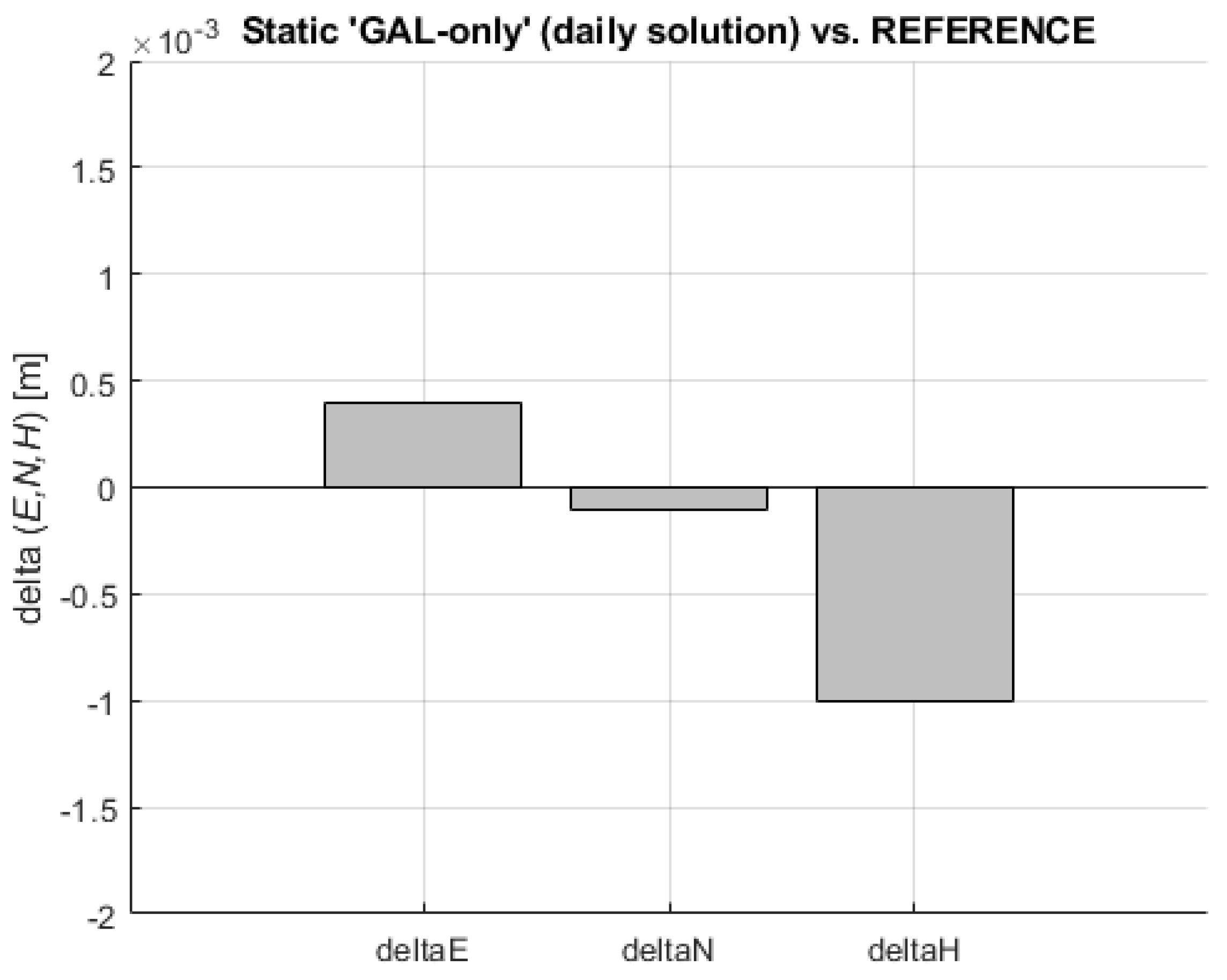
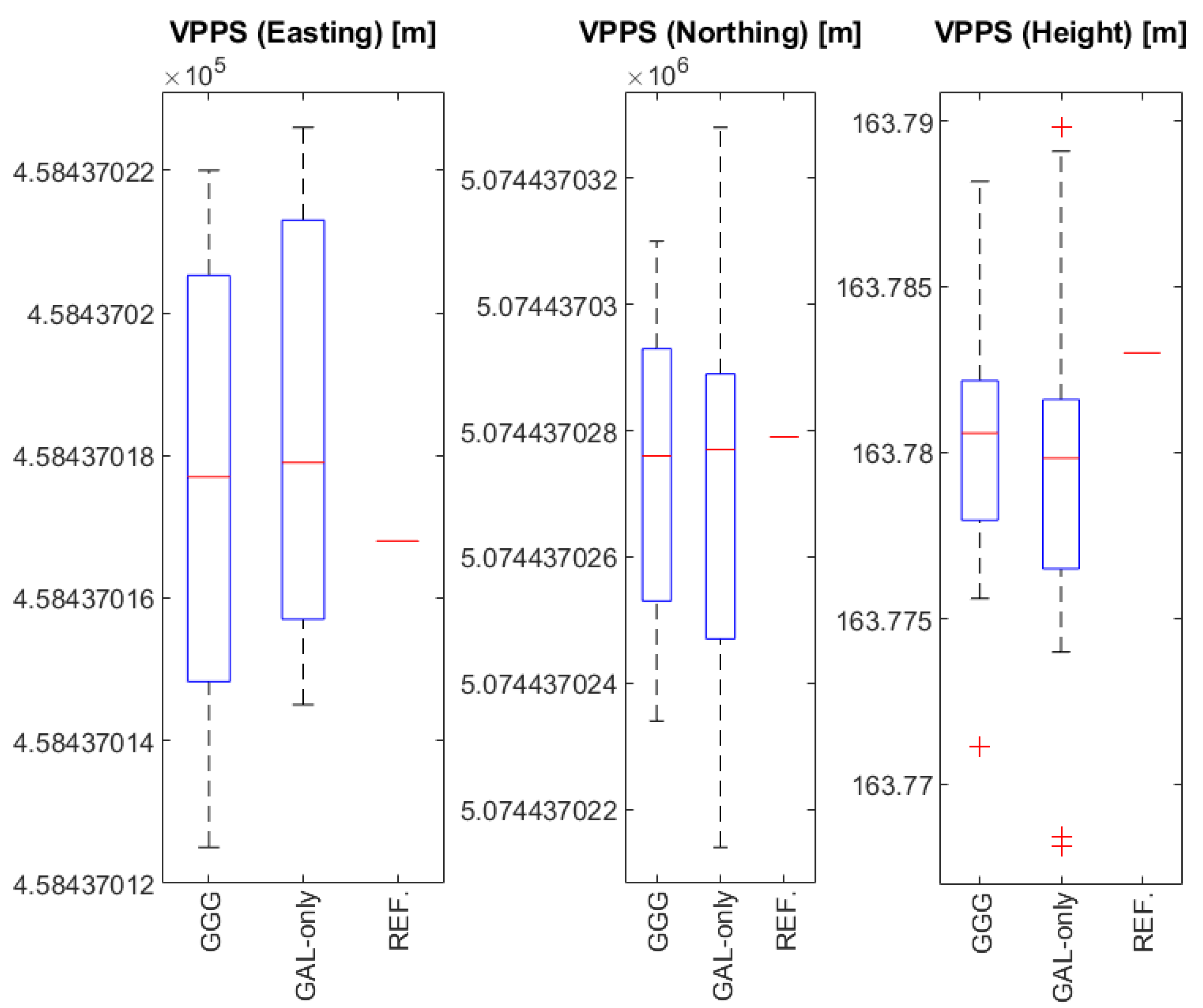
- Easting (Figure 13, left). The range of the Easting results obtained by GGG and ‘GAL-only’ are 0.0095 m and 0.0081 m, respectively. The greater range for GGG is due to the results obtained in session A-6 having higher DOP values (see Figure 9). The IQR for GGG solution is a bit higher (0.0056 m) compared to the ‘GAL-only’ solution (0.0054 m). Interestingly, the median values are almost equal (0.2 mm of difference), whereas there is a small difference between the average values of GGG (0.0176 m) vs. ‘GAL-only’ (0.0184). Both median values compared to the REF value supply the differences of 0.0009 m and 0.0011 m (GGG vs. ‘GAL-only’), which can be considered as a negligible amount. The averaging of VPPS results provides a more accurate and reliable solution. Easting components obtained with GGG and GAL-only observations have shown a great level of similarity (matching), leading to the conclusion that Easting components obtained with ‘GAL-only’ observations can be regarded as equally accurate and reliable as those obtained with GGG observations.
- Northing (Figure 13 middle). There is a small difference between median values: GGG (0.0276 m) vs. GAL-only (0.0277 m). There is a small difference between the average values: GGG (0.0273 m) vs. GAL-only (0.0270 m). The IQR is slightly smaller for GGG (0.0039 m) vs. GAL-only (0.0041 m), but the range for GAL-only is bigger (0.0114 m) compared to GGG (0.0076 m). That means that there is a higher spread of the results obtained with GAL-only observations, which can be seen in STDEV values, as well (0.0021 m vs. 0.0026 m). The higher range for Northing components obtained with GAL-only comes from the results in session A-8 (see Figure 10). Northing components obtained with GGG and GAL-only observations have shown a great level of similarity (matching), leading to the conclusion that Northing components obtained with ‘GAL-only’ observation can be regarded as equally accurate and reliable as those obtained with GGG observations, even though ‘GAL-only’ results have shown a greater level of variability. When the results are averaged and compared to the REFERENCE value, there is a negligible difference < 1 mm.
- Height (Figure 13 right). When it comes to the height components, there is no significant difference between MEDIAN values (GGG: 0.7806 m vs. GAL-only: 0.7799 m) and AVERAGE values (GGG: 0.7803 m vs GAL-only: 0.7794 m). On the other hand, there is a greater RANGE for ‘GAL-only’ results (0.0217 m) compared to GGG results (0.0171 m), that has consequences for the differences in IQR values (GGG: 0.0040 m vs. GAL-only: 0.0051 m). Small differences between the average values (<1 mm) compared to the REFERENCE value give the difference < 4 mm, providing a confirmation that calculating the average results improves the accuracy and reliability of the final value. Four outlier values have been found: one outlier in GGG results and the other three outliers in GAL-only results. Usually, the outliers are found by considering the IQR, Q1, and Q3 values, where the lower and upper outlier gates are calculated with the following expressions:
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Langley, R.B. RTK GPS. GPS World 1998, 9, 70–76. [Google Scholar]
- Wanninger, L. Introduction to Network RT. 2008. Available online: http://www.wasoft.de/e/iagwg451/intro/introduction.html (accessed on 29 November 2022).
- European GNSS Agency (GSA). GNSS User Technology Report, Issue 3; Publications Office of the European Union: Luxembourg, 2020; ISBN 978-92-9206-049-7. [Google Scholar]
- Feng, Y.; Wang, J. GPS RTK Performance Characteristics and Analysis. J. Glob. Position. Syst. 2008, 7, 1–8. [Google Scholar] [CrossRef]
- Hartmann, S. History of RTK—Part 1: A Really Tough Problem to Solve. Am. Surv. 2021, 18, 18–22. [Google Scholar]
- Hartmann, S. History of RTK—Part 2: RTK Roots Run Deep. Am. Surv. 2021, 18, 26–31. [Google Scholar]
- Hartmann, S. History of RTK Part 3: Everybody Wanted It. Am. Surv. 2021, 18, 10–17. [Google Scholar]
- Hartmann, S. History of RTK Part 4: Birth of a Utility. Am. Surv. 2021, 18, 26–32. [Google Scholar]
- European GNSS Agency (GSA). PPP-RTK Market and Technology Report. 2019. Available online: https://www.euspa.europa.eu/simplecount_pdf/tracker?file=calls_for_proposals/rd.03_-_ppp-rtk_market_and_technology_report.pdf (accessed on 29 November 2022).
- El-Mowafy, A. Precise Real-Time Positioning Using Network RTK. In Global Navigation Satellite Systems: Signal, Theory and Applications; Shuanggen, J., Ed.; IntechOpen: London, UK, 2012; pp. 161–188. [Google Scholar] [CrossRef]
- Leica Geosystems, A.G. Networked Reference Stations, Take It to the MAX! 2005. Available online: https://www.smartnetna.com/documents/Leica_GPS_SpiderNET-Take_it_to_the_MAX_June2005_en.pdf (accessed on 29 November 2022).
- Hu, G.R.; Khoo, H.S.; Goh, P.C.; Law, C.L. Development and assessment of GPS virtual reference stations for RTK positioning. J. Geod. 2003, 77, 292–302. [Google Scholar] [CrossRef]
- Lipatnikov, L.A.; Shevchuk, S.O. Cost-Effective Precise Positioning with GNSS, FIG Report Commission 5. In Proceedings of the International Federation of Surveyors (FIG), Copenhagen, Denmark, March 2019; p. 86, ISBN 978-87-92853-87-5. [Google Scholar]
- Vollath, U.; Patra, R.; Chen, X.; Landau, H.; Allison, T. GALILEO/Modernized GPS: A New Challenge to Network RTK. In Proceedings of the 17th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GNSS 2004), Long Beach, CA, USA, 21–24 September 2004; pp. 2855–2863. [Google Scholar]
- Janssen, V. A comparison of the VRS and MAC principles for network RTK. In Proceedings of the International Global Navigation Satellite Systems Society IGNSS Symposium 2009, Surfers Paradise, QLD, Australia, 1–3 December 2009; p. 13. [Google Scholar]
- Schrock, G. The birthplace of VRS. Am. Surv. 2010, 7, 28–32. [Google Scholar]
- Rizos, C.; Han, S. Reference station network based RTK systems-concepts and progress. Wuhan Univ. J. Nat. Sci. 2003, 8, 566–574. [Google Scholar] [CrossRef]
- Landau, H.; Vollath, U.; Chen, X. Virtual Reference Station Systems. J. Glob. Position. Syst. 2002, 1, 137–143. [Google Scholar] [CrossRef]
- Wei, E.; Chai, H.; An, Z.; Liu, J. VRS Virtual Observations Generation Algorithm. J. Glob. Position. Syst. 2006, 5, 76–81. [Google Scholar] [CrossRef]
- Odijk, D.; Wanninger, L. Differential Positioning. In Handbook of Global Navigation Satellite System; Teunissen, P.J.G., Montenbruck, O., Eds.; Springer International Publishing AG: Cham, Switzerland, 2017; pp. 753–780. ISBN 978-3-319-42926-7. [Google Scholar] [CrossRef]
- Petovello, M. GNSS Solution: Virtual Reference Station. Inside GNSS 2011, 6, 28–31. [Google Scholar]
- Chen, X.; Vollath, U.; Landau, H.; Sauer, K. Will GALILEO/Modernized GPS Obsolete Network RTK? In Proceedings of ENC-GNSS 2004, Rotterdam, The Netherlands, 17–21 May 2004.
- Paziewski, J.; Wielgosz, P. Assessment of GPS + Galileo and multi-frequency Galileo single-epoch precise positioning with network corrections. GPS Solut. 2014, 18, 571–579. [Google Scholar] [CrossRef]
- Capua, R. GNSS in Cadastral Surveying: State of the Art and future perspectives in the framework of Galileo. In Proceedings of the FIG Congress 2018—Embracing our Smart World where the Continents Connect: Enhancing the Geospatial Maturity of Societies, Istanbul, Turkey, 6–11 May 2018. [Google Scholar]
- CROPOS. CROPOS—Državna Mreža Referentnih Stanica Republike Hrvatske. 2022. Available online: https://cropos.hr/o-sustavu/cropos-drzavna-mreza-referentnih-stanica-republike-hrvatske (accessed on 29 November 2022). (In Croatian).
- CROPOS. CROPOS—VPPS. 2022. Available online: https://cropos.hr/servisi/vpps (accessed on 29 November 2022). (In Croatian).
- CROPOS. CROPOS GNSS Reference Station Web Server. 2022. Available online: https://gnss.cropos.hr/Map/SensorMap.aspx (accessed on 29 November 2022).
- Geomatika-Smolčak. Modernizacija CROPOS Sustava. 2019. Available online: https://geomatika-smolcak.hr/novosti/modernizacija-cropos-sustava/ (accessed on 29 November 2022). (In Croatian).
- CROPOS. Nove Usluge Sustava CROPOS. 2022. Available online: https://cropos.hr/projekti/unaprjedenje-hrvatskog-pozicijskog-sustava-cropos-povezivanjem-s-europskim-globalnim-satelitskim-sustavom-galileo/164-nove-usluge-sustava-cropos (accessed on 29 November 2022). (In Croatian).
- CROPOS. Zakon o Izmjenama i Dopuni Zakona o Državnoj Izmjeri i Katastru Nekretnina. 2022. Available online: https://www.cropos.hr/obavijesti/170-zakon-o-izmjenama-i-dopuni-zakona-o-drzavnoj-izmjeri-i-katastru-nekretnina (accessed on 29 November 2022). (In Croatian).
- European Commission. About Galileo. 2022. Available online: https://defence-industry-space.ec.europa.eu/eu-space-policy/galileo_en (accessed on 29 November 2022).
- Bonnor, N. A brief history of global navigation satellite systems. J. Navig. 2012, 65, 1–14. [Google Scholar] [CrossRef]
- ESA Earth Observation Portal. Galileo FOC Series. 2022. Available online: https://directory.eoportal.org/web/eoportal/satellite-missions/g/galileo-foc#references (accessed on 29 November 2022).
- European GNSS Service Centre. Galileo Constellation Information. 2022. Available online: https://www.gsc-europa.eu/system-service-status/constellation-information (accessed on 29 November 2022).
- Galileo GNSS. Galileo Frequency Bands. 2013. Available online: https://galileognss.eu/galileo-frequency-bands/ (accessed on 29 November 2022).
- ESA. Galileo Navigation Signals and Frequencies. 2022. Available online: https://www.esa.int/Applications/Navigation/Galileo/Galileo_navigation_signals_and_frequencies (accessed on 29 November 2022).
- Montenbruck, O.; Rizos, C.; Weber, R.; Weber, G.; Neilan, R.; Hugentobler, U. Innovation: Getting a Grip on Multi-GNSS—GPS World. GPSWorld 2013, 24, 44–49. [Google Scholar]
- Odijk, D.; Teunissen, P.J.G.; Khodabandeh, A. Galileo IOV RTK positioning: Standalone and combined with GPS. Surv. Rev. 2013, 46, 267–277. [Google Scholar] [CrossRef]
- Tian, Y.; Sui, L.; Xiao, G.; Zhao, D.; Tian, Y. Analysis of Galileo/BDS/GPS signals and RTK performance. GPS Solut. 2019, 23, 37. [Google Scholar] [CrossRef]
- ESA. Galileo Fixes Europe’s Position in History. 2013. Available online: https://www.esa.int/Applications/Navigation/Galileo_fixes_Europe_s_position_in_history (accessed on 29 November 2022).
- Steigenberger, P.; Hugentobler, U.; Montenbruck, O. First Demonstration of Galileo-Only Positioning. GPSWorld 2013, 24, 14–15. [Google Scholar]
- Talbot, N. Capturing Today’s (and Tomorrow’s) Constellations. XYHT 2022, 9, 16–21. [Google Scholar]
- European GNSS Service Centre. Galileo Open Service—Service Definition Document (OS SDD v1.2). 2022. Available online: https://www.gsc-europa.eu/sites/default/files/sites/all/files/Galileo-OS-SDD_v1.2.pdf (accessed on 29 November 2022).
- European GNSS Agency (GSA). EGNOS and GALILEO for MAPPING & SURVEYING, High Accuracy, Widely Available. 2017. Available online: https://www.euspa.europa.eu/system/files/documents/mappingsurveying_gnss.pdf (accessed on 29 November 2022).
- Mihoković, V.; Zalović, L.; Šugar, D. Single Base RTK Solutions Obtained Individually with GALILEO and Beidou as Well as in Combination with Other Fully Operational GNSS. In Proceedings of the FIG Congress 2018, International Federation of Surveyors (FIG), Istanbul, Turkey, 6–11 May 2018. [Google Scholar]
- Luo, X.; Chen, J.; Richter, B. How Galileo benefits high-precision RTK. GPSWorld 2017, 28, 22–28. [Google Scholar]
- Luo, X.; Schaufler, S.; Branzanti, M.; Chen, J. Assessing the benefits of Galileo to high-precision GNSS positioning—RTK, PPP and post-processing. Adv. Space Res. 2020, 68, 4916–4931. [Google Scholar] [CrossRef]
- Šugar, D.; Skopljak, B.; Bačić, Ž. Multi-Constellation GNSS Baseline Solutions-a Perspective from the User’s and Developer’s Point of View. In Proceedings of the FIG Congress 2018, International Federation of Surveyors (FIG), Istanbul, Turkey, 6–11 May 2018. [Google Scholar]
- Prochniewicz, D.; Szpunar, R.; Kozuchowska, J.; Szabo, V.; Staniszewska, D.; Walo, J. Performance of Network-Based GNSS Positioning Services in Poland: A Case Study. J. Surv. Eng. 2020, 146, 04016017. [Google Scholar] [CrossRef]
- European GNSS Service Centre. Galileo in the Permanent CORS Network in Sweden: A Success Story. 2022. Available online: https://www.gsc-europa.eu/news/galileo-in-the-permanent-cors-network-in-sweden-a-success-story (accessed on 29 November 2022).
- Schrock, G. An Exemplary Real-Time GNSS Network. XYHT 2022, 9, 11–15. [Google Scholar]
- EUSPA. Galileo-Enabled RTK Network Brings Clear Benefits to Surveyors. 2017. Available online: https://www.euspa.europa.eu/newsroom/news/galileo-enabled-rtk-network-brings-clear-benefits-surveyors (accessed on 29 November 2022).
- Hriscu, A. European GNSS for Surveying and Mapping. In Proceedings of the Presented at the Communication Workshop of the PosKEN, Brussels, Belgium, 28 April 2018. [Google Scholar]
- Trimble Inc. Trimble GNSS Planning Online. 2022. Available online: https://www.gnssplanning.com/#/settings (accessed on 29 November 2022).
- National Geographic. Horizon. 2022. Available online: https://education.nationalgeographic.org/resource/horizon (accessed on 29 November 2022).
- BeiDou Navigation Satellite System. Completion and Commissioning of the BeiDou Navigation Satellite System (BDS-3). 2020. Available online: http://en.beidou.gov.cn/WHATSNEWS/202008/t20200803_21013.html (accessed on 29 November 2022).
- Trimble Inc. New Trimble R12 Receiver Boosts Surveying Performance. 2019. Available online: https://investor.trimble.com/news-releases/news-release-details/new-trimble-r12-receiver-boosts-surveying-performance (accessed on 29 November 2022).
- Trimble Inc. Trimble R12—Datasheet. 2019. Available online: https://geospatial.trimble.com/sites/geospatial.trimble.com/files/2020-10/Datasheet%20-%20Trimble%20R12%20GNSS%20Receiver%20English%20(US)%20-%20Screen.pdf (accessed on 29 November 2022).
- State Geodetic Administration—SGA. Regulations on the Fundamental Geodetic Works Performance, National Gazette. 2020. Available online: https://narodne-novine.nn.hr/clanci/sluzbeni/2020_02_15_316.html (accessed on 29 November 2022). (In Croatian).
- State Geodetic Administration—SGA. Appendix 3 to the Regulations on the Fundamental Geodetic Works Performance—CROPOS—Croatian Positiong System. 2022. Available online: https://dgu.gov.hr/UserDocsImages/dokumenti/Pristup%20informacijama/Zakoni%20i%20ostali%20propisi/Pravilnici/Prilozi_1_9_Pravilnika_o_nacinu_izvodjenja_OGR.pdf (accessed on 29 November 2022). (In Croatian)
- Henning, W.; User Guidelines for Single Base Real Time GNSS Positioning. National Geodetic Survey (NGS). 2014. Available online: https://geodesy.noaa.gov/PUBS_LIB/UserGuidelinesForSingleBaseRealTimeGNSSPositioningv.3.1APR2014-1.pdf (accessed on 29 November 2022).
- Odolinski, R.; Swedish User Guidelines for Network RTK. Lantmäteriet. 2010. Available online: https://www.lantmateriet.se/globalassets/kartor-och-geografisk-information/gps-och-geodetisk-matning/publikationer/nkg2010_swedish_userguidelines_odolinski.pdf (accessed on 28 July 2021).
- Hofmann-Wellenhof, B.; Lichtenegger, H.; Collins, J. Global Positioning System, 5th ed.; Springer: Vienna, Austria, 2001; ISBN 978-3-7091-6199-9. [Google Scholar]
- National Geodetic Survey—NGS. Antenna Calibration—FAQ. 2022. Available online: https://geodesy.noaa.gov/ANTCAL/index.xhtml# (accessed on 29 November 2022).
- Blecha, J. Trimble Business Center—Modernized Approaches for GNSS Baseline Processing. 2018. Available online: https://drive.google.com/file/d/16nktMJR2gUE1NRKVrItKqZnruuLI04mN/view (accessed on 29 November 2022).
- Trimble Inc. TBC—Product Bulletin, Trimble Geospatial. 2018. Available online: https://drive.google.com/file/d/14gTxj_VrVCI0fm-zS6uQlGuu8_r21g_q/view (accessed on 29 November 2022).
- Trimble Geospatial. Trimble Business Center Tutorials, Trimble. 2022. Available online: https://geospatial.trimble.com/trimble-business-center-tutorials (accessed on 29 November 2022).
- Trimble Geospatial. Trimble Access Help Portal—Glossary. Trimble. 2022. Available online: https://help.trimblegeospatial.com/TrimbleAccess/latest/en/Glossary.htm (accessed on 29 November 2022).
- Seeber, G. Satellite Geodesy, 2nd ed.; Walter de Gruyter: Berlin, Germany, 2003; p. 589. ISBN 978-3-1101-7549-3. [Google Scholar]
- Trimble Inc. Trimble Access Software General Survey User Guide, Version 2021.10, Trimble. 2021. Available online: https://help.trimblegeospatial.com/TrimbleAccess-PDFs/2021.20/en/TA_General_Survey.pdf (accessed on 29 November 2022).
- Microsoft. Office Support, Create a Box and Whisker Chart. 2022. Available online: https://support.microsoft.com/en-us/office/create-a-box-and-whisker-chart-62f4219f-db4b-4754-aca8-4743f6190f0d (accessed on 29 November 2022).
- Frost, J. Statistic by Jim—Interquartile Range (IQR): How to Find and Use It. 2022. Available online: https://statisticsbyjim.com/basics/interquartile-range/ (accessed on 29 November 2022).
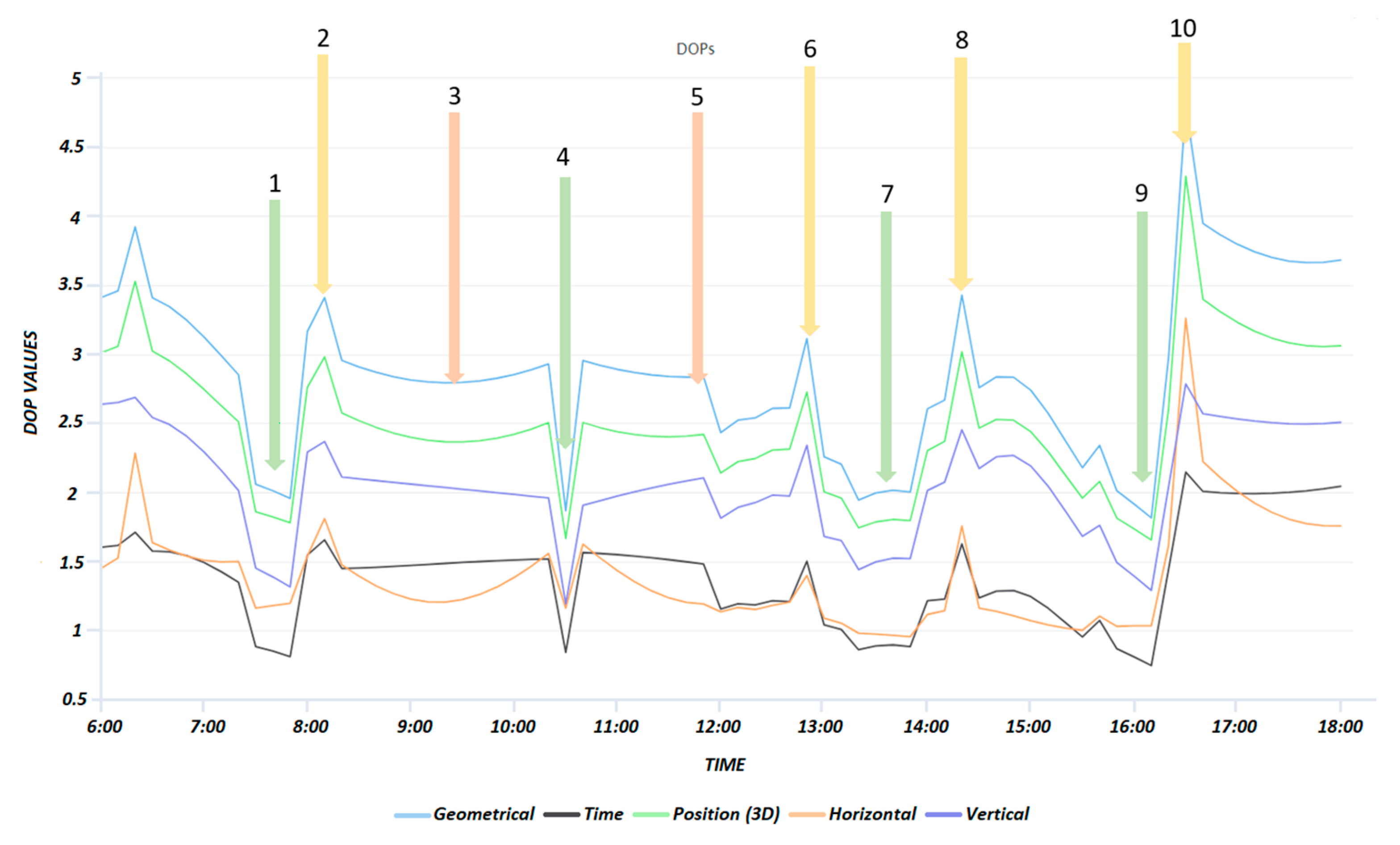





| Session Planned | Start of Session (CET) | Expected Number of GAL SV Visible | Expected PDOP |
|---|---|---|---|
| 1 | 07:50 | 7 | 1.78 |
| 2 | 08:10 | 6 | 2.98 |
| 3 | 09:30 | 6 | 2.37 |
| 4 | 10:30 | 7 | 1.67 |
| 5 | 11:50 | 6 | 2.42 |
| 6 | 12:50 | 7 | 2.73 |
| 7 | 13:30 | 8 | 1.79 |
| 8 | 14:20 | 6 | 3.02 |
| 9 | 16:00 | 7 | 1.74 |
| 10 | 16:30 | 4 | 4.29 |
| Session | Series | Start Time (CET) | PDOP | Number of SV |
|---|---|---|---|---|
| 1. (A-1) | VPPS (GGG) | 07:53:08 | 1.2 | 18 |
| VPPS (GGG) | 07:53:42 | 1.2 | 18 | |
| VPPS (GGG) | 07:54:18 | 1.2 | 18 | |
| VPPS (GAL-only) | 07:56:53 | 3.9 | 5 | |
| VPPS (GAL-only) | 07:58:38 | 3.9 | 5 | |
| VPPS (GAL-only) | 07:59:24 | 3.8 | 5 | |
| STATIC (GGGB) | --- | --- | --- | |
| 2. (A-2) | VPPS (GGG) | 08:10:54 | 1.6 | 15 |
| VPPS (GGG) | 08:12:06 | 1.6 | 15 | |
| VPPS (GGG) | 08:12:43 | 1.6 | 17 | |
| VPPS (GAL-only) | 08:20:50 | 3.3 | 5 | |
| VPPS (GAL-only) | 08:21:34 | 3.3 | 5 | |
| VPPS (GAL-only) | 08:22:10 | 3.3 | 5 | |
| STATIC (GGGB) | 08:25–08:47 | |||
| 3. (A-3) | VPPS (GGG) | 09:31:01 | 1.4 | 16 |
| VPPS (GGG) | 09:31:47 | 1.4 | 16 | |
| VPPS (GGG) | 09:32:24 | 1.4 | 16 | |
| VPPS (GAL-only) | 09:34:55 | 2.4 | 6 | |
| VPPS (GAL-only) | 09:35:32 | 2.4 | 6 | |
| VPPS (GAL-only) | 09:36:11 | 2.4 | 6 | |
| STATIC (GGGB) | 09:38–10:00 | |||
| 4. (A-4) | VPPS (GGG) | 10:31:14 | 1.3 | 18 |
| VPPS (GGG) | 10:31:52 | 1.4 | 18 | |
| VPPS (GGG) | 10:32:33 | 1.4 | 18 | |
| VPPS (GAL-only) | 11:02:30 | 3.0 | 6 | |
| VPPS (GAL-only) | 11:03:06 | 2.4 | 6 | |
| VPPS (GAL-only) | 11:03:43 | 2.4 | 6 | |
| STATIC (GGGB) | 11:05–11:27 | |||
| 5. (A-5) | VPPS (GGG) | 11:53:50 | 1.3 | 20 |
| VPPS (GGG) | 11:54:25 | 1.4 | 19 | |
| VPPS (GGG) | 11:55:00 | 1.4 | 19 | |
| VPPS (GAL-only) | 11:56:50 | 2.4 | 6 | |
| VPPS (GAL-only) | 11:57:26 | 2.4 | 6 | |
| VPPS (GAL-only) | 11:58:01 | 2.4 | 6 | |
| STATIC (GGGB) | 12:01–12:23 | |||
| 6. (A-6) | VPPS (GGG) | 12:54:27 | 1.3 | 18 |
| VPPS (GGG) | 12:55:09 | 1.3 | 18 | |
| VPPS (GGG) | 12:55:43 | 1.3 | 18 | |
| VPPS (GAL-only) | 12:59:33 | 2.7 | 7 | |
| VPPS (GAL-only) | 13:00:07 | 2.7 | 7 | |
| VPPS (GAL-only) | 13:00:43 | 2.7 | 7 | |
| STATIC (GGGB) | 13:02–13:20 | |||
| 7. (A-7) | VPPS (GGG) | 13:56:45 | 1.3 | 23 |
| VPPS (GGG) | 13:57:25 | 1.3 | 23 | |
| VPPS (GGG) | 13:58:01 | 1.3 | 23 | |
| VPPS (GAL-only) | 13:59:39 | 2.4 | 6 | |
| VPPS (GAL-only) | 14:00:12 | 2.4 | 6 | |
| VPPS (GAL-only) | 14:00:54 | 2.4 | 6 | |
| STATIC (GGGB) | 13:30–13:52 | |||
| 8. (A-8) | VPPS (GGG) | 14:26:50 | 1.6 | 23 |
| VPPS (GGG) | 14:27:30 | 1.3 | 23 | |
| VPPS (GGG) | 14:28:06 | 1.3 | 23 | |
| VPPS (GAL-only) | 14:30:22 | 3.0 | 6 | |
| VPPS (GAL-only) | 14:30:56 | 3.0 | 6 | |
| VPPS (GAL-only) | 14:31:33 | 3.0 | 6 | |
| STATIC (GGGB) | 14:32–14:55 | |||
| 9. (A-9) | VPPS (GGG) | 16:13:59 | 1.1 | 25 |
| VPPS (GGG) | 16:14:32 | 1.0 | 26 | |
| VPPS (GGG) | 16:15:08 | 1.0 | 26 | |
| VPPS (GAL-only) | 16:17:08 | 1.6 | 7 | |
| VPPS (GAL-only) | 16:17:43 | 2.1 | 6 | |
| VPPS (GAL-only) | ---- | ---- | ---- | |
| STATIC (GGGB) | 16:31–16:53 |
| Solution | #SAT | PDOP | Hz Precision | Vt Precision | |
|---|---|---|---|---|---|
| GGG | MAX | 26 | 1.620 | 0.0043 | 0.0112 |
| MIN | 15 | 1.012 | 0.0033 | 0.0050 | |
| GAL-only | MAX | 7 | 3.910 | 0.0124 | 0.0218 |
| MIN | 5 | 1.602 | 0.0044 | 0.0062 |
| Session | Number of SV (RINEX): GPS + GLO + GAL | Time (CET) | Number of SV (RINEX): GAL | Number of SV: VPPS (GGG) | Time (CET) | Number of SV: VPPS (GAL-only) | Time (CET) |
|---|---|---|---|---|---|---|---|
| 2. | 6 + 4 + 6 = 16 | 08:25 | 6 | 17 | 08:12 | 5 | 08:22 |
| 3. | 8 + 5 + 6 = 19 | 09:38 | 6 | 16 | 09:32 | 6 | 09:36 |
| 4. | 8 + 6 + 6 = 20 | 11:05 | 6 | 18 | 10:32 | 6 | 11:03 |
| 5. | 8 + 5 + 6 = 19 | 12:01 | 6 | 19 | 11:55 | 6 | 11:58 |
| 6. | 9 + 5 + 8 = 22 | 13:02 | 8 | 18 | 12:55 | 7 | 13:00 |
| 7. | 9 + 7 + 6 = 22 | 13:52 | 6 | 23 | 13:58 | 6 | 14:00 |
| 8. | 8 + 6 + 7 = 21 | 14:32 | 7 | 23 | 14:28 | 6 | 14:31 |
| Solution | Range (E) | Range (N) | Range (H) |
|---|---|---|---|
| VPPS (GGG) | 9.5 mm | 7.6 mm | 17.1 mm |
| VPPS (GAL-only) | 8.1 mm | 11.4 mm | 21.7 mm |
| GPPS (GGGB) | 5.6 mm | 5.4 mm | 5.3 mm |
| GPPS (GAL-only) | 8.7 mm | 7.9 mm | 19.4 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Šugar, D.; Kliman, A.; Bačić, Ž.; Nevistić, Z. Assessment of GNSS Galileo Contribution to the Modernization of CROPOS’s Services. Sensors 2023, 23, 2466. https://doi.org/10.3390/s23052466
Šugar D, Kliman A, Bačić Ž, Nevistić Z. Assessment of GNSS Galileo Contribution to the Modernization of CROPOS’s Services. Sensors. 2023; 23(5):2466. https://doi.org/10.3390/s23052466
Chicago/Turabian StyleŠugar, Danijel, Ana Kliman, Željko Bačić, and Zvonimir Nevistić. 2023. "Assessment of GNSS Galileo Contribution to the Modernization of CROPOS’s Services" Sensors 23, no. 5: 2466. https://doi.org/10.3390/s23052466
APA StyleŠugar, D., Kliman, A., Bačić, Ž., & Nevistić, Z. (2023). Assessment of GNSS Galileo Contribution to the Modernization of CROPOS’s Services. Sensors, 23(5), 2466. https://doi.org/10.3390/s23052466







