Performance Characterization of a Fully Transportable Mid-Infrared Laser Heterodyne Radiometer (LHR)
Abstract
1. Introduction
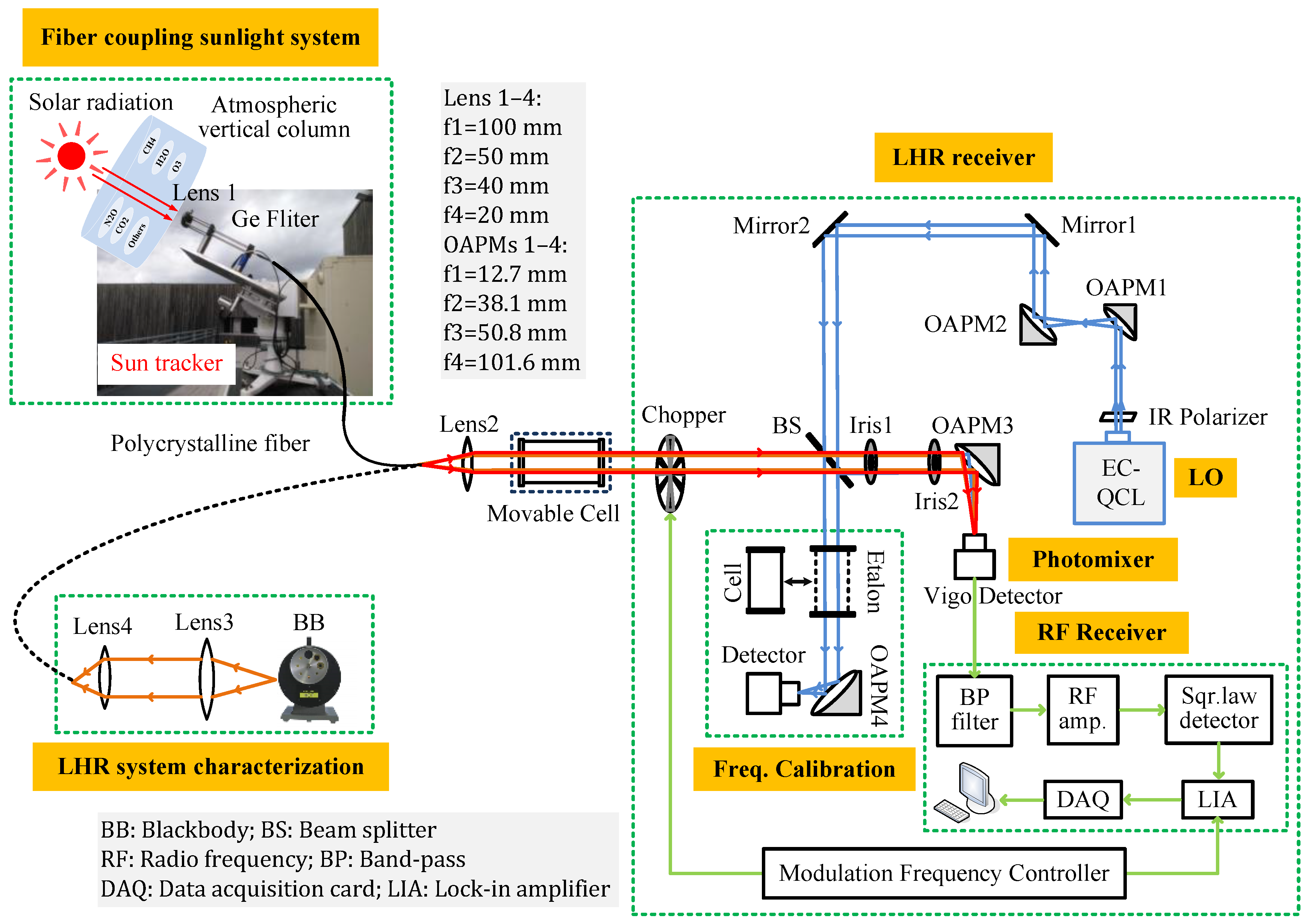
2. Instrumental Description
3. Performance Characterization of the MIR LHR
3.1. SNR of the MIR LHR
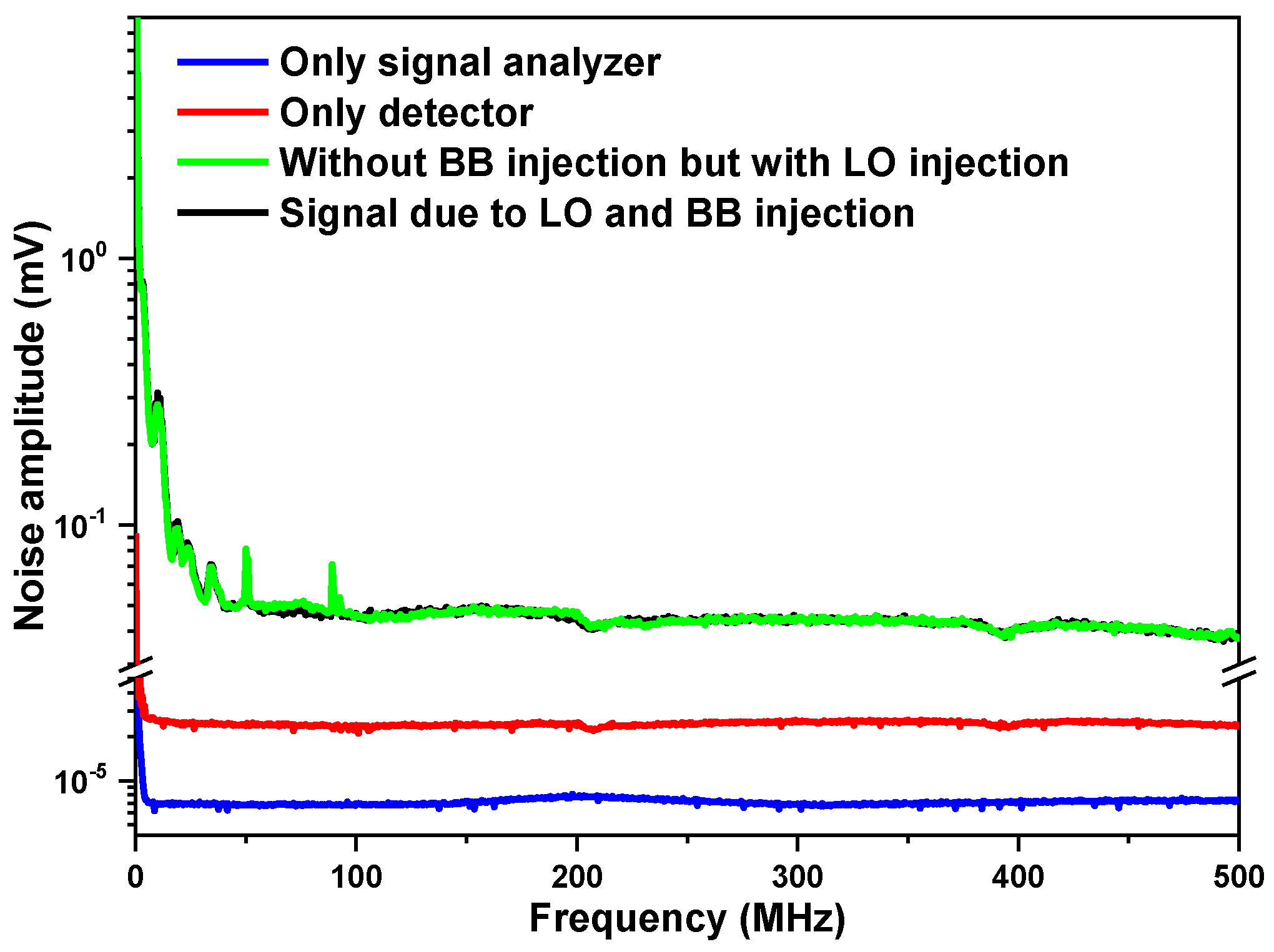
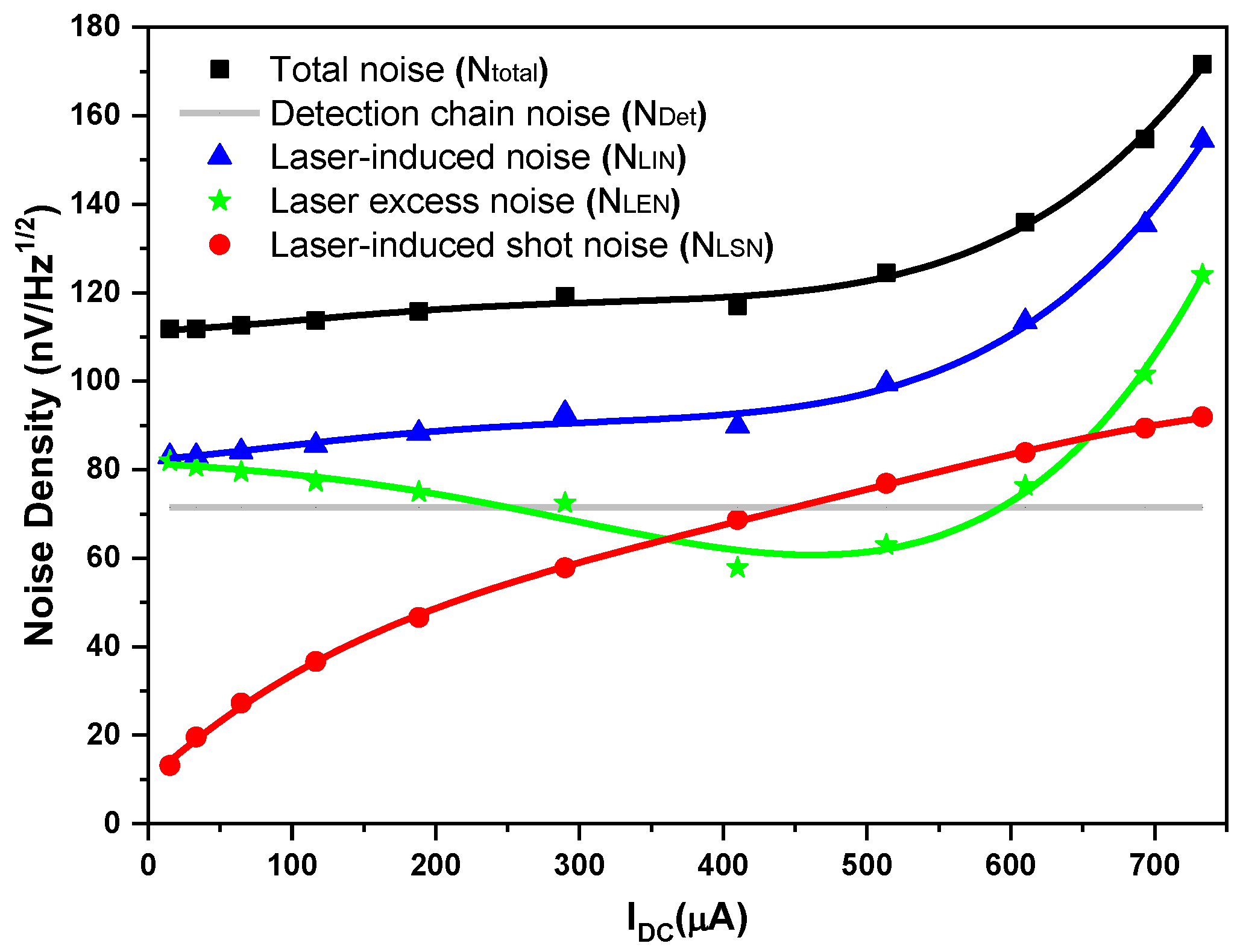
3.1.1. Noise Sources in the MIR LHR
- (1)
- Detection chain noise (NDet)
- (2)
- Laser-induced noise (NLIN)
- (2.1)
- Laser-induced shot noise (NLSN)
- (2.2)
- Laser excess noise (NLEN)
3.1.2. Theoretical SNR vs. Measured SNR
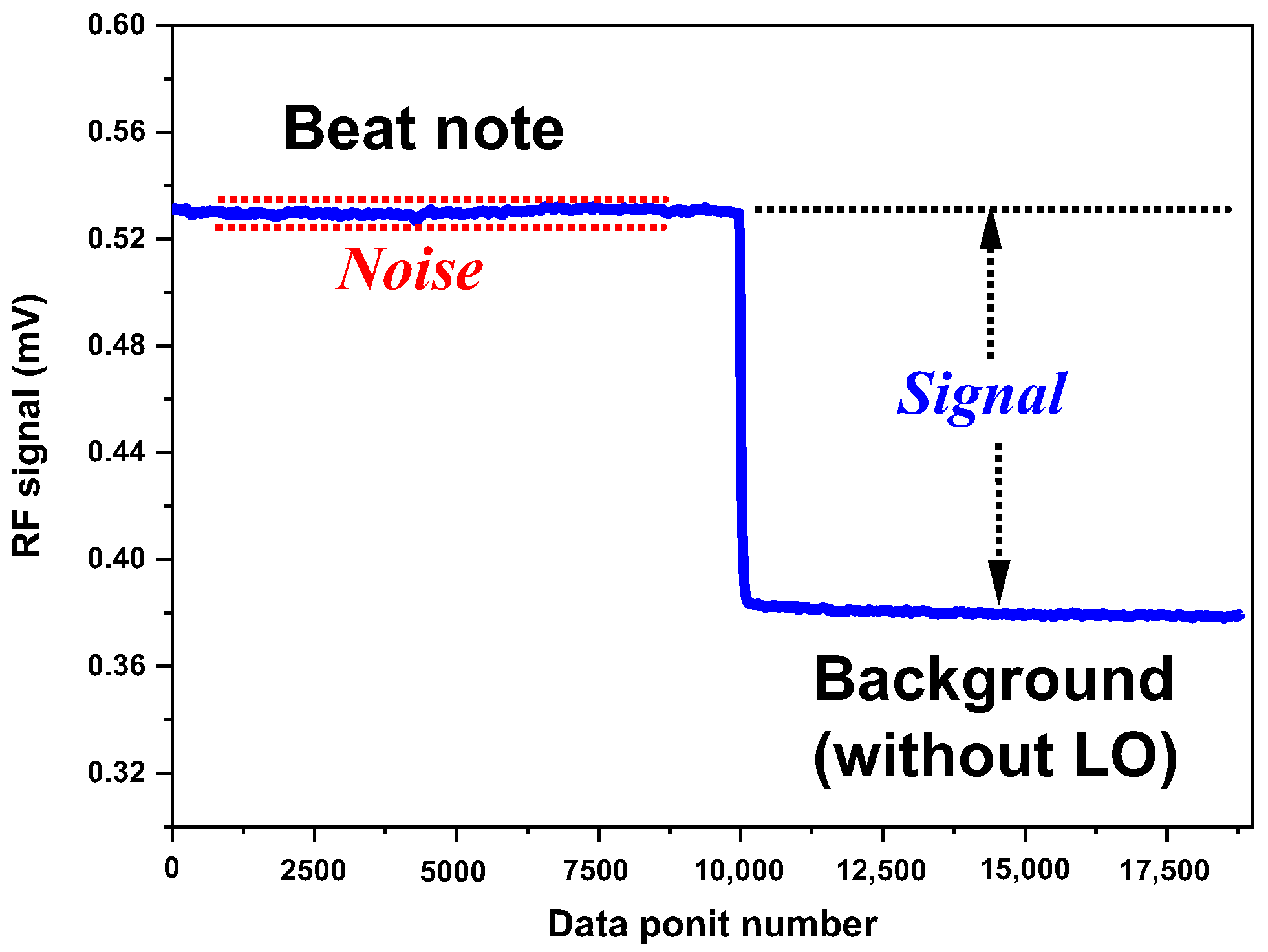
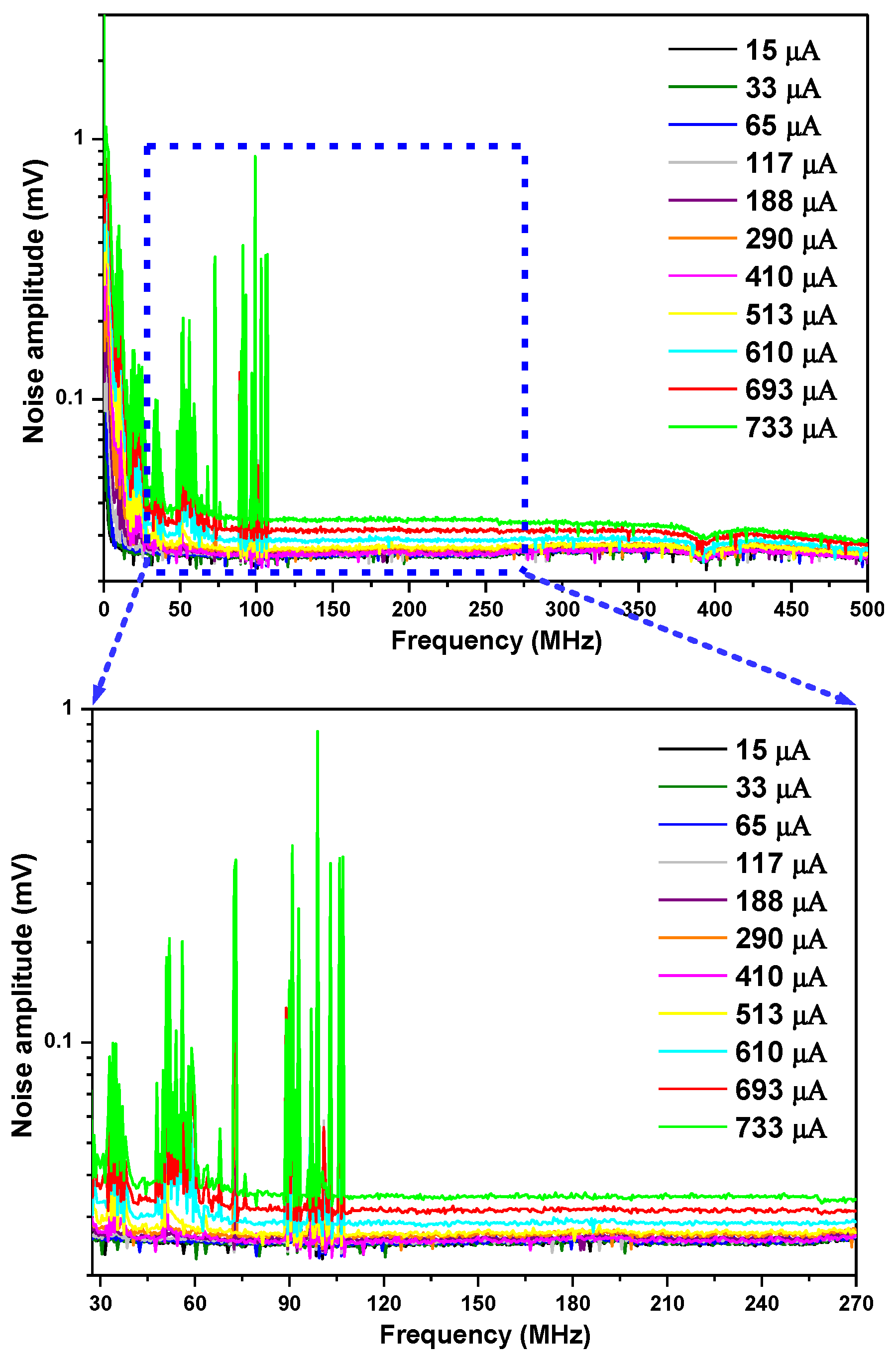
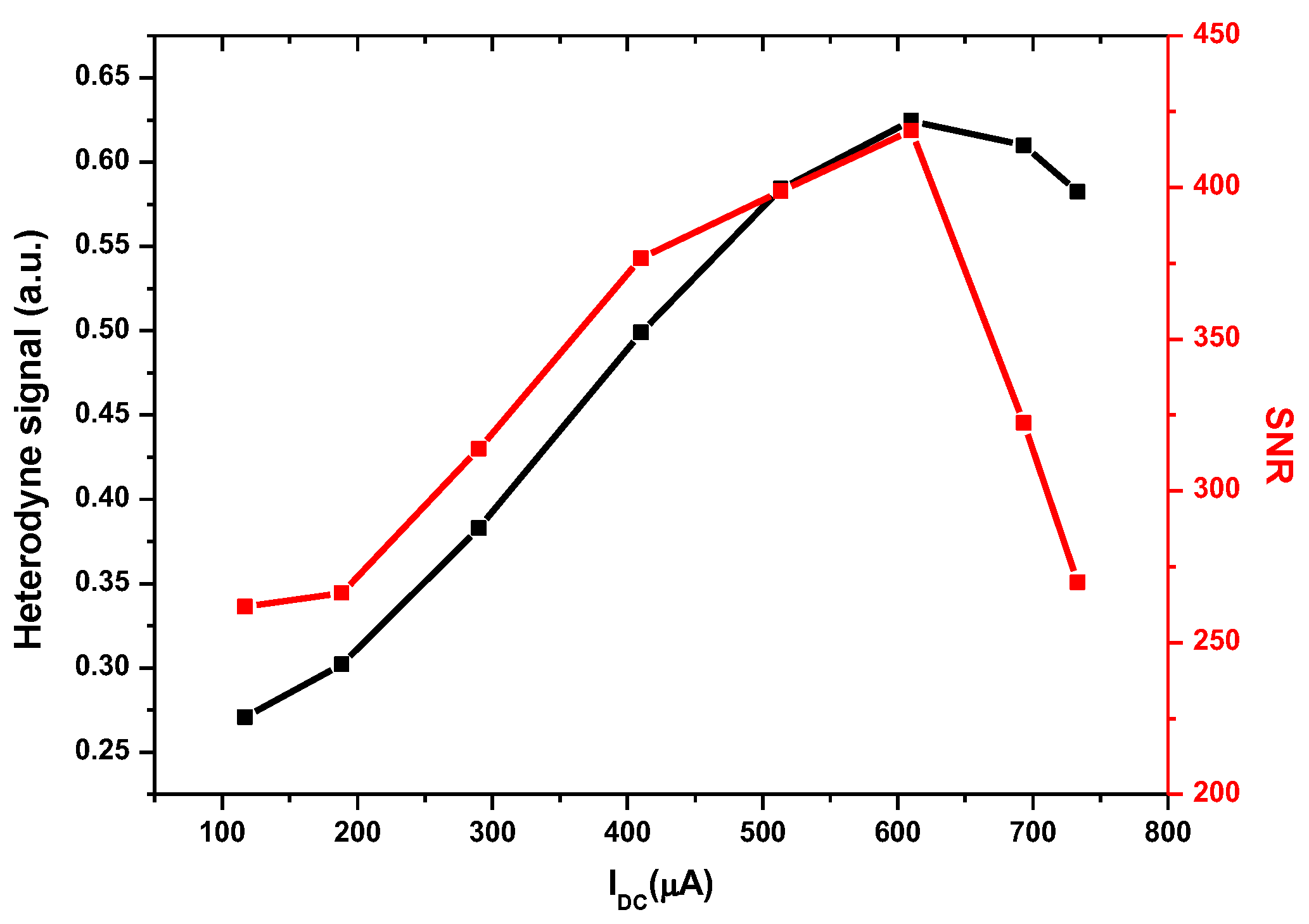
3.1.3. Heterodyne Signal and SNR of the LHR vs. LO Photocurrent
3.2. Spectroscopic Performance of the LHR System
3.2.1. Impact of RF Pass-Band on LHR Spectrum
3.2.2. LHR Spectral Line Shape vs. Scanning Speed of LO Frequency
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pidwirny, M. Atmospheric Composition, Fundamentals of Physical Geography, 2nd ed.; Date Viewed; University of British Columbia Okanagan: Kelowna, BC, Canada, 2006. [Google Scholar]
- Tsai, T. External Cavity Quantum Cascade Lasers for Spectroscopic Applications. Ph.D. Dissertation, Princeton University, Princeton, NJ, USA, 2012. [Google Scholar]
- Qiao, S.; Ma, P.; Tsepelin, V.; Han, G.; Liang, J.; Ren, W.; Zheng, H.; Ma, Y. Super tiny quartz-tuning-fork-based light-induced thermoelastic spectroscopy sensing. Opt. Lett. 2023, 48, 419–422. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Ma, Y. Advances in multipass cell for absorption spectroscopy-based trace gas sensing technology. Chin. Opt. Lett. 2023, 21, 033001. [Google Scholar] [CrossRef]
- Menzies, R.T.; Chahine, M.T. Remote Atmospheric Sensing with an Airborne Laser Absorption Spectrometer. Appl. Opt. 1974, 13, 2840–2849. [Google Scholar] [CrossRef] [PubMed]
- Menzies, R.T. Ozone spectroscopy with a CO2 waveguide laser. Appl. Opt. 1976, 15, 2597–2599. [Google Scholar] [CrossRef]
- Frerking, M.A.; Muehlner, D.J. Infrared heterodyne spectroscopy of atmospheric ozone. Appl. Opt. 1977, 16, 526–528. [Google Scholar] [CrossRef] [PubMed]
- Shen, F.; Wang, G.; Wang, J.; Tan, T.; Wang, G.; Jeseck, P.; Té, Y.V.; Gao, X.; Chen, W. Transportable mid-infrared laser heterodyne radiometer operating in the shot-noise dominated regime. Opt. Lett. 2021, 46, 3171–3174. [Google Scholar] [CrossRef]
- Shen, F. Development of a laser heterodyne radiometer for atmospheric remote sensing. In Instrumentation and Detectors [physics.ins-det]; Université du Littoral Côte d’Opale: Dunkirk, France, 2019. (In English) [Google Scholar]
- Wilson, E.L.; McLinden, M.L.; Miller, J.H. Miniaturized laser heterodyne radiometer for measurements of CO2 in the atmospheric column. Appl. Phys. B 2014, 114, 385–393. [Google Scholar] [CrossRef]
- Melroy, H.R.; Wilson, E.L.; Clarke, G.B.; Ott, L.E.; Mao, J.; Ramanathan, A.K.; McLinden, M.L. Autonomous field measurements of CO2 in the atmospheric column with the miniaturized laser heterodyne radiometer (Mini-LHR). Appl. Phys. B 2015, 120, 609–615. [Google Scholar] [CrossRef]
- Wilson, E.L.; DiGregorio, A.J.; Villanueva, G.; Grunberg, C.E.; Souders, Z.K.; Miletti, M.; Menendez, A.; Grunberg, M.H.; Floyd, M.A.M.; Bleacher, J.E.; et al. A portable miniaturized laser heterodyne radiometer (mini-LHR) for remote measurements of column CH4 and CO2. Appl. Phys. B 2019, 125, 211. [Google Scholar] [CrossRef]
- Zenevich, S.G.; Klimchuk, A.U.; Semenov, V.M.; Spiridonov, M.V.; Rodin, A.V. Measurements of the fully resolved contour of the carbon dioxide absorption line in a band at λ = 1.605 μm in the atmospheric column using high-resolution heterodyne spectroradiometry method. Quantum Electron. 2019, 49, 604–611. [Google Scholar] [CrossRef]
- Rodin, A.; Klimchuk, A.; Nadezhdinskiy, A.; Churbanov, D.; Spiridonov, M. High resolution heterodyne spectroscopy of atmospheric methane NIR absorption. Opt. Express 2014, 22, 13825–13834. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Sun, C.; Wang, G.; Zou, M.; Tan, T.; Liu, K.; Chen, W.; Gao, X. A fibered near-infrared laser heterodyne radiometer for simultaneous remote sensing of atmospheric CO2 and CH4. Opt. Laser Eng. 2020, 129, 106083. [Google Scholar] [CrossRef]
- Wang, J.; Wang, G.; Tan, T.; Zhu, G.; Sun, C.; Cao, Z.; Gao, X.; Chen, W. Mid-infrared laser heterodyne radiometer (LHR) based on a 3.53 μm room-temperature interband cascade laser. Opt. Express 2019, 27, 610–9619. [Google Scholar]
- Xue, Z.; Shen, F.; Li, J.; Liu, X.; Wang, G.; Liu, K.; Gao, X.; Chen, W.; Tan, T. MEMS Modulator-Based Mid-Infrared Laser Heterodyne Radiometer for Atmospheric Remote Sensing. Front. Phys. 2022, 10, 945995. [Google Scholar] [CrossRef]
- Xue, Z.; Shen, F.; Li, J.; Liu, X.; Wang, G.; Liu, K.; Gao, X.; Chen, W.; Tan, T. A MEMS modulator-based dual-channel mid-infrared laser heterodyne radiometer for simultaneous remote sensing for atmospheric N2O, CH4 and HDO. Opt. Express. 2022, 30, 31828–31839. [Google Scholar] [CrossRef] [PubMed]
- Shen, F.; Akil, J.; Wang, G.; Poupin, C.; Cousin, R.; Siffert, S.; Fertein, E.; Ba, T.; Chen, W. Real-time monitoring of N2O production in a catalytic reaction process using mid-infrared quantum cascade laser. J. Quant. Spectrosc. Radiat. Transf. 2018, 221, 1–7. [Google Scholar] [CrossRef]
- Tsai, T.; Rose, R.A.; Weidmann, D.; Wysocki, G. Atmospheric vertical profiles of O3, N2O, CH4, CCl2F2, and H2O retrieved from external-cavity quantum-cascade laser heterodyne radiometer measurements. Appl. Opt. 2012, 51, 8779–8792. [Google Scholar] [CrossRef] [PubMed]
- Weidmann, D.; Tsai, T.; Macleod, N.A.; Wysocki, G. Atmospheric observations of multiple molecular species using ultra-high-resolution external cavity quantum cascade laser heterodyne radiometry. Opt. Lett. 2011, 36, 1951–1953. [Google Scholar] [CrossRef]
- Weidmann, D.; Courtois, D. Infrared 7.6 µm lead-salt diode laser heterodyne radiometry of water vapor in a CH4-air premixed flat flame. Appl. Opt. 2003, 42, 1115–1121. [Google Scholar] [CrossRef]
- Weidmann, D. Radiometrie Heterodyne Infrarouge par Diode Laser Accordable. Ph.D. Dissertation, Reims University, Reims, France, 2002. [Google Scholar]
- Blaney, T.G. Signal-to-noise ratio and other characteristics of heterodyne radiation receivers. Space Sci. Rev. 1975, 17, 691–702. [Google Scholar] [CrossRef]
- Hoffmann, A.; Macleod, N.A.; Huebner, M.; Weidmann, D. Thermal infrared laser heterodyne spectroradiometry for solar occultation atmospheric CO2 measurements. Atmos. Meas. Tech. 2016, 9, 5975–5996. [Google Scholar] [CrossRef]
- Niwuwenhuijzen, H. Photo-electric mixing of coherent and incoherent light. Bull. Astr. Inst. Neth. 1967, 19, 391–404. [Google Scholar]
- Horowitz, P.; Hill, W. The Art of Electronics, 2nd ed.; Cambridge University Press: Cambridge, UK, 1989; pp. 431–432. [Google Scholar]
- Klimchuk, A.; Nadezhdinskii, A.; Ponurovskii, Y.; Shapovalov, Y.; Rodin, A. On the possibility of designing a high-resolution heterodyne spectrometer for near-IR range on the basis of a tunable diode laser. Quantum Electron. 2012, 42, 244–249. [Google Scholar] [CrossRef]
- Gordon, I.E.; Rothman, L.S.; Hill, C.; Kochanov, R.V.; Tan, Y.; Bernath, P.F.; Birk, M.; Boudon, V.; Campargue, A.; Chance, K.V.; et al. The HITRAN2016 molecular spectroscopic database. J. Quant. Spectrosc. Radiat. Transf. 2017, 203, 3–69. [Google Scholar] [CrossRef]
- Olivero, J.J.; Longbothum, R.L. Empirical fits to the Voigt line width: A brief review. J. Quant. Spectrosc. Radiat. Transf. 1977, 17, 233–236. [Google Scholar] [CrossRef]
- Armstrong, B.H. Spectrum line profiles: The Voigt function. J. Quant. Spectrosc. Radiat. Transf. 1967, 7, 61–88. [Google Scholar] [CrossRef]
- IPCC. Climate Change 2007: The Physical Science Basis. Contribution of Working Group I to the Fourth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Shen, F.; Wang, G.; Xue, Z.; Tan, T.; Cao, Z.; Gao, X.; Chen, W. Impact of lock-in time constant on remote monitoring of trace gas in the atmospheric column using laser heterodyne radiometer (LHR). Remote Sens. 2022, 14, 2923. [Google Scholar] [CrossRef]
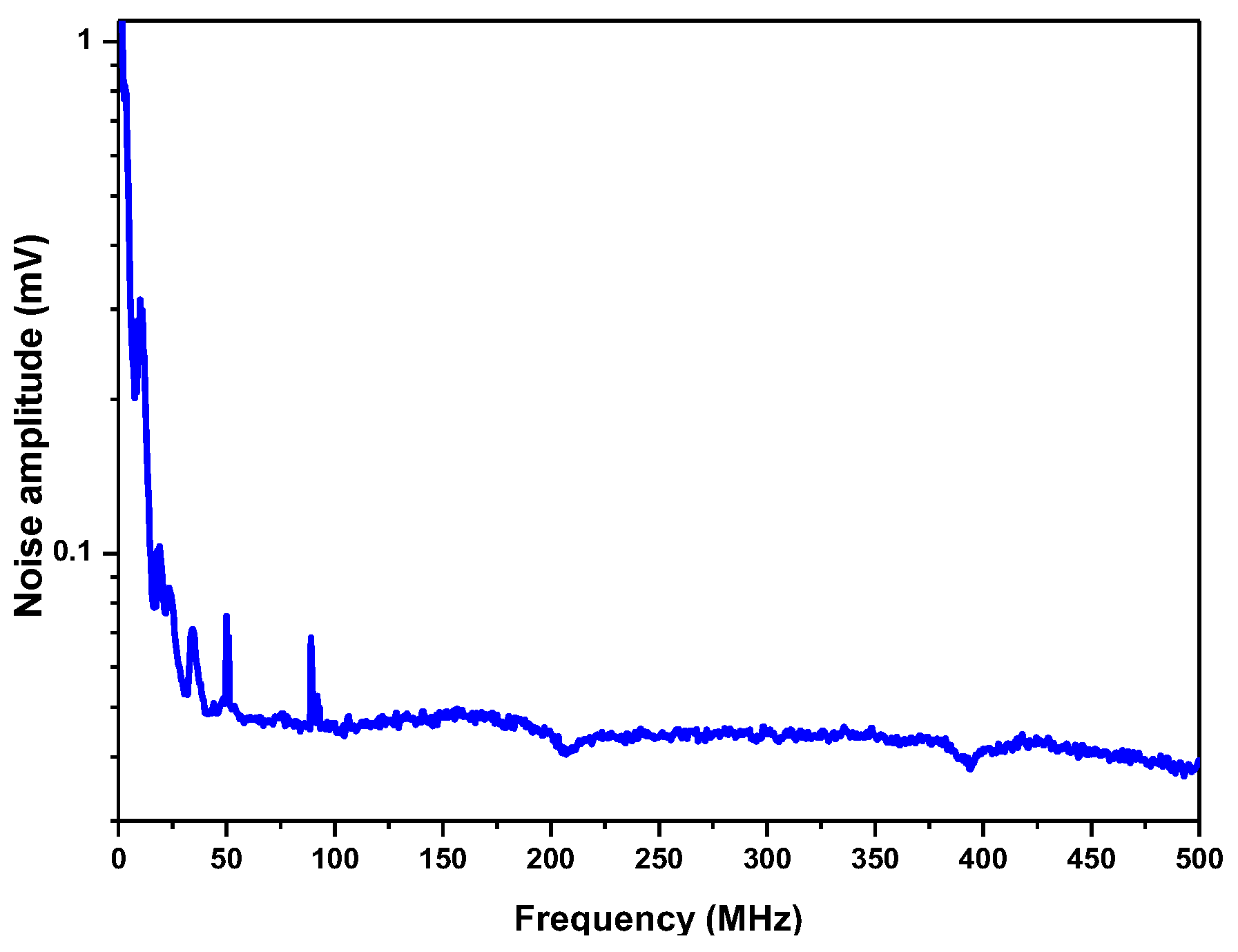
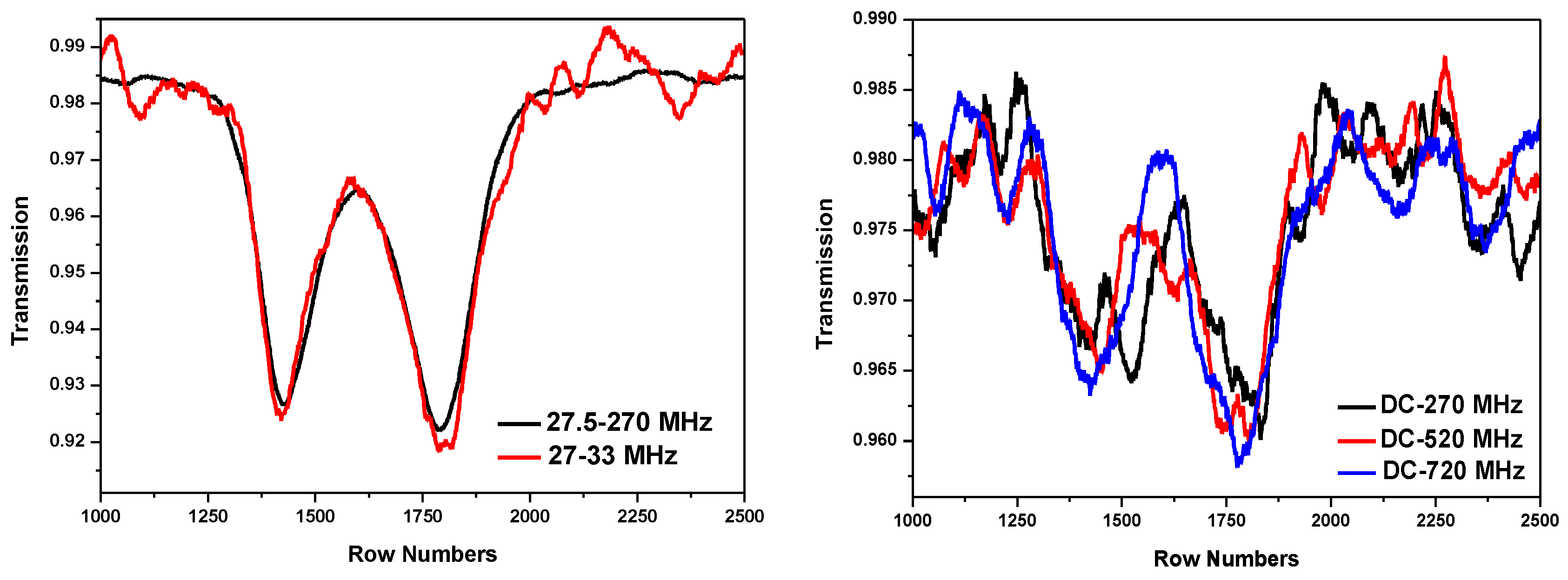

| Pass-Band (MHz) | DC-270 | DC-520 | DC-720 | 27–33 | 27.5–270 |
|---|---|---|---|---|---|
| Noise density () | 0.396 | 0.239 | 0.239 | 0.180 | 0.149 |
| τ (ms) | υsc (cm−1/s) | ΔTscan (ms) | Δνb (cm−1) | Absorption Depth | δν (cm−1) | Fit Residual (1σ) |
|---|---|---|---|---|---|---|
| 1 | 0.1574 | 89 | 0.038 | 0.1142 | 1.6 × 10−4 | 0.0091 |
| 0.3935 | 36 | 0.042 | 0.1037 | 4.0 × 10−4 | 0.0043 | |
| 0.7870 | 18 | 0.048 | 0.0975 | 8.2 × 10−4 | 0.0032 | |
| 1.1799 | 12 | 0.079 | 0.0909 | 1.2 × 10−3 | 0.0021 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, F.; Hu, X.; Lu, J.; Xue, Z.; Li, J.; Tan, T.; Cao, Z.; Gao, X.; Chen, W. Performance Characterization of a Fully Transportable Mid-Infrared Laser Heterodyne Radiometer (LHR). Sensors 2023, 23, 978. https://doi.org/10.3390/s23020978
Shen F, Hu X, Lu J, Xue Z, Li J, Tan T, Cao Z, Gao X, Chen W. Performance Characterization of a Fully Transportable Mid-Infrared Laser Heterodyne Radiometer (LHR). Sensors. 2023; 23(2):978. https://doi.org/10.3390/s23020978
Chicago/Turabian StyleShen, Fengjiao, Xueyou Hu, Jun Lu, Zhengyue Xue, Jun Li, Tu Tan, Zhensong Cao, Xiaoming Gao, and Weidong Chen. 2023. "Performance Characterization of a Fully Transportable Mid-Infrared Laser Heterodyne Radiometer (LHR)" Sensors 23, no. 2: 978. https://doi.org/10.3390/s23020978
APA StyleShen, F., Hu, X., Lu, J., Xue, Z., Li, J., Tan, T., Cao, Z., Gao, X., & Chen, W. (2023). Performance Characterization of a Fully Transportable Mid-Infrared Laser Heterodyne Radiometer (LHR). Sensors, 23(2), 978. https://doi.org/10.3390/s23020978







