0.5 V Versatile Voltage- and Transconductance-Mode Analog Filter Using Differential Difference Transconductance Amplifier
Abstract
1. Introduction
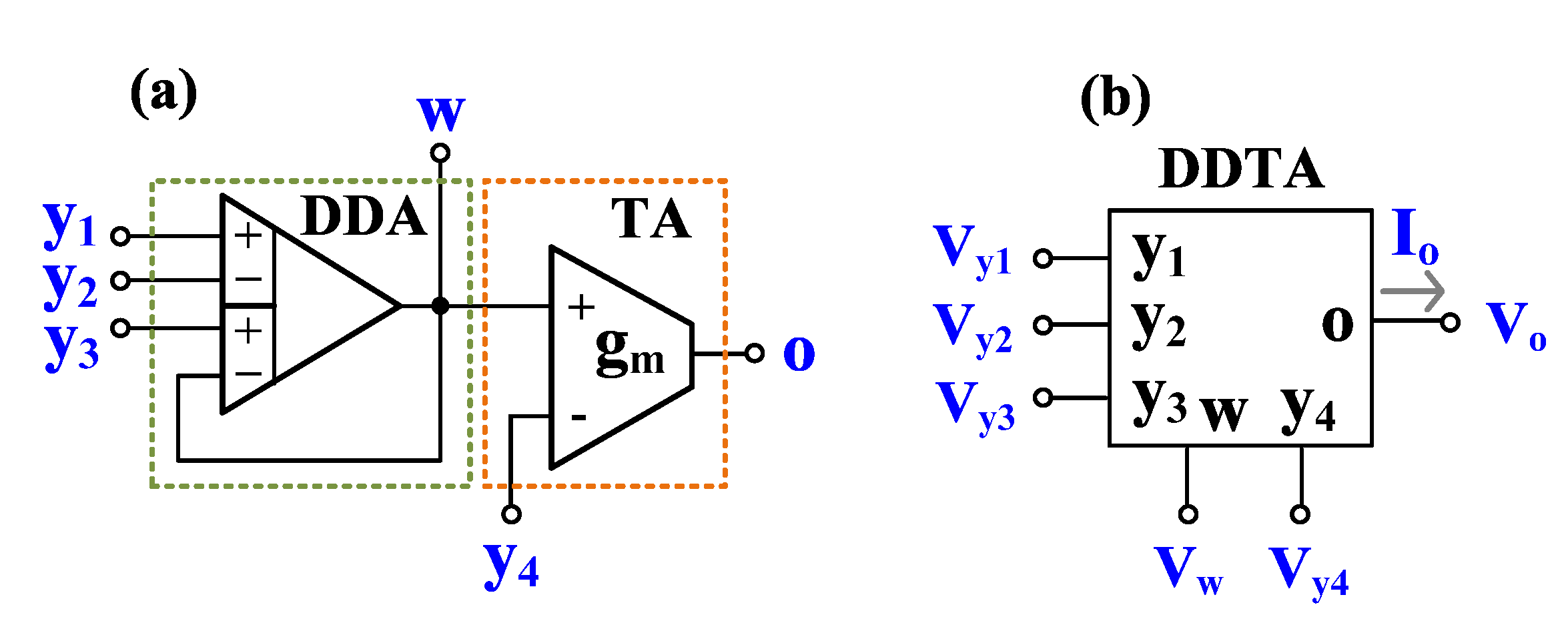
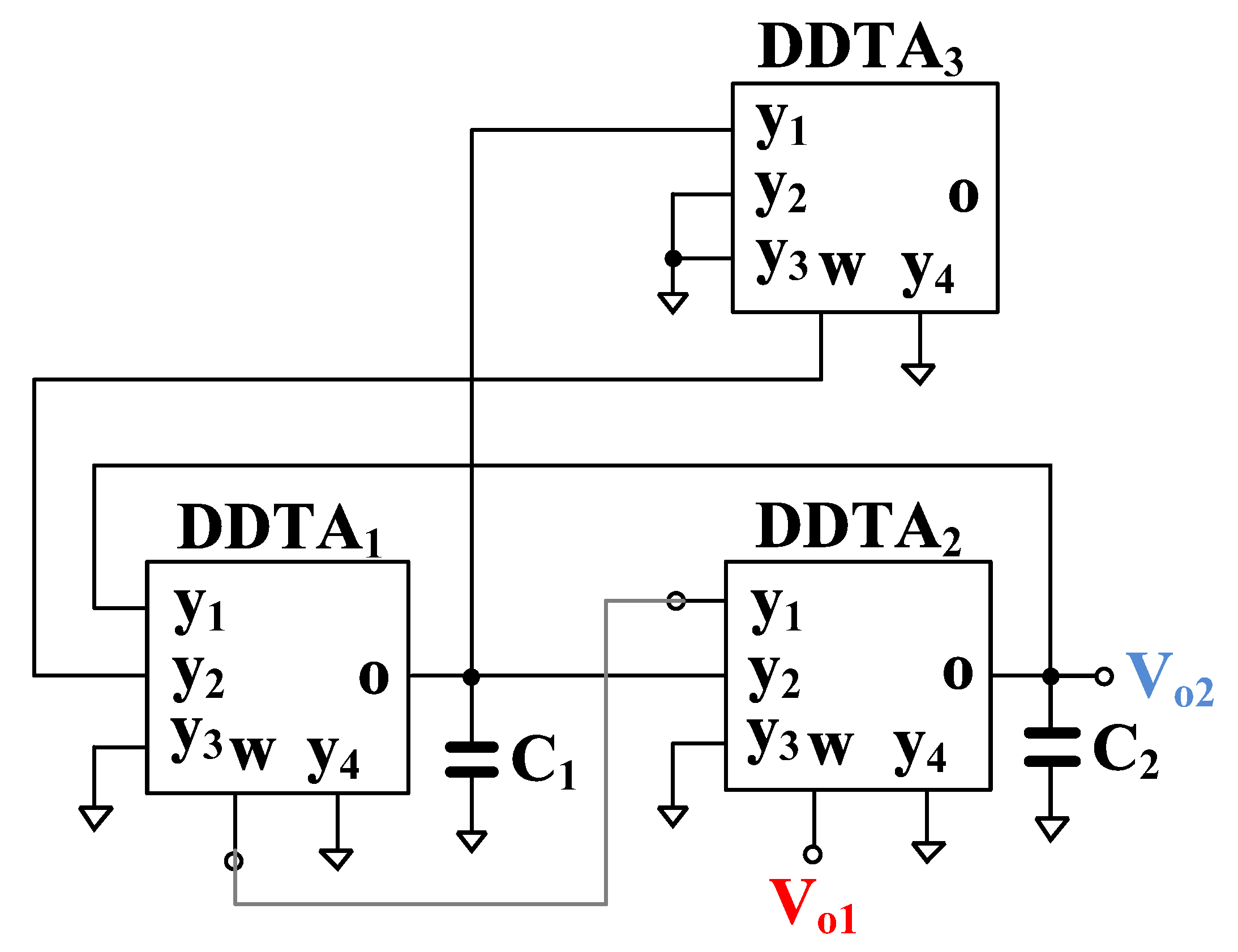
2. Proposed Circuit
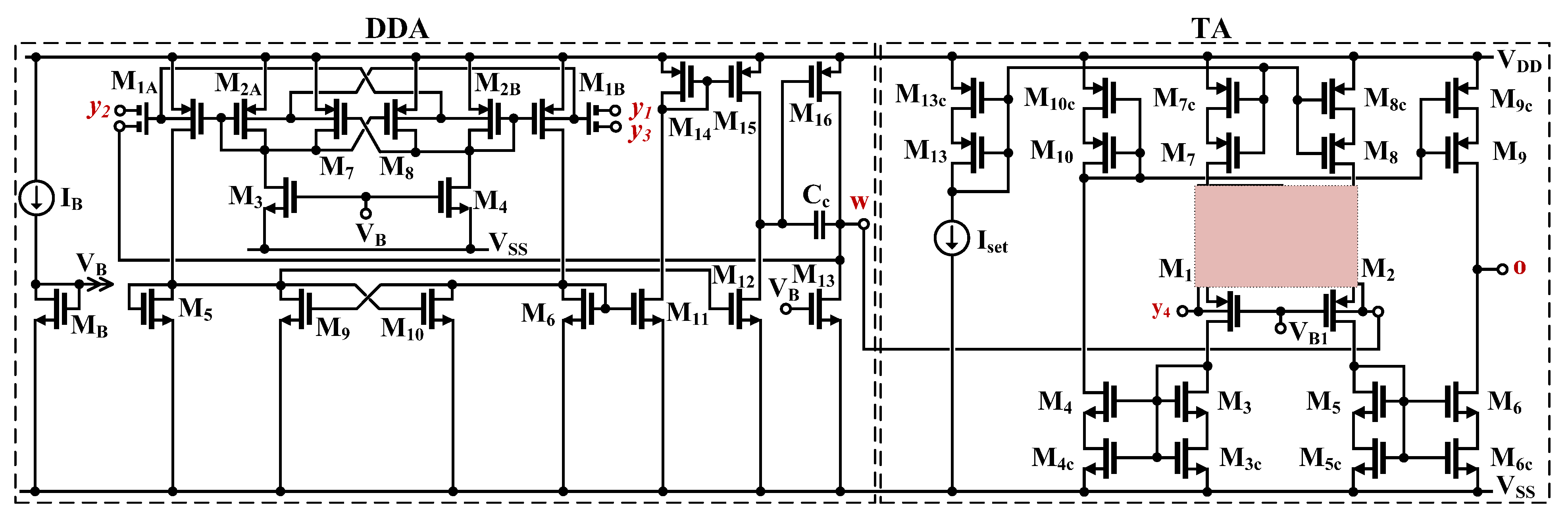
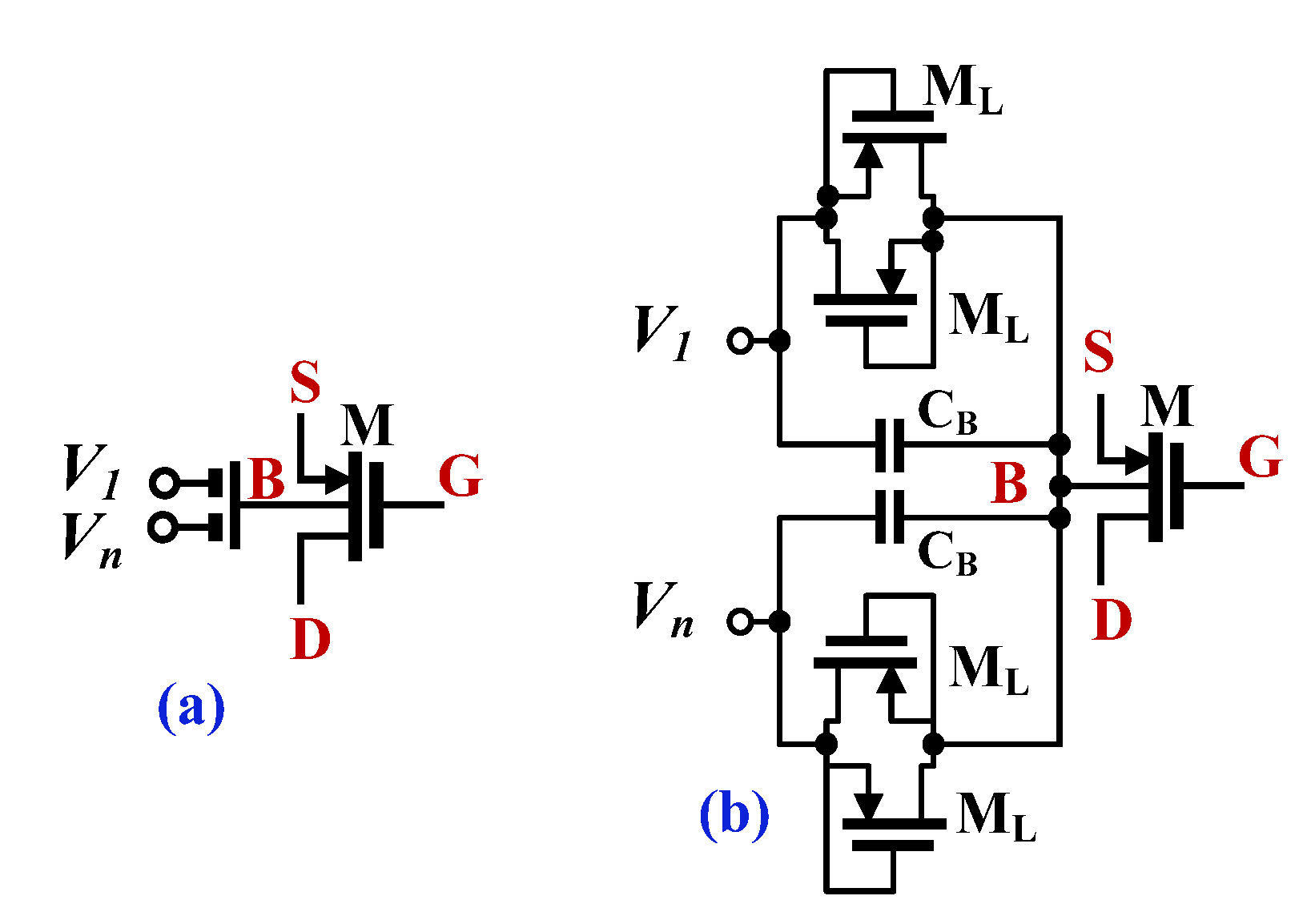
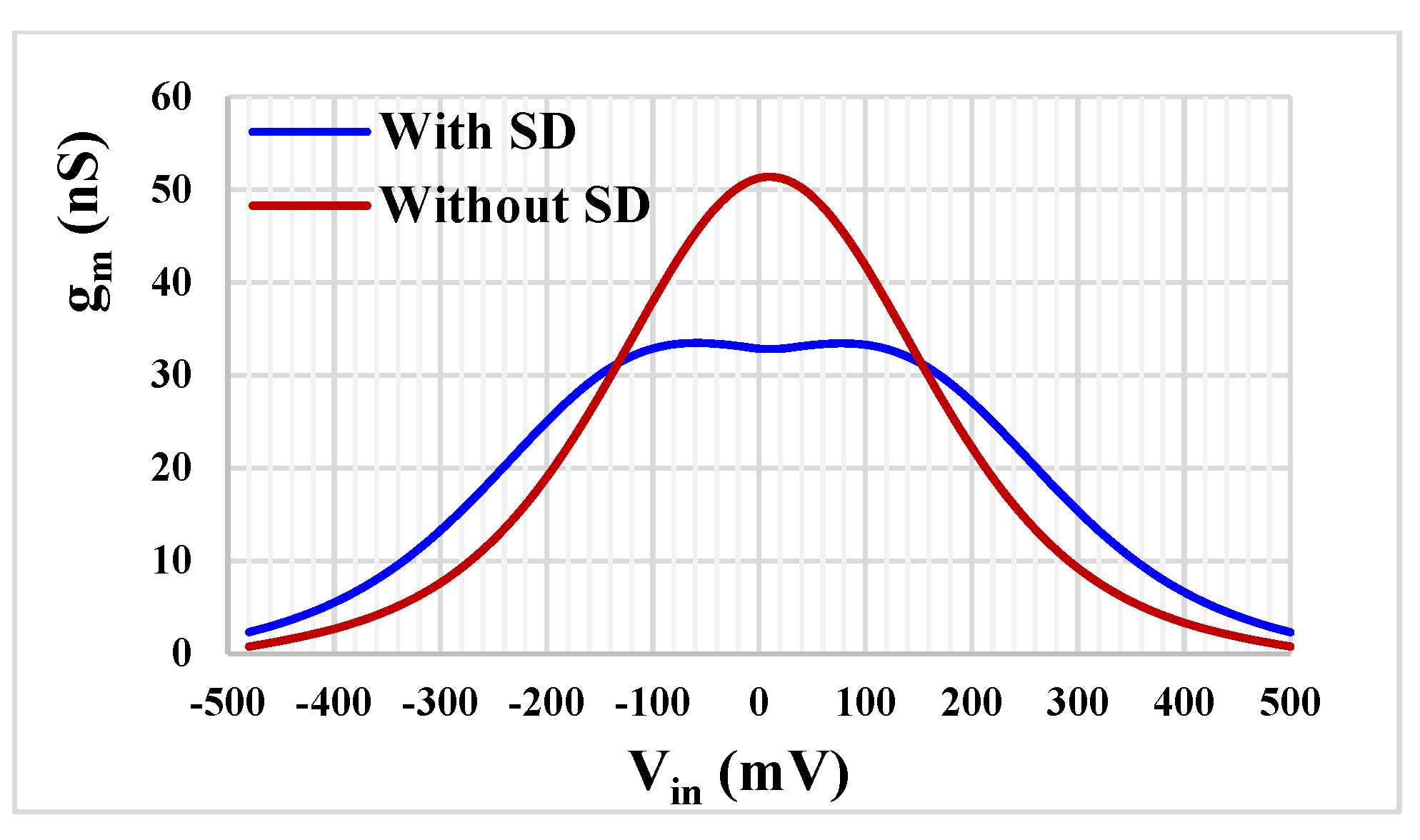
2.1. 0.5 V DDTA
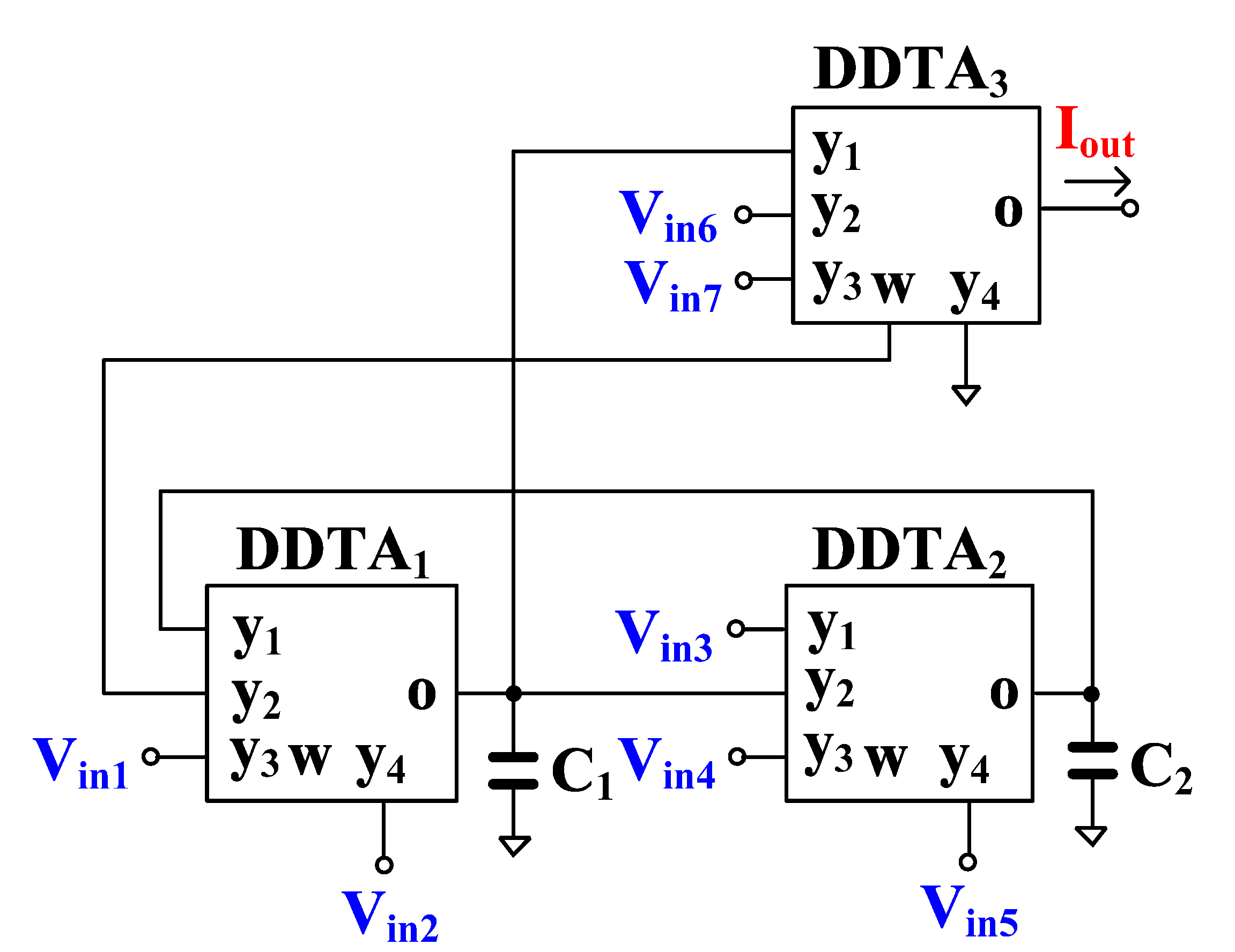
2.2. Versatile Analog Filter
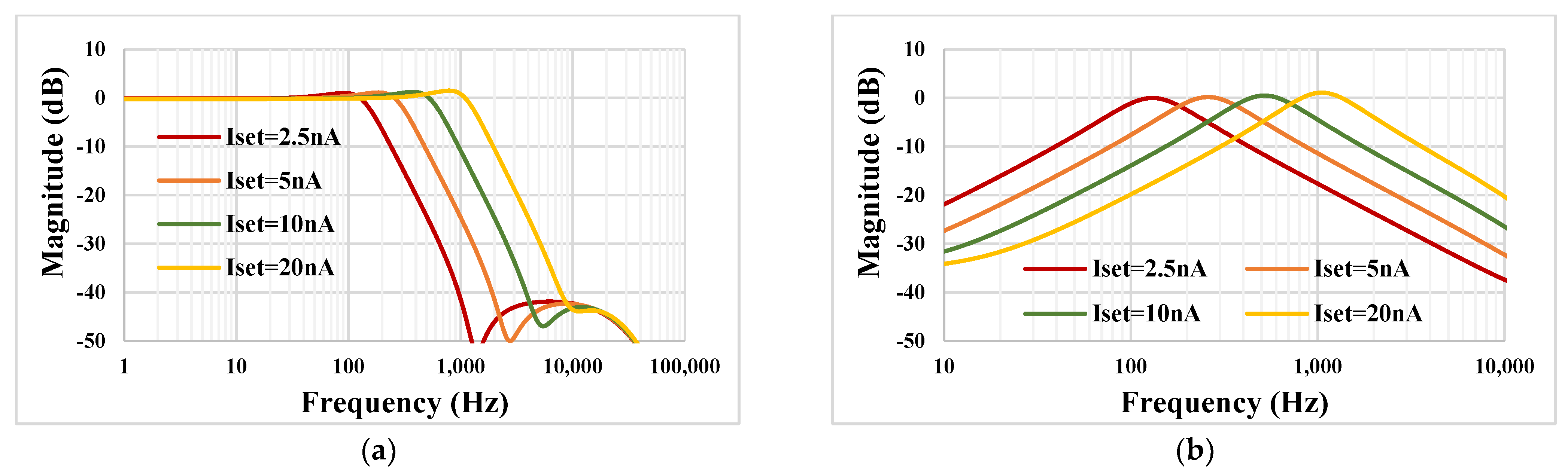
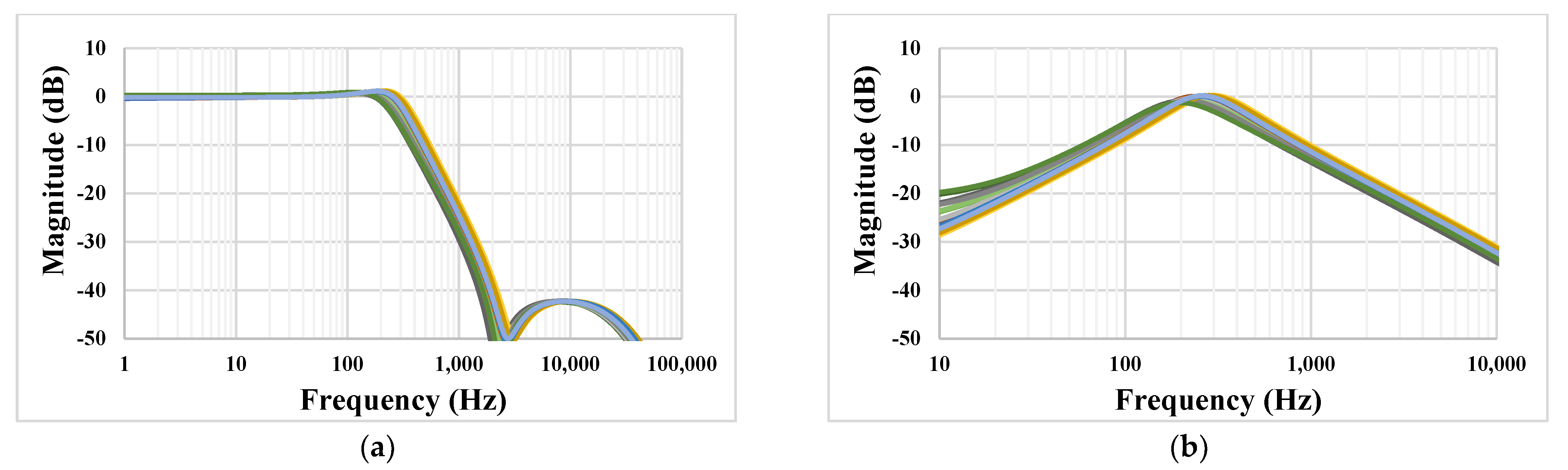
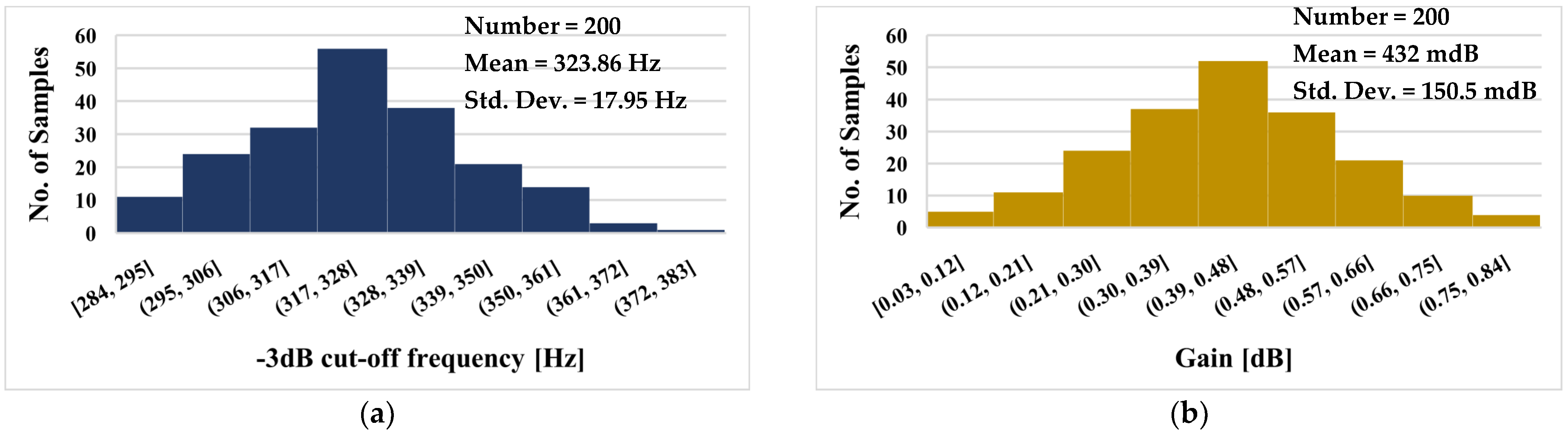
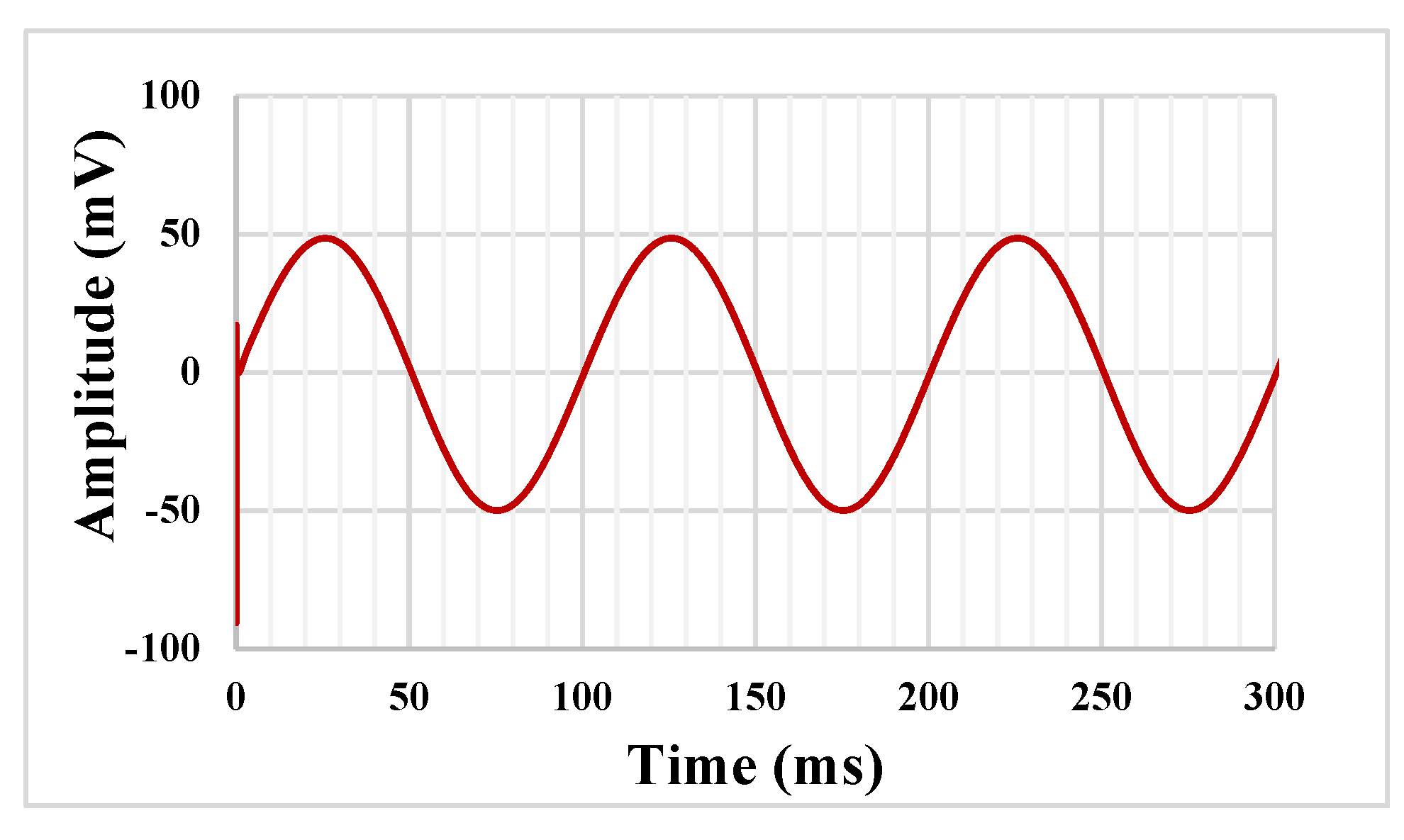
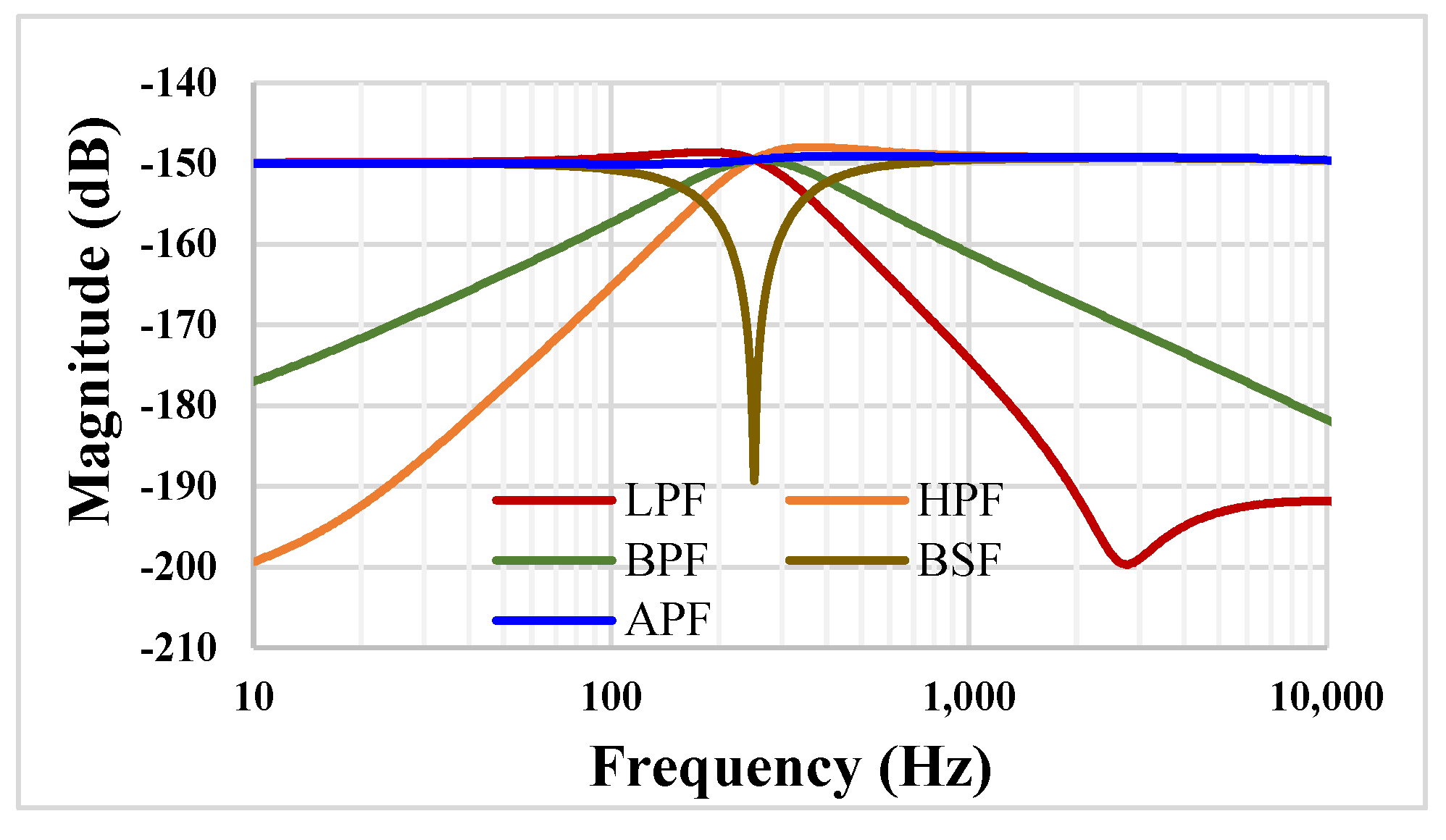
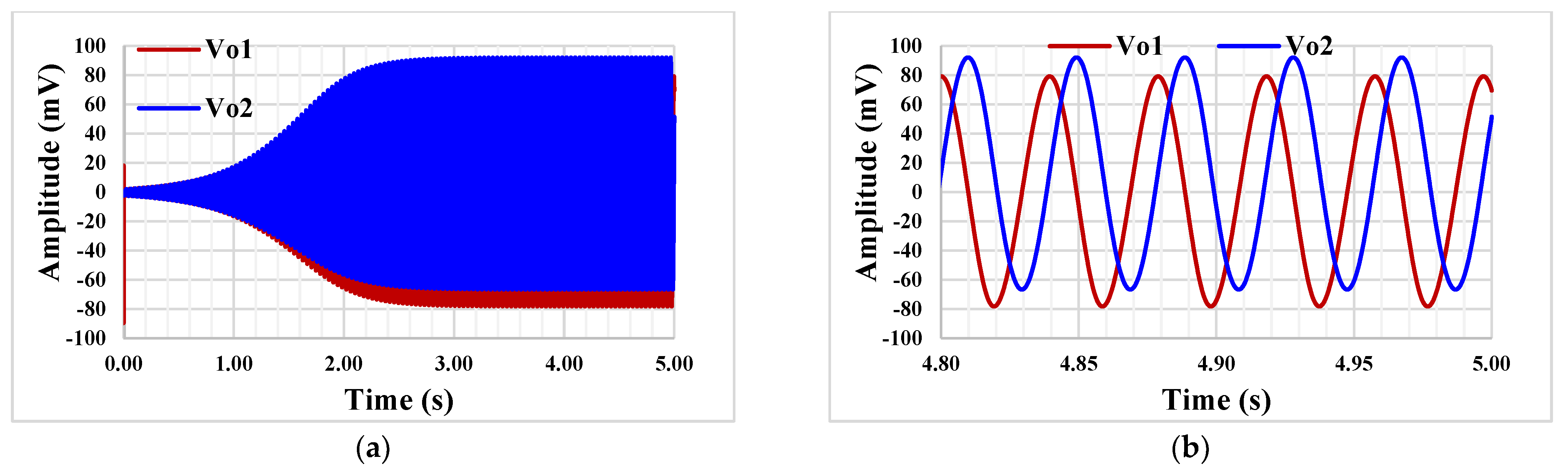
3. Simulation Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Sackinger, E.; Guggenbuhl, W. A versatile building block: The CMOS differential difference amplifier. IEEE J. Solid-State Circuits 1987, 22, 287–294. [Google Scholar] [CrossRef]
- Huang, S.-C.; Ismail, M.; Zarabadi, S.R. A wide range differential difference amplifier: A basic block for analog signal processing in MOS technology. IEEE Trans. Circuits Syst. II Express Briefs 1993, 40, 289–301. [Google Scholar] [CrossRef]
- Duque-Carrillo, J.; Torelli, G.; Perez-Aloe, R.; Valverde, J.; Maloberti, F. Fully differential basic building blocks based on fully differential difference amplifiers with unity-gain difference feedback. IEEE Trans. Circuits Syst. I Regul. Pap. 1995, 42, 190–192. [Google Scholar] [CrossRef]
- Chiu, W.; Liu, S.-I.; Tsao, H.-W.; Chen, J.-J. CMOS differential difference current conveyors and their applications. IEE Proc. Circuits Devices Syst. 1996, 143, 91–96. [Google Scholar] [CrossRef]
- Pandey, N.; Paul, S.K. Differential difference current conveyor transconductance amplifier: A new analog building block for signal processing. J. Electr. Comput. Eng. 2011, 2011, 1–10. [Google Scholar] [CrossRef]
- Kumngern, M. DDTA and DDCCTA: New active elements for analog signal processing. In Proceedings of the 2012 IEEE International Conference on Electronics Design, Systems and Applications (ICEDSA), Kuala Lumpur, Malaysia, 5–6 November 2012; pp. 141–145. [Google Scholar] [CrossRef]
- Sedra, A.; Smith, K. A second-generation current conveyor and its applications. IEEE Trans. Circuit Theory 1970, 17, 132–134. [Google Scholar] [CrossRef]
- Kumngern, M.; Khateb, F.; Dejhan, K.; Phasukkit, P.; Tungjitkusolmun, S. Voltage-mode multifunction biquadratic filters using new ultra-low-power differential difference current conveyors. Radioengineering 2013, 22, 448–457. [Google Scholar]
- Lee, C.-N. Independently tunable plus-type DDCC-based voltage-mode universal biquad filter with MISO and SIMO types. Microelectron. J. 2017, 67, 71–81. [Google Scholar] [CrossRef]
- Abaci, A.; Yuce, E. Single DDCC− based simulated floating inductors and their applications. IET Circuits, Devices Syst. 2020, 14, 796–804. [Google Scholar] [CrossRef]
- Unuk, T.; Yuce, E. Supplementary DDCC+ based universal filter with grounded passive elements. AEU Int. J. Electron. Commun. 2021, 132, 153652. [Google Scholar] [CrossRef]
- Orman, K.; Yesil, A.; Babacan, Y. DDCC-based meminductor circuit with hard and smooth switching behaviors and its circuit implementation. Microelectron. J. 2022, 125, 105462. [Google Scholar] [CrossRef]
- Kumngern, M. CMOS differential difference voltage follower transconductance amplifier. In Proceedings of the 2015 IEEE International Circuits and Systems Symposium (ICSyS), Langkawi, Malaysia, 2–4 September 2015; pp. 133–136. [Google Scholar] [CrossRef]
- Rana, P.; Ranjan, A. Odd- and even-order electronically controlled wave active filter employing differential difference trans-conductance amplifier (DDTA). Int. J. Electron. 2021, 108, 1623–1651. [Google Scholar] [CrossRef]
- Kumngern, M.; Suksaibul, P.; Khateb, F.; Kulej, T. 1.2 V differential difference transconductance amplifier and its application in mixed-mode universal filter. Sensors 2022, 22, 3535. [Google Scholar] [CrossRef] [PubMed]
- Khateb, F.; Kumngern, M.; Kulej, T.; Biolek, D. 0.5 V differential difference transconductance amplifier and its application in voltage-mode universal filter. IEEE Access 2022, 10, 43209–43220. [Google Scholar] [CrossRef]
- Kumngern, M.; Suksaibul, P.; Khateb, F.; Kulej, T. Electronically tunable universal filter and quadrature oscillator using low-voltage differential difference transconductance amplifiers. IEEE Access 2022, 10, 68965–68980. [Google Scholar] [CrossRef]
- Khateb, F.; Kumngern, M.; Kulej, T.; Biolek, D. 0.3-volt rail-to-rail DDTA and its application in a universal filter and quadrature oscillator. Sensors 2022, 22, 2655. [Google Scholar] [CrossRef]
- Horowitz, P.; Hill, W. The Art of Electronics; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Gift, S.J.G. Electronic Circuit Design and Application; Springer Nature Switzerland AG: Cham, Switzerland, 2021. [Google Scholar]
- Tietze, U.; Schenk, C.; Gamm, E. Electronic Circuits: Handbook for Design and Application; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Schaumann, R.; Ghausi, M.S.; Laker, K.R. Design of Analog Filters, Passive, Active RC, and Switched Capacitor; Prentice Hall: Hoboken, NJ, USA, 1990. [Google Scholar]
- Chiu, W.-Y.; Horng, J.-W. High-input and low-output impedance voltage-mode universal biquadratic filter using DDCCs. IEEE Trans. Circuits Syst. II Express Briefs 2007, 54, 649–652. [Google Scholar] [CrossRef]
- Chen, H.-P.; Liao, Y.-Z. High-input and low-output impedance voltage-mode universal biquadratic filter using FDCCIIs. In Proceedings of the 2008 9th International Conference on Solid-State and Integrated-Circuit Technology, Beijing, China, 20–23 October 2008; pp. 1794–1798. [Google Scholar] [CrossRef]
- Liu, S.I. High input impedance filters with low component spread using current-feedback amplifiers. Electron. Lett. 1995, 31, 1042–1043. [Google Scholar] [CrossRef]
- Abuelma’atti, M.T.; Al-Zaher, H.A. New universal filter with one input and five outputs using current-feedbackamplifiers. Analog. Integr. Circuits Signal Process. 1998, 16, 239–244. [Google Scholar] [CrossRef]
- Wang, S.-F.; Chen, H.-P.; Ku, Y.; Li, Y.-F. High-input impedance voltage-mode multifunction filter. Appl. Sci. 2021, 11, 387. [Google Scholar] [CrossRef]
- Koton, J.; Herencsár, N.; Vrba, K. KHN-equivalent voltage-mode filters using universal voltage conveyors. AEU Int. J. Electron. Commun. 2011, 65, 154–160. [Google Scholar] [CrossRef]
- Sangyaem, S.; Siripongdee, S.; Jaikla, W.; Khateb, F. Five-inputs single-output voltage mode universal filter with high input and low output impedance using VDDDAs. Optik 2017, 128, 14–25. [Google Scholar] [CrossRef]
- Kulej, T. 0.5-V bulk-driven CMOS operational amplifier. IET Circuits Devices Syst. 2013, 7, 352–360. [Google Scholar] [CrossRef]
- Kulej, T.; Khateb, F.; Arbet, D.; Stopjakova, V. A 0.3-V high linear rail-to-rail bulk-driven OTA in 0.13 µm CMOS. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 2046–2050. [Google Scholar] [CrossRef]
- Furth, P.; Andreou, A. Linearised differential transconductors in subthreshold CMOS. Electron. Lett. 1995, 31, 545–547. [Google Scholar] [CrossRef]
- Tsukutani, T.; Higashimura, M.; Takahashi, N.; Sumi, Y.; Fukui, Y. Versatile voltage-mode active-only biquad with lossless and lossy integrator loop. Int. J. Electron. 2001, 88, 1093–1101. [Google Scholar] [CrossRef]
- Khateb, F.; Kulej, T.; Kumngern, M.; Psychalinos, C. Multiple-input bulk-driven MOS transistor for low-voltage low-frequency applications. Circuits Syst. Signal Process. 2018, 38, 2829–2845. [Google Scholar] [CrossRef]
- Khateb, F.; Kulej, T.; Akbari, M.; Tang, K.-T. A 0.5-V multiple-input bulk-driven OTA in 0.18-μm CMOS. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2022, 30, 1739–1747. [Google Scholar] [CrossRef]



| Output | Input | Filtering Function | Transfer Functions |
|---|---|---|---|
| Non-inverting LP | |||
| Non-inverting BP | |||
| Non-inverting BP | |||
| Inverting BP | |||
| Non-inverting HP | |||
| Non-inverting HP | |||
| Inverting HP | |||
| Non-inverting BS | |||
| Non-inverting BS | |||
| Non-Inverting LP | |||
| Inverting BP | |||
| Non-inverting BP | |||
| Inverting BP | |||
| Non-inverting BP | |||
| Non-inverting HP | |||
| Non-inverting HP | |||
| Non-inverting HP | |||
| Non-inverting HP | |||
| Non-inverting BS | |||
| Non-inverting BS | |||
| Non-inverting BS | |||
| Non-inverting BS | |||
| Non-inverting AP | |||
| Non-inverting LP | |||
| Non-inverting LP | |||
| Inverting LP | |||
| Non-inverting BP | |||
| Inverting BP | |||
| Non-inverting HP | |||
| Inverting HP | - | ||
| Non-inverting BS | |||
| Inverting BS | |||
| Non-inverting AP | |||
| Inverting AP |
| Output | Input | Filtering Function | Transfer Functions |
|---|---|---|---|
| Non-inverting LP | |||
| Non-inverting LP | |||
| Inverting LP | |||
| Non-inverting BP | |||
| Inverting BP | |||
| Non-inverting HP | |||
| Inverting HP | |||
| Inverting BS | |||
| Non-inverting BS | |||
| Non-inverting AP | |||
| Inverting AP |
| DDA | W/L (µm/µm) | TA | W/L (µm/µm) |
|---|---|---|---|
| M1A, M2A, M1B, M2B M14, M15 | 16/3 | M1, M2 | 5 × 15/1 |
| M3-M8, M11-M12, MB | 8/3 | M3-M6 | 2 × 10/1 |
| M9, M10 | 4/3 | M3c-M6c | 10/1 |
| M16 | 6 × 16/3 | M8, M9, MB1, M11, M12 | 2 × 15/1 |
| M13 | 6 × 8/3 | M8c, M9c, MB1c | 15/1 |
| ML | 4/5 | M7 | 2 × 30/1 |
| MIM capacitor: CB = 0.5 pF, Cc = 6 pF | M7c | 30/1 | |
| Factor | [11] | [15] | [16] | [18] | Proposed |
|---|---|---|---|---|---|
| Number of active devices | 3 DDCC | 5-DDTA | 3 DDTA | 2 DDTA | 3 DDTA |
| Realization | 130 nm | 180 nm | 130 nm | 130 nm | 180 nm |
| Number of passive devices | 2 R, 2 C | 2 C | 2 C | 2 C | 2 C |
| Type of filter | MISO | MIMO | MIMO | MIMO | MIMO |
| Operation mode | VM | VM/CM/TAM/TIM | VM | VM | VM/TIM |
| Number of offered responses | 5 (VM) | 36 (VM/CM/TAM/TIM) | 23 (VM) | 22 (VM) | 34 (VM) 11 (TIM) |
| Active device offers electronic control | No | Yes | Yes | Yes | Yes |
| High-input and low-output impedance of VM | Yes | No | No | No | Yes |
| High-input and high-output impedance of TM | - | Yes | - | - | Yes |
| Orthogonal control of and | Yes | Yes | Yes | Yes | Yes |
| Electronic control of | No | Yes | Yes | Yes | Yes |
| Offer modified into oscillator | No | No | No | Yes | Yes |
| Orthogonal control of CO and FO | - | - | - | Yes | Yes |
| Natural frequency (kHz) | 6370 | 1.04 | 0.254 | 0.08147 | 0.323 |
| Simulated power supply (V) | 0.75 | 1.2 | 0.5 | 0.3 | 0.5 |
| Power dissipation () | 3650 | 330 | 0.616 | 0.715 | 0.646 |
| THD (%) | 3 @120 m Vpp | 1.09@650 mVpp | 0.62 @100 m Vpp | 0.5 @100 m Vpp | 0.8@100 mVpp |
| Dynamic range (dB) | - | - | 49.7 | - | 53.2 |
| Verification of result | Sim/Exp | Sim/Exp | Sim | Sim | Sim |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kulej, T.; Kumngern, M.; Khateb, F.; Arbet, D. 0.5 V Versatile Voltage- and Transconductance-Mode Analog Filter Using Differential Difference Transconductance Amplifier. Sensors 2023, 23, 688. https://doi.org/10.3390/s23020688
Kulej T, Kumngern M, Khateb F, Arbet D. 0.5 V Versatile Voltage- and Transconductance-Mode Analog Filter Using Differential Difference Transconductance Amplifier. Sensors. 2023; 23(2):688. https://doi.org/10.3390/s23020688
Chicago/Turabian StyleKulej, Tomasz, Montree Kumngern, Fabian Khateb, and Daniel Arbet. 2023. "0.5 V Versatile Voltage- and Transconductance-Mode Analog Filter Using Differential Difference Transconductance Amplifier" Sensors 23, no. 2: 688. https://doi.org/10.3390/s23020688
APA StyleKulej, T., Kumngern, M., Khateb, F., & Arbet, D. (2023). 0.5 V Versatile Voltage- and Transconductance-Mode Analog Filter Using Differential Difference Transconductance Amplifier. Sensors, 23(2), 688. https://doi.org/10.3390/s23020688







