A Bayesian Method for Material Identification of Composite Plates via Dispersion Curves †
Abstract
1. Introduction
2. Lamb Wave Propagation in Plates
2.1. Physics of Lamb Waves
2.2. Dispersion Curve Solutions for Orthotropic Media
2.3. Prior Exploration of Model
3. Material Identification Procedure
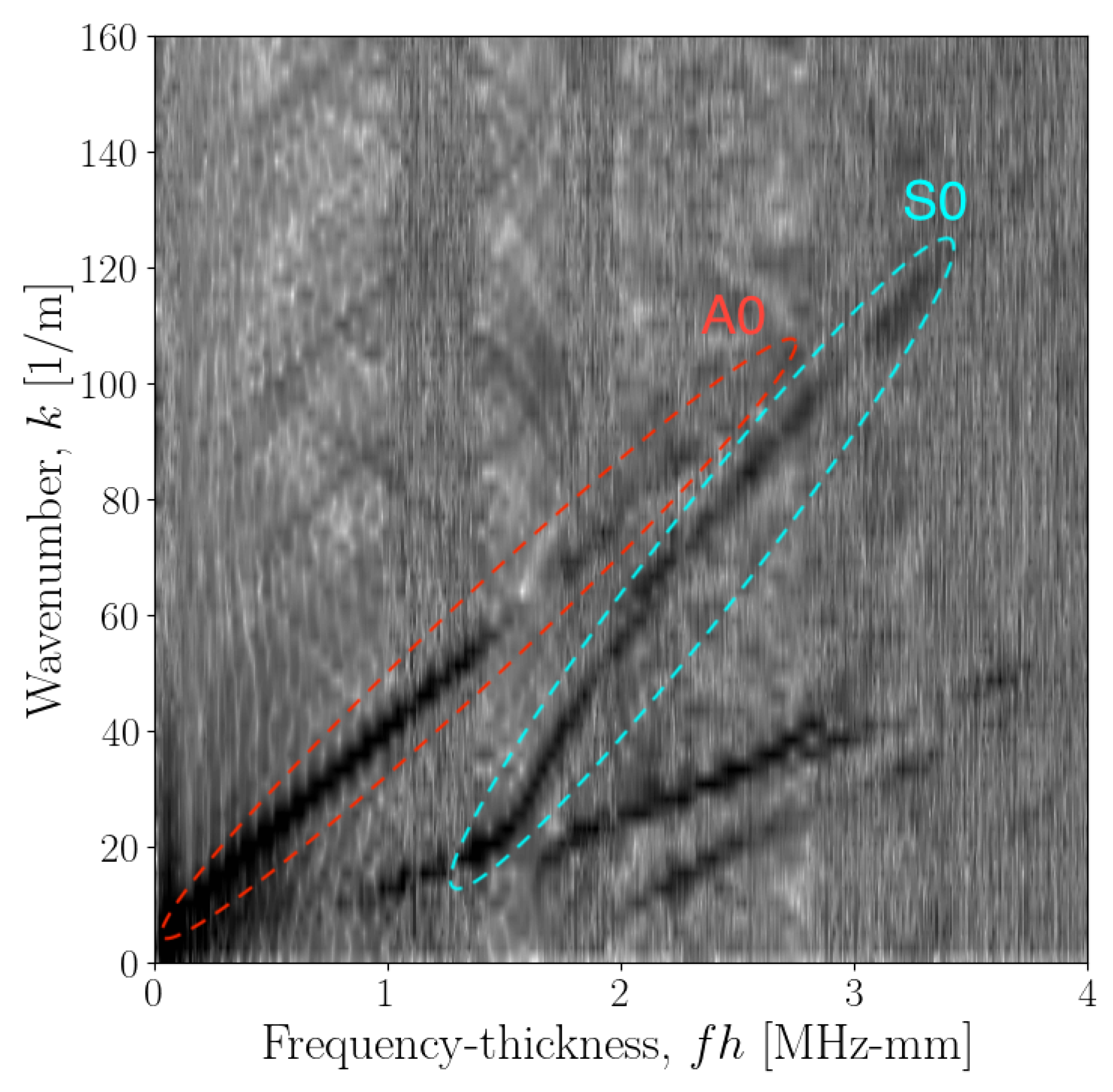
3.1. Measuring Observations of Dispersion Curves
3.2. Estimating Elastic Constants
3.3. Estimating the Posterior Distributions
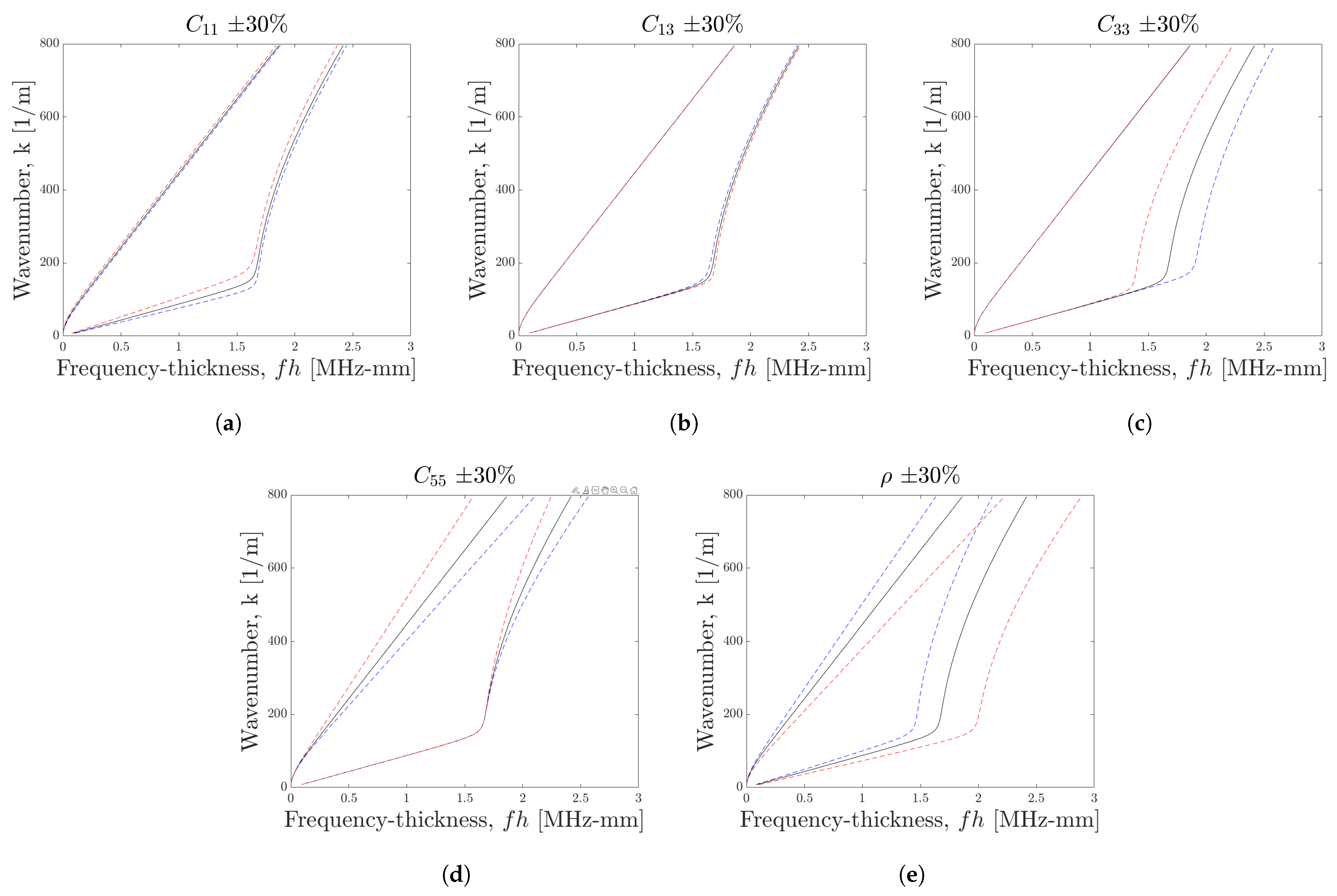
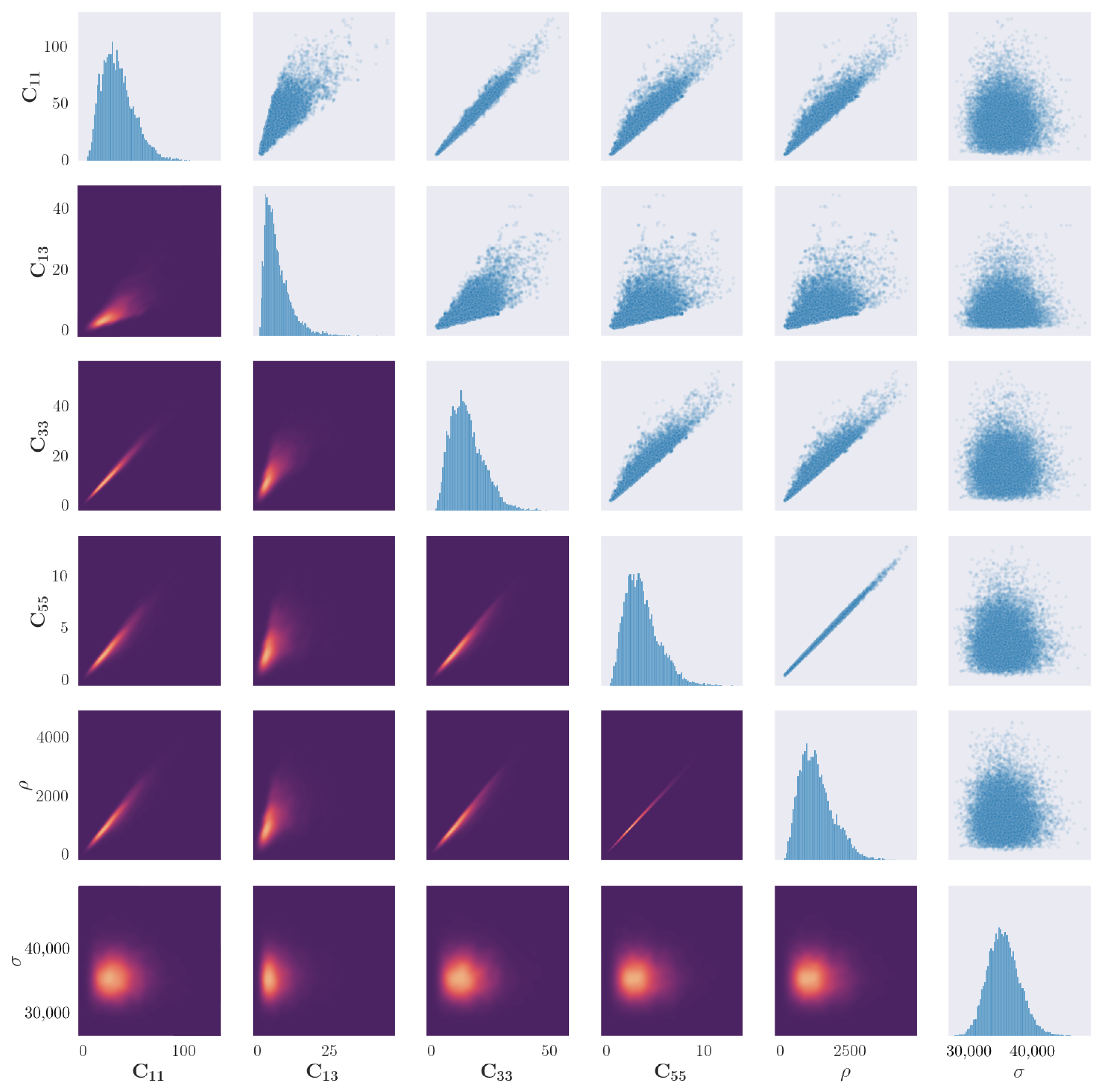
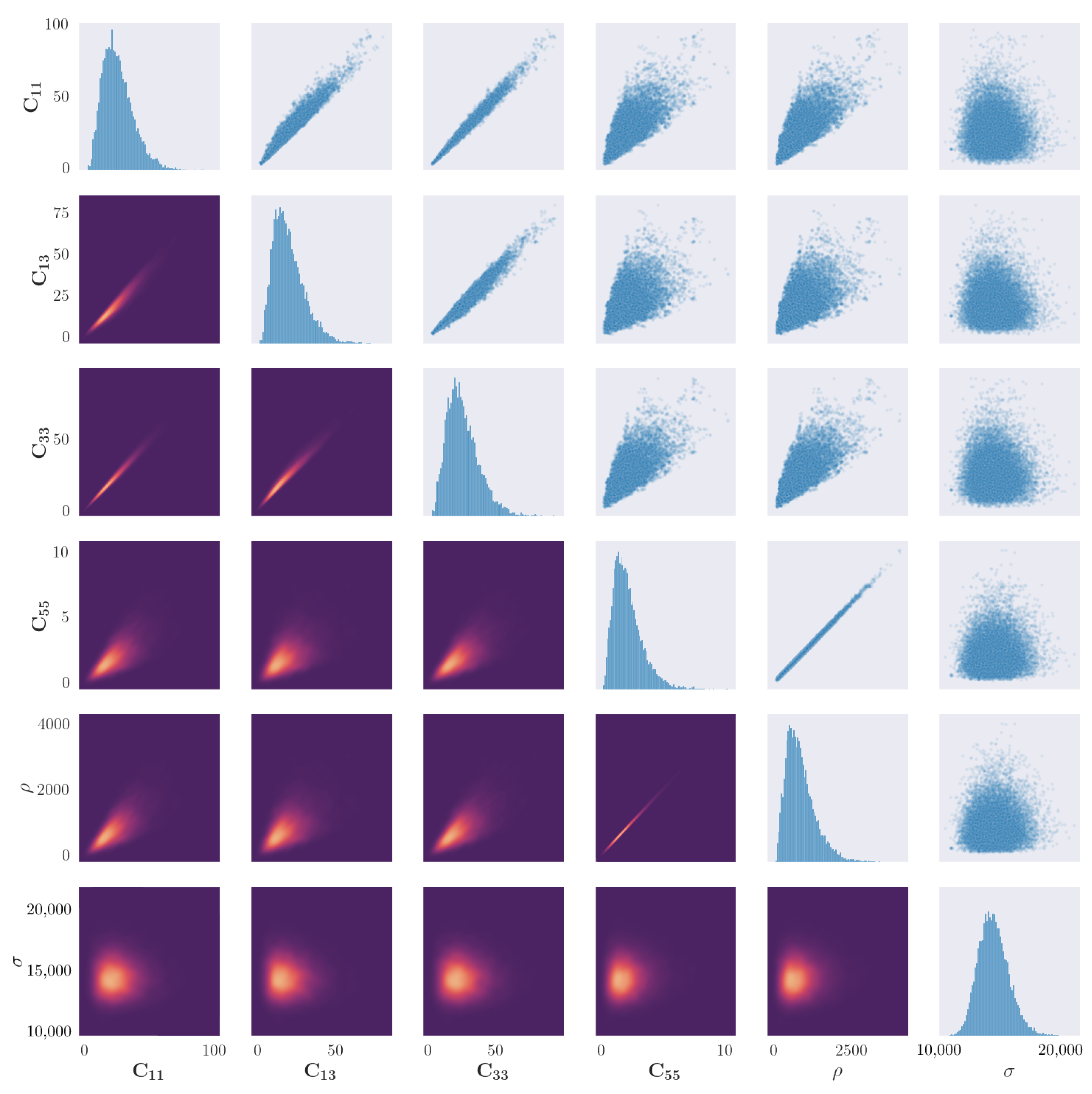
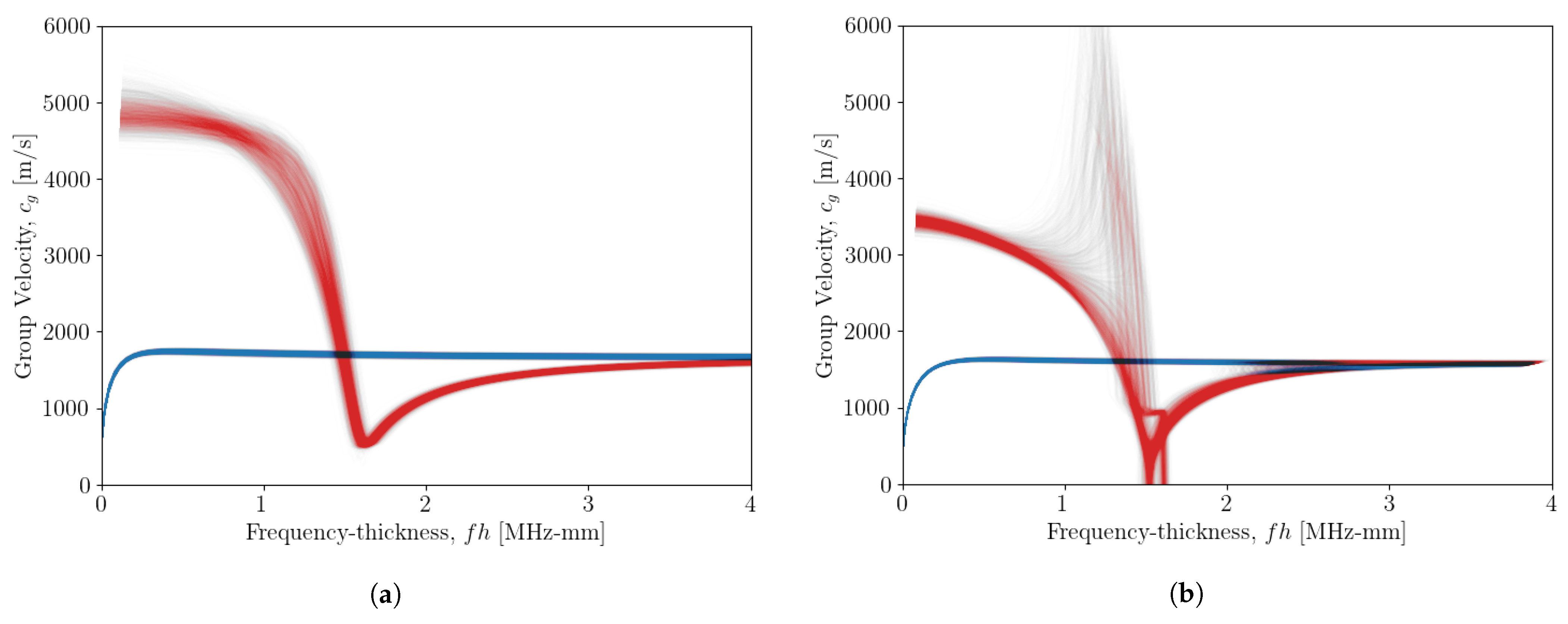
4. Results
4.1. Posterior Distribution of the Material Parameters
4.2. Distribution of Dispersion Curve Models
4.3. Quantifying the Posterior Distributions
4.4. On the Confidence of the Results
5. Conclusions
Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| MCMC | Markov-Chain Monte Carlo |
| HMC | Hamiltonian Monte Carlo |
| NDE | Non-Destructive Evaluation |
| SHM | Structural Health Monitoring |
| UGW | Ultrasonic Guided Wave |
| SH | Shear Horizontal (Wave Mode) |
| GFRP | Glass-Fibre Reinforced Polymer |
| LPE | Legendre Polynomial Expansion |
| 2DFT | Two-Dimensional Fourier Transform |
| PZT | Piezo-electric Transducer |
Appendix A
Appendix B
Appendix B.1
Appendix B.2
Appendix B.3
References
- Rose, J.L. Ultrasonic guided waves in structural health monitoring. Key Eng. Mater. 2004, 270–273, 14–21. [Google Scholar] [CrossRef]
- Viktorov, I.A. Rayleigh and Lamb Waves: Physical Theory and Applications; Plenum Press: New York, NY, USA, 1967. [Google Scholar]
- Worden, K. Rayleigh and Lamb Waves - Basic Principles. Strain 2001, 37, 167–172. [Google Scholar] [CrossRef]
- Rose, J.L. Ultrasonic Waves in Solid Media; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Alleyne, D.N.; Cawley, P. Optimization of Lamb wave inspection techniques. NDT E Int. 1992, 25, 11–22. [Google Scholar] [CrossRef]
- Cawley, P.; Alleyne, D. The use of Lamb waves for the long range inspection of large structures. Ultrasonics 1996, 34, 287–290. [Google Scholar] [CrossRef]
- Guo, N.; Cawley, P. Lamb wave reflection for the quick nondestructive evaluation of large composite laminates. Mater. Eval. 1994, 52, 404–411. [Google Scholar]
- Haywood-Alexander, M.; Dervilis, N.; Worden, K.; Dobie, G.; Rogers, T.J. Informative Bayesian Tools for Damage Localisation by Decomposition of Lamb Wave Signals. J. Sound Vib. 2022, 535, 117063. [Google Scholar] [CrossRef]
- Kundu, T. Acoustic source localization. Ultrasonics 2014, 54, 25–38. [Google Scholar] [CrossRef]
- Solie, L.; Auld, B. Elastic waves in free anisotropic plates. J. Acoust. Soc. Am. 1973, 54, 50–65. [Google Scholar] [CrossRef]
- Kundu, T. Mechanics of Elastic Waves and Ultrasonic Nondestructive Evaluation; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Shorter, P. Wave propagation and damping in linear viscoelastic laminates. J. Acoust. Soc. Am. 2004, 115, 1917–1925. [Google Scholar] [CrossRef]
- Manconi, E.; Sorokin, S. On the effect of damping on dispersion curves in plates. Int. J. Solids Struct. 2013, 50, 1966–1973. [Google Scholar] [CrossRef]
- Fan, Z. Applications of Guided Wave Propagation on Waveguides with Irregular Cross-Section. Ph.D. Thesis, Imperial College London, London, UK, 2010. [Google Scholar]
- Yang, Z.; Wu, Z. Guided Waves Dispersion Analysis in Composite Pipe Using the SAFE Method. In Proceedings of the European Workshop on Structural Health Monitoring; Springer: Berlin/Heidelberg, Germany, 2020; pp. 727–738. [Google Scholar]
- Xiao, D.; Han, Q.; Liu, Y.; Li, C. Guided wave propagation in an infinite functionally graded magneto-electro-elastic plate by the Chebyshev spectral element method. Compos. Struct. 2016, 153, 704–711. [Google Scholar] [CrossRef]
- Moll, J.; Schulte, R.; Hartmann, B.; Fritzen, C.; Nelles, O. Multi-site damage localization in anisotropic plate-like structures using an active guided wave structural health monitoring system. Smart Mater. Struct. 2010, 19, 045022. [Google Scholar] [CrossRef]
- Lefebvre, J.; Zhang, V.; Gazalet, J.; Gryba, T.; Sadaune, V. Acoustic wave propagation in continuous functionally graded plates: An extension of the Legendre polynomial approach. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2001, 48, 1332–1340. [Google Scholar] [CrossRef] [PubMed]
- Eremin, A.A.; Glushkov, E.; Glushkova, N.; Lammering, R. Evaluation of effective elastic properties of layered composite fiber-reinforced plastic plates by piezoelectrically induced guided waves and laser Doppler vibrometry. Compos. Struct. 2015, 125, 449–458. [Google Scholar] [CrossRef]
- Roozen, N.; Leclere, Q.; Ege, K.; Gerges, Y. Estimation of plate material properties by means of a complex wavenumber fit using Hankel’s functions and the image source method. J. Sound Vib. 2017, 390, 257–271. [Google Scholar] [CrossRef]
- Webersen, M.; Johannesmann, S.; Düchting, J.; Claes, L.; Henning, B. Guided ultrasonic waves for determining effective orthotropic material parameters of continuous-fiber reinforced thermoplastic plates. Ultrasonics 2018, 84, 53–62. [Google Scholar] [CrossRef]
- Cui, R.; di Scalea, F.L. On the identification of the elastic properties of composites by ultrasonic guided waves and optimization algorithm. Compos. Struct. 2019, 223, 110969. [Google Scholar] [CrossRef]
- Rautela, M.; Gopalakrishnan, S.; Gopalakrishnan, K.; Deng, Y. Ultrasonic guided waves based identification of elastic properties using 1d-convolutional neural networks. In Proceedings of the 2020 IEEE International Conference on Prognostics and Health Management (ICPHM), Detroit, MI, USA, 8–10 June 2020; pp. 1–7. [Google Scholar]
- Gopalakrishnan, K.; Rautela, M.; Deng, Y. Deep learning based identification of elastic properties using ultrasonic guided waves. In Proceedings of the European Workshop on Structural Health Monitoring; Springer: Berlin/Heidelberg, Germany, 2020; pp. 77–90. [Google Scholar]
- Kudela, P.; Radzienski, M.; Fiborek, P.; Wandowski, T. Elastic constants identification of fibre-reinforced composites by using guided wave dispersion curves and genetic algorithm for improved simulations. Compos. Struct. 2021, 272, 114178. [Google Scholar] [CrossRef]
- Kudela, P.; Radzienski, M.; Fiborek, P.; Wandowski, T. Elastic constants identification of woven fabric reinforced composites by using guided wave dispersion curves and genetic algorithm. Compos. Struct. 2020, 249, 112569. [Google Scholar] [CrossRef]
- Rylander, B.I. Computational Complexity and the Genetic Algorithm; University of Idaho: Moscow, ID, USA, 2001. [Google Scholar]
- Achenbach, J.D. Wave Propagation in Elastic Solids; Elsevier: Amsterdam, The Netherlands, 1973. [Google Scholar]
- Wang, L.; Yuan, F. Group velocity and characteristic wave curves of Lamb waves in composites: Modeling and experiments. Compos. Sci. Technol. 2007, 67, 1370–1384. [Google Scholar] [CrossRef]
- Othmani, C.; Dahmen, S.; Njeh, A.; Ghozlen, M.H.B. Investigation of guided waves propagation in orthotropic viscoelastic carbon–epoxy plate by Legendre polynomial method. Mech. Res. Commun. 2016, 74, 27–33. [Google Scholar] [CrossRef]
- Othmani, C.; Njeh, A.; Ghozlen, M.H.B. Influences of anisotropic fiber-reinforced composite media properties on fundamental guided wave mode behavior: A Legendre polynomial approach. Aerosp. Sci. Technol. 2018, 78, 377–386. [Google Scholar] [CrossRef]
- Mises, R.V.; Pollaczek-Geiringer, H. Praktische Verfahren der Gleichungsauflösung. ZAMM J. Appl. Math. Mech. Z. Angew. Math. Mech. 1929, 9, 152–164. [Google Scholar] [CrossRef]
- Tufts, D.; Melissinos, C. Simple, effective computation of principal eigenvectors and their eigenvalues and application to high-resolution estimation of frequencies. IEEE Trans. Acoust. Speech Signal Process. 1986, 34, 1046–1053. [Google Scholar] [CrossRef]
- Alleyne, D.; Cawley, P. A two-dimensional Fourier transform method for the measurement of propagating multimode signals. J. Acoust. Soc. Am. 1991, 89, 1159–1168. [Google Scholar] [CrossRef]
- Boresi, A.P.; Schmidt, R.J.; Sidebottom, O.M. Advanced Mechanics of Materials; Wiley: New York, NY, USA, 1985; Volume 6. [Google Scholar]
- Dobie, G.; Spencer, A.; Burnham, K.; Pierce, S.G.; Worden, K.; Galbraith, W.; Hayward, G. Simulation of ultrasonic Lamb wave generation, propagation and detection for a reconfigurable air coupled scanner. Ultrasonics 2011, 51, 258–269. [Google Scholar] [CrossRef] [PubMed]
- Le Cam, L. Maximum likelihood: An introduction. In International Statistical Review/Revue Internationale de Statistique; Wiley: New York, NY, USA, 1990; pp. 153–171. [Google Scholar]
- Lehmann, E.L.; Casella, G. Theory of Point Estimation; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Gamerman, D.; Lopes, H.F. Markov Chain Monte Carlo: Stochastic Simulation for Bayesian Inference; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Gilks, W.R.; Richardson, S.; Spiegelhalter, D. Markov Chain Monte Carlo in Practice; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Barber, D. Bayesian Reasoning and Machine Learning; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Neal, R. Handbook of Markov Chain Monte Carlo; chapter MCMC Using Hamiltonian Dynamics; CRC Press: Boca Raton, FL, USA, 2011; pp. 116–162. [Google Scholar]
- Betancourt, M.; Girolami, M. Hamiltonian Monte Carlo for hierarchical models. Curr. Trends Bayesian Methodol. Appl. 2015, 79, 2–4. [Google Scholar]
- Stan Development Team. Stan Modelling Language Users Guide and Reference Manual. Available online: https://mc-stan.org (accessed on 21 November 2021).


| Plate | GFRP |
|---|---|
| Plate dimensions | 800 mm × 700 mm × 16mm |
| PZT Location | 400 mm × 235 mm |
| Actuation signal | 500 kHz chirp |
| Signal record length | 8 ms |
| Sampling frequency | 1.024 MHz |
| Spatial sampling step size | 1.8 mm |
| No. averages | 100 |
| Provided Material Properties | Calculated ECs | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Property | ||||||||||
| Value | 24.5 | 14.6 | 8.2 | −0.46 | −0.28 | 1200 | 28.1 | 7.8 | 16.7 | 8.2 |
| Unit | GPa | GPa | GPa | - | - | GPa | GPa | GPa | GPa | |
| Parameter | Distribution | Definition | |
|---|---|---|---|
| 0 | 90 | |||||
|---|---|---|---|---|---|---|
| 34.69 | 26.49 | 265.9 | 26.19 | 20.29 | 150.6 | |
| 6.917 | 3.912 | 21.67 | 20.12 | 14.82 | 103.3 | |
| 15.24 | 11.62 | 51.39 | 25.87 | 19.94 | 149.1 | |
| 3.649 | 2.771 | 3.052 | 2.141 | 1.458 | 1.426 | |
| 1320.9 | 1003.0 | 396,661 | 874.45 | 594.35 | 232,668 | |
| 35,578 | 35,149 | 14,427 | 14,166 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haywood-Alexander, M.; Dervilis, N.; Worden, K.; Mills, R.S.; Ladpli, P.; Rogers, T.J. A Bayesian Method for Material Identification of Composite Plates via Dispersion Curves. Sensors 2023, 23, 185. https://doi.org/10.3390/s23010185
Haywood-Alexander M, Dervilis N, Worden K, Mills RS, Ladpli P, Rogers TJ. A Bayesian Method for Material Identification of Composite Plates via Dispersion Curves. Sensors. 2023; 23(1):185. https://doi.org/10.3390/s23010185
Chicago/Turabian StyleHaywood-Alexander, Marcus, Nikolaos Dervilis, Keith Worden, Robin S. Mills, Purim Ladpli, and Timothy J. Rogers. 2023. "A Bayesian Method for Material Identification of Composite Plates via Dispersion Curves" Sensors 23, no. 1: 185. https://doi.org/10.3390/s23010185
APA StyleHaywood-Alexander, M., Dervilis, N., Worden, K., Mills, R. S., Ladpli, P., & Rogers, T. J. (2023). A Bayesian Method for Material Identification of Composite Plates via Dispersion Curves. Sensors, 23(1), 185. https://doi.org/10.3390/s23010185








