Wavelength-Tunable Vortex Beam Emitter Based on Silicon Micro-Ring with PN Depletion Diode
Abstract
1. Introduction
2. Principle of Operation
3. Simulation Results
4. Analysis of the Emitted Field Propagation
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185. [Google Scholar] [CrossRef] [PubMed]
- He, H.; Friese, M.E.J.; Heckenberg, N.R.; Rubinsztein-Dunlop, H. Direct Observation of Transfer of Angular Momentum to Absorptive Particles from a Laser Beam with a Phase Singularity. Phys. Rev. Lett. 1995, 75, 826–829. [Google Scholar] [CrossRef]
- Grier, D.G. A revolution in optical manipulation. Nature 2003, 424, 810–816. [Google Scholar] [CrossRef] [PubMed]
- Chapin, S.C.; Germain, V.; Dufresne, E.R. Automated trapping, assembly, and sorting with holographic optical tweezers. Opt. Express 2006, 14, 13095–13100. [Google Scholar] [CrossRef]
- Padgett, M.; Bowman, R. Tweezers with a twist. Nat. Photonics 2011, 5, 343–348. [Google Scholar] [CrossRef]
- Vicente, O.C.; Caloz, C. Bessel beams: A unified and extended perspective. Optica 2021, 8, 451–457. [Google Scholar] [CrossRef]
- Forbes, A.; Nape, I. Quantum mechanics with patterns of light: Progress in high dimensional and multidimensional entanglement with structured light. AVS Quantum Sci. 2019, 1, 011701. [Google Scholar] [CrossRef]
- Ndagano, B.; Nape, I.; Cox, M.A.; Rosales-Guzman, C.; Forbes, A. Creation and Detection of Vector Vortex Modes for Classical and Quantum Communication. J. Light. Technol. 2018, 36, 292–301. [Google Scholar] [CrossRef]
- Mafu, M.; Dudley, A.; Goyal, S.; Giovannini, D.; McLaren, M.; Padgett, M.J.; Konrad, T.; Petruccione, F.; Lütkenhaus, N.; Forbes, A. Higher-dimensional orbital-angular-momentum-based quantum key distribution with mutually unbiased bases. Phys. Rev. A 2013, 88, 032305. [Google Scholar] [CrossRef]
- Zhang, Y.; Agnew, M.; Roger, T.; Roux, F.S.; Konrad, T.; Faccio, D.; Leach, J.; Forbes, A. Simultaneous entanglement swapping of multiple orbital angular momentum states of light. Nat. Commun. 2017, 8, 632. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Nape, I.; Wang, Q.; Vallés, A.; Wang, J.; Forbes, A. Multidimensional entanglement transport through single-mode fiber. Sci. Adv. 2020, 6, eaay0837. [Google Scholar] [CrossRef] [PubMed]
- Richardson, D.J.; Fini, J.M.; Nelson, L.E. Space-division multiplexing in optical fibres. Nat. Photonics 2013, 7, 354–362. [Google Scholar] [CrossRef]
- Ellis, A.D.; Suibhne, N.M.; Saad, D.; Payne, D.N. Communication networks beyond the capacity crunch. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2016, 374, 20150191. [Google Scholar] [CrossRef]
- Turitsyn, K.S.; Turitsyn, S.K. Nonlinear communication channels with capacity above the linear Shannon limit. Opt. Lett. 2012, 37, 3600–3602. [Google Scholar] [CrossRef]
- Sakaguchi, J.; Awaji, Y.; Wada, N.; Kanno, A.; Kawanishi, T.; Hayashi, T.; Taru, T.; Kobayashi, T.; Watanabe, M. Space Division Multiplexed Transmission of 109-Tb/s Data Signals Using Homogeneous Seven-Core Fiber. J. Light. Technol. 2012, 30, 658–665. [Google Scholar] [CrossRef]
- Nakajima, K.; Sillard, P.; Richardson, D.; Li, M.J.; Essiambre, R.J.; Matsuo, S. Transmission media for an SDM-based optical communication system. IEEE Commun. Mag. 2015, 53, 44–51. [Google Scholar] [CrossRef]
- Mizuno, T.; Takara, H.; Shibahara, K.; Sano, A.; Miyamoto, Y. Dense space division multiplexed transmission over multicore and multimode fiber for long-haul transport systems. J. Light. Technol. 2016, 34, 1484–1493. [Google Scholar] [CrossRef]
- Wang, J.; Padgett, M.J.; Ramachandran, S.; Lavery, M.P.; Huang, H.; Yue, Y.; Yan, Y.; Bozinovic, N.; Golowich, S.E.; Willner, A.E. Multimode Communications Using Orbital Angular Momentum. In Optical Fiber Telecommunications; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar] [CrossRef]
- Berdagué, S.; Facq, P. Mode division multiplexing in optical fibers. Appl. Opt. 1982, 21, 1950–1955. [Google Scholar] [CrossRef] [PubMed]
- Bozinovic, N.; Yue, Y.; Ren, Y.; Tur, M.; Kristensen, P.; Huang, H.; Willner, A.E.; Ramachandran, S. Terabit-scale orbital angular momentum mode division multiplexing in fibers. Science 2013, 340, 1545–1548. [Google Scholar] [CrossRef]
- Qu, Z.; Djordjevic, I.B. 500 Gb/s free-space optical transmission over strong atmospheric turbulence channels. Opt. Lett. 2016, 41, 3285. [Google Scholar] [CrossRef]
- Willner, A.E.; Zhao, Z.; Liu, C.; Zhang, R.; Song, H.; Pang, K.; Manukyan, K.; Song, H.; Su, X.; Xie, G.; et al. Perspectives on advances in high-capacity, free-space communications using multiplexing of orbital-angular-momentum beams. APL Photonics 2021, 6, 030901. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhang, R.; Song, H.; Pang, K.; Almaiman, A.; Zhou, H.; Song, H.; Liu, C.; Hu, N.; Su, X.; et al. Modal coupling and crosstalk due to turbulence and divergence on free space THz links using multiple orbital angular momentum beams. Sci. Rep. 2021, 11, 2110. [Google Scholar] [CrossRef] [PubMed]
- Khonina, S.; Kazanskiy, N.; Soifer, V. Optical Vortices in a Fiber: Mode Division Multiplexing and Multimode Self-Imaging. In Recent Progress in Optical Fiber Research; InTech: London, UK, 2012. [Google Scholar] [CrossRef][Green Version]
- Wang, J.; Yang, J.Y.; Fazal, I.M.; Ahmed, N.; Yan, Y.; Huang, H.; Ren, Y.; Yue, Y.; Dolinar, S.; Tur, M.; et al. Terabit free-space data transmission employing orbital angular momentum multiplexing. Nat. Photonics 2012, 6, 488–496. [Google Scholar] [CrossRef]
- Zhao, Y.; Askarpour, A.N.; Sun, L.; Shi, J.; Li, X.; Alù, A. Chirality detection of enantiomers using twisted optical metamaterials. Nat. Commun. 2017, 8, 1–8. [Google Scholar] [CrossRef]
- Tamburini, F.; Anzolin, G.; Umbriaco, G.; Bianchini, A.; Barbieri, C. Overcoming the Rayleigh criterion limit with optical vortices. Phys. Rev. Lett. 2006, 97, 163903. [Google Scholar] [CrossRef] [PubMed]
- Kozawa, Y.; Matsunaga, D.; Sato, S. Superresolution imaging via superoscillation focusing of a radially polarized beam. Optica 2018, 5, 86–92. [Google Scholar] [CrossRef]
- Fu, H.; Wang, S.; Chang, H.; You, Y. A high resolution and large range fiber Bragg grating temperature sensor with vortex beams. Opt. Fiber Technol. 2020, 60, 102369. [Google Scholar] [CrossRef]
- Yue, Z.; Ren, H.; Wei, S.; Lin, J.; Gu, M. Angular-momentum nanometrology in an ultrathin plasmonic topological insulator film. Nat. Commun. 2018, 9, 1–7. [Google Scholar] [CrossRef]
- Fang, J.; Zhou, C.; Mou, Z.; Wang, S.; Yu, J.; Yang, Y.; Gbur, G.J.; Teng, S.; Cai, Y. High order plasmonic vortex generation based on spiral nanoslits. New J. Phys. 2021, 23, 033013. [Google Scholar] [CrossRef]
- Liu, E.; Yan, B.; Zhou, H.; Liu, Y.; Liu, G.; Liu, J. OAM mode-excited surface plasmon resonance for refractive index sensing based on a photonic quasi-crystal fiber. J. Opt. Soc. Am. B 2021, 38, F16–F22. [Google Scholar] [CrossRef]
- Vahala, K.J. Optical microcavities. Nature 2003, 424, 839–846. [Google Scholar] [CrossRef]
- Cai, X.; Wang, J.; Strain, M.J.; Johnson-Morris, B.; Zhu, J.; Sorel, M.; O’Brien, J.L.; Thompson, M.G.; Yu, S. Integrated Compact Optical Vortex Beam Emitters. Science 2012, 338, 363–366. [Google Scholar] [CrossRef]
- Strain, M.J.; Cai, X.; Wang, J.; Zhu, J.; Phillips, D.B.; Chen, L.; Lopez-Garcia, M.; O’Brien, J.L.; Thompson, M.G.; Sorel, M.; et al. Fast electrical switching of orbital angular momentum modes using ultra-compact integrated vortex emitters. Nat. Commun. 2014, 5, 4856. [Google Scholar] [CrossRef]
- Kim, Y.; Han, J.H.; Ahn, D.; Kim, S. Heterogeneously-Integrated Optical Phase Shifters for Next-Generation Modulators and Switches on a Silicon Photonics Platform: A Review. Micromachines 2021, 12, 625. [Google Scholar] [CrossRef] [PubMed]
- Li, R.; Feng, X.; Zhang, D.; Cui, K.; Liu, F.; Huang, Y. Radially Polarized Orbital Angular Momentum Beam Emitter Based on Shallow-Ridge Silicon Microring Cavity. IEEE Photonics J. 2014, 6, 1–10. [Google Scholar] [CrossRef]
- Soref, R. Tutorial: Integrated-photonic switching structures. APL Photonics 2018, 3, 021101. [Google Scholar] [CrossRef]
- Soref, R.; Bennett, B. Electrooptical effects in silicon. IEEE J. Quantum Electron. 1987, 23, 123–129. [Google Scholar] [CrossRef]
- Nedeljkovic, M.; Soref, R.; Mashanovich, G.Z. Free-Carrier Electrorefraction and Electroabsorption Modulation Predictions for Silicon Over the 1–14-µm Infrared Wavelength Range. IEEE Photonics J. 2011, 3, 1171–1180. [Google Scholar] [CrossRef]
- Bogaerts, W.; De Heyn, P.; Van Vaerenbergh, T.; De Vos, K.; Kumar Selvaraja, S.; Claes, T.; Dumon, P.; Bienstman, P.; Van Thourhout, D.; Baets, R. Silicon microring resonators. Laser Photonics Rev. 2012, 6, 47–73. [Google Scholar] [CrossRef]
- Hao, B.; Leger, J. Experimental measurement of longitudinal component in the vicinity of focused radially polarized beam. Opt. Express 2007, 15, 3550. [Google Scholar] [CrossRef]
- Lerman, G.M.; Levy, U. Effect of radial polarization and apodization on spot size under tight focusing conditions. Opt. Express 2008, 16, 4567. [Google Scholar] [CrossRef] [PubMed]
- Khonina, S.N.; Degtyarev, S.A. Analysis of the formation of a longitudinally polarized optical needle by a lens and axicon under tightly focused conditions. J. Opt. Technol. 2016, 83, 197. [Google Scholar] [CrossRef]
- Helseth, L. Optical vortices in focal regions. Opt. Commun. 2004, 229, 85–91. [Google Scholar] [CrossRef]
- Rashid, M.; Maragò, O.M.; Jones, P.H. Focusing of high order cylindrical vector beams. J. Opt. A Pure Appl. Opt. 2009, 11, 065204. [Google Scholar] [CrossRef]
- Khonina, S.N. Vortex beams with high-order cylindrical polarization: Features of focal distributions. Appl. Phys. B 2019, 125, 100. [Google Scholar] [CrossRef]
- Almazov, A.A.; Khonina, S.N.; Kotlyar, V.V. Using phase diffraction optical elements to shape and select laser beams consisting of a superposition of an arbitrary number of angular harmonics. J. Opt. Technol. 2005, 72, 391. [Google Scholar] [CrossRef]
- Khonina, S.N.; Podlipnov, V.V.; Karpeev, S.V.; Ustinov, A.V.; Volotovsky, S.G.; Ganchevskaya, S.V. Spectral control of the orbital angular momentum of a laser beam based on 3D properties of spiral phase plates fabricated for an infrared wavelength. Opt. Express 2020, 28, 18407. [Google Scholar] [CrossRef] [PubMed]
- Lavery, M.P.J.; Speirits, F.C.; Barnett, S.M.; Padgett, M.J. Detection of a Spinning Object Using Light’s Orbital Angular Momentum. Science 2013, 341, 537–540. [Google Scholar] [CrossRef]
- Cvijetic, N.; Milione, G.; Ip, E.; Wang, T. Detecting Lateral Motion using Light’s Orbital Angular Momentum. Sci. Rep. 2015, 5, 15422. [Google Scholar] [CrossRef] [PubMed]
- Ren, H.; Wang, X.; Li, C.; He, C.; Wang, Y.; Pan, A.; Maier, S.A. Orbital-Angular-Momentum-Controlled Hybrid Nanowire Circuit. Nano Lett. 2021, 21, 6220–6227. [Google Scholar] [CrossRef]
- Li, S.; Ding, Y.; Guan, X.; Tan, H.; Nong, Z.; Wang, L.; Liu, L.; Zhou, L.; Yang, C.; Yvind, K.; et al. Compact high-efficiency vortex beam emitter based on a silicon photonics micro-ring. Opt. Lett. 2018, 43, 1319. [Google Scholar] [CrossRef] [PubMed]
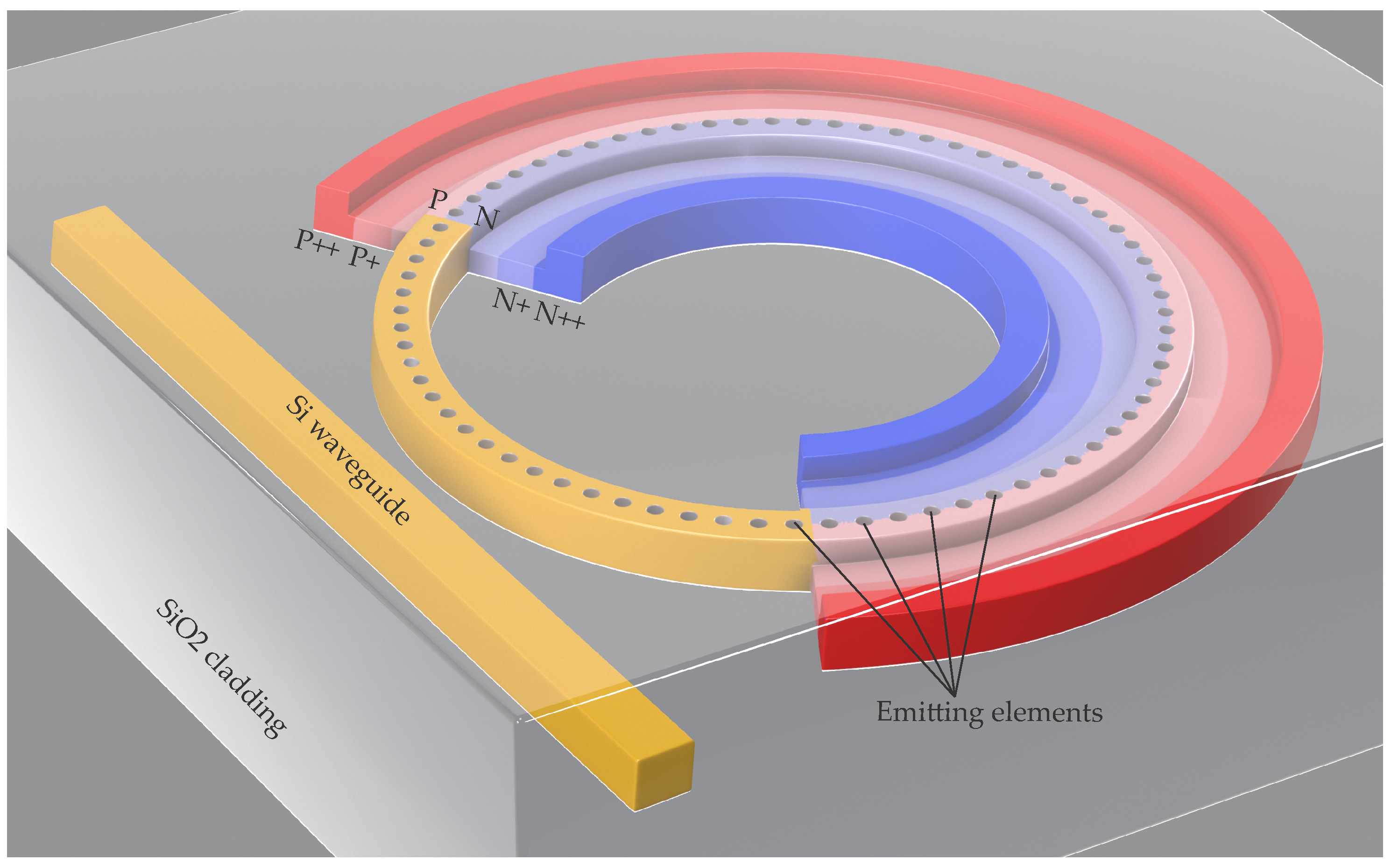

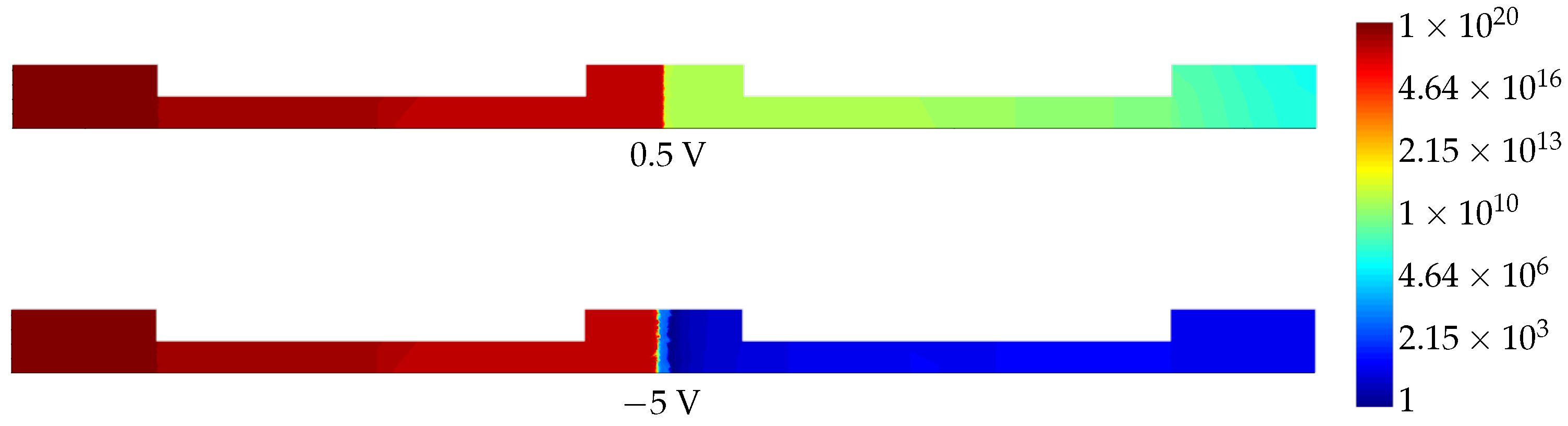

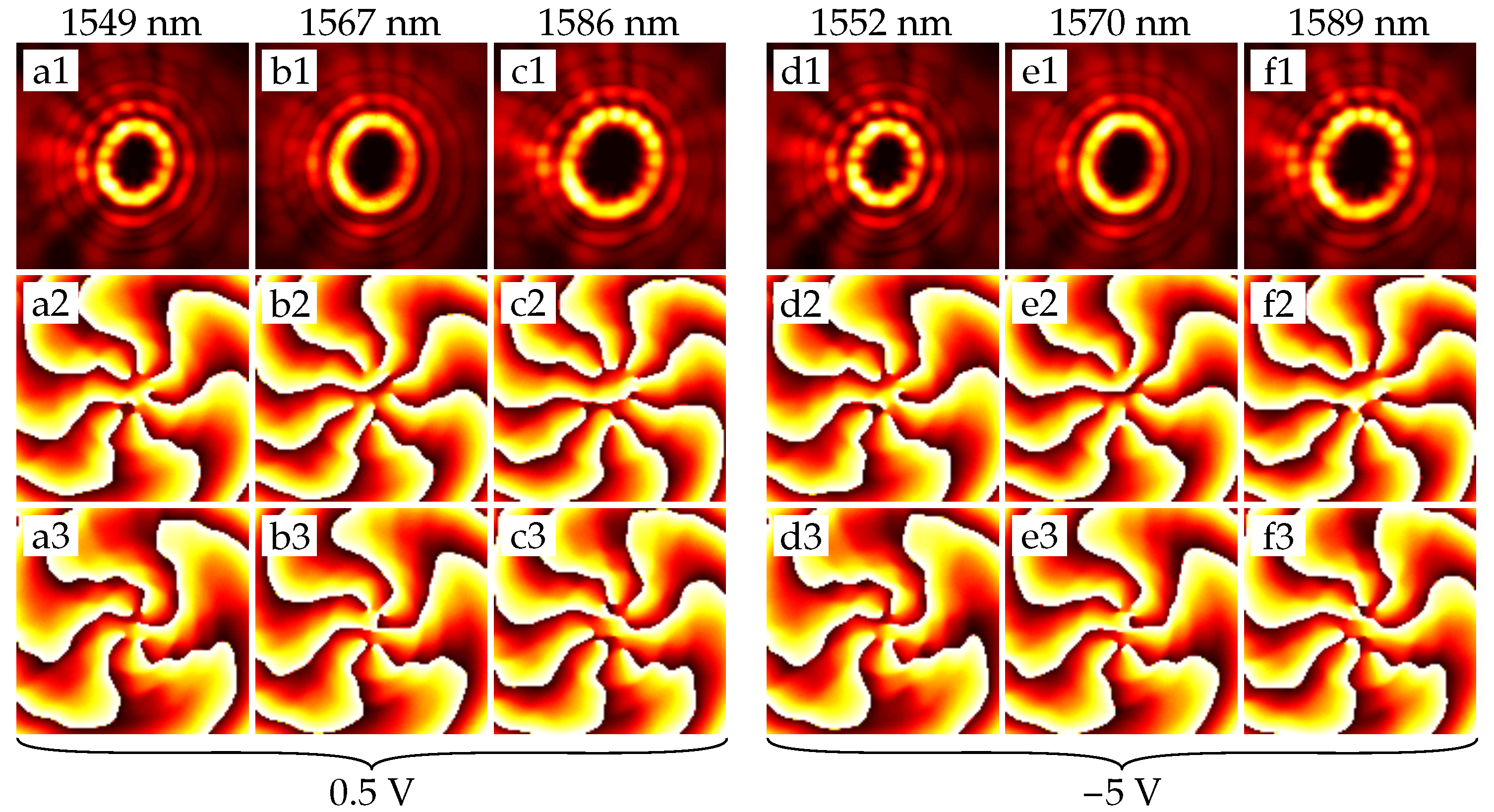

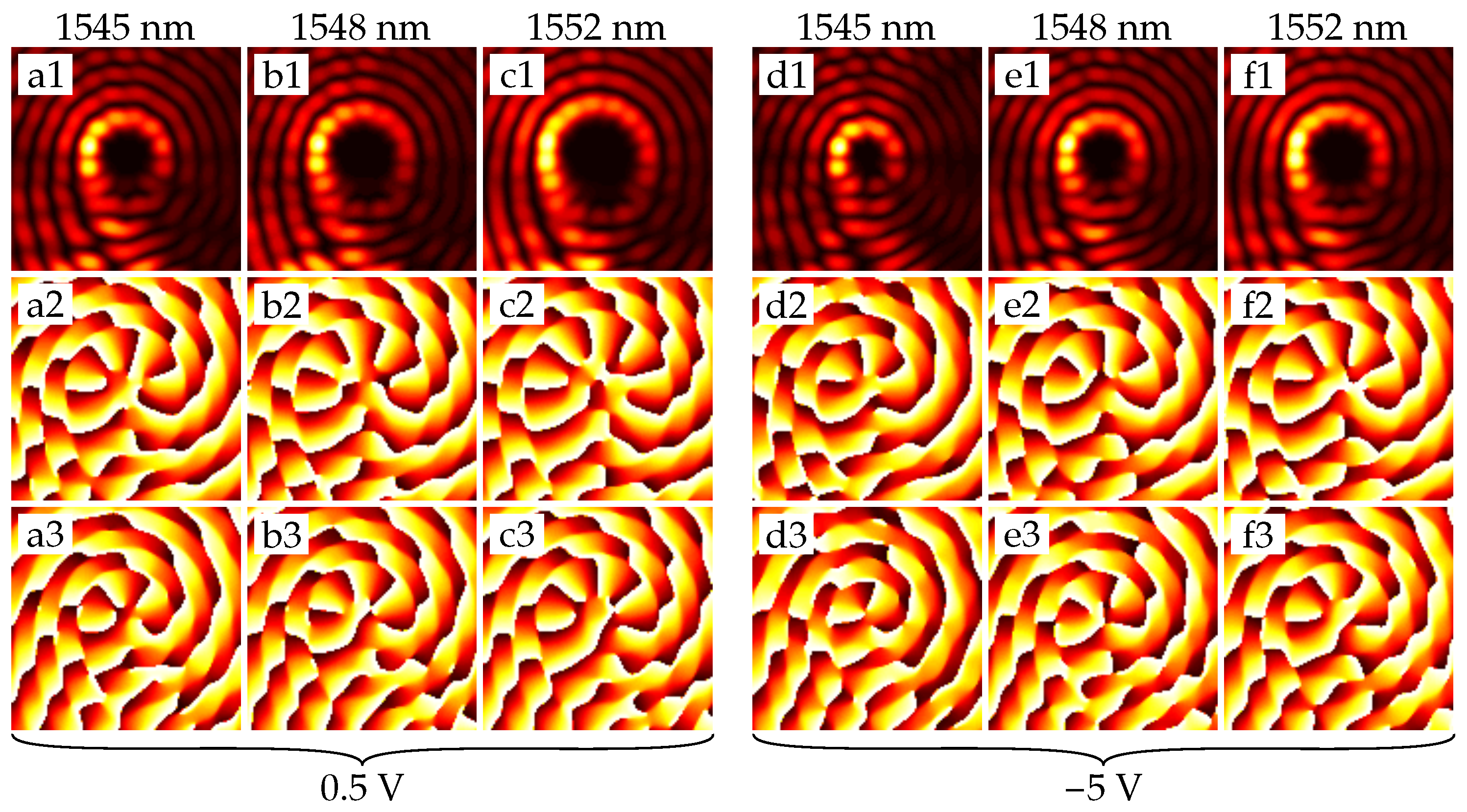


| Dimension | w1 | w2 | h1 | h2 | h3 | d |
|---|---|---|---|---|---|---|
| Value | 0.545 | 0.5 | 0.22 | 0.11 | 0.07 | 0.15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stepanov, I.V.; Fatkhiev, D.M.; Lyubopytov, V.S.; Kutluyarov, R.V.; Grakhova, E.P.; Neumann, N.; Khonina, S.N.; Sultanov, A.K. Wavelength-Tunable Vortex Beam Emitter Based on Silicon Micro-Ring with PN Depletion Diode. Sensors 2022, 22, 929. https://doi.org/10.3390/s22030929
Stepanov IV, Fatkhiev DM, Lyubopytov VS, Kutluyarov RV, Grakhova EP, Neumann N, Khonina SN, Sultanov AK. Wavelength-Tunable Vortex Beam Emitter Based on Silicon Micro-Ring with PN Depletion Diode. Sensors. 2022; 22(3):929. https://doi.org/10.3390/s22030929
Chicago/Turabian StyleStepanov, Ivan V., Denis M. Fatkhiev, Vladimir S. Lyubopytov, Ruslan V. Kutluyarov, Elizaveta P. Grakhova, Niels Neumann, Svetlana N. Khonina, and Albert K. Sultanov. 2022. "Wavelength-Tunable Vortex Beam Emitter Based on Silicon Micro-Ring with PN Depletion Diode" Sensors 22, no. 3: 929. https://doi.org/10.3390/s22030929
APA StyleStepanov, I. V., Fatkhiev, D. M., Lyubopytov, V. S., Kutluyarov, R. V., Grakhova, E. P., Neumann, N., Khonina, S. N., & Sultanov, A. K. (2022). Wavelength-Tunable Vortex Beam Emitter Based on Silicon Micro-Ring with PN Depletion Diode. Sensors, 22(3), 929. https://doi.org/10.3390/s22030929







