Multispectral High Temperature Thermography
Abstract
1. Introduction
- the ability to measure the true temperature T and estimate the effective emissivity εeff;
- minimization of the uncertainty of the temperature measurement results achieved through the optimal choice of spectral sections for registration of thermal radiation and the elimination of the influence of the deviation of the calibration curves from the calculated dependences;
- determination of the maximum body temperature Tmax and its dependence on time, as well as the possibility of video recording of the temperature field and its subsequent frame-by-frame viewing, which is necessary when monitoring complex heat engineering processes;
- invariance of the results of determining the maximum temperature Tmax to changes in the size of the image of the monitored bodies;
- invariance of the measured values of Tmax to nonstationarity of the noise dispersion of the used photodetector matrix, i.e., the dependence of its noise on the value of the incident thermal radiation flux.
2. Design and Quality Indicators of Thermographic Equipment
- accuracy parameters the root-mean-square value of the error with which the temperature can be measured;
- instantaneous field of view;
- frame formation time or frequency of their repetition;
- resolution.
3. Formation of a Digital Image of the Temperature Field
4. Determining Temperature When Using Multiple Spectral Regions
4.1. Determination of T Using Three Narrow Spectral Regions
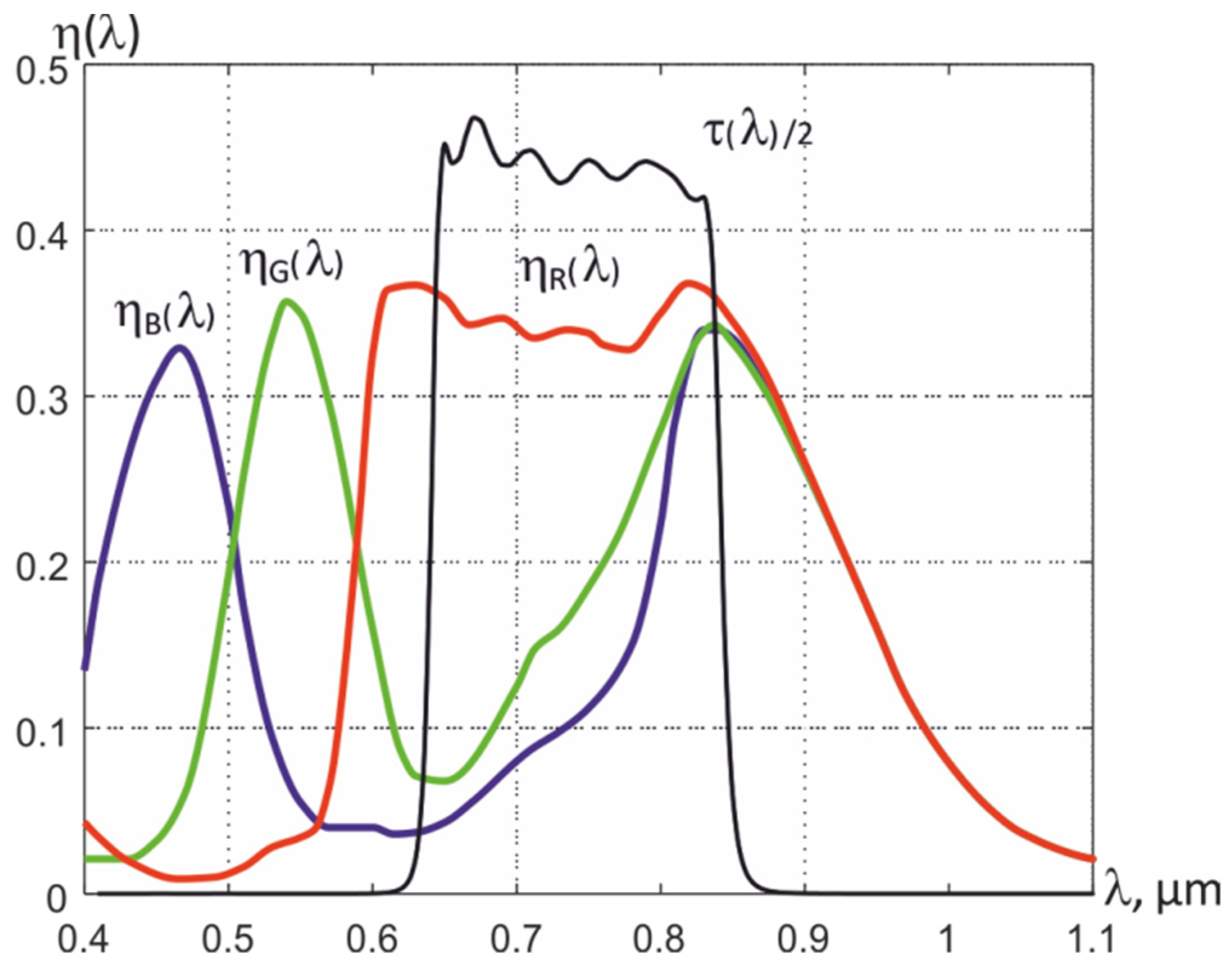
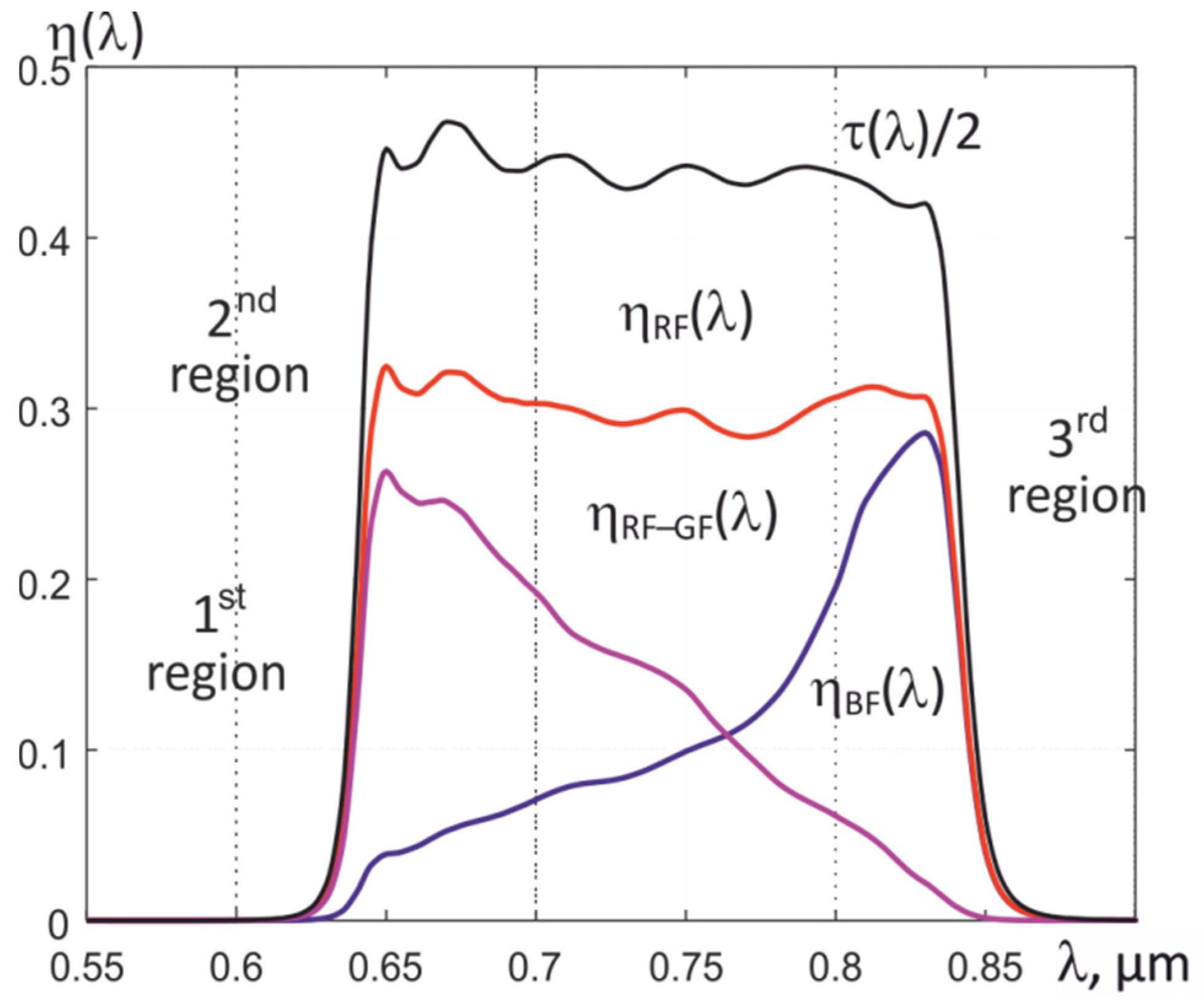
4.2. Formation of Three Spectrum Regions, Shifted in the NIR Range
4.3. Temperature Determination Method
5. Tehnique of Averaging Signals over the Area of Maximum Heating
5.1. Ensuring the Invariance of the Measured Values of the Maximum Temperature Tmax to the Image Size and the Nonstationarity of the Matrix Noise
5.2. Method for Obtaining Averaged Values on the High-Temperature Slope of Histograms
- (1)
- After receiving the frame, histograms are calculated for all three of its layers with the width of pockets or bins equal to one or one level of the ADC conversion range.
- (2)
- Carry out operations to clean the high-temperature sections of the histograms from single noise emissions, assessing the probability of their occurrence, taking into account the current values of the standard deviation equal to . This ensures the correct position on the histogram of the averaging intervals with width , where Vkmax are the rightmost points of the histograms after clearing.
- (3)
- Determine the left boundaries of the areas of integration of the histograms .
- (4)
- Calculate the dependences of two sums: the dependence of the area of the histogram section and the sum on the current number n of the histogram bins. Moreover, they are summed from right to left, which starts from the maximum values of n. These dependences correspond to the integrals and , where W(x) is the normal distribution.
- (5)
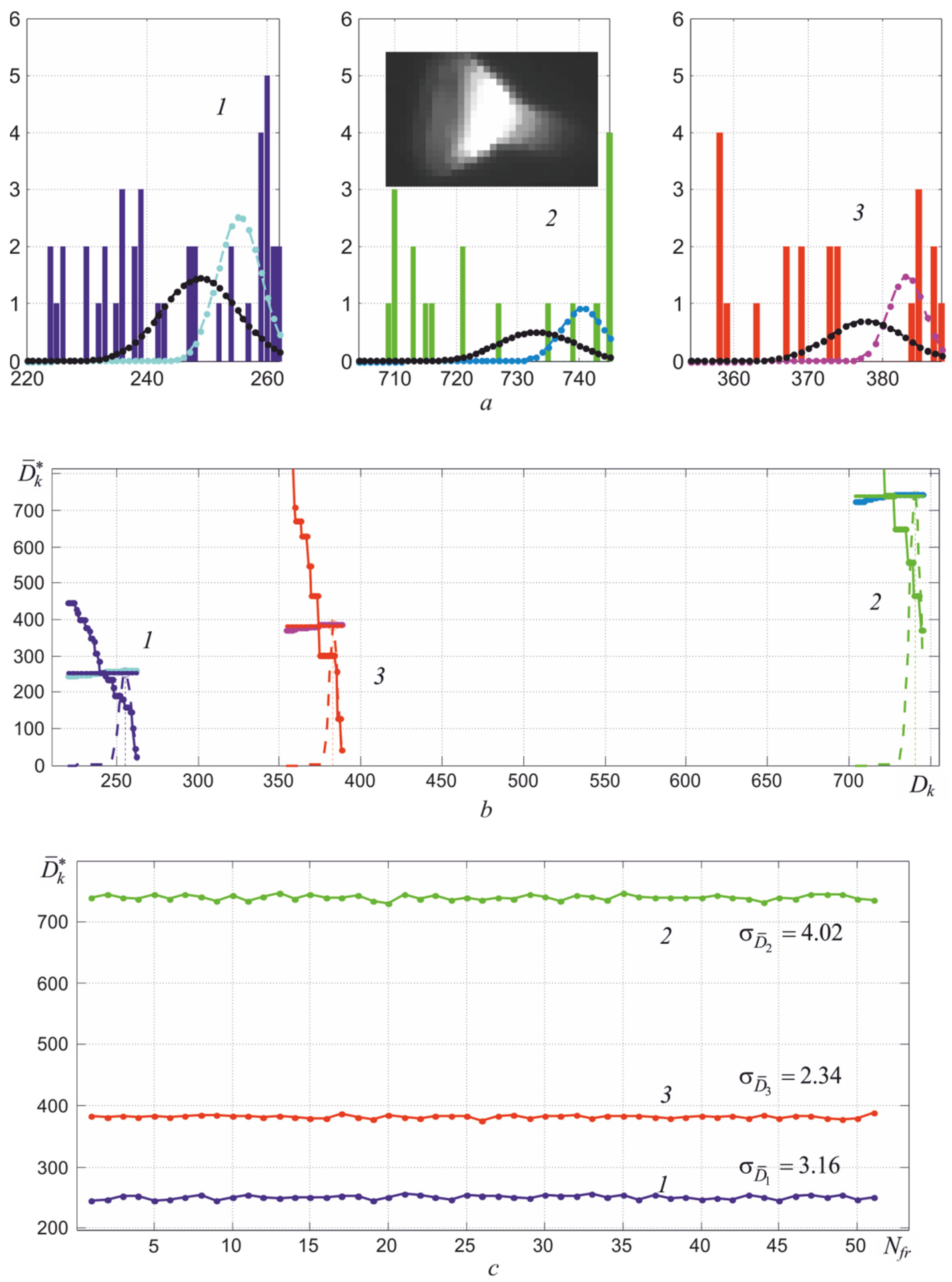
- Normalize dependencies by dividing them by , which corresponds to the relationship . As seen in Figure 16, the values of the elements of the series gradually decrease to the desired average value when the point x moves from the maximum value D to the left, since the value of the integral is in the denominator of the ratio . When moving to the left, the ratio has a small steepness, determined by the value of σ, which makes it possible to obtain a slightly biased estimate with a small number of pixels involved in averaging.
- (6)
- Find an estimate of the mean values , where is determined by enumerating the values of the dependencies and starting from the maximum value of n to their intersection. The value is taken as the estimate , where nl = xthl.
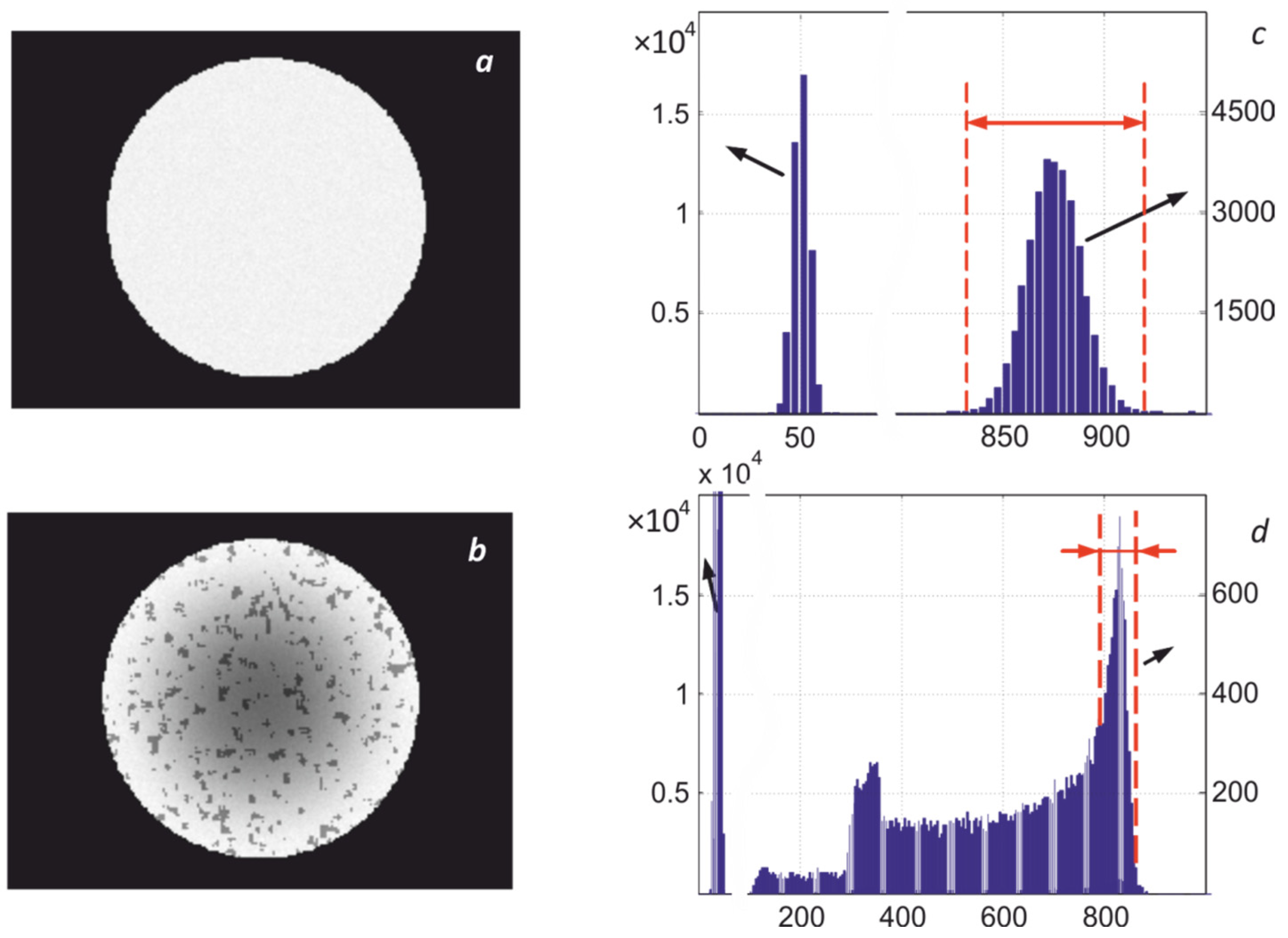
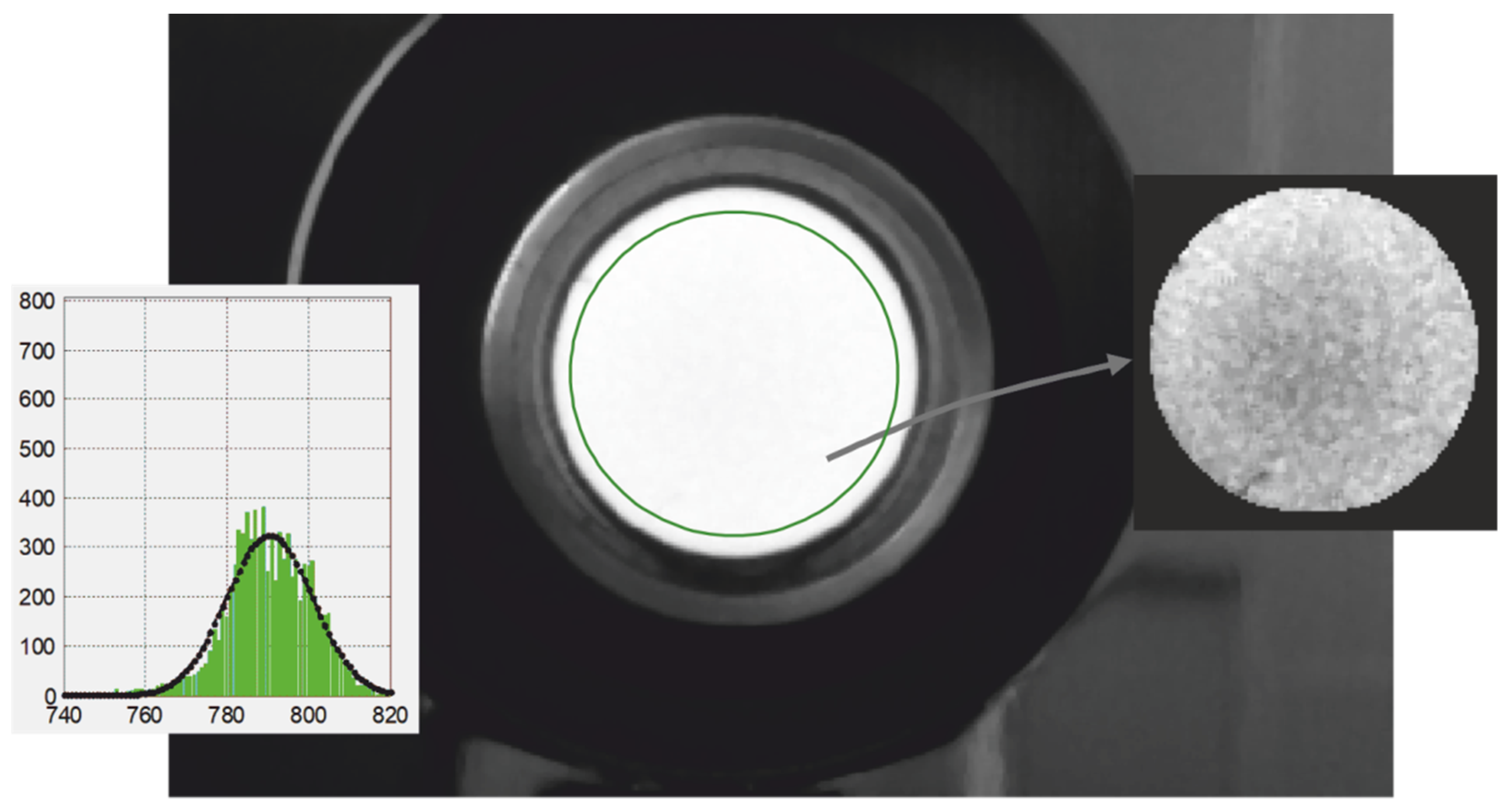
5.3. Example Illustrating Obtaining for Small Objects with Uneven Heating
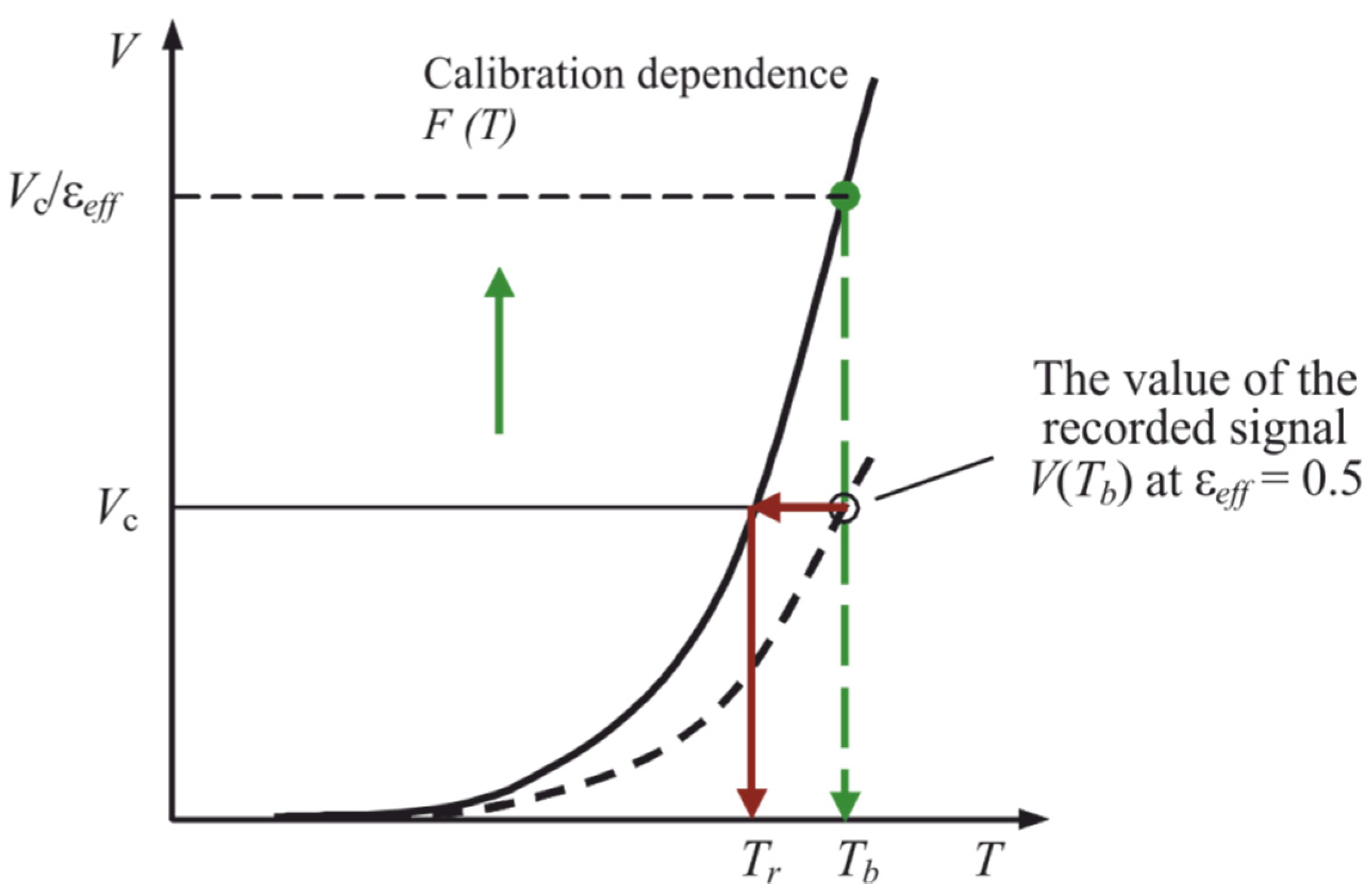
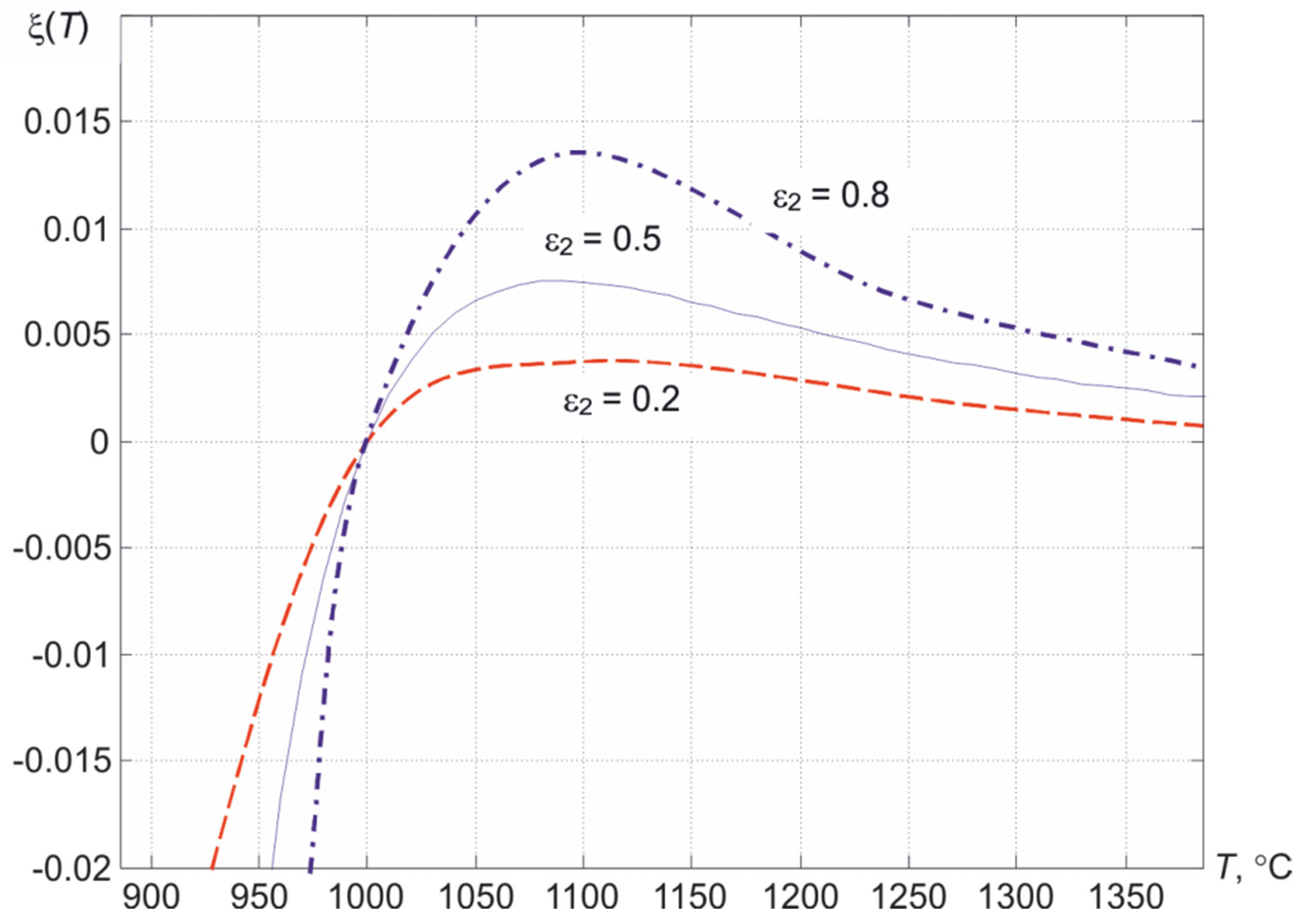
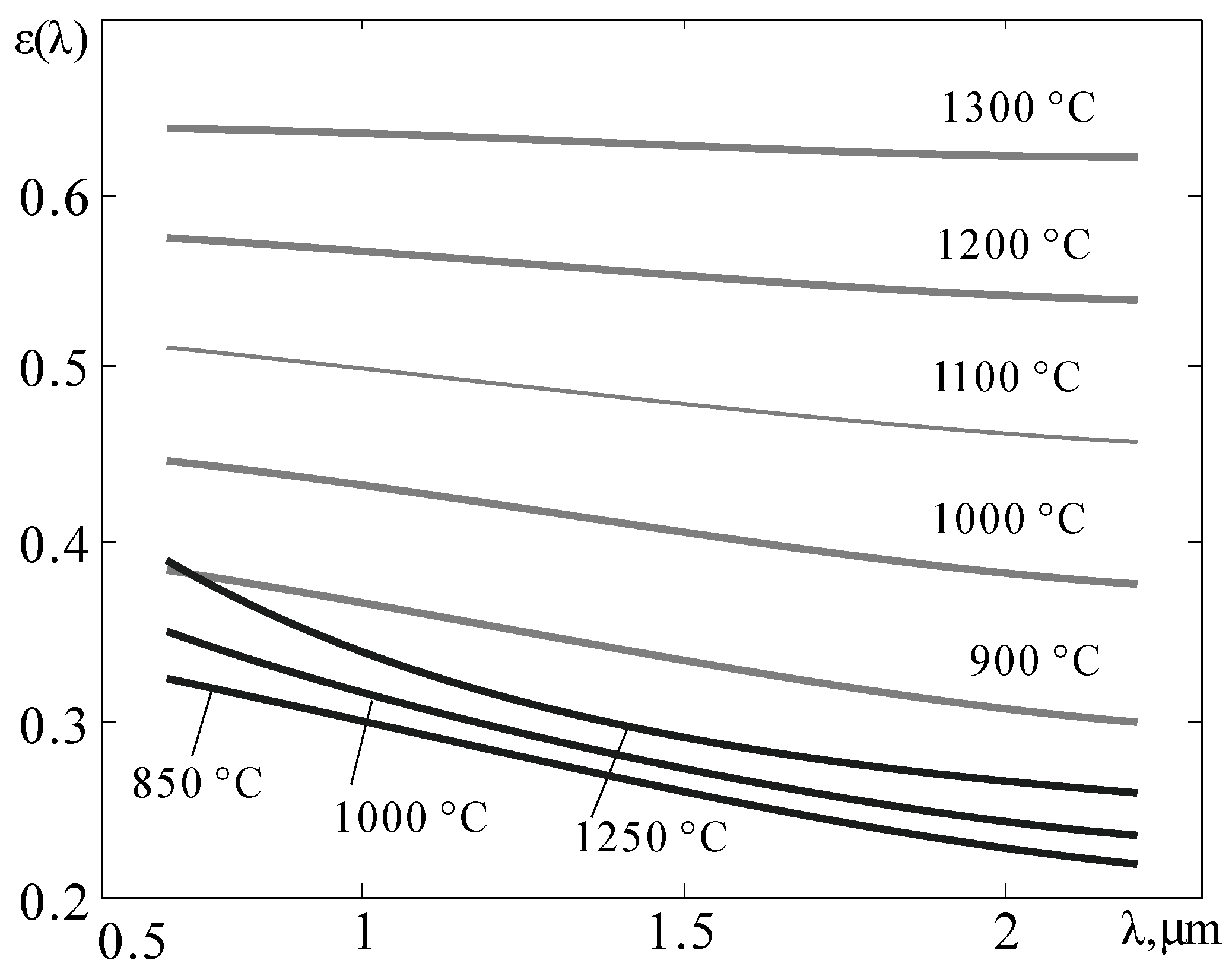
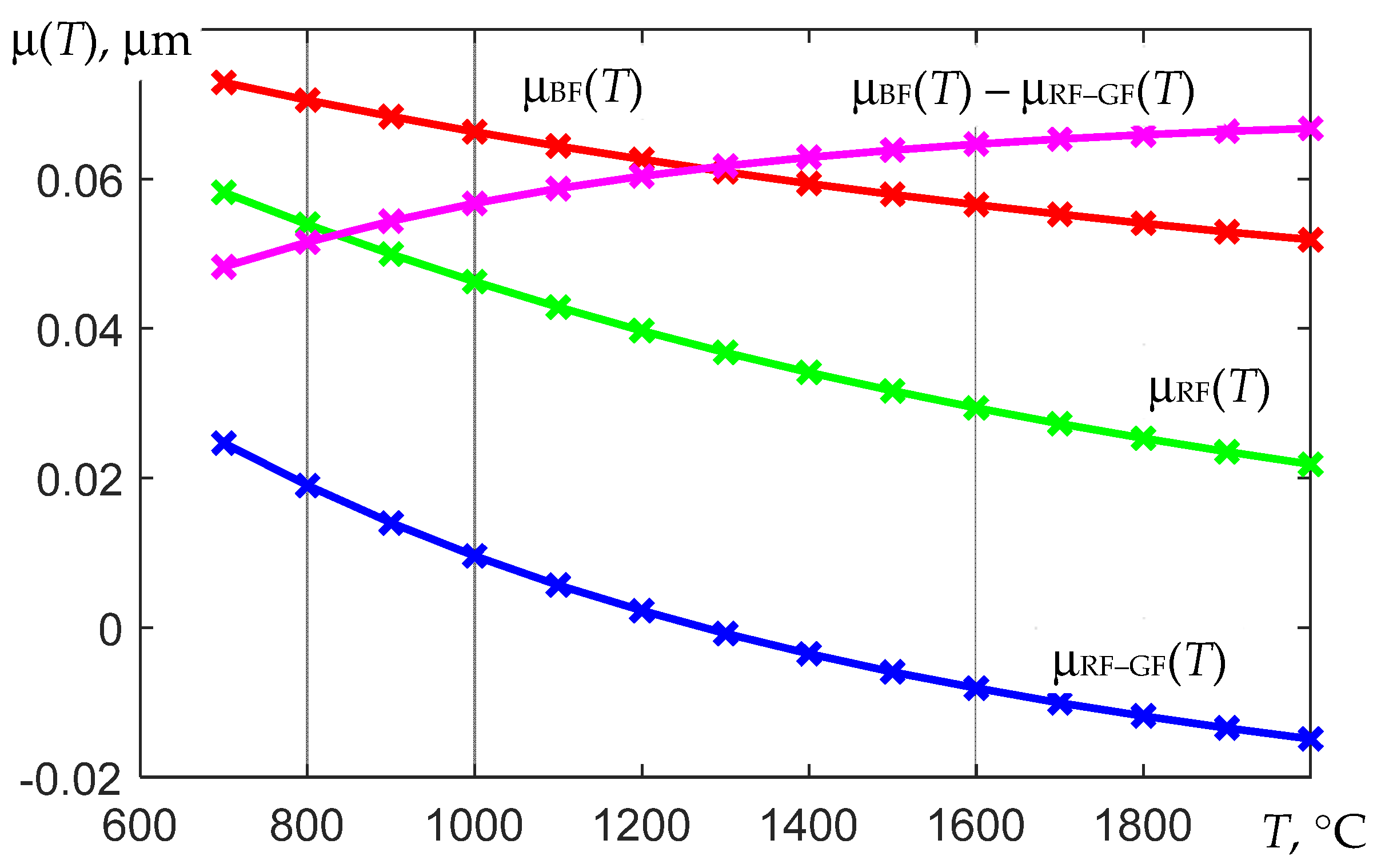
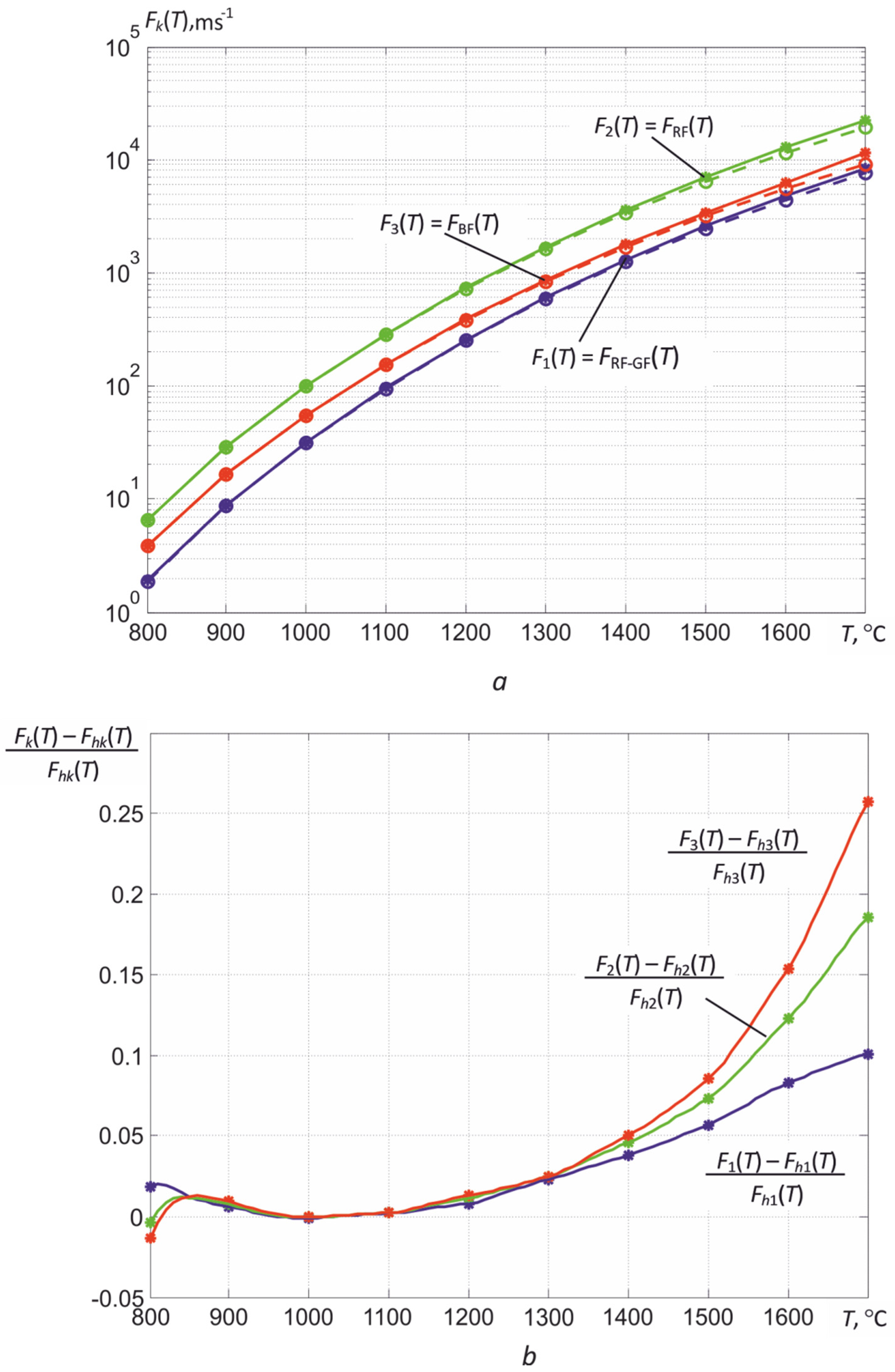
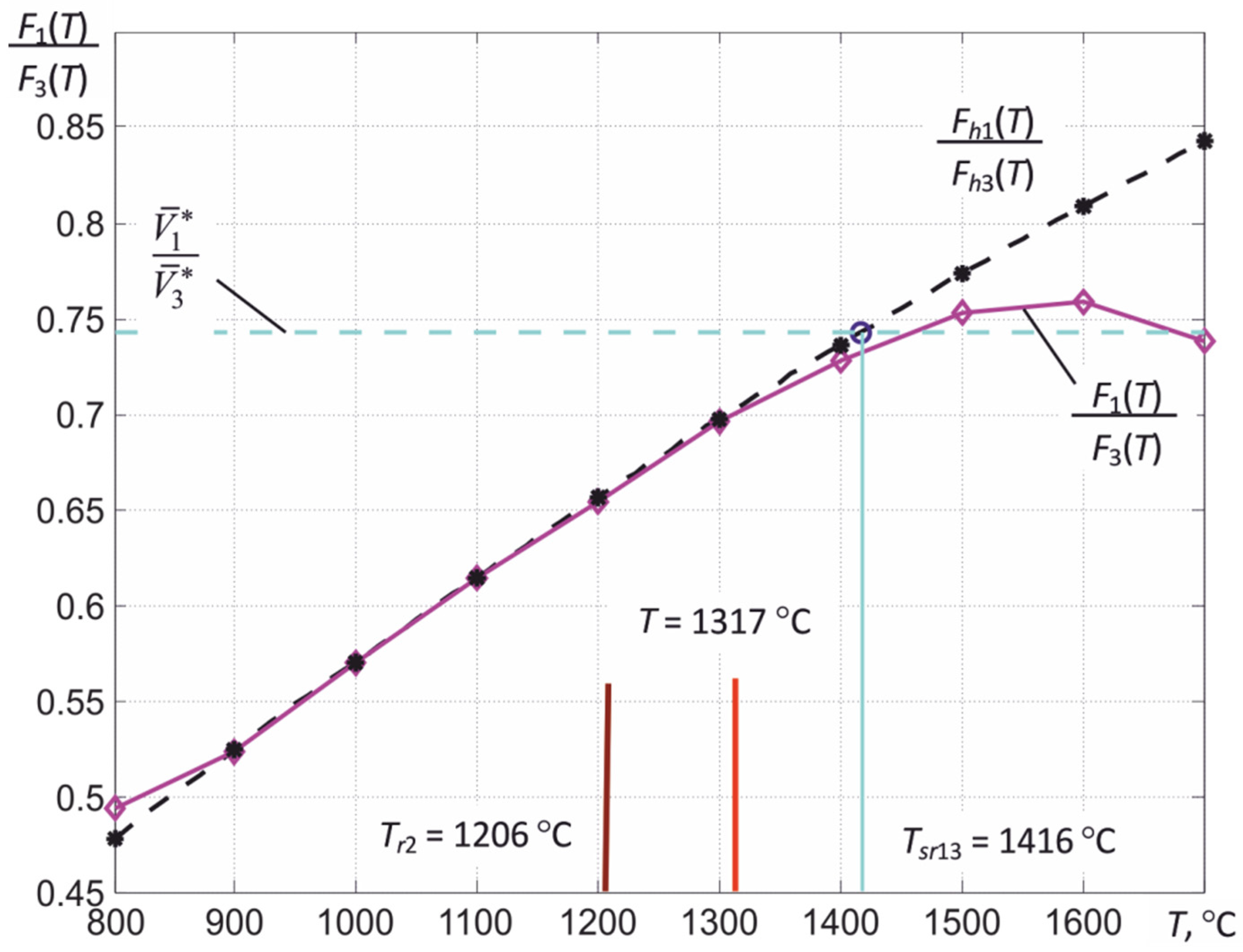
6. Correction of the Deviation of the Real Characteristics of the Photodetector Array from the Calculated Ones
7. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vavilov, V.; Burleigh, D. Infrared Thermography and Thermal Nondestructive Testing; Springer International Publishing: New York, NY, USA, 2020. [Google Scholar]
- Vollmer, M. Infrared Thermal Imaging: Fundamentals, Research and Applications, 2nd ed.; Wiley: Hoboken, NJ, USA, 2018. [Google Scholar]
- Firago, V.A. Correction of signals in a microbolometric array raising the validity of the measuring object’s temperature. Part 1. J. Eng. Phys. Thermophys. 2021, 94, 272–285. [Google Scholar] [CrossRef]
- Ruddock, R.W. Basic Infrared Thermography Principles, 1st ed.; Reliabilityweb.com Press: Fort Myers, FL, USA, 2010. [Google Scholar]
- Saadlaoui, Y.; Sijobert, J.; Doubenskaia, M.; Bertrand, P.; Feulvarch, E.; Bergheau, J.-M. Experimental Study of Thermomechanical Processes: Laser Welding and Melting of a Powder Bed. Crystals 2020, 10, 246. [Google Scholar] [CrossRef]
- Altenburg, S.J.; Straße, A.; Gumenyuk, A.; Maierhofer, C. In-situ monitoring of a laser metal deposition (LMD) process: Comparison of MWIR, SWIR and high-speed NIR thermography. Quant. InfraRed Thermogr. J. 2020, 1–18. [Google Scholar] [CrossRef]
- Yadav, P.; Rigo, O.; Arvieu, C.; Le Guen, E.; Lacoste, E. In Situ Monitoring Systems of The SLM Process: On the Need to Develop Machine Learning Models for Data Processing. Crystals 2020, 10, 524. [Google Scholar] [CrossRef]
- Advanced Energy. Understanding Two-Color (Ratio) Pyrometer Accuracy; Technical Note; Advanced Energy: Denver, CO, USA, 2020. [Google Scholar]
- Rockett, T.; Boone, N.; Richards, R.; Willmott, J.R. Thermal imaging metrology using high dynamic range near-infrared photovoltaic-mode camera. Sensors 2021, 21, 6151. [Google Scholar] [CrossRef] [PubMed]
- Firago, V.; Wojcik, W. High-temperature three-colour thermal imager. Przegląd Elektrotech. 2015, 91, 208–214. [Google Scholar] [CrossRef][Green Version]
- Dagel, D.; Grossetete, G.; Maccallum, D.O. Measurement of Laser Weld Temperatures for 3D Model Input; SAND2016-10703, 648545; Sandia National Laboratories: Albuquerque, NM, USA, 2016. [Google Scholar] [CrossRef]
- Qu, D.-X.; Berry, J.; Calta, N.P.; Crumb, M.F.; Guss, G.; Matthews, M.J. Temperature Measurement of Laser-Irradiated Metals Using Hyperspectral Imaging. Phys. Rev. Appl. 2020, 14, 014031–014043. [Google Scholar] [CrossRef]
- Schager, A.; Zauner, G.; Mayr, G.; Burgholzer, P. Extension of the thermographic signal reconstruction technique for an automated segmentation and depth estimation of subsurface defects. J. Imaging 2020, 6, 96. [Google Scholar] [CrossRef] [PubMed]
- LumaSense Technologies. MIKRON Thermal Imaging Cameras MSC640. Available online: https://www.advancedenergy.com/globalassets/resources-root/data-sheets/en-ti-mcs640-mcs640hd-data-sheet.pdf (accessed on 15 October 2018).
- Firago, V.; Wojcik, W.; Volkova, I. The principles of reducing temperature measurement uncertainty of modern thermal imaging system. Przegląd Elektrotech. 2016, 92, 117–120. [Google Scholar] [CrossRef][Green Version]
- Firago, V.A.; Sen’kov, A.G.; Savkova, Y.N.; Golub, T.V. Pirometricheskiy kontrol temperatury nagrevayemykh metallov na predpriyatiyakh mashinostroyeniya. Kontrol. Diagn. 2011, 5, 17–25. [Google Scholar]
- Manoi, A.; Saunders, P. Size-of-source Effect in Infrared Thermometers with Direct Reading of Temperature. Int. J. Thermophys. 2017, 38, 101. [Google Scholar] [CrossRef]
- Minkina, S.; Dudzik, W. Measurements in Infrared Thermography. In Infrared Thermography: Errors and Uncertainties; John Wiley & Sons, Ltd.: Chichester, UK, 2009. [Google Scholar]
- Chrzanowski, K. Testing Thermal Imagers: Practical Guidebook; Military University of Technology: Warsaw, Poland, 2010. [Google Scholar]
- Firago, V.; Sencov, A.; Wojcik, W. Pyrometry of hot metals with changing and nonuniform emissivity. Przeglad Electrotech. 2010, 86, 104–108. [Google Scholar]
- Snopko, V.N. Osnovy Metodov Pirometrii po Spektru Teplovogo Izlucheniya; Institut Fiziki Imeni B.I. Stepanova NAN Belarusi: Minsk, Belarus, 1999. [Google Scholar]
- IMEC. Hyperspectral Imaging. Sensors. Available online: https://www.imec-int.com/en/hyperspectral-imaging (accessed on 17 September 2018).
- Sheyndlin, A.Y. (Ed.) Izluchatel’nyye Svoystva Tverdykh Materialov: Spravochnik; Energiya: Moscow, Russia, 1974. [Google Scholar]
- Mani, M.; Lane, B.M.; Donmez, M.A.; Feng, S.C.; Moylan, S.P. A review on measurement science needs for real-time control of additive manufacturing metal powderbed fusion processes. Int. J. Prod. Res. 2017, 55, 1400–1418. [Google Scholar] [CrossRef]
- Hooper, P.A. Melt pool temperature and cooling rates in laser powder bed fusion. Addit. Manuf. 2018, 22, 548–559. [Google Scholar] [CrossRef]
- Firago, V.A.; Wojcik, W.; Dzhunisbekov, M.S. Monitoring of the Metal Surface Temperature during Laser Processing. Russ. Metall. 2019, 11, 1224–1230. [Google Scholar] [CrossRef]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wójcik, W.; Firago, V.; Smolarz, A.; Shedreyeva, I.; Yeraliyeva, B. Multispectral High Temperature Thermography. Sensors 2022, 22, 742. https://doi.org/10.3390/s22030742
Wójcik W, Firago V, Smolarz A, Shedreyeva I, Yeraliyeva B. Multispectral High Temperature Thermography. Sensors. 2022; 22(3):742. https://doi.org/10.3390/s22030742
Chicago/Turabian StyleWójcik, Waldemar, Vladimir Firago, Andrzej Smolarz, Indira Shedreyeva, and Bakhyt Yeraliyeva. 2022. "Multispectral High Temperature Thermography" Sensors 22, no. 3: 742. https://doi.org/10.3390/s22030742
APA StyleWójcik, W., Firago, V., Smolarz, A., Shedreyeva, I., & Yeraliyeva, B. (2022). Multispectral High Temperature Thermography. Sensors, 22(3), 742. https://doi.org/10.3390/s22030742






