Recent Progress in Long-Range Brillouin Optical Correlation Domain Analysis
Abstract
:1. Introduction
2. Operating Principle
2.1. SBS Interactions
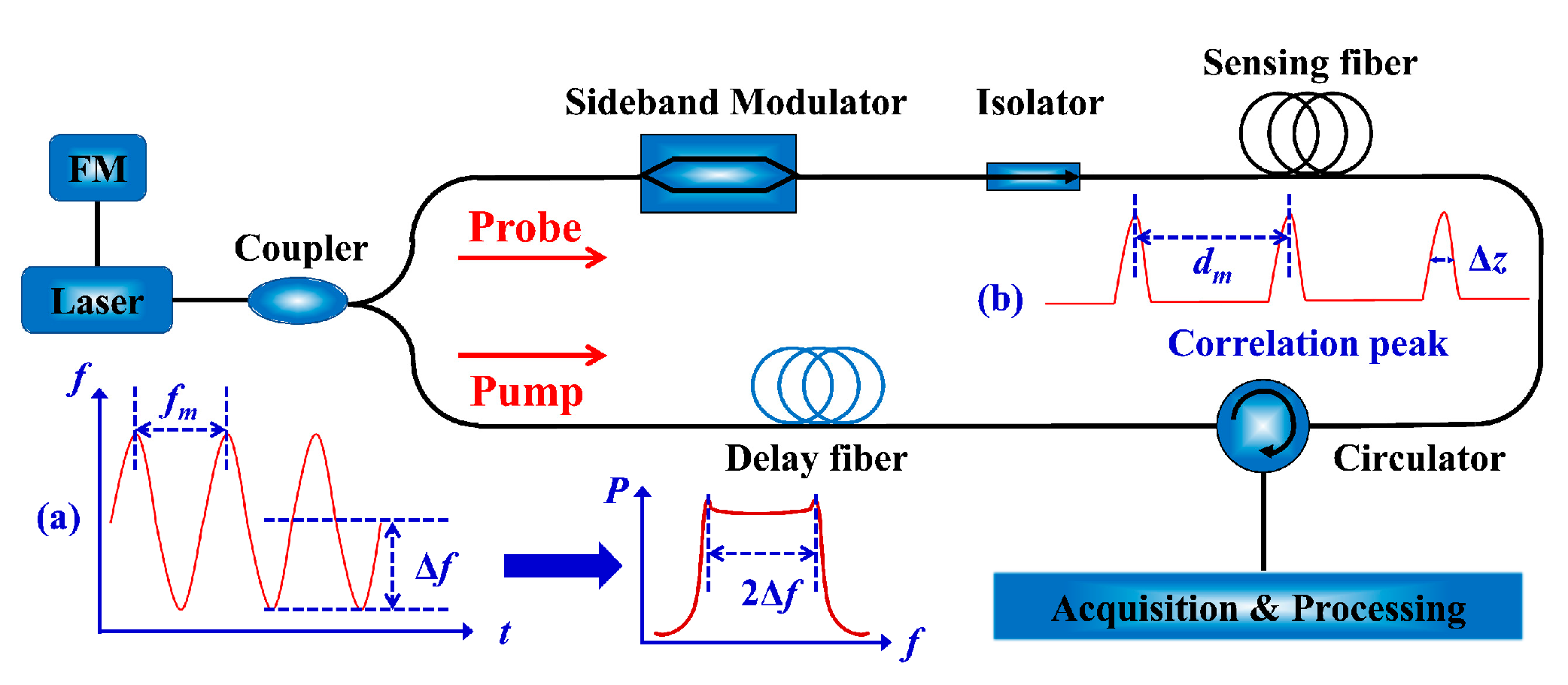
2.2. Basic Sine-FM BOCDA
2.3. Basic PRBS-PM BOCDA
2.4. Basic Broadband Source-Based BOCDA
3. Measurement Range Enlargement
3.1. Improvement in Measurement SNR
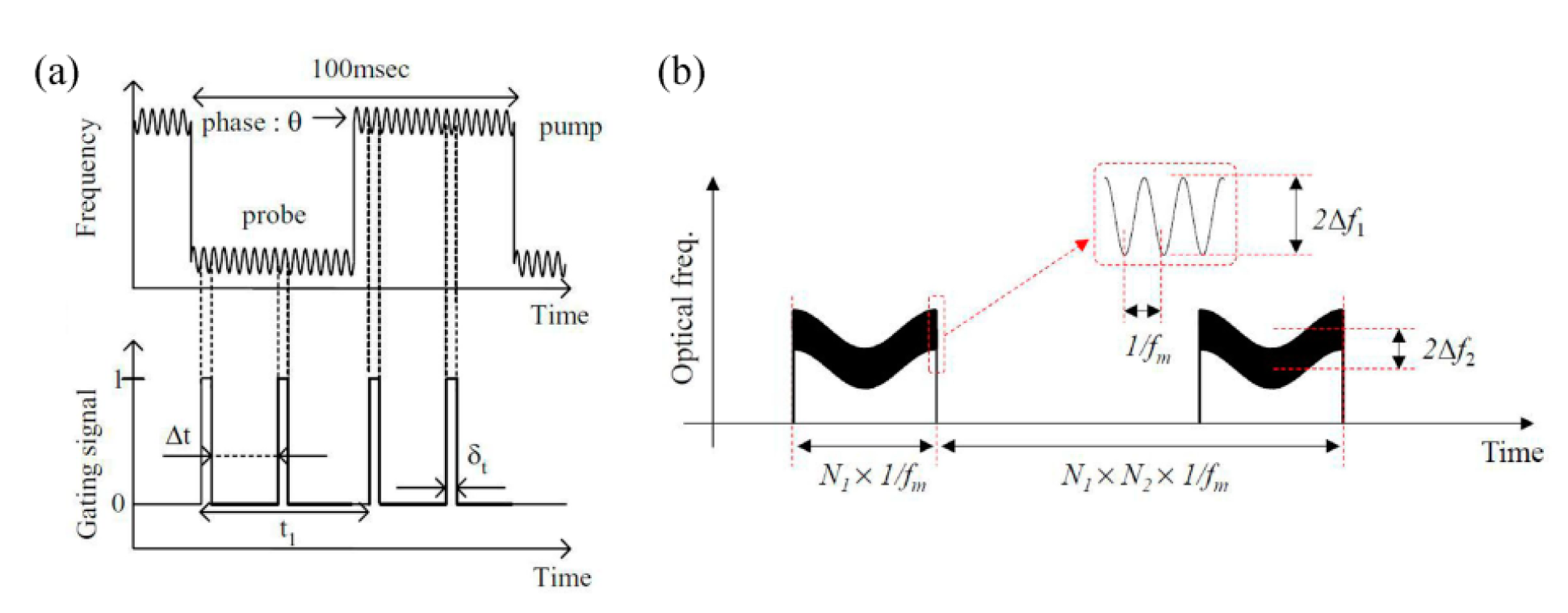
3.1.1. Sine-FM BOCDA
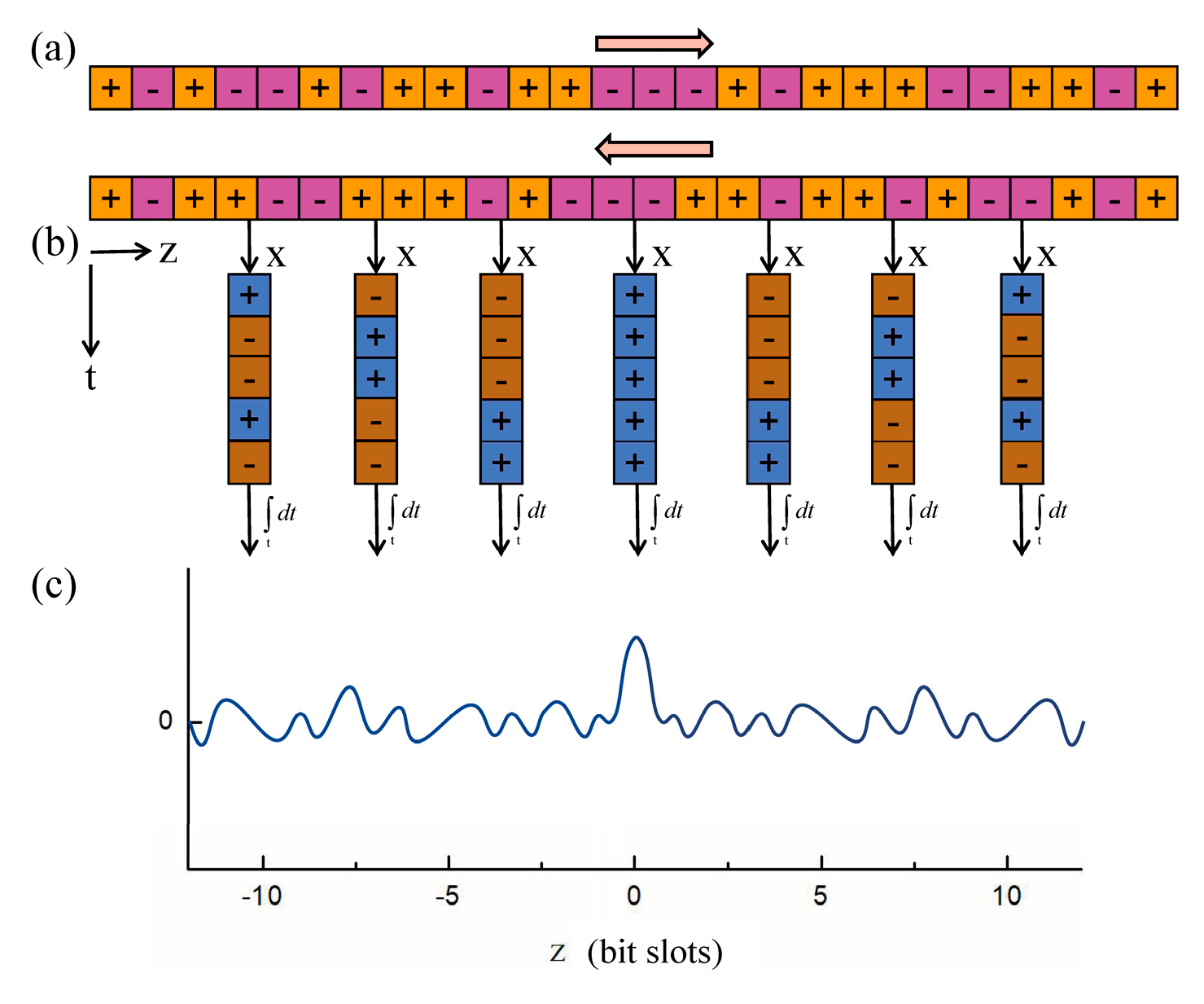
3.1.2. PM-BOCDA
3.1.3. Chaos-Based BOCDA
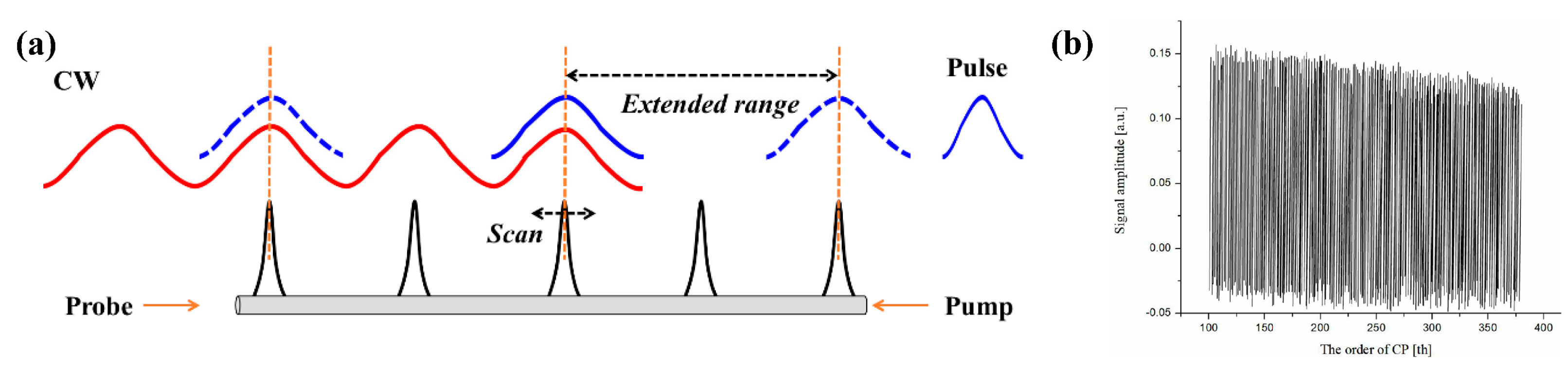
3.2. Concurrent Interrogation of Multiple CPs
3.2.1. Sine-FM BOCDA
3.2.2. PM BOCDA
4. Discussions
- (1)
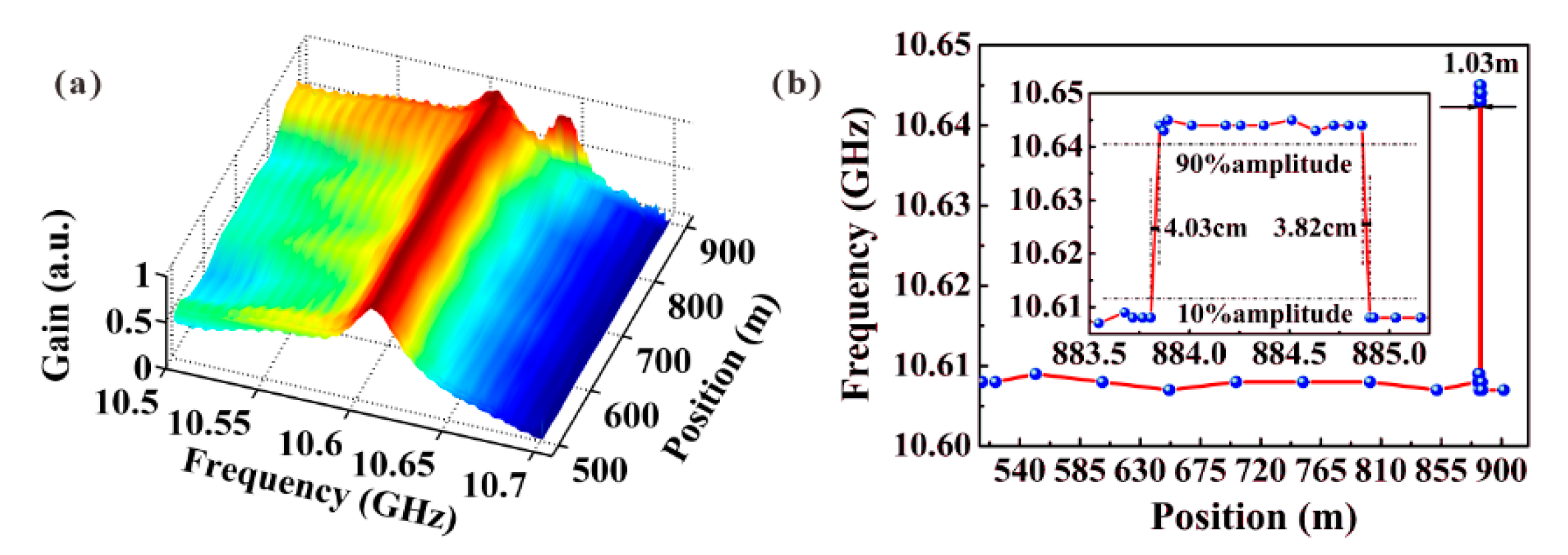
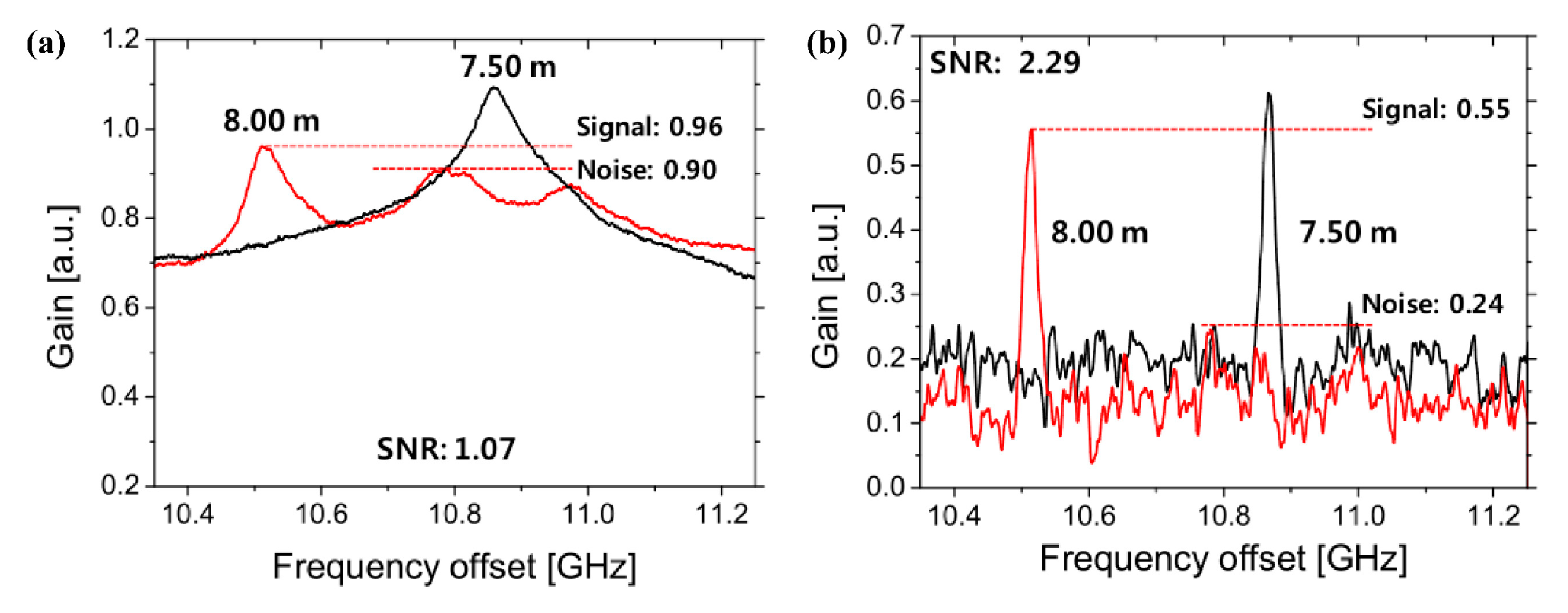
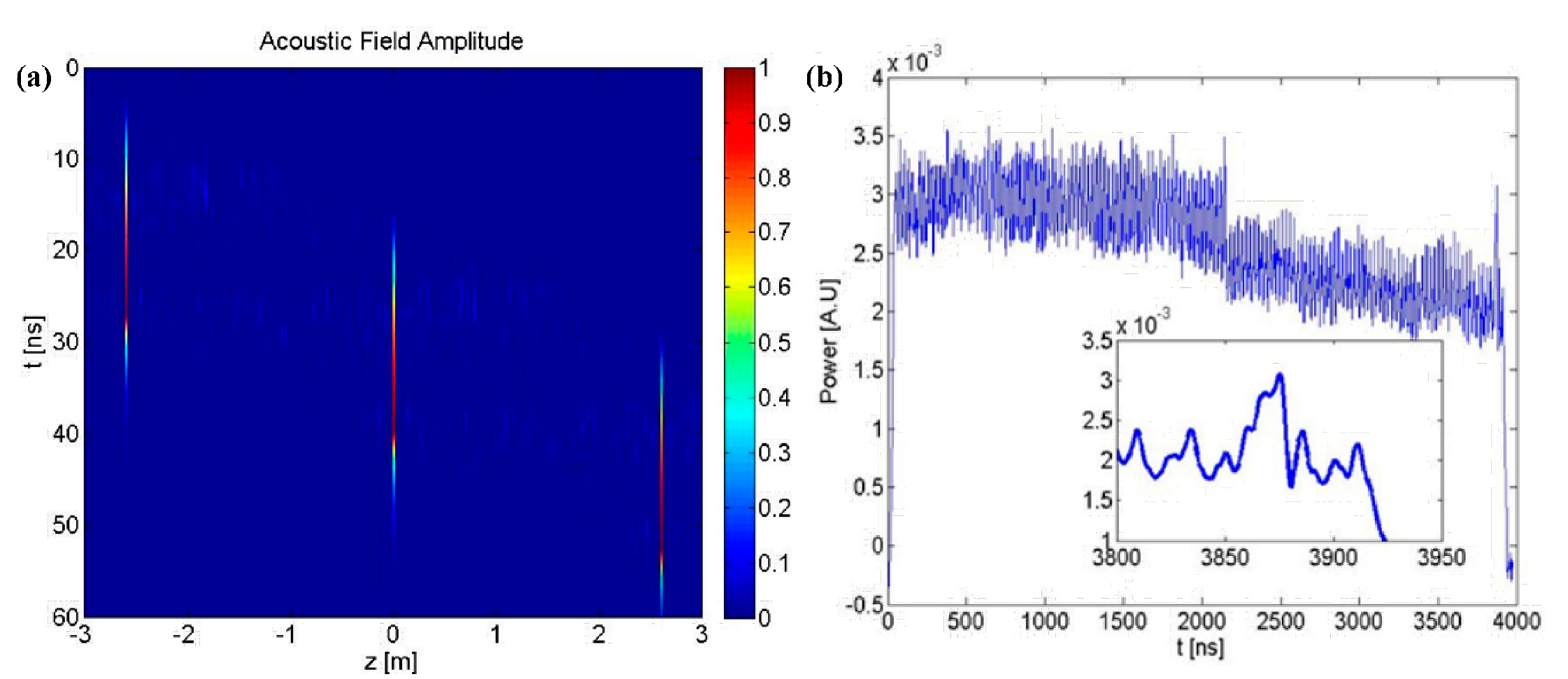
- In the sine-FM BOCDA systems, the temporal gating scheme is incipiently proposed to extend the interval of adjacent CPs and then the double modulation scheme is overlaid to maximize the MR in the single-CP system, where the differential measurement is employed to eliminate the intrinsic noise structure and the measurement SNR is significantly promoted. Further, the time-division multiplexing scheme is demonstrated to simultaneously interrogate a sequence of CPs at a single time trace of probe power, supplementing the DRA to compensate for the pump loss; therefore, the MR extends to 52.1 km, which is the longest sensing range conducted by BOCDA systems.
- (2)
- In the PM BOCDA systems, the OSNR is promoted by using the perfect Golomb code or incoherent pulse compression, i.e., a combination of amplitude and phase sequence coding. Based on the previous trials of time-domain data processing, an optimized time-division multiplexing scheme, including a careful choice of the sampling interval, pulse width, and PRBS length, experimentally enlarges the MR to 17.5 km with a SR of 8.3 mm, which is the best coupling performance between the MR and SR in Brillouin sensors.
- (3)
- In the broadband source-based BOCDA systems, the noise-like property ensures that there is a sole CP along the fiber, so the MR can be extended due to the gain anti-interference in principle. ASE-based BOCDA is proposed and demonstrated with a 5 cm short-range precise measurement in the proof-of-concept experiment. Notably, the chaos-based BOCDA promotes forceful competitiveness in coupling the long MR and high SR, owing to the superior SNR. After suppressing the chaos TDS, a time-gated scheme is proposed to restrain the noise background and promote the MR to 10.2 km with a SR of 9 cm. In addition, the inherently high-incoherence source, used as the sensing signal, can largely avoid the modulation bandwidth limitation to simply achieve a millimeter-scale SR.
- (1)
- Inferior SNR. In all the BOCDA schemes, a narrow and feeble acoustic field is essential to achieve a centimeter-level SR so that a trade-off problem is severe between the SNR and sensing distance.
- (2)
- Time-consuming measurement. The measurement speed of BOCDA schemes is limited by two aspects: one is the scanning of CP interval and the other is the frequency sweeping near the central BFS, although the inspired methods, including time-domain data processing using the single-pulse or double-pulse pair [62], as well as scanning-free BOCDA [63,64,65], have been verifiably proposed to shorten the measurement time.
- (3)
- SR deterioration. In the sine-FM and PM systems, the scanning of periodic CPs is achieved by adjusting the frequency of the sine wave or the rate of the bit sequence. As a result, the CP width also changes slightly during the localization process, leading to the SR worsening in principle [29]. Consequently, the SR will be gradually deteriorated by increasing the sensing distance, which limits the further expansion of MR, despite the fact that the phase-shift keying [66] and short-pulse optical source [67] have been adopted to achieve a sub-millimeter SR. It is noticeable that the SR of the chaos-based scheme is only dependent on the chaos bandwidth and will not change with an increase in the MR [49], implying that the chaos-based BOCDA has performed forceful competitiveness in coupling the long MR and high SR.
- (4)
- Ultra-long delay line. In the sine-FM and PM systems, in order to achieve a fully distributed measurement, the zeroth-order CP, invariably operating at the middle of the fiber, must be moved outside the FUT and only the higher-order CPs can ensure a lower measurement error in localization or SR. Consequently, the ultra-long delay fiber is obligatory to select the high-order CP in current schemes. For example, a 250 km delay fiber is opted in 50 km MR schemes [35]. In chaos-based BOCDA, the delay line, whose length may be shorter than the periodic schemes, must be programmable to localize the single CP by adjusting the delay length.
- (5)
- To obtain a higher performance, the stability, simplicity, and cost should also be considered, which is equally important in practical applications.
- (6)
- To open up more suitable applications. The unique advantage of ultra-high SR and distributed sensing can be employed for precise measurement, including simultaneous strain and temperature measurement [68], the distributed analysis of SBS over different waveguides [44,69,70,71], opto-mechanics community [72,73,74], and so on.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lu, P.; Lalam, N.; Badar, M.; Liu, B.; Chorpening, B.T.; Buric, M.P.; Ohodnicki, P.R. Distributed optical fiber sensing: Review and perspective. Appl. Phys. Rev. 2019, 6, 041302. [Google Scholar] [CrossRef]
- Murray, J.B.; Cerjan, A.; Redding, B. Distributed Brillouin fiber laser sensor. Optica 2022, 9, 80. [Google Scholar] [CrossRef]
- Bao, X.; Chen, L. Recent Progress in Brillouin Scattering Based Fiber Sensors. Sensors 2011, 11, 4152–4187. [Google Scholar] [CrossRef]
- Min, R.; Liu, Z.; Pereira, L.; Yang, C.; Sui, Q.; Marques, C. Optical fiber sensing for marine environment and marine structural health monitoring: A review. Opt. Laser Technol. 2021, 140, 107082. [Google Scholar] [CrossRef]
- Bao, X.; Zhou, Z.; Wang, Y. Review: Distributed time-domain sensors based on Brillouin scattering and FWM enhanced SBS for temperature, strain and acoustic wave detection. PhotoniX 2021, 2, 14. [Google Scholar] [CrossRef]
- Sun, X.Z.; Yang, Z.S.; Hong, X.B.; Zaslawski, S.; Wang, S.; Soto, M.A.; Gao, X.; Wu, J.; Thevenaz, L. Genetic-optimised aperiodic code for distributed optical fibre sensors. Nat. Commun. 2020, 11, 5774. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.Z.; Dong, Y.K.; Ba, D.X.; Bao, X.Y. High spatial resolution: An integrative review of its developments on the Brillouin optical time- and correlation-domain analysis. Meas. Sci. Technol. 2020, 31, 052001. [Google Scholar] [CrossRef]
- Choi, B.-H.; Seo, D.-C.; Kwon, Y.-S.; Kwon, I.-B. Application of the Proposed Fiber Optic Time Differential BOCDA Sensor System for Impact Damage Detection of a Composite Cylinder. Appl. Sci. 2021, 11, 10247. [Google Scholar] [CrossRef]
- Hotate, K. Brillouin Optical Correlation-Domain Technologies Based on Synthesis of Optical Coherence Function as Fiber Optic Nerve Systems for Structural Health Monitoring. Appl. Sci. 2019, 9, 187. [Google Scholar] [CrossRef]
- Zadok, A.; Preter, E.; London, Y. Phase-Coded and Noise-Based Brillouin Optical Correlation-Domain Analysis. Appl. Sci. 2018, 8, 1482. [Google Scholar] [CrossRef]
- Hotate, K. Measurement of Brillouin gain spectrum distribution along an optical fiber with a high spatial resolution using a novel correlation-based technique: Demonstration of 45 cm spatial resolution. In Proceedings of the 13th International Conference on Optical Fiber Sensors, Kyongju, Korea, 1 September 1999; Volume 3746, pp. 611–614. [Google Scholar] [CrossRef]
- Hotate, K.; Hasegawa, T. Measurement of Brillouin gain spectrum distribution along an optical fiber using a correlation-based technique-proposal, experiment and simulation. IEICE Trans. Electron. 2000, E83-C, 405–412. [Google Scholar]
- Song, K.Y.; He, Z.Y.; Hotate, K. Distributed strain measurement with millimeter-order spatial resolution based on Brillouin optical correlation domain analysis. Opt. Lett. 2006, 31, 2526–2528. [Google Scholar] [CrossRef]
- Song, K.Y.; He, Z.Y.; Hotate, K. Optimization of Brillouin optical correlation domain analysis system based on intensity modu-lation scheme. Opt. Express 2006, 14, 4256–4263. [Google Scholar] [CrossRef]
- Song, K.Y.; He, Z.Y.; Hotate, K. Effects of intensity modulation of light source on Brillouin optical correlation domain analysis. J. Light. Technol. 2007, 25, 1238–1246. [Google Scholar] [CrossRef]
- Miyake, D.; Ito, F. Brillouin optical correlation domain analysis based on intensity modulation. Opt. Fiber Technol. 2021, 66, 102656. [Google Scholar] [CrossRef]
- Wang, B.; Fan, X.Y.; Fu, Y.X.; He, Z.Y. Enhancement of strain/temperature measurement range and spatial resolution in Brillouin optical correlation domain analysis based on convexity extraction algorithm. IEEE Access 2019, 7, 32128–32136. [Google Scholar] [CrossRef]
- Song, K.Y.; Choi, J.H. Measurement error induced by the power-frequency delay of the light source in optical correlation-domain distributed Brillouin sensors. Opt. Lett. 2018, 43, 5078–5081. [Google Scholar] [CrossRef] [PubMed]
- Song, K.Y.; Youn, J.H.; Choi, J.H. Suppression of systematic errors in Brillouin optical correlation domain analysis based on injection-locking. J. Light. Technol. 2019, 37, 4421–4425. [Google Scholar] [CrossRef]
- Song, K.Y.; Hotate, K. Enlargement of measurement range in a Brillouin optical correlation domain analysis system using double lock-in amplifiers and a single-sideband modulator. IEEE Photonics Technol. Lett. 2006, 18, 499–501. [Google Scholar] [CrossRef]
- Hotate, K.; Arai, H.; Song, K.Y. Range-enlargement of simplified Brillouin optical correlation domain analysis based on a tem-poral gating scheme. SICE J. Control Meas. Syst. Integr. 2008, 1, 271–274. [Google Scholar] [CrossRef]
- Jeong, J.H.; Lee, S.B.; Jhon, Y.M.; Song, K.Y.; Jeong, J.M.; Lee, K. Extension of measurement range in Brillouin optical correlation domain analysis by pump-probe switching. Appl. Phys. B Lasers Opt. 2014, 116, 91–96. [Google Scholar] [CrossRef]
- Wang, B.; Fan, X.Y.; Du, J.B.; He, Z.Y. Performance enhancement of Brillouin optical correlation domain analysis based on fre-quency chirp magnification. Chin. Opt. Lett. 2017, 15, 120601. [Google Scholar] [CrossRef]
- Zadok, A.; Antman, Y.; Primerov, N.; Denisov, A.; Sancho, J.M.; Thevenaz, L. Random-access distributed fiber sensing. Laser Photonics Rev. 2012, 6, L1–L5. [Google Scholar] [CrossRef]
- Cohen, R.; London, Y.; Antman, Y.; Zadok, A. Brillouin optical correlation domain analysis with 4 millimeter resolution based on amplified spontaneous emission. Opt. Express 2014, 22, 12070–12078. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.Z.; Zhang, M.T.; Zhang, M.J.; Liu, Y.; Feng, C.K.; Wang, Y.H.; Wang, Y.C. Chaotic Brillouin optical correlation-domain analysis. Opt. Lett. 2018, 43, 1722–1725. [Google Scholar] [CrossRef]
- Jeong, J.H.; Lee, K.; Song, K.Y.; Jeong, J.M.; Lee, S.B. Bidirectional measurement for Brillouin optical correlation domain analysis. Opt. Express 2012, 20, 11091–11096. [Google Scholar] [CrossRef]
- Jeong, J.H.; Lee, K.; Jeong, J.M.; Lee, S.B. Measurement range enlargement in Brillouin optical correlation domain analysis using multiple correlation peaks. J. Opt. Soc. Korea 2012, 16, 210–214. [Google Scholar] [CrossRef]
- Ryu, G.; Kim, G.-T.; Song, K.Y.; Lee, S.B.; Lee, K. Brillouin Optical Correlation Domain Analysis Enhanced by Time-Domain Data Processing for Concurrent Interrogation of Multiple Sensing Points. J. Light. Technol. 2017, 35, 5311–5316. [Google Scholar] [CrossRef]
- Denisov, A.; Soto, M.A.; Thévenaz, L. Going beyond 1000000 resolved points in a Brillouin distributed fiber sensor: Theoretical analysis and experimental demonstration. Light. Sci. Appl. 2016, 5, e16074. [Google Scholar] [CrossRef] [PubMed]
- Jeong, J.H.; Lee, K.; Song, K.Y.; Jeong, J.M.; Lee, S.B. Differential measurement scheme for Brillouin optical correlation domain analysis. Opt. Express 2012, 20, 27094–27101. [Google Scholar] [CrossRef] [PubMed]
- Antman, Y.; Levanon, N.; Zadok, A. Low-noise delays from dynamic Brillouin gratings based on perfect Golomb coding of pump waves. Opt. Lett. 2012, 37, 5259–5261. [Google Scholar] [CrossRef]
- Zhang, J.; Feng, C.; Zhang, M.; Liu, Y.; Wu, C.; Wang, Y. Brillouin optical correlation domain analysis based on chaotic laser with suppressed time delay signature. Opt. Express 2018, 26, 6962–6972. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Y.; Zhang, M.; Zhang, Q.; Li, M.; Wu, C.; Qiao, L.; Wang, Y. Time-gated chaotic Brillouin optical correlation domain analysis. Opt. Express 2018, 26, 17597–17607. [Google Scholar] [CrossRef]
- Ryu, G.; Kim, G.-T.; Song, K.Y.; Lee, S.B.; Lee, K. 50 km-Range Brillouin Optical Correlation Domain Analysis with First-Order Backward Distributed Raman Amplification. J. Light. Technol. 2020, 38, 5199–5204. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics, 3rd ed.; Academic Press: Cambridge, MA, USA, 2008. [Google Scholar]
- Nikles, M.; Thevenaz, L.; Robert, P. Brillouin gain spectrum characterization in single-mode optical fibers. J. Light. Technol. 1997, 15, 1842–1851. [Google Scholar] [CrossRef]
- Brown, A.; Colpitts, B.; Brown, K. Distributed sensor based on dark-pulse Brillouin scattering. IEEE Photonics Technol. Lett. 2005, 17, 1501–1503. [Google Scholar] [CrossRef]
- Wang, F.; Bao, X.; Chen, L.; Li, Y.; Snoddy, J.; Zhang, X. Using pulse with a dark base to achieve high spatial and frequency resolution for the distributed Brillouin sensor. Opt. Lett. 2008, 33, 2707–2709. [Google Scholar] [CrossRef]
- Li, W.; Bao, X.; Li, Y.; Chen, L. Differential pulse-width pair BOTDA for high spatial resolution sensing. Opt. Express 2008, 16, 21616–21625. [Google Scholar] [CrossRef]
- Soto, M.A.; Thevenz, L. Modeling and evaluating the performance of Brillouin distributed optical fiber sensors. Opt. Express 2013, 21, 31347–31366. [Google Scholar] [CrossRef]
- Hotate, K. Application of synthesized coherence function to distributed optical sensing. Meas. Sci. Technol. 2002, 13, 1746–1755. [Google Scholar] [CrossRef]
- Hotate, K.; He, Z. Synthesis of optical-coherence function and its applications in distributed and multiplexed optical sensing. J. Light. Technol. 2006, 24, 2541–2557. [Google Scholar] [CrossRef]
- Zarifi, A.; Stiller, B.; Merklein, M.; Li, N.; Vu, K.; Choi, D.-Y.; Ma, P.; Madden, S.; Eggleton, B.J. Highly localized distributed Brillouin scattering response in a photonic integrated circuit. APL Photonics 2018, 3, 036101. [Google Scholar] [CrossRef]
- Qiao, L.; Lv, T.; Xu, Y.; Zhang, M.; Zhang, J.; Wang, T.; Zhou, R.; Wang, Q.; Xu, H. Generation of flat wideband chaos based on mutual injection of semiconductor lasers. Opt. Lett. 2019, 44, 5394–5397. [Google Scholar] [CrossRef]
- Yang, Q.; Qiao, L.; Zhang, M.; Zhang, J.; Wang, T.; Gao, S.; Chai, M.; Mohiuddin, P.M.; Mengmeng, C.; Promi, M.M. Generation of a broadband chaotic laser by active optical feedback loop combined with a high nonlinear fiber. Opt. Lett. 2020, 45, 1750–1753. [Google Scholar] [CrossRef]
- Yang, Q.; Qiao, L.J.; Wei, X.J.; Zhang, B.X.; Chai, M.M.; Zhang, J.Z.; Zhang, M.J. Flat broadband chaos generation using a semiconductor laser subject to asymmetric dual-path optical feedback. J. Light Technol. 2021, 39, 6246–6252. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, Y. Review on Chaotic Lasers and Measurement Applications. J. Light. Technol. 2020, 39, 3711–3723. [Google Scholar] [CrossRef]
- Wang, Y.H.; Zhang, M.J.; Zhang, J.H.; Qiao, L.J.; Wang, T.; Zhang, Q.; Zhao, L.; Wang, Y.C. Millimeter-level-spatial-resolution Brillouin optical correlation-domain analysis based on broadband chaotic laser. J. Light Technol. 2019, 37, 3706–3712. [Google Scholar] [CrossRef]
- Song, K.Y.; Youn, J.H. Effects of Differential Measurement Scheme on Brillouin Optical Correlation-Domain Analysis. J. Light. Technol. 2021, 39, 2609–2617. [Google Scholar] [CrossRef]
- Li, M.; Zhang, X.; Zhang, J.; Zhang, J.; Zhang, M.; Qiao, L.; Wang, T. True random coding for Brillouin optical correlation domain analysis. OSA Contin. 2019, 2, 2234–2243. [Google Scholar] [CrossRef]
- London, Y.; Antman, Y.; Cohen, R.; Kimelfeld, N.; Levanon, N.; Zadok, A. High-resolution long-range distributed Brillouin analysis using dual-layer phase and amplitude coding. Opt. Express 2014, 22, 27144–27158. [Google Scholar] [CrossRef]
- Hotate, K.; Arai, H. Enlargement of measurement range of simplified BOCDA fiber-optic distributed strain sensing system using a temporal gating scheme. In Proceedings of the 17th International Conference on Optical Fibre Sensors, Bruges, Belgium, 23 May 2005; Volume 5855, pp. 184–187. [Google Scholar] [CrossRef]
- Kim, Y.H.; Lee, K.; Song, K.Y. Brillouin optical correlation domain analysis with more than 1 million effective sensing points based on differential measurement. Opt. Express 2015, 23, 33241–33248. [Google Scholar] [CrossRef] [PubMed]
- Mizuno, Y.; He, Z.; Hotate, K. Measurement range enlargement in Brillouin optical correlation-domain reflectometry based on double-modulation scheme. Opt. Express 2010, 18, 5926–5933. [Google Scholar] [CrossRef] [PubMed]
- Ryu, G.; Kim, G.; Song, K.Y.; Lee, S.B.; Lee, K. BOCDA system enhanced by concurrent interrogation of multiple correlation peaks with a 10 km sensing range. In Proceedings of the 2017 25th Optical Fiber Sensors Conference (OFS), Jeju, Korea, 24 April 2017; pp. 1–4. [Google Scholar] [CrossRef]
- Alem, M.; Soto, M.A.; Thévenaz, L. Modelling the depletion length induced by modulation instability in distributed optical fibre sensors. In Proceedings of the 23rd Optical Fiber Sensors Conference, Santander, Spain, 2 June 2014; Volume 9157, pp. 821–824. [Google Scholar] [CrossRef]
- Li, J.; Zhang, M. Physics and applications of Raman distributed optical fiber sensing. Light. Sci. Appl. 2022, 11, 128. [Google Scholar] [CrossRef]
- Elooz, D.; Antman, Y.; Levanon, N.; Zadok, A. High-resolution long-reach distributed Brillouin sensing based on combined time-domain and correlation-domain analysis. Opt. Express 2014, 22, 6453–6463. [Google Scholar] [CrossRef]
- London, Y.; Antman, Y.; Preter, E.; Levanon, N.; Zadok, A. Brillouin Optical Correlation Domain Analysis Addressing 440,000 Resolution Points. J. Light. Technol. 2016, 34, 4421–4429. [Google Scholar] [CrossRef]
- Antman, Y.; Yaron, L.; Langer, T.; Tur, M.; Levanon, N.; Zadok, A. Experimental demonstration of localized Brillouin gratings with low off-peak reflectivity established by perfect Golomb codes. Opt. Lett. 2013, 38, 4701–4704. [Google Scholar] [CrossRef]
- Shlomi, O.; Preter, E.; Ba, D.; London, Y.; Antman, Y.; Zadok, A. Double-pulse pair Brillouin optical correlation-domain analysis. Opt. Express 2016, 24, 26867–26876. [Google Scholar] [CrossRef]
- Preter, E.; Ba, D.; London, Y.; Shlomi, O.; Antman, Y.; Zadok, A. High-resolution Brillouin optical correlation domain analysis with no spectral scanning. Opt. Express 2016, 24, 27253–27267. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, L.; Zhang, M.; Zhang, J.; Qiao, L.; Wang, T.; Gao, S.; Zhang, Q.; Wang, Y. Dynamic strain measurement by a single-slope-assisted chaotic Brillouin optical correlation-domain analysis. Opt. Lett. 2020, 45, 1822–1825. [Google Scholar] [CrossRef]
- Zhao, L.; Wang, Y.; Hu, X.; Guo, Y.; Zhang, J.; Qiao, L.; Wang, T.; Gao, S.; Zhang, M. Improvement of Strain Measurement Accuracy and Resolution by Dual-Slope-Assisted Chaotic Brillouin Optical Correlation Domain Analysis. J. Light. Technol. 2021, 39, 3312–3318. [Google Scholar] [CrossRef]
- Ba, D.X.; Li, Y.; Yan, J.L.; Zhang, X.P.; Dong, Y.K. Phase-coded Brillouin optical correlation domain analysis with 2-mm res-olution based on phase-shift keying. Opt. Express 2019, 27, 36197–36205. [Google Scholar] [CrossRef]
- Matsumoto, M.; Akai, S. High-Spatial-Resolution Brillouin Optical Correlation Domain Analysis Using Short-Pulse Optical Sources. J. Light. Technol. 2019, 37, 6007–6014. [Google Scholar] [CrossRef]
- Zhang, X.C.; Liu, S.S.; Zhang, J.Z.; Qiao, L.J.; Wang, T.; Gao, S.H.; Zhang, M.J. Simultaneous strain and temperature meas-urement based on chaotic Brillouin optical correlation-domain analysis in large-effective-area fibers. Photonic Sens. 2021, 11, 377–386. [Google Scholar] [CrossRef]
- Shin, H.; Qiu, W.; Jarecki, R.; Cox, J.A.; Olsson, R.H.; Starbuck, A.; Wang, Z.; Rakich, P.T. Tailorable stimulated Brillouin scattering in nanoscale silicon waveguides. Nat. Commun. 2013, 4, 1944. [Google Scholar] [CrossRef]
- Van Laer, R.; Kuyken, B.; Van Thourhout, D.; Baets, R. Interaction between light and highly confined hypersound in a silicon photonic nanowire. Nat. Photonics 2015, 9, 199–203. [Google Scholar] [CrossRef]
- Kittlaus, E.A.; Otterstrom, N.; Rakich, P.T. On-chip inter-modal Brillouin scattering. Nat. Commun. 2017, 8, 15819. [Google Scholar] [CrossRef] [PubMed]
- Bashan, G.; Diamandi, H.H.; London, Y.; Preter, E.; Zadok, A. Optomechanical time-domain reflectometry. Nat. Commun. 2018, 9, 2991. [Google Scholar] [CrossRef]
- Bashan, G.; London, Y.; Diamandi, H.H.; Zadok, A. Distributed cladding mode fiber-optic sensor. Optica 2020, 7, 85. [Google Scholar] [CrossRef]
- Pang, C.; Hua, Z.J.; Zhou, D.W.; Zhang, H.Y.; Chen, L.; Bao, X.Y.; Dong, Y.K. Opto-mechanical time-domain analysis based on coherent forward stimulated Brillouin scattering probing. Optica 2020, 7, 176–184. [Google Scholar] [CrossRef]







| Categories | Enhanced Techniques | Performances 1 | Merits | Disadvantages | |
|---|---|---|---|---|---|
| Sine-FM BOCDA | Temporal gating | 1 km@7 cm [21] | Longest MR | SR deterioration Bandwidth limitation | Inferior SNR Ultra-long delay line Time-consuming |
| Differential measurement | 10.5 km@1 cm [54] | ||||
| Time-division multiplexing | 10.15 km@5 cm [56] | ||||
| Distributed Raman amplification | 52.1 km@7 cm [35] | ||||
| PM BOCDA | Golomb codes | 0.4 km@2 cm [59] | Best coupling of MR/SR | ||
| Incoherent pulse compression | 8.8 km@2 cm [60] | ||||
| Time-division multiplexing | 17.5 km@0.83 cm [30] | ||||
| Chaos-based BOCDA | Ordinary chaos | 0.9 km@4 cm [26] | Inherently high- incoherence source | Localization by variable delay line | |
| TDS suppression | 3.2 km@7 cm [33] | ||||
| Temporal gating | 10.2 km@9 cm [34] | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zhang, M. Recent Progress in Long-Range Brillouin Optical Correlation Domain Analysis. Sensors 2022, 22, 6062. https://doi.org/10.3390/s22166062
Wang Y, Zhang M. Recent Progress in Long-Range Brillouin Optical Correlation Domain Analysis. Sensors. 2022; 22(16):6062. https://doi.org/10.3390/s22166062
Chicago/Turabian StyleWang, Yahui, and Mingjiang Zhang. 2022. "Recent Progress in Long-Range Brillouin Optical Correlation Domain Analysis" Sensors 22, no. 16: 6062. https://doi.org/10.3390/s22166062
APA StyleWang, Y., & Zhang, M. (2022). Recent Progress in Long-Range Brillouin Optical Correlation Domain Analysis. Sensors, 22(16), 6062. https://doi.org/10.3390/s22166062







