Study on a Detection Technique for Scholte Waves at the Seafloor
Abstract
:1. Introduction
2. Detection Principle
2.1. Acoustic Model
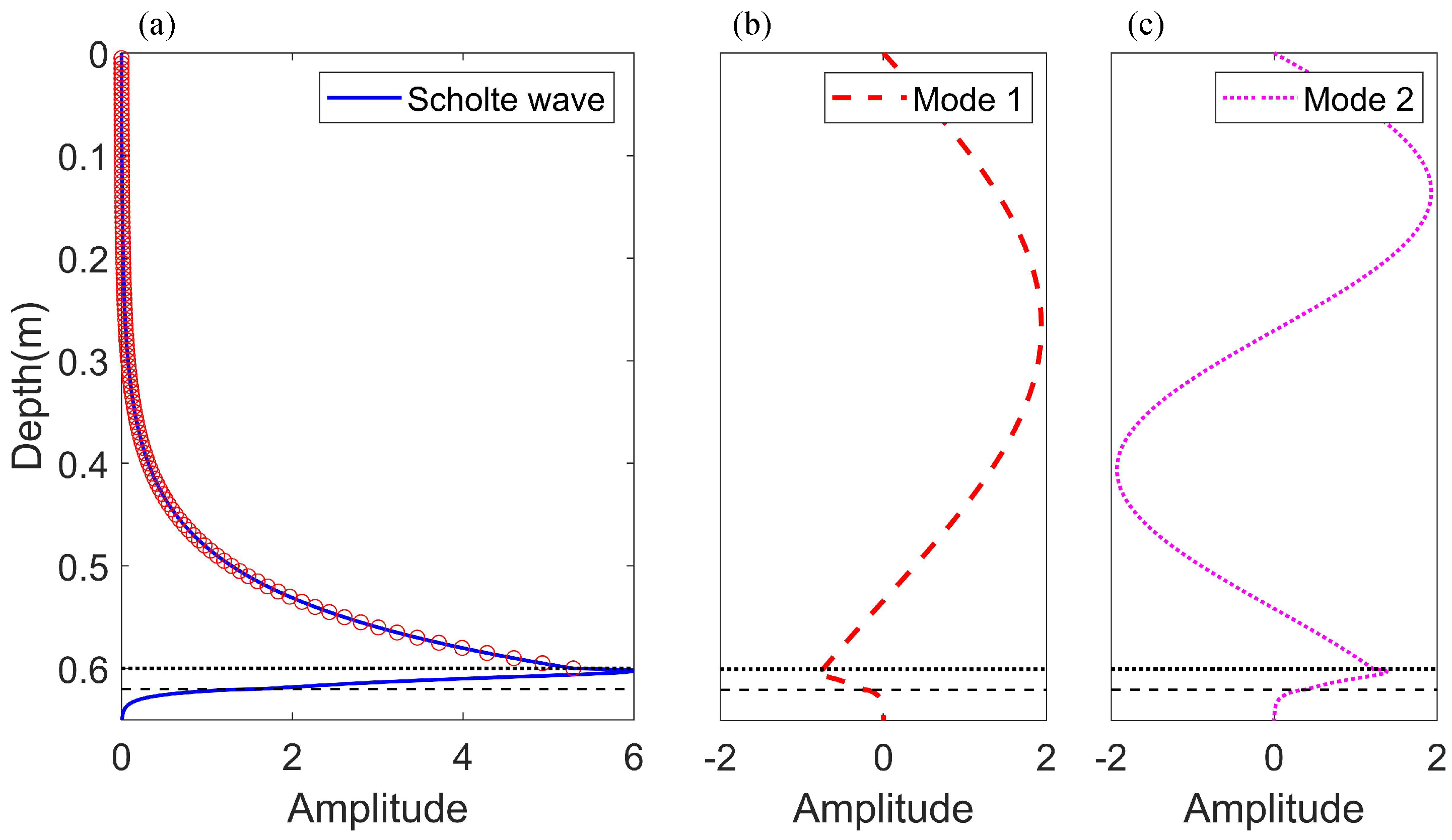
2.2. Elastic Normal Modes
3. Tank Experiment
3.1. Acoustic Field Analysis for the Laboratory Environment
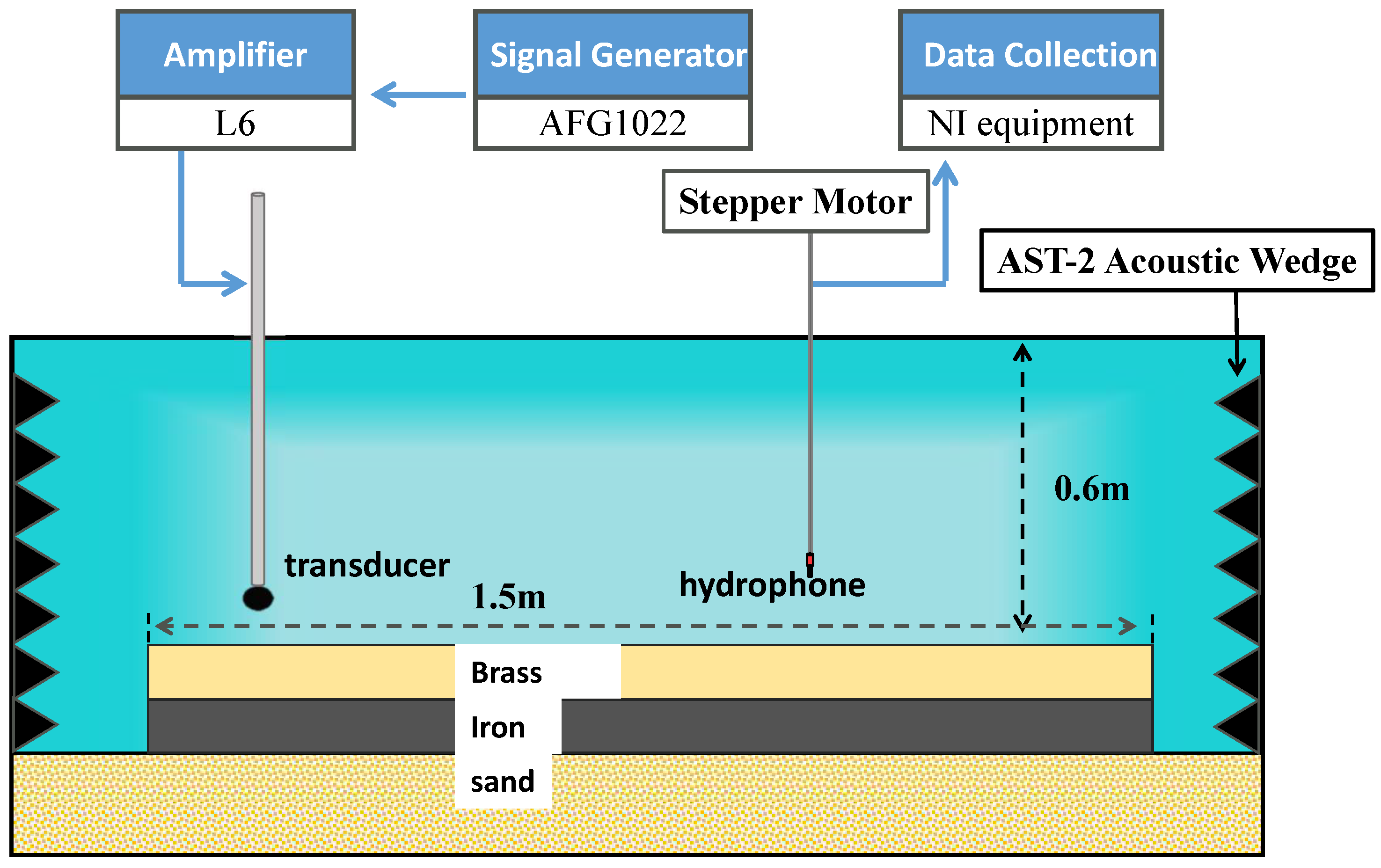
3.2. Experiment Settings
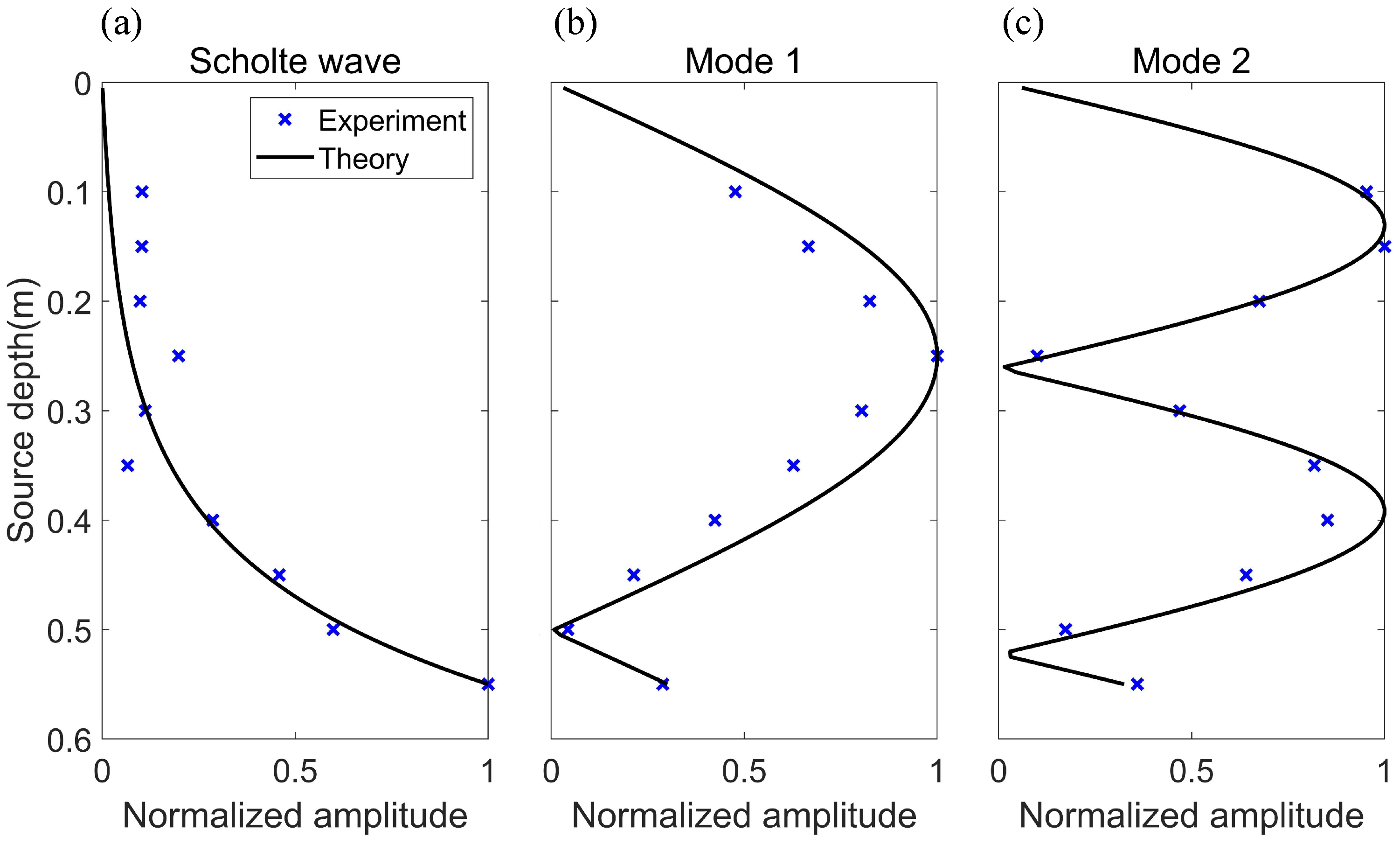
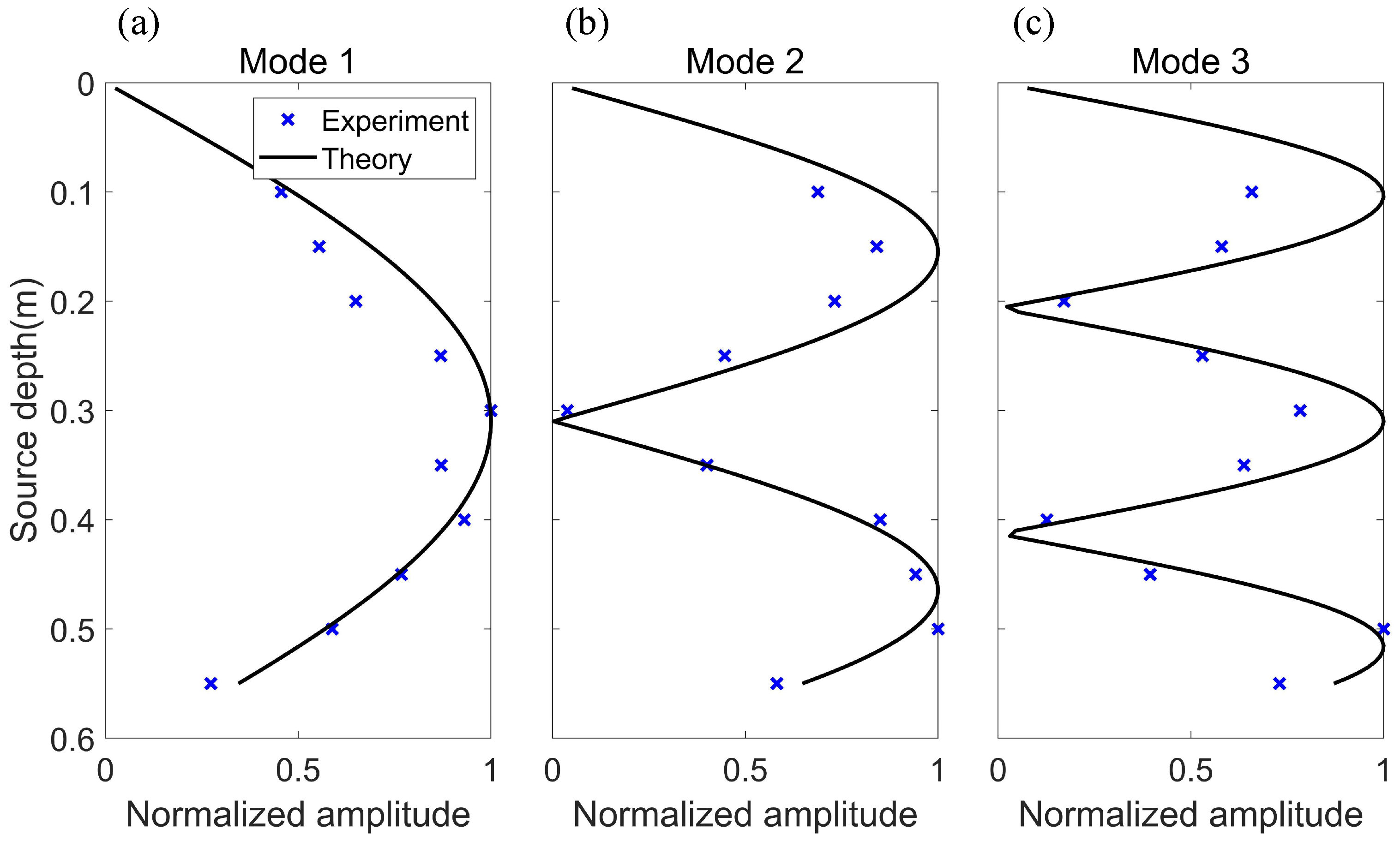
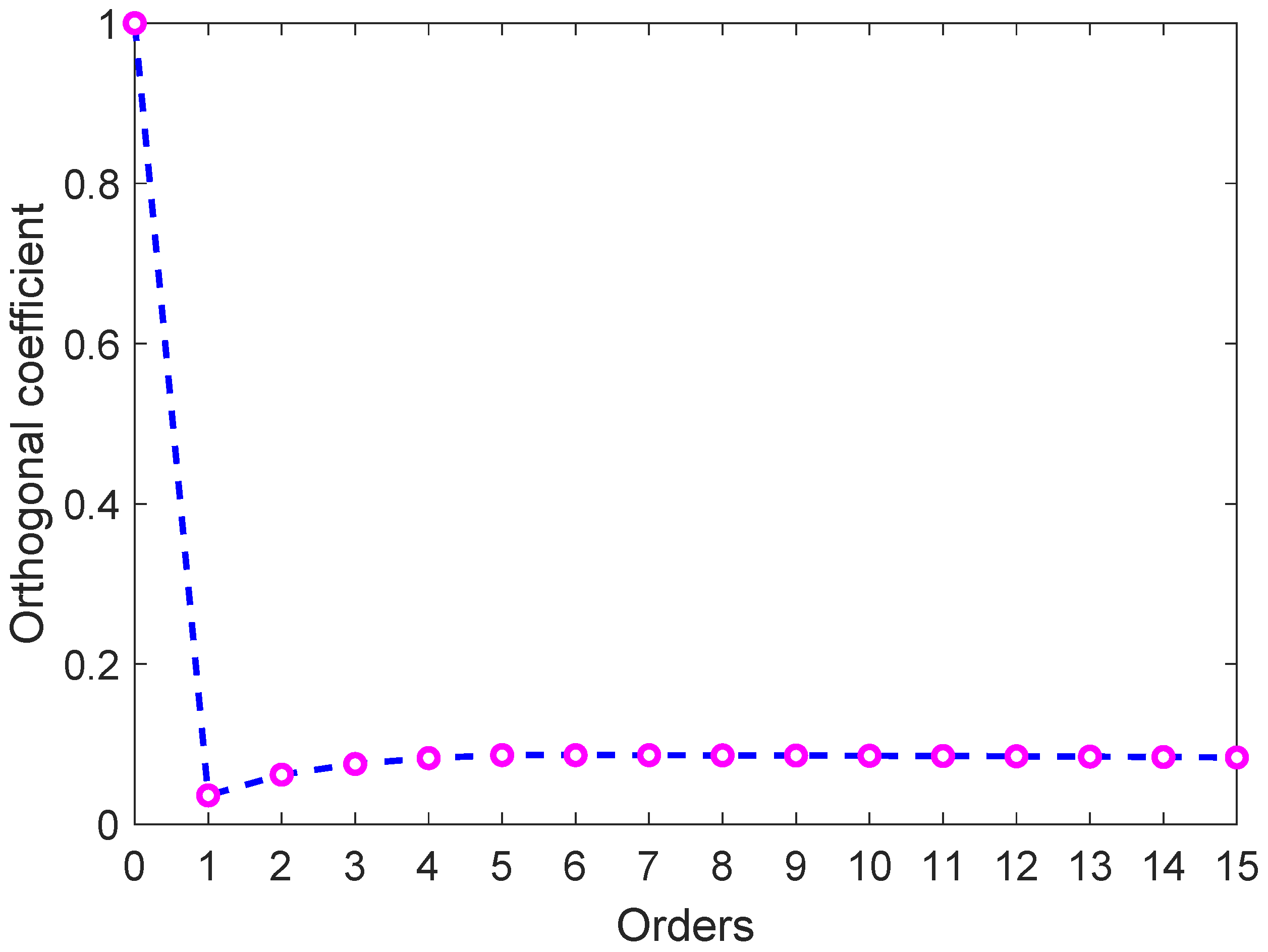
3.3. Experimental Data Analysis
4. Sediment Effect
4.1. Experiment Setting
4.2. Experimental Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Scholte, J. The range of existence of Rayleigh and Stoneley waves. Geophys. Suppl. Mon. Not. R. Soc. 1947, 5, 120–126. [Google Scholar] [CrossRef] [Green Version]
- Socco, L.V.; Foti, S.; Boiero, D. Surface-wave analysis for building near-surface velocity models—Established approaches and new perspectives. Geophysics 2010, 75, 75A83–75A102. [Google Scholar] [CrossRef]
- Nguyen, X.N.; Dahm, T.; Grevemeyer, I. Inversion of Scholte wave dispersion and waveform modeling for shallow structure of the Ninetyeast Ridge. J. Seismol. 2009, 13, 543–559. [Google Scholar] [CrossRef] [Green Version]
- Ritzwoller, M.H.; Levshin, A.L. Estimating shallow shear velocities with marine multicomponent seismic data. Geophysics 2002, 67, 1991–2004. [Google Scholar] [CrossRef]
- Zywicki, D.J.; Rix, G.J. Mitigation of near-field effects for seismic surface wave velocity estimation with cylindrical beamformers. J. Geotech. Geoenvironmental Eng. 2005, 131, 970–977. [Google Scholar] [CrossRef]
- Potty, G.R.; Miller, J.H. Measurement and modeling of Scholte wave dispersion in coastal waters. In Proceedings of the AIP Conference Proceedings, Ft. Worth, TX, USA, 5–10 August 2012; American Institute of Physics: NewYork, NY, USA, 2012; Volume 1495, pp. 500–507. [Google Scholar]
- Dong, Y.; Piao, S.; Gong, L.; Zheng, G.; Iqbal, K.; Zhang, S.; Wang, X. Scholte wave dispersion modeling and subsequent application in seabed shear-wave velocity profile inversion. J. Mar. Sci. Eng. 2021, 9, 840. [Google Scholar] [CrossRef]
- Godin, O.A.; Deal, T.J.; Dong, H. Physics-based characterization of soft marine sediments using vector sensors. J. Acoust. Soc. Am. 2021, 149, 49–61. [Google Scholar] [CrossRef] [PubMed]
- TenCate, J.A.; Muir, T.G.; Caiti, A.; Kristensen, Å.; Manning, J.F.; Shooter, J.A.; Koch, R.A.; Michelozzi, E. Beamforming on seismic interface waves with an array of geophones on the shallow sea floor. IEEE J. Ocean. Eng. 1995, 20, 300–310. [Google Scholar] [CrossRef] [Green Version]
- Ren, B.; Li, H. Characteristics of Scholte Wave for Target Detection. In Advances in Wireless Communications and Applications; Springer: Berlin/Heidelberg, Germany, 2021; pp. 115–121. [Google Scholar]
- Williams, E.F.; Fernández-Ruiz, M.R.; Magalhaes, R.; Vanthillo, R.; Zhan, Z.; González-Herráez, M.; Martins, H.F. Scholte wave inversion and passive source imaging with ocean-bottom DAS. Lead. Edge 2021, 40, 576–583. [Google Scholar] [CrossRef]
- Rauch, D. Seismic Interface Waves in Coastal Waters: A Review, SACLANTCEN SR-42; SACLANT ASW Research Centre: La Spezia, Italy, 1980. [Google Scholar]
- Wang, X.; Xia, C.; Liu, X. A case study: Imaging OBS multiples of South China Sea. Mar. Geophys. Res. 2012, 33, 89–95. [Google Scholar] [CrossRef]
- Du, S.; Cao, J.; Zhou, S.; Qi, Y.; Jiang, L.; Zhang, Y.; Qiao, C. Observation and inversion of very-low-frequency seismo-acoustic fields in the South China Sea. J. Acoust. Soc. Am. 2020, 148, 3992–4001. [Google Scholar] [CrossRef] [PubMed]
- Zhu-Bo, L.I.; Pan, F.R. Present Situation and Prospect of Submarine Seismic Observation Technology. N. China Earthq. Sci. 2015, 33, 56–63. [Google Scholar]
- Wang, S.; Qiu, X.; Zhao, M.; Li, P.; Liu, L.; Zhang, Y.; Xie, Z. Signal transfer and noise level of Ocean Bottom Seismometers. Chin. J. Geophys. 2019, 62, 3199–3207. [Google Scholar]
- Cao, J.; Qi, Y.; Zhou, S.; Du, S.; Peng, Z.; Zhang, Y.; Qiao, C. Anomalous dispersion observed in signal arrivals at a deep-sea floor receiver. JASA Express Lett. 2021, 1, 076004. [Google Scholar] [CrossRef]
- Dong, Y.; Piao, S.; Gong, L. Effect of three-dimensional seamount topography on very low frequency sound field in deep water. J. Harbin Eng. Univ. 2020, 41, 1464–1470. [Google Scholar]
- Tang, K.; Cheng, G.; Liu, B. Seismo-acoustic signal extraction from shallow sea seabed seimic wave field based on FK method. J. Phys. Conf. Ser. 2022, 2246, 012023. [Google Scholar] [CrossRef]
- Yuan, D.; Nazarian, S. Automated surface wave method: Inversion technique. J. Geotech. Eng. 1993, 119, 1112–1126. [Google Scholar] [CrossRef]
- Meng, X.; Zhang, J.; Zhao, S.; Wang, X. Study on the Influence of Shallow Sea Sediments on the Frequency Dispersion of Scholte Wave in Ship Seismic Wave. In Proceedings of the ICMLCA 2021; 2nd International Conference on Machine Learning and Computer Application, VDE, Shenyang, China, 12–14 November 2021; pp. 1–4. [Google Scholar]
- Schneiderwind, J.D.; Collis, J.M.; Simpson, H.J. Elastic Pekeris waveguide normal mode solution comparisons against laboratory data. J. Acoust. Soc. Am. 2012, 132, EL182–EL188. [Google Scholar] [CrossRef] [PubMed]
- Hamilton, E.L. Geoacoustic modeling of the sea floor. J. Acoust. Soc. Am. 1980, 68, 1313–1340. [Google Scholar] [CrossRef]
- Heacock, J.G. The Earth’s Crust: Its Nature and Physical Properties; American Geophysical Union: Washington, DC, USA, 1977; Volume 20, pp. 16–24. [Google Scholar]
- Jensen, F.B.; Kuperman, W.A.; Porter, M.B.; Schmidt, H.; Tolstoy, A. Computational Ocean Acoustics; Springer: Berlin/Heidelberg, Germany, 2011; Volume 794, pp. 375–380. [Google Scholar]
- Ewing, W.M.; Jardetzky, W.S.; Press, F.; Beiser, A. Elastic Waves in Layered Media, 1st ed.; McGraw-Hill Book Company, Inc.: New York, NY, USA, 1957; pp. 156–189. [Google Scholar]
- Hall, M.; Gordon, D.F.; White, D. Improved methods for determining eigenfunctions in multilayered normal-mode problems. J. Acoust. Soc. Am. 1983, 73, 153–162. [Google Scholar] [CrossRef]
- Porter, M.B. The KRAKEN Normal Mode Program; Technical Report; Naval Research Lab: Washington, DC, USA, 1992. [Google Scholar]
- Simon, B.; Isakson, M.; Ballard, M. Modeling acoustic wave propagation and reverberation in an ice covered environment using finite element analysis. In Proceedings of the Meetings on Acoustics 175ASA, Minneapolis, MN, USA, 7–11 May 2018; Acoustical Society of America: NewYork, NY, USA, 2018; Volume 33, p. 070002. [Google Scholar]
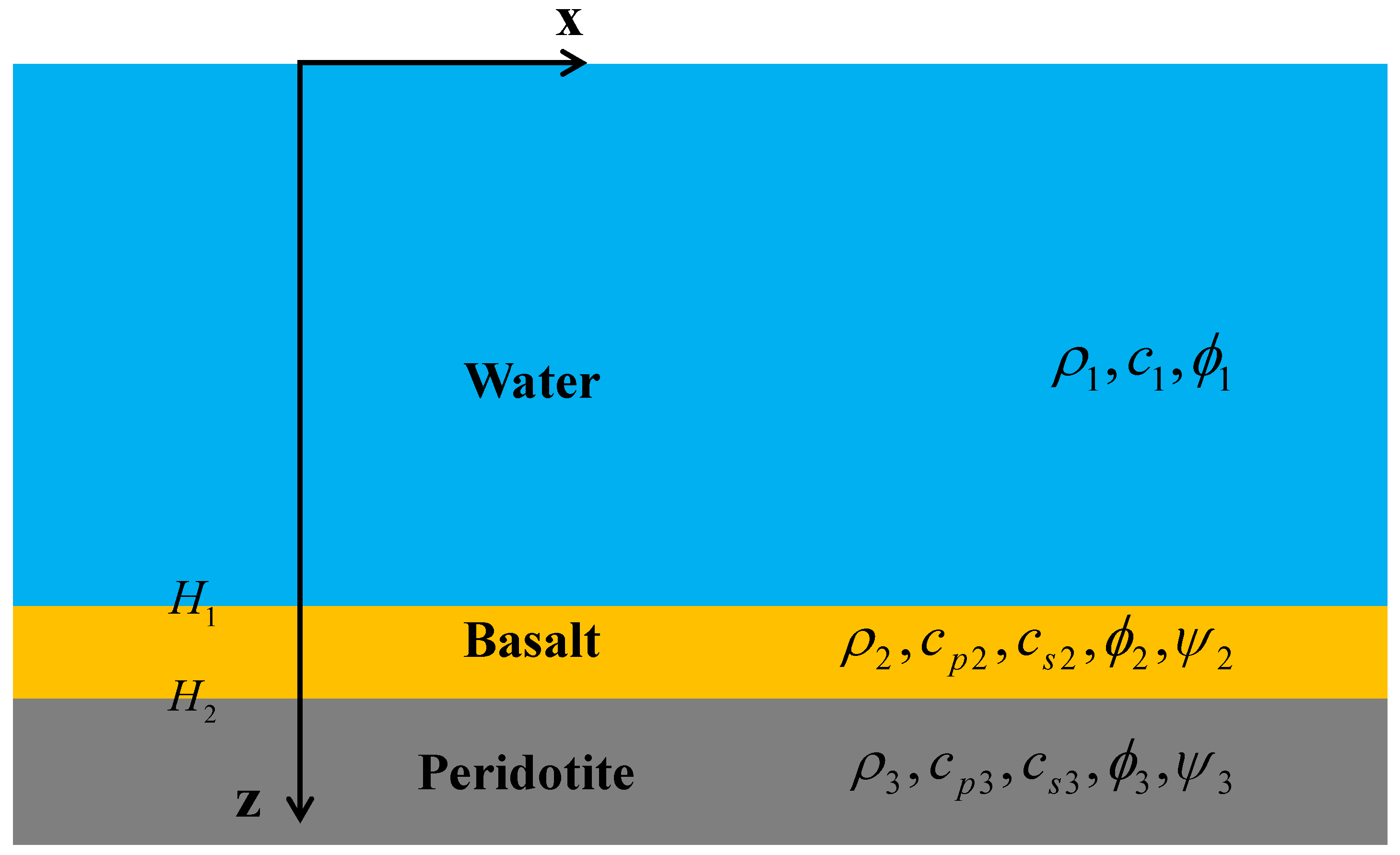






| Media | Layer i | Depth | (g/cm) | (m/s) | (m/s) |
|---|---|---|---|---|---|
| Seawater | 1 | 3000 | 1 | 1500 | - |
| Basalt | 2 | 3100 | 2.7 | 5250 | 2500 |
| Peridotite | 3 | - | 3.28 | 6500 | 4000 |
| Order | Phase Velocity (m/s) |
|---|---|
| 0 | 1489.741 |
| 1 | 1500.537 |
| 2 | 1502.139 |
| 3 | 1504.784 |
| 4 | 1508.457 |
| Media | Layer i | Depth | (g/cm) | (m/s) | (m/s) |
|---|---|---|---|---|---|
| Water | 1 | 0.6 | 1 | 1485 | - |
| Brass | 2 | 0.62 | 8.54 | 4640 | 2050 |
| Iron | 3 | 0.64 | 7.7 | 5850 | 3230 |
| Media | Layer i | Depth | (g/cm) | (m/s) | (m/s) |
|---|---|---|---|---|---|
| Seawater | 1 | 0.6 | 1 | 1500 | - |
| Silt | 2 | 0.601 | 1.2 | 1600 | - |
| Basalt | 3 | 0.621 | 8.54 | 4640 | 2050 |
| Peridotite | 4 | 0.641 | 7.7 | 5850 | 3230 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, M.; Wang, L.; Yu, G.; Ren, Y.; Peng, L. Study on a Detection Technique for Scholte Waves at the Seafloor. Sensors 2022, 22, 5344. https://doi.org/10.3390/s22145344
Liang M, Wang L, Yu G, Ren Y, Peng L. Study on a Detection Technique for Scholte Waves at the Seafloor. Sensors. 2022; 22(14):5344. https://doi.org/10.3390/s22145344
Chicago/Turabian StyleLiang, Minshuai, Liang Wang, Gaokun Yu, Yun Ren, and Linhui Peng. 2022. "Study on a Detection Technique for Scholte Waves at the Seafloor" Sensors 22, no. 14: 5344. https://doi.org/10.3390/s22145344
APA StyleLiang, M., Wang, L., Yu, G., Ren, Y., & Peng, L. (2022). Study on a Detection Technique for Scholte Waves at the Seafloor. Sensors, 22(14), 5344. https://doi.org/10.3390/s22145344






