Full-Field Operational Modal Analysis of an Aircraft Composite Panel from the Dynamic Response in Multi-Impact Test
Abstract
1. Introduction
2. Experimental Methodology and Analysis
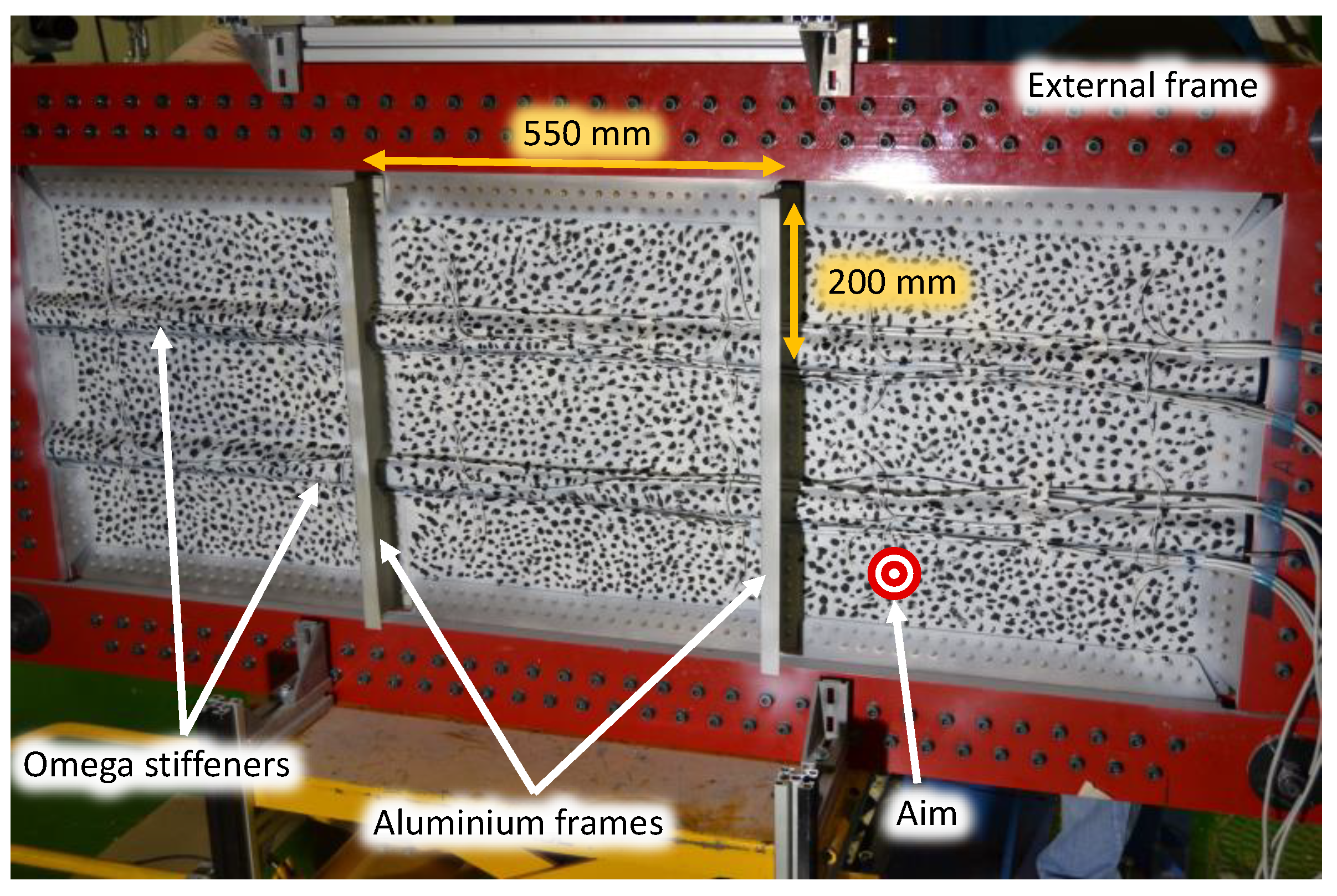
2.1. Specimen Description
2.2. Multihit Test Setup
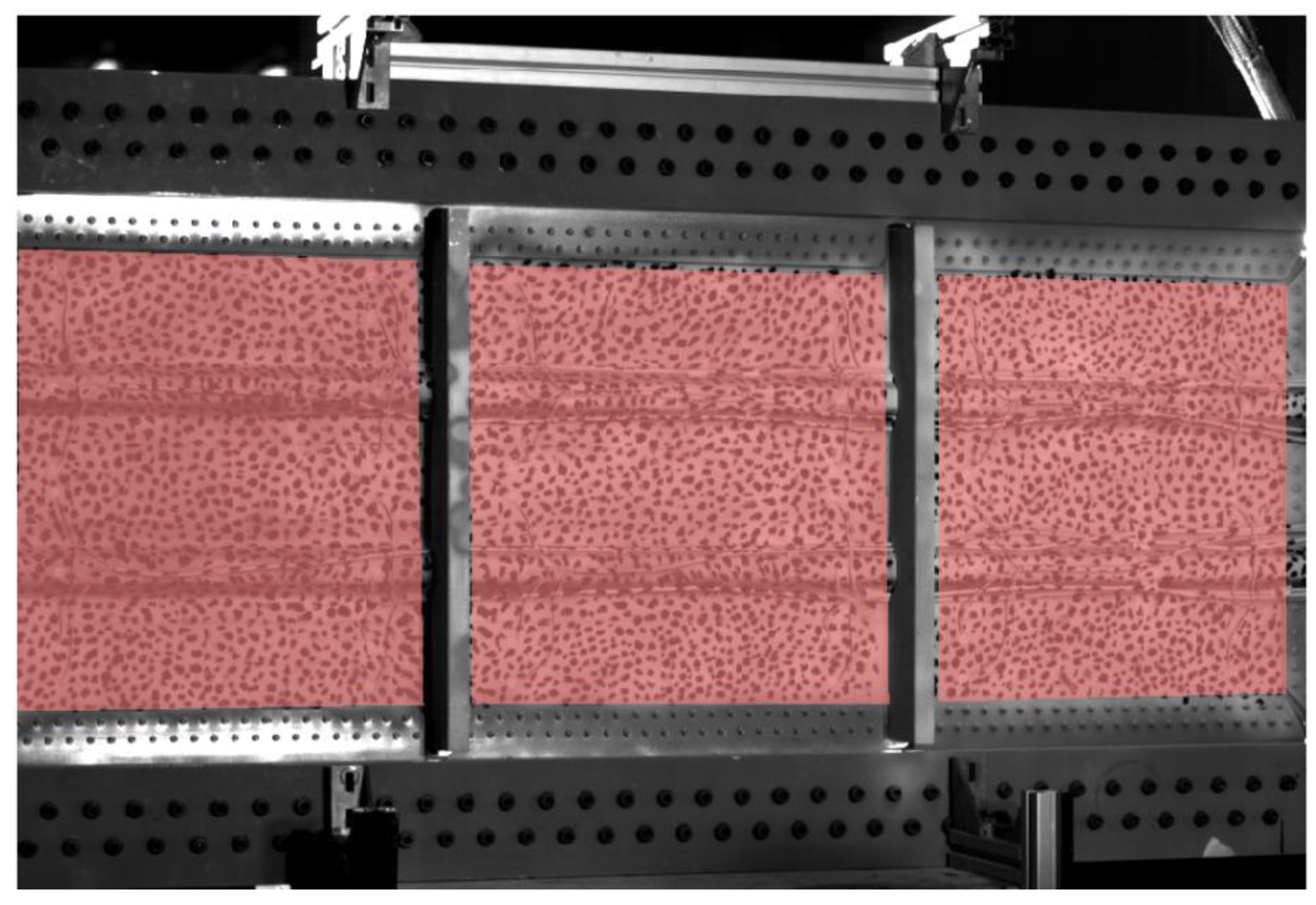
2.3. Time Series DIC Measurement
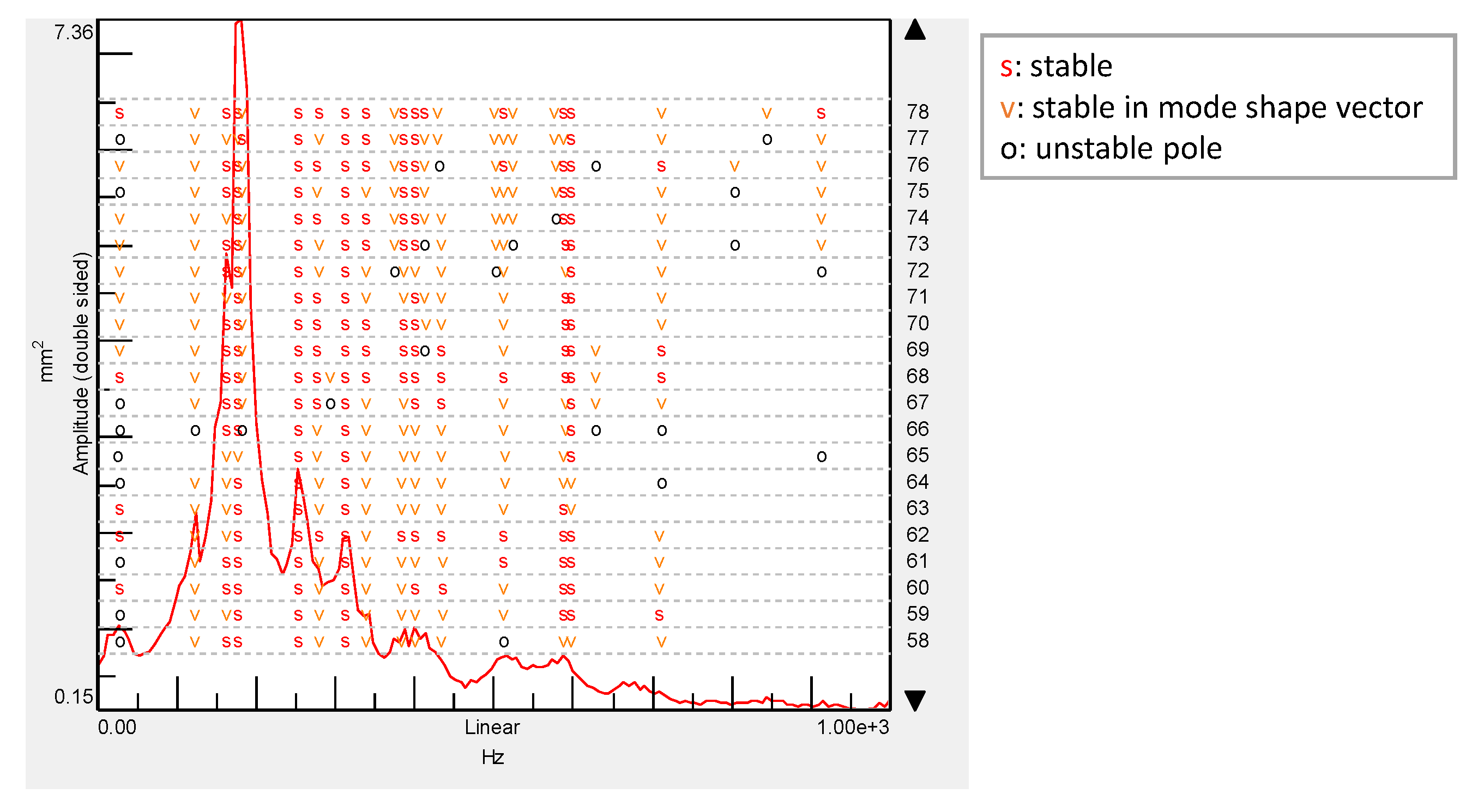
2.4. Operational Modal Analysis
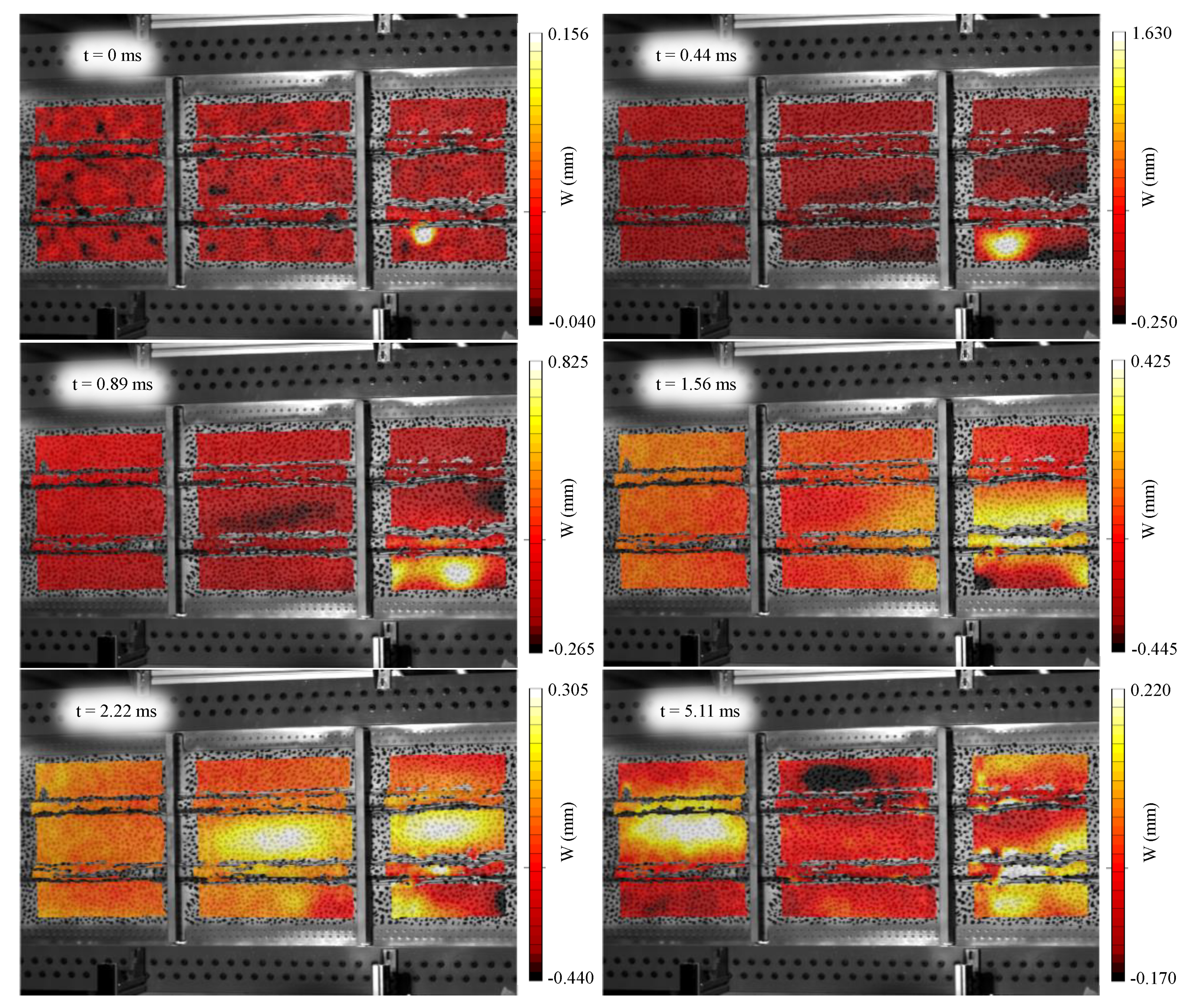
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Costanza, G.; Tata, M.E. Dynamic and static compressive behaviour of aluminium foam. In Proceedings of the Innovations in Structural Engineering and Construction; Xie, Y.M., Patnaikuni, I., Eds.; Taylor & Francis LTD: Melbourn, Australia, 2008; pp. 919–922. [Google Scholar]
- Zhou, Y.; Sun, Y.; Huang, T. SPH-FEM Design of Laminated Plies under Bird-Strike Impact. Aerospace 2019, 6, 112. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory, Practice, and Application, 2nd ed.; Roberts, J.B., Ed.; Research Studies Press LTD: Baldock, Hertfordshire, UK, 2000; ISBN 978-0863802188. [Google Scholar]
- McConnell, K.G.; Varoto, P.S. Vibration Testing: Theory and Practice, 2nd ed.; Wiley: Hoboken, NJ, USA, 2008; ISBN 9780471666516. [Google Scholar]
- Baqersad, J.; Poozesh, P.; Niezrecki, C.; Avitabile, P. Photogrammetry and optical methods in structural dynamics—A review. Mech. Syst. Signal Process. 2017, 86, 17–34. [Google Scholar] [CrossRef]
- Warren, C.; Niezrecki, C.; Avitabile, P.; Pingle, P. Comparison of FRF measurements and mode shapes determined using optically image based, laser, and accelerometer measurements. Mech. Syst. Signal Process. 2011, 25, 2191–2202. [Google Scholar] [CrossRef]
- Helfrick, M.N.; Niezrecki, C.; Avitabile, P.; Schmidt, T. 3D digital image correlation methods for full-field vibration measurement. Mech. Syst. Signal Process. 2011, 25, 917–927. [Google Scholar] [CrossRef]
- Reu, P.L.; Rohe, D.P.; Jacobs, L.D. Comparison of DIC and LDV for practical vibration and modal measurements. Mech. Syst. Signal Process. 2017, 86, 2–16. [Google Scholar] [CrossRef]
- Ha, N.S.; Jin, T.; Goo, N.S. Modal analysis of an artificial wing mimicking an Allomyrina dichotoma beetle’s hind wing for flapping-wing micro air vehicles by noncontact measurement techniques. Opt. Lasers Eng. 2013, 51, 560–570. [Google Scholar] [CrossRef]
- Ehrhardt, D.A.; Allen, M.S.; Yang, S.; Beberniss, T.J. Full-field linear and nonlinear measurements using Continuous-Scan Laser Doppler Vibrometry and high speed Three-Dimensional Digital Image Correlation. Mech. Syst. Signal Process. 2017, 86, 82–97. [Google Scholar] [CrossRef]
- Molina-Viedma, A.J.; Felipe-Sesé, L.; López-Alba, E.; Díaz, F.A. 3D mode shapes characterisation using phase-based motion magnification in large structures using stereoscopic DIC. Mech. Syst. Signal Process. 2018, 108, 140–155. [Google Scholar] [CrossRef]
- Srivastava, V.; Baqersad, J. An optical-based technique to obtain operating deflection shapes of structures with complex geometries. Mech. Syst. Signal Process. 2019, 128, 69–81. [Google Scholar] [CrossRef]
- Molina-Viedma, Á.J.; López-Alba, E.; Felipe-Sesé, L.; Díaz, F.A. Operational Deflection Shape Extraction from Broadband Events of an Aircraft Component Using 3D-DIC in Magnified Images. Shock. Vib. 2019, 2019, 1–9. [Google Scholar] [CrossRef]
- Berke, R.B.; Sebastian, C.M.; Chona, R.; Patterson, E.A.; Lambros, J. High Temperature Vibratory Response of Hastelloy-X: Stereo-DIC Measurements and Image Decomposition Analysis. Exp. Mech. 2016, 56, 231–243. [Google Scholar] [CrossRef]
- Warburton, J.R.; Lu, G.; Buss, T.M.; Docx, H.; Matveev, M.Y.; Jones, I.A. Digital Image Correlation Vibrometry with Low Speed Equipment. Exp. Mech. 2016, 56, 1219–1230. [Google Scholar] [CrossRef]
- Trebuňa, F.; Hagara, M. Experimental modal analysis performed by high-speed digital image correlation system. Measurement 2014, 50, 78–85. [Google Scholar] [CrossRef]
- Huňady, R.; Hagara, M. A new procedure of modal parameter estimation for high-speed digital image correlation. Mech. Syst. Signal Process. 2017, 93, 66–79. [Google Scholar] [CrossRef]
- Molina-Viedma, Á.J.; López-Alba, E.; Felipe-Sesé, L.; Díaz, F.A. Full-field modal analysis during base motion excitation using high-speed 3D digital image correlation. Meas. Sci. Technol. 2017, 28, 105402. [Google Scholar] [CrossRef]
- Patil, K.; Srivastava, V.; Baqersad, J. A multi-view optical technique to obtain mode shapes of structures. Measurement 2018, 122, 358–367. [Google Scholar] [CrossRef]
- Molina-Viedma, Á.J.; Felipe-Sesé, L.; López-Alba, E.; Díaz, F.A. Comparative of conventional and alternative Digital Image Correlation techniques for 3D modal characterisation. Measurement 2020, 151, 107101. [Google Scholar] [CrossRef]
- Huňady, R.; Pavelka, P.; Lengvarský, P. Vibration and modal analysis of a rotating disc using high-speed 3D digital image correlation. Mech. Syst. Signal Process. 2019, 121, 201–214. [Google Scholar] [CrossRef]
- Ha, N.S.; Vang, H.M.; Goo, N.S. Modal Analysis Using Digital Image Correlation Technique: An Application to Artificial Wing Mimicking Beetle’s Hind Wing. Exp. Mech. 2015, 55, 989–998. [Google Scholar] [CrossRef]
- Wu, R.; Zhang, D.; Yu, Q.; Jiang, Y.; Arola, D. Health monitoring of wind turbine blades in operation using three-dimensional digital image correlation. Mech. Syst. Signal Process. 2019, 130, 470–483. [Google Scholar] [CrossRef]
- Felipe-Sesé, L.; Díaz, F.A. Damage methodology approach on a composite panel based on a combination of Fringe Projection and 2D Digital Image Correlation. Mech. Syst. Signal Process. 2018, 101, 467–479. [Google Scholar] [CrossRef]
- Molina-Viedma, Á.J.; Pieczonka, L.; Mendrok, K.; López-Alba, E.; Díaz, F.A. Damage identification in frame structures using high-speed digital image correlation and local modal filtration. Struct. Control Heal. Monit. 2020, 27, e2586. [Google Scholar] [CrossRef]
- Molina-Viedma, Á.; López-Alba, E.; Felipe-Sesé, L.; Díaz, F.; Rodríguez-Ahlquist, J.; Iglesias-Vallejo, M. Modal Parameters Evaluation in a Full-Scale Aircraft Demonstrator under Different Environmental Conditions Using HS 3D-DIC. Materials 2018, 11, 230. [Google Scholar] [CrossRef] [PubMed]
- Felipe-Sesé, L.; Molina-Viedma, Á.J.; López-Alba, E.; Díaz, F.A. FP + DIC for low-cost 3D full-field experimental modal analysis in industrial components. Mech. Syst. Signal Process. 2019, 128, 329–339. [Google Scholar] [CrossRef]
- Amaro, A.M.; Reis, P.N.B.; de Moura, M.F.S.F.; Neto, M.A. Influence of multi-impacts on GFRP composites laminates. Compos. Part B Eng. 2013, 52, 93–99. [Google Scholar] [CrossRef]
- Horsfall, I.; Austin, S.J.; Bishop, W. Structural ballistic armour for transport aircraft. Mater. Des. 1999, 21, 19–25. [Google Scholar] [CrossRef]
- Hazell, P.J.; Roberson, C.J.; Moutinho, M. The design of mosaic armour: The influence of tile size on ballistic performance. Mater. Des. 2008, 29, 1497–1503. [Google Scholar] [CrossRef]
- Rodríguez-Millán, M.; Vaz-Romero, A.; Rusinek, A.; Rodríguez-Martínez, J.A.; Arias, A. Experimental Study on the Perforation Process of 5754-H111 and 6082-T6 Aluminium Plates Subjected to Normal Impact by Conical, Hemispherical and Blunt Projectiles. Exp. Mech. 2014, 54, 729–742. [Google Scholar] [CrossRef]
- Garcia-Gonzalez, D.; Rusinek, A.; Jankowiak, T.; Arias, A. Mechanical impact behavior of polyether–ether–ketone (PEEK). Compos. Struct. 2015, 124, 88–99. [Google Scholar] [CrossRef]
- Garcia-Gonzalez, D.; Rodriguez-Millan, M.; Rusinek, A.; Arias, A. Investigation of mechanical impact behavior of short carbon-fiber-reinforced PEEK composites. Compos. Struct. 2015, 133, 1116–1126. [Google Scholar] [CrossRef]
- Russell, B.P. Multi-hit ballistic damage characterisation of 304 stainless steel plates with finite elements. Mater. Des. 2014, 58, 252–264. [Google Scholar] [CrossRef]
- Deka, L.J.; Bartus, S.D.; Vaidya, U.K. Multi-site impact response of S2-glass/epoxy composite laminates. Compos. Sci. Technol. 2009, 69, 725–735. [Google Scholar] [CrossRef]
- Garzon-Hernandez, S.; Garcia-Gonzalez, D.; Arias, A. Multi-impact mechanical behaviour of short fibre reinforced composites. Compos. Struct. 2018, 202, 241–252. [Google Scholar] [CrossRef]
- Rizo-Patron, S.; Sirohi, J. Operational Modal Analysis of a Helicopter Rotor Blade Using Digital Image Correlation. Exp. Mech. 2017, 57, 367–375. [Google Scholar] [CrossRef]
- Chang, Y.-H.; Wang, W.; Chang, J.-Y.; Mottershead, J.E. Compressed sensing for OMA using full-field vibration images. Mech. Syst. Signal Process. 2019, 129, 394–406. [Google Scholar] [CrossRef]
- Chang, Y.-H.; Wang, W.; Siebert, T.; Chang, J.-Y.; Mottershead, J.E. Basis-updating for data compression of displacement maps from dynamic DIC measurements. Mech. Syst. Signal Process. 2019, 115, 405–417. [Google Scholar] [CrossRef]
- Uehara, D.; Sirohi, J. Full-field optical deformation measurement and operational modal analysis of a flexible rotor blade. Mech. Syst. Signal Process. 2019, 133, 106265. [Google Scholar] [CrossRef]
- Hu, Y.; Guo, W.; Zhu, W.; Xu, Y. Local damage detection of membranes based on Bayesian operational modal analysis and three-dimensional digital image correlation. Mech. Syst. Signal Process. 2019, 131, 633–648. [Google Scholar] [CrossRef]
- Green Regional Aircraft (GRA) | Clean Sky. Available online: https://www.cleansky.eu/green-regional-aircraft-gra (accessed on 16 February 2021).
- Díaz-Maroto, P.F.; Fernández-López, A.; García-Alonso, J.; Iglesias, M.; Güemes, A. Buckling detection of an omega-stiffened aircraft composite panel using distributed fibre optic sensors. Thin-Walled Struct. 2018, 132, 375–384. [Google Scholar] [CrossRef]
- Correlated Solutions Correlated Solutions. Available online: http://correlatedsolutions.com/ (accessed on 10 February 2021).
- Guillaume, P.; Verboven, P.; Vanlanduit, S.; Van Der Auweraer, H.; Peeters, B. A poly-reference implementation of the least-squares complex frequency-domain estimator. Proc. IMAC 2003, 21, 183–192. [Google Scholar]
- Cuadrado, M.; Pernas-Sánchez, J.; Artero-Guerrero, J.A.; Varas, D. Model updating of uncertain parameters of carbon/epoxy composite plates using digital image correlation for full-field vibration measurement. Measurement 2020, 159, 107783. [Google Scholar] [CrossRef]
- Allemang, R.J.; Brown, D.L. A correlation coefficient for modal vector analysis. In Proceedings of the First International Modal Analysis Conference, Orlando, FL, USA, 8–10 November 1982; pp. 110–116. [Google Scholar]
- Molina-Viedma, A.J.; Felipe-Sesé, L.; López-Alba, E.; Díaz, F. High frequency mode shapes characterisation using Digital Image Correlation and phase-based motion magnification. Mech. Syst. Signal Process. 2018, 102, 245–261. [Google Scholar] [CrossRef]
- Javh, J.; Slavič, J.; Boltežar, M. High frequency modal identification on noisy high-speed camera data. Mech. Syst. Signal Process. 2018, 98, 344–351. [Google Scholar] [CrossRef]





| Natural Frequency (Hz) | Damping Ratio (%) |
|---|---|
| 123.6 | 0.89 |
| 179.0 | 3.07 |
| 254.3 | 2.62 |
| 278.5 | 2.15 |
| 313.0 | 1.78 |
| 400.7 | 0.82 |
| 434.3 | 0.88 |
| 513.2 | 0.18 |
| 589.9 | 0.61 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Molina-Viedma, Á.; López-Alba, E.; Felipe-Sesé, L.; Díaz, F. Full-Field Operational Modal Analysis of an Aircraft Composite Panel from the Dynamic Response in Multi-Impact Test. Sensors 2021, 21, 1602. https://doi.org/10.3390/s21051602
Molina-Viedma Á, López-Alba E, Felipe-Sesé L, Díaz F. Full-Field Operational Modal Analysis of an Aircraft Composite Panel from the Dynamic Response in Multi-Impact Test. Sensors. 2021; 21(5):1602. https://doi.org/10.3390/s21051602
Chicago/Turabian StyleMolina-Viedma, Ángel, Elías López-Alba, Luis Felipe-Sesé, and Francisco Díaz. 2021. "Full-Field Operational Modal Analysis of an Aircraft Composite Panel from the Dynamic Response in Multi-Impact Test" Sensors 21, no. 5: 1602. https://doi.org/10.3390/s21051602
APA StyleMolina-Viedma, Á., López-Alba, E., Felipe-Sesé, L., & Díaz, F. (2021). Full-Field Operational Modal Analysis of an Aircraft Composite Panel from the Dynamic Response in Multi-Impact Test. Sensors, 21(5), 1602. https://doi.org/10.3390/s21051602







