Measurement of Flow Fluctuation in the Flow Standard Facility Based on Singular Value Decomposition
Abstract
1. Introduction
2. Flow Stability of the Flow Standard Facility
3. Principle of Proposed Measuring Method Based on SVD
4. Simulation Experiment
4.1. Generation and Pre-Processing of Simulated Flow Fluctuation Signal
4.2. Parameters Setting of Measuring Method Based on SVD
4.3. Simulation Results
5. Experiment and Result
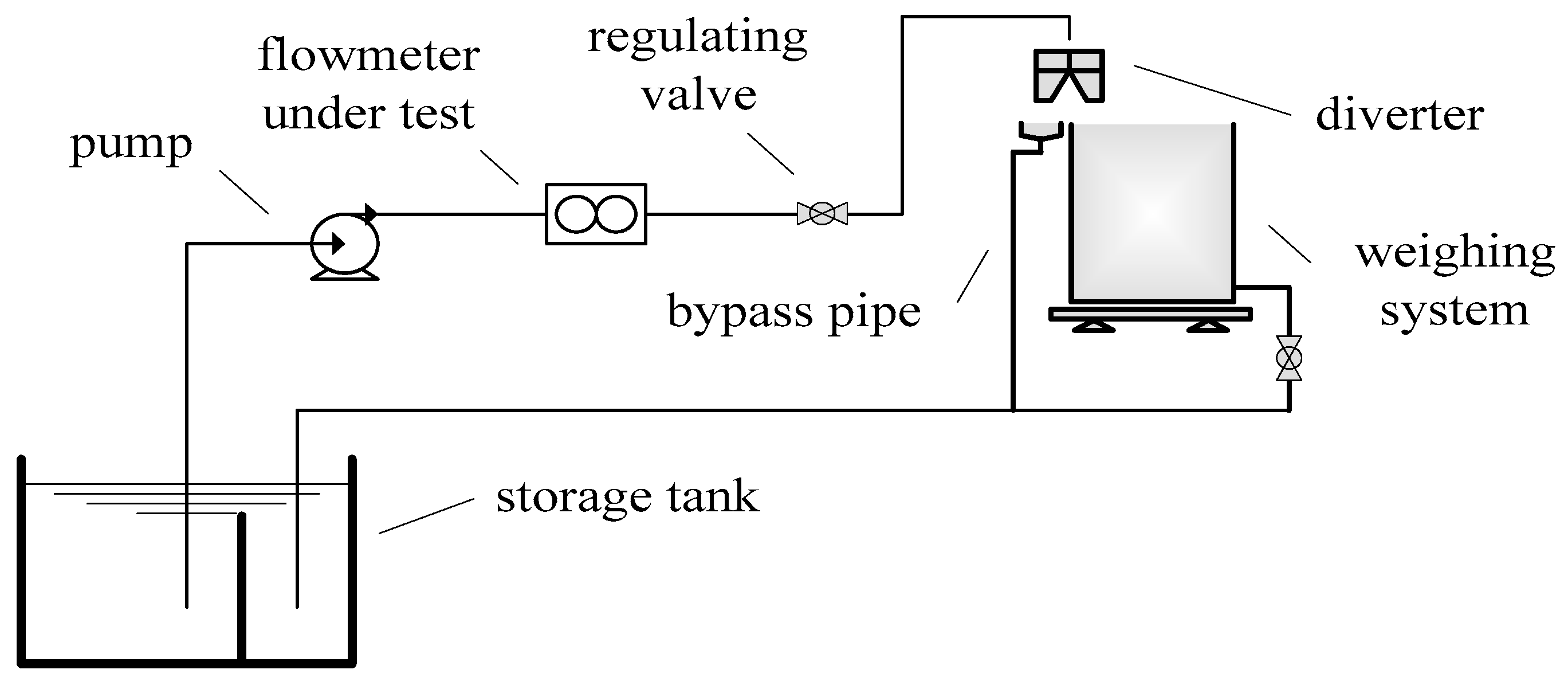
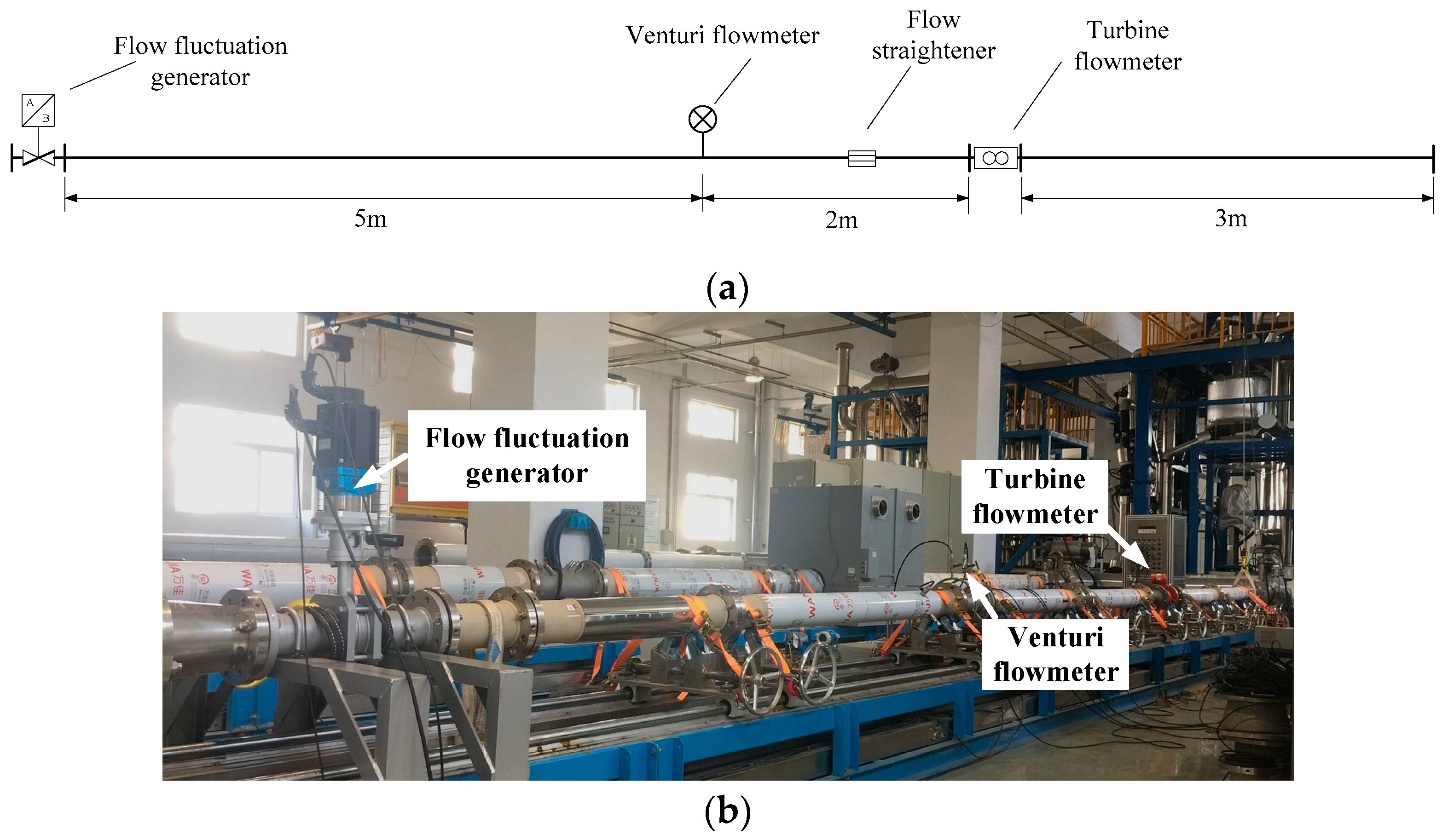
5.1. Experiment Setup
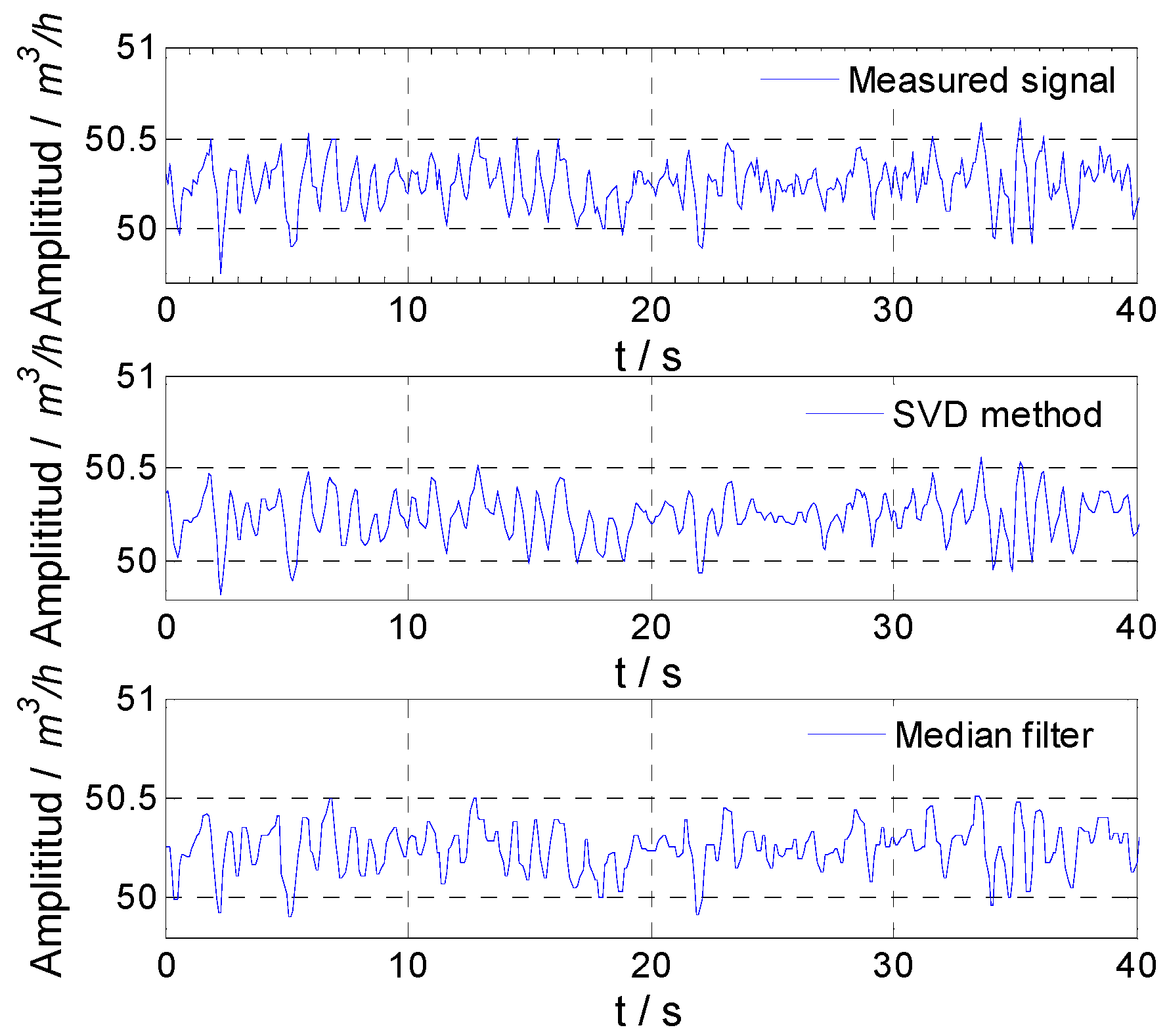
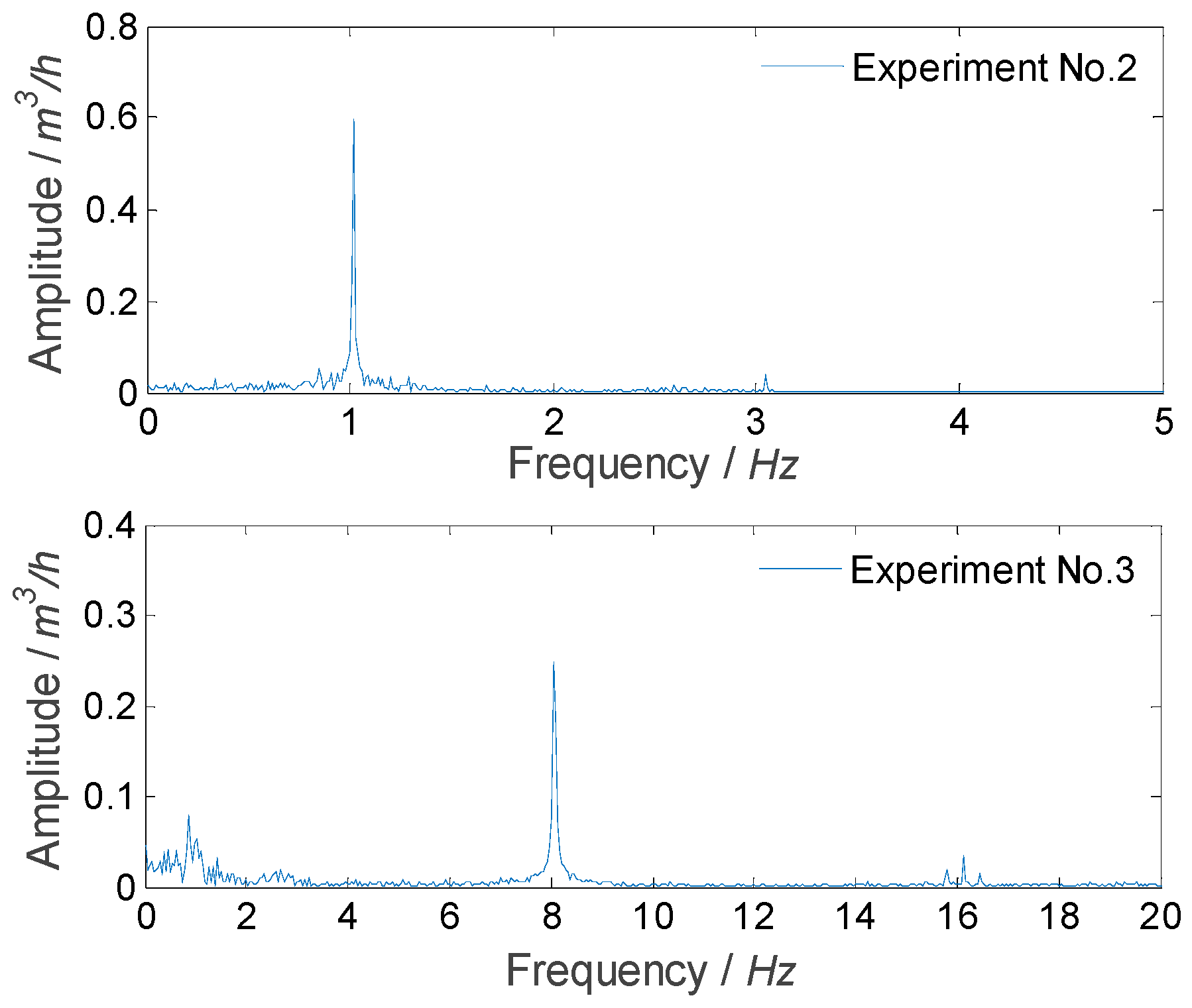
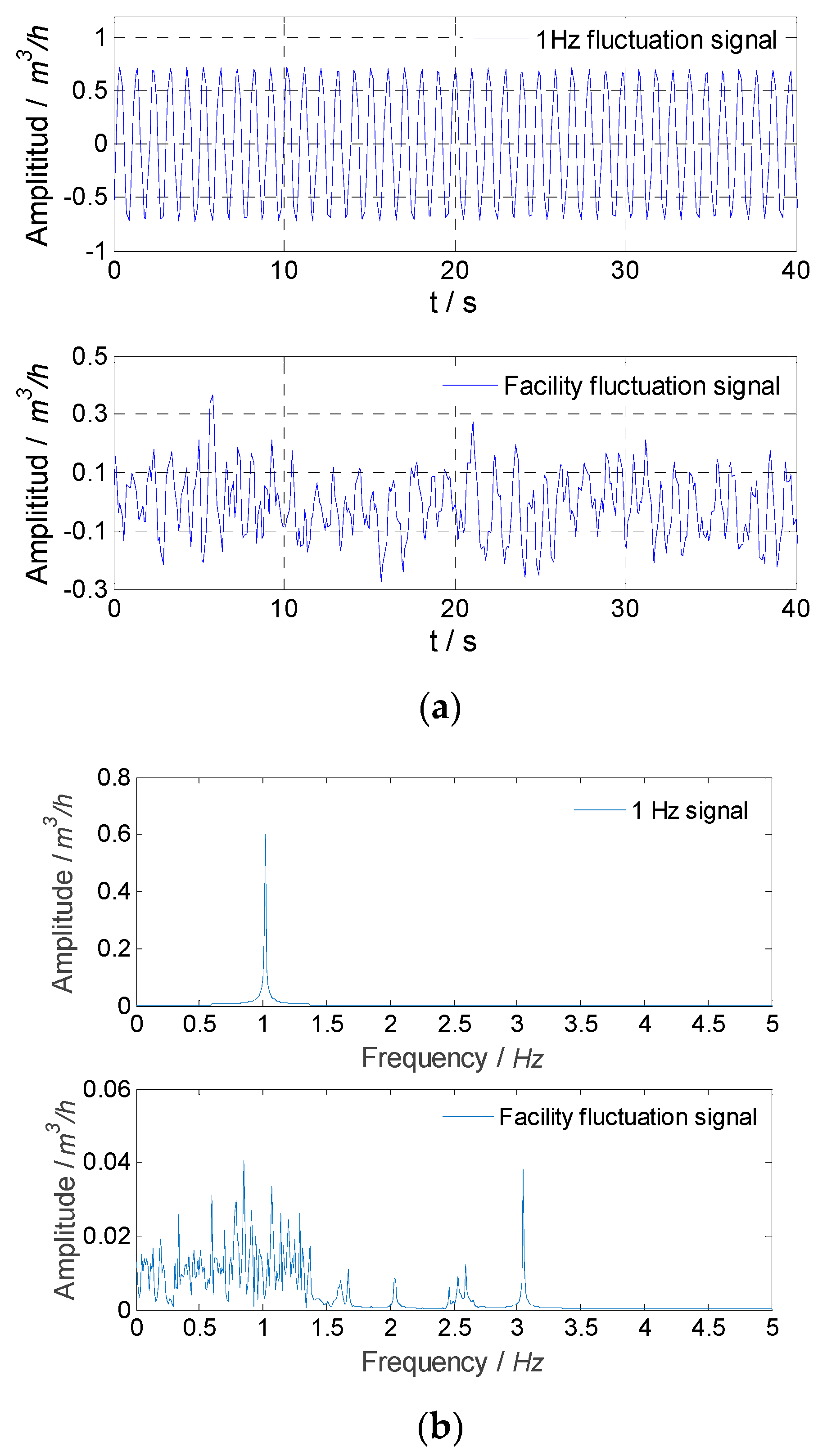
5.2. Experiment Result
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jaiswal, S.K.; Yadav, S.; Agarwal, R. Design and development of a novel water flow measurement system. Measurement 2017, 105, 120–129. [Google Scholar] [CrossRef]
- Meng, T.; Fan, S.; Wang, C.; Shi, H. Influence analysis of fluctuation parameters on flow stability based on uncertainty method. Rev. Sci. Instrum. 2018, 89, 055005. [Google Scholar] [CrossRef] [PubMed]
- Engel, R.; Baade, H.J. Quantifying impacts on the measurement uncertainty in flow calibration arising from dynamic flow effects. Flow Meas. Instrum. 2015, 44, 51–60. [Google Scholar] [CrossRef]
- Berrebi, J.; Deventer, J.V.; Delsing, J. Reducing the flow measurement error caused by pulsations in flows. Flow Meas. Instrum. 2004, 15, 311–315. [Google Scholar] [CrossRef][Green Version]
- Meng, T.; Fan, S.; Wang, C.; Shi, H.; Li, X. Flow stability evaluation method based on flow-pressure correlation. Flow Meas. Instrum. 2018, 64, 155–163. [Google Scholar] [CrossRef]
- Reis, M.N.; Hanriot, S. Incompressible pulsating flow for low Reynolds numbers in orifice plates. Flow Meas. Instrum. 2017, 54, 146–157. [Google Scholar] [CrossRef]
- Blythman, R.; Persoons, T.; Jeffers, N.; Nolan, K.P.; Murray, D.B. Localised dynamics of laminar pulsatile flow in a rectangular channel. Int. J. Heat Fluid Flow 2017, 66, 8–17. [Google Scholar] [CrossRef]
- International Organization for Standardization. ISO/TR 3313: 2018. Measurement of Fluid Flow in Closed Conduits—Guidelines on the Effects of Flow Pulsations on Flow-Measurement Instruments; International Organization for Standardization: Geneva, Switzerland, 2018. [Google Scholar]
- Berrebi, J.; Martinsson, P.E.; Willatzen, M.; Delsing, J. Ultrasonic flow metering errors due to pulsating flow. Flow Meas. Instrum. 2004, 15, 179–185. [Google Scholar] [CrossRef][Green Version]
- Zhu, Y. Study on the measurement error of differential pressure flowmeter in pulsating flow. Chin. J. Sci. Instrum. 2006, 27, 894–897. [Google Scholar]
- Gajan, P.; Mottram, R.C.; Hebrard, P.; Andriamihafy, H.; Platet, B. The influence of pulsating flows on orifice plate flowmeters. Flow Meas. Instrum. 1992, 3, 118–129. [Google Scholar] [CrossRef]
- International Organization for Standardization. ISO 9368-1: 1990. Measurement of Liquid Flow in Closed Conduits by the Weighing Method-Procedures for Checking Installations—Part 1: Static Weighing Systems; International Organization for Standardization: Geneva, Switzerland, 1990. [Google Scholar]
- Meng, T.; Shi, H.; Wang, C.; Wu, B. Application of principal component analysis in measurement of flow fluctuation. Measurement 2020, 173, 108503. [Google Scholar] [CrossRef]
- Guo, H.X.; Xie, K. An improved method of adaptive median filter. J. Image Graph. 2007, 12, 1185–1188. [Google Scholar]
- Liu, F.; Zhang, G.Q. Application of wavelet transform and FK algorithm in filtering. Oil Geophys. Prospect. 1996, 76, 2078–2088. [Google Scholar]
- Ke, X.; Xu, J.W.; Yang, D. Application of wavelet transform in signal filtering. J. Univ. Sci. Technol. Beijing 1997, 19, 382–389. [Google Scholar]
- Loizou, P.C. Speech Enhancement: Theory and Practice; CRC Press, Inc.: Boca Raton, FL, USA, 2007. [Google Scholar]
- Dendrinos, M.; Bakamidis, S.; Carayannis, G. Speech enhancement from noise: A regenerative approach. Speech Commun. 1991, 10, 45–57. [Google Scholar] [CrossRef]
- De Moor, B. The singular value decomposition and long and short spaces of noisy matrices. IEEE Trans. Signal Process. 1993, 41, 2826–2838. [Google Scholar] [CrossRef]
- Sun, L.; Dang, S.; Zhang, T.; Liu, Y. Experiment on the influence of flow stability on flowmeter measuring performance. J. Tianjin Univ. 2018, 51, 422–432. [Google Scholar]
- Li, J.H.; Su, Y.X. Uncertainty of the commutator and stability measurement in liquid flowmeter standard system. Acta Metrol. Sin. 2008, 5, 437–440. [Google Scholar]
- China Institute of Metrology. JJG 164. Liquid Flow Standard Device; China Institute of Metrology: Hangzhou, China, 2000. [Google Scholar]
- Zhou, X.Y.; Gao, C.W.; Cao, J.H. Principal component analysis method and its application in data noise reducing. Ordnance Ind. Autom. 2014, 33, 55–58. [Google Scholar]
- Duan, H.M. Standard Device and Standard Meter Flow Standard Device for Liquid Flow; China Metrology Press: Beijing, China, 2004. [Google Scholar]
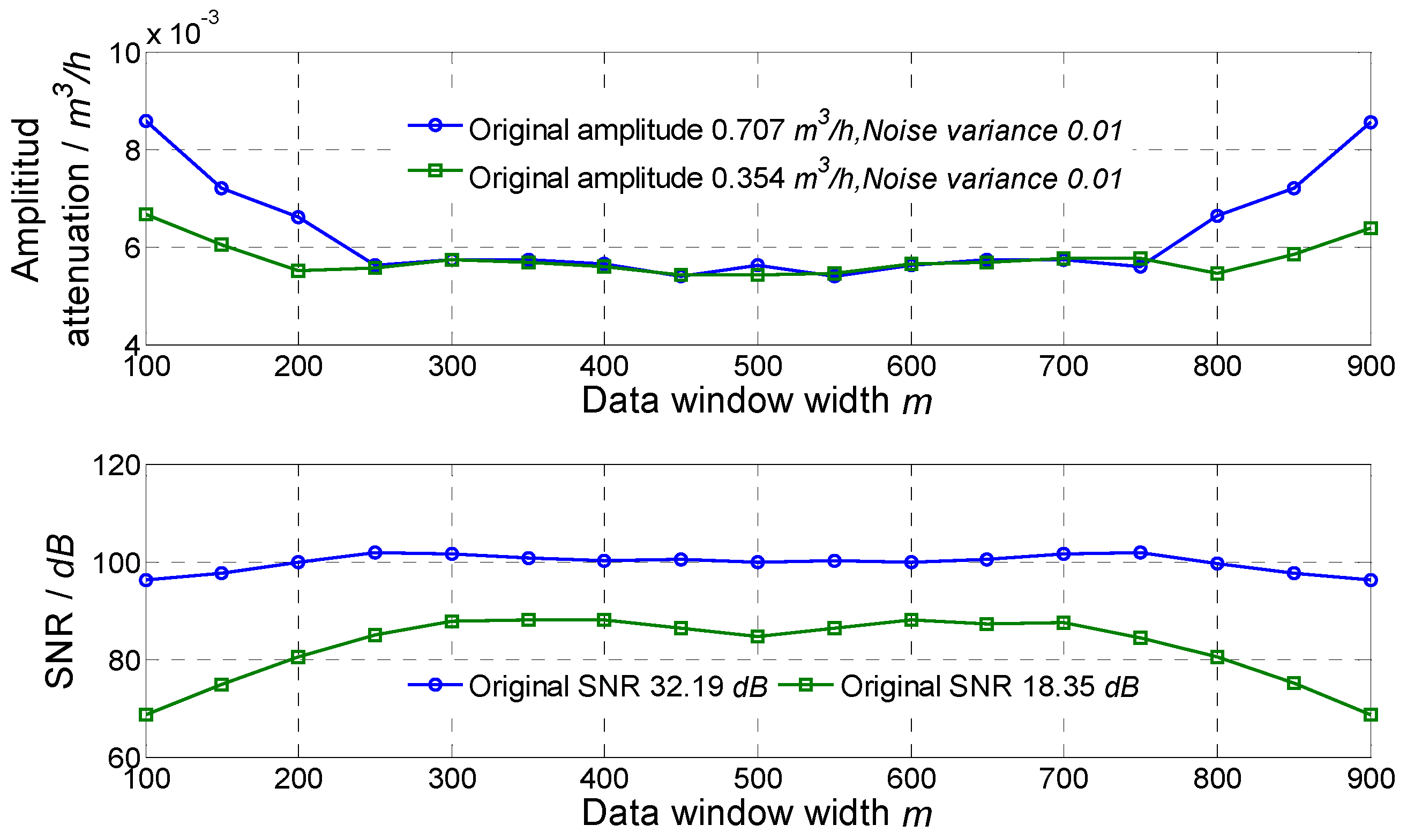





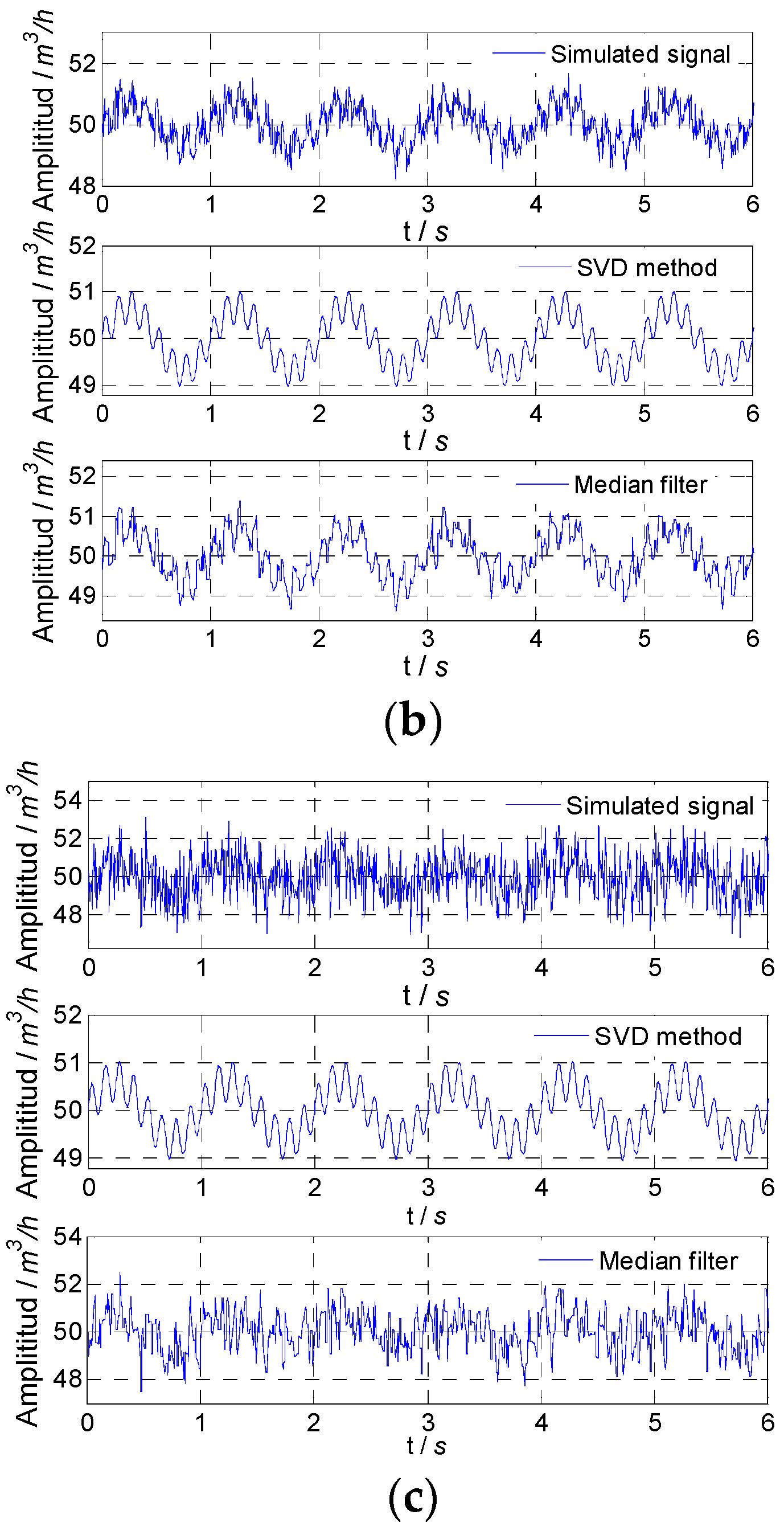
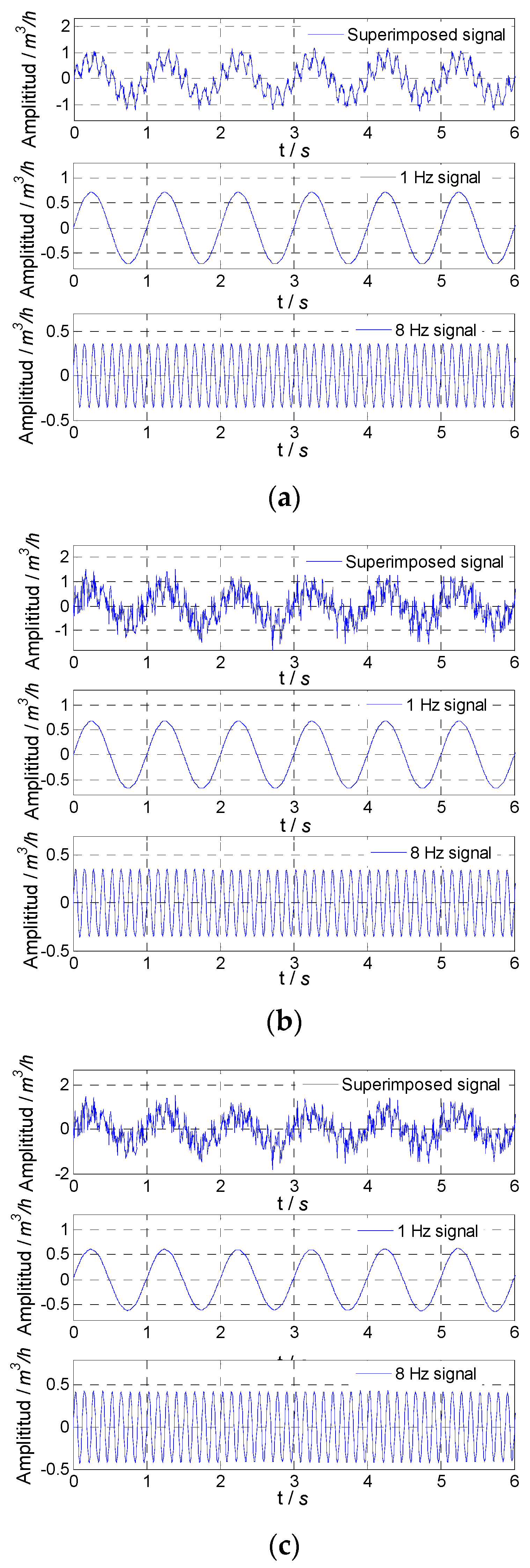





| Noise | Variance | 0.01 | 0.1 | 1 |
| SNR | 32.19 dB | 9.16 dB | −13.86 dB | |
| SVD Method | Attenuation | 5.40 × 10−3 m3/h | 2.52 × 10−2 m3/h | 2.50 × 10−2 m3/h |
| SNR | 100.53 dB | 77.26 dB | 66.90 dB | |
| Median Filter | Attenuation | 5.61 × 10−2 m3/h | 1.50 × 10−1 m3/h | 4.99 × 10−1 m3/h |
| SNR | 47.62 dB | 23.15 dB | −20.14 dB |
| Noise | Variance | 0.01 | 0.1 | 1 |
| SNR | 18.32 dB | −4.70 dB | −27.73 dB | |
| SVD Method | Attenuation | 5.41 × 10−3 m3/h | 2.54 × 10−2 m3/h | 2.87 × 10−2 m3/h |
| SNR | 86.42 dB | 62.40 dB | 48.49 dB | |
| Median Filter | Attenuation | 1.15 × 10−2 m3/h | 2.49 × 10−2 m3/h | 3.64 × 10−2 m3/h |
| SNR | 25.37 dB | 3.52 dB | −21.06 dB |
| Noise | Variance | 0.01 | 0.1 | 1 |
| SNR | 34.42 dB | 11.39 dB | −11.63 dB | |
| SVD Method | Attenuation | 5.22 × 10−3 m3/h | −6.41 × 10−3 m3/h | 7.66 × 10−2 m3/h |
| SNR | 119.18 dB | 103.64 dB | 46.56 dB | |
| Median Filter | Attenuation | 5.71 × 10−2 m3/h | 1.58 × 10−1 m3/h | 4.78 × 10−1 m3/h |
| SNR | 49.91 dB | 26.79 dB | −9.51 dB |
| Noise | Variance | 0.01 | 0.1 | 1 |
| SNR | 34.42 dB | 11.39 dB | −11.63 dB | |
| 1 Hz Signal | Attenuation | −1.49 × 10−3 m3/h | 1.85 × 10−2 m3/h | 5.97 × 10−2 m3/h |
| SNR | 102.44 dB | 69.03 dB | 52.72 dB | |
| 8 Hz Signal | Attenuation | 1.10 × 10−3 m3/h | 3.15 × 10−3 m3/h | −1.45 × 10−2 m3/h |
| SNR | 96.16 dB | 82.17 dB | 36.27 dB |
| Signal | Noise Variance | 0 | 0.01 | 0.1 | 1 |
| 1 HZ Signal | Simulated fluctuation | 1.00% | 1.03% | 1.22% | 2.23% |
| Median filter | 1.00% | 1.09% | 1.64% | ||
| SVD method | 1.01% | 1.03% | 1.01% | ||
| 8 HZ Signal | Simulated fluctuation | 0.50% | 0.54% | 0.83% | 2.04% |
| Median filter | 0.51% | 0.67% | 1.40% | ||
| SVD method | 0.51% | 0.53% | 0.51% |
| Noise Variance | 0 | 0.01 | 0.1 | 1 |
| Simulated Fluctuation | 1.12% | 1.14% | 1.27% | 2.28% |
| Median Filter | 1.12% | 1.17% | 1.69% | |
| SVD Method | 1.12% | 1.09% | 1.06% | |
| 1 Hz Signal | 1.00% | 1.00% | 0.97% | 0.94% |
| 8 Hz Signal | 0.50% | 0.50% | 0.50% | 0.49% |
| Experiment No. | 1 | 2 | 3 |
|---|---|---|---|
| Direct calculation | 0.25% | 1.01% | 0.57% |
| Median filter | 0.22% | 0.95% | 0.47% |
| SVD method | 0.22% | 0.99% | 0.53% |
| Sinusoidal fluctuation | / | 0.97% | 0.48% |
| Facility fluctuation signal | / | 0.22% | 0.22% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, T.; Wei, H.; Gao, F.; Shi, H. Measurement of Flow Fluctuation in the Flow Standard Facility Based on Singular Value Decomposition. Sensors 2021, 21, 6850. https://doi.org/10.3390/s21206850
Meng T, Wei H, Gao F, Shi H. Measurement of Flow Fluctuation in the Flow Standard Facility Based on Singular Value Decomposition. Sensors. 2021; 21(20):6850. https://doi.org/10.3390/s21206850
Chicago/Turabian StyleMeng, Tao, Huanchang Wei, Feng Gao, and Huichao Shi. 2021. "Measurement of Flow Fluctuation in the Flow Standard Facility Based on Singular Value Decomposition" Sensors 21, no. 20: 6850. https://doi.org/10.3390/s21206850
APA StyleMeng, T., Wei, H., Gao, F., & Shi, H. (2021). Measurement of Flow Fluctuation in the Flow Standard Facility Based on Singular Value Decomposition. Sensors, 21(20), 6850. https://doi.org/10.3390/s21206850






