A Novel Gold Film-Coated V-Shape Dual-Core Photonic Crystal Fiber Polarization Beam Splitter Covering the E + S + C + L + U Band
Abstract
1. Introduction
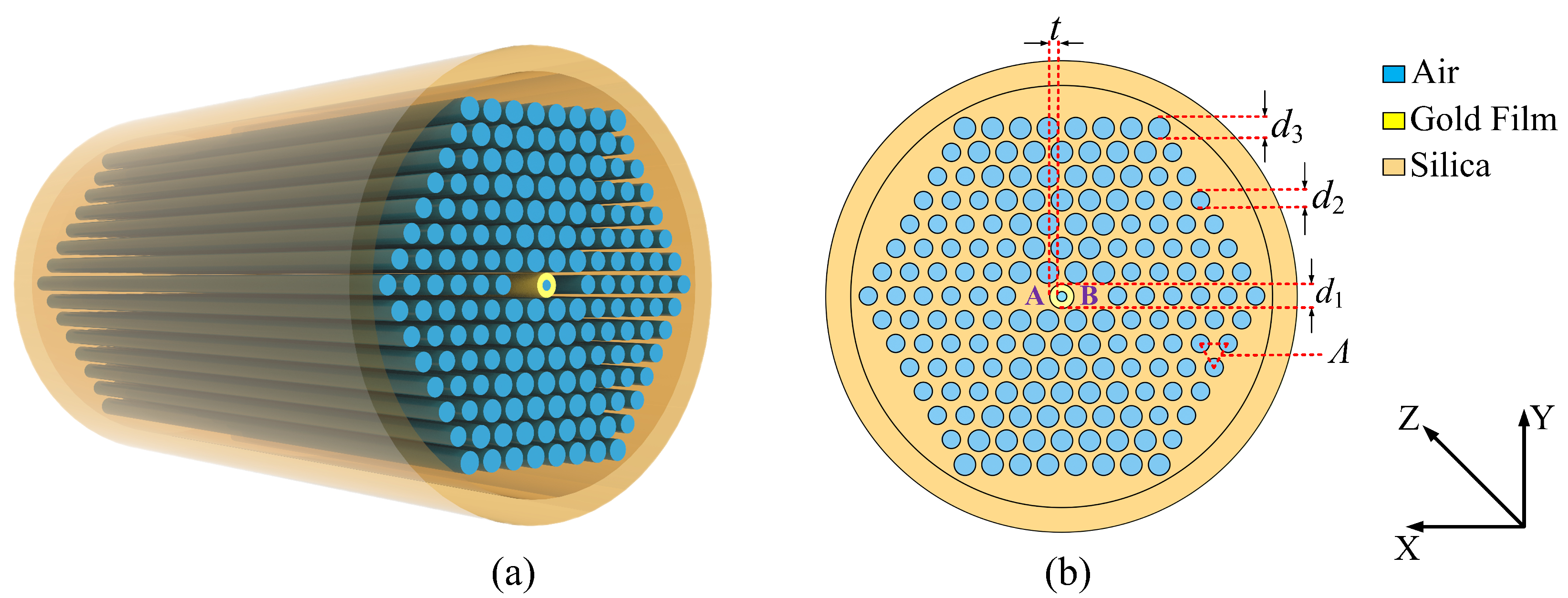
2. Design of the V-DC-PCF PBS
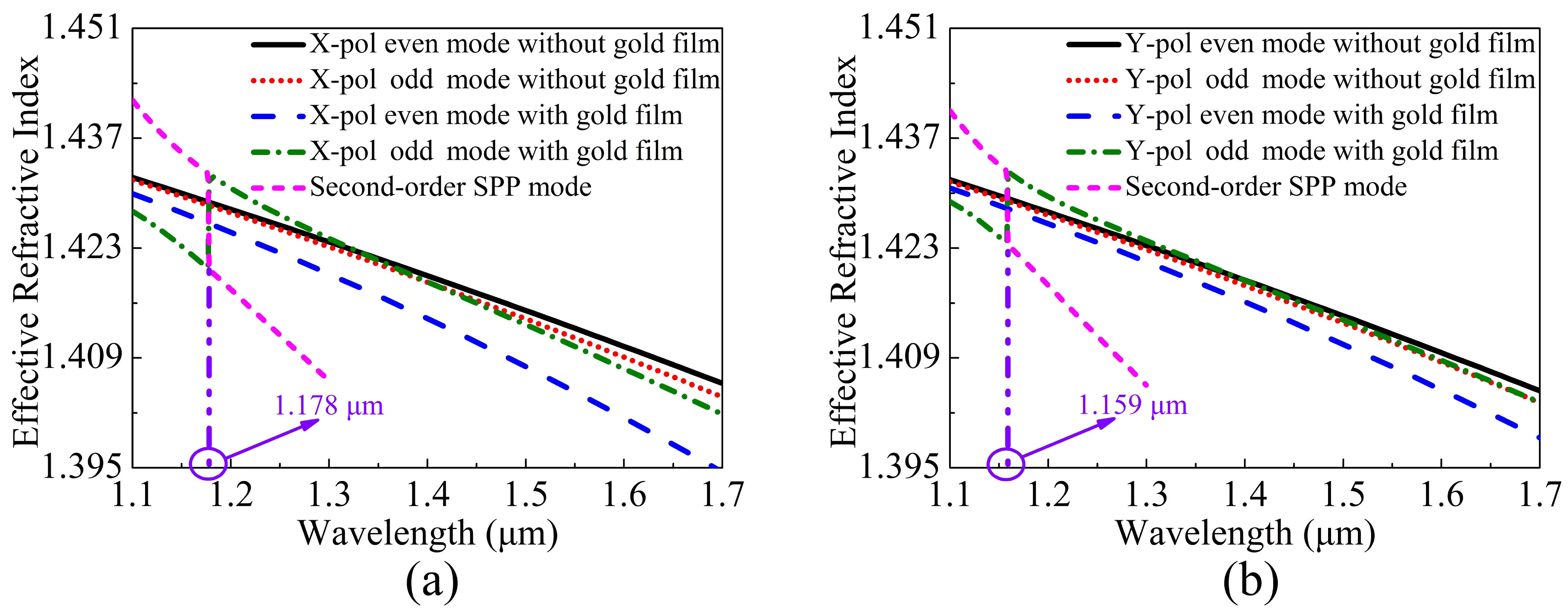
3. Simulation Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Knight, J.C.; Birks, T.A.; Russell, P.S.J.; Atkin, D.M. All-silica single-mode optical fiber with photonic crystal cladding. Opt. Lett. 1996, 21, 1547–1549. [Google Scholar] [CrossRef]
- Liu, Q.; Ma, Z.; Wu, Q.; Wang, W.L. The biochemical sensor based on liquid-core photonic crystal fiber filled with gold, silver and aluminum. Opt. Laser Technol. 2020, 130, 106363. [Google Scholar] [CrossRef]
- Úsuga-Restrepo, J.E.; Guimarães, W.M.; Franco, M.A.R. All-fiber circular polarization beam splitter based on helically twisted twincore photonic crystal fiber coupler. Opt. Laser Technol. 2020, 58, 102285. [Google Scholar]
- Feng, X.X.; Du, H.J.; Li, S.G.; Zhang, Y.N.; Liu, Q.; Gao, X.Y. A polarization filter based on photonic crystal fiber with asymmetry around gold-coated holes. Plasmonics 2018, 13, 1271–1275. [Google Scholar] [CrossRef]
- Yu, R.W.; Chen, Y.X.; Shui, L.L.; Xiao, L.M. Hollow-core photonic crystal fiber gas sensing. Sensors 2020, 20, 2996. [Google Scholar] [CrossRef]
- Yao, C.Y.; Xiao, L.M.; Gao, S.F.; Wang, Y.Y.; Wang, P.; Kand, R.F.; Jine, W.; Rena, W. Sub-ppm CO detection in a sub-meter-long hollow-core negative curvature fiber using absorption spectroscopy at 2.3 μm. Sens. Actuat. B Chem. 2020, 303, 127238. [Google Scholar] [CrossRef]
- Wang, J.; Pei, L.; Wang, J.S.; Ruan, Z.L.; Zheng, J.J.; Li, J.; Ning, T.G. Magnetic field and temperature dual-parameter sensor based on magnetic fluid materials filled photonic crystal fiber. Opt. Express 2020, 28, 1456–1471. [Google Scholar] [CrossRef]
- Shakya, A.K.; Singh, S. Design of dual polarized tetra core PCF based plasmonic RI sensor for visible-IR spectrum. Opt. Commun. 2021, 478, 126372. [Google Scholar] [CrossRef]
- Chu, L.H.; Liu, M.; Shum, P.; Fu, Y.B. Simultaneous achievement of an ultrashort length and a high extinction ratio polarization splitter based on the dual-core photonic crystal fiber with Ge20Sb15Se65 glass. Appl. Opt. 2019, 58, 7892–7896. [Google Scholar] [CrossRef]
- Rahman, M.M.; Khaleque, A.; Rahman, M.T.; Rabbi, F. Gold-coated photonic crystal fiber based polarization filter for dual communication windows. Opt. Commun. 2020, 461, 125293. [Google Scholar] [CrossRef]
- Reyes-Vera, E.; Úsuga-Restrepo, J.; Gomez, F.; Gómez-Cardona, N. Novel multiband polarization beam splitter based on a dual-core transversally chirped microstructured optical fiber. Third Int. Conf. Appl. Opt. Photonics 2017, 10453, 1045334. [Google Scholar]
- Jiang, L.H.; Zheng, Y.; Hou, L.T.; Zheng, K.; Peng, J.Y.; Zhao, X.T. An ultrabraoadband polarization splitter based on square-lattice dualcore photonic crystal fiber with a gold wire. Opt. Commun. 2015, 351, 50–56. [Google Scholar] [CrossRef]
- Khaleque, A.; Hattori, H.T. Ultra-broadband and compact polarization splitter based on gold filled dual-core photonic crystal fiber. J. Appl. Phys. 2015, 118, 143101. [Google Scholar] [CrossRef]
- Jimenez-Durango, C.; Reyes-Vera, E.; Gomez-Cardona, N. Ultra-short polarization beam splitter to operate in two communication bands based on a gold-filled dual-core photonic crystal fiber. In Latin America Optics and Photonics Conference; OSA Technical Digest (Optical Society of America): Washington, DC, USA, 2018. [Google Scholar]
- Chen, H.L.; Li, S.G.; An, G.W.; Li, J.S.; Fan, Z.K.; Han, Y. Polarization splitter based on d-shaped dual-core photonic crystal fibers with gold film. Plasmonics 2015, 10, 57–61. [Google Scholar] [CrossRef]
- Sun, B.; Chen, M.Y.; Zhang, Y.K.; Zhou, J. Polarization-dependent coupling characteristics of metal-wire filled dual-core photonic crystal fiber. Opt. Quant. Electron. 2015, 47, 441–451. [Google Scholar] [CrossRef]
- An, G.W.; Li, S.G.; Yan, X.; Yuan, Z.Y.; Zhang, X.N. High-birefringence photonic crystal fiber polarization filter based on surface plasmon resonance. Appl. Opt. 2016, 55, 1262–1266. [Google Scholar] [CrossRef]
- Wang, X.Y.; Yan, X.; Li, S.G.; Zhang, X.N. Tunable surface plasmon resonance polarization beam splitter based on dual-core photonic crystal fiber with magnetic fluid. Opt. Quant. Electron. 2017, 49, 368. [Google Scholar] [CrossRef]
- Jiang, H.M.; Wang, E.L.; Zhang, J.; Hu, L.; Mao, Q.P.; Li, Q.; Xie, K. Polarization splitter based on dual-core photonic crystal fiber. Opt. Express 2014, 22, 30461–30466. [Google Scholar] [CrossRef]
- Zi, J.C.; Li, S.G.; An, G.W.; Fan, Z.K. Short-length polarization splitter based on dual-core photonic crystal fiber with hexagonal lattice. Opt. Commun. 2016, 363, 80–84. [Google Scholar] [CrossRef]
- Wang, E.L.; Jiang, H.M.; Xie, K.; Chen, C.; Hu, Z.J. Olarization splitter based on dual core liquid crystal-filled holey fiber. J. Appl. Phys. 2016, 120, 114501. [Google Scholar] [CrossRef]
- He, F.T.; Shi, W.J.; Hui, Z.Q.; Zhan, F.; Zhang, Y.K. A dual-core PCF polarization splitter with five elliptical air holes based on tellurite glass. Opt. Quant. Electron. 2017, 49, 363. [Google Scholar] [CrossRef]
- Wang, J.S.; Pei, L.; Weng, S.J.; Wu, L.Y.; Ning, T.G.; Li, J. Ultrashort polarization beam splitter based on liquid-filled dual-core photonic crystal fiber. Appl. Opt. 2018, 57, 3847–3852. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.Y.; Li, S.G.; Liu, Q.; Fan, Z.K.; Wang, G.Y.; Zhao, Y.Y. High-extinction ratio and short-length polarization splitter based on microstructured optical fiber with tellurite glass. Opt. Mater. 2017, 66, 542–546. [Google Scholar] [CrossRef]
- Lou, J.B.; Cheng, T.L.; Li, S.G. Ultra-short polarization beam splitter with square lattice and gold film based on dual-core photonic crystal fiber. Optik 2019, 179, 128–134. [Google Scholar] [CrossRef]
- Xiao, L.M.; Birks, T.A.; Loh, W.H. Hydrophobic photonic crystal fibers. Opt. Lett. 2011, 36, 4662–4664. [Google Scholar] [CrossRef]
- Zhao, T.T.; Lou, S.Q.; Wang, X.; Zhang, W.; Wang, Y.L. Simultaneous measurement of curvature, strain and temperature using a twin-core photonic crystal fiber-based sensor. Sensors 2018, 18, 2145. [Google Scholar] [CrossRef]
- Wiegandt, F.; Anderson, P.N.; Yu, F.; Treacher, D.J.; Lloyd, D.T.; Mosley, P.J.; Hooler, S.M.; Walmsley, I.A. Quasi-phase-matched high-harmonic generation in gas-filled hollow-core photonic crystal fiber. Optica 2019, 6, 442–447. [Google Scholar] [CrossRef]
- Mridha, M.K.; Novoa, D.; Hosseini, P.; Russel, P.S.J. Thresholdless deep and vacuum ultraviolet Raman frequency conversion in hydrogen-filled photonic crystal fiber. Optica 2019, 6, 731–734. [Google Scholar] [CrossRef]
- Davtyan, S.; Chen, Y.; Frosz, M.H.; Russell, P.S.J.; Novoa, D. Robust excitation and raman conversion of guided vortices in a chiral gas-filled photonic crystal fiber. Opt. Lett. 2020, 45, 1766–1769. [Google Scholar] [CrossRef]
- Fujisawa, T.; Saitoh, K. Geometric-phase-induced arbitrary polarization and orbital angular momentum generation in helically twisted birefringent photonic crystal fiber. Photonics Res. 2020, 8, 1278–1288. [Google Scholar] [CrossRef]
- Ning, D.; Lothar, W.; Markus, A.S.; Nicolai, G.; Russell, P.S.J. High index-contrast all-solid photonic crystal fibers by pressure-assisted melt infiltration of silica matrices. J. Non-Cryst. Solids 2010, 356, 1829–1836. [Google Scholar]
- Knight, J.C.; Broeng, J.; Birks, T.A.; Russell, P.S.J. Photonic band gap guidance in optical fibers. Science 1998, 282, 1476–1478. [Google Scholar] [CrossRef] [PubMed]
- Feng, X.; Mairaj, A.K.; Hewak, D.W.; Monro, T.M. Nonsilica glasses for holey fibers. J. Lightw. Technol. 2005, 23, 2046–2054. [Google Scholar] [CrossRef]
- Li, Y.; Itoh, K.; Watanabe, W.; Yamada, K.; Kuroda, D.; Nishii, J.J.; Jiang, Y.Y. Three-dimensional hole drilling of silica glass from the rear surface with femtosecond laser pulses. Opt. Lett. 2001, 26, 1912–1914. [Google Scholar] [CrossRef]
- Cook, K.; Canning, J.; Leon-saval, S.; Reid, Z.; Hossainmd, A.; Comatt, J.E.; Luo, Y.H.; Peng, G.D. Air-structured optical fiber drawn from a 3D-printed preform. Opt. Lett. 2015, 40, 3966–3969. [Google Scholar] [CrossRef]
- Urich, A.; Maier, R.R.J.; Yu, F.; Knight, J.C.; Hand, D.P.; Shephard, J.D. Silica hollow core microstructured fibres for mid-infrared surgical applications. J. Non Cryst. Solids 2013, 377, 236–239. [Google Scholar] [CrossRef]
- Liang, L.B.; Ju, B.; Long, X.Q.; Liu, J.T.; Rong, S.Y.; Xia, C.M.; Chen, Y.; Hou, Z.Y.; Zhou, G.Y.; Zhao, N. Fabrication and optical properties of Tm3+/Al3+ co-doped photonic crystal fiber based on CO2 laser sintering technology. J. Abbr. 2008, 10, 142–149. [Google Scholar] [CrossRef]
- Chen, Y.; Zhao, N.; Liu, J.T.; Zhu, M.M.; Mai, Y.F.; Zhou, G.Y.; Hou, Z.Y.; Xia, C.M.; Zheng, Y.; Chen, Z.Q. Investigation of photo-darkening effect in ytterbium-doped microstructure optical fiber through the laser sintering fabrication method. J. Non-Cryst. Solids 2019, 521, 119468. [Google Scholar] [CrossRef]
- Sazio, P.J.A.; Correa, A.A.; Finlayson, C.E.; Hayes, J.R.; Scheidemantel, T.J.; Baril, N.F.; Jackson, B.R.; Won, D.J.; Zhang, F.; Margine, E.R.; et al. Microstructured optical fibers as high-pressure microfluidic reactors. Science 2006, 311, 1583–1586. [Google Scholar] [CrossRef]
- Schmidt, M.A.; Sempere, L.N.P.; Tyagi, H.K.; Poulton, C.G.; Russell, P.S.J. Waveguiding and plasmon resonances in two-dimensional photonic lattices of gold and silver nanowires. Phys. Rev. B 2008, 77, 033417. [Google Scholar] [CrossRef]
- Zhan, X.; Wang, R.; Cox, F.M.; Kuhlmey, B.T.; Large, M.C.J. Selective coating of holes in microstructured optical fiber and its application to in-fiber absorptive polarizers. Opt. Express 2007, 15, 16270–16278. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.W.; Schmidt, M.A.; Tyagi, H.K.; Sempere, L.P.; Russel, P.S.J. Polarization dependent coupling to plasmon modes on submicron gold wire in photonic crystal fiber. Appl. Phys. Lett. 2008, 93, 111102. [Google Scholar] [CrossRef]
- Lee, H.W.; Schmidt, M.A.; Russell, R.F.; Joly, N.Y.; Tyagi, H.K.; Uebel, P.; Russel, P.S.J. Pressure-assisted melt-filling and optical characterization of Au nano-wires in microstructured fibers. Opt. Express 2011, 19, 12180–12189. [Google Scholar] [CrossRef] [PubMed]
- Boehm, J.; François, A.; Ebendorff-Heidepriem, H.; Monro, T.M. Chemical deposition of silver for the fabrication of surface plasmon microstructured optical fibre sensors. Plasmonics 2011, 6, 133–136. [Google Scholar] [CrossRef]
- Lee, H.W.; Schmidt, M.A.; Russel, P.S.J. Excitation of a nanowire “molecule” in gold-filled photonic crystal fiber. Opt. Lett. 2012, 37, 2946–2948. [Google Scholar] [CrossRef]
- Li, B.Y.; Sheng, Z.C.; Wu, M.; Liu, X.Y.; Zhou, G.Y.; Liu, J.T.; Hou, Z.Y.; Xia, C.M. Sensitive real-time monitoring of refractive indices and components using a microstructure optical fiber microfluidic sensor. Opt. Lett. 2018, 43, 5070–5073. [Google Scholar] [CrossRef]
- Li, B.Y.; Wu, M.; Liu, X.Y.; Zhou, G.Y.; Liu, J.T.; Hou, Z.Y.; Xia, C.M. Surface plasmon resonance on the v-type microstructured optical fiber embedded with dual copper wires. Plasmonics 2019, 14, 383–387. [Google Scholar] [CrossRef]
- Paul, B.K.; Khalek, M.A.; Chakma, S.; Ahmed, K. Chalcogenide embedded quasi photonic crystal fiber for nonlinear optical applications. Ceram. Int. 2018, 44, 18955–18959. [Google Scholar] [CrossRef]
- Li, B.; Cheng, T.L.; Chen, J.X.; Yan, X. Graphene-enhanced surface plasmon resonance liquid refractive index sensor based on photonic crystal fiber. Sensors 2019, 19, 3666. [Google Scholar] [CrossRef]
- Younis, B.M.; Heikal, A.M.; Hameed, M.F.O.; Obayya, S.S.A. Highly wavelength-selective asymmetric dual-core liquid photonic crystal fiber polarization splitter. J. Opt. Soc. Am. B 2018, 35, 1020–1028. [Google Scholar] [CrossRef]
- Qu, Y.W.; Yuan, J.H.; Zhou, X.; Li, F.; Yan, B.B.; Wu, Q.; Wang, K.R.; Sang, X.Z.; Long, K.P.; Yu, C.X. Surface plasmon resonance-based silicon dual-core photonic crystal fiber polarization beam splitter at the mid-infrared spectral region. J. Opt. Soc. Am. B 2020, 37, 2221–2230. [Google Scholar] [CrossRef]
- Chiang, J.S.; Sun, N.H.; Lin, S.C.; Liu, W.F. Analysis of an ultrashort PCF-based polarization splitter. J. Lightw. Technol. 2010, 28, 707–713. [Google Scholar] [CrossRef]
- Li, J.H.; Wang, J.Y.; Wang, R.; Liu, Y. A novel polarization splitter based on dual-core hybrid photonic crystal fibers. Opt. Laser Technol. 2011, 43, 795–800. [Google Scholar] [CrossRef]
- Liu, Q.; Li, S.G.; Fan, Z.K.; Zhang, W.; Zi, J.C.; Li, H. Numerical analysis of high extinction ratio photonic crystal fiber polarization splitter based on ZnTe glass. Opt. Fiber Technol. 2015, 21, 193–197. [Google Scholar] [CrossRef]
- Paul, B.K.; Ahmed, K. Si7N3 material filled novel heptagonal photonic crystal fiber for laser applications. Ceram. Int. 2019, 45, 1215–1218. [Google Scholar] [CrossRef]
- Ahmed, K.; Paul, B.K.; Jabin, M.A.; Biswas, B. FEM analysis of birefringence, dispersion and nonlinearity of graphene coated photonic crystal fiber. Ceram. Int. 2019, 45, 15343–15347. [Google Scholar] [CrossRef]
- Hui, Z.Q.; Zhang, Y.K.; Soliman, A.H. Mid-infrared dual-rhombic air hole Ge20Sb15Se65 chalcogenide photonic crystal fiber with high birefringence and high nonlinearity. Ceram. Int. 2018, 44, 10383–10392. [Google Scholar] [CrossRef]












| 0.6961663 | 0.4079426 | 0.8974794 | 0.0684043 | 0.1162414 | 9.896161 |
| 5.9673 | 1.09 | 2113.6 | 15.92 | 650.07 | 104.86 |
| Ref. | DC-PCF Structure | Gold Film or Wire | SB | SL | Max IL |
|---|---|---|---|---|---|
| [12] | Square lattice with circular air holes | Gold wire | O + E + S + C + L + U band | 4.036 mm | 0.8 dB |
| [13] | Hexagonal lattice with elliptical air holes | Gold wire | S + C + L + U band | 254.6 | N/A |
| [14] | Hexagonal lattice with circular air holes | Gold wire | O and C bands | 830 | N/A |
| [15] | D-shape hexagonal lattice with circular air holes | Gold film | C band | 0.782 mm | 1.5 dB |
| [16] | Hexagonal lattice with circular air holes | Gold wire | S + C + L + U band | 577.5 | N/A |
| [17] | Rectangle lattice with circular air holes | Two gold wires | E + C band | 1 mm | N/A |
| [18] | Hexagonal lattice with circular air holes | Gold film | S + C + L band | 5.112 mm | N/A |
| [24] | Hexagonal lattice with circular air holes | Elliptical gold wire | C band | 1.079 mm | N/A |
| [25] | Square lattice with circular air holes | Gold film | S + C + L band | 47.26 | N/A |
| This work | Hexagonal lattice with circular air holes | Gold film | E + S + C + L + U band | 188 | <0.22 dB |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qu, Y.; Yuan, J.; Qiu, S.; Zhou, X.; Li, F.; Yan, B.; Wu, Q.; Wang, K.; Sang, X.; Long, K.; et al. A Novel Gold Film-Coated V-Shape Dual-Core Photonic Crystal Fiber Polarization Beam Splitter Covering the E + S + C + L + U Band. Sensors 2021, 21, 496. https://doi.org/10.3390/s21020496
Qu Y, Yuan J, Qiu S, Zhou X, Li F, Yan B, Wu Q, Wang K, Sang X, Long K, et al. A Novel Gold Film-Coated V-Shape Dual-Core Photonic Crystal Fiber Polarization Beam Splitter Covering the E + S + C + L + U Band. Sensors. 2021; 21(2):496. https://doi.org/10.3390/s21020496
Chicago/Turabian StyleQu, Yuwei, Jinhui Yuan, Shi Qiu, Xian Zhou, Feng Li, Binbin Yan, Qiang Wu, Kuiru Wang, Xinzhu Sang, Keping Long, and et al. 2021. "A Novel Gold Film-Coated V-Shape Dual-Core Photonic Crystal Fiber Polarization Beam Splitter Covering the E + S + C + L + U Band" Sensors 21, no. 2: 496. https://doi.org/10.3390/s21020496
APA StyleQu, Y., Yuan, J., Qiu, S., Zhou, X., Li, F., Yan, B., Wu, Q., Wang, K., Sang, X., Long, K., & Yu, C. (2021). A Novel Gold Film-Coated V-Shape Dual-Core Photonic Crystal Fiber Polarization Beam Splitter Covering the E + S + C + L + U Band. Sensors, 21(2), 496. https://doi.org/10.3390/s21020496







