1. Introduction
Multiferroic magnetoelectric (ME) composites attract large attention both from academe and industry due to their applications in sensor technology, high-frequency engineering, energy harvesting devices, random access memories, etc. [
1,
2,
3,
4,
5,
6]. Conventional ME layered composites are built from rigid constitutive materials (Young’s modulus
Y ~ 10
11 Pa). A significant reduction of the effective Young’s modulus below 10
9 Pa would allow one to decrease the resonance frequency of a composite layered multiferroic structure (~1–100 kHz in conventional multilayered composites), where the efficiency of ME coupling is maximum, to the low-frequency range of 1 to 100 Hz. This would be advantageous for a number of applications such as vibration energy harvesting [
7] or low-frequency magnetic-field sensing [
8,
9]. This justifies the interest in mechanically soft ME materials because of their absence in nature. At the present state of technology, polymer materials would be a natural choice. A comprehensive review of piezoelectric (PE) polymer materials is given in Ref. [
10]. Low resonance frequencies (in order of a few hundred Hz) can also be achieved in compositions of a PE polymer with a ferromagnetic metal if the thickness of the magnetostrictive (MS) layer is of the order of several micrometers [
11].
Two papers considered theoretically novel concepts on how to design ME soft materials. Alameh et al. [
12] proposed an approach that does not require the materials themselves to be ME, or piezoelectric (PE) or exhibit any exotic atomistic features that conventional hard crystalline multiferroics do. As long as the magnetic permeability of the soft matter is larger than that of vacuum, an emergent ME effect should appear due to the interaction of deformation and a pre-existing electric field. Rambausek and Keip [
13] demonstrated that the strain-mediated ME coupling induced by finite deformations in soft composites could be of significant magnitude due to the shape effect as a specific non-local phenomenon in magneto- and electro-elasticity. It was found that, among ellipsoidal bodies, the shape-influenced ME coupling should be most pronounced for those of spherical to moderately prolate shape.
Zhang et al. proposed an alternative way to design compliant ME composites and demonstrated several experimental realizations [
14,
15,
16]. The idea was to embed a hard-magnetic material (NdFeB), surrounded by an electrically conductive helix coil, into a soft silicone matrix. When the resulting material was deformed, e.g., compressed along the coil axis, an open-circuit output voltage up to
100 µV or the short-circuit output current up to
60 µA was observed between coil terminals due to electromagnetic induction. Therefore, the authors observed a direct PE effect and not a ME effect in their material realizations. It is plausible that such materials may display ME properties due to the interaction of hard-magnetic filler material with an external magnetic field.
A new, alternative line of research explores the so-called magnetoactive elastomers (MAEs), in which micrometer-sized ferromagnetic particles are embedded into a soft polymer matrix [
17,
18,
19,
20,
21,
22,
23]. MAEs are known to possess superior properties as far as the magnitude of the magneto-mechanical effects is concerned. For example, giant (~10
−1) magnetostriction can be observed using compliant MAEs [
24]. Relevant results were reported by Sheridan et al. [
25] and Kalita et al. [
26], who studied the significant bending of MAE cantilevers in external magnetic fields. To the best of our knowledge, the ME coupling in a layered composite with an MAE layer was first reported by Feng et al. [
27] who cured an MAE layer on a polyvinylidene fluoride (PVDF) film. Makarova et al. published a series of papers [
28,
29,
30] devoted to the development of ME polymer-based composite materials using silicone matrices. Initially, they reported multiferroic ferroelectric/ferromagnetic-polymer composite systems, consisting of ferroelectric lead zirconate titanate (PZT) particles, ferromagnetic NdFeB (or barium ferrite) particles, and silicone matrix [
28]. In the second paper, the authors fabricated composite materials based on ferroelectric porous structure and either MAE or magnetic-fluid filler [
29]. The maximum value of the coefficient of the converse ME transformation for the sample with Fe microparticles was found to be ≈36 pT·m/V, which is three orders of magnitude smaller than can be observed in conventional (stiff) laminated composites [
31]. Very recently, the same group reported the first realization of layered structures comprising a commercially available PE polymer (PVDF) and MAE and pointed out their perspectives for sensors and energy harvesting devices [
30]. The direct ME effect was observed as a voltage appearing between the electrodes of the PE polymer due to the bending of the composite structure in a non-uniform magnetic field. Unfortunately, the magnetic field gradient was not specified. The amplitude of voltage oscillations during magnetic-field pulse excitations (pulse duration ~10 ms) reached about 650 mV. The work [
30] demonstrated that a significant direct ME effect is feasible in multiferroic composite materials comprising MAE layers. However, further research is required, because the unconventional experimental configuration in [
30] (in particular, the external magnetic field is non-uniform) did not allow one to determine the ME coupling coefficient. Additionally, it remains unclear how the physical properties of the MAE layer(s) should be optimized in order to achieve the maximum ME voltage coefficient.
Very recently, Tan et al. [
32] published a new concept for developing soft ME materials, which they called the magnetoelectric electret (MEE). The key idea was to deposit net charges on the interface between two layers of materials, which are different in magnetic properties [
12]. One layer was a polytetrafluoroethylene (PTFE) thin film and the other layer was an MAE. Before bonding these two layers together, a layer of surface charge was deposited onto one surface of the PTFE thin film by the corona charging technique. In a uniform magnetic field, applied perpendicularly to the charged surface, the two-layered structure deformed asymmetrically, i.e., one layer (MAE) deformed more than the other layer (PTFE). This magnetic-field-induced asymmetric deformation resulted in voltage differences between the upper and lower surfaces of the MEE. The theoretical explanation was based on the concept of a Maxwell stress [
33]. The material exhibited a room temperature ME coefficient of about 0.24 V/A at the magnetic field of ≈48 kA/m and a low frequency of ≈1 Hz.
Hitherto, the usage of MAEs in mechanically soft ME multiferroic composites is in the initial stage, although the published results are very promising. The aim of this paper is to investigate in detail the behavior of laminated structures comprising an isotropic MAE layer and a commercially available PVDF-based vibration sensor in pulsed uniform magnetic fields, directed almost perpendicularly to the sample plane. In particular, the maximum induced voltage exceeds that reported in [
30] by more than eight-fold. Moreover, we report the first, to the best of our knowledge, measurement of the magnetically induced ME coupling coefficient in such a material [
34] and its peculiar dependence on the external magnetic field. The paper is organized as follows: In the following section, the fabrication methods of composite cantilevers and the experimental setup are described. In particular, the effect of the fabrication method on the ME response is addressed. In
Section 3, the magnetic properties of employed MAE materials are presented. Dependences of the induced voltage and the cantilever deflection on the composition of the MAE layer (volume fraction of the filler and the softness of the elastomer matrix) as well as on its thickness are presented. From these dependencies, the guidelines for designing MAE-PVDF structures with the maximum response to the (almost) perpendicular magnetic field are derived. Transient behavior is studied. In
Section 4, the underlying physical effects of the cantilever’s deflection and generated voltage are discussed. Furthermore, we present the first measurement of the ME coupling coefficient for this novel type of structure and discuss its physical significance. Conclusions are drawn in
Section 5, where also the future work is discussed.
2. Materials and Methods
2.1. Fabrication of Composite Structures
Planar structures comprised an MAE layer bonded to a commercially available PVDF-based vibration sensor (LDT0-028K, Measurement Specialties, Hampton, VA, USA) [
35]. The latter is a flexible component comprising a 28 μm thick PE PVDF polymer film with screen-printed silver ink electrodes, laminated to a 0.125 mm polyester substrate, and fitted with two crimped contacts. The PE film is displaced from the mechanical neutral axis, therefore bending creates very high strain within the PE polymer and high voltages are generated. In the following, this electronic component will be denoted as the PE polymer (PEP).
The synthesis of MAE materials followed the known path [
36,
37]. The entire technological process is illustrated in
Figure 1. The base polymer VS 100000 (vinyl-functional polydimethylsiloxane) for addition-curing silicones, the chain extenders Modifier 715 (SiH-terminated polydimethylsiloxane), the reactive diluent polymer MV 2000 (monovinyl functional polydimethylsiloxane), the crosslinker CL 210 (dimethylsiloxane-methyl hydrogen siloxane copolymer), the Pt catalyst 510, and the inhibitor DVS were provided by Evonik Hanse GmbH, Geesthacht, Germany. The silicone oil AK 10 (linear, nonreactive polydimethylsiloxane) was purchased from Wacker Chemie AG, Burghausen, Germany. A carbonyl iron powder (CIP) (type SQ, mean particle size of 3.9–5.0 μm, BASF SE Carbonyl Iron Powder and Metal Systems, Ludwigshafen, Germany) was used as the ferromagnetic filling. CIP is fabricated by thermal decomposition of iron pentacarbonyl (Fe(CO)
5), which is previously distilled to high purity [
38]. The minimum iron content in SQ particles is 99.5% [
39]. It is well known that CIP particles have a spherical shape, which was obtained by scanning electron microscopy [
40,
41].
The polymer VS 100000, the polymer MV 2000, the modifier 715 and the silicone oil AK 10 were put together and blended with an electric mixer to form an initial compound. In the next step, the initial compound was mixed together with the CIP particles and the CL 210. The crosslinking reaction was activated by the Pt-Catalyst 510. For the control of the Pt-catalyst’s activity, the inhibitor DVS was used. PE substrates were fixed in a specifically designed plastic mold and their top side was covered with a thin layer of a silicone adhesive (Permatex® High Temp Red RTV Silicone Gasket Maker, Solon, OH, USA). The uncured MAE in form of a viscous liquid was poured onto the adhesive layer to the desired thickness of the MAE layer. The rest of the material was put into another mold (Petri dish with a diameter of about 33 mm, Greiner Bio-One International GmbH, Kremsmünster, Austria) for further characterization and quality control. The resulting structure was cured in a universal oven (Memmert UF30, Memmert GmbH, Schwabach, Germany) for 24 h at 55 °C with air circulation. The curing was performed in the absence of a magnetic field. Therefore, the MAE material can be considered as random heterogeneous, i.e., no chain-like aggregates could be formed during crosslinking.
For the purposes of this study, two types of matrix were synthesized. We denote them as “soft” and “rigid” to emphasize the difference in the elastic moduli of the matrices. The word “soft” refers to the samples with the lower elastic modulus, while the word “rigid” refers to the samples with the higher elastic modulus. The variation in the elastic modulus was achieved by changing the amount of cross-linker added to the initial compound.
Table 1 summarizes typical mechanical properties of the two matrices and the MAE materials on their basis. The shear storage modulus
was obtained from rheological measurements as described in
Section 2.2 below.
As expected, the effective shear modulus of the MAE material increases with increasing concentration of iron particles and, for the same fraction of the filler, it is higher for composites with the rigid matrix.
Note that the curing of the MAE layer can be carried out directly on the PEP, i.e., step (2b) can be skipped. However, as it will be explained below, a thin layer of a silicone glue improves adhesion between MAE and PEP layers. Consequently, a thin layer of a silicone glue allows one to enhance the ME response of the composite cantilever.
In the present paper, the results obtained on 19 different cantilevers from 6 different material combinations are presented.
2.2. Material Characterization
To characterize the MAE materials using rheological measurements, fully cured MAE samples in the form of disk-shaped plate with a diameter of 20 mm and a thickness of 1 mm were cut from a Petri dish. Magnetorheological measurements were performed using a commercially available rheometer (Anton Paar, model Physica MCR 301). The angular oscillation frequency ω was kept constant at 10 rad/s. To avoid slippage, the normal force of approximately 1 N was applied. The moduli were measured at constant strain amplitude γ = 0.01%, which corresponds to the linear viscoelastic regime. The measurement time was 20 s per data point.
The MAE samples for magnetic measurements were also cut out of a mold. They were of cylindrical shape with a diameter of approximately 6 mm and a height of about 1 mm. Magnetization curves were measured in-plane (perpendicular to the cylinder axis) at room temperature by varying the external magnetic field from 1.5 T to −1.5 T and back again, using a vibrating sample magnetometer (model 7407, Lake Shore Cryotronics, Inc., Westerville, OH, USA).
2.3. Experimental Setup
To realize a cantilever, a fabricated structure was clamped at one end (on the side of electrical connectors) in a specifically designed 3D printed holder from the polylactic acid (PLA) material. The resulting dimensions of the cantilever are shown in
Figure 2a. The MAE material was always bonded to the “negative” side of the PE element. The side “polarity” is defined by the PE element manufacturer. On this side, the manufacturer’s logotype is imprinted, see
Figure 1. The cantilever was always clamped in such a way that the MAE was on its top side.
The cantilever in the holder was placed between the poles of a large electromagnet (EM2 model, MAGMESS Magnetmesstechnik Jürgen Ballanyi e.K., Bochum, Germany), cf.
Figure 2b. The pole diameter was 9.2 cm and the vertical distance between the poles was 3.4 cm. The vertical magnetic field of the electromagnet was highly uniform over the entire space where the cantilever could move. We verified that the uniformity of a magnetic field was better than 99.9% over the right circular cylinder with a radius of 2 cm and a height of 3.4 cm, which symmetry axis coincided with the symmetry axes of the magnetic poles. The electromagnet was powered by a bipolar power supply (FAST-PS 1k5, CAEN ELS s.r.l., Basovizza, Italy). The deflection of a cantilever was recorded using a CMOS-based camera (Alvium 1800 U-319 m, Allied Vision Technologies GmbH, Stadtroda, Germany) with a suitable lens (Edmund Optic Double Gauss Focusable, 25 mm C-mount F4.0 1.3”, Barrington, New Jersey, NJ, USA) and a backlight LED illumination (LED Illuminant G4 Pen). The generated voltage by a PE element was measured using a data acquisition board (NI USB-6212, National Instruments, Austin, TX, USA). The generated electrical charge was obtained by electrical current integration using a charge amplifier (Kistler 5018A, Winterthur, Switzerland). The entire experimental process was automated using the LabVIEW software.
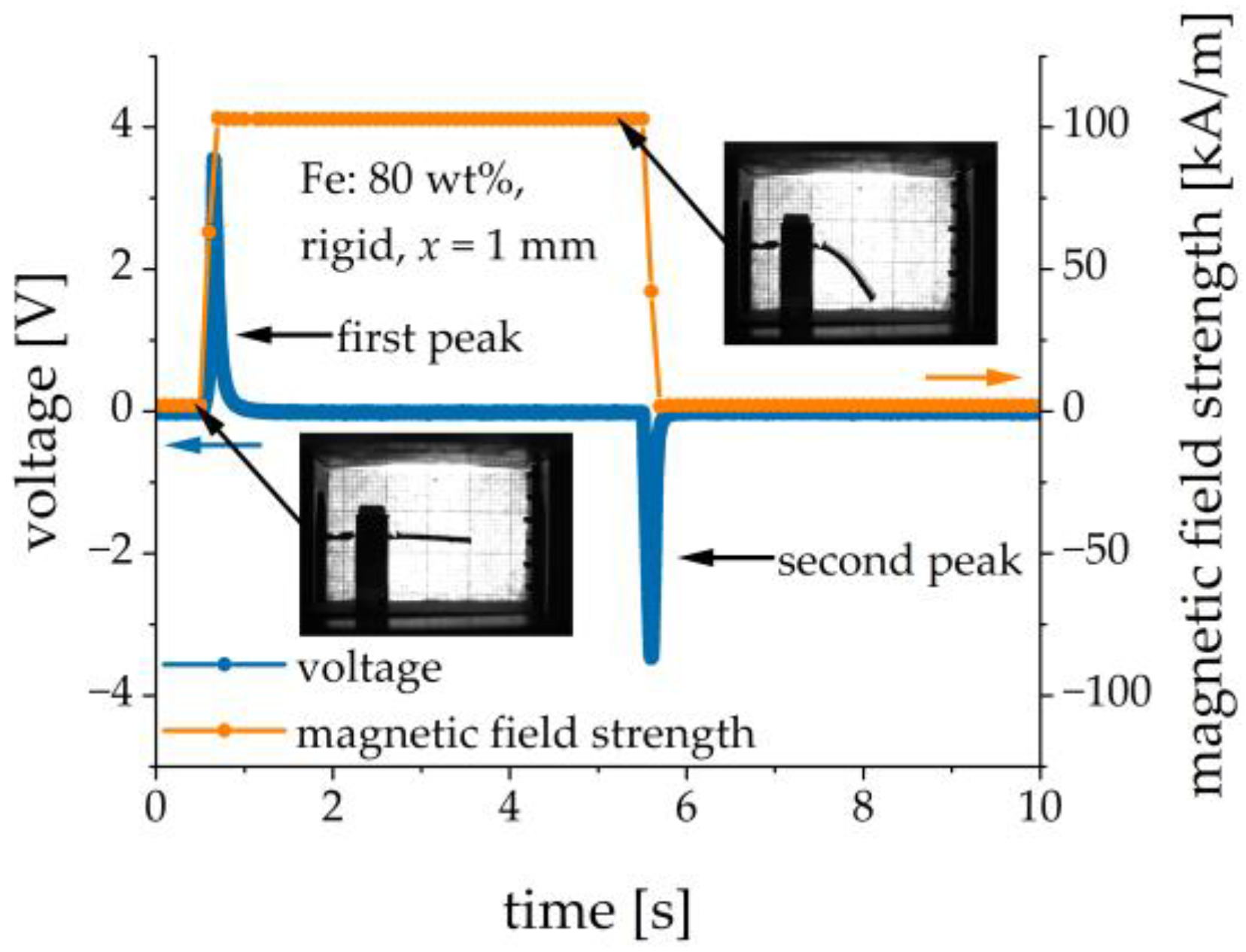
The “negative” side of the PE element was connected electrically to the conducting shield (“ground”) of the coaxial cable, while the “positive” side was connected to the core electrode (“signal”). With such a choice of the signal polarity, the first voltage peak was positive, when the composite cantilever was deflected down upon application of an external magnetic field. When an external magnetic field was switched off then, the second voltage peak was negative, see
Section 3.1 for details. If the cantilever was deflected up when a magnetic field was switched on and off, the polarity of two voltage peaks was changed to the opposite.
2.4. Image Processing
To quantify the deflection of the composite cantilever, image processing was performed. For this purpose, a Python script using the OpenCV library was written. The cantilever’s deflection
was determined as the vertical displacement of the “mass center” of the cantilever from its initial position in the absence of a magnetic field as presented in
Figure 3. The “mass center” shown as a colored dot in
Figure 3 was defined as the average pixel coordinate of the cantilever’s contour. We found that such an image analysis works more reliably than tracking the tip of the cantilever. The deflection of the free tip of the cantilever is approximately twice larger than the deflection of the “mass center”.
2.5. Measurement Protocol
The experimental setup was calibrated in such a way that the electric current through the electromagnet coils could be re-calculated into the magnetic field strength. The resulting magnetic field strength was directly proportional to the excitation current. Pulsed magnetic field excitations were studied. The magnetic field was first switched on. In general, the rate of the electrical current change could be set to some value. In the following experiments, the electrical current ramp rate was set to the highest possible value of 15 A/s, which corresponded to the magnetic-field ramp rate of approximately 770
. The resulting ramp of the magnetic field was practically linear. The magnetic field was kept constant for a few seconds and then it was switched off at the same rate. Normally, the magnetic field remained switched on for 2 s and the switch-off pauses between different settings of the magnetic field were also 2 s. Only in
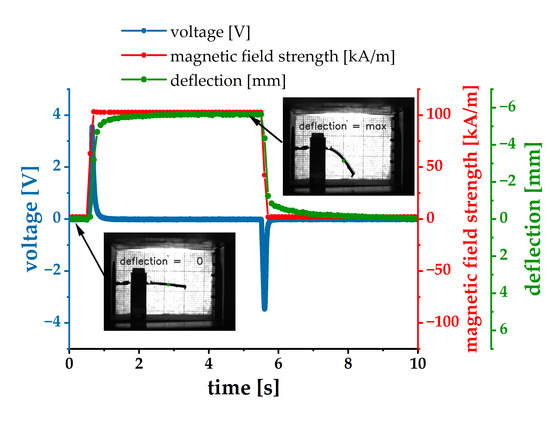
Section 3.5, where the transient behavior was studied, the magnetic field remained switched on for 5 s, in order to determine the time constant more precisely. The duration of the transient process for the electrical current (~0.1 s) was much less than a few seconds. The excitation current, the cantilever’s deflection (via image analysis) and the output voltage were measured as functions of time.
Figure 4 shows the typical transient behavior of the applied magnetic field and the resulting output voltage. Two voltage peaks of different polarities were observed, see
Figure 4. In the following, we refer to the measurements of the amplitude of the first voltage peak and to the maximum deflection of the cantilever in a particular magnetic field, which was normally reached just before the magnetic field was switched off. To remove some parasitic noise at mains frequency, a notch peak filtering at 50 Hz with the 3dB bandwidth of 10 Hz was employed for the output voltage.
Contrary to the results reported in [
30], we did not observe pronounced damped oscillations of the output voltage at a frequency of about 40 Hz, both in unfiltered and filtered signals, when the magnetic field was switched off. In most cases, we just observed the monotonic decay of the trailing edges of output voltage pulses. For some samples with heavier MAE layers (i.e., with the large MAE thickness and higher concentration of Fe particles), we detected oscillations of the output voltage at roughly 25 Hz overlapping the decay of the trailing edge. We attributed these oscillations to those at the resonant frequency of the composite cantilever with added mass, as is described in the datasheet for this PE element. These oscillations at the resonant frequency seem to be strongly damped by the presence of a relatively thick viscoelastic MAE layer. However, a possible presence of the damped oscillations of the composite cantilever does not influence the following considerations or conclusions.
4. Discussion
The bending mechanism of the composite MAE–PEP cantilever is described in Ref. [
26]. If a soft-magnetic planar MAE cantilever would be directed perpendicular to the uniform magnetic field, it would not be bent. A slight inclination of the normal to the MAE plane with respect to the external magnetic field leads to the torque acting on the MAE layer by the magnetic forces, because the magnetization of the MAE layer and the external magnetic field are not collinear. This torque tries to align the MAE slab parallel to the external magnetic field, in order to minimize its demagnetizing factor. Because the MAE layer is mechanically coupled to a flexible PE substrate, the entire structure is bent. The composite structure is made from flexible materials, therefore, the resulting deformation of the PE material is high, which leads to high output voltages. The maximum amplitude of the first voltage peak in the present work is 5.5 V, which is about 8.5-fold larger than the maximum voltage reported in [
30]. It is observed on the sample with 80 wt% of Fe, rigid matrix and a thickness
of 4 mm sample in a magnetic field
kA/m. The appearance of the electrical voltage between the electrodes of the PE material as a result of an applied magnetic field can be considered as the manifestation of the direct ME effect in an MAE-PEP structure. It is the mechanical strain that mediates the ME coupling in our case like in traditional (layered) ME heterostructures. In traditional layered heterostructures, the application of an external magnetic field perpendicular to the sample’s plane is not efficient, because of the large demagnetizing factor. In our case, the magnetostrictive material (MAE) is extremely soft (Young’s modulus ~10–100 kPa), while the PEP is flexible. This leads to large deformations and large output voltages.
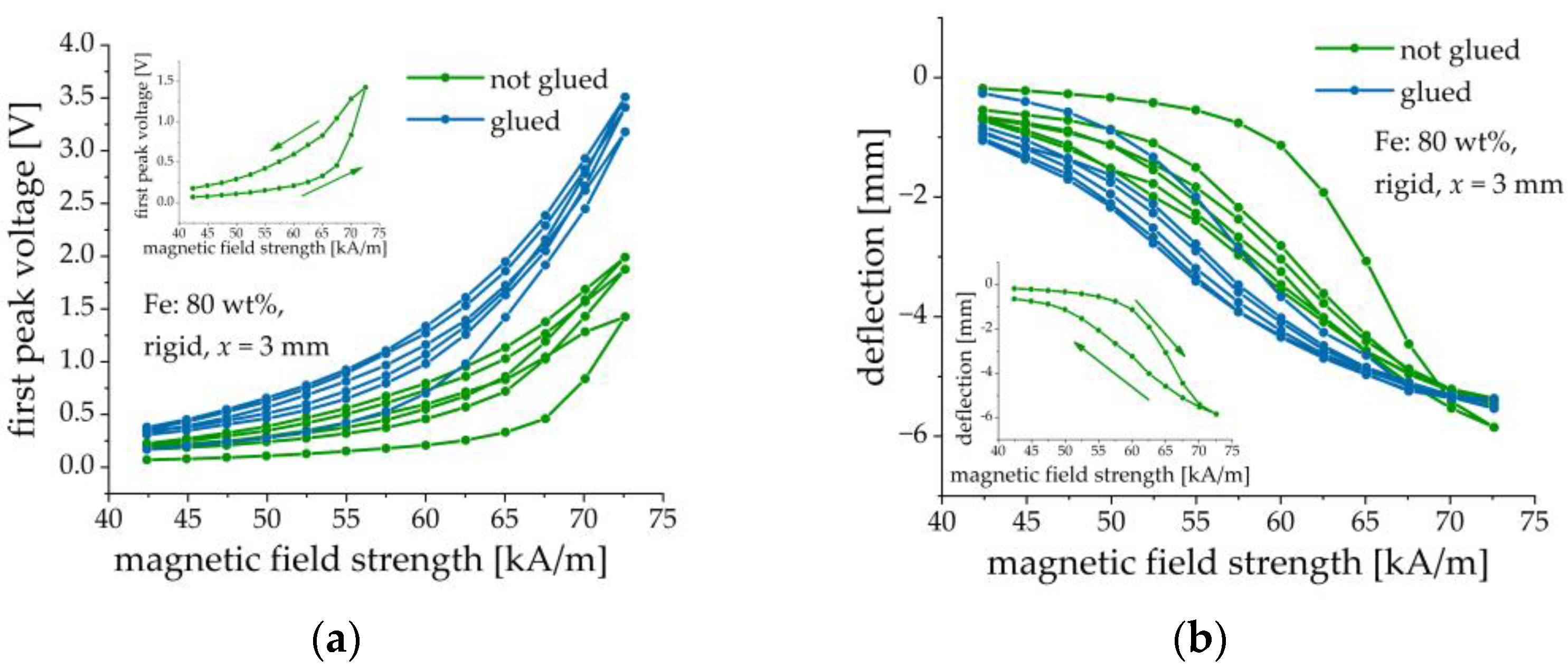
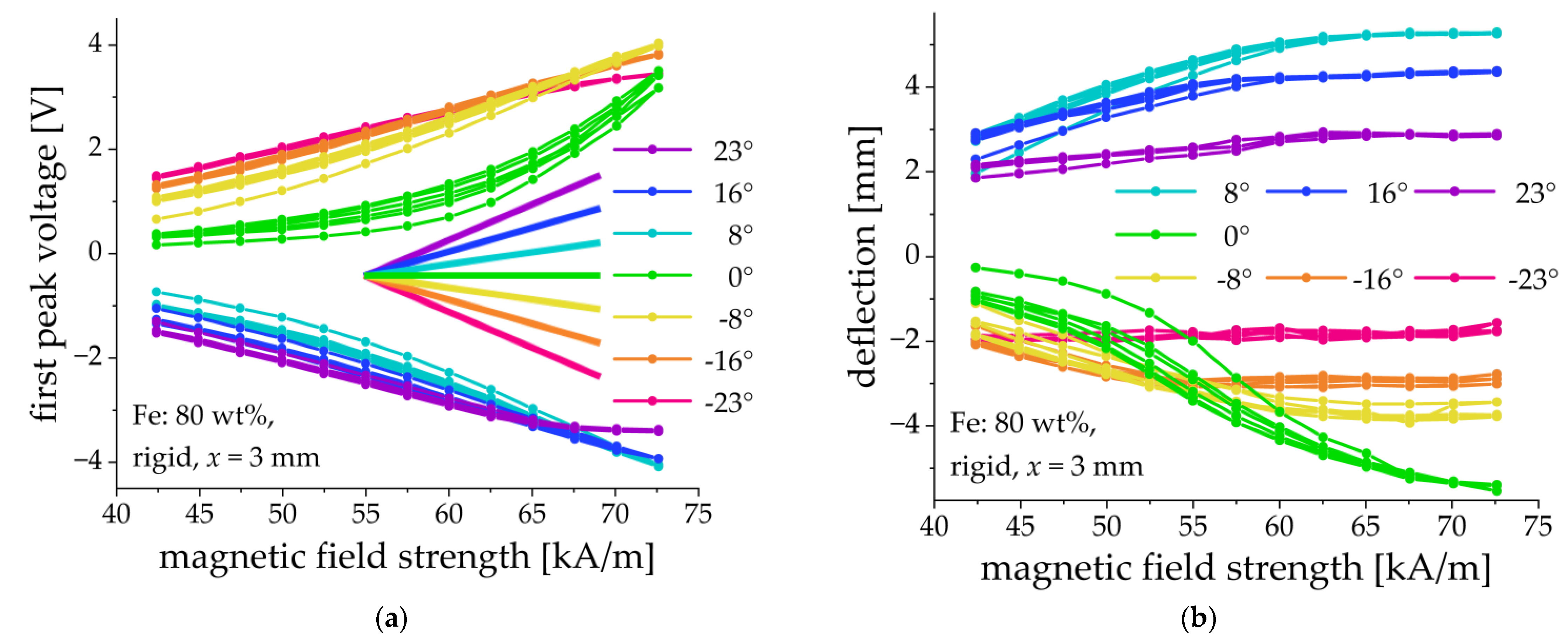
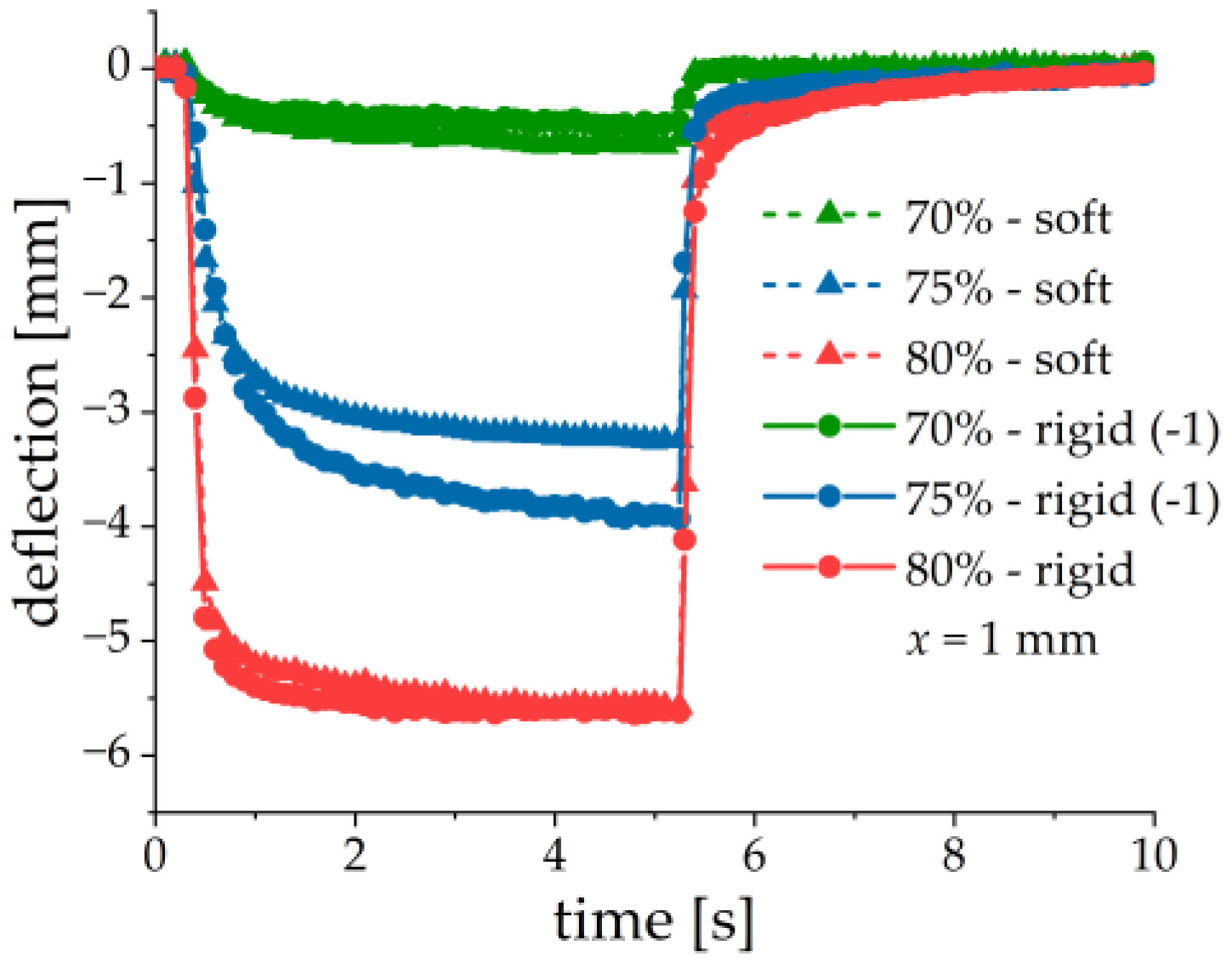
The main factor affecting the amplitude of the output voltage and the cantilever’s deflection seems to be the total quantity of iron in the MAE layer, because of the total magnetic moment induced in it and the resulting torque on the composite structure, see
Figure 11 and
Figure 12. A larger amount of iron filler leads to a higher magneto-mechanical response of the cantilever and to more efficient ME coupling. MAE layers with soft and rigid matrices give similar results because the difference in their stiffness can be neglected in comparison with the stiffness of the PEP.
The usage of an additional adhesive layer between the MAE and PEP provides improvements to the composite structures, not only as far as the structure mechanical stability (avoiding delamination) is concerned, but also increases the output voltage and reduces the hysteresis.
The direction of deflection can be efficiently controlled by the initial tilt of the clamping point in order to compensate for the effect of gravity. However, if the MAE-PEP cantilever is fixed horizontally, such a position is unstable in a magnetic field and depends on the geometrical details, such as the deviation of the PE substrate from the planarity.
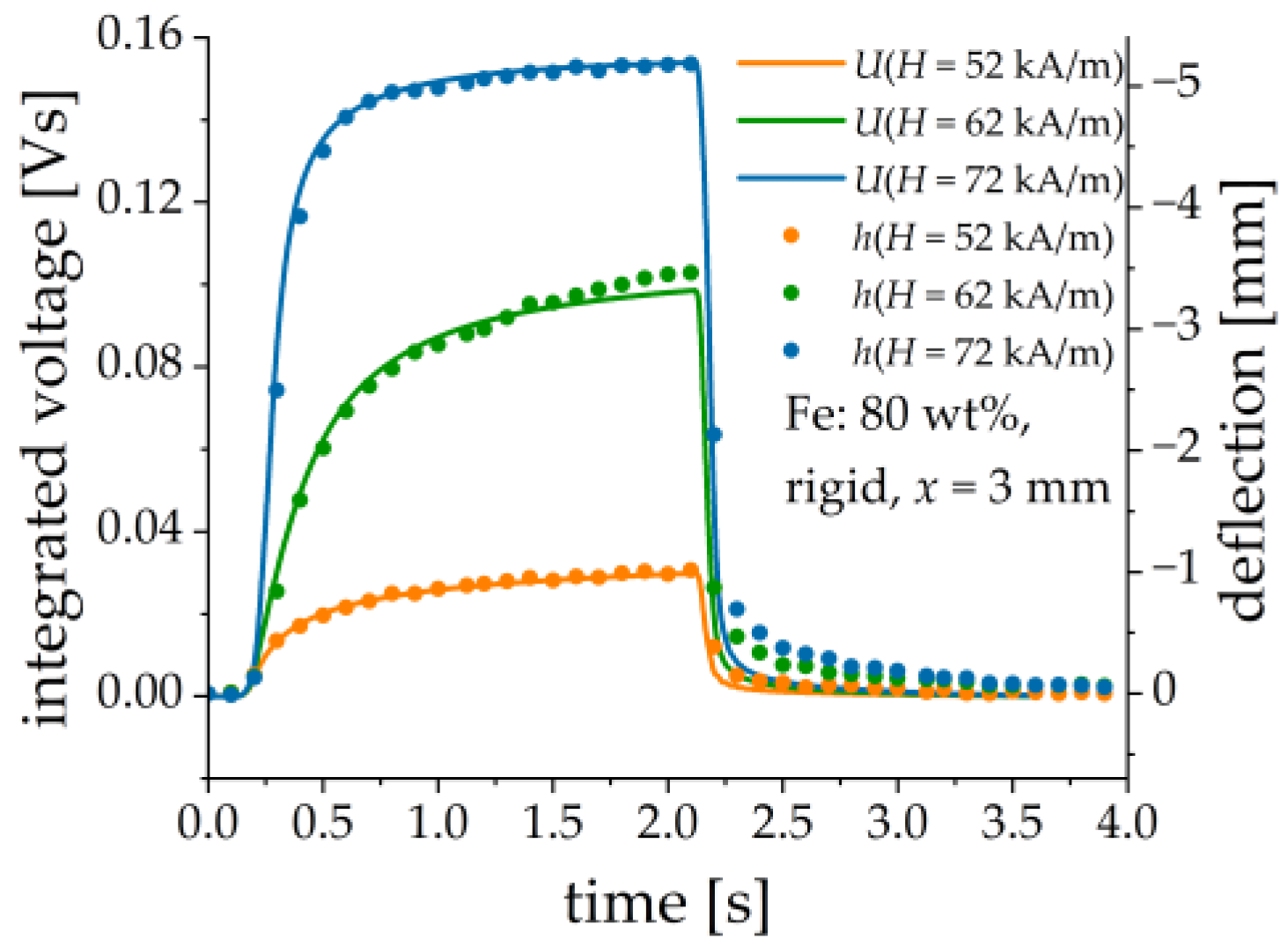
It is found that the output voltage can be reasonably described as proportional to the first-time derivative of the cantilever’s deflection, see
Figure 14. This can be explained by a simple model. Assume that the generated charge is linearly proportional to the deflection
h:
, where
is some constant. The simplified equivalent circuit of the PE transducer is a current source with the source current
and a capacitor
and a resistor
connected in parallel. The values of concentrated elements
and
are determined by the PE element and the measurement circuit, e.g., the connecting cable. The corresponding time constant of the equivalent circuit is
. The time dependence of the voltage
is described by the following inhomogeneous differential equation of the first order:
If
, the solution to Equation (1) can be written as:
The voltage is proportional to , if the time constant is small enough, that can be also seen from Equation (1) when .
Let us estimate the magnetically induced ME coupling coefficient:
for a particular MAE-PEP heterostructure with 80 wt% of Fe, rigid matrix and
mm. This sample is selected among others for demonstration because it has a high ME response and the largest working range of
. In (3) the external magnetic field
is directed vertically and
is the resulting electrical polarization. From the material equation
we obtain that
, where
is the induced electric field,
is the electric displacement,
is the vacuum permittivity,
is the relative permittivity of PE material, and
is the surface charge density on the electrodes of PE material. The surface charge density is measured by integration of the output current
by a charge amplifier, divided by the area
of the PE layer from the datasheet:
, where
is the generated electric charge. The material constant
is assumed to have the value
[
49].
Figure 15 presents the results of the measurements. A slow decline of the induced electrical charge in the constant magnetic field is attributed to the non-perfect characteristics (leakage) of the charge amplifier. We did not use this part of the transient behavior of the charge
for the calculation of the ME coupling coefficient. In
Figure 15a the measuring rate for
is 20 pts/s and 1000 pts/s for the charge
. Therefore, a cubic spline algorithm was used to interpolate between measured points of
. As described above, the deflection of the MAE-PEP cantilever is delayed with respect to the applied magnetic field. Therefore, a clear hysteresis behavior of the electrical polarization with respect to the applied magnetic field is observed in
Figure 15b.
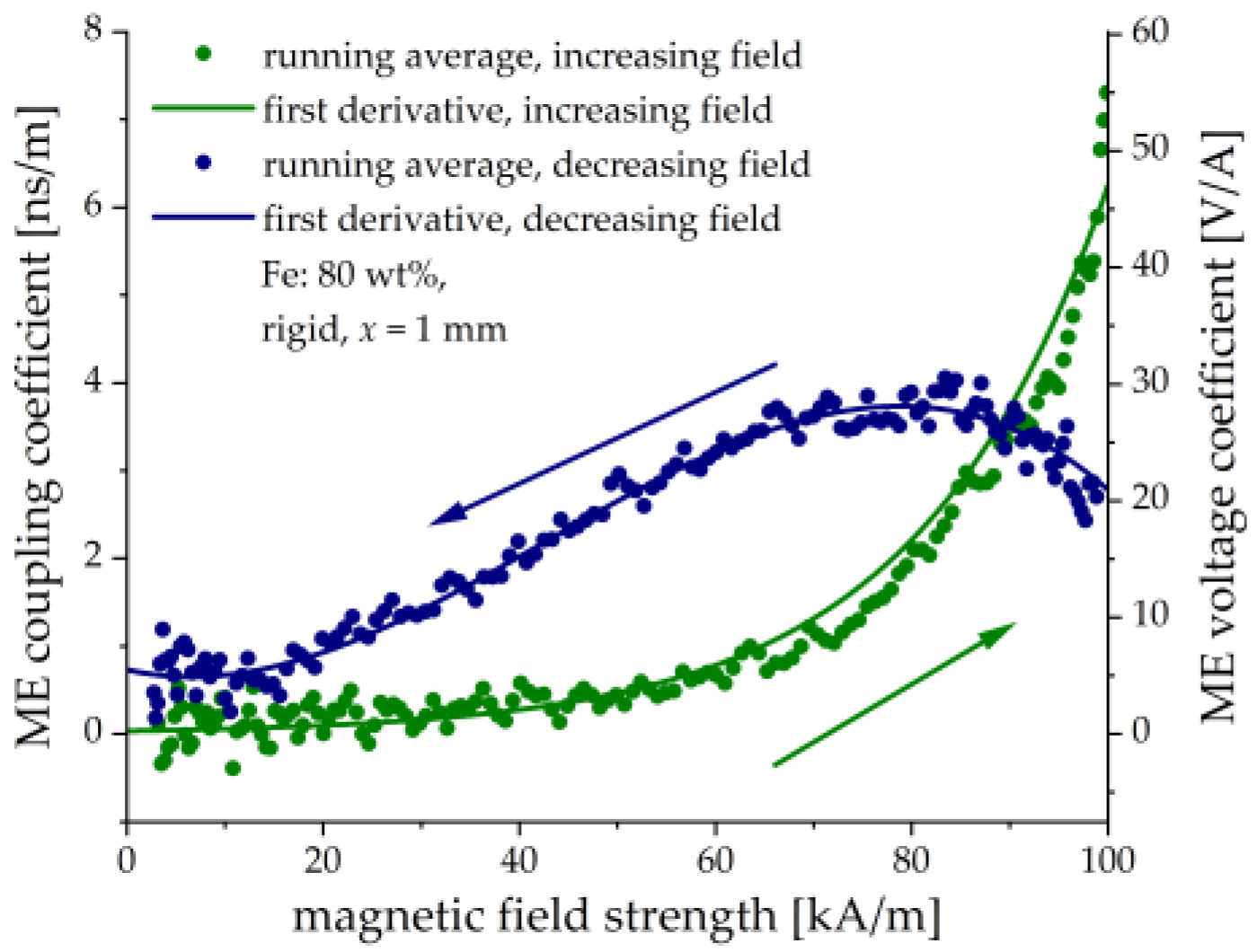
To determine the ME coupling coefficient
, the derivatives in (1) have to be calculated numerically from experimental data with some noise, which generally results in the scattering of calculated values. The dots in
Figure 16 represent the numerical first derivatives between the neighboring points and subsequent usage of the running average over five points. The continuous lines show the first derivatives of the fitted analytical functions. For the increasing magnetic field, the following empirical dependence of the polarization
on the magnetic field
is used:
. For the decreasing magnetic field, a polynomial dependence is used:
. The derivatives are then calculated analytically. We also recalculated the ME coupling coefficient
into the magnetically induced voltage ME coefficient
[
34,
50].
It is seen in
Figure 16 that the field behavior of the ME coupling coefficient differs strongly for the ascending and descending magnetic fields. There is a monotonic increase in
with the increasing magnetic field and a local maximum in the decreasing magnetic field. We are not aware of any similar behavior observed in traditional (metal/ceramics) layered heterostructures. Of course, the observed hysteresis is related to the delayed response between the applied magnetic field and the induced electrical polarization. It is seen from
Figure 15, that the electric charge continues to grow even when that magnetic field reached the steady-state. This is because the cantilever’s deflection still takes place. Formally, such behavior would result in an infinite ME coupling coefficient, which is non-physical and, hence not shown in
Figure 16. The reason is that Equation (3) implies the instantaneous ME response, which is not the case in our paper. We intended to investigate the field dependence of the ME coupling coefficient and its transient behavior in more detail in the following works. The maximum value of the ME voltage coefficient is about 50 V/A (≈40 V/(cm Oe) in cgs units). It compares well with conventional layered heterostructures, in particular taking into account that it was obtained in a quasi-static magnetic field on the non-optimized structure. The ME coefficient can be increased by using thicker MAE layers, optimized PE substrates with large PE coefficients and flexibility and, likely, by operation at the resonant frequency of the resulting heterostructure. Further research is required on whether it is possible to significantly reduce the time constant for the ME response and to minimize hysteresis.