Interval State Estimation in Active Distribution Systems Considering Multiple Uncertainties
Abstract
:1. Introduction
- A more accurate ISE model including the multiple uncertainties caused by the the non-Gaussian measurement noise, inaccurate line parameters, stochastic power outputs of DG, and plug-in EV are proposed for DSSE. To the best of our knowledge, there is no existing ISE model that includes such kind of uncertainties completely.
- A new ISE algorithm based on the MKO and ICP is proposed for the distribution systems to deal with the multiple uncertainties mentioned above and reduce the conservativeness of state estimation results.
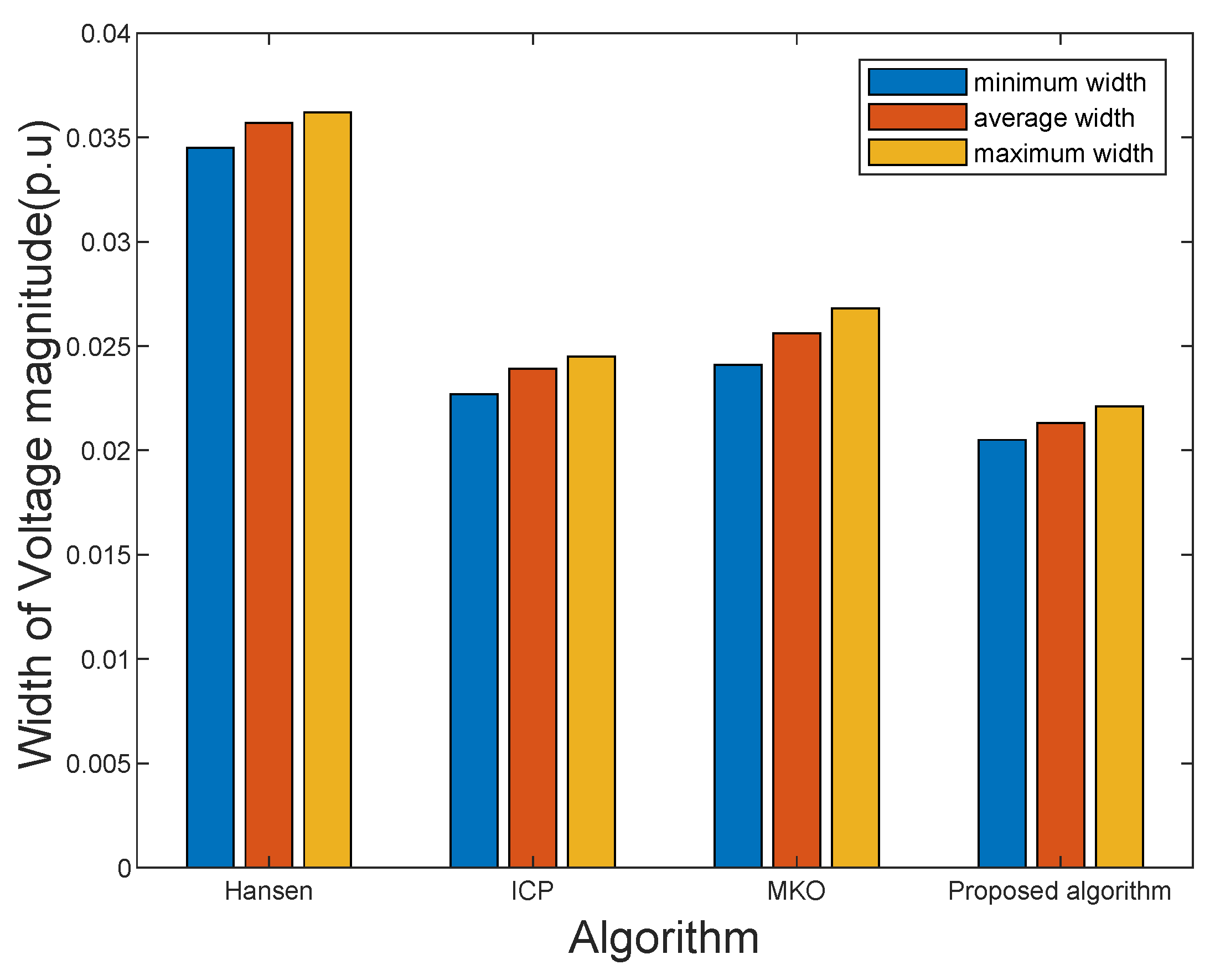
- The proposed algorithm can obtain more tighter upper and lower bounds of state estimation results than other existing methods such as the Hansen, Krawczyk, KM-ICP, MKO, and ICP algorithms.
2. Distribution System State Estimation Model
2.1. Measurement Model
2.2. ISE Model
3. Proposed ISE Approach
3.1. Proposed ISE Model
3.2. Proposed ISE Algorithm
4. Simulation and Results
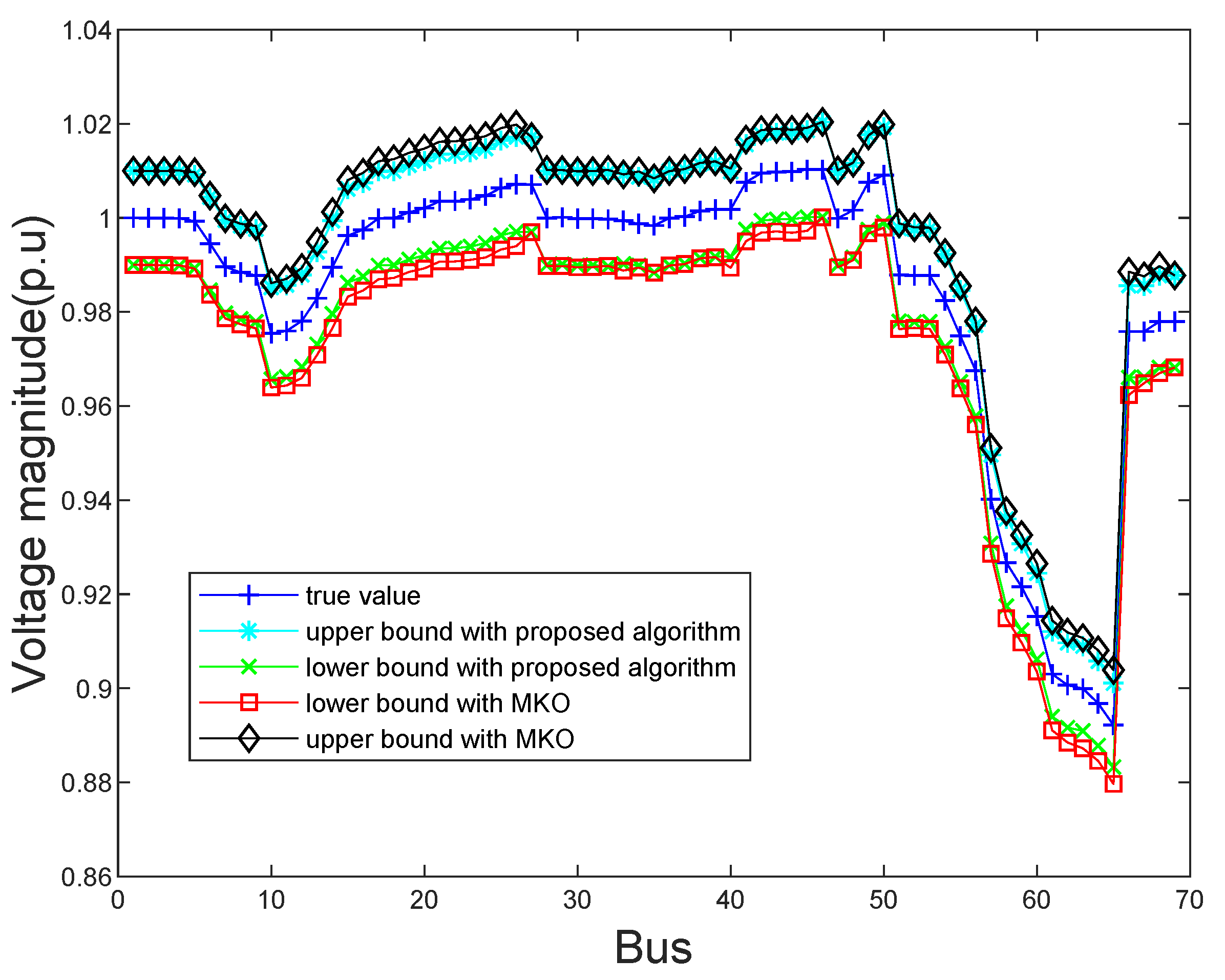
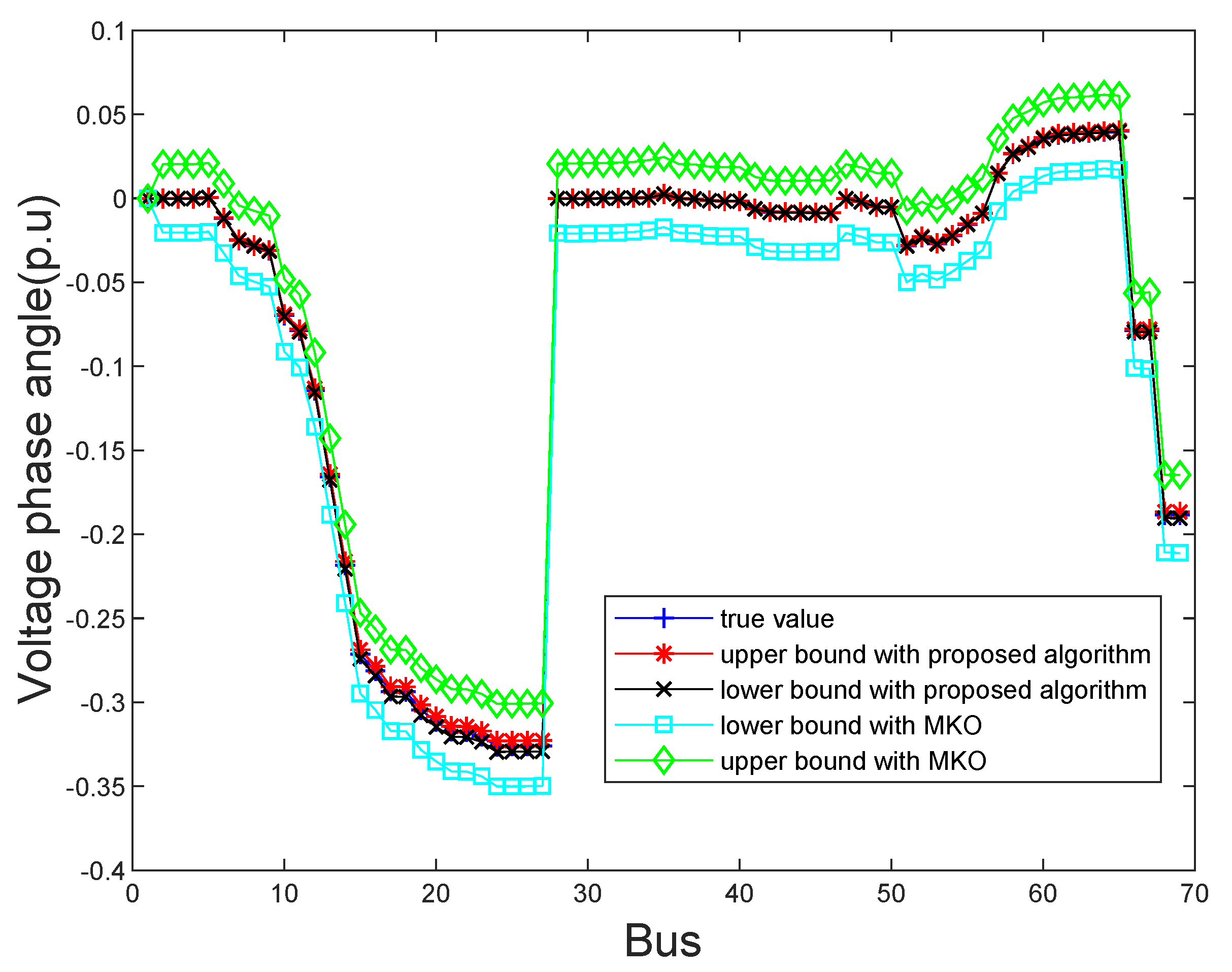
4.1. Result Analysis
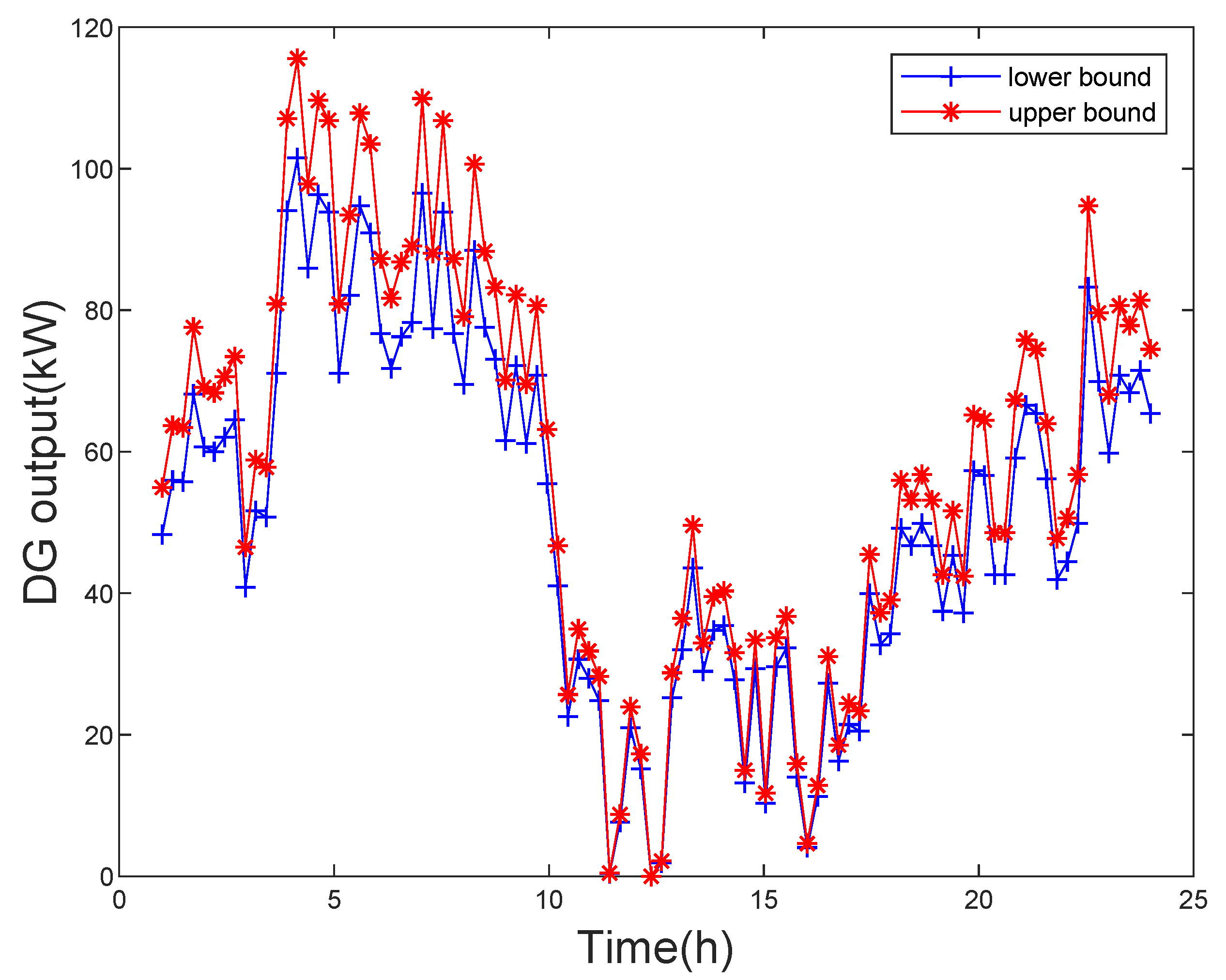
4.2. Effect of DG Units Uncertainty
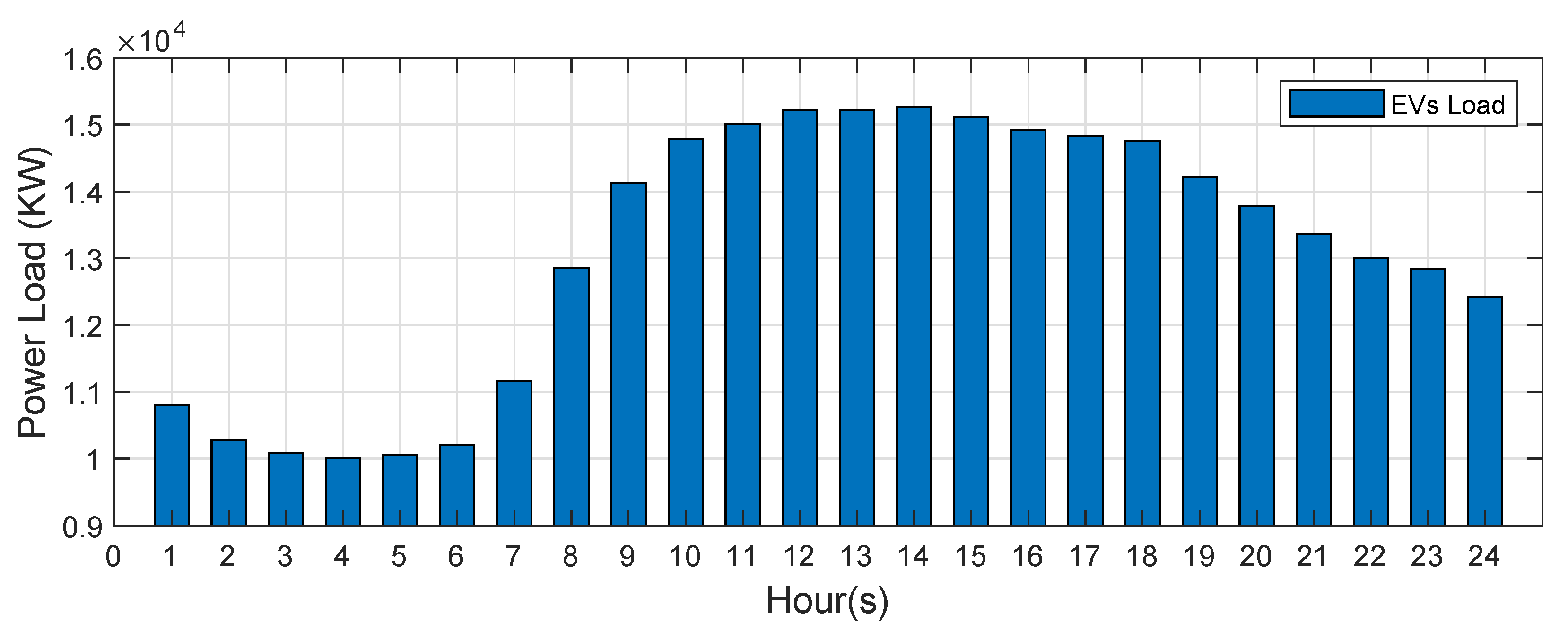
4.3. Effect of EV Units Uncertainty
4.4. Calculation Efficiency
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Fang, Z.; Lin, Y.; Song, S.; Song, C.; Lin, X.; Cheng, G. Active distribution system state estimation incorporating photovoltaic generation system model. Electr. Power Syst. Res. 2020, 182, 106247. [Google Scholar] [CrossRef]
- Abur, A.; Gómez-Expósito, A. Power System State Estimation: Theory and Implementation; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Göl, M.; Abur, A. LAV Based Robust State Estimation for Systems Measured by PMUs. IEEE Trans. Smart Grid 2014, 5, 1808–1814. [Google Scholar] [CrossRef]
- Decentralized three-phase distribution system static state estimation based on phasor measurement units. Electr. Power Syst. Res. 2018, 160, 327–336. [CrossRef]
- Robust pseudo-measurement modeling for three-phase distribution systems state estimation. Electr. Power Syst. Res. 2020, 180, 106138. [CrossRef]
- Primadianto, A.; Lu, C. A Review on Distribution System State Estimation. IEEE Trans. Power Syst. 2017, 32, 3875–3883. [Google Scholar] [CrossRef]
- Huang, Y.; Xu, Q.; Hu, C.; Sun, Y.; Lin, G. Probabilistic State Estimation Approach for AC/MTDC Distribution System Using Deep Belief Network With Non-Gaussian Uncertainties. IEEE Sens. J. 2019, 19, 9422–9430. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, J.; Li, Z. Interval State Estimation With Uncertainty of Distributed Generation and Line Parameters in Unbalanced Distribution Systems. IEEE Trans. Power Syst. 2020, 35, 762–772. [Google Scholar] [CrossRef] [Green Version]
- Martínez-Parrales, R.; Fuerte-Esquivel, C.; Alcaide-Moreno, B. Analysis of bad data in power system state estimation under non-gaussian measurement noise. Electr. Power Syst. Res. 2020, 186, 106424. [Google Scholar] [CrossRef]
- Chen, T.; Cao, Y.; Sun, L.; Qing, X.; Zhang, J. A Distributed Robust Power System State Estimation Approach Using t-Distribution Noise Model. IEEE Syst. J. 2020, 15, 1066–1076. [Google Scholar] [CrossRef]
- Kuhar, U.; Pantoš, M.; Kosec, G.; Švigelj, A. The Impact of Model and Measurement Uncertainties on a State Estimation in Three-Phase Distribution Networks. IEEE Trans. Smart Grid 2019, 10, 3301–3310. [Google Scholar] [CrossRef]
- Huang, M.; Wei, Z.; Pau, M.; Ponci, F.; Sun, G. Interval State Estimation for Low-Voltage Distribution Systems Based on Smart Meter Data. IEEE Trans. Instrum. Meas. 2019, 68, 3090–3099. [Google Scholar] [CrossRef]
- Schmidt, M.; Schegner, P. Deriving power uncertainty intervals for low voltage grid state estimation using affine arithmetic. Electr. Power Syst. Res. 2020, 189, 106703. [Google Scholar] [CrossRef]
- Vaccaro, A.; Villacci, D. Radial Power Flow Tolerance Analysis by Interval Constraint Propagation. IEEE Trans. Power Syst. 2009, 24, 28–39. [Google Scholar] [CrossRef]
- Muscas, C.; Pau, M.; Pegoraro, P.A.; Sulis, S. Uncertainty of Voltage Profile in PMU-Based Distribution System State Estimation. IEEE Trans. Instrum. Meas. 2016, 65, 988–998. [Google Scholar] [CrossRef]
- Cheng, G.; Song, S.; Lin, Y.; Huang, Q.; Lin, X.; Wang, F. Enhanced state estimation and bad data identification in active power distribution networks using photovoltaic power forecasting. Electr. Power Syst. Res. 2019, 177, 105974. [Google Scholar] [CrossRef]
- Pegoraro, P.A.; Sulis, S. Robustness-Oriented Meter Placement for Distribution System State Estimation in Presence of Network Parameter Uncertainty. IEEE Trans. Instrum. Meas. 2013, 62, 954–962. [Google Scholar] [CrossRef]
- Mousavi-Seyedi, S.S.; Aminifar, F.; Afsharnia, S. Parameter Estimation of Multiterminal Transmission Lines Using Joint PMU and SCADA Data. IEEE Trans. Power Deliv. 2015, 30, 1077–1085. [Google Scholar] [CrossRef]
- Pegoraro, P.A.; Brady, K.; Castello, P.; Muscas, C.; von Meier, A. Line Impedance Estimation Based on Synchrophasor Measurements for Power Distribution Systems. IEEE Trans. Instrum. Meas. 2019, 68, 1002–1013. [Google Scholar] [CrossRef]
- Xu, J.; Wu, Z.; Yu, X.; Hu, Q.; Dou, X. An Interval Arithmetic-Based State Estimation Framework for Power Distribution Networks. IEEE Trans. Ind. Electron. 2019, 66, 8509–8520. [Google Scholar] [CrossRef]
- Pegoraro, P.A.; Angioni, A.; Pau, M.; Monti, A.; Muscas, C.; Ponci, F.; Sulis, S. Bayesian Approach for Distribution System State Estimation With Non-Gaussian Uncertainty Models. IEEE Trans. Instrum. Meas. 2017, 66, 2957–2966. [Google Scholar] [CrossRef] [Green Version]
- Valverde, G.; Saric, A.T.; Terzija, V. Stochastic Monitoring of Distribution Networks Including Correlated Input Variables. IEEE Trans. Power Syst. 2013, 28, 246–255. [Google Scholar] [CrossRef]
- Moore, R.E.; Kearfott, R.B.; Cloud, M.J. Introduction to Interval Analysis; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2009. [Google Scholar]
- Wu, Z.; Zhan, H.; Gu, W.; Zheng, S.; Li, B. Interval State Estimation of Distribution Network With Power Flow Constraint. IEEE Access 2018, 6, 40826–40835. [Google Scholar] [CrossRef]
- Ngo, V.; Wu, W.; Lou, Y. Revised constraint-propagation method for distribution interval state estimation. IET Gener. Transm. Distrib. 2020, 14, 1329–1336. [Google Scholar] [CrossRef]
- Sparse and numerically stable implementation of a distribution system state estimation based on Multifrontal QR factorization. Electr. Power Syst. Res. 2020, 189, 106734. [CrossRef]
- Batko, W.; Pawlik, P. Uncertainty Evaluation in Modelling of Acoustic Phenomena with Uncertain Parameters Using Interval Arithmetic. Acta Phys. Pol. 2012, 121, A152–A155. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, Z.; Wu, X. The Multiobjective Based Large-Scale Electric Vehicle Charging Behaviours Analysis. Complexity 2018, 2018, 1968435. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.; Ponci, F.; Monti, A.; Muscas, C.; Pegoraro, P.A.; Sulis, S. Optimal Meter Placement for Robust Measurement Systems in Active Distribution Grids. IEEE Trans. Instrum. Meas. 2014, 63, 1096–1105. [Google Scholar] [CrossRef]
- Ghosh, A.K.; Lubkeman, D.L.; Downey, M.J.; Jones, R.H. Distribution circuit state estimation using a probabilistic approach. IEEE Trans. Power Syst. 1997, 12, 45–51. [Google Scholar] [CrossRef]
- Zhao, J.; Mili, L. A Framework for Robust Hybrid State Estimation With Unknown Measurement Noise Statistics. IEEE Trans Ind. Inform. 2018, 14, 1866–1875. [Google Scholar] [CrossRef]
- Wang, S.; Zhao, J.; Huang, Z.; Diao, R. Assessing Gaussian Assumption of PMU Measurement Error Using Field Data. IEEE Trans. Power Deliv. 2018, 33, 3233–3236. [Google Scholar] [CrossRef]
- Kotz, S.; Nadarajah, S. Multivariate T-Distributions and Their Applications; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Zhao, J.; Mili, L. Sparse State Recovery Versus Generalized Maximum-Likelihood Estimator of a Power System. IEEE Trans. Power Syst. 2018, 33, 1104–1106. [Google Scholar] [CrossRef]
- Yu, Y.; Wang, Z.; Lu, C. A Joint Filter Approach for Reliable Power System State Estimation. IEEE Trans. Instrum. Meas. 2019, 68, 87–94. [Google Scholar] [CrossRef]
- Huang, Y.; Werner, S.; Huang, J.; Kashyap, N.; Gupta, V. State Estimation in Electric Power Grids: Meeting New Challenges Presented by the Requirements of the Future Grid. IEEE Signal Process. Mag. 2012, 29, 33–43. [Google Scholar] [CrossRef] [Green Version]
- Göl, M.; Abur, A. A Hybrid State Estimator For Systems With Limited Number of PMUs. IEEE Trans. Power Syst. 2015, 30, 1511–1517. [Google Scholar] [CrossRef]




| System | Hansen [23] | Krawczyk [24] | ICP [14] | KM-ICP [25] | MKO [8] | Proposed Algorithm |
|---|---|---|---|---|---|---|
| IEEE33 | 0.029 | 0.0282 | 0.0199 | 0.0192 | 0.0253 | 0.0177 |
| Improvement | 38.9 | 37.2 | 30.03 | 7.81 | 11.05 | 0 |
| IEEE69 | 0.0276 | 0.0261 | 0.0209 | 0.0203 | 0.0227 | 0.0189 |
| Improvement | 31.52 | 27.58 | 16.7 | 6.89 | 9.56 | 0 |
| IEEE123 | 0.0357 | 0.0354 | 0.0239 | 0.0235 | 0.0256 | 0.0213 |
| Improvement | 40.4 | 39.8 | 18.9 | 10.6 | 12.4 | 0 |
| Number | Bus Node |
|---|---|
| 0 | - |
| 3 | 21,24,32 |
| 6 | 14,19,24,27,32,33 |
| 9 | 2,3,14,19,21,24,27,32,33 |
| System | Hansen [23] | Krawczyk [24] | ICP [14] | KM-ICP [25] | MKO [8] | Proposed Algorithm |
|---|---|---|---|---|---|---|
| IEEE33 | 0.1146 | 0.1247 | 0.2018 | 0.1976 | 0.1672 | 0.3025 |
| IEEE69 | 0.2457 | 0.2488 | 1.0279 | 0.2319 | 1.3671 | 1.6142 |
| IEEE123 | 14.3678 | 14.7462 | 27.623 | 12.9574 | 29.373 | 32.33 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, T.; Ren, H.; Amaratunga, G.A.J. Interval State Estimation in Active Distribution Systems Considering Multiple Uncertainties. Sensors 2021, 21, 4644. https://doi.org/10.3390/s21144644
Chen T, Ren H, Amaratunga GAJ. Interval State Estimation in Active Distribution Systems Considering Multiple Uncertainties. Sensors. 2021; 21(14):4644. https://doi.org/10.3390/s21144644
Chicago/Turabian StyleChen, Tengpeng, He Ren, and Gehan A. J. Amaratunga. 2021. "Interval State Estimation in Active Distribution Systems Considering Multiple Uncertainties" Sensors 21, no. 14: 4644. https://doi.org/10.3390/s21144644
APA StyleChen, T., Ren, H., & Amaratunga, G. A. J. (2021). Interval State Estimation in Active Distribution Systems Considering Multiple Uncertainties. Sensors, 21(14), 4644. https://doi.org/10.3390/s21144644







