Microcantilever: Dynamical Response for Mass Sensing and Fluid Characterization
Abstract
1. Introduction
2. Cantilever Mechanics and Dynamical Response
2.1. Euler–Bernoulli Beam
2.2. Harmonic Oscillations with a Single Degree of Freedom
2.2.1. Simple Harmonic Oscillator
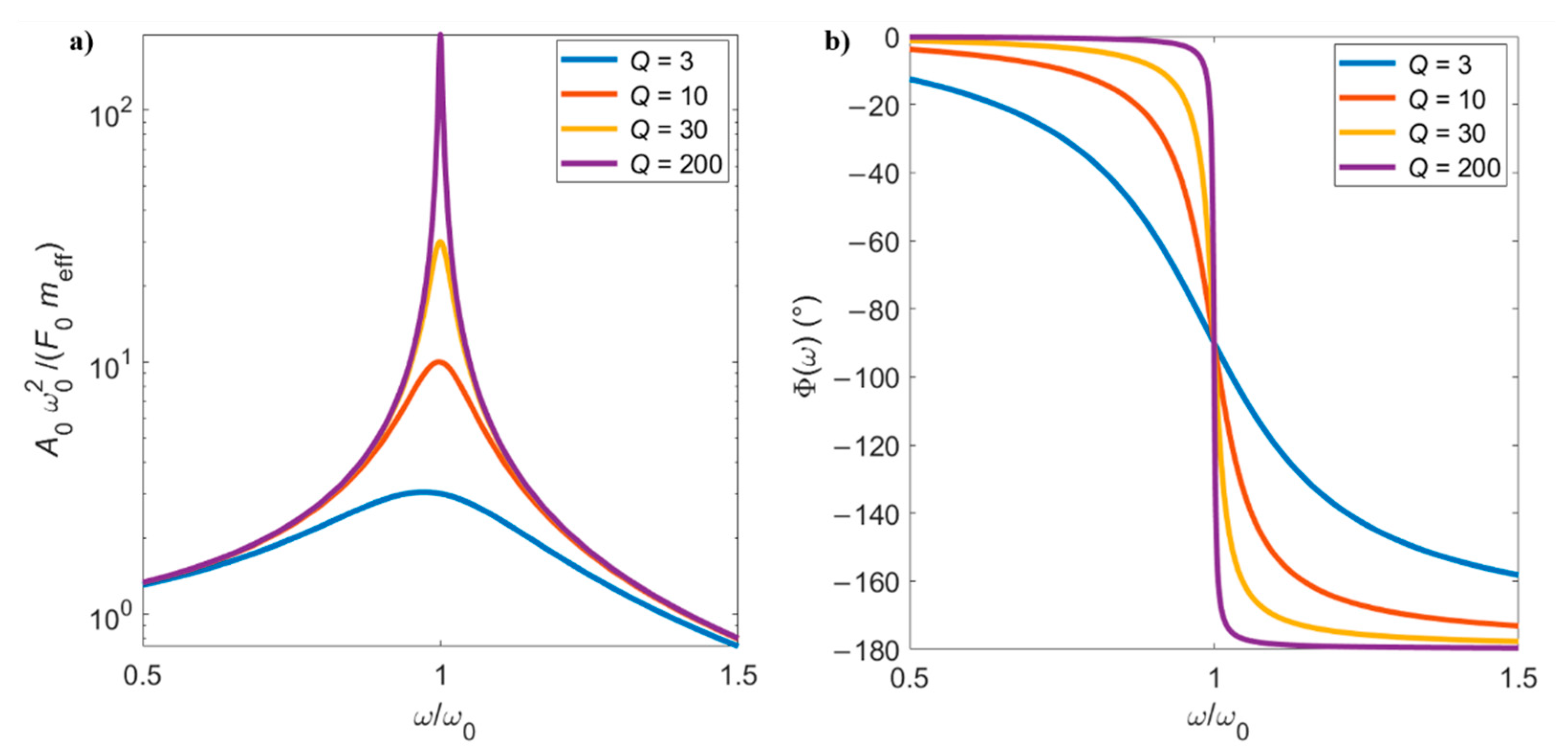
2.2.2. Forced Damped Harmonic Oscillator
2.2.3. General One-Degree-of-Freedom Equation of Motion for Microcantilevers
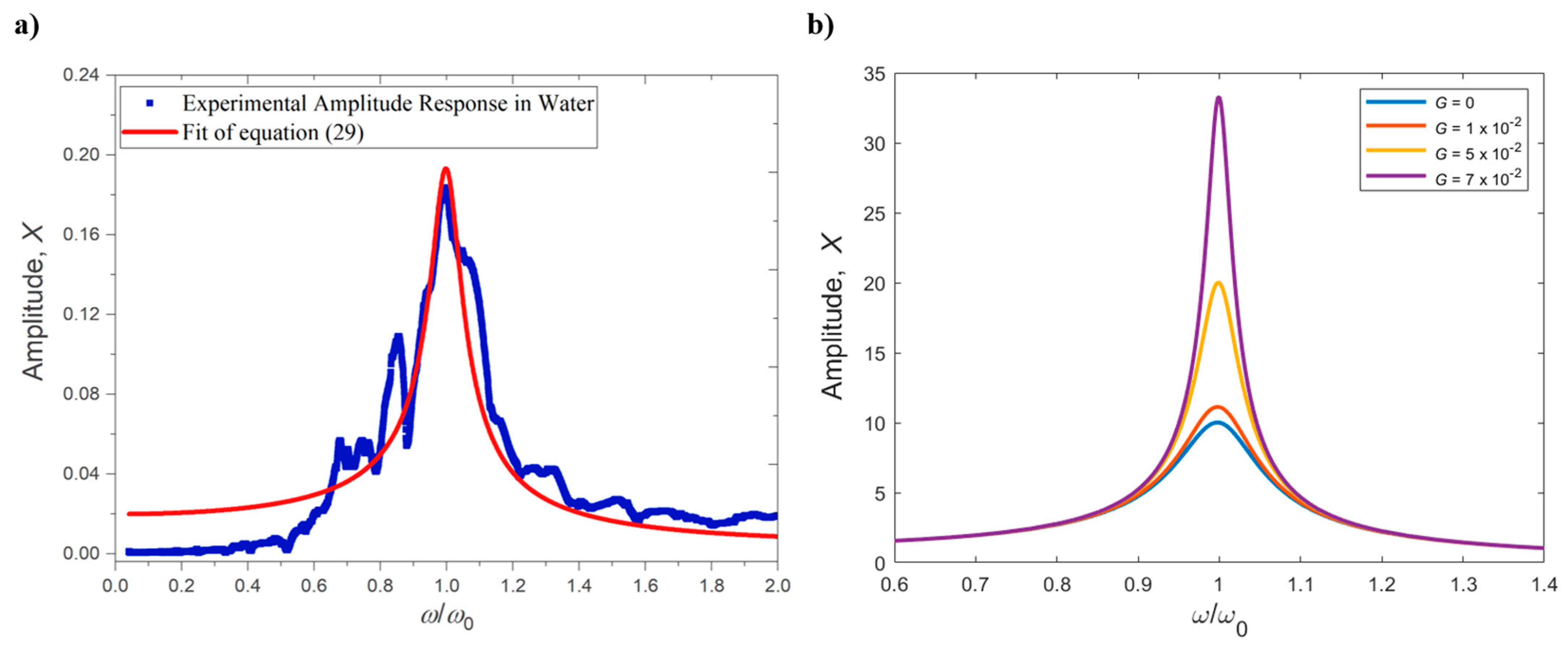
2.3. Operation in Dissipative Fluids
3. Excitation Schemes and Noise
3.1. Excitation Strategies
3.1.1. External or Open-Loop Excitation Mechanisms
3.1.2. Feedback or Closed-Loop Excitation Mechanisms
3.2. Detection Mechanisms
3.3. Noise
3.3.1. Time Domain—Allan deviation
3.3.2. Frequency Domain—Spectral Densities
3.3.3. Conversion between Frequency and Time Domain—Power Law Spectral Densities
3.3.4. Physical Origins of Noise
3.3.5. Minimum Detectable Frequency Shift,
4. Mass Sensing
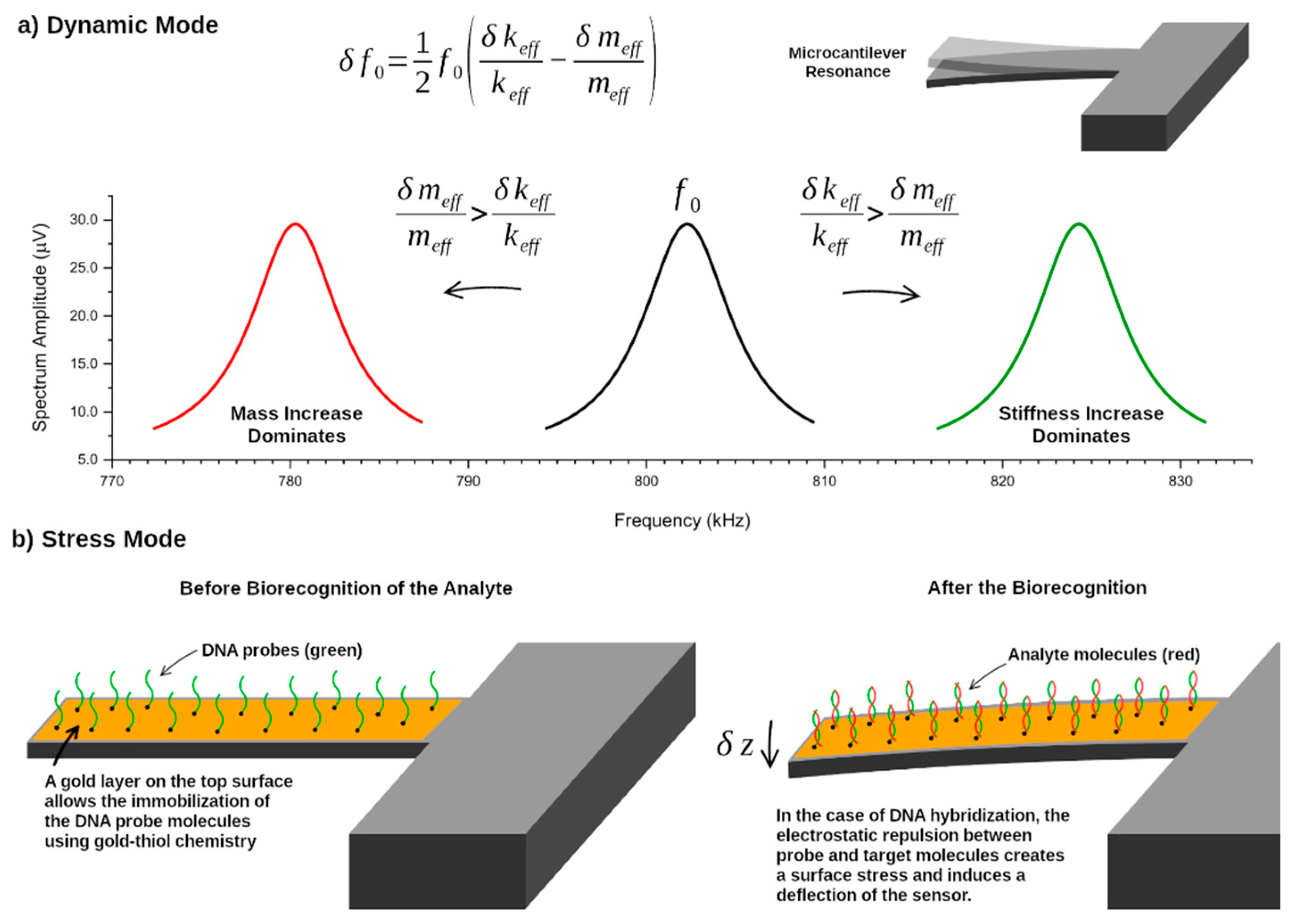
4.1. Dynamic vs Static Sensing Modes
4.2. Mass Sensitivity
4.3. Limits of Detection (LoD)
5. Viscosity Sensing
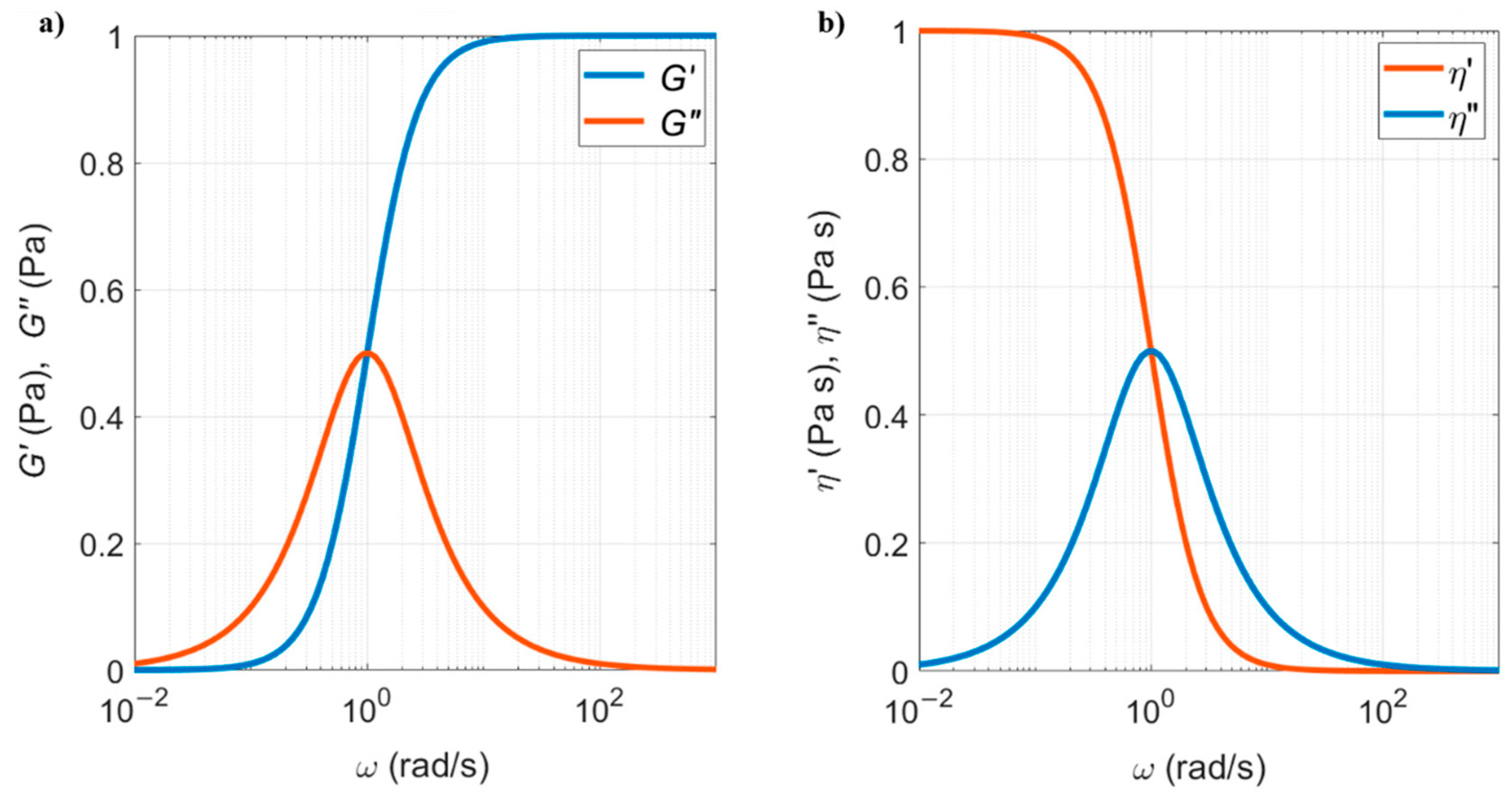
5.1. Viscoelastic Materials
5.2. Measuring Rheological Properties of Fluids Using Microcantilevers
5.2.1. Newtonian Fluids
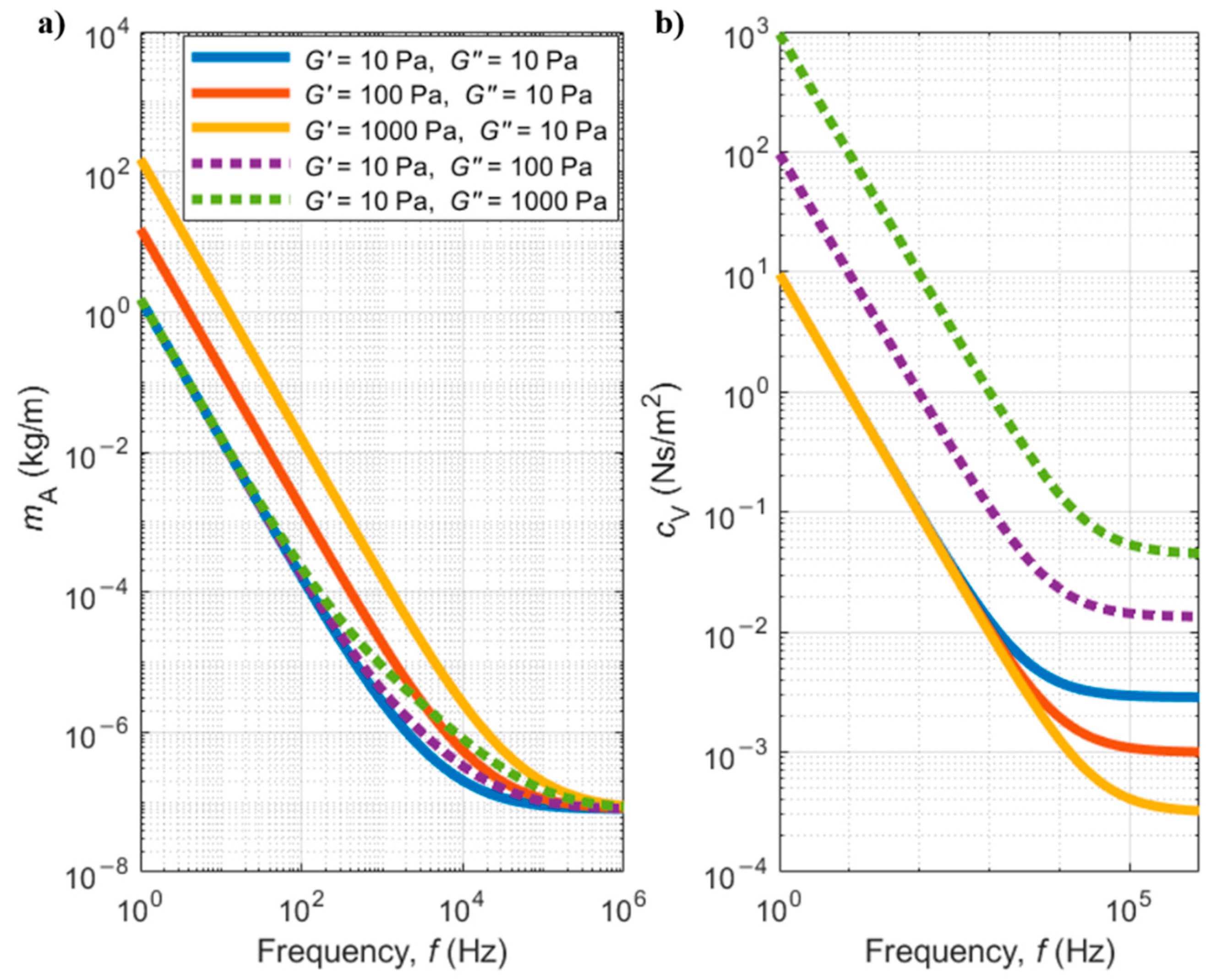
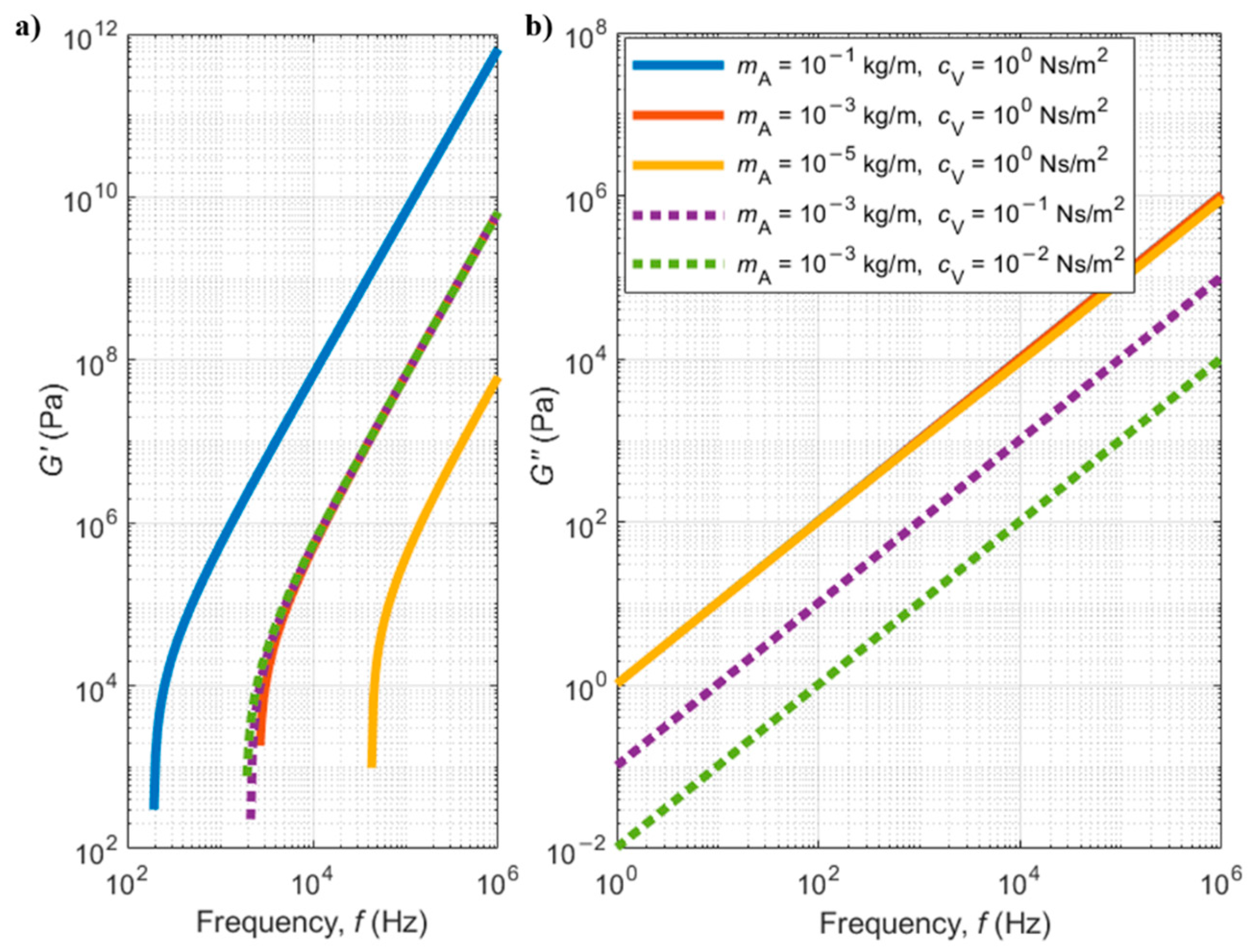
5.2.2. Viscoelastic Fluids
6. Outlook and Further Challenges
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
List of Symbols (in Order of Appearance in the Text)
| L | cantilever length |
| w | cantilever width |
| h | cantilever thickness |
| t | time |
| x | space coordinate (distance from the cantilever support) |
| time-varying distributed load acting on the beam at a distance x from the support, per unit length | |
| time-varying deflection of the beam at a distance x from the support | |
| shear forces acting on the element of the beam | |
| bending moment acting on the element of the beam | |
| density of the structural material | |
| area of rectangular beam cross section | |
| second moment of area of the rectangular cross section beam | |
| E | Young’s modulus of the structural material |
| temporal term solution of harmonic oscillation | |
| spacial term solution of harmonic oscillation | |
| constants of spacial term solution of harmonic oscillation | |
| natural (undamped) resonance frequency of mode n | |
| natural (undamped) radial resonance frequency of mode n | |
| displacement of the one-degree-of-freedom microcantilever from the equilibrium position (z = 0) | |
| velocity of the one-degree-of-freedom microcantilever | |
| acceleration of the one-degree-of-freedom microcantilever | |
| effective spring constant of the microcantilever | |
| effective mass of the nth resonant mode of the microcantilever | |
| total mass of the microcantilever | |
| intrinsic viscous damping coefficient | |
| Q | quality factor |
| excitation frequency | |
| excitation harmonic force at , with amplitude | |
| amplitude of the motion at | |
| phase between the applied external force and the motion at | |
| resonance frequency of the nth mode of intrinsically damped resonators | |
| mass of the cantilever per unit length | |
| intrinsic viscous damping coefficient per unit length | |
| time-varying distributed hydrodynamic load, acting on the beam at a distance x, per unit length | |
| added mass by interactions with the surrounding fluid, per unit length | |
| added damping coefficient by interactions with the surrounding fluid, per unit length | |
| resonance frequency of the nth mode of extrinsically damped resonators with added mass and damping | |
| quality factor of the nth mode | |
| real part of the hydrodynamic load acting on a microcantilever with rectangular cross section | |
| imaginary part of the hydrodynamic load acting on a microcantilever with rectangular cross section | |
| density of the fluid | |
| viscosity of the fluid | |
| thickness of the layer in which the velocity of the fluid drops by a factor of 1/e | |
| Reynolds number | |
| a1, a2, b1, b2 | Maali’s constants for |
| τ | integration time |
| Allan deviation for time windows of duration τ | |
| consecutive ith frequency measurements | |
| nominal carrier frequency | |
| spectral density of frequency fluctuations | |
| spectral density of phase fluctuations | |
| measured root mean squared (rms) value of normalized frequency | |
| measured root mean squared (rms) value of normalized phase | |
| width of the frequency band in Hz | |
| power density in one single sideband due to phase modulation by noise, for a 1 Hz bandwidth (dBm/Hz) | |
| total power of the carrier (dBm) | |
| single-sideband phase noise, the ratio of to (dBc/Hz) | |
| cut-off frequency of an infinitely sharp low-pass filter | |
| , , , , | constants to fit power-laws to random walk frequency noise, flicker of frequency, white frequency noise, flicker of phase and white phase noise, respectively |
| , , , , | numerical constants for conversion between frequency (spectral densities) and time (Allan deviation) domains |
| minimum measurable frequency shift | |
| LoD | limit of detection |
| shift in the natural (undamped) resonance frequency | |
| shift in the damped resonance frequency of microcantilevers with added mass and damping; | |
| infinitesimal change of the effective stiffness of the cantilever induced by the adsorbate | |
| infinitesimal change of the effective mass of the cantilever induced by the adsorbate | |
| infinitesimal change of the added mass induced by the fluid | |
| infinitesimal change in the viscosity of the fluid | |
| infinitesimal change in the density of the fluid | |
| S | sensitivity |
| , | mass sensitivity in vacuum and in fluid |
| viscosity sensitivity | |
| , | applied shear stress and shear stress rate |
| , | shear strain and shear strain rate of a viscous dashpot |
| , | shear strain and shear strain rate of an elastic spring |
| , | shear strain and shear strain rate of the spring-dashpot series |
| elasticity constant of the fluid | |
| characteristic relaxation time of the fluid | |
| frequency of the applied shear stress and induced total strain response | |
| phase between applied stress and total strain response | |
| amplitude of the shear stress | |
| amplitude of the total strain response | |
| dynamic elastic modulus | |
| , | elastic and viscous parts of the dynamic elastic modulus |
| complex dynamic viscosity | |
| , | viscous and elastic parts of the dynamic viscosity |
| general ratio of amplitudes of the transfer function |
References
- Leissa, A.W.; Qatu, M.S. Vibrations of Continuous Systems; McGraw-Hill: New York, NY, USA, 2011. [Google Scholar]
- Bao, M. Analysis and Design Principles of MEMS Devices; Elsevier Science: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Beards, C.F. Structural Vibration: Analysis and Damping; Arnold: London, UK, 1996. [Google Scholar]
- Younis, M.I. MEMS Linear and Nonlinear Statics and Dynamics; Springer Science and Business Media LLC: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Meirovitch, L.; Parker, R. Fundamentals of Vibrations. Appl. Mech. Rev. 2001, 54, B100–B101. [Google Scholar] [CrossRef]
- Kaajakari, V.; Mattila, T.; Oja, A.; Seppa, H. Nonlinear Limits for Single-Crystal Silicon Microresonators. J. Microelectromech. Syst. 2004, 13, 715–724. [Google Scholar] [CrossRef]
- Dohn, S.; Svendsen, W.; Boisen, A.; Hansen, O. Mass and Position Determination of Attached Particles on Cantilever Based Mass Sensors. Rev. Sci. Instruments 2007, 78, 103303. [Google Scholar] [CrossRef] [PubMed]
- Lifshitz, R.; Cross, M. Nonlinear Dynamics of Nanomechanical and Micromechanical Resonators. Rev. Nonlinear Dyn. Complex. 2009, 1–52. [Google Scholar] [CrossRef]
- Kovacic, I.; Brennan, M. The Duffing Equation—Nonlinear Oscillators and Their Behaviour; Wiley-VCH Verlag: Weinheim, Germany, 2011. [Google Scholar]
- Villanueva, L.G.; Karabalin, R.B.; Matheny, M.H.; Chi, D.; Sader, J.E.; Roukes, M.L. Nonlinearity in Nanomechanical Cantilevers. Phys. Rev. B 2013, 87, 1–8. [Google Scholar] [CrossRef]
- Venstra, W.J.; Westra, H.J.R.; Van Der Zant, H.S.J. Mechanical Stiffening, Bistability, and Bit Operations in A Microcantilever. Appl. Phys. Lett. 2010, 97, 193107. [Google Scholar] [CrossRef]
- Zega, V.; Nitzan, S.; Li, M.; Ahn, C.H.; Ng, E.; Hong, V.; Yang, Y.; Kenny, T.; Corigliano, A.; Horsley, D.A. Predicting the Closed-Loop Stability and Oscillation Amplitude of Nonlinear Parametrically Amplified Oscillators. Appl. Phys. Lett. 2015, 106, 233111. [Google Scholar] [CrossRef]
- Villanueva, L.G.; Karabalin, R.B.; Matheny, M.H.; Kenig, E.; Cross, M.C.; Roukes, M.L. A Nanoscale Parametric Feedback Oscillator. Nano Lett. 2011, 11, 5054–5059. [Google Scholar] [CrossRef]
- Karabalin, R.B.; Masmanidis, S.C.; Roukes, M.L. Efficient Parametric Amplification in High and Very High Frequency Piezoelectric Nanoelectromechanical Systems. Appl. Phys. Lett. 2010, 97, 183101. [Google Scholar] [CrossRef]
- Van Leeuwen, R.; Karabacak, D.M.; Van Der Zant, H.S.J.; Venstra, W.J. Nonlinear Dynamics of a Microelectromechanical Oscillator with Delayed Feedback. Phys. Rev. B 2013, 88, 1–5. [Google Scholar] [CrossRef]
- Mestrom, R.M.C.; Fey, R.H.B.; Nijmeijer, H. Phase Feedback for Nonlinear MEM Resonators in Oscillator Circuits. IEEE/ASME Trans. Mechatron. 2009, 14, 423–433. [Google Scholar] [CrossRef]
- Mouro, J.; Chu, V.; Conde, J.P. Dynamics of Hydrogenated Amorphous Silicon Flexural Resonators for Enhanced Performance. J. Appl. Phys. 2016, 119, 154501. [Google Scholar] [CrossRef]
- Naik, T.; Longmire, E.K.; Mantell, S.C. Dynamic Response of a Cantilever in Liquid near a Solid Wall. Sens. Actuators A Phys. 2003, 102, 240–254. [Google Scholar] [CrossRef]
- Sader, J.E. Frequency Response of Cantilever Beams Immersed in Viscous Fluids with Applications to the Atomic Force Microscope. J. Appl. Phys. 1998, 84, 64–76. [Google Scholar] [CrossRef]
- Maali, A.; Hurth, C.; Boisgard, R.; Jai, C.; Cohen-Bouhacina, T.; Aimé, J.-P. Hydrodynamics of Oscillating Atomic Force Microscopy Cantilevers in Viscous Fluids. J. Appl. Phys. 2005, 97, 074907. [Google Scholar] [CrossRef]
- Ekinci, K.L.; Yakhot, V.; Rajauria, S.; Colosqui, C.; Karabacak, D.M. High-Frequency Nanofluidics: A Universal Formulation of the Fluid Dynamics of MEMS and NEMS. Lab Chip 2010, 10, 3013–3025. [Google Scholar] [CrossRef]
- Wambsganss, M.W.; Chen, S.S.; Jendrzejczyk, A.J. Added Mass and Damping of a Vibrating Rod in Confined Viscous Fluids. J. Appl. Mech. 1976, 43, 325–329. [Google Scholar] [CrossRef][Green Version]
- Tuck, E.O. Calculation of Unsteady Flows Due to Small Motions of Cylinders in a Viscous Fluid. J. Eng. Math. 1969, 3, 29–44. [Google Scholar] [CrossRef]
- Chon, J.W.M.; Mulvaney, P.; Sader, J.E. Experimental Validation of Theoretical Models for the Frequency Response of Atomic Force Microscope Cantilever Beams Immersed in Fluids. J. Appl. Phys. 2000, 87, 3978–3988. [Google Scholar] [CrossRef]
- Green, C.P.; Sader, J.E. Torsional Frequency Response of Cantilever Beams Immersed in Viscous Fluids with Applications to the Atomic Force Microscope. J. Appl. Phys. 2002, 92, 6262–6274. [Google Scholar] [CrossRef]
- Green, C.P.; Sader, J.E. Frequency Response of Cantilever Beams Immersed in Viscous Fluids near a Solid Surface with Applications to the Atomic Force Microscope. J. Appl. Phys. 2005, 98, 114913. [Google Scholar] [CrossRef]
- Van Eysden, C.A.; Sader, J.E. Small Amplitude Oscillations of a Flexible Thin Blade in a Viscous Fluid: Exact Analytical Solution. Phys. Fluids 2006, 18, 123102. [Google Scholar] [CrossRef]
- Van Eysden, C.A.; Sader, J.E. Frequency Response of Cantilever Beams Immersed in Viscous Fluids with Applications to the Atomic Force Microscope: Arbitrary Mode Order. J. Appl. Phys. 2007, 101, 44908. [Google Scholar] [CrossRef]
- Abdolvand, R.; Bahreyni, B.; Lee, J.E.-Y.; Nabki, F. Micromachined Resonators: A Review. Micromachines 2016, 7, 160. [Google Scholar] [CrossRef] [PubMed]
- Labuda, A.; Kobayashi, K.; Kiracofe, D.; Suzuki, K.; Grütter, P.H.; Yamada, H. Comparison of Photothermal and Piezoacoustic Excitation Methods for Frequency and Phase Modulation Atomic Force Microscopy in Liquid Environments. AIP Adv. 2011, 1, 22136. [Google Scholar] [CrossRef]
- Asakawa, H.; Fukuma, T. Spurious-Free Cantilever Excitation in Liquid by Piezoactuator with Flexure Drive Mechanism. Rev. Sci. Instrum. 2009, 80, 103703. [Google Scholar] [CrossRef]
- Dufour, I.; Maali, A.; Amarouchene, Y.; Ayela, C.; Caillard, B.; Darwiche, A.; Guirardel, M.; Kellay, H.; Lemaire, E.; Mathieu, F.; et al. The Microcantilever: A Versatile Tool for Measuring the Rheological Properties of Complex Fluids. J. Sens. 2012, 2012, 1–9. [Google Scholar] [CrossRef]
- Pini, V.; Tiribilli, B.; Gambi, C.M.C.; Vassalli, M. Dynamical Characterization of Vibrating AFM Cantilevers Forced by Photothermal Excitation. Phys. Rev. B 2010, 81, 2–6. [Google Scholar] [CrossRef]
- Lee, J.O.; Choi, K.; Choi, S.-J.; Kang, M.-H.; Seo, M.-H.; Kim, I.-D.; Yu, K.; Yoon, J.-B. Nanomechanical Encoding Method Using Enhanced Thermal Concentration on a Metallic Nanobridge. ACS Nano 2017, 11, 7781–7789. [Google Scholar] [CrossRef]
- Zaghloul, U.; Piazza, G. Sub-1-Volt Piezoelectric Nanoelectromechanical Relays with Millivolt Switching Capability. IEEE Electron. Device Lett. 2014, 35, 669–671. [Google Scholar] [CrossRef]
- Rana, S.; Mouro, J.; Bleiker, S.J.; Reynolds, J.D.; Chong, H.M.; Niklaus, F.; Pamunuwa, D. Nanoelectromechanical Relay without Pull-in Instability for High-Temperature Non-Volatile Memory. Nat. Commun. 2020, 11, 1181–1910. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez, T.R.; Garcia, R. Theory of Q Control in Atomic Force Microscopy. Appl. Phys. Lett. 2003, 82, 4821–4823. [Google Scholar] [CrossRef]
- Moreno-Moreno, M.; Raman, A.; Gómez-Herrero, J.; Reifenberger, R. Parametric Resonance Based Scanning Probe Microscopy. Appl. Phys. Lett. 2006, 88, 193108. [Google Scholar] [CrossRef]
- Prakash, G.; Raman, A.; Rhoads, J.; Reifenberger, R.G. Parametric Noise Squeezing and Parametric Resonance of Microcantilevers in Air and Liquid Environments. Rev. Sci. Instrum. 2012, 83, 065109. [Google Scholar] [CrossRef]
- Prakash, G.; Hu, S.; Raman, A.; Reifenberger, R. Theoretical Basis of Parametric-Resonance-Based Atomic Force Microscopy. Phys. Rev. B 2009, 79, 094304. [Google Scholar] [CrossRef]
- Garcia, R. Dynamic Atomic Force Microscopy Methods. Surf. Sci. Rep. 2002, 47, 197–301. [Google Scholar] [CrossRef]
- Miller, J.M.; Shin, D.D.; Kwon, H.-K.; Shaw, S.W.; Kenny, T.W. Phase Control of Self-Excited Parametric Resonators. Phys. Rev. Appl. 2019, 12, 044053. [Google Scholar] [CrossRef]
- Mouro, J.; Tiribilli, B.; Paoletti, P. Nonlinear Behaviour of Self-Excited Microcantilevers in Viscous Fluids. J. Micromech. Microeng. 2017, 27, 095008. [Google Scholar] [CrossRef]
- Khalil, H.K. Nonlinear Systems, New International Edition; Pearson: London, UK, 2014; p. XVIII. [Google Scholar]
- Basso, M.; Paoletti, P.; Tiribilli, B.; Vassalli, M. Modelling and Analysis of Autonomous Micro-Cantilever Oscillations. Nanotechnology 2008, 19, 475501. [Google Scholar] [CrossRef]
- Basso, M.; Paoletti, P.; Tiribilli, B.; Vassalli, M. AFM Imaging via Nonlinear Control of Self-Driven Cantilever Oscillations. IEEE Trans. Nanotechnol. 2010, 10, 560–565. [Google Scholar] [CrossRef]
- Mouro, J.; Tiribilli, B.; Paoletti, P. A Versatile Mass-Sensing Platform with Tunable Nonlinear Self-Excited Microcantilevers. IEEE Trans. Nanotechnol. 2018, 17, 751–762. [Google Scholar] [CrossRef]
- Mouro, J.; Tiribilli, B.; Paoletti, P. Measuring Viscosity with Nonlinear Self-Excited Microcantilevers. Appl. Phys. Lett. 2017, 111, 144101. [Google Scholar] [CrossRef]
- Kim, W.; Kouh, T. Simple Optical Knife-Edge Effect Based Motion Detection Approach for a Microcantilever. Appl. Phys. Lett. 2020, 116, 163104. [Google Scholar] [CrossRef]
- Ulčinas, A.; Picco, L.M.; Berry, M.; Hörber, J.H.; Miles, M.J. Detection and Photothermal Actuation of Microcantilever Oscillations in Air and Liquid Using a Modified DVD Optical Pickup. Sens. Actuators A Phys. 2016, 248, 6–9. [Google Scholar] [CrossRef]
- Pooser, R.C.; Lawrie, B. Ultrasensitive Measurement of Microcantilever Displacement below the Shot-Noise Limit. Optica 2015, 2, 393–399. [Google Scholar] [CrossRef]
- Boisen, A.; Thundat, T. Design & Fabrication of Cantilever Array Biosensors. Mater. Today 2009, 12, 32–38. [Google Scholar] [CrossRef]
- Hwang, K.S.; Lee, S.-M.; Kim, S.K.; Lee, J.H.; Kim, T.S. Micro-and Nanocantilever Devices and Systems for Biomolecule Detection. Annu. Rev. Anal. Chem. 2009, 2, 77–98. [Google Scholar] [CrossRef]
- Arlett, J.; Myers, E.; Roukes, M.L. Comparative Advantages of Mechanical Biosensors. Nat. Nanotechnol. 2011, 6, 203–215. [Google Scholar] [CrossRef]
- Braind, O.; Dufour, I.; Heinrich, S.M.; Josse, F. Resonant MEMS—Fundamentals, Implementation and Application; Wiley-VCH Verlag: Weinheim, Germany, 2015. [Google Scholar]
- Sansa, M.; Sage, E.; Bullard, E.C.; Gély, M.; Alava, T.; Colinet, E.; Naik, A.K.; Villanueva, G.; Duraffourg, L.; Roukes, M.L.; et al. Frequency Fluctuations in Silicon Nanoresonators. Nat. Nanotechnol. 2016, 11, 552–558. [Google Scholar] [CrossRef]
- Feng, X.L.; White, C.J.; Hajimiri, A.; Roukes, M.L. A Self-Sustaining Ultrahigh-Frequency Nanoelectromechanical Oscillator. Nat. Nanotechnol. 2008, 3, 342–346. [Google Scholar] [CrossRef]
- Ekinci, K.L.; Yang, Y.T.; Roukes, M.L. Ultimate Limits to Inertial Mass Sensing Based upon Nanoelectromechanical Systems. J. Appl. Phys. 2004, 95, 2682–2689. [Google Scholar] [CrossRef]
- Vig, J.; Kim, Y. Noise in Microelectromechanical System Resonators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 1999, 46, 1558–1565. [Google Scholar] [CrossRef] [PubMed]
- IEEE Standard Definitions of Physical Quantities for Fundamental Frequency and Time Metrology-Random Instabilities in IEEE Std Std 1139-2008; IEEE: Piscataway, NJ, USA, 2009. [CrossRef]
- Riley, W.J. Handbook of Frequency Stability Analysis; NIST: Gaithersburg, MD, USA, 2008; p. 31. [Google Scholar]
- Pinto, R.M.R.; Brito, P.; Chu, V.; Conde, J.P. Thin-Film Silicon MEMS for Dynamic Mass Sensing in Vacuum and Air: Phase Noise, Allan Deviation, Mass Sensitivity and Limits of Detection. J. Microelectromech. Syst. 2019, 28, 390–400. [Google Scholar] [CrossRef]
- Rutman, J.; Walls, F. Characterization of Frequency Stability in Precision Frequency Sources. Proc. IEEE 1991, 79, 952–960. [Google Scholar] [CrossRef]
- Cutler, L.; Searle, C. Some Aspects of the Theory and Measurement of Frequency Fluctuations in Frequency Standards. Proc. IEEE 1966, 54, 136–154. [Google Scholar] [CrossRef]
- Rutman, J. ; Characterization of Frequency Stability: A Transfer Function Approach and Its Application to Measurements via Filtering of Phase Noise. IEEE Trans. Instrum. Meas. 1974, 23, 40–48. [Google Scholar] [CrossRef]
- Comment on “Characterization of Frequency Stability”. IEEE Trans. Instrum. Meas. 1972, 21, 85. [CrossRef]
- Allan, D.W. Time and Frequency Characterization Estimation and Prediction of Precision Clocks and Oscillators Allan IEEE Transaction on Ultrasonics Ferroelectrics. IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 1987, 34, 647–654. [Google Scholar] [CrossRef]
- Cleland, A.N.; Roukes, M.L. Noise Processes in Nanomechanical Resonators. J. Appl. Phys. 2002, 92, 2758–2769. [Google Scholar] [CrossRef]
- Yang, Y.T.; Callegari, C.; Feng, X.L.; Roukes, M.L. Surface Adsorbate Fluctuations and Noise in Nanoelectromechanical Systems. Nano Lett. 2011, 11, 1753–1759. [Google Scholar] [CrossRef]
- Chaste, J.; Eichler, A.; Moser, E.J.; Ceballos, G.; Rurali, R.; Bachtold, A. A Nanomechanical Mass Sensor with Yoctogram Resolution. Nat. Nanotechnol. 2012, 7, 301–304. [Google Scholar] [CrossRef] [PubMed]
- Naik, A.K.; Hanay, M.S.; Hiebert, W.K.; Feng, X.L.; Roukes, M.L. Towards Single-Molecule Nanomechanical Mass Spectrometry. Nat. Nanotechnol. 2009, 4, 445–450. [Google Scholar] [CrossRef] [PubMed]
- Hanay, M.S.; Kelber, S.; Naik, A.K.; Chi, D.; Hentz, S.; Bullard, E.C.; Colinet, E.; Duraffourg, L.; Roukes, M.L. Single-Protein Nanomechanical Mass Spectrometry in Real Time. Nat. Nanotechnol. 2012, 7, 602–608. [Google Scholar] [CrossRef] [PubMed]
- Domínguez-Medina, S.; Fostner, S.; Defoort, M.; Sansa, M.; Stark, A.-K.; Halim, M.A.; Vernhes, E.; Gely, M.; Jourdan, G.; Alava, T.; et al. Neutral Mass Spectrometry of Virus Capsids above 100 Megadaltons with Nanomechanical Resonators. Science 2018, 362, 918–922. [Google Scholar] [CrossRef]
- Gupta, A.; Akin, D.; Bashir, R. Single Virus Particle Mass Detection Using Microresonators with Nanoscale Thickness. Appl. Phys. Lett. 2004, 84, 1976–1978. [Google Scholar] [CrossRef]
- Then, D.; Vidic, A.; Ziegler, C. A Highly Sensitive Self-Oscillating Cantilever Array for the Quantitative and Qualitative Analysis of Organic Vapor Mixtures. Sens. Actuators B Chem. 2006, 117, 1–9. [Google Scholar] [CrossRef]
- Vančura, C.; Li, Y.; Lichtenberg, J.; Kirstein, K.-U.; Hierlemannb, A.; Josse, F. Liquid-Phase Chemical and Biochemical Detection Using Fully Integrated Magnetically Actuated Complementary Metal Oxide Semiconductor Resonant Cantilever Sensor Systems. Anal. Chem. 2007, 79, 1646–1654. [Google Scholar] [CrossRef]
- Li, M.; Tang, H.X.; Roukes, M.L. Ultra-Sensitive NEMS-Based Cantilevers for Sensing, Scanned Probe and Very High-Frequency Applications. Nat. Nanotechnol. 2007, 2, 114–120. [Google Scholar] [CrossRef]
- Zhou, X.; Wu, S.; Liu, H.; Wu, X.; Zhang, Q. Nanomechanical Label-Free Detection of Aflatoxin B1 Using a Microcantilever. Sens. Actuators B Chem. 2016, 226, 24–29. [Google Scholar] [CrossRef]
- Drummond, T.G.; Hill, M.G.; Barton, J.K. Electrochemical DNA Sensors. Nat. Biotechnol. 2003, 21, 1192–1199. [Google Scholar] [CrossRef]
- Conde, J.P.; Madaboosi, N.; Soares, R.R.G.; Fernandes, J.T.S.; Novo, P.; Moulas, G.; Chu, V. Lab-on-Chip Systems for Integrated Bioanalyses. Essays Biochem. 2016, 60, 121–131. [Google Scholar] [CrossRef] [PubMed]
- Alvarez, M.; Lechuga, L.M. Microcantilever-Based Platforms as Biosensing Tools. Analyst 2010, 135, 827–836. [Google Scholar] [CrossRef] [PubMed]
- Mathew, R.; Sankar, A.R. A Review on Surface Stress-Based Miniaturized Piezoresistive SU-8 Polymeric Cantilever Sensors. Nano. Micro Lett. 2018, 10, 1–41. [Google Scholar] [CrossRef] [PubMed]
- Pinto, R.M.R.; Chu, V.; Conde, J.P. Label-Free Biosensing of DNA in Microfluidics using Amorphous Silicon Capacitive Micro-Cantilevers. IEEE Sens. J. 2020, 20, 1. [Google Scholar] [CrossRef]
- Johnson, B.N.; Mutharasan, R. Biosensing Using Dynamic-Mode Cantilever Sensors: A Review. Biosens. Bioelectron. 2012, 32, 1–18. [Google Scholar] [CrossRef]
- Lachut, M.J.; Sader, J.E. Effect of Surface Stress on the Stiffness of Cantilever Plates. Phys. Rev. Lett. 2007, 99, 206102. [Google Scholar] [CrossRef]
- Karabalin, R.B.; Villanueva, G.; Matheny, M.H.; Sader, J.E.; Roukes, M.L. Stress-Induced Variations in the Stiffness of Micro- and Nanocantilever Beams. Phys. Rev. Lett. 2012, 108, 236101. [Google Scholar] [CrossRef]
- Sohi, A.N.; Nieva, P.M. Size-Dependent Effects of Surface Stress on Resonance Behavior of Microcantilever-Based Sensors. Sens. Actuators A Phys. 2018, 269, 505–514. [Google Scholar] [CrossRef]
- Tamayo, J.; Kosaka, P.M.; Ruz, J.J.; Paulo, A.S.; Calleja, M. Biosensors Based on Nanomechanical Systems. Chem. Soc. Rev. 2013, 42, 1287–1311. [Google Scholar] [CrossRef]
- Tamayo, J.; Ramos, D.; Mertens, J.; Calleja, M. Effect of the Adsorbate Stiffness on the Resonance Response of Microcantilever Sensors. Appl. Phys. Lett. 2006, 89, 224104. [Google Scholar] [CrossRef]
- Gil-Santos, E.; Ramos, D.; Martínez, J.; Fernández-Regúlez, M.; García, R.; San Paulo, Á.; Calleja, M.; Tamayo, J. Nanomechanical Mass Sensing and Stiffness Spectrometry Based on Two-Dimensional Vibrations of Resonant Nanowires. Nat. Nanotechnol. 2010, 5, 641–645. [Google Scholar] [CrossRef] [PubMed]
- Olcum, S.; Cermak, N.; Wasserman, C.; Manalis, S.R. High-speed multiple-mode mass-sensing resolves dynamic nanoscale mass distributions. Nat. Commun. 2015, 6, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Malvar, O.; Ruz, J.J.; Kosaka, P.M.; Domínguez, C.M.; Gil-Santos, E.; Calleja, M.; Tamayo, J. Mass and Stiffness Spectrometry of Nanoparticles and Whole Intact Bacteria by Multimode Nanomechanical Resonators. Nat. Commun. 2016, 7, 13452. [Google Scholar] [CrossRef] [PubMed]
- Dufour, I.; Heinrich, S.M.; Josse, F. Strong-Axis Bending Mode Vibrations for Resonant Microcantilever (Bio)Chemical Sensors in Gas or Liquid Phase. In Proceedings of the IEEE International Frequency Control Symposium and Exposition, Montreal, QC, Canada, 23–27 August 2004; pp. 193–199. [Google Scholar] [CrossRef]
- Thundat, T.; Warmack, R.J.; Chen, G.Y.; Allison, D.P. Thermal and Ambient-Induced Deflections of Scanning Force Microscope Cantilevers. Appl. Phys. Lett. 1994, 64, 2894–2896. [Google Scholar] [CrossRef]
- Gupta, A.; Akin, D.; Bashir, R. Detection of Bacterial Cells and Antibodies Using Surface Micromachined Thin Silicon Cantilever Resonators. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. 2004, 22, 2785. [Google Scholar] [CrossRef]
- Xie, H.; Vitard, J.; Haliyo, S.; Régnier, S. Enhanced Sensitivity of Mass Detection Using the First Torsional Mode of Microcantilevers. Meas. Sci. Technol. 2008, 19. [Google Scholar] [CrossRef]
- Ghatkesar, M.K.; Braun, T.; Barwich, V.; Ramseyer, J.-P.; Gerber, C.; Hegner, M.; Lang, H.P. Resonating Modes of Vibrating Microcantilevers in Liquid. Appl. Phys. Lett. 2008, 92, 043106. [Google Scholar] [CrossRef]
- Parkin, J.D.; Hähner, G. Mass Determination and Sensitivity Based on Resonance Frequency Changes of the Higher Flexural Modes of Cantilever Sensors. Rev. Sci. Instrum. 2011, 82, 35108. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhang, M.; Duan, X.; Zhang, H.; Pang, W. A Flexible, Gigahertz, and Free-Standing Thin Film Piezoelectric MEMS Resonator with High Figure of Merit. Appl. Phys. Lett. 2017, 111, 023505. [Google Scholar] [CrossRef]
- Yu, H.; Chen, Y.; Xu, P.; Xu, T.; Bao, Y.; Li, X. μ-‘Diving Suit’ for Liquid-Phase High-Q Resonant Detection. Lab Chip 2016, 16, 902–910. [Google Scholar] [CrossRef]
- Lassagne, B.; García-Sánchez, D.; Aguasca, A.; Bachtold, A. Ultrasensitive Mass Sensing with a Nanotube Electromechanical Resonator. Nano Lett. 2008, 8, 3735–3738. [Google Scholar] [CrossRef] [PubMed]
- Jensen, K.H.; Kim, K.; Zettl, A. An Atomic-Resolution Nanomechanical Mass Sensor. Nat. Nanotechnol. 2008, 3, 533–537. [Google Scholar] [CrossRef] [PubMed]
- Davis, Z.; Abadal, G.; Helbo, B.; Hansen, O.; Campabadal, F.; Pérez-Murano, F.; Esteve, J.; Figueras, E.; Verd, J.; Barniol, N.; et al. Monolithic Integration of Mass Sensing Nano-Cantilevers with CMOS Circuitry. Sens. Actuators A Phys. 2003, 105, 311–319. [Google Scholar] [CrossRef]
- Teva, J.; Abadal, G.; Torres, F.; Verd, J.; Pérez-Murano, F.; Barniol, N. A Femtogram Resolution Mass Sensor Platform, Based on SOI Electrostatically Driven Resonant Cantilever. Part I: Electromechanical Model and Parameter Extraction. Ultramicroscopy 2006, 106, 800–807. [Google Scholar] [CrossRef]
- Lu, J.; Ikehara, T.; Zhang, Y.; Mihara, T.; Itoh, T.; Maeda, R. Characterization and Improvement on Quality Factor of Microcantilevers with Self-Actuation and Self-Sensing Capability. Microelectron. Eng. 2009, 86, 1208–1211. [Google Scholar] [CrossRef]
- Narducci, M.; Figueras, E.; Lopez, M.J.; Gràcia, I.; Santander, J.; Ivanov, P.; Fonseca, L.; Cané, C. Sensitivity Improvement of a Microcantilever Based Mass Sensor. Microelectron. Eng. 2009, 86, 1187–1189. [Google Scholar] [CrossRef]
- Linden, J.; Thyssen, A.; Oesterschulze, E. Suspended Plate Microresonators with High Quality Factor for the Operation in Liquids. Appl. Phys. Lett. 2014, 104, 191906. [Google Scholar] [CrossRef]
- Badarlis, A.; Pfau, A.; Kalfas, A. Measurement and Evaluation of the Gas Density and Viscosity of Pure Gases and Mixtures Using a Micro-Cantilever Beam. Sensors 2015, 15, 24318–24342. [Google Scholar] [CrossRef]
- Gfeller, K.Y.; Nugaeva, N.; Hegner, M. Micromechanical Oscillators as Rapid Biosensor for the Detection of Active Growth of Escherichia Coli. Biosens. Bioelectron. 2005, 21, 528–533. [Google Scholar] [CrossRef]
- Ramos, D.; Mertens, J.; Calleja, M.; Tamayo, J. Phototermal Self-Excitation of Nanomechanical Resonators in Liquids. Appl. Phys. Lett. 2008, 92, 173108. [Google Scholar] [CrossRef]
- Villarroya, M.; Verd, J.; Teva, J.; Abadal, G.; Forsen, E.; Murano, F.P.; Uranga, A.; Figueras, E.; Montserrat, J.; Esteve, J.; et al. System on Chip Mass Sensor Based on Polysilicon Cantilevers Arrays for Multiple Detection. Sens. Actuators A Phys. 2006, 132, 154–164. [Google Scholar] [CrossRef]
- Verd, J.; Uranga, A.; Abadal, G.; Teva, J.L.; Torres, F.; López, J.; Pérez-Murano, F.; Esteve, J.; Barniol, N. Monolithic CMOS MEMS Oscillator Circuit for Sensing in the Attogram Range. IEEE Electron. Device Lett. 2008, 29, 146–148. [Google Scholar] [CrossRef]
- Jin, D.; Li, X.; Liu, J.; Zuo, G.; Wang, Y.; Liu, M.; Yu, H. High-Mode Resonant Piezoresistive Cantilever Sensors for Tens-Femtogram Resoluble Mass Sensing in Air. J. Micromech. Microeng. 2006, 16, 1017–1023. [Google Scholar] [CrossRef]
- Lochon, F.; Fadel, L.; Dufour, I.; Rebière, D.; Pistré, J. Silicon Made Resonant Microcantilever: Dependence of the Chemical Sensing Performances on the Sensitive Coating Thickness. Mater. Sci. Eng. C 2006, 26, 348–353. [Google Scholar] [CrossRef]
- Burg, T.P.; Godin, M.; Knudsen, S.M.; Shen, W.; Carlson, G.; Foster, J.S.; Babcock, K.; Manalis, S.R. Weighing of Biomolecules, Single Cells and Single Nanoparticles in Fluid. Nat. Cell Biol. 2007, 446, 1066–1069. [Google Scholar] [CrossRef]
- Ivaldi, P.; Abergel, J.; Matheny, M.H.; Villanueva, G.; Karabalin, R.B.; Roukes, M.L.; Andreucci, P.; Hentz, S.; Defaÿ, E. 50 nm Thick AlN Film-Based Piezoelectric Cantilevers for Gravimetric Detection. J. Micromech. Microeng. 2011, 21, 085023. [Google Scholar] [CrossRef]
- Vig, J.R.; Walls, F. A Review of Sensor Sensitivity and Stability. In Proceedings of the IEEE/EIA International Frequency Control Symposium and Exhibition. Institute of Electrical and Electronics Engineers (IEEE), Kansas City, MO, USA, 7–9 June 2000; pp. 30–33. [Google Scholar]
- Ahmed, N.; Nino, D.F.; Moy, V.T. Measurement of Solution Viscosity by Atomic Force Microscopy. Rev. Sci. Instrum. 2001, 72, 2731–2734. [Google Scholar] [CrossRef]
- Boskovic, S.; Chon, J.W.M.; Mulvaney, P.; Sader, J.E. Rheological Measurements Using Microcantilevers. J. Rheol. 2002, 46, 891. [Google Scholar] [CrossRef]
- Vančura, C.; Dufour, I.; Heinrich, S.M.; Josse, F.; Hierlemann, A. Analysis of Resonating Microcantilevers Operating in a Viscous Liquid Environment. Sens. Actuators A Phys. 2008, 141, 43–51. [Google Scholar] [CrossRef]
- Castille, C.; Dufour, I.; Lucat, C. Longitudinal Vibration Mode of Piezoelectric Thick-Film Cantilever-Based Sensors in Liquid Media. Appl. Phys. Lett. 2010, 96, 154102. [Google Scholar] [CrossRef]
- Youssry, M.; Belmiloud, N.; Caillard, B.; Ayela, C.; Pellet, C.; Dufour, I. A Straightforward Determination of Fluid Viscosity and Density Using Microcantilevers: From Experimental Data to Analytical Expressions. Sens. Actuators A Phys. 2011, 172, 40–46. [Google Scholar] [CrossRef]
- Dufour, I.; Lemaire, E.; Caillard, B.; Debéda, H.; Lucat, C.; Heinrich, S.; Josse, F.; Brand, O. Effect of Hydrodynamic Force on Microcantilever Vibrations: Applications to Liquid-Phase Chemical Sensing. Sens. Actuators B Chem. 2014, 192, 664–672. [Google Scholar] [CrossRef]
- Belmiloud, N.; Dufour, I.; Colin, A.; Nicu, L. Rheological Behavior Probed by Vibrating Microcantilevers. Appl. Phys. Lett. 2008, 92, 041907. [Google Scholar] [CrossRef]
- Iglesias, L.; Boudjiet, M.; Dufour, I. Discrimination and Concentration Measurement of Different Binary Gas Mixtures with a Simple Resonator through Viscosity and Mass Density Measurements. Sens. Actuators B Chem. 2019, 285, 487–494. [Google Scholar] [CrossRef]
- Liu, W.; Wu, C. Rheological Study of Soft Matters: A Review of Microrheology and Microrheometers. Macromol. Chem. Phys. 2017, 219, 1–10. [Google Scholar] [CrossRef]
- Waigh, T.A. Advances in the Microrheology of Complex Fluids. Rep. Prog. Phys. 2016, 79, 074601. [Google Scholar] [CrossRef]
- Garcia, R. Nanomechanical Mapping of Soft Materials with the Atomic Force Microscope: Methods, Theory and Applications. Chem. Soc. Rev. 2020, 49, 5850–5884. [Google Scholar] [CrossRef]
- Hecht, F.M.; Rheinlaender, J.; Schierbaum, N.; Goldmann, W.H.; Fabry, B.; Schäffer, T.E. Imaging Viscoelastic Properties of Live Cells by AFM: Power-Law Rheology on the Nanoscale. Soft Matter. 2015, 11, 4584–4591. [Google Scholar] [CrossRef]
- Efremov, Y.M.; Okajima, T.; Raman, A. Measuring Viscoelasticity of Soft Biological Samples Using Atomic Force Microscopy. Soft Matter. 2020, 16, 64–81. [Google Scholar] [CrossRef]
- Haviland, D.B.; Van Eysden, C.A.; Forchheimer, D.; Platz, D.; Kassa, H.G.; Leclère, P. Probing Viscoelastic Response of Soft Material Surfaces at the Nanoscale. Soft Matter. 2016, 12, 619–624. [Google Scholar] [CrossRef]
- Belmiloud, N.; Dufour, I.; Nicu, L.; Colin, A.; Pistre, J. Vibrating Microcantilever used as Viscometer and Microrheometer. In Proceedings of the 5th IEEE Conference on Sensors, Daegu, Korea, 22–25 October 2006; Volume 4, pp. 753–756. [Google Scholar] [CrossRef]
- Youssry, M.; Lemaire, E.; Caillard, B.; Colin, A.; Dufour, I. On-Chip Characterization of the Viscoelasticity of Complex Fluids Using Microcantilevers. Meas. Sci. Technol. 2012, 23, 125306. [Google Scholar] [CrossRef]
- Lemaire, E.; Heinisch, M.; Caillard, B.; Jakoby, B.; Dufour, I. Comparison and Experimental Validation of Two Potential Resonant Viscosity Sensors in the Kilohertz Range. Meas. Sci. Technol. 2013, 24, 084005. [Google Scholar] [CrossRef]
- Meister, A.; Gabi, M.; Behr, P.; Studer, P.; Vörös, J.; Niedermann, P.; Bitterli, J.; Polesel-Maris, J.; Liley, M.; Heinzelmann, H.; et al. FluidFM: Combining Atomic Force Microscopy and Nanofluidics in a Universal Liquid Delivery System for Single Cell Applications and Beyond. Nano Lett. 2009, 9, 2501–2507. [Google Scholar] [CrossRef] [PubMed]
- Van Hoorn, C.H.; Chavan, D.C.; Tiribilli, B.; Margheri, G.; Mank, A.J.G.; Ariese, F.; Iannuzzi, D. Opto-Mechanical Probe for Combining Atomic Force Microscopy and Optical Near-Field Surface Analysis. Opt. Lett. 2014, 39, 4800–4803. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mouro, J.; Pinto, R.; Paoletti, P.; Tiribilli, B. Microcantilever: Dynamical Response for Mass Sensing and Fluid Characterization. Sensors 2021, 21, 115. https://doi.org/10.3390/s21010115
Mouro J, Pinto R, Paoletti P, Tiribilli B. Microcantilever: Dynamical Response for Mass Sensing and Fluid Characterization. Sensors. 2021; 21(1):115. https://doi.org/10.3390/s21010115
Chicago/Turabian StyleMouro, João, Rui Pinto, Paolo Paoletti, and Bruno Tiribilli. 2021. "Microcantilever: Dynamical Response for Mass Sensing and Fluid Characterization" Sensors 21, no. 1: 115. https://doi.org/10.3390/s21010115
APA StyleMouro, J., Pinto, R., Paoletti, P., & Tiribilli, B. (2021). Microcantilever: Dynamical Response for Mass Sensing and Fluid Characterization. Sensors, 21(1), 115. https://doi.org/10.3390/s21010115






