Dielectric Spectroscopy and Application of Mixing Models Describing Dielectric Dispersion in Clay Minerals and Clayey Soils
Abstract
1. Introduction
- Free water, which exhibits bulk properties of a continuous background phase.
- Macroscopically confined water that exhibits bulk properties but is confined by the configuration of the solid, water and air phases, e.g., in aggregates or foams, as a discontinuous inclusion phase.
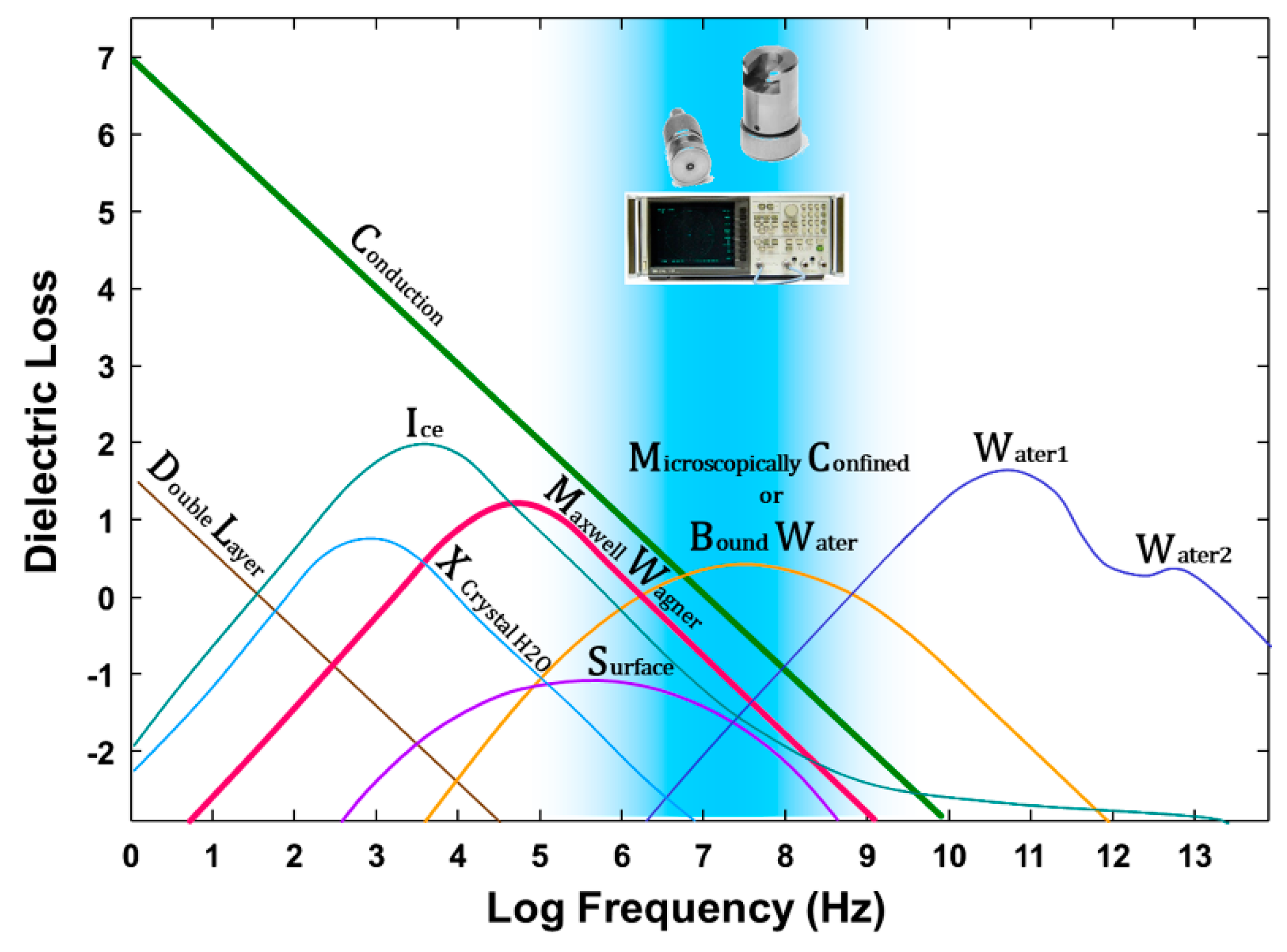
- Microscopically confined water where the dielectric response of the water is modified, for example, by:
- ○
- Extrinsic electric fields, including dipole forces, charged surfaces or hydration around ions.
- ○
- Extrinsic geometry, causing structural alteration due to being trapped, caged or structurally modified by proximity to a surface.
- Measure the dielectric dispersion of well-defined clay minerals and associated clayey soils, and to compare these with other unsaturated porous media where either macro- or microscopically confined water should be dominant.
- Use simple models to test the predictive capability of the geometrical modeling approach for water-saturated dispersive clayey soils.
- Compare the dispersive data for unsaturated clayey soils with the mixing model bounds we might expect for coarse granular materials.
2. Materials and Methods
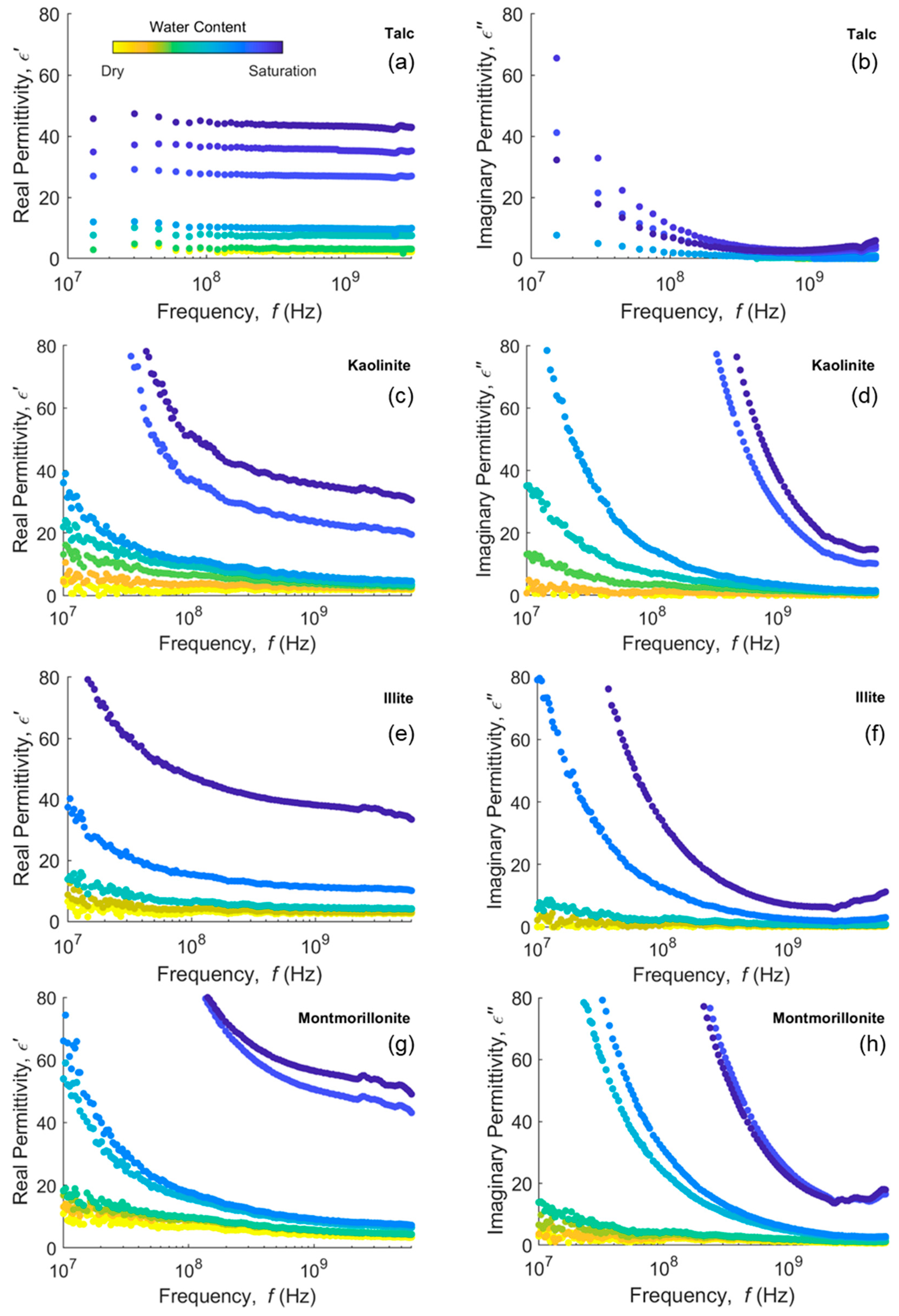
2.1. Clay Minerals
2.2. Clayey Soils
2.3. Vector Network Analyzer (VNA) Measurements
3. Theory and Modeling
4. Results and Discussion
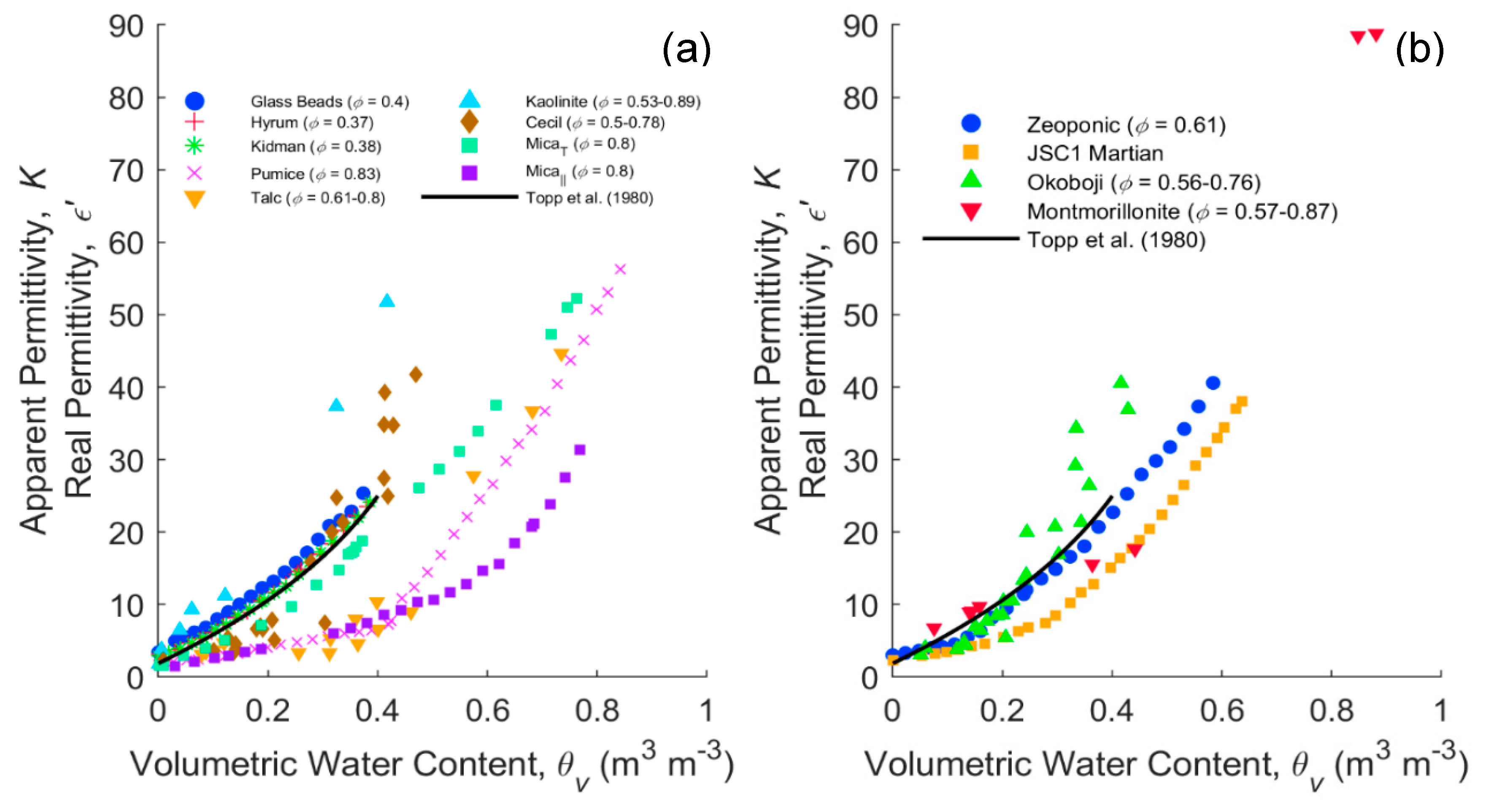
4.1. Clay Minerals and Clayey Soils Dispersion
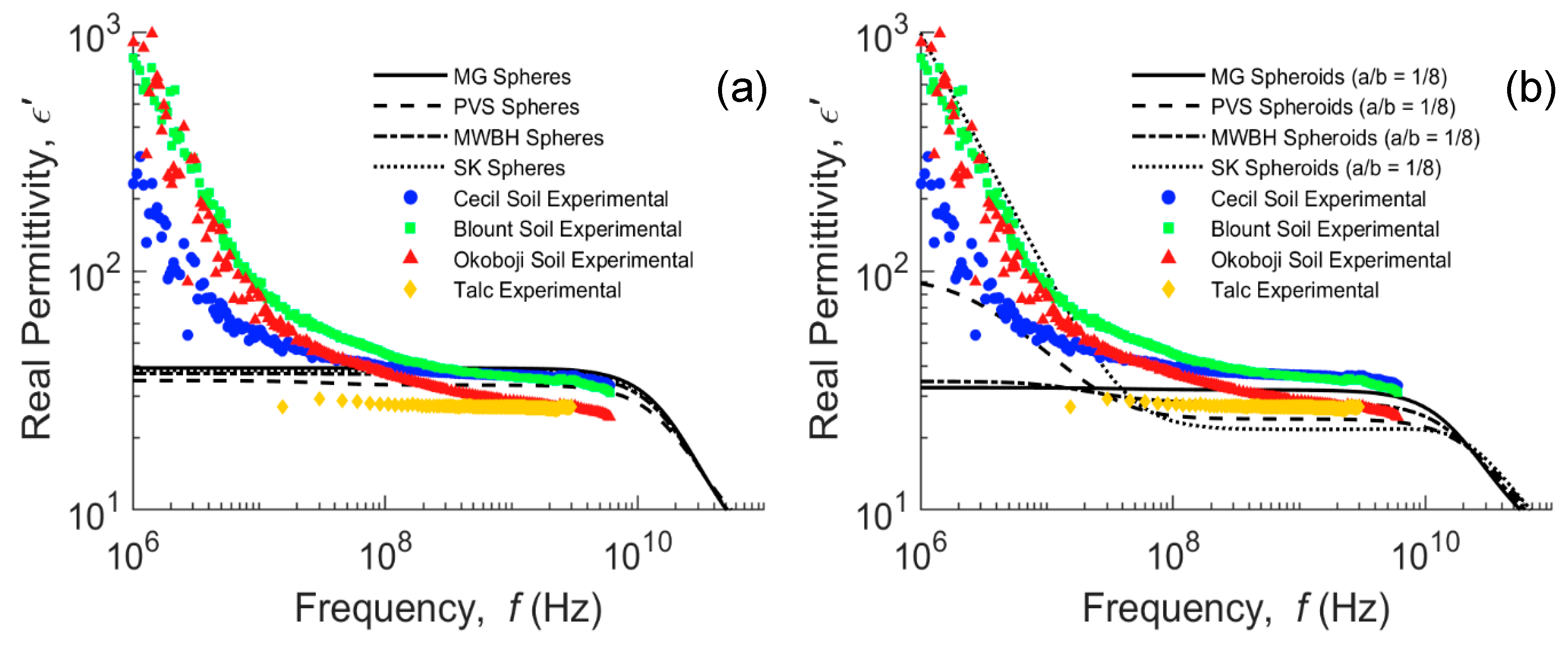
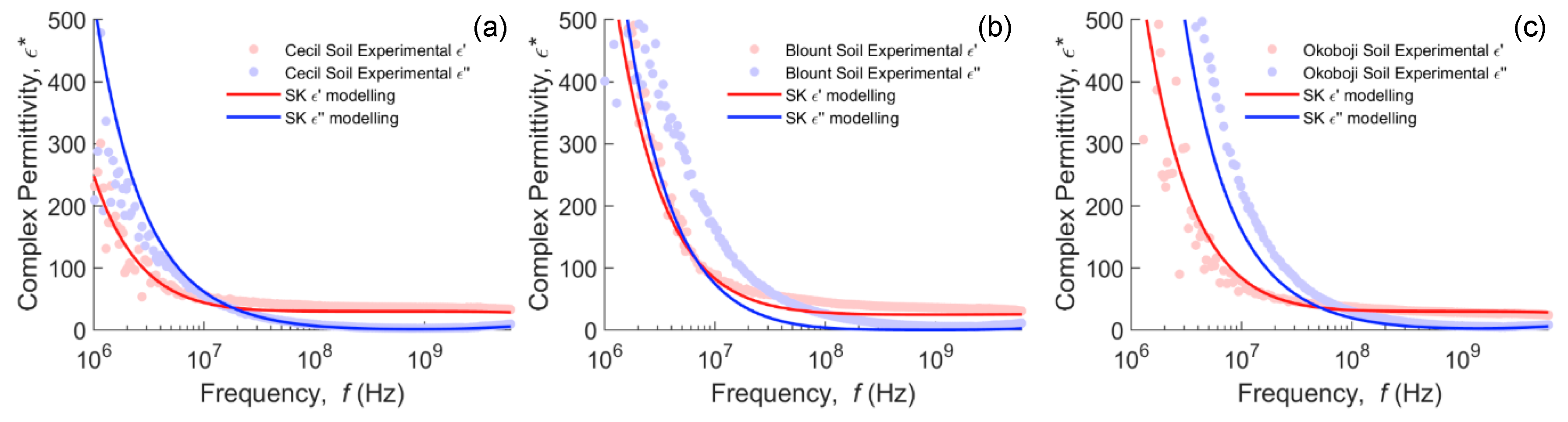
4.2. Modeling of Water-Saturated Clay Mineral Soils
4.3. Comparison of Media with Confined Water
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Topp, G.C.; Davis, J.L.; Annan, A.P. Electromagnetic determination of soil water content: Measurements in coaxial transmission lines. Water Resour. Res. 1980, 16, 574–582. [Google Scholar] [CrossRef]
- Bridge, B.J.; Sabburg, J.; Habash, K.O.; Ball, J.A.R.; Hancock, N.H. The dielectric behaviour of clay soils and its application to time domain reflectometry. Aust. J. Soil Res. 1996, 34, 825–835. [Google Scholar] [CrossRef]
- Dirksen, C.; Dasberg, S. Improved Calibration of Time Domain Reflectometry Soil Water Content Measurements. Soil Sci. Soc. Am. J. 1993, 57, 660–667. [Google Scholar] [CrossRef]
- Dobson, M.C.; Ulaby, F.T.; Hallikainen, M.T.; El-Rayes, M.A. Microwave Dielectric Behavior of Wet Soil-Part II: Dielectric Mixing Models. IEEE Trans. Geosci. Remote Sens. 1985, GE-23, 35–46. [Google Scholar] [CrossRef]
- Perdok, U.D.; Kroesbergen, B.; Hilhorst, M.A. Influence of gravimetric water content and bulk density on the dielectric properties of soil. Eur. J. Soil Sci. 1996, 47, 367–371. [Google Scholar] [CrossRef]
- Hallikainen, M.; Ulaby, F.; Dobson, M.; El-rayes, M.; Wu, L. Microwave Dielectric Behavior of Wet Soil-Part 1: Empirical Models and Experimental Observations. IEEE Trans. Geosci. Remote Sens. 1985, GE-23, 25–34. [Google Scholar] [CrossRef]
- Jones, S.B.; Friedman, S.P. Particle shape effects on the effective permittivity of anisotropic or isotropic media consisting of aligned or randomly oriented ellipsoidal particles. Water Resour. Res. 2000, 36, 2821–2833. [Google Scholar] [CrossRef]
- Jones, S.B.; Or, D. Surface area, geometrical and configurational effects on permittivity of porous media. J. Non-Cryst. Solids 2002, 305, 247–254. [Google Scholar] [CrossRef]
- Cosenza, P.; Camerlynck, C.; Tabbagh, A. Differential effective medium schemes for investigating the relationship between high-frequency relative dielectric permittivity and water content of soils. Water Resour. Res. 2003, 39, 9. [Google Scholar] [CrossRef]
- Friedman, S.P. A saturation degree-dependent composite spheres model for describing the effective dielectric constant of unsaturated porous media. Water Resour. Res. 1998, 34, 2949–2961. [Google Scholar] [CrossRef]
- Robinson, D.A.; Friedman, S.P. Effect of particle size distribution on the effective dielectric permittivity of saturated granular media. Water Resour. Res. 2001, 37, 33–40. [Google Scholar] [CrossRef]
- Blonquist, J.M.; Jones, S.B.; Lebron, I.; Robinson, D.A. Microstructural and phase configurational effects determining water content: Dielectric relationships of aggregated porous media. Water Resour. Res. 2006, 42, 5. [Google Scholar] [CrossRef]
- Wagner, N.; Scheuermann, A. On the relationship between matric potential and dielectric properties of organic free soils: A sensitivity study. Can. Geotech. J. 2009, 46, 1202–1215. [Google Scholar] [CrossRef]
- Robinson, D.A.; Cooper, J.D.; Gardner, C.M.K. Modelling the relative permittivity of soils using soil hygroscopic water content. J. Hydrol. 2002, 255, 39–49. [Google Scholar] [CrossRef]
- Belyaeva, T.A.; Bobrov, P.P.; Kroshka, E.S.; Repin, A.V. Complex dielectric permittivity of saline soils and rocks at frequencies from 10 kHz to 8 GHz. In Proceedings of the Progress in Electromagnetics Research Symposium, St. Petersburg, Russia, 22–25 May 2017; pp. 3046–3051. [Google Scholar]
- Dyck, M.; Miyamoto, T.; Iwata, Y.; Kameyama, K. Bound Water, Phase Configuration, and Dielectric Damping Effects on TDR-Measured Apparent Permittivity. Vadose Zone J. 2019, 18. [Google Scholar] [CrossRef]
- He, H.; Dyck, M. Application of Multiphase Dielectric Mixing Models for Understanding the Effective Dielectric Permittivity of Frozen Soils. Vadose Zone J. 2013, 12. [Google Scholar] [CrossRef]
- Loewer, M.; Igel, J.; Minnich, C.; Wagner, N. Electrical and Dielectric Properties of Soils in the mHz to GHz Frequency Range. In Proceedings of the 11th International Conference on Electromagnetic Wave Interaction with Water and Moist Substances (ISEMA), Florence, Italy, 23–27 May 2016. [Google Scholar]
- Mironov, V.L.; Karavayskiy, A.Y.; Lukin, Y.I.; Molostov, I.P. A dielectric model of thawed and frozen Arctic soils considering frequency, temperature, texture and dry density. Int. J. Remote Sens. 2020, 41, 3845–3865. [Google Scholar] [CrossRef]
- Park, C.H.; Montzka, C.; Jagdhuber, T.; Jonard, F.; De Lannoy, G.; Hong, J.; Jackson, T.J.; Wulfmeyer, V. A dielectric mixing model accounting for soil organic matter. Vadose Zone J. 2019, 18, 190036. [Google Scholar] [CrossRef]
- Teschke, O.; Ceotto, G.; de Souza, E.F. Interfacial water dielectric-permittivity-profile measurements using atomic force microscopy. Phys. Rev. E Stat. Phys. Plasmas Fluids Relat. Interdiscip. Top. 2001, 64, 10. [Google Scholar] [CrossRef]
- Thorp, J.M. The dielectric behaviour of vapours adsorbed on porous solids. Trans. Faraday Soc. 1959, 55, 442–454. [Google Scholar] [CrossRef]
- Bockris, J.O.; Devanathan, M.A.V.; Müller, K. On the structure of charged interfaces. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. R. Soc. 1963, 274, 55–79. [Google Scholar]
- Sposito, G.; Prost, R. Structure of Water Adsorbed on Smectites. Chem. Rev. 1982, 82, 553–573. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and absorption in dielectrics I. Alternating current characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef]
- McBride, M.B.; Baveye, P. Mobility of Anion Spin Probes in Hectorite Gels: Viscosity of Surficial Water. Soil Sci. Soc. Am. J. 1995, 59, 388–394. [Google Scholar] [CrossRef]
- Pashley, R.M.; Israelachvili, J.N. Molecular layering of water in thin films between mica surfaces and its relation to hydration forces. J. Colloid Interface Sci. 1984, 101, 511–523. [Google Scholar] [CrossRef]
- Raviv, U.; Laurat, P.; Klein, J. Fluidity of water confined to subnanometre films. Nature 2001, 413, 51–54. [Google Scholar] [CrossRef]
- Mulla, D.J.; Cushman, J.H.; Low, P.F. Molecular Dynamics and Statistical Mechanics of Water Near an Uncharged Silicate Surface. Water Resour. Res. 1984, 20, 619–628. [Google Scholar] [CrossRef]
- Bonthuis, D.J.; Gekle, S.; Netz, R.R. Profile of the static permittivity tensor of water at interfaces: Consequences for capacitance, hydration interaction and ion adsorption. Langmuir 2012, 28, 7679–7694. [Google Scholar] [CrossRef]
- Hasted, J.B.; Ritson, D.M.; Collie, C.H. Dielectric properties of aqueous ionic solutions. Parts I and II. J. Chem. Phys. 1948, 16, 1–21. [Google Scholar] [CrossRef]
- Grahame, D.C. Effects of dielectric saturation upon the diffuse double layer and the free energy of hydration of ions. J. Chem. Phys. 1950, 18, 903–909. [Google Scholar] [CrossRef]
- Gordillo, M.C.; Martí, J. Hydrogen bond structure of liquid water confined in nanotubes. Chem. Phys. Lett. 2000, 329, 341–345. [Google Scholar] [CrossRef]
- Mashl, R.J.; Joseph, S.; Aluru, N.R.; Jakobsson, E. Anomalously immobilized water: A new water phase induced by confinement in nanotubes. Nano Lett. 2003, 3, 589–592. [Google Scholar] [CrossRef]
- Thompson, W.H. Perspective: Dynamics of confined liquids. J. Chem. Phys. 2018, 149, 170901. [Google Scholar] [CrossRef] [PubMed]
- Robinson, D.A.; Friedman, S.P. Electrical conductivity and dielectric permittivity of sphere packings: Measurements and modelling of cubic lattices, randomly packed monosize spheres and multi-size mixtures. Phys. A Stat. Mech. Appl. 2005, 358, 447–465. [Google Scholar] [CrossRef]
- Sen, P.N.; Scala, C.; Cohen, M.H. A self-similar model for sedimentary rocks with application to the dielectric constant of fused glass beads. Geophysics 1981, 46, 781–795. [Google Scholar] [CrossRef]
- Maxwell Garnett, J.C. Colours in Metal Glasses and in Metallic Films. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1904, 203, 385–420. [Google Scholar]
- Doyle, T.E.; Tew, A.T.; Jain, R.; Robinson, D.A. Effects of aggregation on the permittivity of random media containing monodisperse spheres. J. Appl. Phys. 2009, 106, 114104. [Google Scholar] [CrossRef]
- Hasted, J.B. Aqueous Dielectrics; Chapman and Hall Ltd.: London, UK, 1973. [Google Scholar]
- Davidson, D.W.; Cole, R.H. Dielectric Relaxation in Glycerine. J. Chem. Phys. 1950, 18, 1417. [Google Scholar] [CrossRef]
- Davidson, D.W.; Cole, R.H. Dielectric relaxation in glycerol, propylene glycol, and n-propanol. J. Chem. Phys. 1951, 19, 1484–1490. [Google Scholar] [CrossRef]
- Debye, P. Polar Molecules; Dover Publications, Inc.: New York, NY, USA, 1929. [Google Scholar]
- Havriliak, S.; Negami, S. A complex plane representation of dielectric and mechanical relaxation processes in some polymers. Polymer 1967, 8, 161–210. [Google Scholar] [CrossRef]
- Tyč, S.; Schwartz, L.M.; Sen, P.N.; Wong, P.Z. Geometrical models for the high-frequency dielectric properties of brine saturated sandstones. J. Appl. Phys. 1988, 64, 2575–2582. [Google Scholar] [CrossRef]
- Chen, Y.; Or, D. Geometrical factors and interfacial processes affecting complex dielectric permittivity of partially saturated porous media. Water Resour. Res. 2006, 42. [Google Scholar] [CrossRef]
- Haslund, E.; Hansen, B.D.; Hilfer, R.; Nøst, B. Measurement of local porosities and dielectric dispersion for a water-saturated porous medium. J. Appl. Phys. 1994, 76, 5473–5480. [Google Scholar] [CrossRef]
- Wagner, N.; Scheuermann, A.; Schwing, M.; Bonitz, F.; Kupfer, K. On the coupled hydraulic and dielectric material properties of soils: Combined numerical and experimental investigations. In Proceedings of the 9th International Conference on Electromagnetic Wave Interaction with Water and Moist Substances, Kansas City, MO, USA, 31 May–3 June 2011; pp. 152–161. [Google Scholar]
- Wagner, N.; Schwing, M.; Scheuermann, A. Numerical 3-D FEM and Experimental Analysis of the Open-Ended Coaxial Line Technique for Microwave Dielectric Spectroscopy on Soil. IEEE Trans. Geosci. Remote Sens. 2014, 52, 880–893. [Google Scholar] [CrossRef]
- Hanai, T. Dielectric Theory on the Interfacial Polarization for Two-Phase Mixtures. Bull. Inst. Chem. Res. Kyoto Univ. 1962, 39, 341–367. [Google Scholar]
- Maxwell, J.C. A Treatise on Electricity and Magnetism, 2nd ed.; Clarendon Press: Oxford, UK, 1881. [Google Scholar]
- Sillars, R.W. The properties of a dielectric containing semiconducting particles of various shapes. J. Inst. Electr. Eng. 1937, 80, 378–394. [Google Scholar]
- Von Hippel, A.R. Dielectrics and Waves, 2nd ed.; MIT Press: Cambridge, MA, USA, 1954. [Google Scholar]
- Wagner, K.W. Erklärung der dielektrischen Nachwirkungsvorgänge auf Grund Maxwellscher Vorstellungen. Arch. Für Elektrotechnik 1914, 2, 371–387. [Google Scholar] [CrossRef]
- Lauer, K.; Wagner, N.; Felix-Henningsen, P. A new technique for measuring broadband dielectric spectra of undisturbed soil samples. Eur. J. Soil Sci. 2012, 63, 224–238. [Google Scholar] [CrossRef]
- Robinson, D.A. Measurement of the Solid Dielectric Permittivity of Clay Minerals and Granular Samples Using a Time Domain Reflectometry Immersion Method. Vadose Zone J. 2004, 3, 705–713. [Google Scholar] [CrossRef]
- Robinson, D.A.; Friedman, S.P. A method for measuring the solid particle permittivity or electrical conductivity of rocks, sediments, and granular materials. J. Geophys. Res. Solid Earth 2003, 108. [Google Scholar] [CrossRef]
- Mishra, P.N.; Bore, T.; Scheuermann, A.; Li, L. Characterisation of pore fluid salinity dependent evaporative dewatering of kaolin using dielectric spectroscopy. J. Rock Mech. Geotech. Eng. 2020, 12, 112–125. [Google Scholar] [CrossRef]
- Hewlett-Packard User’s Manual HP 85070B Dielectric Probe Kit. Available online: http://literature.cdn.keysight.com/litweb/pdf/85070-90009.pdf (accessed on 1 November 2020).
- Sihvola, A.H. Electromagnetic Mixing Formulas and Applications; Michael Faraday House: Stevenage, UK, 1999. [Google Scholar]
- Sangani, A.S.; Acrivos, A. The Effective Conductivity of a Periodic Array of Spheres. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1983, 386, 263–275. [Google Scholar]
- Bruggeman, D.A.G. Berechnung verschiedener physikalischer Konstanten von heterogenen Substanzen. I. Dielektrizitätskonstanten und Leitfähigkeiten der Mischkörper aus isotropen Substanzen. Ann. Phys. 1935, 416, 636–664. [Google Scholar] [CrossRef]
- Cosenza, P.; Ghorbani, A.; Camerlynck, C.; Rejiba, F.; Guérin, R.; Tabbagh, A. Effective medium theories for modelling the relationships between electromagnetic properties and hydrological variables in geomaterials: A review. Near Surf. Geophys. 2009, 7, 563–578. [Google Scholar] [CrossRef]
- Asami, K. Characterization of heterogeneous systems by dielectric spectroscopy. Prog. Polym. Sci. 2002, 27, 1617–1659. [Google Scholar] [CrossRef]
- Boned, C.; Peyrelasse, J. Some comments on the complex permittivity of ellipsoids dispersed in continuum media. J. Phys. D Appl. Phys. 1983, 16, 1777–1784. [Google Scholar] [CrossRef]
- Friedman, S.P.; Robinson, D.A. Particle shape characterization using angle of repose measurements for predicting the effective permittivity and electrical conductivity of saturated granular media. Water Resour. Res. 2002, 38, 1236. [Google Scholar] [CrossRef]
- Sihvola, A.H.; Kong, J.A. Effective permittivity of dielectric mixtures. IEEE Trans. Geosci. Remote Sens. 1988, 26, 420–429. [Google Scholar] [CrossRef]
- Polder, D.; van Santen, J.H. The effective permeability of mixtures of solids. Physica 1946, 12, 257–271. [Google Scholar] [CrossRef]
- Kaatze, U. The dielectric properties of water in its different states of interaction. J. Solut. Chem. 1997, 26, 1049–1112. [Google Scholar] [CrossRef]
- Kaatze, U. Complex permittivity of water as a function of frequency and temperature. J. Chem. Eng. Data 1989, 34, 371–374. [Google Scholar] [CrossRef]
- Endres, A.L. Size scale considerations in modelling the electrical conductivity of porous rocks and soils. Explor. Geophys. 2000, 31, 418–423. [Google Scholar] [CrossRef]
- Endres, A.L.; Bertrand, E.A. A pore-size scale model for the dielectric properties of water-saturated clean rocks and soils. Geophysics 2006, 71, F185–F193. [Google Scholar] [CrossRef]
- Garboczi, E.J.; Snyder, K.A.; Douglas, J.F.; Thorpe, M.F. Geometrical percolation threshold of overlapping ellipsoids. Phys. Rev. E 1995, 52, 819–828. [Google Scholar] [CrossRef] [PubMed]
- Rowlands, W.N.; O’brien, R.W. The dynamic mobility and dielectric response of kaolinite particles. J. Colloid Interface Sci. 1995, 175, 190–200. [Google Scholar] [CrossRef]
- Calvet, R. Absorption dipolaire et conductivité de l’eau adsorbée sur la montmorillonite calcique. In Proceedings of the International Clay Conference, Madrid, Spain, 23–30 June 1972; Volume 2, pp. 519–528. [Google Scholar]
- Blonquist, J.M.; Robinson, D.A.; Humphries, S.D.; Jones, S.B. Improved Dielectric and Electrical Conductivity Anisotropy Measurements Using TDR in Unsaturated Mica. Vadose Zone J. 2011, 10, 1097–1104. [Google Scholar] [CrossRef]
- Robinson, D.A.; Jones, S.B.; Blonquist, J.M.; Friedman, S.P. A Physically Derived Water Content/Permittivity Calibration Model for Coarse-Textured, Layered Soils. Soil Sci. Soc. Am. J. 2005, 69, 1372–1378. [Google Scholar] [CrossRef]
- Robinson, D.A.; Jones, S.B.; Blonquist, J.M.; Heinse, R.; Lebron, I.; Doyle, T.E. The Dielectric Response of the Tropical Hawaiian Mars Soil Simulant JSC Mars-1. Soil Sci. Soc. Am. J. 2009, 73, 1113–1118. [Google Scholar] [CrossRef]
- Weast, R.C.; Astel, M.J.; Beyer, W.H. Handbook of Chemistry and Physics, 67th ed.; CRC Press Inc.: Boca Ratón, FL, USA, 1986. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
González-Teruel, J.D.; Jones, S.B.; Soto-Valles, F.; Torres-Sánchez, R.; Lebron, I.; Friedman, S.P.; Robinson, D.A. Dielectric Spectroscopy and Application of Mixing Models Describing Dielectric Dispersion in Clay Minerals and Clayey Soils. Sensors 2020, 20, 6678. https://doi.org/10.3390/s20226678
González-Teruel JD, Jones SB, Soto-Valles F, Torres-Sánchez R, Lebron I, Friedman SP, Robinson DA. Dielectric Spectroscopy and Application of Mixing Models Describing Dielectric Dispersion in Clay Minerals and Clayey Soils. Sensors. 2020; 20(22):6678. https://doi.org/10.3390/s20226678
Chicago/Turabian StyleGonzález-Teruel, Juan D., Scott B. Jones, Fulgencio Soto-Valles, Roque Torres-Sánchez, Inmaculada Lebron, Shmulik P. Friedman, and David A. Robinson. 2020. "Dielectric Spectroscopy and Application of Mixing Models Describing Dielectric Dispersion in Clay Minerals and Clayey Soils" Sensors 20, no. 22: 6678. https://doi.org/10.3390/s20226678
APA StyleGonzález-Teruel, J. D., Jones, S. B., Soto-Valles, F., Torres-Sánchez, R., Lebron, I., Friedman, S. P., & Robinson, D. A. (2020). Dielectric Spectroscopy and Application of Mixing Models Describing Dielectric Dispersion in Clay Minerals and Clayey Soils. Sensors, 20(22), 6678. https://doi.org/10.3390/s20226678





