Hybrid Sensor Device for Simultaneous Surface Plasmon Resonance and Surface Acoustic Wave Measurements
Abstract
1. Introduction
2. Materials and Methods
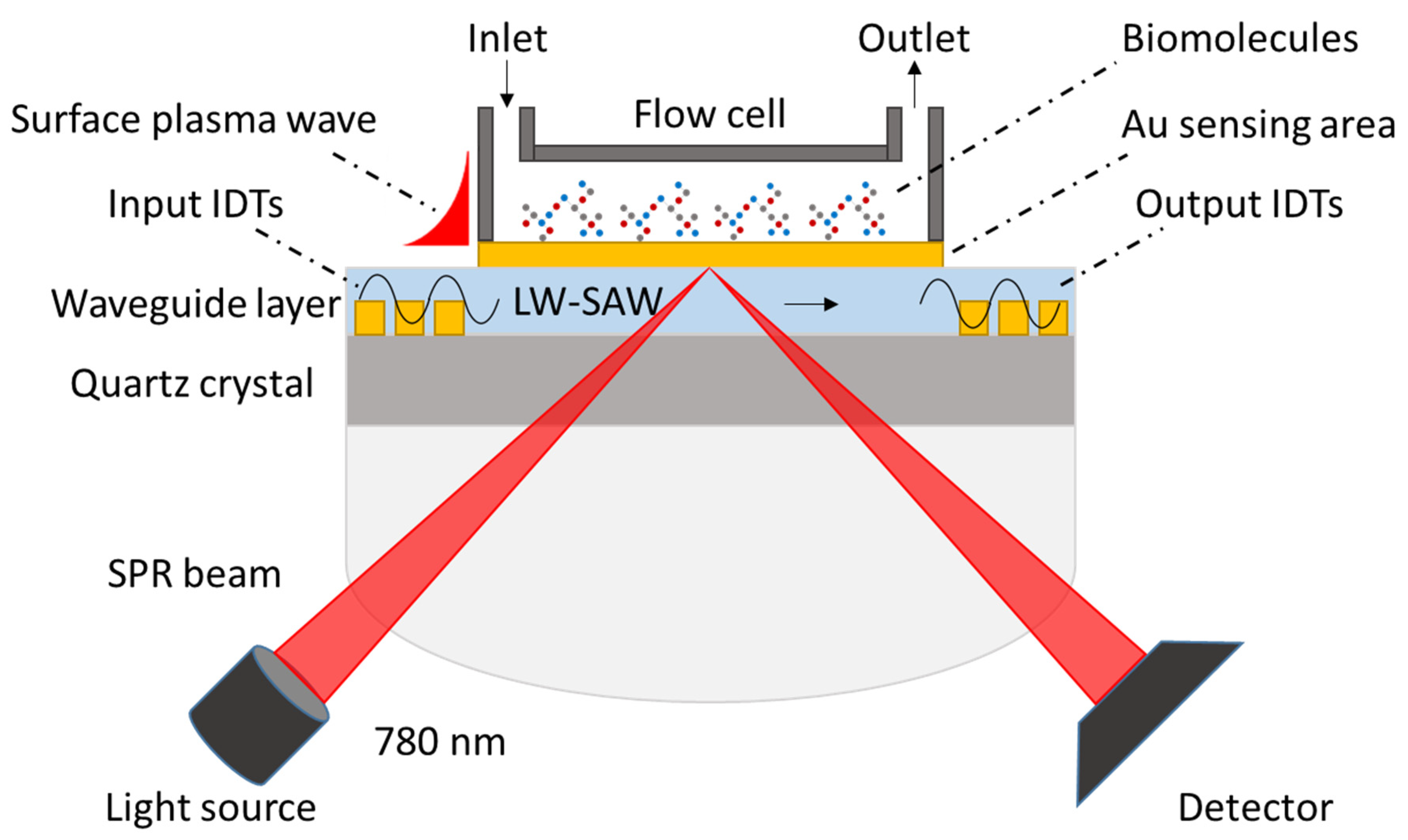
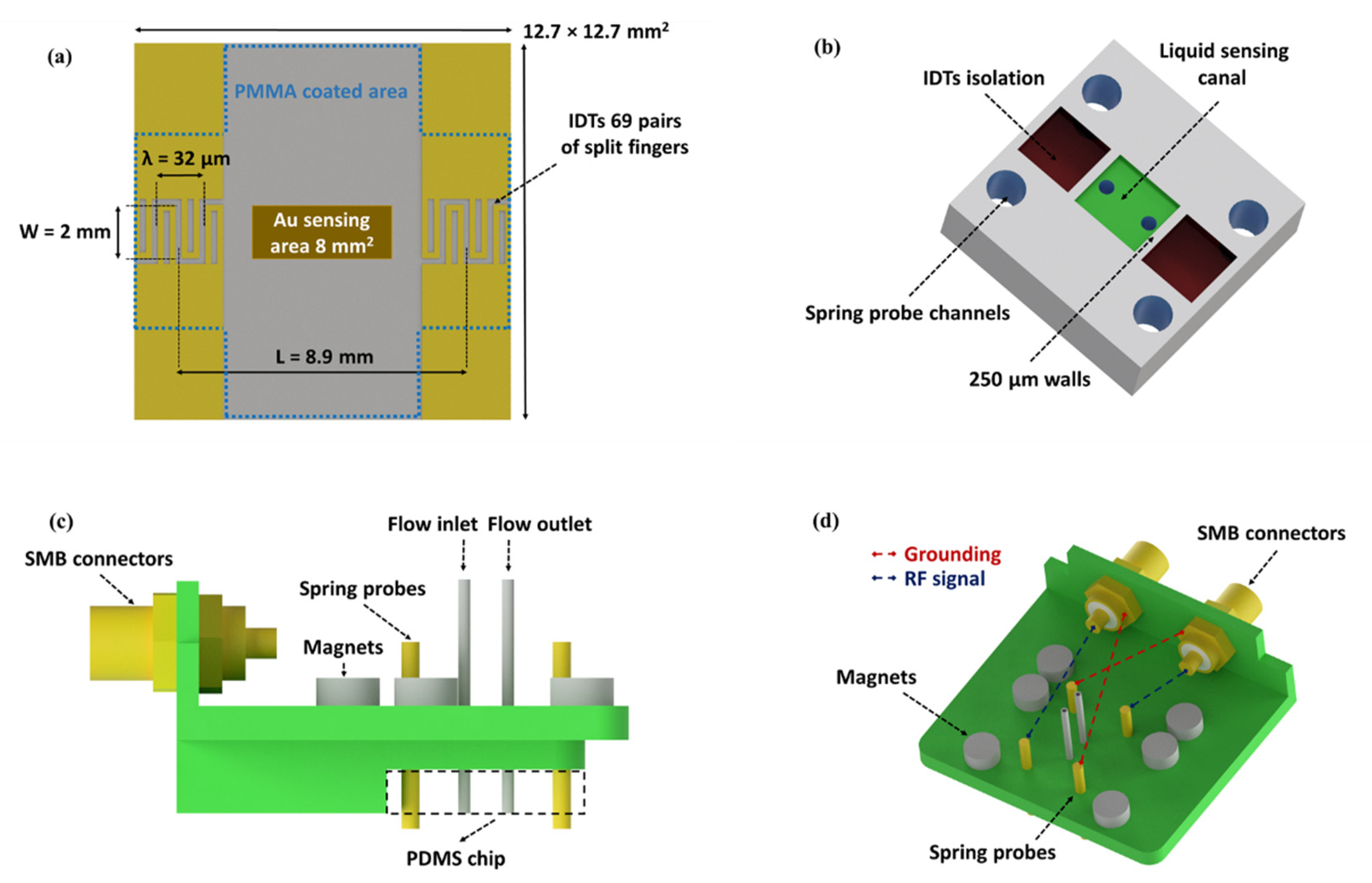
2.1. SPR/LW-SAW Sensor Device
2.2. PMMA Guiding Layer
2.3. Au Sensing Area Sputter Coating
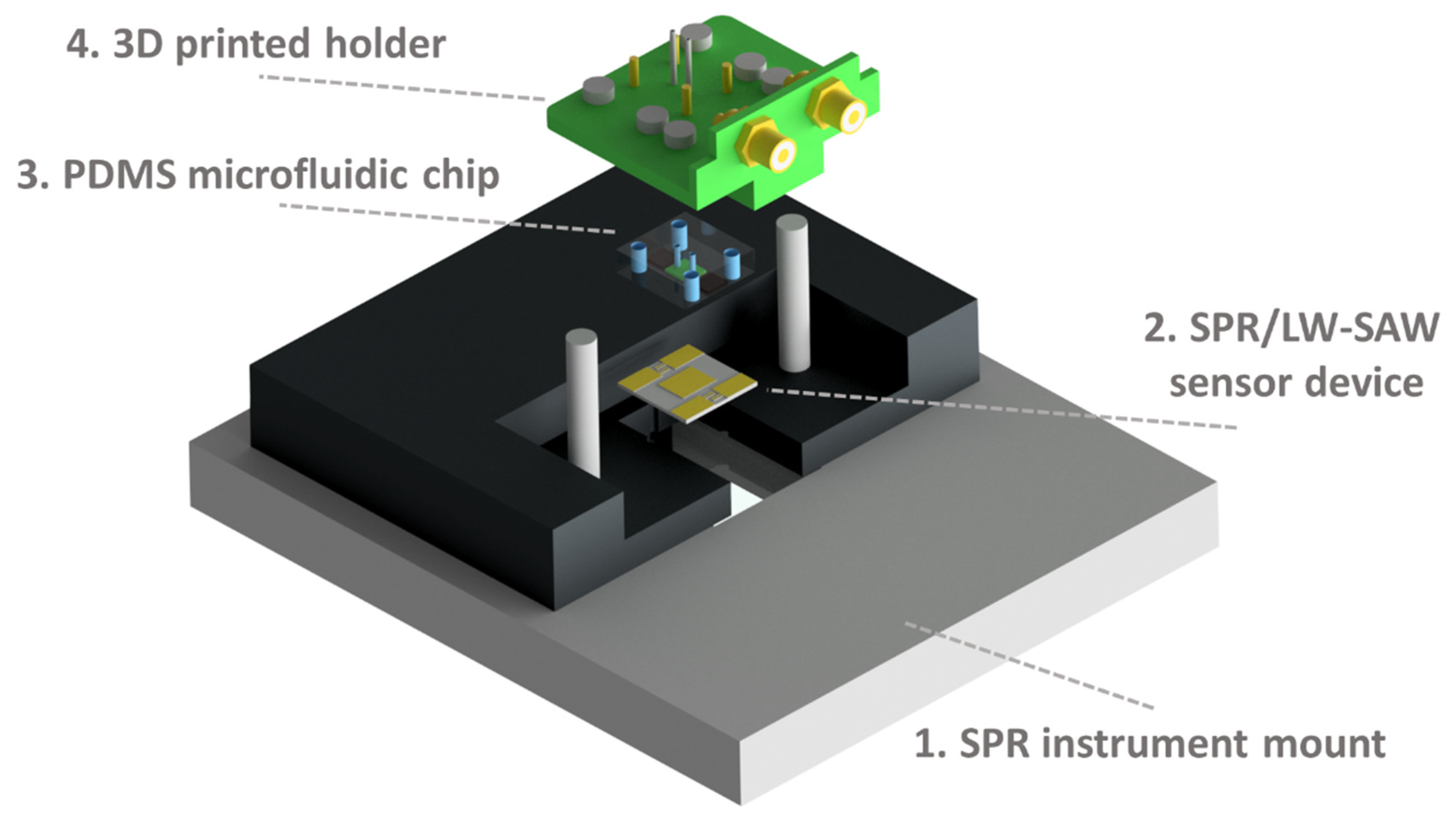
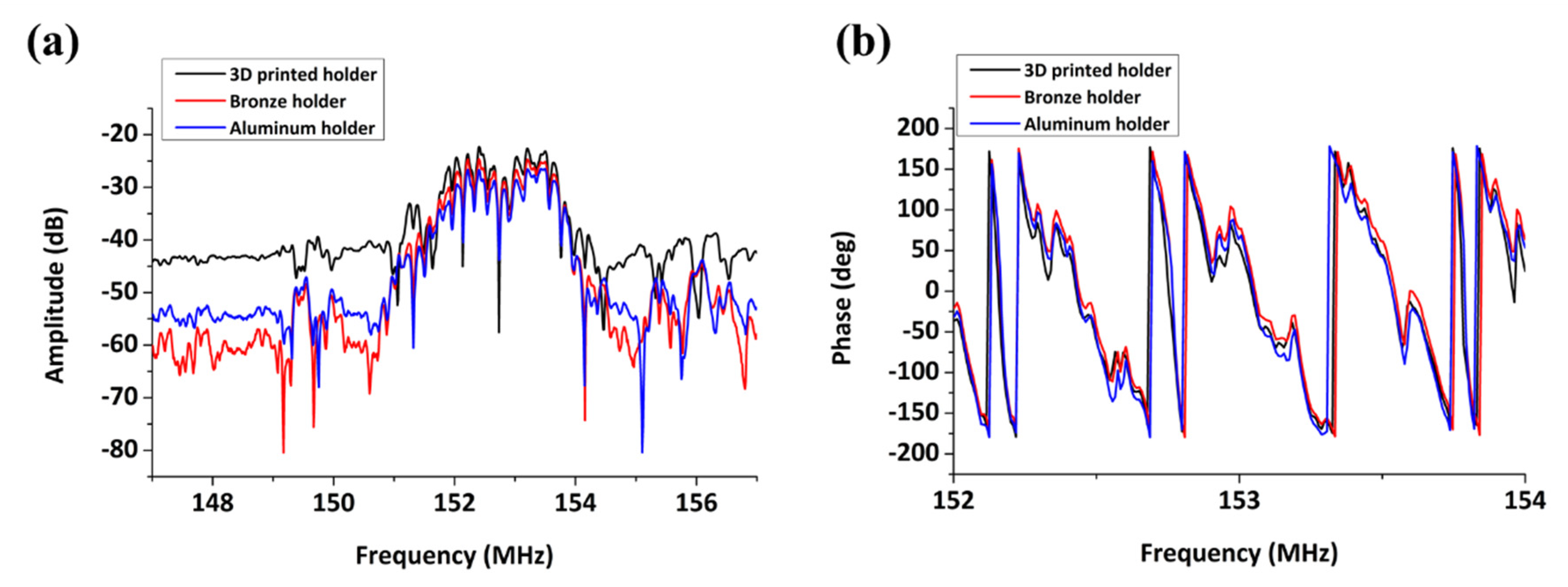
2.4. 3D-Printed Holder
2.5. PDMS Microfluidics
2.6. Assembly and Instrumentation
2.7. SPR/LW-SAW Device for Liquid Studies
3. Results and Discussion
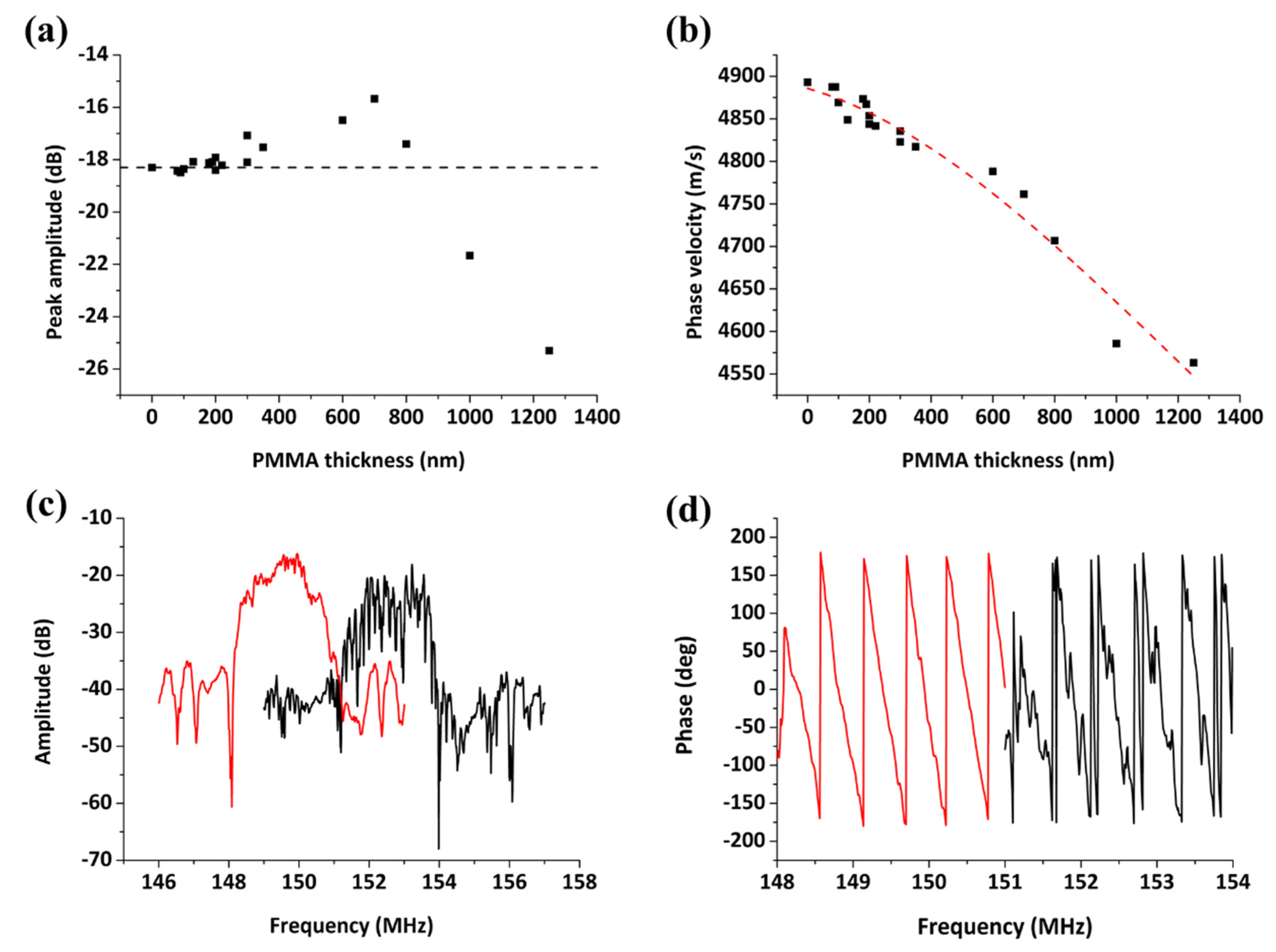
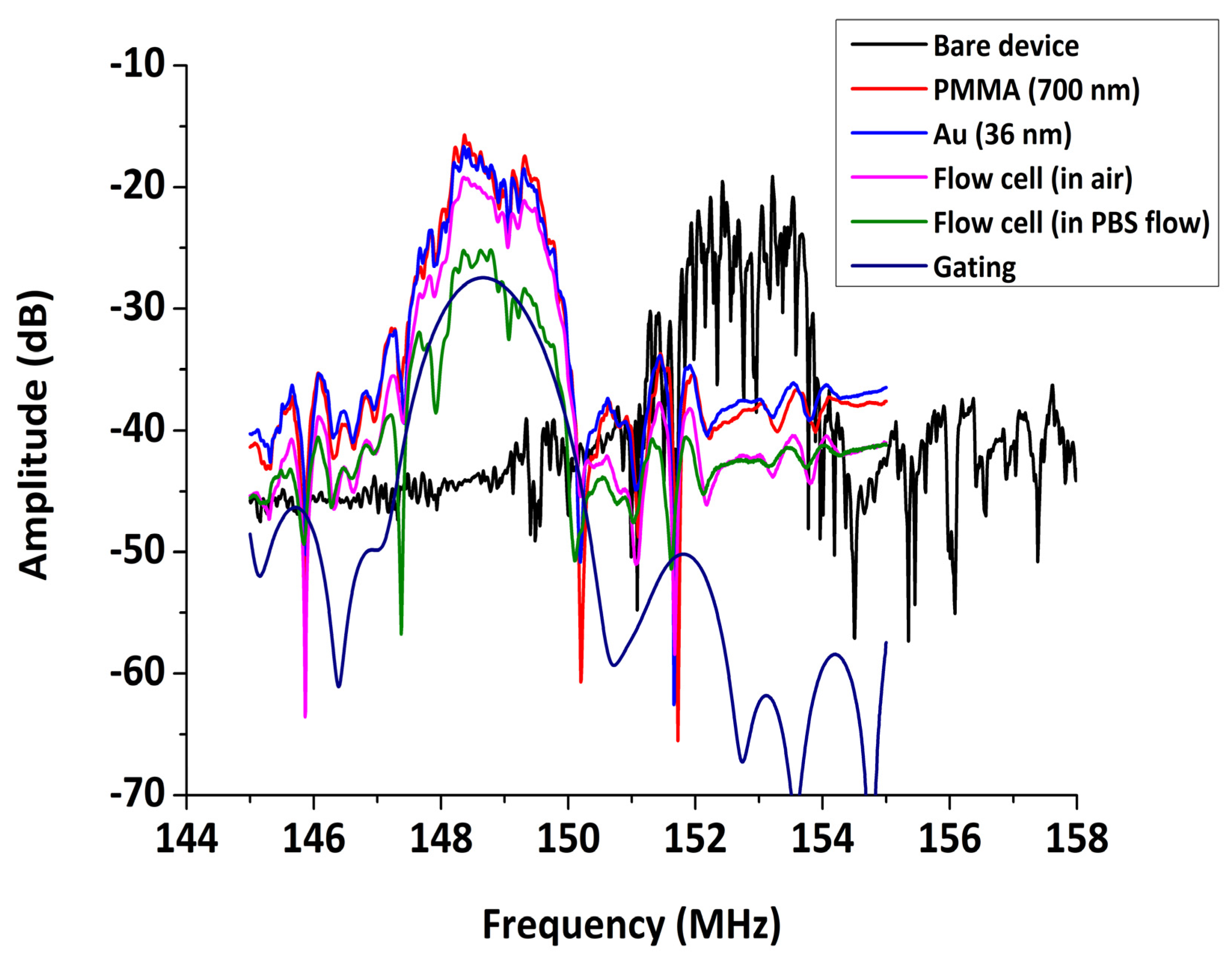
3.1. Love-Wave Device Optimization
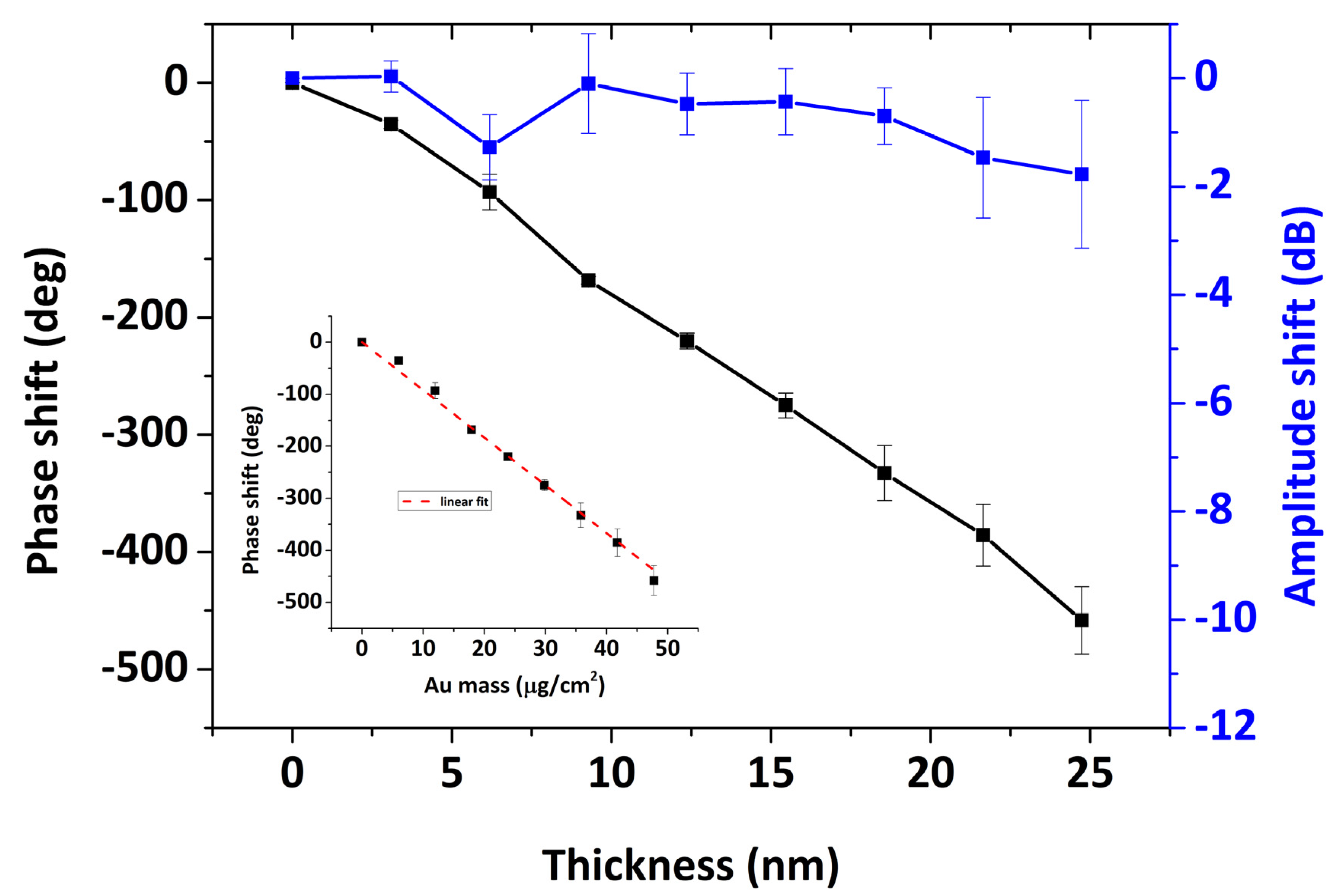
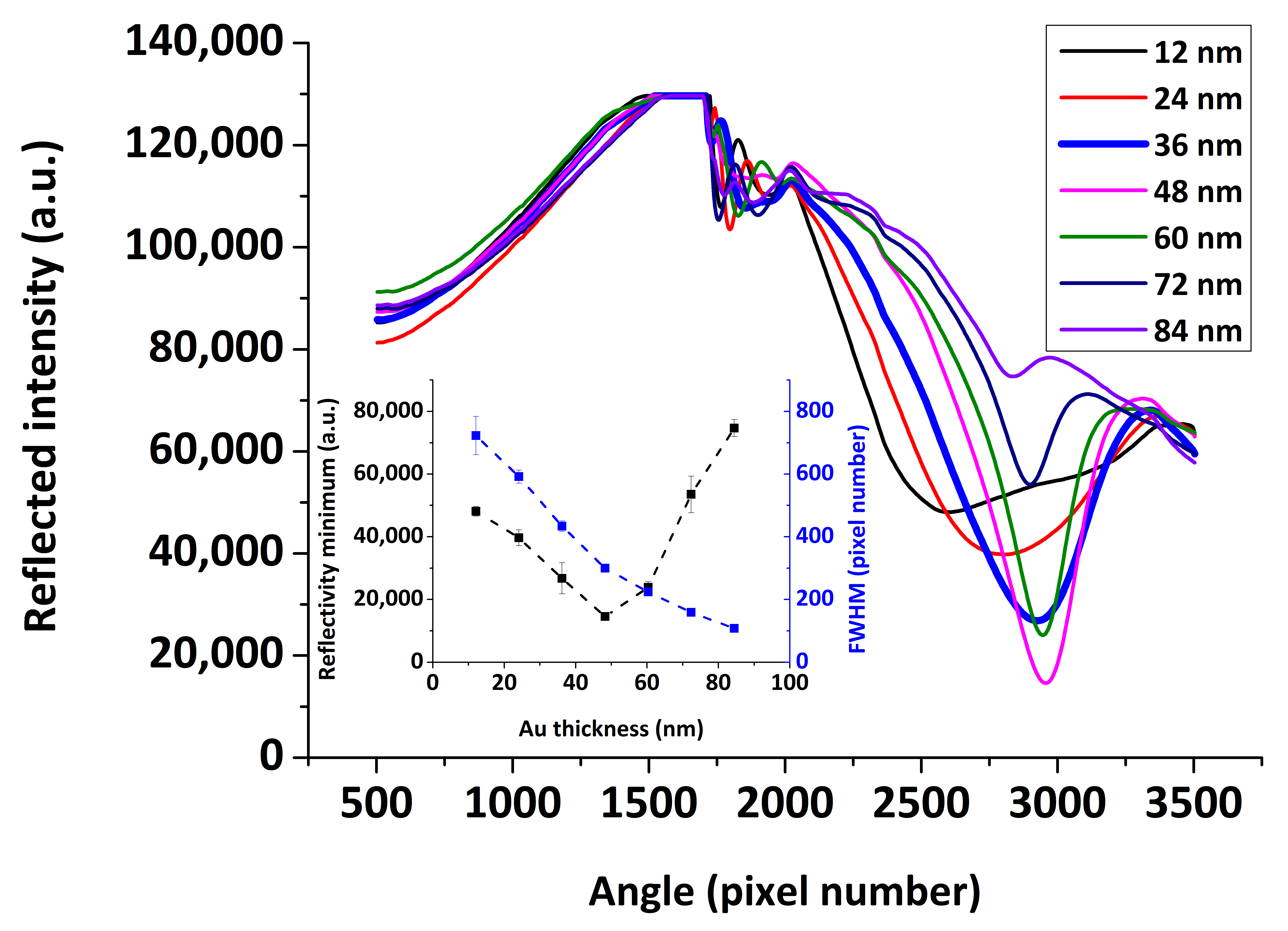
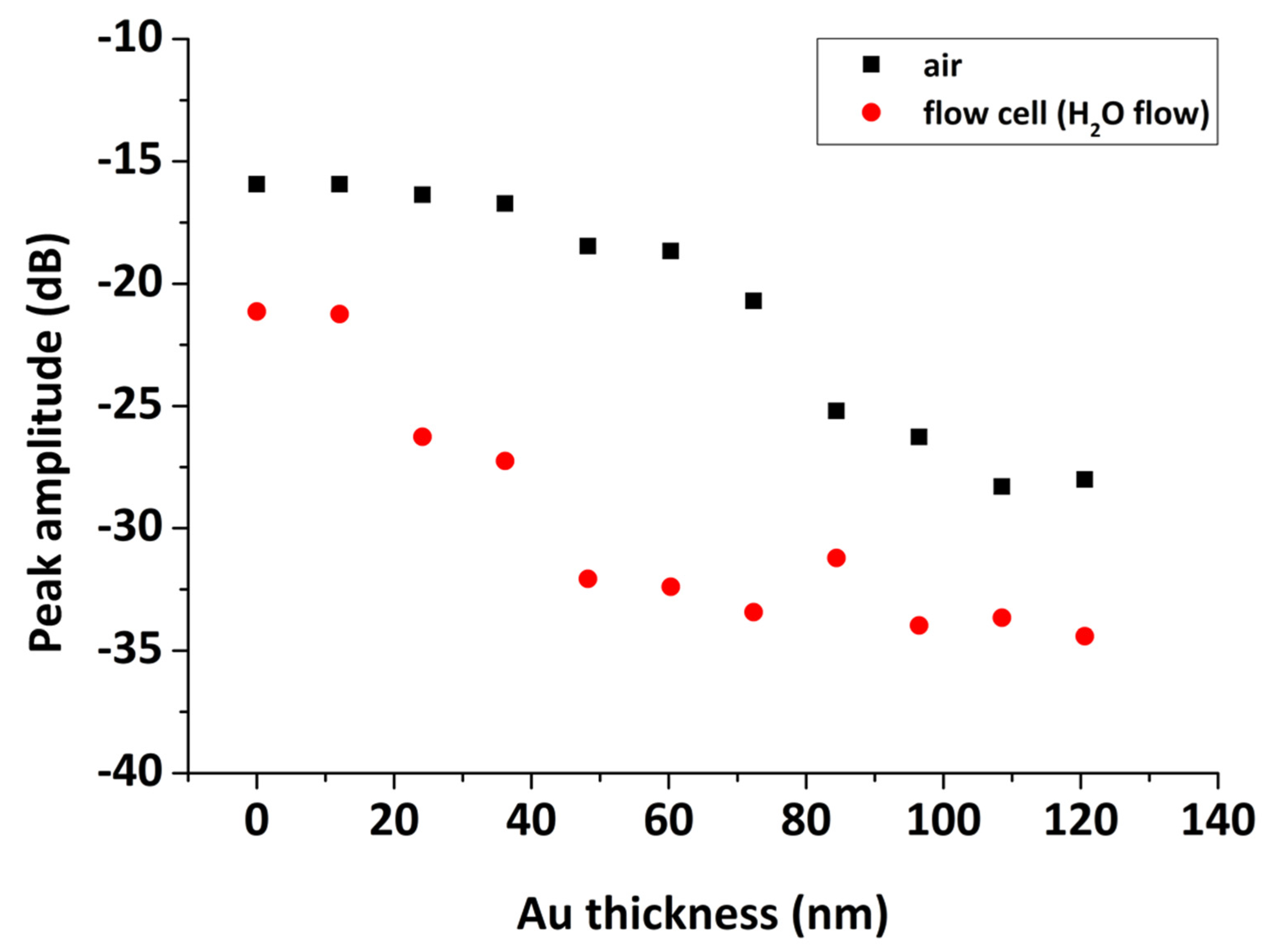
3.2. Au Layer Optimization for a Combined SPR/LW-SAW Geometry
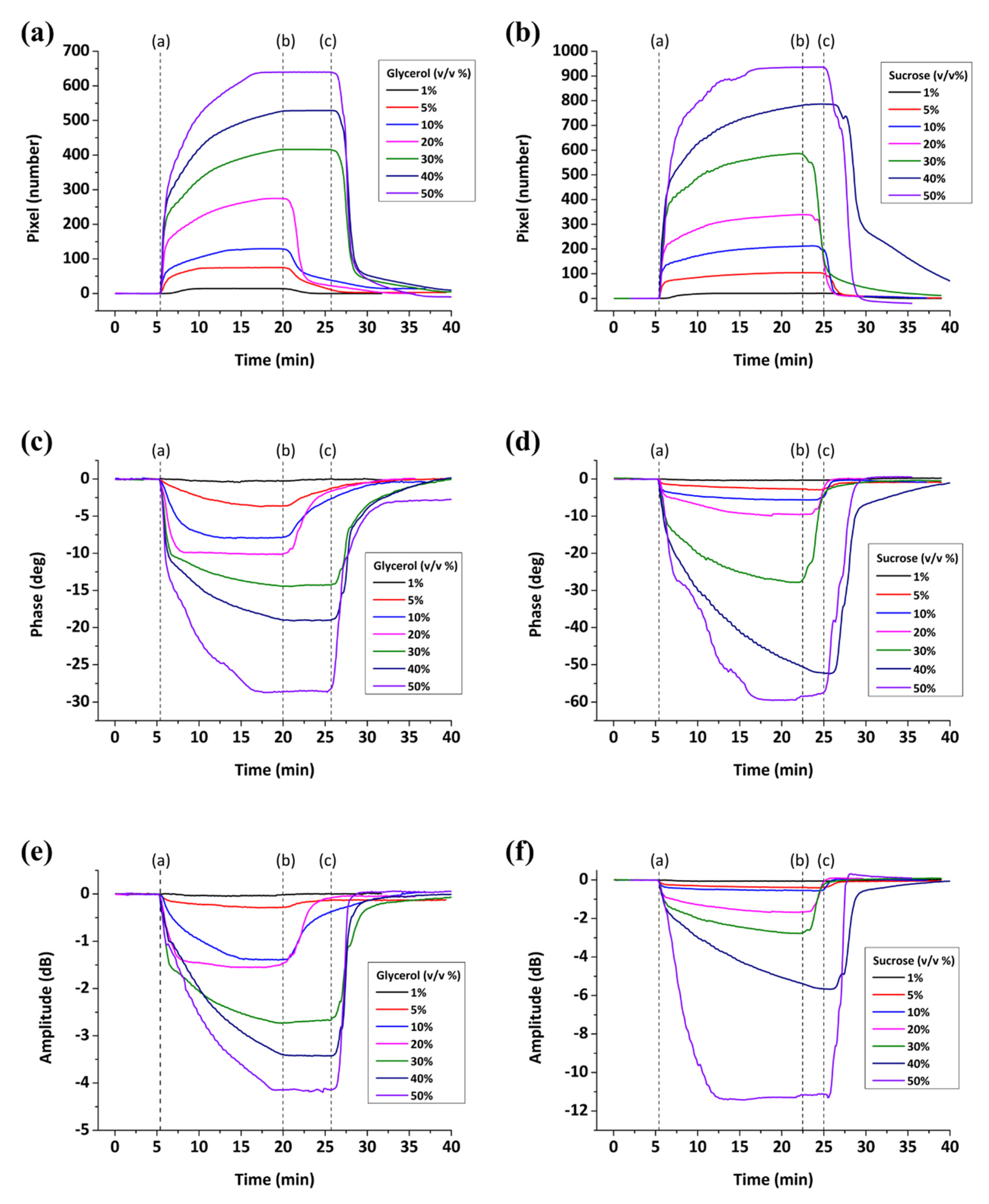
3.3. SPR/LW-SAW Calibration (Glycerol/Water and Sucrose/Water Solution Sensing)
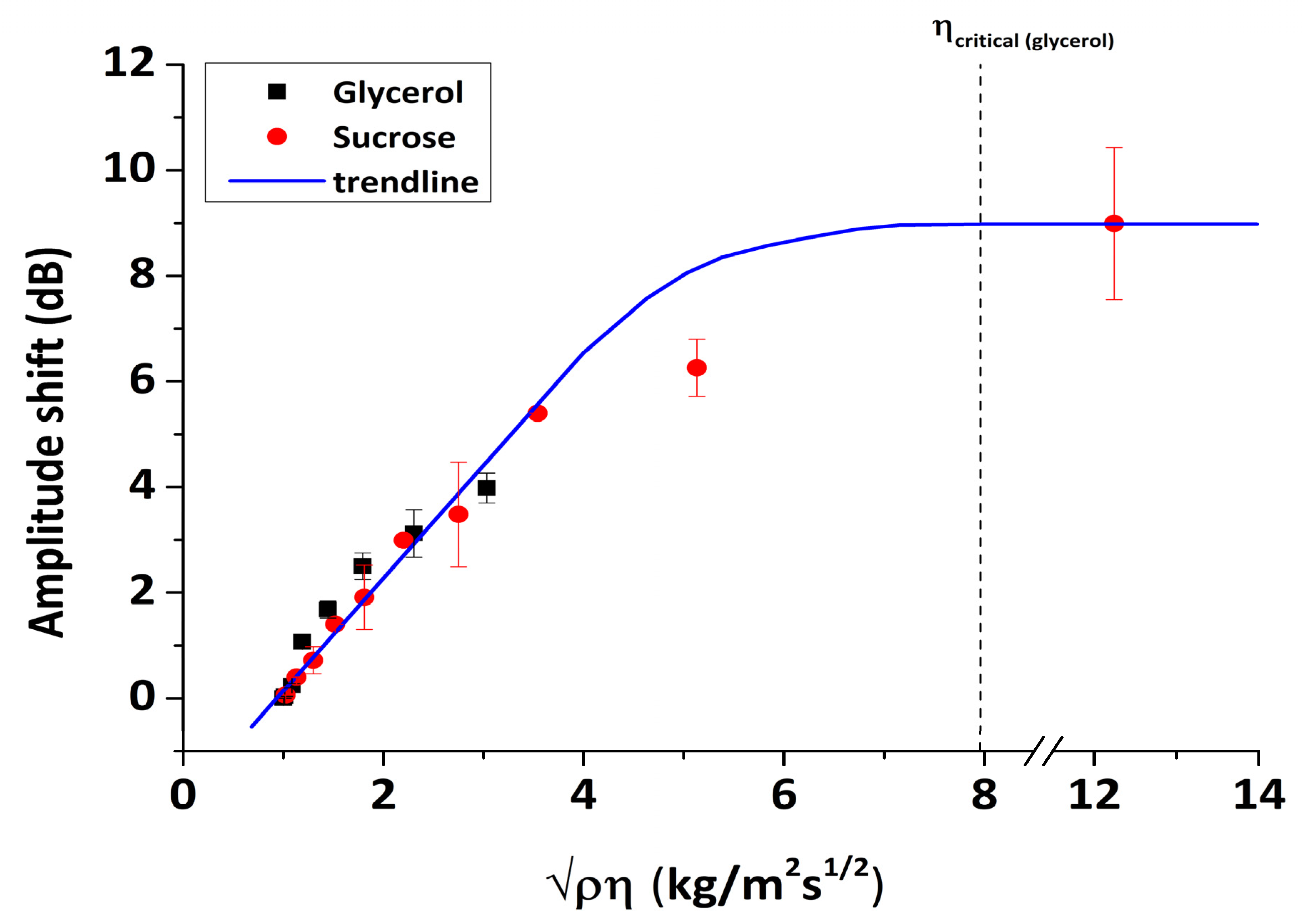
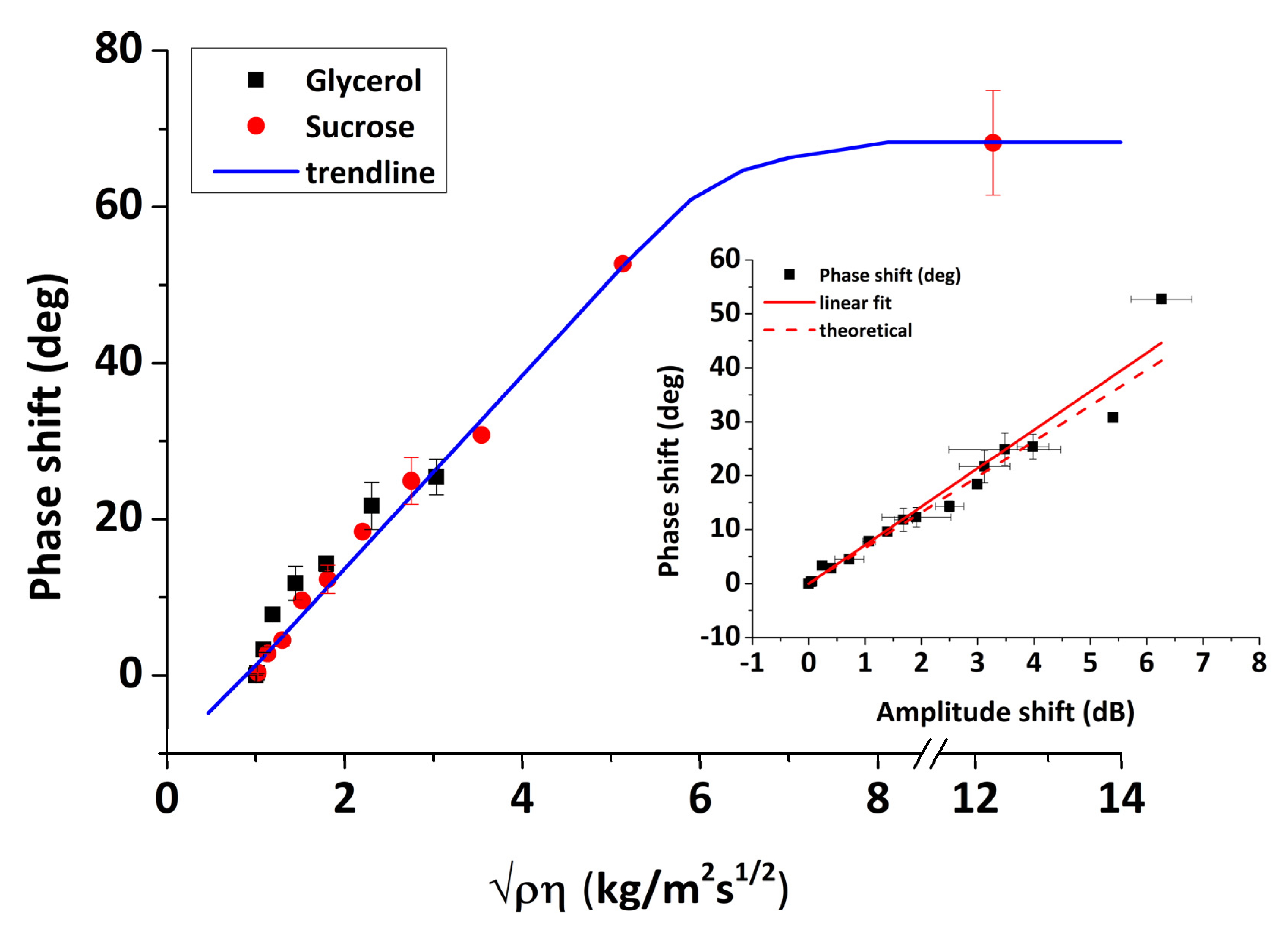
3.3.1. SPR Calibration
3.3.2. LW-SAW Calibration
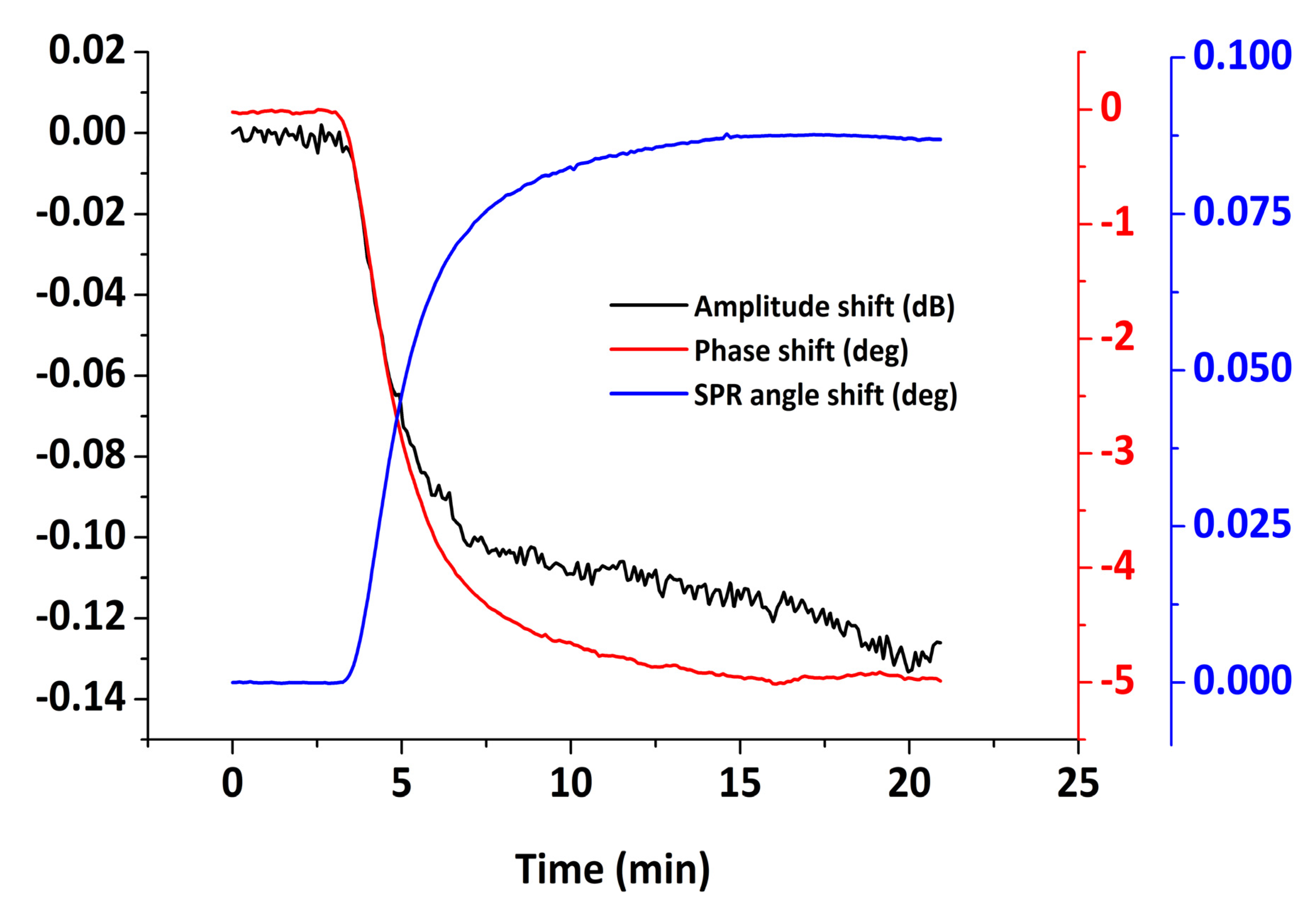
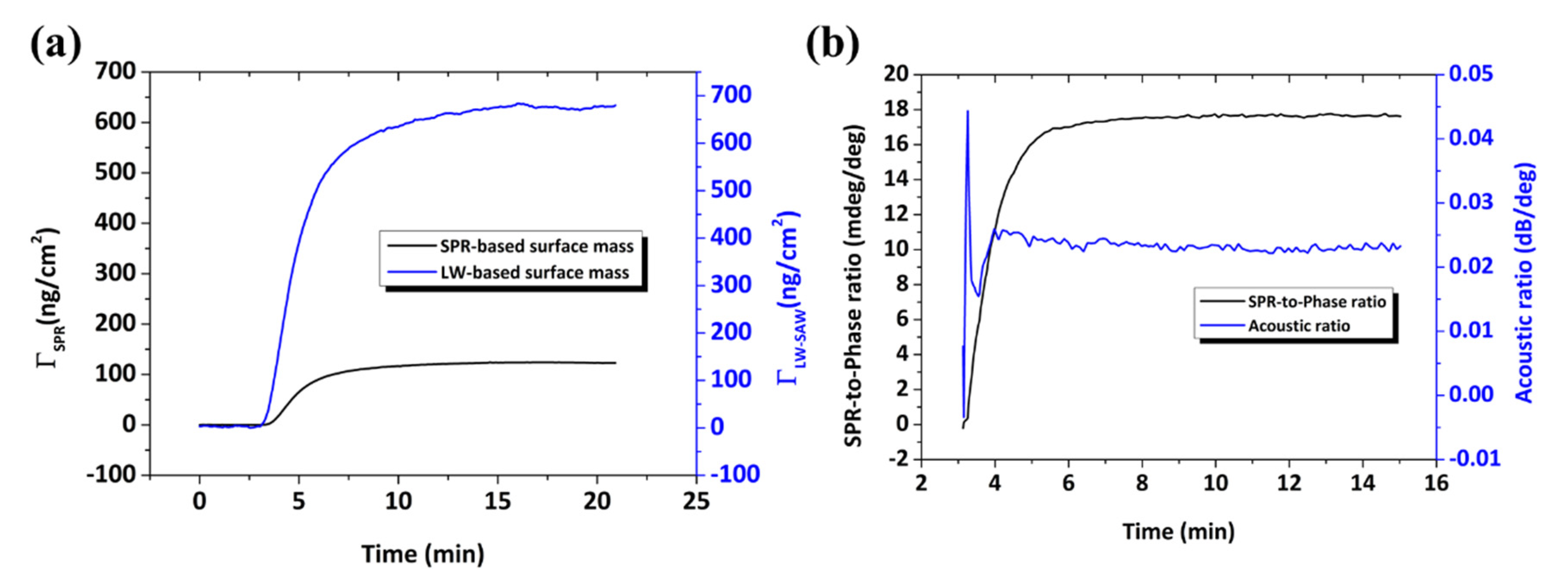
3.4. BSA Adsorption
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Konradi, R.; Textor, M.; Reimhult, E. Using complementary acoustic and optical techniques for quantitative monitoring of biomolecular adsorption at interfaces. Biosensors 2012, 2, 341–376. [Google Scholar] [CrossRef] [PubMed]
- Tsortos, A.; Papadakis, G.; Gizeli, E. On the Hydrodynamic Nature of DNA Acoustic Sensing. Anal. Chem. 2016, 88, 6472–6478. [Google Scholar] [CrossRef] [PubMed]
- Manaka, Y.; Kudo, Y.; Yoshimine, H.; Kawasaki, T.; Kajikawa, K.; Okahata, Y. Simultaneous anomalous reflection and quartz-crystal microbalance measurements of protein bindings on a gold surface. Chem. Commun. 2007, 3574–3576. [Google Scholar] [CrossRef] [PubMed]
- Laschitsch, A.; Menges, B.; Johannsmann, D. Simultaneous determination of optical and acoustic thicknesses of protein layers using surface plasmon resonance spectroscopy and quartz crystal microweighing. Appl. Phys. Lett. 2000, 14, 2252–2254. [Google Scholar] [CrossRef]
- Bailey, L.E.; Kambhampati, D.; Kanazawa, K.K.; Knoll, W.; Frank, C.W. Using surface plasmon resonance and the quartz crystal microbalance to monitor in situ the interfacial behavior of thin organic films. Langmuir 2002, 18, 479–489. [Google Scholar] [CrossRef]
- Zong, Y.; Xu, F.; Su, X.; Knoll, W. Quartz Crystal Microbalance with Integrated Surface Plasmon Grating Coupler. Anal. Chem. 2008, 80, 5246–5250. [Google Scholar] [CrossRef]
- Kim, J.; Kim, S.; Ohashi, T.; Muramatsu, H.; Chang, S.M.; Kim, W.S. Construction of simultaneous SPR and QCM sensing platform. Bioprocess. Biosyst. Eng. 2010. [Google Scholar] [CrossRef]
- Shinbo, K.; Ishikawa, H.; Baba, A.; Ohdaira, Y.; Kato, K.; Kaneko, F. Fabrication of a quartz-crystal-microbalance/surface-plasmon-resonance hybrid sensor and its use for detection of polymer thin-film deposition and evaluation of moisture sorption phenomena. Appl. Phys. Express. 2012, 5, 1–3. [Google Scholar] [CrossRef]
- Hao, D.; Hu, C.; Grant, J.; Glidle, A.; Cumming, D.R.S. Biosensors and Bioelectronics Hybrid localized surface plasmon resonance and quartz crystal microbalance sensor for label free biosensing. Biosens. Bioelectron. 2018, 100, 23–27. [Google Scholar] [CrossRef]
- Wang, G.; Rodahl, M.; Edvardsson, M.; Svedhem, S.; Ohlsson, G.; Höök, F.; Kasemo, B. A combined reflectometry and quartz crystal microbalance with dissipation setup for surface interaction studies. Rev. Sci. Instrum. 2008, 79. [Google Scholar] [CrossRef]
- Dahlin, A.B.; Jönsson, P.; Jonsson, M.P.; Schmid, E.; Zhou, Y.; Höök, F. Synchronized quartz crystal microbalance and nanoplasmonic sensing of biomolecular recognition reactions. ACS Nano 2008, 2, 2174–2182. [Google Scholar] [CrossRef] [PubMed]
- Rodenhausen, K.B.; Kasputis, T.; Pannier, A.K.; Gerasimov, J.Y.; Lai, R.Y.; Solinsky, M.; Tiwald, T.E.; Wang, H.; Sarkar, A.; Hofmann, T.; et al. Combined optical and acoustical method for determination of thickness and porosity of transparent organic layers below the ultra-thin film limit. Rev. Sci. Instrum. 2011. [Google Scholar] [CrossRef] [PubMed]
- Rasmusson, A.; Gizeli, E. Comparison of Poly(methylmethacrylate) and Novolak waveguide coatings for an acoustic biosensor. J. Appl. Phys. 2001, 90, 5911–5914. [Google Scholar] [CrossRef]
- Harding, G.L.; Du, J. Design and properties of quartz-based Love wave acoustic sensors incorporating silicon dioxide and PMMA guiding layers. Smart Mater. Struct. 1999, 6, 716–720. [Google Scholar] [CrossRef]
- Gizeli, E.; Goddard, N.J.; Lowe, C.R.; Stevenson, A.C. A Love plate biosensor utilising a polymer layer. Sens. Actuators B Chem. 1992, 6, 131–137. [Google Scholar] [CrossRef]
- Friedt, J.M.; Francis, L.; Reekmans, G.; de Palma, R.; Campitelli, A.; Sleytr, U.B. Simultaneous surface acoustic wave and surface plasmon resonance measurements: Electrodeposition and biological interactions monitoring. J. Appl. Phys. 2004, 95, 1677–1680. [Google Scholar] [CrossRef]
- Friedt, J.M.; Francis, L.A. Combined surface acoustic wave and surface plasmon resonance measurement of collagen and fibrinogen layer physical properties. Sens. Bio-Sens. Res. 2016. [Google Scholar] [CrossRef]
- Zhou, C.; Friedt, J.-M.; Angelova, A.; Choi, K.-H.; Laureyn, W.; Frederix, F.; Francis, A.L.; Campitelli, A.; Engelborghs, Y.; Borghs, G. Human immunoglobulin adsorption investigated by means of quartz crystal microbalance dissipation, atomic force microscopy, surface acoustic wave, and surface plasmon resonance techniques. Langmuir 2004, 20, 5870–5878. [Google Scholar] [CrossRef]
- Francis, L.A.; Friedt, J.M.; Zhou, C.; Bertrand, P. In situ evaluation of density, viscosity, and thickness of adsorbed soft layers by combined surface acoustic wave and surface plasmon resonance. Anal. Chem. 2006, 78, 4200–4209. [Google Scholar] [CrossRef]
- Bender, F.; Roach, P.; Tsortos, A.; Papadakis, G.; Newton, M.I.; McHale, G.; Gizeli, E. Development of a combined surface plasmon resonance/surface acoustic wave device for the characterization of biomolecules. Meas. Sci. Technol. 2009, 20. [Google Scholar] [CrossRef]
- Papadakis, G.; Friedt, J.M.; Eck, M.; Rabus, D.; Jobst, G.; Gizeli, E. Optimized acoustic biochip integrated with microfluidics for biomarkers detection in molecular diagnostics. Biomed. Microdevices 2017, 19, 1–11. [Google Scholar] [CrossRef]
- Kretschmann, E. Die Bestimmung optischer Konstanten von Metallen durch Anregung von Oberflächenplasmaschwingungen. Zeitschrift Fur Phys. 1971, 241, 313–324. [Google Scholar] [CrossRef]
- Badia, A.; Chen, C.I.; Norman, L.L. Calibration of a fan-shaped beam surface plasmon resonance instrument for quantitative adsorbed thin film studies-No metal film thickness or optical properties required. Sens. Actuators B Chem. 2013, 176, 736–745. [Google Scholar] [CrossRef]
- McHale, G. Generalized concept of shear horizontal acoustic plate mode and Love wave sensors. Meas. Sci. Technol. 2003, 14, 1847–1853. [Google Scholar] [CrossRef]
- McHale, G.; Newton, M.I.; Martin, F.; Gizeli, E.; Melzak, K.A. Resonant conditions for Love wave guiding layer thickness. Appl. Phys. Lett. 2001, 79, 3542–3543. [Google Scholar] [CrossRef]
- Newton, M.I.; McHale, G.; Martin, F. Experimental study of Love wave devices with thick guiding layers. >Sens. Actuators A Phys. 2004, 109, 180–185. [Google Scholar] [CrossRef]
- Newton, M.I.; Roach, P.; McHale, G. ST quartz acoustic wave sensors with sectional guiding layers. Sensors 2008, 7, 4384. [Google Scholar] [CrossRef]
- Knoll, W. Interfaces and Thin Films as Seen by Bound Electromagnetic Waves. Annu. Rev. Phys. Chem. 1998, 49, 569–638. [Google Scholar] [CrossRef]
- Martin, S.J.; Ricco, A.J.; Niemczyk, T.M.; Frye, G.C. Characterization of SH acoustic plate mode liquid sensors. Sens. Actuators 1989, 20, 253–268. [Google Scholar] [CrossRef]
- Ricco, A.J.; Martin, S.J. Thin metal film characterization and chemical sensors: Monitoring electronic conductivity, mass loading and mechanical properties with surface acoustic wave devices. Thin Solid Films 1991, 206, 94–101. [Google Scholar] [CrossRef]
- Francis, L.A.; Friedit, J.-M.; Palma, D.; Zhou, C.; Bartic, C.; Campitelli, A.; Bertrand, P. Techniques to evaluate the mass sensitivity of Love mode surface acoustic wave biosensors. In Proceedings of the 2004 IEEE International Frequency Control Symposium and Exposition, Montreal, QC, Canada, 23–27 August 2004; pp. 241–249. [Google Scholar] [CrossRef]
- Martin, S.J.; Frye, G.C.; Senturia, S.D. Dynamics and Response of Polymer-Coated Surface Acoustic Wave Devices: Effect of Viscoelastic Properties and Film Resonance. Anal. Chem. 1994, 66, 2201–2219. [Google Scholar] [CrossRef]
- Kovacs, G.; Vellekoop, M.J.; Haueis, R.; Lubking, G.W.; Venema, A. A love wave sensor for (bio)chemical sensing in liquids. Sens. Actuators A. Phys. 1994, 43, 38–43. [Google Scholar] [CrossRef]
- Raether, H. Tracts in Modern Physics. In Surface Plasmons on Smooth and Rough Surfaces and on Gratings; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Lide, D.R. CRC Handbook of Physics and Chemistry, 82nd ed.; Taylor & Francis: Abingdon, UK, 2001. [Google Scholar]
- Stenberg, E.; Persson, B.; Roos, H.; Urbaniczky, C. Quantitative determination of surface concentration of protein with surface plasmon resonance using radiolabeled proteins. J. Colloid Interface Sci. 1991, 143, 513–526. [Google Scholar] [CrossRef]
- Jung, L.S.; Campbell, C.T.; Chinowsky, T.M.; Mar, M.N.; Yee, S.S. Quantitative interpretation of the response of surface plasmon resonance sensors to adsorbed films. Langmuir 1998, 14, 5636–5648. [Google Scholar] [CrossRef]
- Jakoby, B.; Vellekoop, M.J. Viscosity sensing using a Love-wave device. Sens. Actuators A Phys. 1998, 68, 275–281. [Google Scholar] [CrossRef]
- Mitsakakis, K.; Tsortos, A.; Kondoh, J.; Gizeli, E. Parametric study of SH-SAW device response to various types of surface perturbations. Sens. Actuators B Chem. 2009, 138, 408–416. [Google Scholar] [CrossRef]
- Liedberg, B.; Lundström, I.; Stenberg, E. Principles of biosensing with an extended coupling matrix and surface plasmon resonance. Sens. Actuators B Chem. 1993, 11, 63–72. [Google Scholar] [CrossRef]
- Liedberg, B.; Nylander, C.; Lunström, I. Surface plasmon resonance for gas detection and biosensing. Sens. Actuators 1983. [Google Scholar] [CrossRef]
- Winspall. Available online: http://res-tec.de/index.html (accessed on 4 June 2020).
- RefractiveIndex.INFO. Available online: https://refractiveindex.info/ (accessed on 28 September 2020).
- Weiss, M.; Welsch, W.; Schickfus, M.V.; Hunklinger, S. Viscoelastic Behavior of Antibody Films on a Shear Horizontal Acoustic Surface Wave Sensor. Anal. Chem. 1998, 70, 2881–2887. [Google Scholar] [CrossRef]
- Ricco, A.J.; Martin, S.J. Acoustic wave viscosity sensor. Appl. Phys. Lett. 1987, 50, 1474–1476. [Google Scholar] [CrossRef]
- Ouali, F.F.; Doy, N.; McHale, G.; Hardacre, C.; Ge, R.; Allen, R.W.K.; Maclnnes, M.J.; Newton, I.M. Determination of the physical properties of room temperature ionic liquids using a love wave device. Anal. Chem. 2011, 83, 6717–6721. [Google Scholar] [CrossRef] [PubMed]
- Reimhult, K.; Petersson, K.; Krozer, A. QCM-D analysis of the performance of blocking agents on gold and polystyrene surfaces. Langmuir 2008, 24, 8695–8700. [Google Scholar] [CrossRef] [PubMed]
- Goda, T.; Miyahara, Y. Interpretation of protein adsorption through its intrinsic electric charges: A comparative study using a field-effect transistor, surface plasmon resonance, and quartz crystal microbalance. Langmuir 2012, 28, 14730–14738. [Google Scholar] [CrossRef]
- Kubiak-Ossowska, K.; Tokarczyk, K.; Jachimska, B.; Mulheran, P.A. Bovine Serum Albumin Adsorption at a Silica Surface Explored by Simulation and Experiment. J. Phys. Chem. B 2017, 121, 3975–3986. [Google Scholar] [CrossRef]
- Jachimska, B.; Tokarczyk, K.; Łapczyńska, M.; Puciul-Malinowska, A.; Zapotoczny, S. Structure of bovine serum albumin adsorbed on silica investigated by quartz crystal microbalance. Colloids Surfaces A. Physicochem. Eng. Asp. 2016, 489, 163–172. [Google Scholar] [CrossRef]
- Rubio-Lara, J.A.; Bergler, F.; Attwood, S.J.; Edwardson, J.M.; Welland, M.E. Ultraflat Gold QCM Electrodes Fabricated with Pressure-Forming Template Stripping for Protein Studies at the Nanoscale. Langmuir 2019, 35, 8889–8895. [Google Scholar] [CrossRef]
- Hagiwara, T.; Nattawut, P.; Shibata, M.; Sakiyama, T. Monitoring of adsorption behaviors of bovine serum albumin onto a stainless steel surface by the quartz crystal microbalance based on admittance analysis. Biosci. Biotechnol. Biochem. 2017, 81, 783–789. [Google Scholar] [CrossRef]
- Sander, M.; Madliger, M.; Schwarzenbach, R.P. Adsorption of transgenic insecticidal Cry1Ab protein to SiO2. 1. Forces driving adsorption. Environ. Sci. Technol. 2010, 44, 8870–8876. [Google Scholar] [CrossRef]
- McMeekin, T.L.; Wilensky, M.; Groves, M.L. Refractive indices of proteins in relation to amino acid composition and specific volume. Biochem. Biophys. Res. Commun. 1962. [Google Scholar] [CrossRef]
- Zhao, H.; Brown, P.H.; Schuck, P. On the distribution of protein refractive index increments. Biophys. J. 2011, 100, 2309–2317. [Google Scholar] [CrossRef] [PubMed]
- Perlmann, G.E.; Longsworth, L.G. The Specific Refractive Increment of Some Purified Proteins. J. Am. Chem. Soc. 1948, 70, 2719–2724. [Google Scholar] [CrossRef] [PubMed]
- Peña, Á.B.; Radhakrishnan, R.; Ketelhut, S.; Schnekenburger, J.; Kemper, B. Refractive index determination of buffer solutions from visible to near-infrared spectral range for multispectral quantitative phase imaging using a calibrated Abbe refractometer. Quantitative Ph. Imaging 2019, 5. [Google Scholar] [CrossRef]
- Benesch, J.; Askendal, A.; Tengvall, P. Quantification of adsorbed human serum albumin at solid interfaces: A comparison between radioimmunoassay (RIA) and simple null ellipsometry. Colloids Surfaces B Biointerfaces 2000, 18, 71–81. [Google Scholar] [CrossRef]
- Hook, F.F.; Vörös, J.; Rodahl, M.; Kurrat, R.; Böni, P.; Ramsden, J.J.; Spencer, M.T.N.D.; Tengvall, P.; Gold, J.; Kasemo, B.A. Comparative study of protein adsorption on titanium oxide surfaces using in situ ellipsometry, optical waveguide lightmode spectroscopy, and quartz crystal microbalance/dissipation. Colloids Surfaces B Biointerfaces 2002, 24, 155–170. [Google Scholar] [CrossRef]
- Vörös, J. The density and refractive index of adsorbing protein layers. Biophys. J. 2004, 87, 553–561. [Google Scholar] [CrossRef]
- Sauerbrey, G. Verwendung von Schwingquarzen zur Wägung dünner Schichten und zur Mikrowägung. Zeitschrift für Phys. 1959. [Google Scholar] [CrossRef]
- Diaconu, G.; Schäfer, T. Study of the interactions of proteins with a solid surface using complementary acoustic and optical techniques. Biointerphases 2014. [Google Scholar] [CrossRef]
- Höök, F.; Kasemo, B.; Nylander, T.; Fant, C.; Sott, K.; Elwing, H. Variations in coupled water, viscoelastic properties, and film thickness of a Mefp-1 protein film during adsorption and cross-linking: A quartz crystal microbalance with dissipation monitoring, ellipsometry, and surface plasmon resonance study. Anal. Chem. 2001. [Google Scholar] [CrossRef]
- Tsortos, A.; Papadakis, G.; Mitsakakis, K.; Melzak, K.a.; Gizeli, E. Quantitative Determination of Size and Shape of Surface-Bound DNA Using an Acoustic Wave Sensor. Biophys. J. 2008, 94, 2706–2715. [Google Scholar] [CrossRef]
- Papadakis, G.; Tsortos, A.; Gizeli, E. Acoustic characterization of nanoswitch structures: Application to the DNA holliday junction. Nano Lett. 2010, 10, 5093–5097. [Google Scholar] [CrossRef] [PubMed]
- Papadakis, G.; Tsortos, A.; Mitsakakis, K.; Gizeli, E. Characterization of DNA-Hv1 histone interactions; discrimination of DNA size and shape. FEBS Lett. 2010, 584, 935–940. [Google Scholar] [CrossRef]
- Melzak, K.A.; Bender, F.; Tsortos, A.; Gizeli, E. Probing mechanical properties of liposomes using acoustic sensors. Langmuir 2008, 24, 9172–9180. [Google Scholar] [CrossRef]
- Mitsakakis, K.; Tsortos, A.; Gizeli, E. Quantitative determination of protein molecular weight with an acoustic sensor; significance of specific versus non-specific binding. Analyst 2014, 139, 3918–3925. [Google Scholar] [CrossRef] [PubMed]
- Saitakis, M.; Tsortos, A.; Gizeli, E. Probing the interaction of a membrane receptor with a surface-attached ligand using whole cells on acoustic biosensors. Biosens. Bioelectron. 2010, 25, 1688–1693. [Google Scholar] [CrossRef] [PubMed]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Samarentsis, A.G.; Pantazis, A.K.; Tsortos, A.; Friedt, J.-M.; Gizeli, E. Hybrid Sensor Device for Simultaneous Surface Plasmon Resonance and Surface Acoustic Wave Measurements. Sensors 2020, 20, 6177. https://doi.org/10.3390/s20216177
Samarentsis AG, Pantazis AK, Tsortos A, Friedt J-M, Gizeli E. Hybrid Sensor Device for Simultaneous Surface Plasmon Resonance and Surface Acoustic Wave Measurements. Sensors. 2020; 20(21):6177. https://doi.org/10.3390/s20216177
Chicago/Turabian StyleSamarentsis, Anastasios G., Alexandros K. Pantazis, Achilleas Tsortos, Jean-Michel Friedt, and Electra Gizeli. 2020. "Hybrid Sensor Device for Simultaneous Surface Plasmon Resonance and Surface Acoustic Wave Measurements" Sensors 20, no. 21: 6177. https://doi.org/10.3390/s20216177
APA StyleSamarentsis, A. G., Pantazis, A. K., Tsortos, A., Friedt, J.-M., & Gizeli, E. (2020). Hybrid Sensor Device for Simultaneous Surface Plasmon Resonance and Surface Acoustic Wave Measurements. Sensors, 20(21), 6177. https://doi.org/10.3390/s20216177






