Accounting for Modeling Errors and Inherent Structural Variability through a Hierarchical Bayesian Model Updating Approach: An Overview
Abstract
1. Introduction
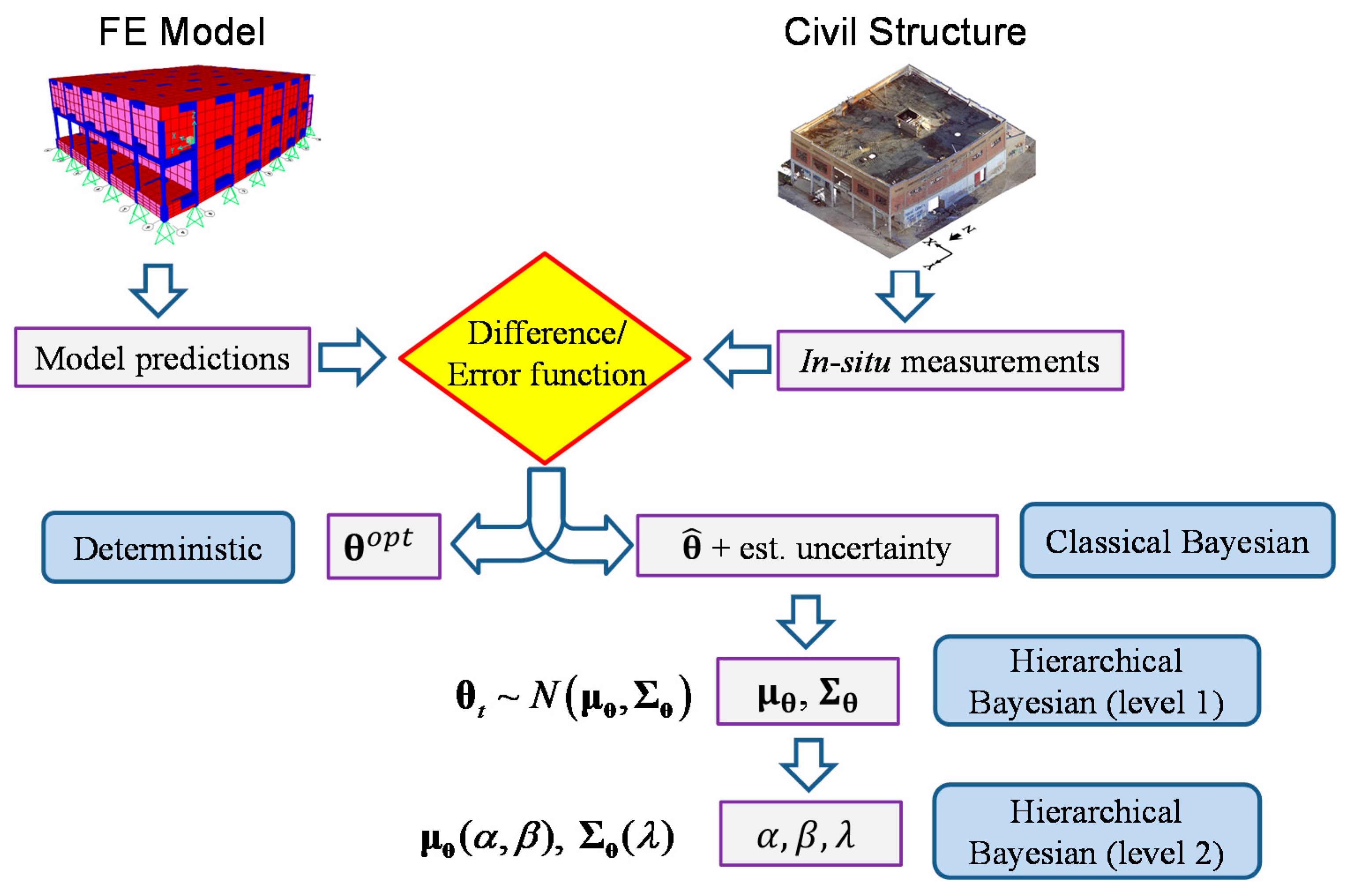
2. Uncertainty Quantification and Propagation through Hierarchical Bayesian Modeling
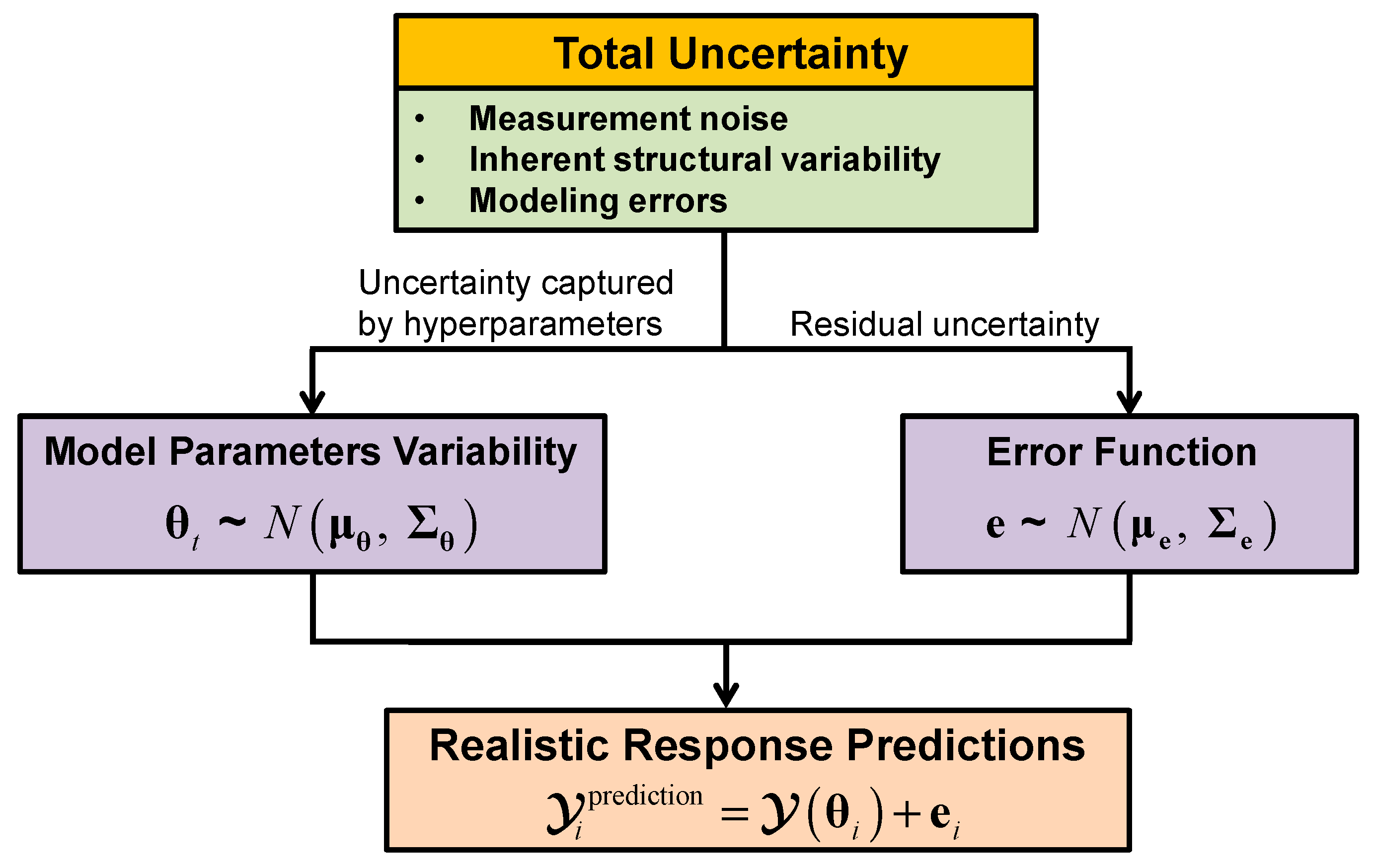
2.1. Sources of Uncertainty
2.1.1. Measurement Noise
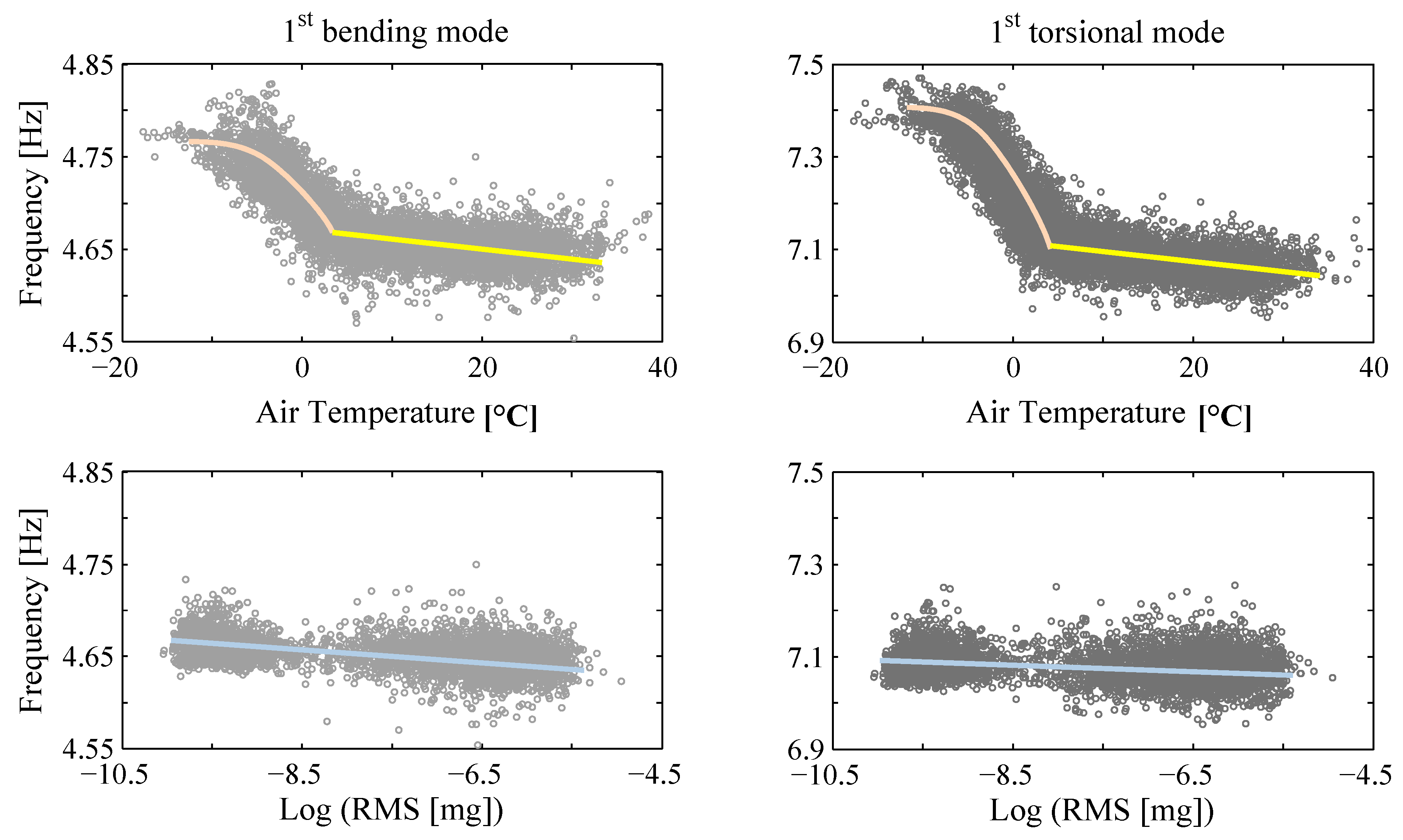
2.1.2. Changing Ambient and Environmental Conditions
2.1.3. Modeling Errors
2.2. Hierarchical Bayesian Model Updating
2.2.1. Hyperparameters
2.2.2. Error Function
2.2.3. Model Updating Process
2.3. Probabilistic Response Prediction
2.4. Numerical Methods for Estimating Posterior Distributions
2.4.1. Sampling Approach
2.4.2. Simplified Approach for Estimating Map Values
3. Applications to Three Full-Scale Civil Structures
3.1. Application 1: Footbridge at Tufts University Campus
3.1.1. Test Structure and Measured Data
3.1.2. Hierarchical Bayesian Modeling at Different Information Levels
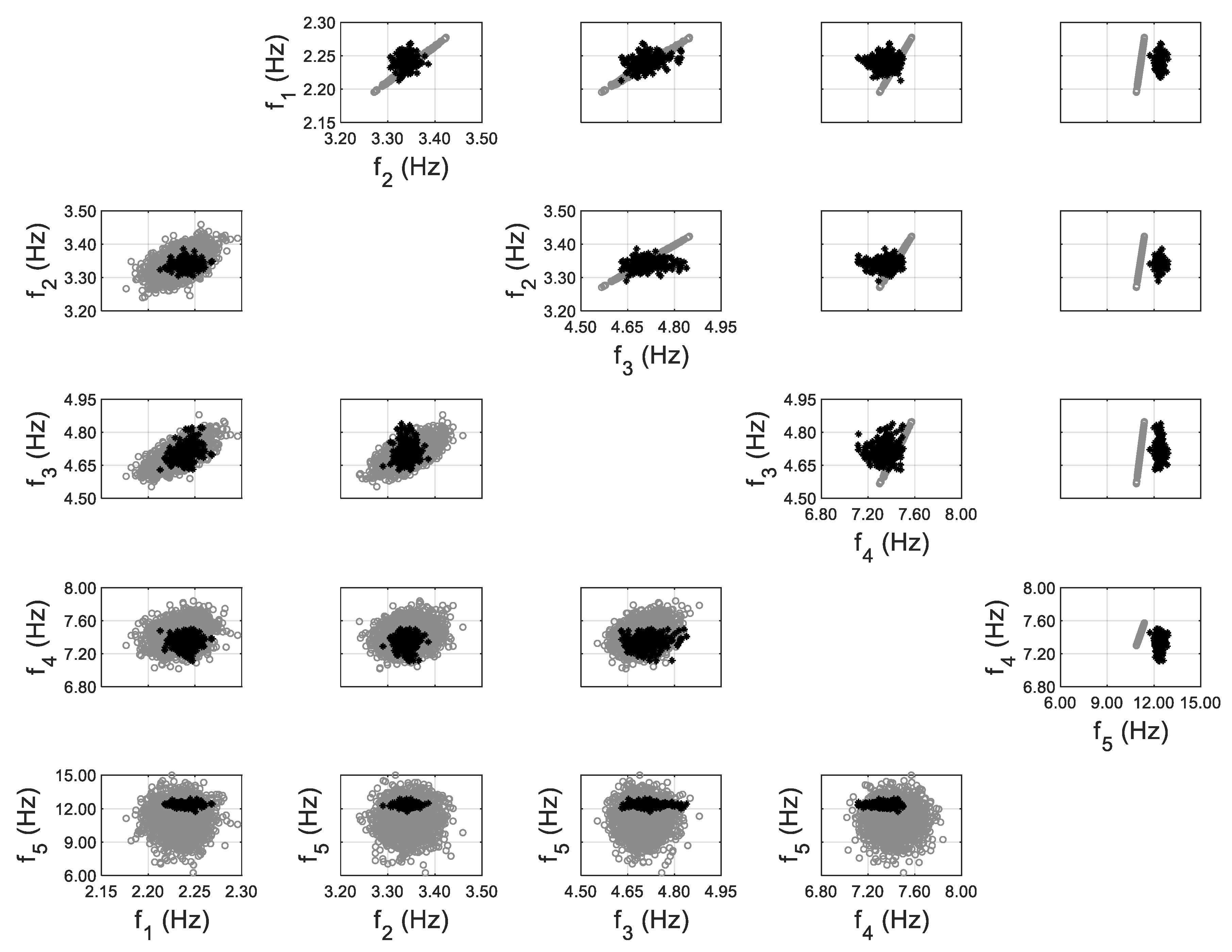
3.1.3. Model Updating Results and Response Predictions
3.2. Application 2: Ten-Story RC Building in Utica, NY
3.2.1. Test Structure and Measured Data
3.2.2. Hierarchical Bayesian Model Updating with Zero-Mean and Non-Zero-Mean Error Function
3.2.3. Model Predictions for Future Building Conditions
3.3. Application 3: Two-Story RC Building in El Centro, California
3.3.1. Test Structure and Measured Data
3.3.2. Hierarchical Bayesian Modeling of Stiffness-Amplitude Relationship
3.3.3. Time History Response Predictions
4. Summary and Conclusions
- (1)
- In the application of the Dowling Hall footbridge, different information levels are considered for stiffness hyperparameters formulation and compared for their performance. It is found that the stiffness variability is reduced when information about ambient temperatures and excitation amplitudes is considered, thus providing tighter confidence intervals for model-predictions. In this study, error function must be included in model predictions to provide realistic confidence bounds.
- (2)
- In the application of the 10-story building, the effects of error bias are studied, and it is found that more accurate and tighter prediction bounds are obtained when the error bias of the fifth mode (which was observed to be biased) is considered. It is also found that the estimated error distribution may not be valid outside the calibration range. Therefore, special precautions should be taken when the calibrated model is used for extrapolation.
- (3)
- In the application of the two-story building, the stiffness mean vector is assumed to have a linear relationship with the vibration levels. Accurate predictions are observed for modal parameters and acceleration time histories using the calibrated model when the stiffness-amplitude dependency is explicitly considered, while inaccurate results are observed when this correlation is neglected.
- (a)
- The hierarchical framework is capable of quantifying structural inherent variability and modeling errors, through postulating probability distributions for structural parameters, and estimating the hyperparameters of these distributions. The estimated structural parameters uncertainty would converge to a constant variation level depending on MAP values of hyperparameters, while parameters uncertainty using classical Bayesian methods is reduced infinitely with more data.
- (b)
- More accurate and robust prediction bounds are achieved by hierarchical framework through propagating of parameters variability and error function. This is often more valuable than just obtaining an accurate prediction fit with measurements. Moreover, more reasonable prediction bounds are obtained compared to the classical Bayesian approach, even when only propagating parameters variability, which is especially useful for predictions of unobserved quantities where error function estimate is not available.
- (c)
- Different relationships and factors that contribute to structural parameters uncertainty can be embedded into the hyperparameters, e.g., ambient temperature and excitation amplitude, in the hierarchical framework, which would reduce the parameters variability and provide tighter prediction confidence bounds.
- (d)
- The hierarchical framework is capable of quantifying the residual prediction errors, by estimating the distribution parameters of error function. The inclusion and propagation of error function into response predictions is important and necessary in some cases when a significant amount of uncertainties is retained in the error function, e.g., Applications 1–3. Considering non-zero-mean error distribution in the presence of error bias reduces the error covariance matrix, thus providing tighter confidence bounds.
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Mottershead, J.E.; Friswell, M.I. Model updating in structural dynamics: A survey. J. Sound Vib. 1993, 167, 347–375. [Google Scholar] [CrossRef]
- Doebling, S.W.; Farrar, C.R.; Prime, M.B.; Shevitz, D.W. Damage identification and health monitoring of structural and mechanical systems from changes in their vibration characteristics: A literature review. Los. Alamos Natl. Lab. 1996. [Google Scholar] [CrossRef]
- Sohn, H.; Farrar, C.R.; Hemez, F.M.; Shunk, D.D.; Stinemates, D.W.; Nadler, B.R.; Czarnecki, J.J. A review of structural health monitoring literature: 1996–2001. In Proceedings of the Third World Conference on Structural Control, Como, Italy, 7–12 April 2002. [Google Scholar]
- Yan, Y.; Cheng, L.; Wu, Z.; Yam, L. Development in vibration-based structural damage detection technique. Mech. Syst. Signal Process. 2007, 21, 2198–2211. [Google Scholar] [CrossRef]
- Moaveni, B.; He, X.; Conte, J.P.; De Callafon, R.A. Damage identification of a composite beam using finite element model updating. Comput.-Aided Civ. Infrastruct. Eng. 2008, 23, 339–359. [Google Scholar] [CrossRef]
- Song, M.; Yousefianmoghadam, S.; Mohammadi, M.E.; Moaveni, B.; Stavridis, A.; Wood, R.L. An application of finite element model updating for damage assessment of a two-story reinforced concrete building and comparison with lidar. Struct. Health Monit. 2018, 17, 1129–1150. [Google Scholar] [CrossRef]
- Brownjohn, J.M.; Xia, P.Q. Dynamic assessment of curved cable-stayed bridge by model updating. J. Struct. Eng. 2000, 126, 252–260. [Google Scholar] [CrossRef]
- Brownjohn, J.M.W.; Moyo, P.; Omenzetter, P.; Lu, Y. Assessment of highway bridge upgrading by dynamic testing and finite-element model updating. J. Bridge Eng. 2003, 8, 162–172. [Google Scholar] [CrossRef]
- Teughels, A.; Maeck, J.; De Roeck, G. Damage assessment by FE model updating using damage functions. Comput. Struct. 2002, 80, 1869–1879. [Google Scholar] [CrossRef]
- Teughels, A.; De Roeck, G. Structural damage identification of the highway bridge Z24 by FE model updating. J. Sound Vib. 2004, 278, 589–610. [Google Scholar] [CrossRef]
- Reynders, E.; Roeck, G.D.; Gundes Bakir, P.; Sauvage, C. Damage identification on the Tilff Bridge by vibration monitoring using optical fiber strain sensors. J. Eng. Mech. 2007, 133, 185–193. [Google Scholar] [CrossRef]
- Zhang, Q.; Chang, T.Y.P.; Chang, C.C. Finite-element model updating for the Kap Shui Mun cable-stayed bridge. J. Bridge Eng. 2001, 6, 285–293. [Google Scholar] [CrossRef]
- Jaishi, B.; Ren, W.X. Damage detection by finite element model updating using modal flexibility residual. J. Sound Vib. 2006, 290, 369–387. [Google Scholar] [CrossRef]
- Moaveni, B.; Conte, J.P.; Hemez, F.M. Uncertainty and sensitivity analysis of damage identification results obtained using finite element model updating. Comput.-Aided Civ. Infrastruct. Eng. 2009, 24, 320–334. [Google Scholar] [CrossRef]
- Moaveni, B.; He, X.; Conte, J.P.; Restrepo, J.I. Damage identification study of a seven-story full-scale building slice tested on the UCSD-NEES shake table. Struct. Saf. 2010, 32, 347–356. [Google Scholar] [CrossRef]
- Moaveni, B.; Stavridis, A.; Lombaert, G.; Conte, J.P.; Shing, P.B. Finite-element model updating for assessment of progressive damage in a 3-story infilled RC frame. J. Struct. Eng. 2012, 139, 1665–1674. [Google Scholar] [CrossRef]
- Bassoli, E.; Vincenzi, L.; D’Altri, A.M.; de Miranda, S.; Forghieri, M.; Castellazzi, G. Ambient vibration-based finite element model updating of an earthquake-damaged masonry tower. Struct. Control. Health Monit. 2018, 25, e2150. [Google Scholar] [CrossRef]
- Nozari, A.; Behmanesh, I.; Yousefianmoghadam, S.; Moaveni, B.; Stavridis, A. Effects of variability in ambient vibration data on model updating and damage identification of a 10-story building. Eng. Struct. 2017, 151, 540–553. [Google Scholar] [CrossRef]
- Yuen, K.V. Bayesian Methods for Structural Dynamics and Civil Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Beck, J.L.; Katafygiotis, L.S. Updating models and their uncertainties. I: Bayesian statistical framework. J. Eng. Mech. 1998, 124, 455–461. [Google Scholar]
- Hastings, W.K. Monte Carlo sampling methods using Markov chains and their applications. Biometrika 1970, 57, 97–109. [Google Scholar] [CrossRef]
- Haario, H.; Saksman, E.; Tamminen, J. An adaptive Metropolis algorithm. Bernoulli 2001, 7, 223–242. [Google Scholar] [CrossRef]
- Ching, J.; Chen, Y.C. Transitional Markov chain Monte Carlo method for Bayesian model updating, model class selection, and model averaging. J. Eng. Mech. 2007, 133, 816–832. [Google Scholar] [CrossRef]
- Beck, J.L.; Au, S.K. Bayesian updating of structural models and reliability using Markov chain Monte Carlo simulation. J. Eng. Mech. 2002, 128, 380–391. [Google Scholar] [CrossRef]
- Sohn, H.; Law, K.H. A Bayesian probabilistic approach for structure damage detection. Earthq. Eng. Struct. Dyn. 1997, 26, 1259–1281. [Google Scholar] [CrossRef]
- Yuen, K.V.; Beck, J.L.; Au, S.K. Structural damage detection and assessment by adaptive Markov chain Monte Carlo simulation. Struct. Control. Health Monit. 2004, 11, 327–347. [Google Scholar] [CrossRef]
- Ching, J.; Beck, J.L. New Bayesian model updating algorithm applied to a structural health monitoring benchmark. Struct. Health Monit. 2004, 3, 313–332. [Google Scholar] [CrossRef]
- Muto, M.; Beck, J.L. Bayesian updating and model class selection for hysteretic structural models using stochastic simulation. J. Vib. Control. 2008, 14, 7–34. [Google Scholar] [CrossRef]
- Behmanesh, I.; Moaveni, B.; Papadimitriou, C. Probabilistic damage identification of a designed 9-story building using modal data in the presence of modeling errors. Eng. Struct. 2017, 131, 542–552. [Google Scholar] [CrossRef]
- Song, M.; Renson, L.; Noël, J.P.; Moaveni, B.; Kerschen, G. Bayesian model updating of nonlinear systems using nonlinear normal modes. Struct. Control. Health Monit. 2018, 25, e2258. [Google Scholar] [CrossRef]
- Ntotsios, E.; Papadimitriou, C.; Panetsos, P.; Karaiskos, G.; Perros, K.; Perdikaris, P.C. Bridge health monitoring system based on vibration measurements. Bull. Earthq. Eng. 2009, 7, 469. [Google Scholar] [CrossRef]
- Lam, H.; Peng, H.; Au, S. Development of a practical algorithm for Bayesian model updating of a coupled slab system utilizing field test data. Eng. Struct. 2014, 79, 182–194. [Google Scholar] [CrossRef]
- Lam, H.F.; Yang, J.; Au, S.K. Bayesian model updating of a coupled-slab system using field test data utilizing an enhanced Markov chain Monte Carlo simulation algorithm. Eng. Struct. 2015, 102, 144–155. [Google Scholar] [CrossRef]
- Behmanesh, I.; Moaveni, B. Probabilistic identification of simulated damage on the Dowling Hall footbridge through Bayesian finite element model updating. Struct. Control. Health Monit. 2015, 22, 463–483. [Google Scholar] [CrossRef]
- De Falco, A.; Girardi, M.; Pellegrini, D.; Robol, L.; Sevieri, G. Model parameter estimation using Bayesian and deterministic approaches: The case study of the Maddalena Bridge. Procedia Struct. Integr. 2018, 11, 210–217. [Google Scholar] [CrossRef]
- Goulet, J.A.; Michel, C.; Smith, I.F. Hybrid probabilities and error-domain structural identification using ambient vibration monitoring. Mech. Syst. Signal Process. 2013, 37, 199–212. [Google Scholar] [CrossRef][Green Version]
- Astroza, R.; Alessandri, A. Effects of model uncertainty in nonlinear structural finite element model updating by numerical simulation of building structures. Struct. Control. Health Monit. 2019, 26, e2297. [Google Scholar] [CrossRef]
- Simoen, E.; Papadimitriou, C.; Lombaert, G. On prediction error correlation in Bayesian model updating. J. Sound Vib. 2013, 332, 4136–4152. [Google Scholar] [CrossRef]
- Arendt, P.D.; Apley, D.W.; Chen, W. Quantification of model uncertainty: Calibration, model discrepancy, and identifiability. J. Mech. Des. 2012, 134. [Google Scholar] [CrossRef]
- Behmanesh, I.; Moaveni, B.; Lombaert, G.; Papadimitriou, C. Hierarchical Bayesian model updating for structural identification. Mech. Syst. Signal Process. 2015, 64, 360–376. [Google Scholar] [CrossRef]
- Vanik, M.W.; Beck, J.L.; Au, S. Bayesian probabilistic approach to structural health monitoring. J. Eng. Mech. 2000, 126, 738–745. [Google Scholar] [CrossRef]
- Simoen, E.; De Roeck, G.; Lombaert, G. Dealing with uncertainty in model updating for damage assessment: A review. Mech. Syst. Signal Process. 2015, 56, 123–149. [Google Scholar] [CrossRef]
- Gelman, A.; Stern, H.S.; Carlin, J.B.; Dunson, D.B.; Vehtari, A.; Rubin, D.B. Bayesian Data Analysis; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Sedehi, O.; Papadimitriou, C.; Katafygiotis, L.S. Probabilistic hierarchical Bayesian framework for time-domain model updating and robust predictions. Mech. Syst. Signal Process. 2019, 123, 648–673. [Google Scholar] [CrossRef]
- Sedehi, O.; Katafygiotis, L.S.; Papadimitriou, C. Hierarchical Bayesian operational modal analysis: Theory and computations. Mech. Syst. Signal Process. 2020, 140, 106663. [Google Scholar] [CrossRef]
- Peeters, B.; De Roeck, G. One-year monitoring of the Z24-Bridge: Environmental effects versus damage events. Earthq. Eng. Struct. Dyn. 2001, 30, 149–171. [Google Scholar] [CrossRef]
- Farrar, C.R.; Doebling, S.W.; Cornwell, P.J.; Straser, E.G. Variability of modal parameters measured on the Alamosa Canyon Bridge. In Proceedings of the International Modal Analysis Conference, Orlando, FL, USA, 3–6 February 1997. [Google Scholar]
- Sohn, H.; Dzwonczyk, M.; Straser, E.G.; Kiremidjian, A.S.; Law, K.H.; Meng, T. An experimental study of temperature effect on modal parameters of the Alamosa Canyon Bridge. Earthq. Eng. Struct. Dyn. 1999, 28, 879–897. [Google Scholar] [CrossRef]
- Clinton, J.F.; Bradford, S.C.; Heaton, T.H.; Favela, J. The observed wander of the natural frequencies in a structure. Bull. Seismol. Soc. Am. 2006, 96, 237–257. [Google Scholar] [CrossRef]
- Moser, P.; Moaveni, B. Environmental effects on the identified natural frequencies of the Dowling Hall Footbridge. Mech. Syst. Signal Process. 2011, 25, 2336–2357. [Google Scholar] [CrossRef]
- Ballesteros, G.C.; Angelikopoulos, P.; Papadimitriou, C.; Koumoutsakos, P. Bayesian hierarchical models for uncertainty quantification in structural dynamics. Vulnerability Uncertain. Risk. 2014, 1615–1624. [Google Scholar] [CrossRef]
- Song, M.; Astroza, R.; Ebrahimian, H.; Moaveni, B.; Papadimitriou, C. Adaptive Kalman filters for nonlinear finite element model updating. Mech. Syst. Signal Process. 2020, 143, 106837. [Google Scholar] [CrossRef]
- Sedehi, O.; Papadimitriou, C.; Katafygiotis, L.S. Data-driven uncertainty quantification and propagation in structural dynamics through a hierarchical Bayesian framework. Probabilistic Eng. Mech. 2020, 60, 103047. [Google Scholar] [CrossRef]
- Behmanesh, I.; Moaveni, B. Accounting for environmental variability, modeling errors, and parameter estimation uncertainties in structural identification. J. Sound Vib. 2016, 374, 92–110. [Google Scholar] [CrossRef]
- Behmanesh, I.; Yousefianmoghadam, S.; Nozari, A.; Moaveni, B.; Stavridis, A. Uncertainty quantification and propagation in dynamic models using ambient vibration measurements, application to a 10-story building. Mech. Syst. Signal Process. 2018, 107, 502–514. [Google Scholar] [CrossRef]
- Song, M.; Moaveni, B.; Papadimitriou, C.; Stavridis, A. Accounting for amplitude of excitation in model updating through a hierarchical Bayesian approach: Application to a two-story reinforced concrete building. Mech. Syst. Signal Process. 2019, 123, 68–83. [Google Scholar] [CrossRef]
- Song, M.; Behmanesh, I.; Moaveni, B.; Papadimitriou, C. Hierarchical Bayesian Calibration and Response Prediction of a 10-Story Building Model, In Model Validation and Uncertainty Quantification; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Song, M.; Behmanesh, I.; Moaveni, B.; Papadimitriou, C. Modeling Error Estimation and Response Prediction of a 10-Story Building Model through a Hierarchical Bayesian Model Updating Framework. Front. Built Environ. 2019, 5, 7. [Google Scholar] [CrossRef]
- Sevieri, G.; De Falco, A. Dynamic structural health monitoring for concrete gravity dams based on the Bayesian inference. J. Civ. Struct. Health Monit. 2020, 10, 235–250. [Google Scholar] [CrossRef]
- Papadimitriou, C.; Lombaert, G. The effect of prediction error correlation on optimal sensor placement in structural dynamics. Mech. Syst. Signal Process. 2012, 28, 105–127. [Google Scholar] [CrossRef]
- Andrieu, C.; Thoms, J. A tutorial on adaptive MCMC. Stat. Comput. 2008, 18, 343–373. [Google Scholar] [CrossRef]
- Kruschke, J. Doing Bayesian Data Analysis: A Tutorial with R; Academic Press: Massachusetts, UK, 2014. [Google Scholar]
- Sevieri, G.; Andreini, M.; De Falco, A.; Matthies, H.G. Concrete gravity dams model parameters updating using static measurements. Eng. Struct. 2019, 196, 109231. [Google Scholar] [CrossRef]
- Peeters, B.; De Roeck, G. Reference-based stochastic subspace identification for output-only modal analysis. Mech. Syst. Signal Process. 1999, 13, 855–878. [Google Scholar] [CrossRef]
- Available online: https://www.mathworks.com/products/matlab.html (accessed on 20 May 2020).
- Juang, J.N.; Pappa, R.S. An eigensystem realization algorithm for modal parameter identification and model reduction. J. Guid. Control. Dyn. 1985, 8, 620–627. [Google Scholar] [CrossRef]
- James, G.; Carne, T.G.; Lauffer, J.P. The natural excitation technique (NExT) for modal parameter extraction from operating structures. Modal Anal.-Int. J. Anal. Exp. Modal Anal. 1995, 10, 260. [Google Scholar]
- Available online: https://opensees.berkeley.edu/workshop/neesOSworkshopSept2004_presentations/B9%20FEDEASLab%20Presentation.pdf (accessed on 22 May 2020).
- Yousefianmoghadam, S.; Song, M.; Stavridis, A.; Moaveni, B. System identification of a two-story infilled RC building in different damage states. Improv. Seism. Perform. Exist. Build. Other Struct. 2015, 2015, 607–618. [Google Scholar]
- Yousefianmoghadam, S.; Song, M.; Mohammadi, M.E.; Packard, B.; Stavridis, A.; Moaveni, B.; Wood, R.L. Nonlinear dynamic tests of a reinforced concrete frame building at different damage levels. Earthq. Eng. Struct. Dyn. 2020, 49, 924–945. [Google Scholar] [CrossRef]
- Yousefianmoghadam, S. Investigation of the Linear and Non-linear Dynamic Behavior of Actual RC Buildings through Tests and Simulations. Ph.D. Thesis, University at Buffalo, Buffalo, NY, USA, 2018. [Google Scholar]
- Available online: https://opensees.berkeley.edu/ (accessed on 25 May 2020).






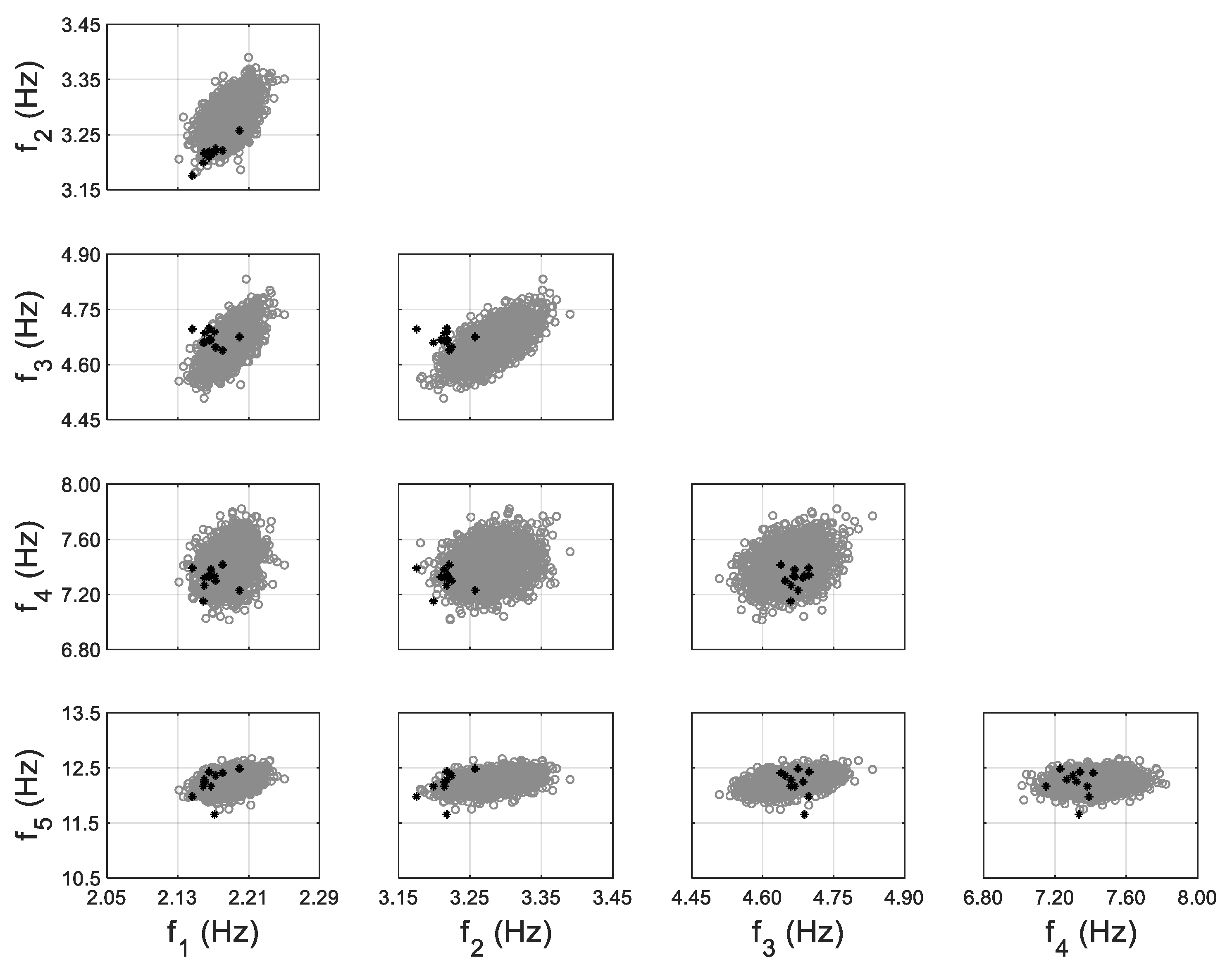
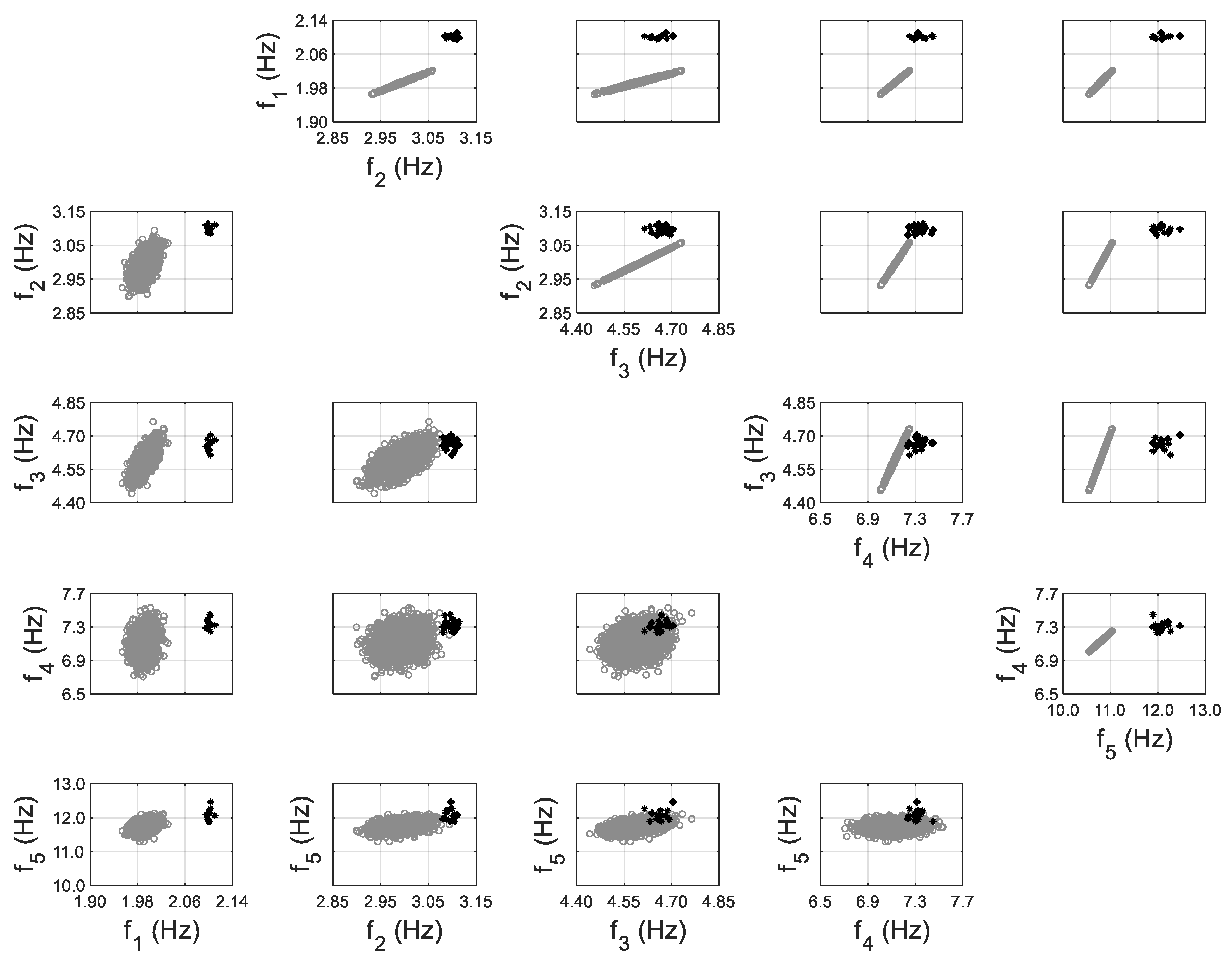


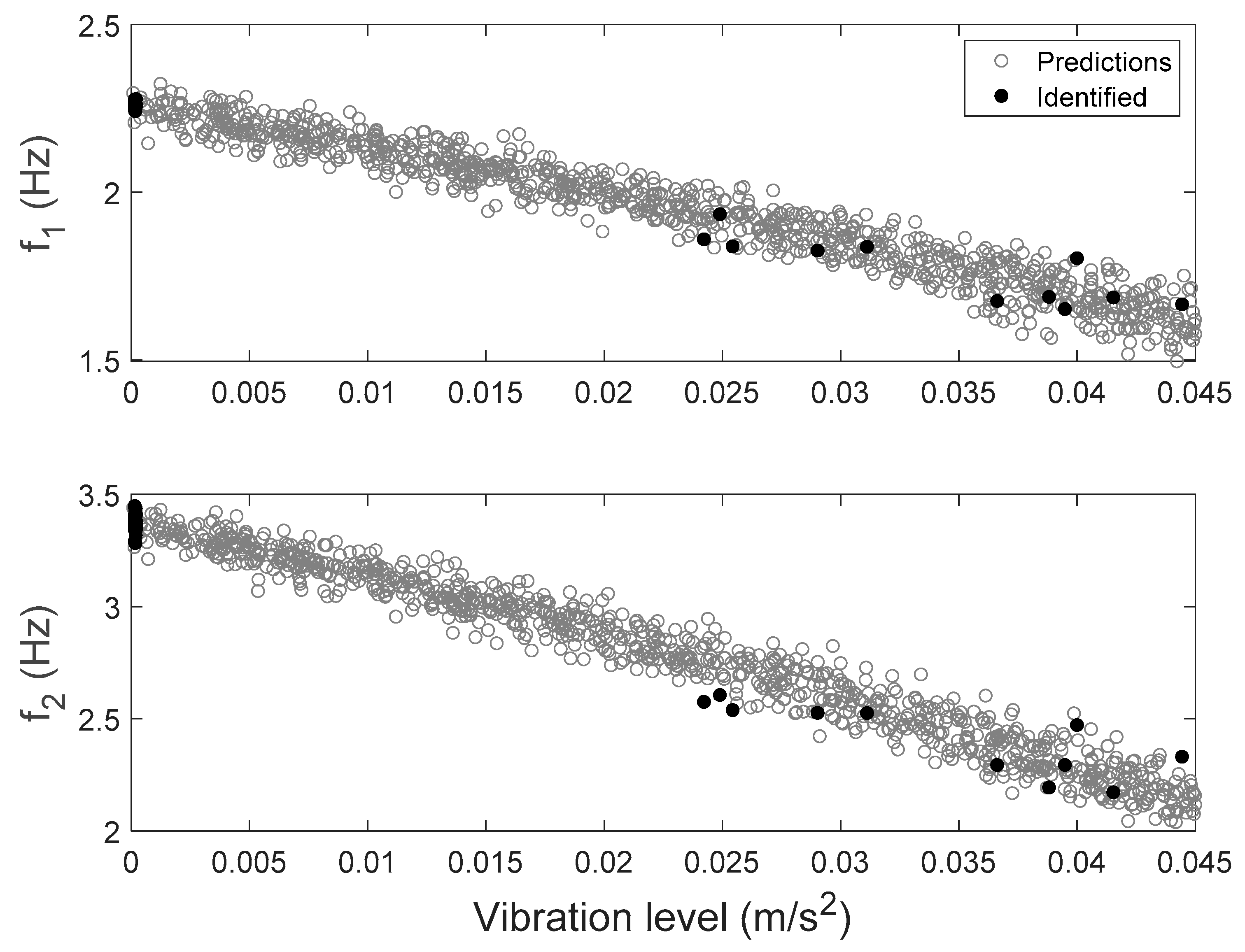

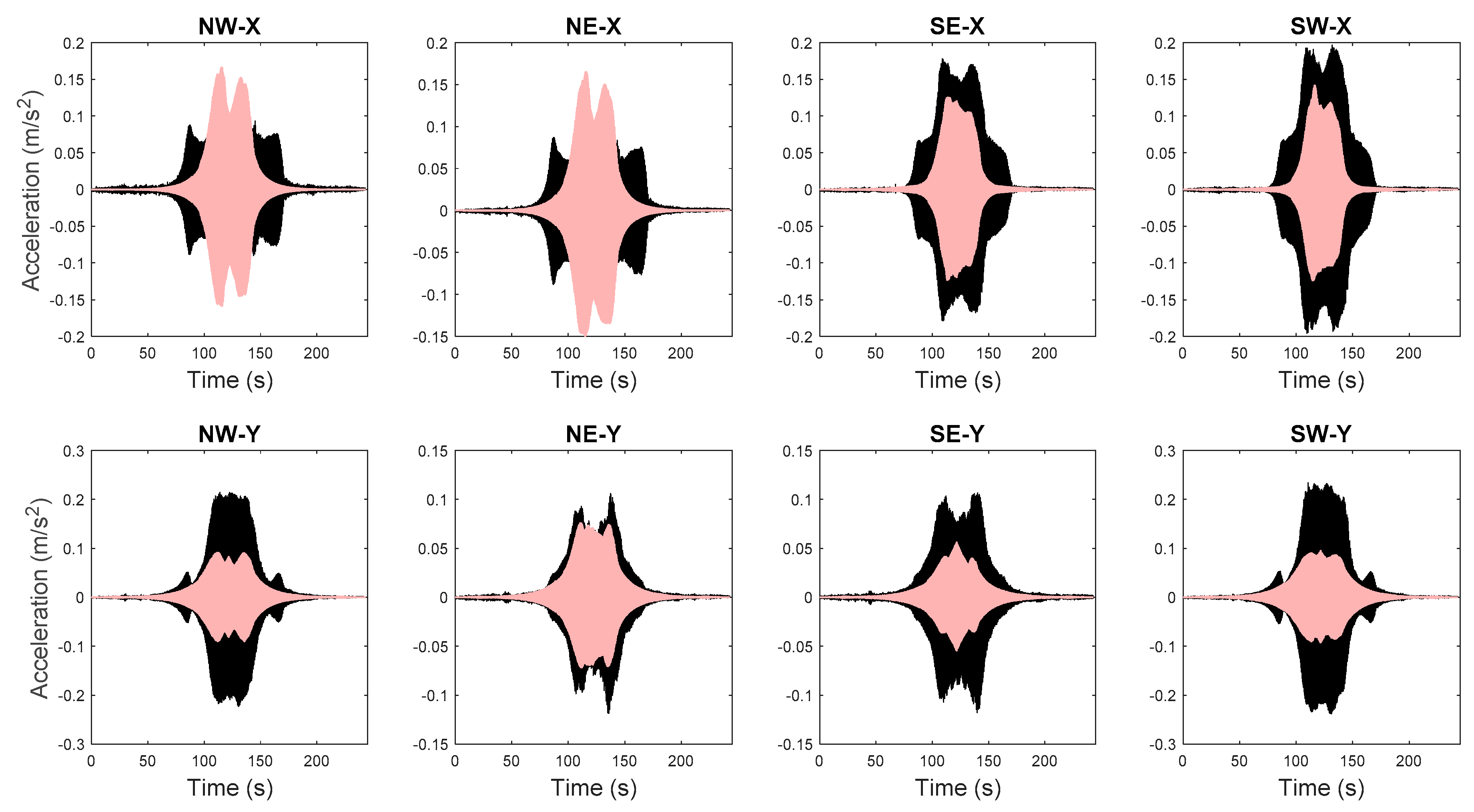
| Q | S | R | ϒ | Y | ||||
|---|---|---|---|---|---|---|---|---|
| Mean | 1.013 | −0.005 | 0.198 | −1.101 | 3.147 | −0.013 | 0.040 | −4.254 |
| Standard deviation | 0.0030 | 0.0001 | 0.0027 | 0.0513 | 0.0861 | 0.0004 | 0.0002 | 0.0012 |
| Zero-Mean Error Function | Non-Zero-Mean Error Function for 5th Mode | ||
|---|---|---|---|
| Hyperparameters | 0.995 | 0.995 | |
| 1.132 | 1.129 | ||
| 0.021 | 0.021 | ||
| 0.015 | 0.014 | ||
| Error covariance (for eigenvalues) | 0.010 | 0.011 | |
| 0.014 | 0.014 | ||
| 0.007 | 0.007 | ||
| 0.034 | 0.034 | ||
| 0.188 | 0.021 | ||
| Error mean | 0 | −0.185 |
| Hyperparameters | 0.11 | −2.41 | 0.011 | Error covariance | 0.02 | ||
| 0.85 | −4.68 | 0.008 | 0.98 | ||||
| 0.08 | −0.44 | 0.010 | 1.32 | ||||
| 0.40 | −4.91 | 0.017 | 1.98 | ||||
| 1.82 | −22.66 | 0.065 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, M.; Behmanesh, I.; Moaveni, B.; Papadimitriou, C. Accounting for Modeling Errors and Inherent Structural Variability through a Hierarchical Bayesian Model Updating Approach: An Overview. Sensors 2020, 20, 3874. https://doi.org/10.3390/s20143874
Song M, Behmanesh I, Moaveni B, Papadimitriou C. Accounting for Modeling Errors and Inherent Structural Variability through a Hierarchical Bayesian Model Updating Approach: An Overview. Sensors. 2020; 20(14):3874. https://doi.org/10.3390/s20143874
Chicago/Turabian StyleSong, Mingming, Iman Behmanesh, Babak Moaveni, and Costas Papadimitriou. 2020. "Accounting for Modeling Errors and Inherent Structural Variability through a Hierarchical Bayesian Model Updating Approach: An Overview" Sensors 20, no. 14: 3874. https://doi.org/10.3390/s20143874
APA StyleSong, M., Behmanesh, I., Moaveni, B., & Papadimitriou, C. (2020). Accounting for Modeling Errors and Inherent Structural Variability through a Hierarchical Bayesian Model Updating Approach: An Overview. Sensors, 20(14), 3874. https://doi.org/10.3390/s20143874








