A Seven-Rod Dielectric Sensor for Determination of Soil Moisture in Well-Defined Sample Volumes
Abstract
1. Introduction
- -
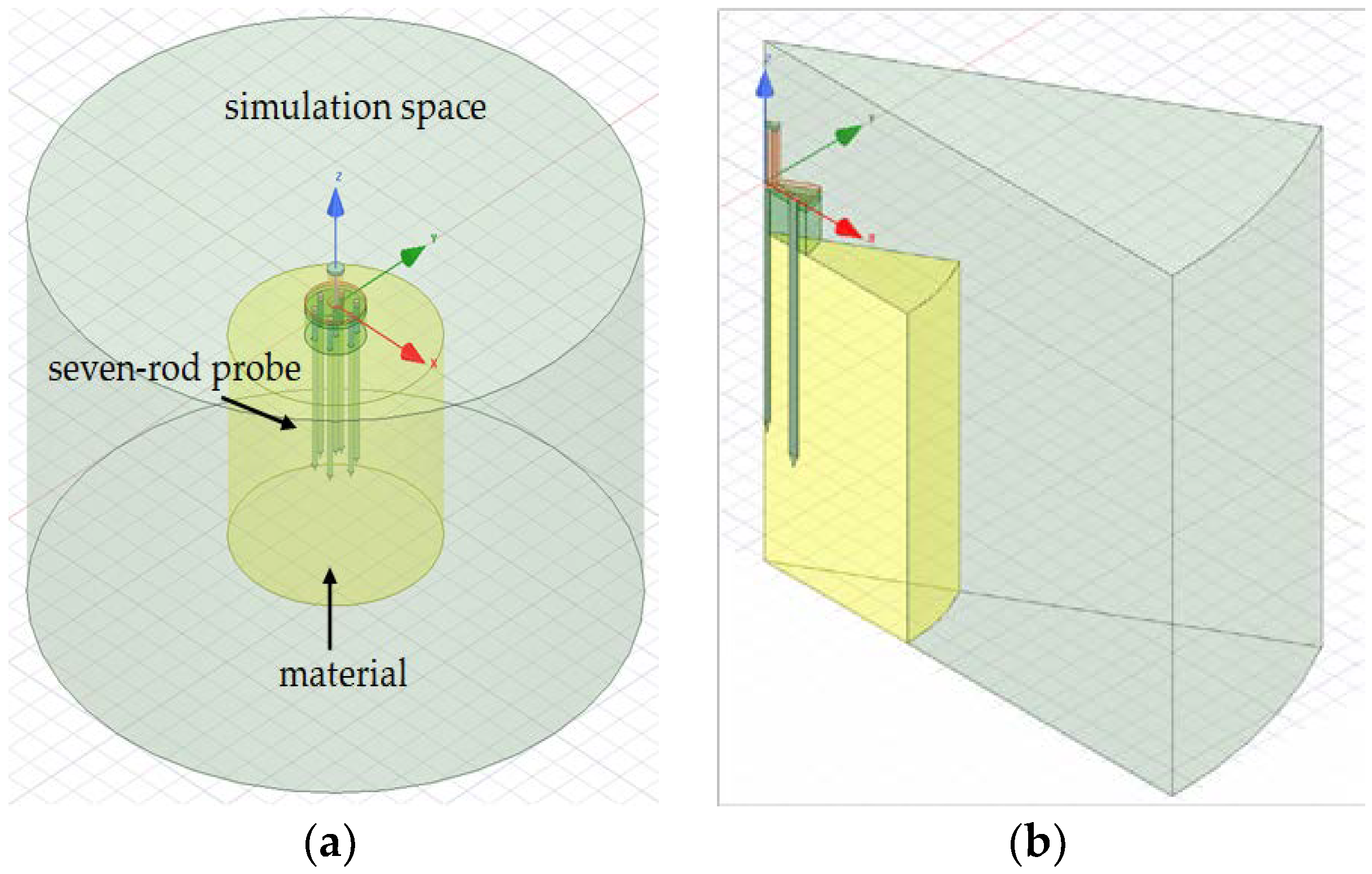
- electromagnetic simulations used to verify the sensitivity zone of the probe using Ansys HFSS software;
- -
- measurements of the values of three soils of different texture and moisture content using the seven-rod probe connected to a vector network analyzer (VNA) in the determined operational frequency range of the probe;
- -
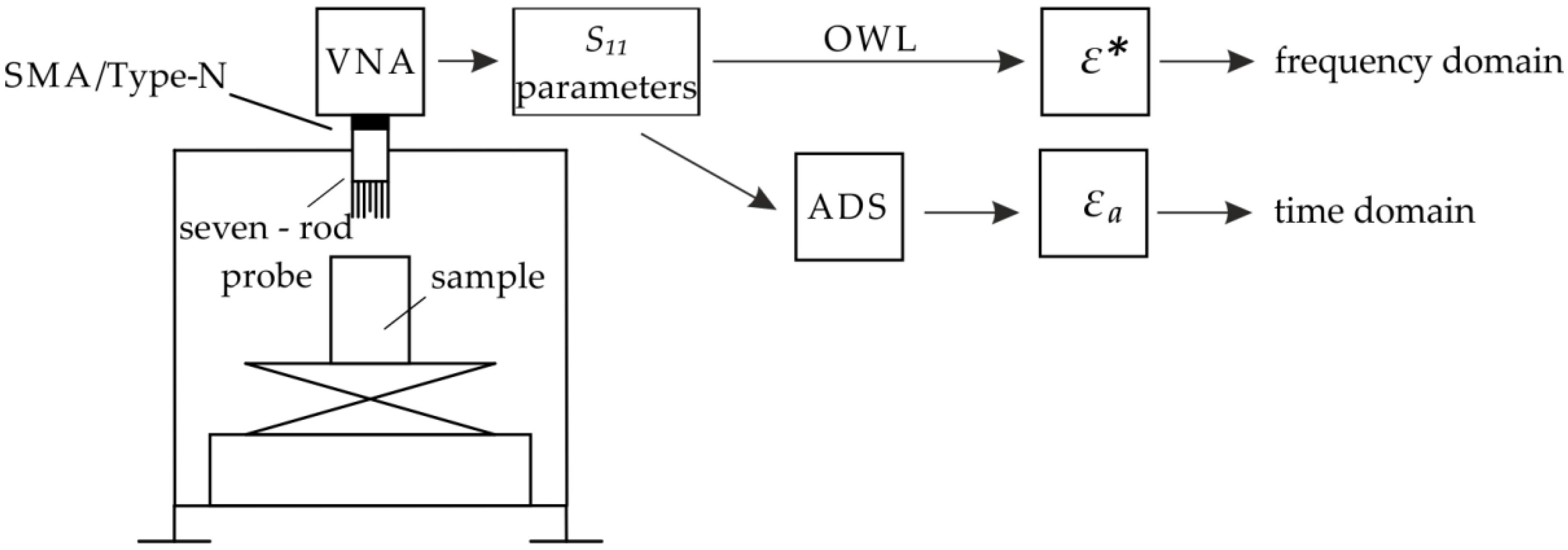
- conversion of parameters obtained from VNA measurements to complex permittivity using a bilinear equation (Open-Water-Liquid calibration);
- -
- transient-state electronic simulations in the Keysight Advanced Design System (ADS), which enabled converting the parameters measured by the VNA from the frequency domain to the time domain in order to obtain and to verify that the probe could be used also as a TDR probe;
- -
- a comparison between , , and volumetric moisture content from the experiment;
- -
- determination of the permittivity–moisture content calibration curve and its comparison to Topp’s equation as reference.
2. Materials and Methods
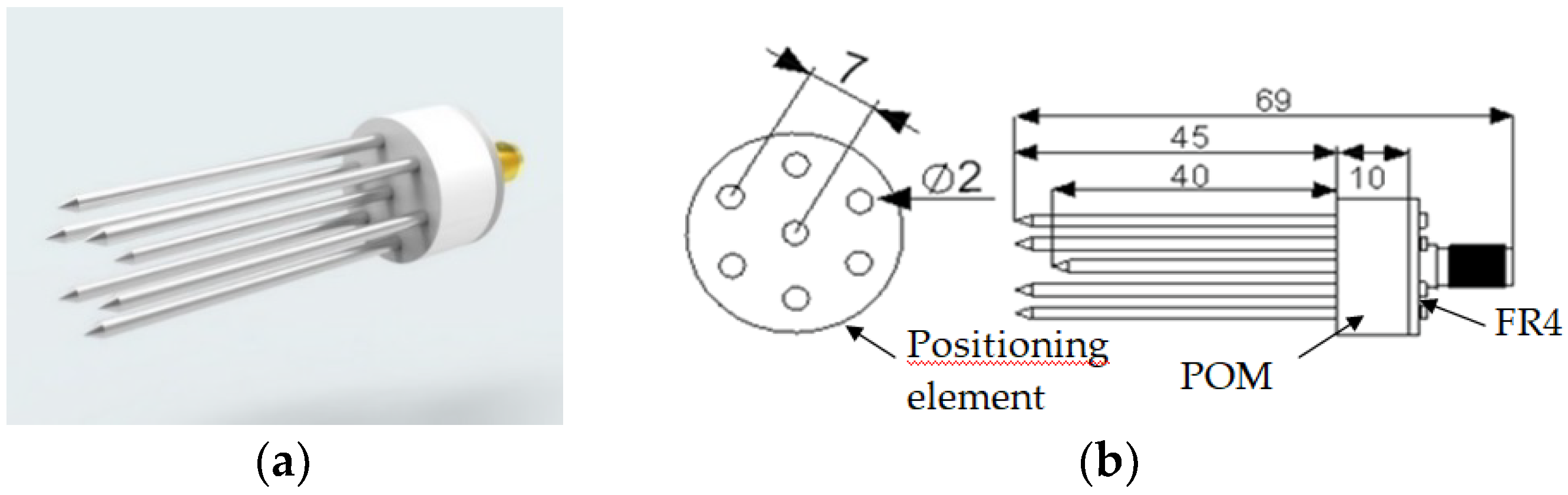
2.1. Sensitivity Zone—Simulation and Experiment
2.2. Soil Characteristics and Measurements
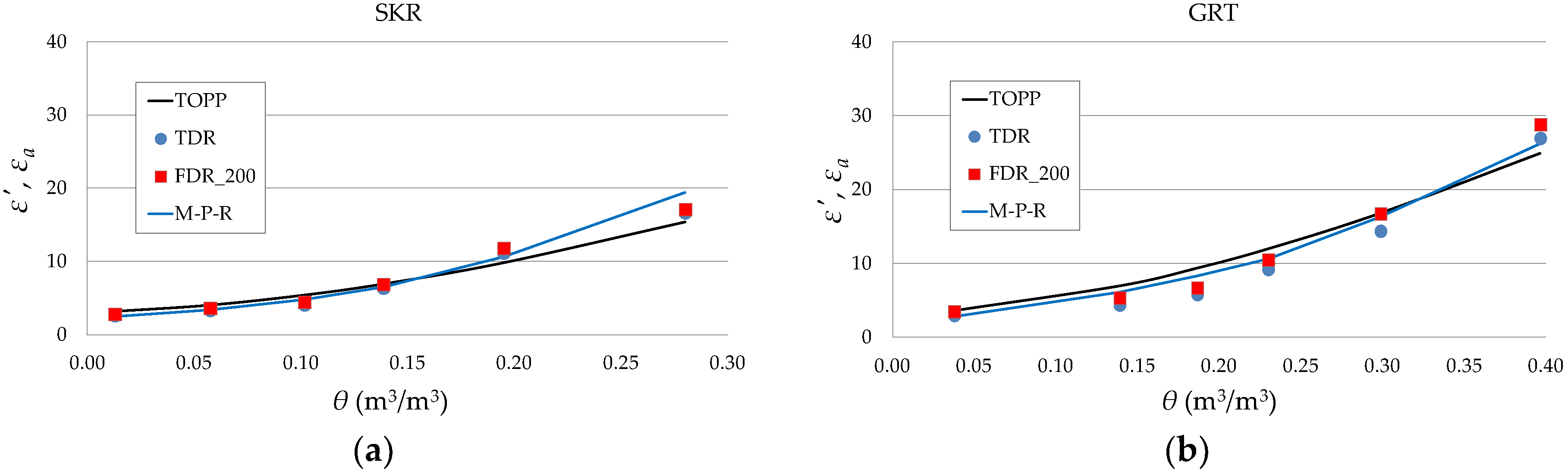
3. Results and Discussion
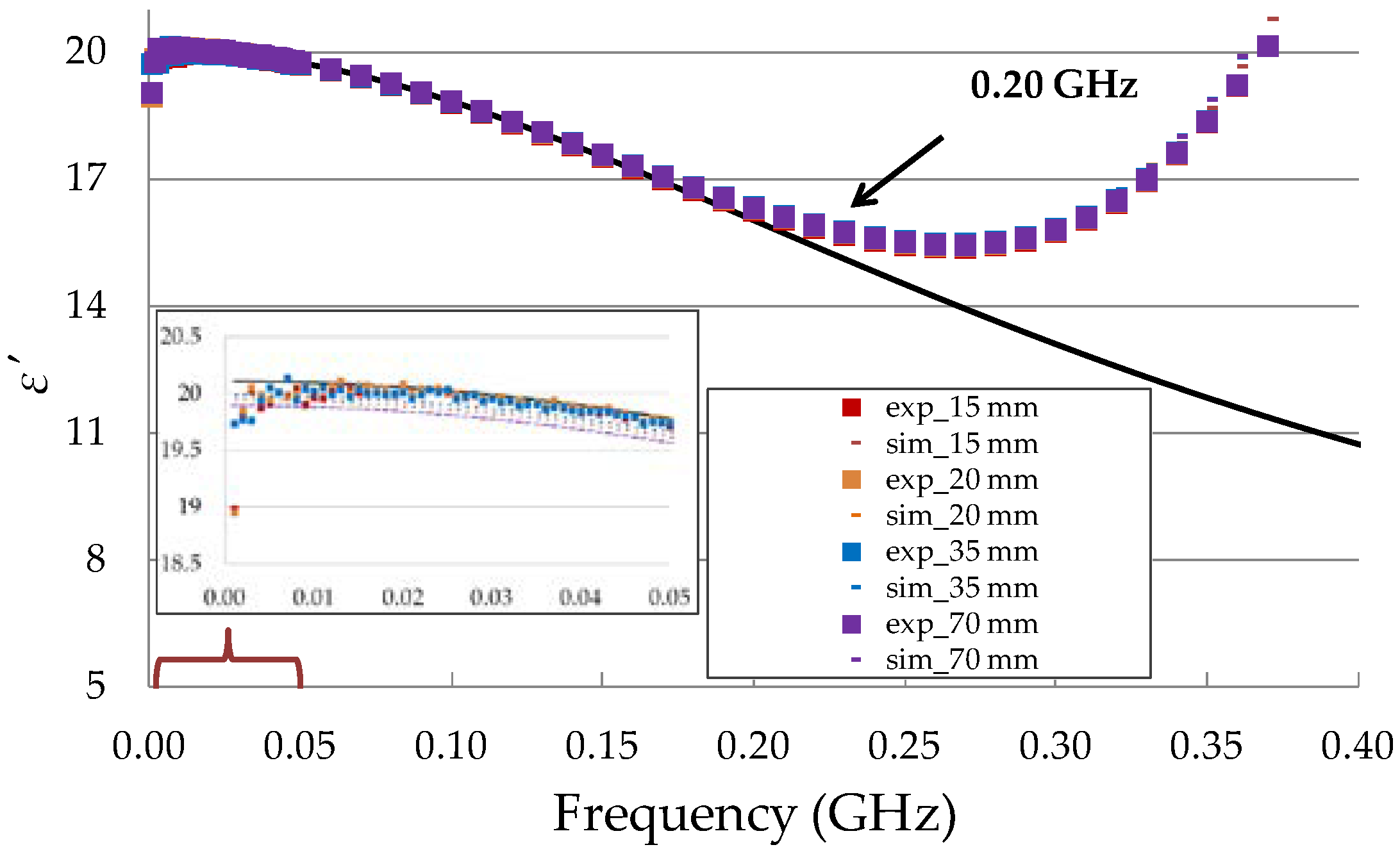
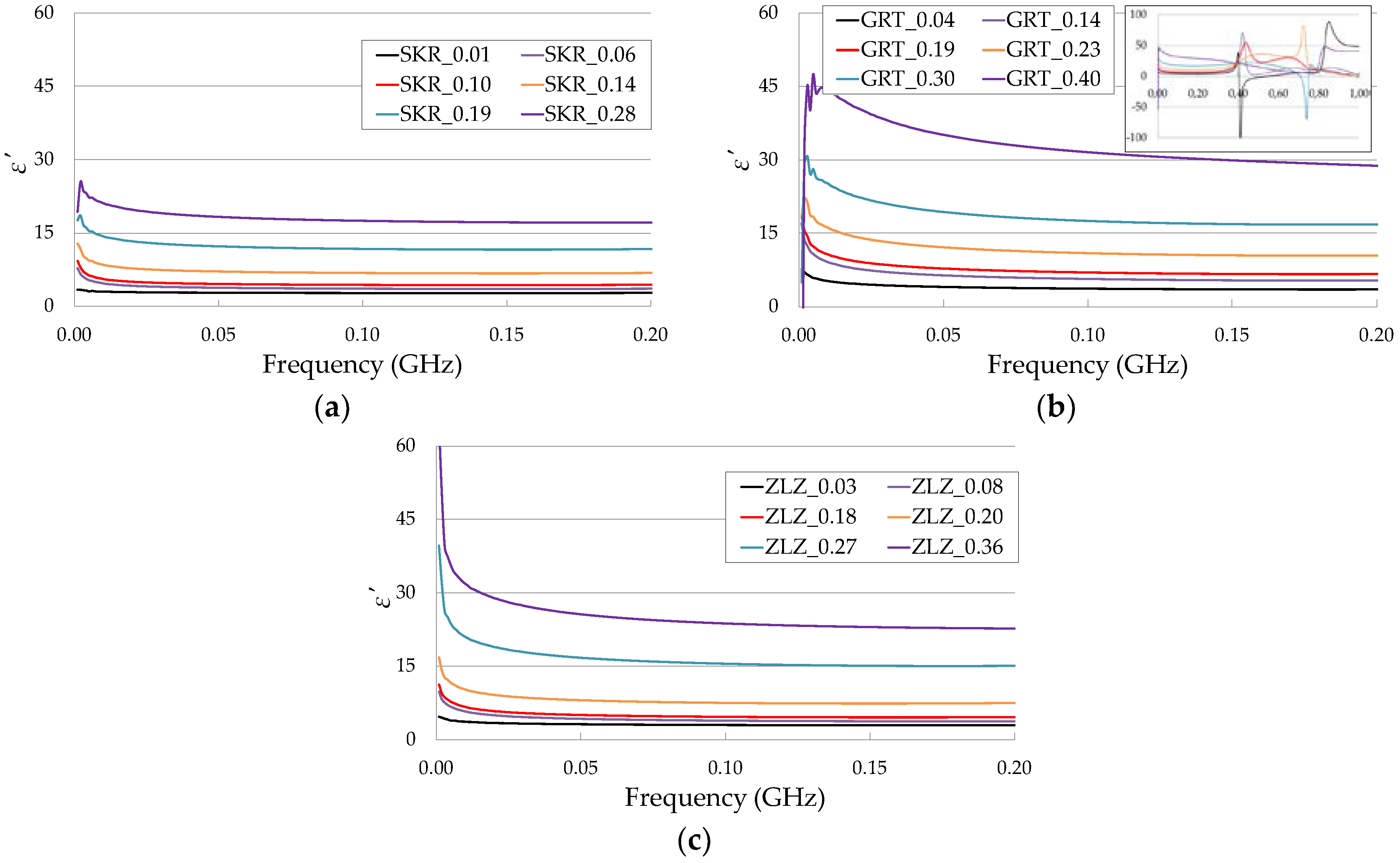
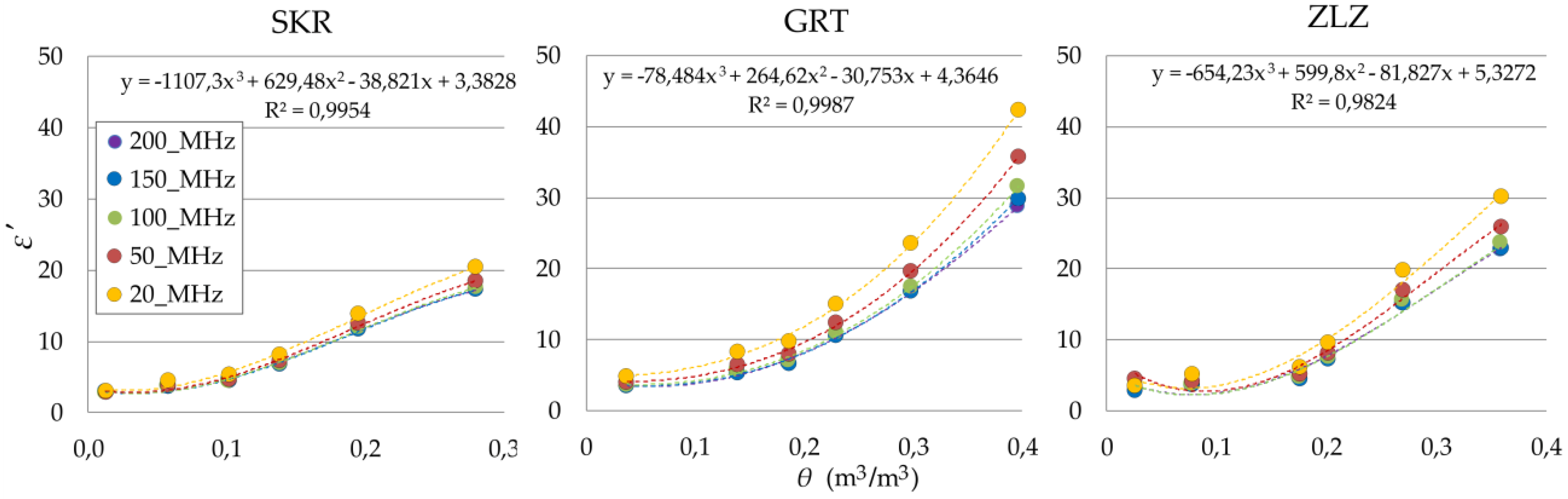
3.1. Frequency Domain Analysis
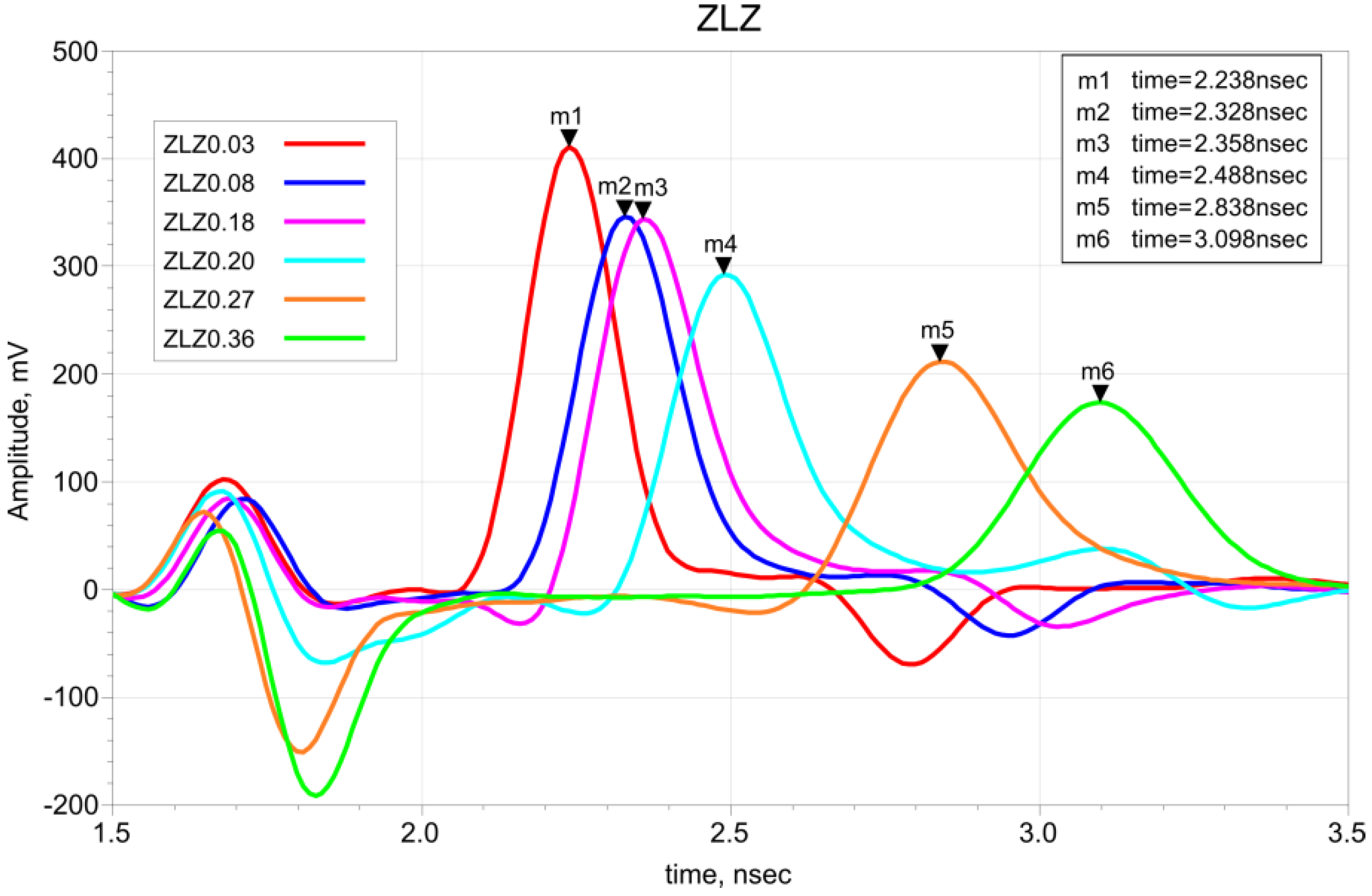
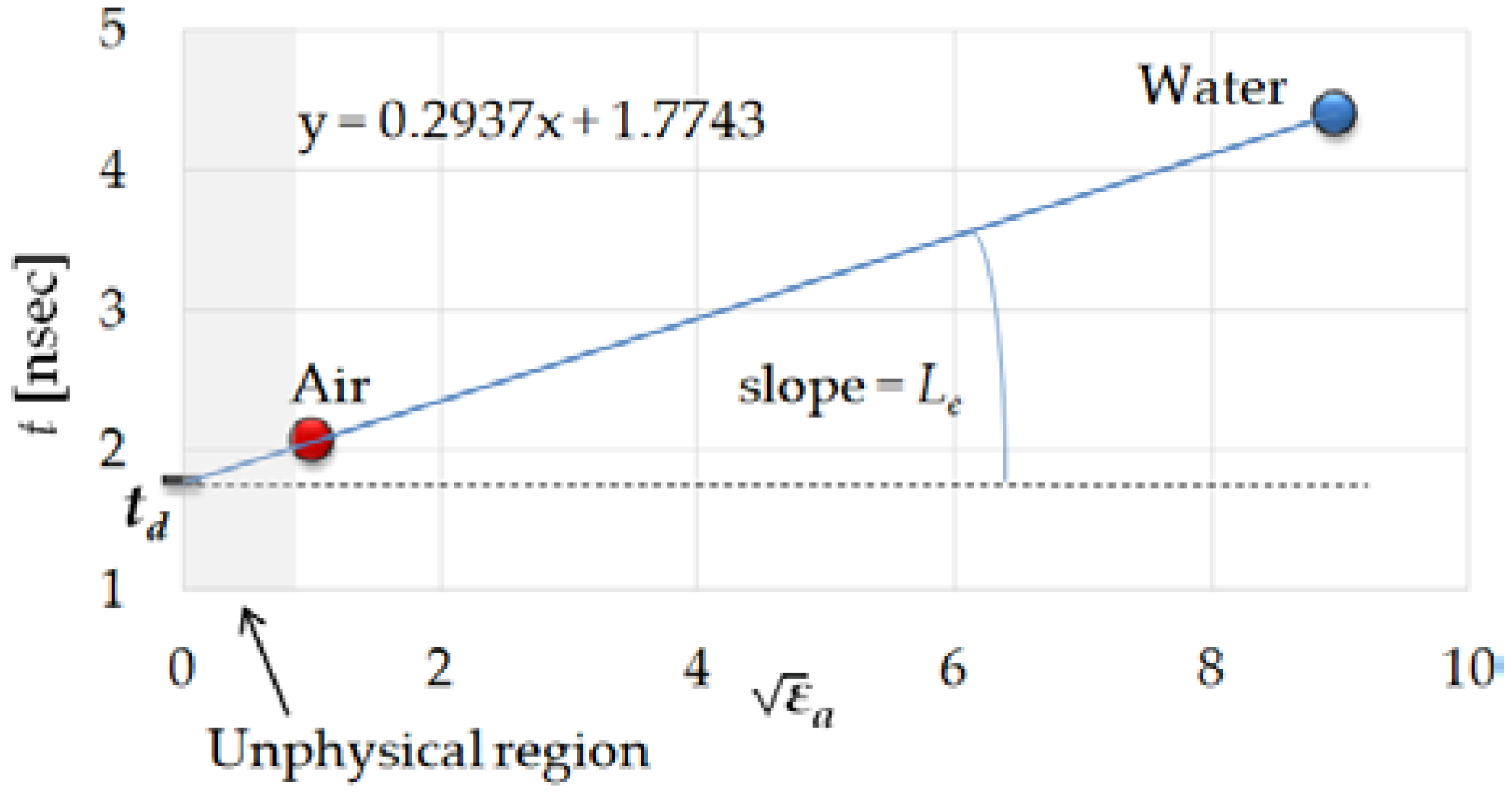
3.2. Time Domain Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Suchorab, Z.; Widomski, M.K.; Łagód, G.; Barnat-Hunek, D.; Majerek, D. A Noninvasive TDR Sensor to Measure the Moisture Content of Rigid Porous Materials. Sensors 2018, 18, 3935. [Google Scholar] [CrossRef]
- Dobriyal, P.; Qureshi, A.; Badola, R.; Hussain, S.A. A review of the methods available for estimating soil moisture and its implications for water resource management. J. Hydrol. 2012, 458–459, 110–117. [Google Scholar] [CrossRef]
- Nosalewicz, A.; Siecińska, J.; Kondracka, K.; Nosalewicz, M. The functioning of Festuca arundinacea and Lolium perenne under drought is improved to a different extend by the previous exposure to water deficit. Environ. Exp. Bot. 2018, 156, 271–278. [Google Scholar] [CrossRef]
- Walczak, A.; Dawid, M.; Janik, G.; Rzeszowski, M.; Skierucha, W.; Wilczek, A. Dielectric sensors in flood early warning systems. In Proceedings of the 12th International Conference on Electromagnetic Wave Interaction with Water and Moist Substances (ISEMA), Lublin, Poland, 4–7 June 2018. [Google Scholar]
- Skierucha, W.; Wilczek, A.; Szypłowska, A. Dielectric spectroscopy in agrophysics. Int. Agrophys. 2012, 26, 187–197. [Google Scholar] [CrossRef]
- Sandrolini, L.; Reggiani, U.; Artioli, M. Electric and Magnetic Characterization of Materials. In Behaviour of Electromagnetic Waves in Different Media and Structures; InTech: Rijeka, Croatia, 2011. [Google Scholar]
- Skierucha, W.; Wilczek, A.; Szypłowska, A.; Sławiński, C.; Lamorski, K. A TDR-based soil moisture monitoring system with simultaneous measurement of soil temperature and electrical conductivity. Sensors 2012, 12, 13545–13566. [Google Scholar] [CrossRef] [PubMed]
- Or, D.; Wraith, J.M.; Friedman, S.P.; Jones, S.B.; Robinson, D.A. A Review of Advances in Dielectric and Electrical Conductivity Measurement in Soils Using Time Domain Reflectometry. Vadose Zone J. 2013, 2, 444–475. [Google Scholar]
- Canone, D.; Previati, M.; Ferraris, S.; Haverkamp, R. A new coaxial time domain reflectometry probe for water content measurement in forest floor litter. Vadose Zone J. 2009, 8, 363–372. [Google Scholar] [CrossRef]
- Topp, G.C.; Davis, J.L.; Annan, A.P. Electromagnetic determination of soil water content: Measurements in coaxial transmission lines. Water Resour. Res. 1980, 16, 574–582. [Google Scholar] [CrossRef]
- Skierucha, W.; Wilczek, A. A FDR sensor for measuring complex soil dielectric permittivity in the 10–500 MHz frequency range. Sensors 2010, 10, 3314–3329. [Google Scholar] [CrossRef] [PubMed]
- Wilczek, A.; Szyplowska, A.; Skierucha, W.; Cieśla, J.; Pichler, V.; Janik, G. Determination of soil pore water salinity using an FDR sensor working at various frequencies up to 500 MHz. Sensors 2012, 12, 10890–10905. [Google Scholar] [CrossRef]
- Matula, S.; Bát’ková, K.; Legese, W.L. Laboratory performance of five selected soil moisture sensors applying factory and own calibration equations for two soil media of different bulk density and salinity levels. Sensors 2016, 16, 1912. [Google Scholar] [CrossRef]
- Chen, Y.; Or, D. Effects of Maxwell-Wagner polarization on soil complex dielectric permittivity under variable temperature and electrical conductivity. Water Resour. Res. 2006, 42, 1–14. [Google Scholar] [CrossRef]
- Logsdon, S.; Laird, D. Cation and Water Content Effects on Dipole Rotation Activation Energy of Smectites. Soil Sci. Soc. Am. J. 2010, 68, 1586. [Google Scholar] [CrossRef]
- Saarenketo, T. Electrical properties of water in clay and silty soils. J. Appl. Geophys. 1998, 40, 73–88. [Google Scholar] [CrossRef]
- Xu, J.; Ma, X.; Logsdon, S.D.; Horton, R. Short, Multineedle Frequency Domain Reflectometry Sensor Suitable for Measuring Soil Water Content. Soil Sci. Soc. Am. J. 2012, 76, 1929. [Google Scholar] [CrossRef]
- Szypłwoska, A.; Kafarski, M.; Wilczek, A.; Lewandowski, A.; Skierucha, W. Salinity index determination of Poros materials Rusing open-ended probes. Meas. Sci. Technol. 2017, 28, 014006. [Google Scholar] [CrossRef]
- La Gioia, A.; Porter, E.; Shahzad, A.; Merunka, I.; O’Halloran, M.; Salahuddin, S.; Jones, M. Open-Ended Coaxial Probe Technique for Dielectric Measurement of Biological Tissues: Challenges and Common Practices. Diagnostics 2018, 8, 40. [Google Scholar] [CrossRef] [PubMed]
- Szypłowska, A.; Wilczek, A.; Kafarski, M.; Skierucha, W. Soil Complex Dielectric Permittivity Spectra Determination Using Electrical Signal Reflections in Probes of Various Lengths. Vadose Zone J. Adv. Crit. Zo. Sci. 2015, 15. [Google Scholar] [CrossRef]
- Logsdon, S.D. Soil Dielectric Spectra from Vector Network Analyzer Data. Soil Sci. Soc. Am. J. 2010, 69, 983. [Google Scholar] [CrossRef]
- Lewandowski, A.; Wilczek, A.; Szypłowska, A.; Kafarski, M.; Szerement, J.; Skierucha, W. A Multiconductor Probe for Broadband Dielectric-Spectrum Measurements. In Proceedings of the 2018 12th International Conference on Electromagnetic Wave Interaction with Water and Moist Substances (ISEMA), Lublin, Poland, 4–7 June 2018. [Google Scholar]
- Kafarski, M.; Wilczek, A.; Szypłowska, A.; Lewandowski, A.; Pieczywek, P.; Janik, G.; Skierucha, W. Evaluation of apple maturity with two types of dielectric probes. Sensors 2018, 18, 121. [Google Scholar] [CrossRef]
- Bao, J.Z.; Davis, C.C.; Swicord, M.L. Microwave dielectric measurements of erythrocyte suspensions. Biophys. J. 1994, 66, 2173–2180. [Google Scholar] [CrossRef]
- Buckley, F.; Maryott, A.A. Table of Dielectric Dispersion Data for Pure Liquids and Dilute Solutions; Bureau of Standards Circular. Natl. Bur. Stand. Circ. 1958, 589, 7–8. [Google Scholar]
- Bieganowski, A.; Ryżak, M.; Sochan, A.; Barna, G.; Beczek, M.; Polakowski, C.; Makó, A. Laser Diffractometry in the Measurements of Soil and Sediment Particle Size Distribution. Adv. Agron. 2018, 151, 215–279. [Google Scholar]
- Pennell, K.D. Specific Surface Area Specific Surface Area; Elsevier Inc.: Amsterdam, The Netherlands, 2017; ISBN 9780124095489. [Google Scholar]
- PN-ISO 11465:1999. Jakość Gleby. Oznaczanie Zawartości Suchej Masy Gleby i Wody w Glebie w Przeliczeniu na Suchą Masę Gleby. Metoda Wagowa. (Soil quality. Determination of Dry Matter and Water Content on a Mass Basis. Gravimetric Method). Warsaw, Poland, 1999. Available online: http://sklep.pkn.pl/pn-iso-11465-1999p.html (accessed on 6 April 2019).
- Wilczek, A.; Szypłowska, A.; Kafarski, M.; Skierucha, W. A time-domain reflectometry method with variable needle pulse width for measuring the dielectric properties of materials. Sensors 2016, 16, 191. [Google Scholar] [CrossRef] [PubMed]
- Brochure—EEsof EDA EMPro 3D Electromagnetic Modeling and Simulation Environment Integrated with Your ADS Design Flow; Keysight Technologies: Santa Rosa, CA, USA, 2017.
- Skierucha, W.; Wilczek, A.; Kafarski, M.; Szyplowska, A. Estimation of Electromagnetic Sensor Measurement Volume Using Combined 3D EM Simulation and Electronic Design Software. In Proceedings of the 2018 12th International Conference on Electromagnetic Wave Interaction with Water and Moist Substances (ISEMA), Lublin, Poland, 4–7 June 2018; pp. 1–9. [Google Scholar]
- Liu, C.; Zhang, L.; Peng, J.; Srinivasakannan, C.; Liu, B.; Xia, H.; Zhou, J.; Xu, L. Temperature and moisture dependence of the dielectric properties of silica sand. J. Microw. Power Electromagn. Energy 2013, 47, 199–209. [Google Scholar] [CrossRef]
- Kelleners, T.J.; Robinson, D.A.; Shouse, P.J.; Ayars, J.E.; Skaggs, T.H. Frequency Dependence of the Complex Permittivity and Its Impact on Dielectric Sensor Calibration in Soils This research was conducted at the George, E.; Brown, Jr. Salinity Laboratory, USDA-ARS, Riverside, CA. The mention of trade or manufacturer names is m. Soil Sci. Soc. Am. J. 2005, 69, 67–76. [Google Scholar]
- Skierucha, W.; Wilczek, A.; Alokhina, O. Calibration of a TDR probe for low soil water content measurements. Sens. Actuators A Phys. 2008, 147, 544–552. [Google Scholar] [CrossRef]
- Malicki, M.A.; Plagge, R.; Roth, C.H. Improving the calibration of dielectric TDR soil moisture determination taking into account the solid soil. Eur. J. Soil Sci. 2018, 47, 357–366. [Google Scholar] [CrossRef]
- Roth, K.; Schulin, R.; Fluhler, H.; Attinger, W. Calibration of time domain reflectometry for water content measurement using a composite dielectric approach. Water Resour. Res. 1990, 26, 2267–2273. [Google Scholar] [CrossRef]
- Keshavarzi, M.; Ojaghlou, H.; Nazemi, A.; Ashraf, S.; Ababaei, B. Effect of soil texture and organic matter on the accuracy of Time domain reflectometry method for estimating soil moisture. Soil Water Pollut. 2015, 368–373. [Google Scholar]


| Texture [%] | Specific Surface Area [m2/g] | |||
|---|---|---|---|---|
| Clay | Silt | Sand | ||
| SKR | 3.7 | 28.8 | 67.5 | 3.06 ± 0.06 |
| GRT | 10.1 | 76.5 | 13.4 | 16.40 ± 0.02 |
| ZLZ | 5.1 | 44.2 | 50.7 | 15.41 ± 0.30 |
| ϕ | Simulation | Experiment |
|---|---|---|
| 70 mm | 0.042 | 0.023 |
| 35 mm | 0.016 | 0.012 |
| 20 mm | 0.014 | 0.026 |
| 15 mm | 0.025 | 0.027 |
| SKR | GRT | ZLZ | |||
|---|---|---|---|---|---|
| θ [m3/m3] | ρ [g/cm3] | θ [m3/m3] | ρ [g/cm3] | θ [m3/m3] | ρ [g/cm3] |
| 0.01 | 1.54 | 0.04 | 1.35 | 0.03 | 1.38 |
| 0.06 | 1.36 | 0.14 | 1.24 | 0.08 | 1.29 |
| 0.10 | 1.31 | 0.19 | 1.25 | 0.18 | 1.21 |
| 0.14 | 1.36 | 0.23 | 1.25 | 0.20 | 1.27 |
| 0.19 | 1.60 | 0.30 | 1.44 | 0.27 | 1.51 |
| 0.28 | 1.96 | 0.40 | 1.63 | 0.36 | 1.86 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szerement, J.; Woszczyk, A.; Szypłowska, A.; Kafarski, M.; Lewandowski, A.; Wilczek, A.; Skierucha, W. A Seven-Rod Dielectric Sensor for Determination of Soil Moisture in Well-Defined Sample Volumes. Sensors 2019, 19, 1646. https://doi.org/10.3390/s19071646
Szerement J, Woszczyk A, Szypłowska A, Kafarski M, Lewandowski A, Wilczek A, Skierucha W. A Seven-Rod Dielectric Sensor for Determination of Soil Moisture in Well-Defined Sample Volumes. Sensors. 2019; 19(7):1646. https://doi.org/10.3390/s19071646
Chicago/Turabian StyleSzerement, Justyna, Aleksandra Woszczyk, Agnieszka Szypłowska, Marcin Kafarski, Arkadiusz Lewandowski, Andrzej Wilczek, and Wojciech Skierucha. 2019. "A Seven-Rod Dielectric Sensor for Determination of Soil Moisture in Well-Defined Sample Volumes" Sensors 19, no. 7: 1646. https://doi.org/10.3390/s19071646
APA StyleSzerement, J., Woszczyk, A., Szypłowska, A., Kafarski, M., Lewandowski, A., Wilczek, A., & Skierucha, W. (2019). A Seven-Rod Dielectric Sensor for Determination of Soil Moisture in Well-Defined Sample Volumes. Sensors, 19(7), 1646. https://doi.org/10.3390/s19071646





