Magnetoconductivity and Terahertz Response of a HgCdTe Epitaxial Layer
Abstract
1. Introduction
2. Materials and Methods
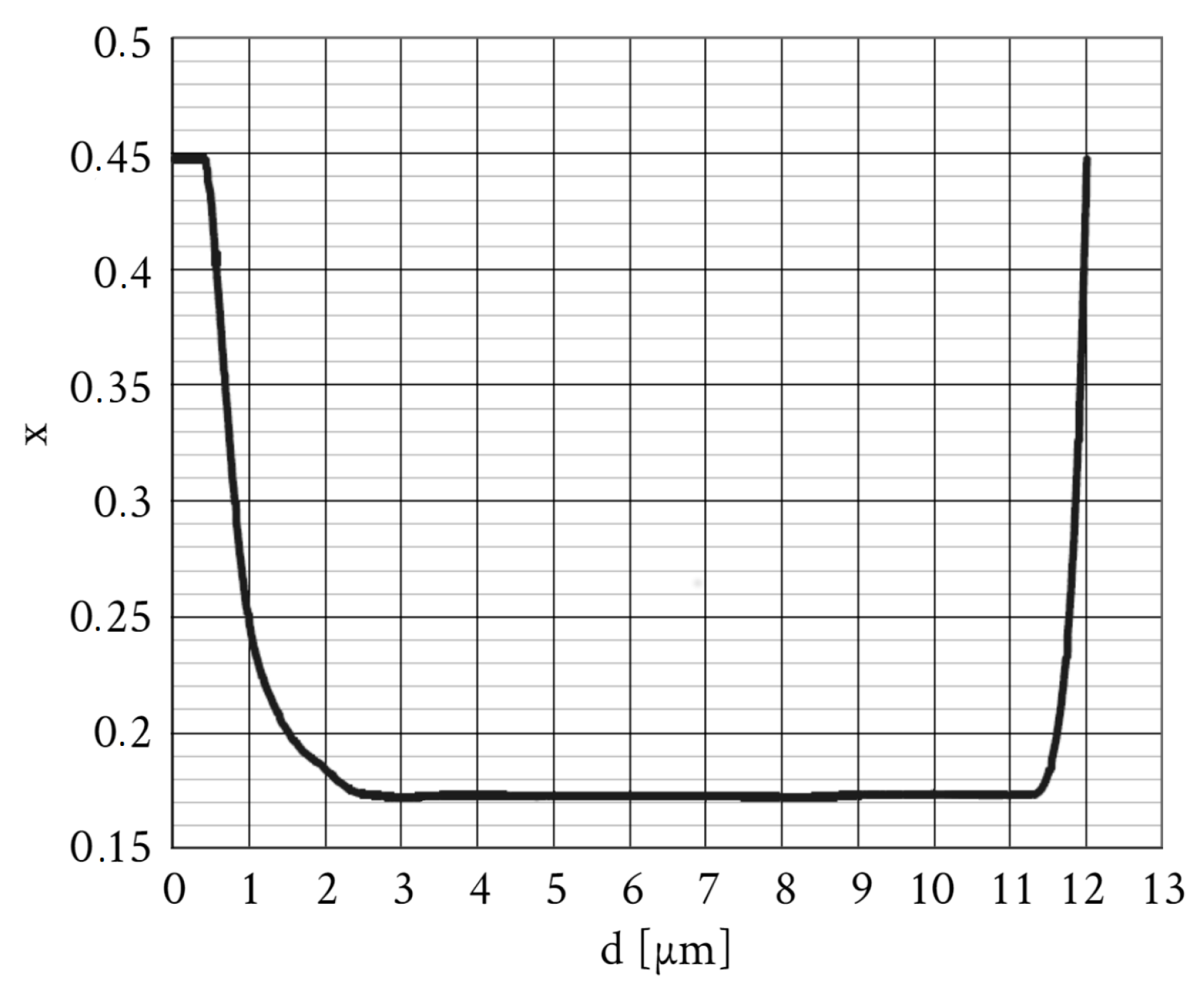
The Sample and Experimental Procedures
3. Results
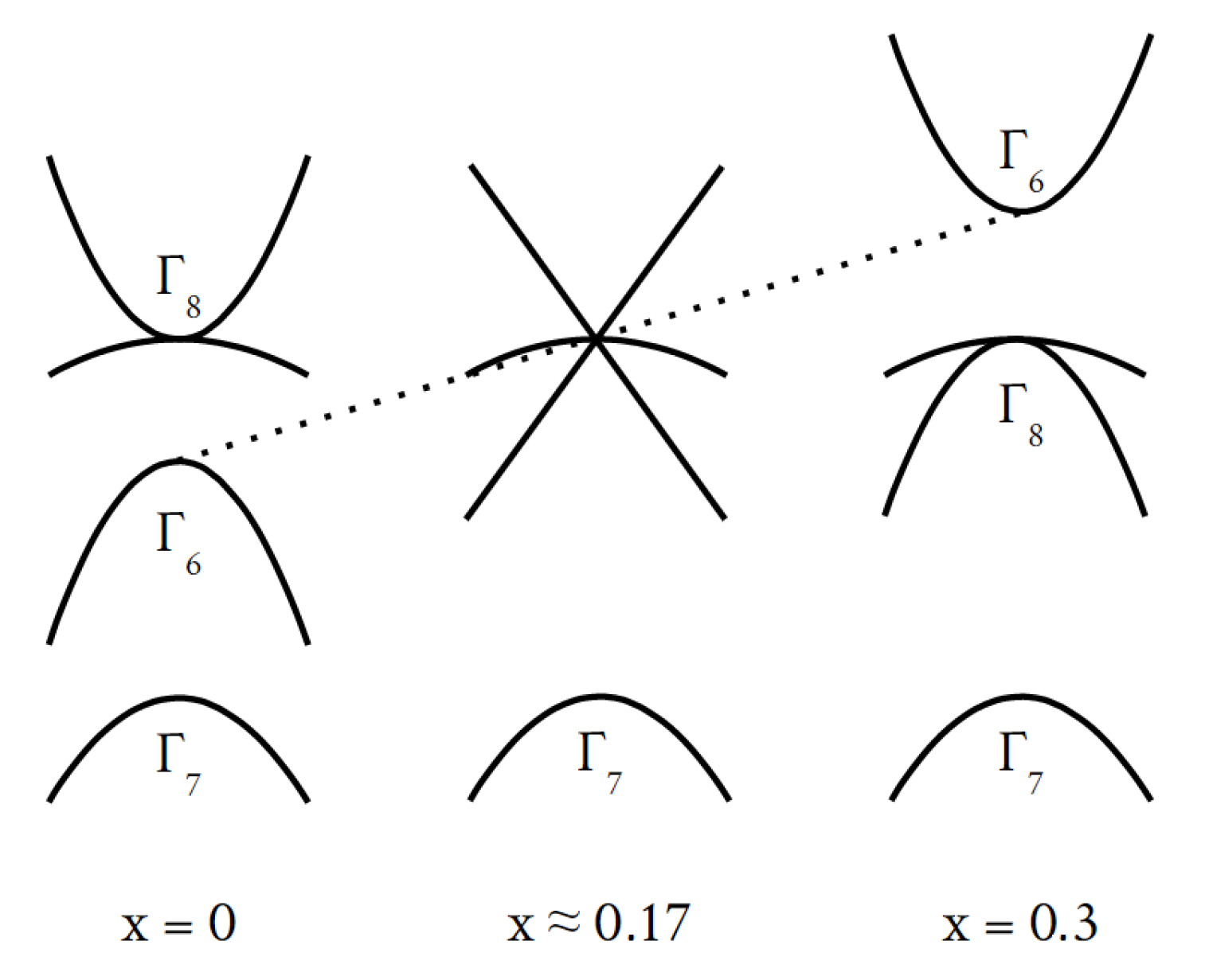
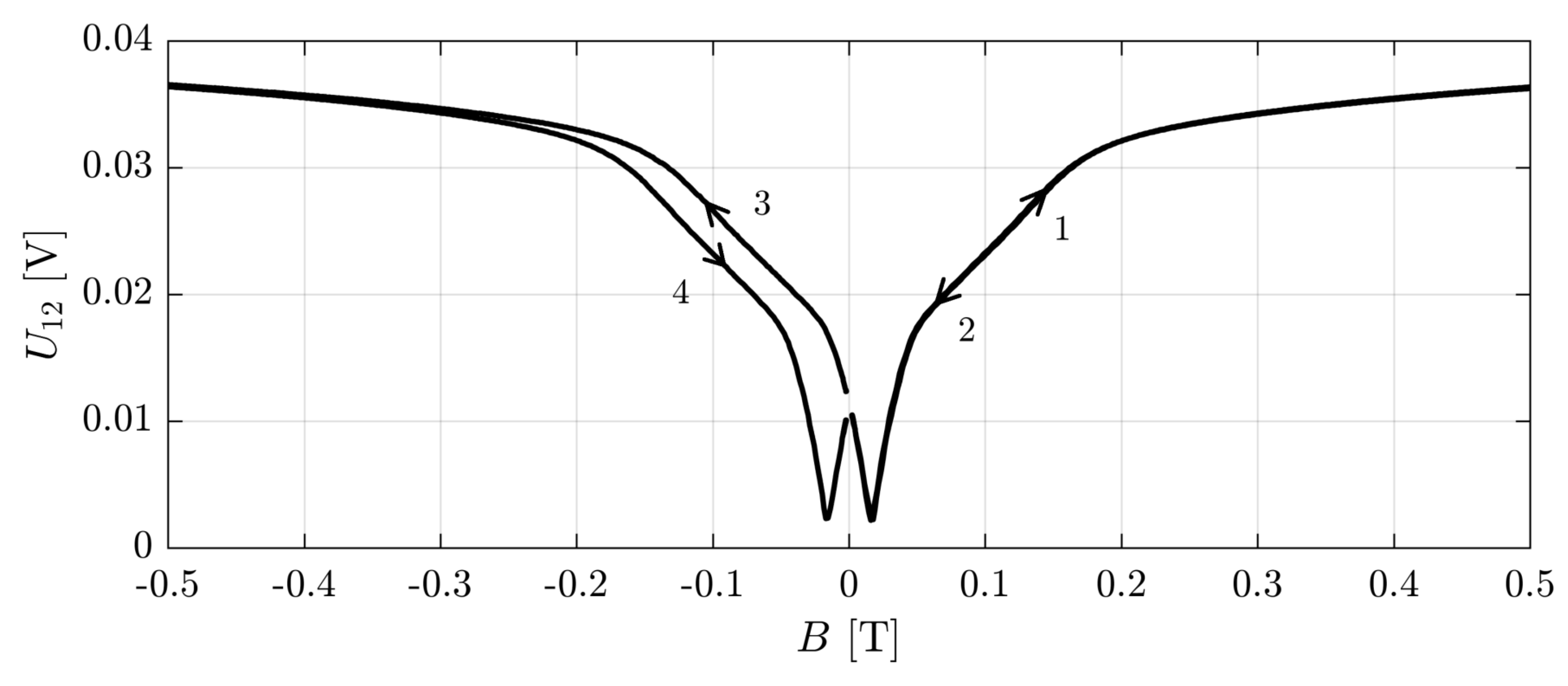
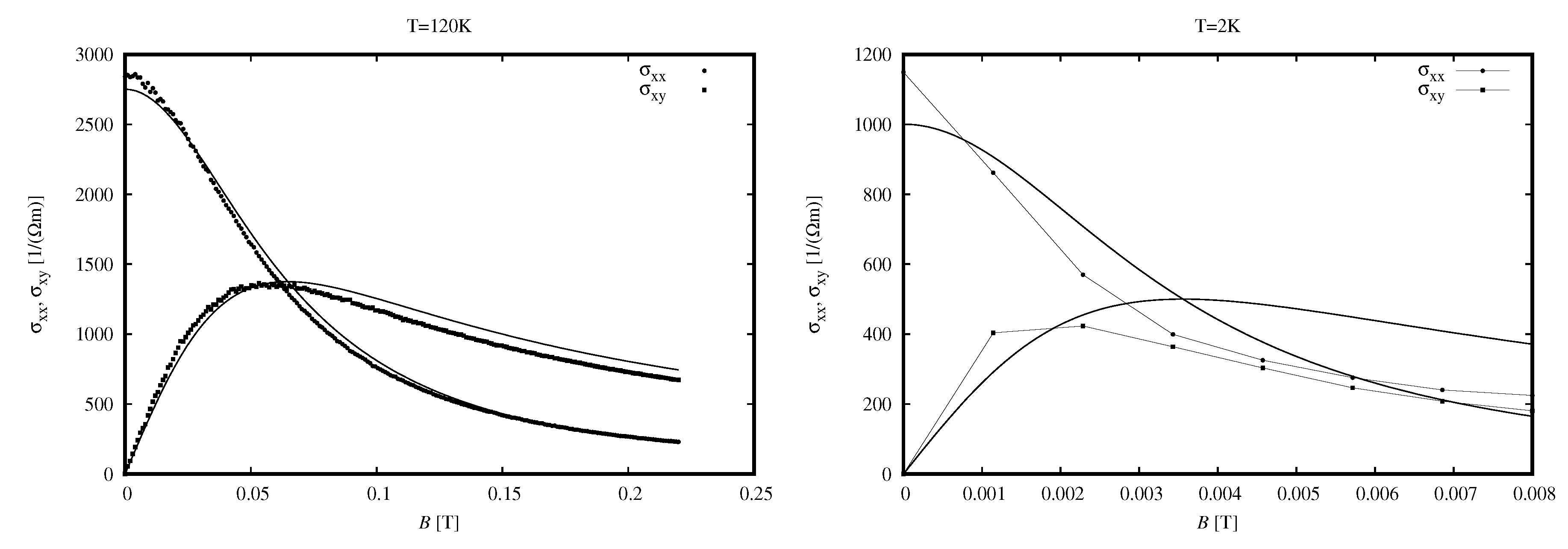
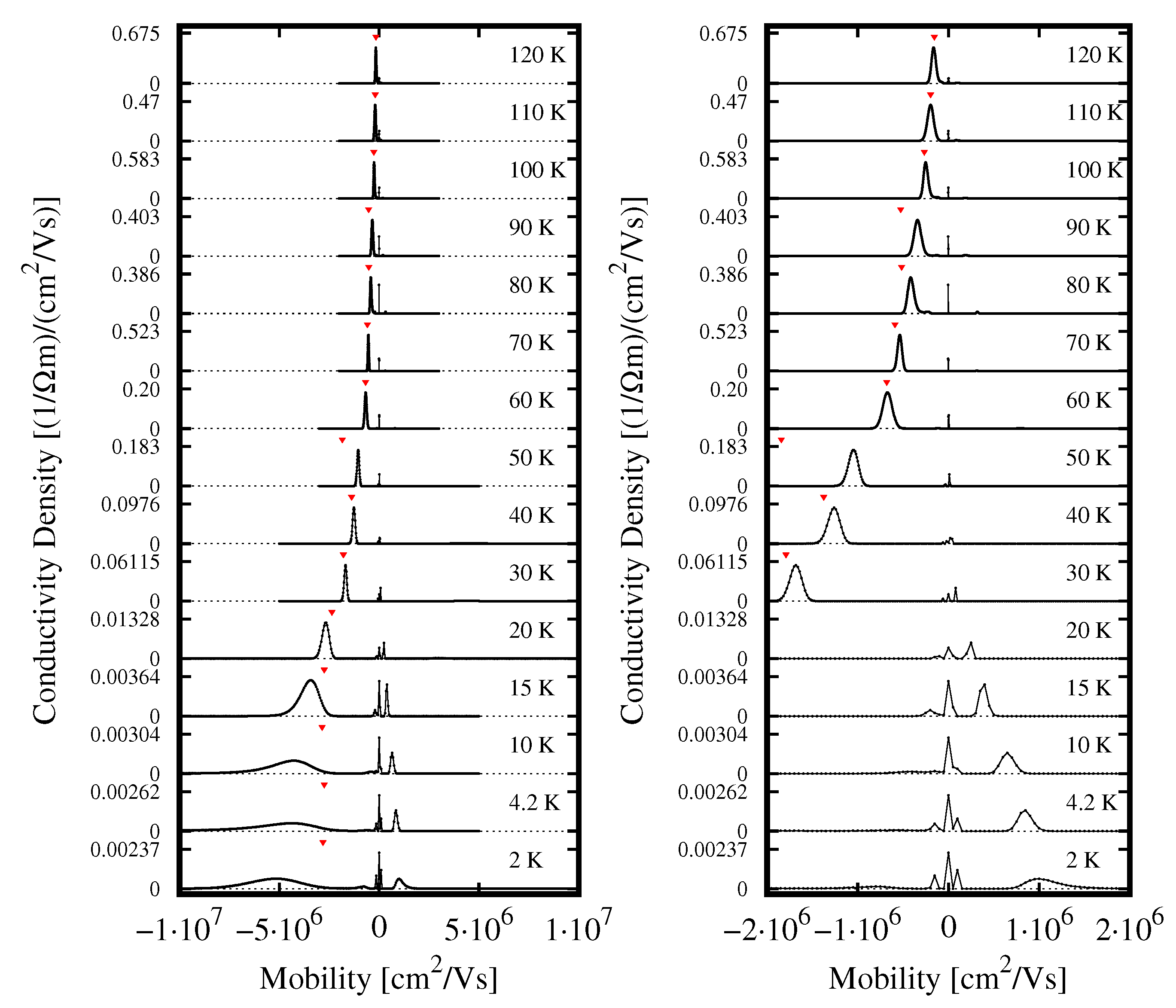
3.1. Tensor of Magnetoconductivity and Mobility of Carriers in the Epitaxial Layer
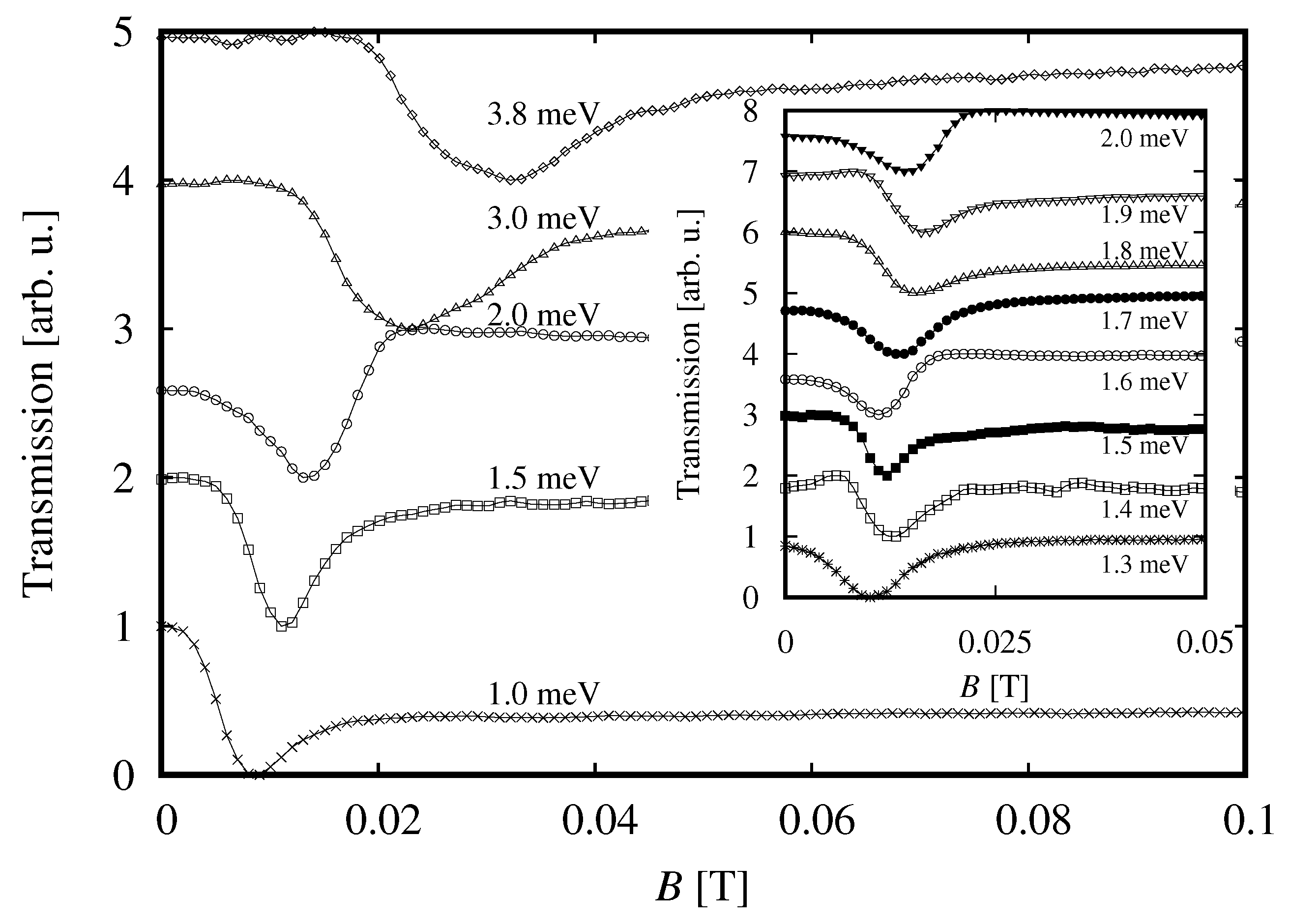
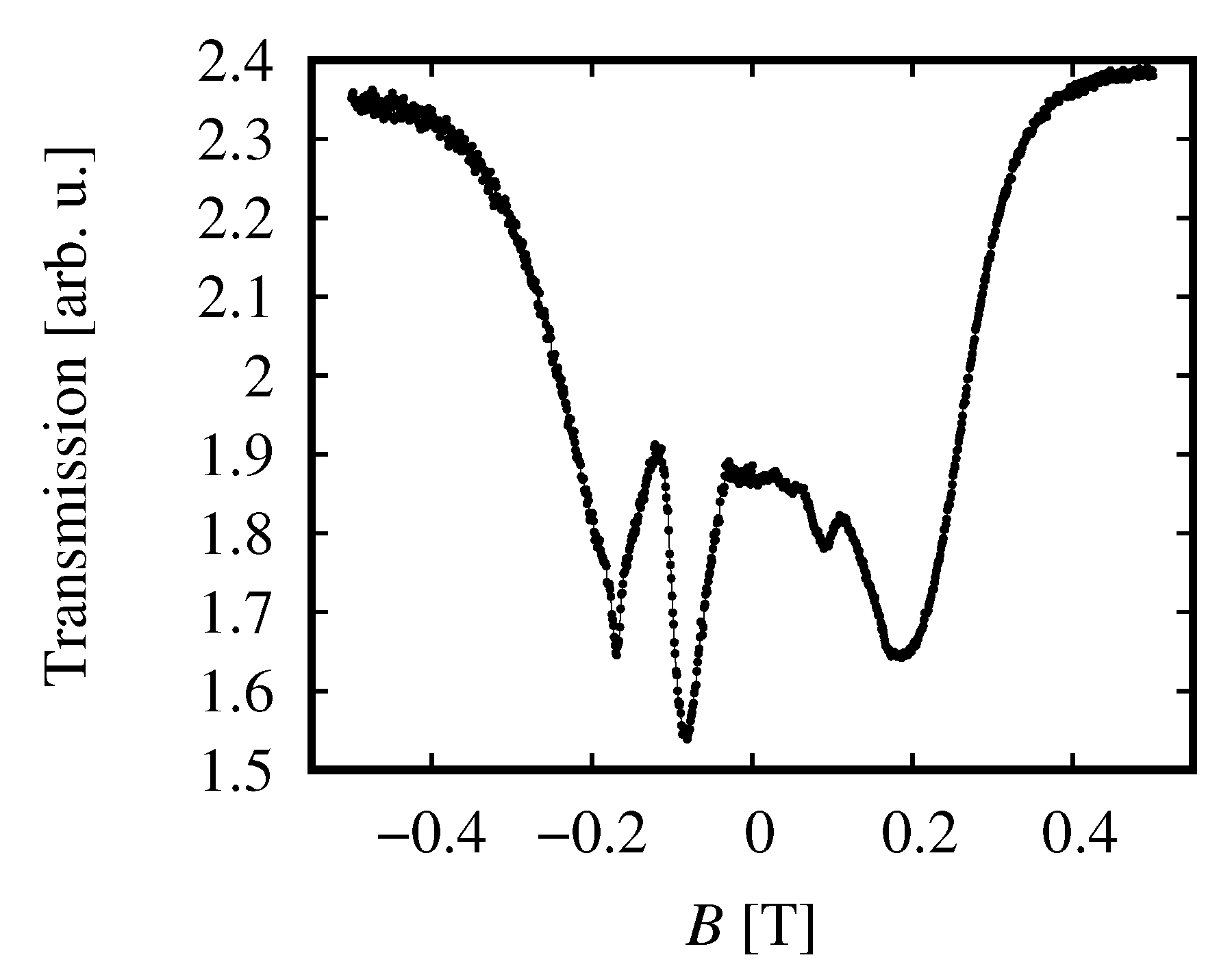
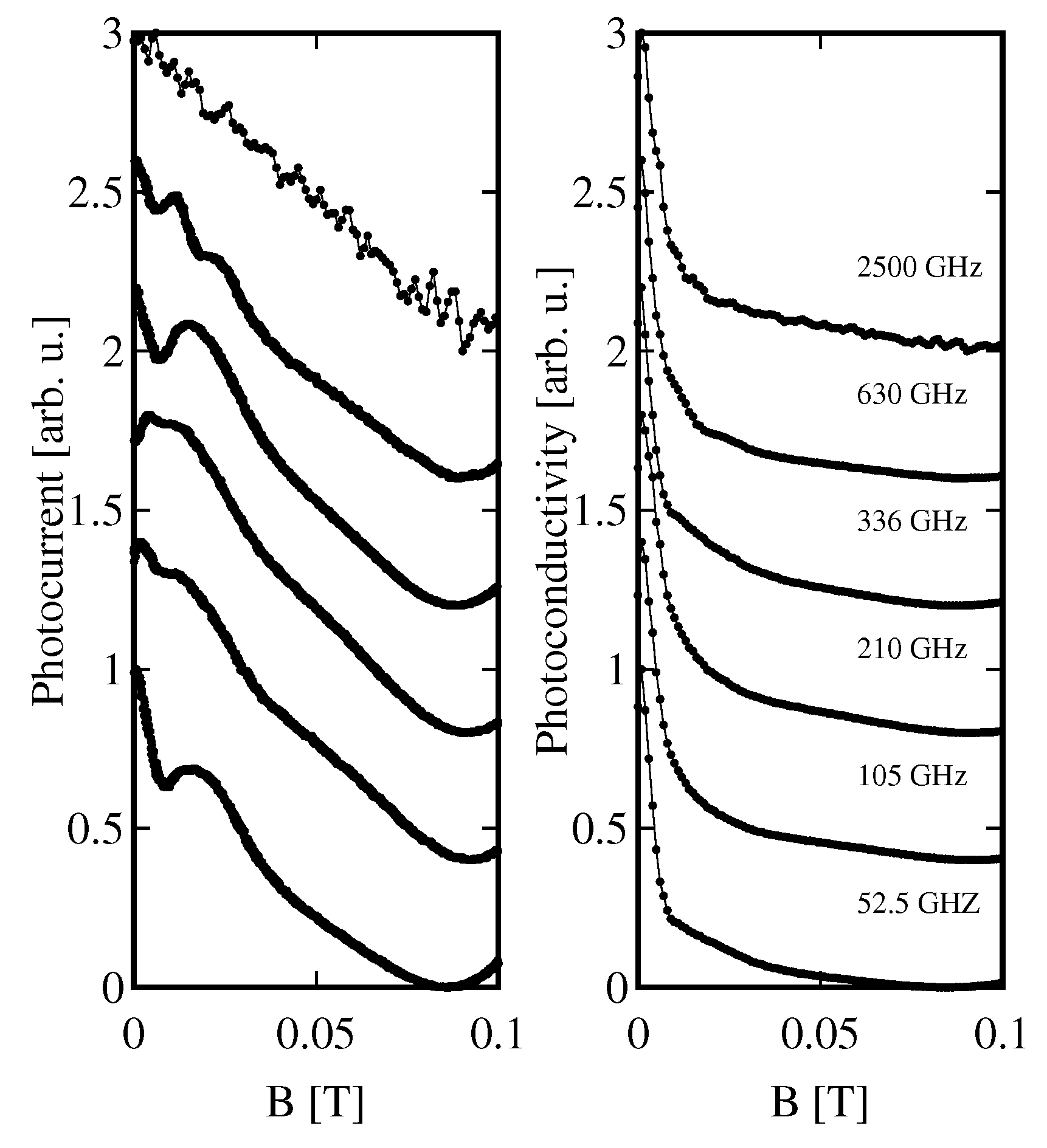
3.2. THz Spectroscopy of the Epitaxial Layer
4. Discussion
4.1. Magnetoconductivity
4.2. THz Spectroscopy
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A

References
- Brucherseifer, M.; Nagel, M.; Haring Bolivar, P.; Kurz, H.; Bosserhoff, A.; Büttner, R. Label-free probing of the binding state of DNA by time-domain terahertz sensing. Appl. Phys. Lett. 2000, 77, 4094. [Google Scholar] [CrossRef]
- Zangeneh-Nejad, F.; Safian, R. A graphene-based THz ring resonator for label-free sensing. IEEE Sens. J. 2015, 16, 4338–4344. [Google Scholar] [CrossRef]
- Zangeneh-Nejad, F.; Safian, R. Hybrid graphene–molybdenum disulphide based ring resonator for label-free sensing. Opt. Commun. 2016, 371, 9–14. [Google Scholar] [CrossRef]
- Padilla, W.J.; Basov, D.N.; Smith, D.R. Negative refractive index metamaterials. Mater. Today 2006, 9, 28–35. [Google Scholar] [CrossRef]
- Debus, C.; Haring Bolivar, P. Frequency selective surfaces for high sensitivity terahertz sensing. Appl. Phys. Lett. 2007, 91, 184102. [Google Scholar] [CrossRef]
- Singh, R.; Cao, W.; Al-Naib, I.; Cong, L.; Withayachumnankul, W.; Zhang, W. Ultrasensitive terahertz sensing with high-Q Fano resonances in metasurfaces. Appl. Phys. Lett. 2014, 105, 171101. [Google Scholar] [CrossRef]
- Kampfrath, T.; Tanaka, K.; Nelson, K.A. Resonant and nonresonant control over matter and light by intense terahertz transients. Nat. Photonics 2013, 7, 680–690. [Google Scholar] [CrossRef]
- Berry, C.W.; Wang, N.; Hashemi, M.R.; Unlu, M.; Jarrahi, M. Significant performance enhancement in photoconductive terahertz optoelectronics by incorporating plasmonic contact electrodes. Nat. Commun. 2013, 4, 1622. [Google Scholar] [CrossRef]
- Yang, S.-H.; Hashemi, M.R.; Berry, C.W.; Jarrahi, M. 7.5% Optical-to-Terahertz Conversion Efficiency Offered by Photoconductive Emitters With Three-Dimensional Plasmonic Contact Electrodes. IEEE Trans. Terahertz Sci. Technol. 2014, 4, 575–581. [Google Scholar] [CrossRef]
- Łusakowski, J. Plasmon—Terahertz photon interaction in high-electron-mobility heterostructures. Semicond. Sci. Technol. 2017, 32, 013004. [Google Scholar] [CrossRef]
- Zangeneh-Nejad, F.; Safian, R. Significant enhancement in the efficiency of photoconductive antennas using a hybrid graphene molybdenum disulphide structure. J. Nanophotonics 2016, 10, 036005. [Google Scholar] [CrossRef]
- Billot, R.; Agnese, P.; Augueres, J.-L.; Beguin, A.; Bouere, A.; Boulade, O.; Cara, C.; Cloue, C.; Doumayrou, E.; Duband, L.; et al. The Herschel/PACS 2560 bolometers imaging camera. In Proceedings of the Space Telescopes and Instrumentation I, Orlando, FL, USA, 24–31 May 2006. [Google Scholar] [CrossRef]
- Putley, E.H. Indium antimonide submillimeter photoconductive detectors. Appl. Opt. 1965, 4, 649–657. [Google Scholar] [CrossRef]
- Vasilyev, Y.B.; Usikova, A.A.; Il’inskaya, N.D.; Petrov, P.V.; Ivanov, Y.L. Highly sensitive submillimeter InSb photodetectors. Semiconductors 2008, 42, 1258–1260. [Google Scholar] [CrossRef]
- Chochol, J.; Postava, K.; Čada, M.; Vanwolleghem, M.; Halagačka, L.; Lampin, J.-F.; Pištora, J. Magneto-optical properties of InSb for THz applications. AIP Adv. 2016, 6, 115021. [Google Scholar] [CrossRef]
- Graf, M.; Scalari, G.; Hofstetter, D.; Faist, J.; Beere, H.; Linfield, E.; Ritchie, D.; Davies, G. Terahertz range quantum well infrared photodetector. Appl. Phys. Lett. 2004, 84, 475–477. [Google Scholar] [CrossRef]
- Luo, H.; Liu, C.H.; Song, C.Y.; Wasilewski, Z.R. Background-limited terahertz quantum—Well photodetector. Appl. Phys. Lett. 2005, 86, 231103. [Google Scholar] [CrossRef]
- Palaferri, D.; Todorov, Y.; Chen, Y.N.; Madeo, J.; Vasanelli, A.; Li, L.H.; Davies, A.G.; Linfield, E.H.; Sirtori, C. Patch antenna terahertz photodetectors. Appl. Phys. Lett. 2015, 106, 161102. [Google Scholar] [CrossRef]
- Tretyakov, I.; Ryabchun, S.; Finkel, M.; Maslennikova, A.; Kaurova, N.; Lobastova, A.; Voronov, B.; Gol’tsman, G. Low noise and wide bandwidth of NbN hot-electron bolometer mixers. Appl. Phys. Lett. 2011, 98, 033507. [Google Scholar] [CrossRef]
- Tretyakov, I.; Ryabchun, S.; Finkel, M.; Maslennikov, S.; Maslennikova, A.; Kaurova, N.; Lobastova, A.; Voronov, B.; Gol’tsman, G. Ultrawide Noise Bandwidth of NbN Hot-Electron Bolometer Mixers With In Situ Gold Contacts. IEEE Trans. Appl. Supercond. 2011, 21, 620–623. [Google Scholar] [CrossRef]
- Ryabchun, S.; Tong, C.Y.E.; Paine, S.; Lobanov, Y.; Blundell, R.; Gol’sman, G. Temperature Resolution of an HEB Receiver at 810 GHz. IEEE Trans. Appl. Supercond. 2009, 19, 293–296. [Google Scholar] [CrossRef]
- Hubers, W.-W.; Pavlov, S.G.; Holldack, K.; Schade, U.; Wustefeld, G. Long wavelength response of unstressed and stressed Ge:Ga detectors. Proc. SPIE 2006, 6275, 627505. [Google Scholar] [CrossRef]
- Poglitsch, A.; Katterloher, R.O.; Hoenle, R.; Beeman, J.W.; Haller, E.E.; Richter, H.; Groezinger, U.; Haegel, N.M.; Krabbe, A. Far-infrared photoconductor arrays for Herschel and SOFIA. Proc. SPIE 2003, 4855, 115–128. [Google Scholar] [CrossRef]
- Haller, E.E.; Hueschen, M.R.; Richards, P.L. Ge:Ga hotoconductors in low infrared backgrounds. Appl. Phys. Lett. 1979, 34, 495–497. [Google Scholar] [CrossRef]
- Lawson, W.D.; Nielsen, S.; Putley, E.H.; Young, A.S. Preparation and properties of HgTe and mixed crystals of HgTe-CdTe. J. Phys. Chem. Sol. 1959, 9, 325–329. [Google Scholar] [CrossRef]
- Dornhaus, R.; Nimtz, G. The properties and applications of the Hg1-xCdxTe system. In Narrow-Gap Semiconductors; Solid-State Physics, Springer Tracts in Modern Physics; Springer: Berlin/Heidelberg, Germany, 1976; Volume 78, p. 1. ISBN 978-3-540-07774-9. [Google Scholar]
- Rogalski, A. HgCdTe infrared detector material: History, status and outlook. Rep. Prog. Phys. 2005, 68, 2267–2336. [Google Scholar] [CrossRef]
- Downs, C.; Vandervelde, T.E. Progress in infrared detectors since 2000. Sensors 2013, 13, 5054–5098. [Google Scholar] [CrossRef]
- Kane, E.O. Band structure of Indium Antimonide. J. Phys. Chem. Solids 1957, 1, 249–261. [Google Scholar] [CrossRef]
- Weiler, M.H.; Aggarwal, R.L.; Lax, B. Interband magnetoreflectance in semiconducting Hg1-xCdxTe alloys. Phys. Rev. B 1977, 16, 3603–3607. [Google Scholar] [CrossRef]
- Rogalski, A. Infrared detectors: Status and trends. Prog. Quantum Electron. 2003, 27, 59–210. [Google Scholar] [CrossRef]
- Zawadzki, W. Semirelativity in semiconductors: A review. J. Phys. Condens. Matter 2017, 29, 373004. [Google Scholar] [CrossRef]
- Teppe, F.; Marcinkiewicz, M.; Krishtopenko, S.S.; Ruffenach, S.; Consejo, C.; Kadykov, A.M.; Desrat, W.; But, D.; Knap, W.; Ludwig, J.; et al. Temperature-driven massless Kane fermions in HgCdTe crystals. Nat. Commun. 2016, 7, 12576. [Google Scholar] [CrossRef] [PubMed]
- Orlita, M.; Basko, D.M.; Zholudev, M.S.; Teppe, F.; Knap, W.; Gavrilenko, V.I.; Mikhailov, N.N.; Dvoretskii, S.A.; Neugebauer, P.; Faugeras, C.; et al. Observation of three-dimensional massless Kane fermions in a zinc-blende crystal. Nat. Phys. 2014, 10, 233–238. [Google Scholar] [CrossRef]
- Moore, J.E. The birth of topological insulators. Nature 2010, 46, 194–198. [Google Scholar] [CrossRef] [PubMed]
- Tomaka, G.; Grendysa, J.; Marchewka, M.; Śliż, P.; Becker, C.R.; Stadler, A.; Sheregii, E.M. Topological insulators based on the semi-metallic HgCdTe. Opto-Electron. Rev. 2017, 25, 188–197. [Google Scholar] [CrossRef]
- Knap, W.; Dur, D.; Raymond, A.; Meny, C.; Leotin, J.; Huant, S.; Etienne, B. A far-infrared spectrometer based on cyclotron resonance emission sources. Rev. Sci. Instrum. 1992, 63, 3293–3297. [Google Scholar] [CrossRef]
- Białek, M.; Czapkiewicz, M.; Wróbel, J.; Umansky, V.; Łusakowski, J. Plasmon dispersions in high electron mobility terahertz detectors. Appl. Phys. Lett. 2007, 104, 263514. [Google Scholar] [CrossRef]
- Białek, M.; Grigelionis, I.; Nogajewski, K.; Łusakowski, J. Plasmon—Photon coupling in high-electron-mobility heterostructures: Tutorial on magnetoplasmon spectroscopy. In Spin Orbitronics and Topological Properties of Nanostructures; Dugaev, V., Tralle, I., Wal, A., Barnaś, J., Eds.; World Scientific: Singapore, 2016; ISBN 978-0-00-098737-2. [Google Scholar]
- Knap, W.; Łusakowski, J.; Karpierz, K.; Orsal, B.; Robert, J.-L. Improved performance of magnetically tunable GaAs and InP far-infrared detectors. J. Appl. Phys. 1992, 72, 680–683. [Google Scholar] [CrossRef]
- Szot, M.; Karpierz, K.; Kossut, J.; Grynberg, M. Potential fluctuations in 2D MBE CdTe/CdMgTe quantum well, experimental proof of the nature of fluctuations. AIP Conf. Proc. 2007, 893, 251–252. [Google Scholar] [CrossRef]
- Yavorskiy, D.; Karpierz, K.; Grynberg, M.; Knap, W.; Łusakowski, J. Indium antimonide detector for spectral characterization of terahertz sources. J. Appl. Phys. 2018, 123, 064502. [Google Scholar] [CrossRef]
- Bąk, M.M.; Yavorskiy, D.; Karpierz, K.; Łusakowski, J.; But, D.; Przybytek, J.; Yahniuk, I.; Cywiński, G.; Knap, W.; Teppe, F.; et al. Magnetoconductivity of a Mercury Cadmium Telluride Resonant THz Detector. Acta Phys. Polonica 2018, 134, 973–977. [Google Scholar] [CrossRef]
- Dziuba, Z. Analysis of Electrical Conduction in Epitaxial Layer Structures Using the Mobility Spectrum Technique. Phys. Stat. Sol. A 1996, 153, 445–457. [Google Scholar] [CrossRef]
- Kiatgamolchai, S.; Myronov, M.; Mironov, O.A.; Kantser, V.G.; Parker, E.H.; Whall, T.E. Mobility spectrum computational analysis using a maximum entropy approach. Phys. Rev. E 2002, 036705–1–9. [Google Scholar] [CrossRef] [PubMed]
- Tunable THz Polarization Converter. Available online: http://www.tydexoptics.com/products/thz_optics/tunable_thz_polarization_converter/ (accessed on 7 December 2018).
- Nagai, M.; Mukai, N.; Minowa, Y.; Ashida, M.; Takayanagi, J.; Ohtake, H. Achromatic THz wave plate composed of stacked parallel metal plates. Opt. Lett. 2014, 39, 146–149. [Google Scholar] [CrossRef] [PubMed]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yavorskiy, D.; Karpierz, K.; Baj, M.; Bąk, M.M.; Mikhailov, N.N.; Dvoretsky, S.A.; Gavrilenko, V.I.; Knap, W.; Teppe, F.; Łusakowski, J. Magnetoconductivity and Terahertz Response of a HgCdTe Epitaxial Layer. Sensors 2018, 18, 4341. https://doi.org/10.3390/s18124341
Yavorskiy D, Karpierz K, Baj M, Bąk MM, Mikhailov NN, Dvoretsky SA, Gavrilenko VI, Knap W, Teppe F, Łusakowski J. Magnetoconductivity and Terahertz Response of a HgCdTe Epitaxial Layer. Sensors. 2018; 18(12):4341. https://doi.org/10.3390/s18124341
Chicago/Turabian StyleYavorskiy, Dmitriy, Krzysztof Karpierz, Michał Baj, Małgorzata M. Bąk, Nikolai N. Mikhailov, Sergey A. Dvoretsky, Vladimir I. Gavrilenko, Wojciech Knap, Frederic Teppe, and Jerzy Łusakowski. 2018. "Magnetoconductivity and Terahertz Response of a HgCdTe Epitaxial Layer" Sensors 18, no. 12: 4341. https://doi.org/10.3390/s18124341
APA StyleYavorskiy, D., Karpierz, K., Baj, M., Bąk, M. M., Mikhailov, N. N., Dvoretsky, S. A., Gavrilenko, V. I., Knap, W., Teppe, F., & Łusakowski, J. (2018). Magnetoconductivity and Terahertz Response of a HgCdTe Epitaxial Layer. Sensors, 18(12), 4341. https://doi.org/10.3390/s18124341




