IRNSS/NavIC L5 Attitude Determination
Abstract
:1. Introduction
2. GNSS Observational Model
2.1. Unconstrained Model
2.2. Multivariate-Constrained Model
2.3. Attitude Determination
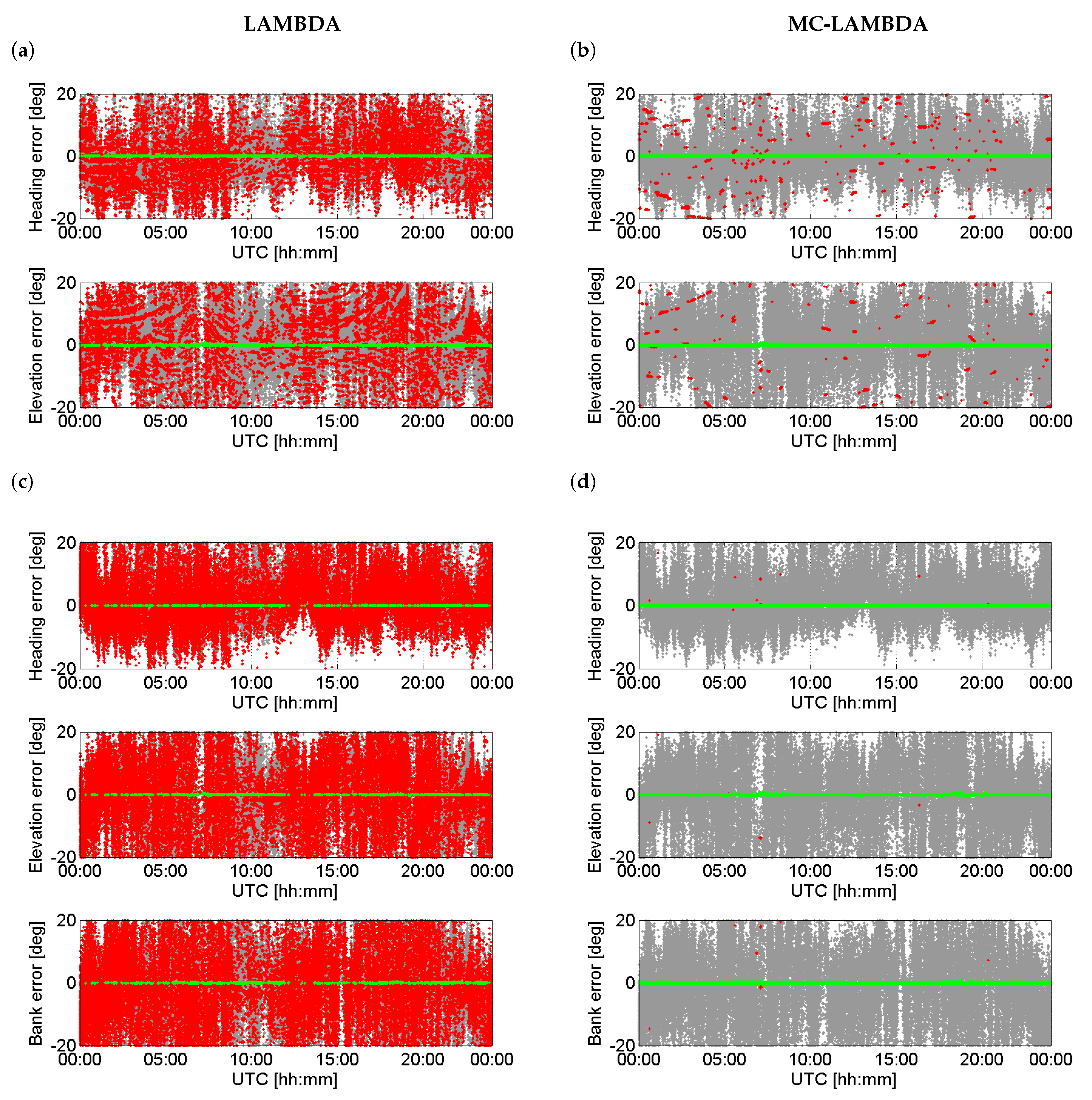
3. Numerical Analysis
3.1. Measurement Set-Up
3.2. Baseline Solution: From Unconstrained to Constrained
3.3. Attitude Determination Performance
3.4. Ambiguity Resolution Performance
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Indian Space Research Organisation (ISRO). Indian Regional Navigation Satellite System: Signal in Space ICD for Standard Positioning Service; Version 1.0; ISRO Satellite Centre: Bengaluru, India, 2014.
- Indian Space Research Organisation (ISRO). PSLV-C33/IRNSS-1G. Available online: http://www.isro.gov.in/sites/default/files/pslv-c33-brochure.pdf (accessed on 1 June 2016).
- Thoelert, S.; Montenbruck, O.; Meurer, M. IRNSS-1A: Signal and clock characterization of the Indian regional navigation system. GPS Solut. 2014, 18, 147–152. [Google Scholar] [CrossRef]
- Kumari, A.; Samal, K.; Rajarajan, D.; Swami, U.; Babu, R.; Kartik, A.; Rathnakara, S.C.; Ganeshan, A.S. Precise Modeling of Solar Radiation Pressure for IRNSS Satellite. J. Nat. Sci. Res. 2015, 5, 35–43. [Google Scholar]
- Babu, R.; Mula, P.; Ratnakara, S.C.; Ganeshan, A.S. IRNSS Satellite Parameter Estimation Using Combination Strategy. Glob. J. Sci. Front. Res. 2015, 15, 3. [Google Scholar]
- Chandrasekhar, M.V.; Rajarajan, D.; Satyanarayana, G.; Tirmal, N.; Rathnakara, S.C.; Ganeshan, A.S. Modernized IRNSS Broadcast Ephemeris Parameters. Control Theory Inform. 2015, 5, 1–9. [Google Scholar]
- Ganeshan, A.S.; Ratnakara, S.C.; Srinivasan, N.; Rajaram, B.; Tirmal, N.; Anbalagan, K. First position fix with IRNSS. Inside GNSS. July 2015, pp. 48–52. Available online: http://www.insidegnss.com/node/4545 (accessed on 19 April 2016).
- Zaminpardaz, S.; Teunissen, P.J.G.; Nadarajah, N. IRNSS stand-alone positioning: First results in Australia. J. Spat. Sci. 2016, 61, 5–27. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, S.R. IRNSS orbit determination and broadcast ephemeris assessment. In Proceedings of the 2015 International Technical Meeting of The Institute of Navigation, Dana Point, CA, USA, 26–28 January 2015; pp. 185–193.
- Nadarajah, N.; Khodabandeh, A.; Teunissen, P.J.G. Assessing the IRNSS L5-signal in combination with GPS, Galileo, and QZSS L5/E5a-signals for positioning and navigation. GPS Solut. 2016, 20, 289–297. [Google Scholar] [CrossRef]
- Cohen, C. Attitude Determination Using GPS. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 1992. [Google Scholar]
- Lu, G. Development of a GPS Multi-Antenna System for Attitude Determination. Ph.D. Thesis, University of Calgary, Calgary, AB, Canada, 1995. [Google Scholar]
- Madsen, J.; Lightsey, E.G. Robust spacecraft attitude determination using global positioning system receivers. J. Spacecr. Rockets 2004, 41, 635–644. [Google Scholar] [CrossRef]
- Psiaki, M.L. Batch algorithm for global-positioning-system attitude determination and integer ambiguity resolution. J. Guid. Control Dyn. 2006, 29, 1070–1079. [Google Scholar] [CrossRef]
- Hodgart, M.S.; Purivigraipong, S. New approach to resolving instantaneous integer ambiguity resolution for spacecraft attitude determination using GPS signals. In Proceedings of the IEEE Position Location and Navigation Symposium, San Diego, CA, USA, 13–16 March 2000; pp. 132–139.
- Li, Y.; Zhang, K.; Roberts, C.; Murata, M. On-the-Fly GPS-Based Attitude Determination Using Single- and Double-Differenced Carrier Phase Measurements. GPS Solut. 2004, 8, 93–102. [Google Scholar] [CrossRef]
- Wang, B.; Miao, L.; Wang, S.; Shen, J. A constrained LAMBDA method for GPS attitude determination. GPS Solut. 2009, 13, 97–107. [Google Scholar] [CrossRef]
- Giorgi, G.; Teunissen, P.J.G.; Verhagen, S.; Buist, P.J. Testing a new multivariate GNSS carrier phase attitude determination method for remote sensing platforms. Adv. Space Res. 2010, 46, 118–129. [Google Scholar] [CrossRef]
- Teunissen, P.J.; Giorgi, G.; Buist, P.J. Testing of a new single-frequency GNSS carrier phase attitude determination method: Land, ship and aircraft experiments. GPS Solut. 2011, 15, 15–28. [Google Scholar] [CrossRef]
- Teunissen, P.J. The least-squares ambiguity decorrelation adjustment: A method for fast GPS integer ambiguity estimation. J. Geod. 1995, 70, 65–82. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. A canonical theory for short GPS baselines. Part I: The baseline precision. J. Geod. 1997, 71, 320–336. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. An optimality property of the integer least-squares estimator. J. Geod. 1999, 73, 587–593. [Google Scholar] [CrossRef]
- Park, C.; Teunissen, P.J.G. A new carrier phase ambiguity estimation for GNSS attitude determination systems. In Proceedings of the International GPS/GNSS Symposium, Tokyo, Japan, 15–18 November 2003; Volume 8, pp. 283–290.
- Park, C.; Teunissen, P.J.G. Integer least squares with quadratic equality constraints and its application to GNSS attitude determination systems. Int. J. Control Autom. Syst. 2009, 7, 566–576. [Google Scholar] [CrossRef]
- Teunissen, P. The LAMBDA method for the GNSS compass. Artif. Satell. 2006, 41, 89–103. [Google Scholar] [CrossRef]
- Buist, P.J. The baseline constrained LAMBDA method for single epoch, single-frequency attitude determination applications. In Proceedings of the 20th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GNSS 2007), Fort Worth, TX, USA, 25–28 September 2007; pp. 2962–2973.
- Giorgi, G.; Teunissen, P.J.G.; Buist, P.J. A search and shrink approach for the baseline constrained LAMBDA method: Experimental results. In Proceedings of the International Symposium on GPS/GNSS, Tokyo, Japan, 11–14 November 2008; Yasuda, A., Ed.; Tokyo University of Marine Science and Technology: Tokyo, Japan, 2008; pp. 797–806. [Google Scholar]
- Giorgi, G.; Buist, P.J. Single-epoch, single-frequency, standalone full attitude determination: Experimental results. In Proceedings of the Fourth ESA Workshop on Satellite Navigation User Equipment Technologies (NAVITEC), Noordwijk, The Netherlands, 10–12 December 2008.
- Harville, D.A. Matrix Algebra From A Statistician’s Perspective; Springer: New York, NY, USA, 1997. [Google Scholar]
- Magnus, J.R.; Neudecker, H. Matrix Differential Calculus with Applications in Statistics and Econometrics; John Wiley & Sons: Hoboken, NJ, USA, 1995. [Google Scholar]
- Euler, H.J.; Goad, C.C. On optimal filtering of GPS dual frequency observations without using orbit information. Bull. Geod. 1991, 65, 130–143. [Google Scholar] [CrossRef]
- Teunissen, P. A general multivariate formulation of the multi-antenna GNSS attitude determination problem. Artif. Satell. 2007, 42, 97–111. [Google Scholar] [CrossRef]
- Kuipers, J.B. Quaternions and Rotation Sequences; Princeton University Press: Princeton, NJ, USA, 2002. [Google Scholar]
- Teunissen, P.J.G. The affine constrained GNSS attitude model and its multivariate integer least-squares solution. J. Geod. 2012, 86, 547–563. [Google Scholar] [CrossRef]
- Teunissen, P.J. Integer least-squares theory for the GNSS compass. J. Geod. 2010, 84, 433–447. [Google Scholar] [CrossRef]
- Teunissen, P.J.G.; Amiri-Simkooei, A.R. Least-squares variance component estimation. J. Geod. 2008, 82, 65–82. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. Estimation in Nonlinear Models. In Proceedings of the II Hotine-Marussi Symposium on Mathematical Geodesy, Pisa, Italy, 5–8 June 1989.
- Nadarajah, N.; Teunissen, P. Instantaneous GPS/Galileo/QZSS/SBAS Attitude Determination: A Single- Frequency (L1/E1) Robustness Analysis under Constrained Environments. Navigation 2014, 61, 65–75. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. Success probability of integer GPS ambiguity rounding and bootstrapping. J. Geod. 1998, 72, 606–612. [Google Scholar] [CrossRef]
- Verhagen, S. On the reliability of integer ambiguity resolution. Navigation 2005, 52, 99–110. [Google Scholar] [CrossRef]
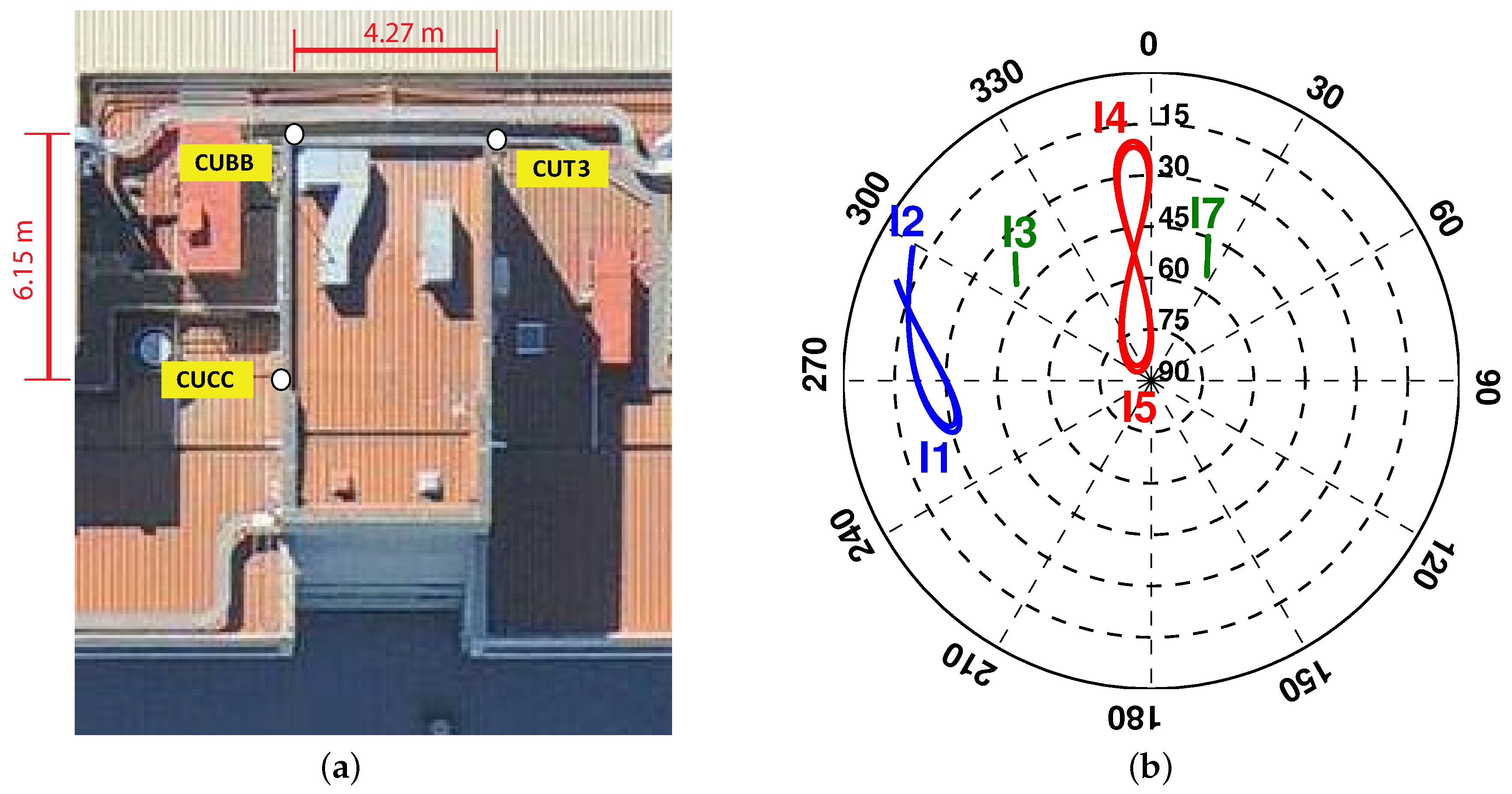
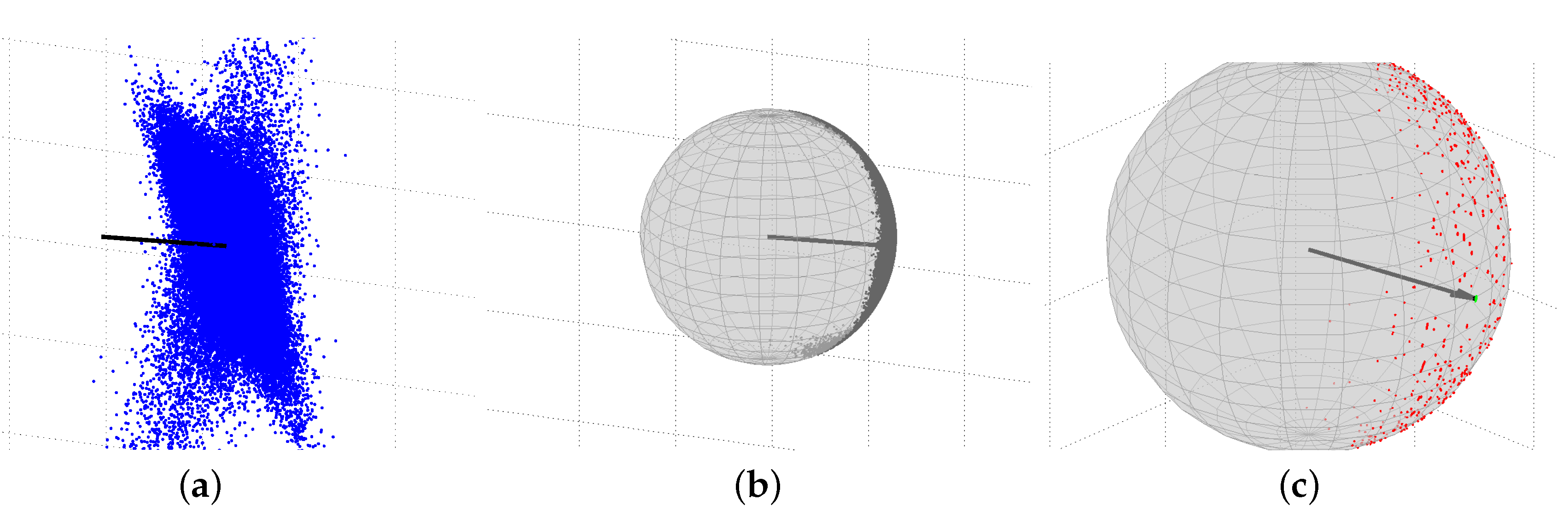

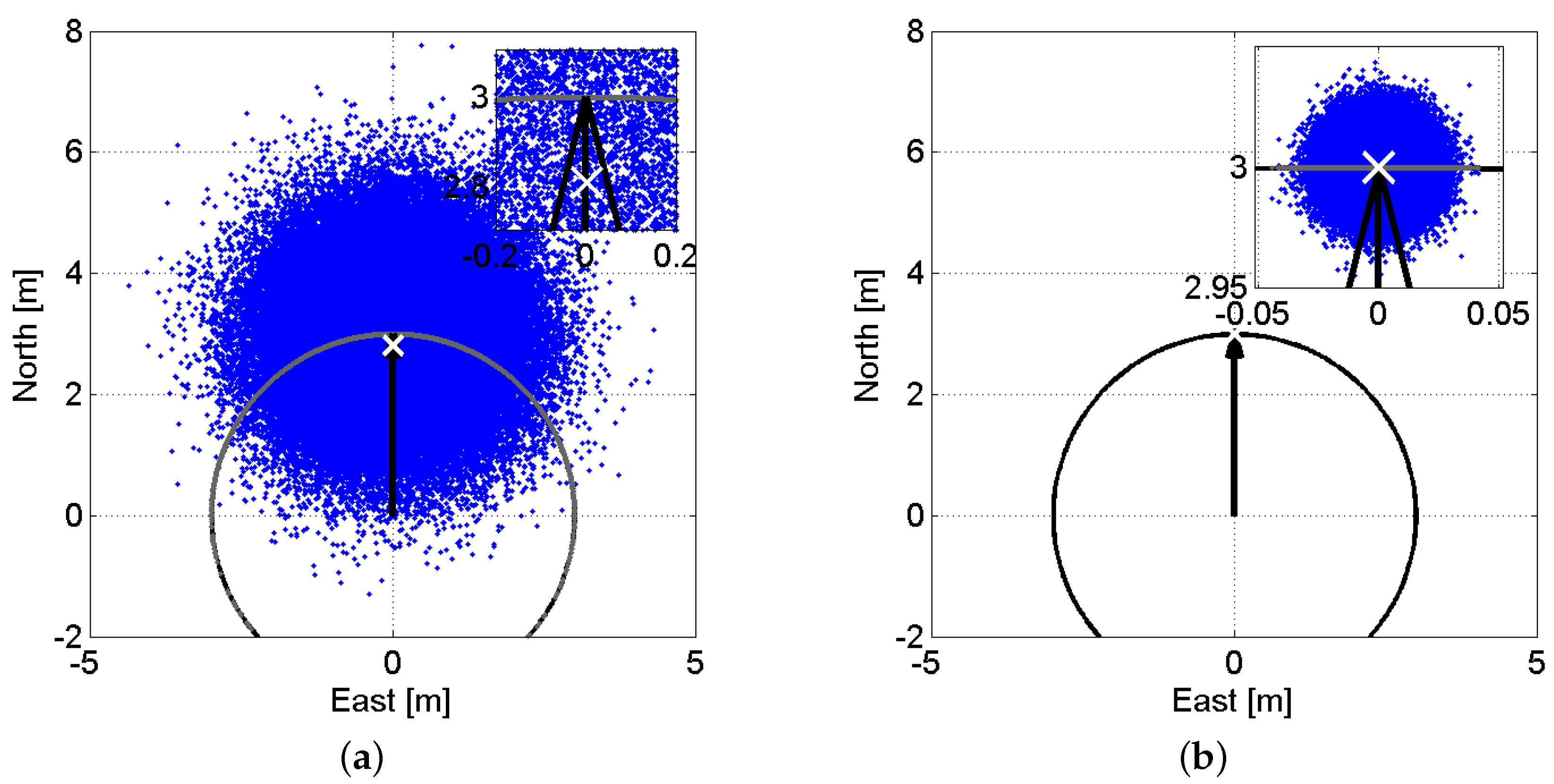
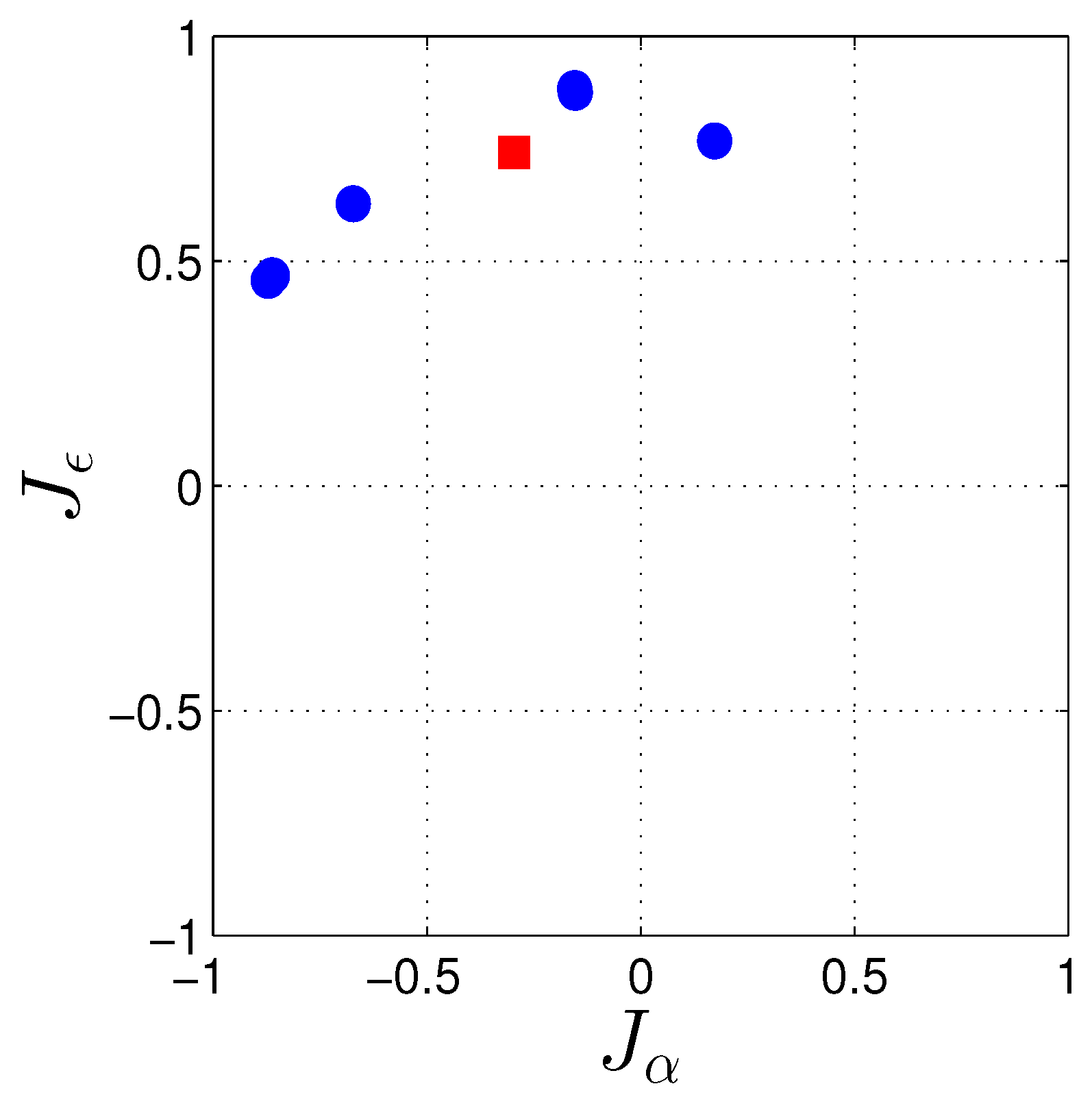
| Satellite | Type | Longitude | Inclination | Launch Date |
|---|---|---|---|---|
| IRNSS-1A (I1) | IGSO | E | July 2013 | |
| IRNSS-1B (I2) | IGSO | E | April 2014 | |
| IRNSS-1C (I3) | GEO | E | – | October 2014 |
| IRNSS-1D (I4) | IGSO | E | March 2015 | |
| IRNSS-1E (I5) | IGSO | E | January 2016 | |
| IRNSS-1F (I6) | GEO | E | – | March 2016 |
| IRNSS-1G (I7) | GEO | E | – | April 2016 |
| Scenario | Ambiguity-Float | Ambiguity-Fixed | ||||||
|---|---|---|---|---|---|---|---|---|
| Linear Array | Planar Array | Linear Array | Planar Array | |||||
| Emp | Form | Emp | Form | Emp | Form | Emp | Form | |
| heading STD [deg] | 13.36 | 7.27 | 12.66 | 6.33 | 0.04 | 0.05 | 0.04 | 0.05 |
| elevation STD [deg] | 19.73 | 17.91 | 20.41 | 16.46 | 0.09 | 0.14 | 0.09 | 0.13 |
| bank STD [deg] | – | – | 25.99 | 21.21 | – | – | 0.11 | 0.16 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zaminpardaz, S.; Teunissen, P.J.G.; Nadarajah, N. IRNSS/NavIC L5 Attitude Determination. Sensors 2017, 17, 274. https://doi.org/10.3390/s17020274
Zaminpardaz S, Teunissen PJG, Nadarajah N. IRNSS/NavIC L5 Attitude Determination. Sensors. 2017; 17(2):274. https://doi.org/10.3390/s17020274
Chicago/Turabian StyleZaminpardaz, Safoora, Peter J.G. Teunissen, and Nandakumaran Nadarajah. 2017. "IRNSS/NavIC L5 Attitude Determination" Sensors 17, no. 2: 274. https://doi.org/10.3390/s17020274
APA StyleZaminpardaz, S., Teunissen, P. J. G., & Nadarajah, N. (2017). IRNSS/NavIC L5 Attitude Determination. Sensors, 17(2), 274. https://doi.org/10.3390/s17020274






