A Possible Mechanism for Evading Temperature Quantum Decoherence in Living Matter by Feshbach Resonance
Abstract
:1. Introduction
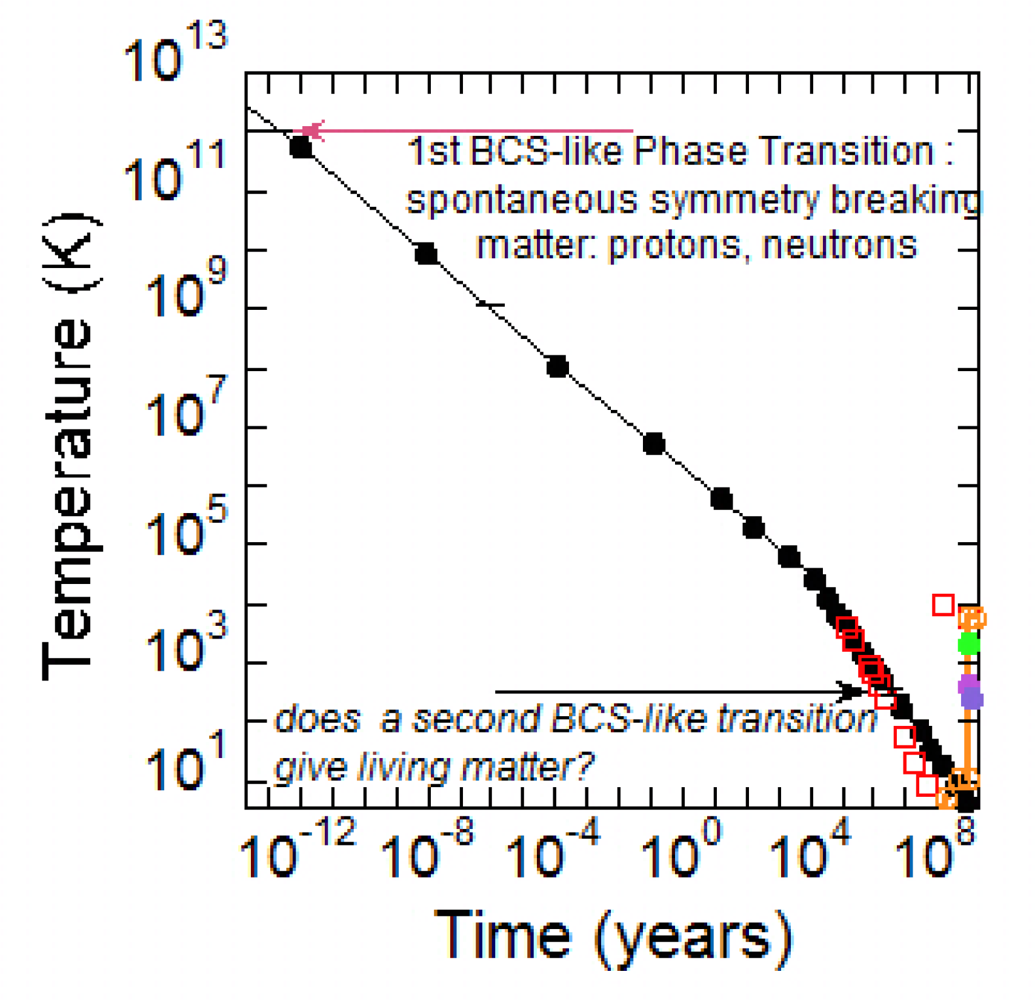
2. Mass Formation by a BCS-like Phase Transition at High Temperature in the Early Universe
3. The Emergence of Life in the Universe Timescale
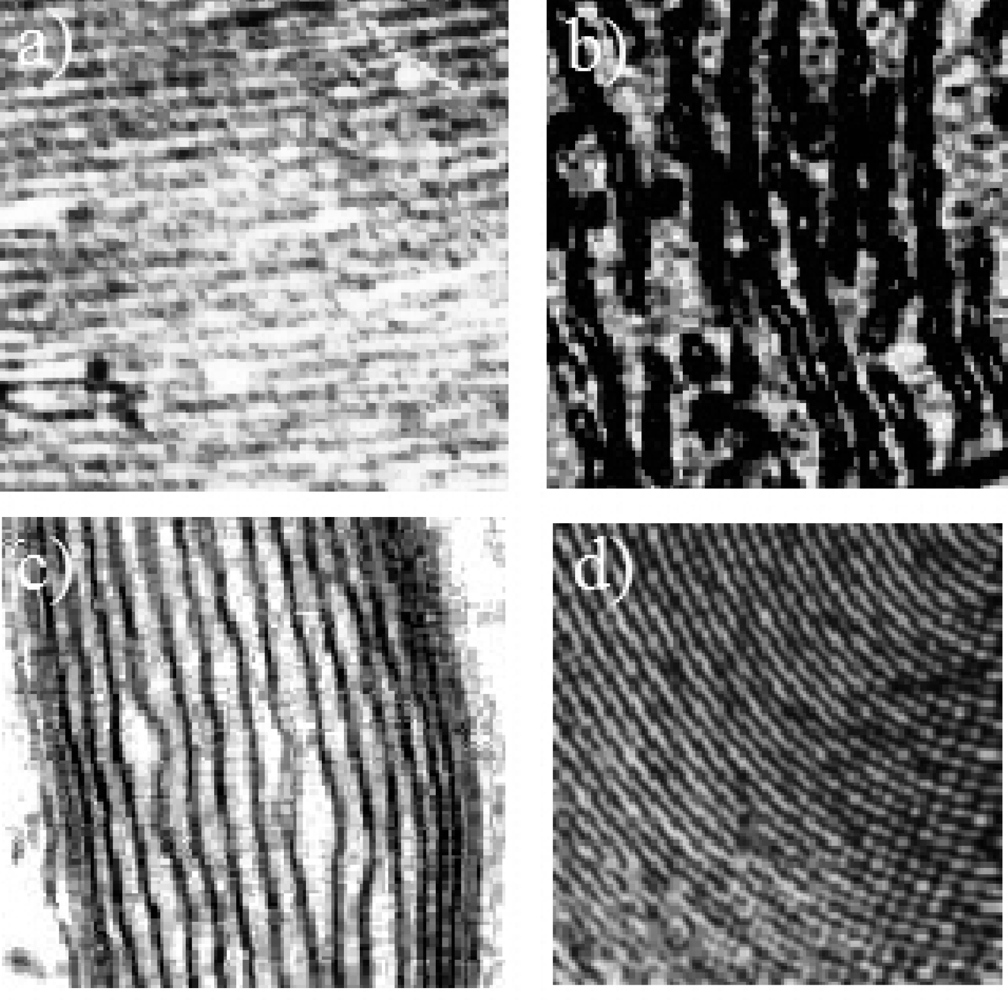
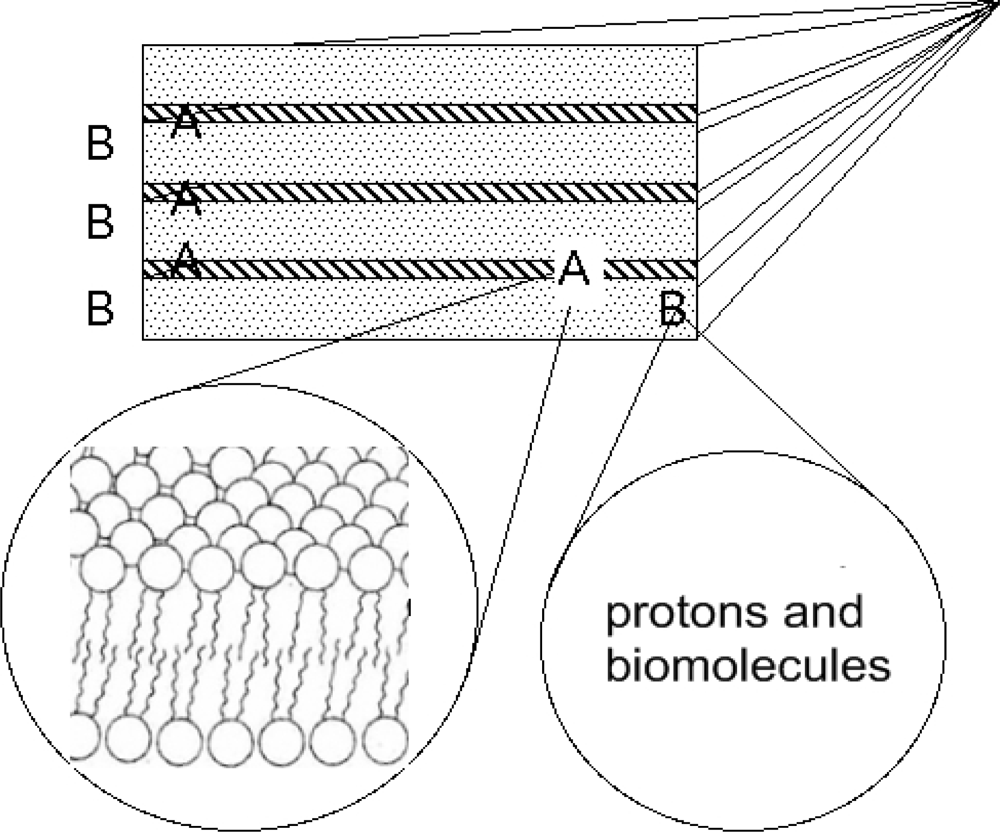
4. An Early Ubiquitous Biological Nano-architecture: The 1D Superlattice of Membranes
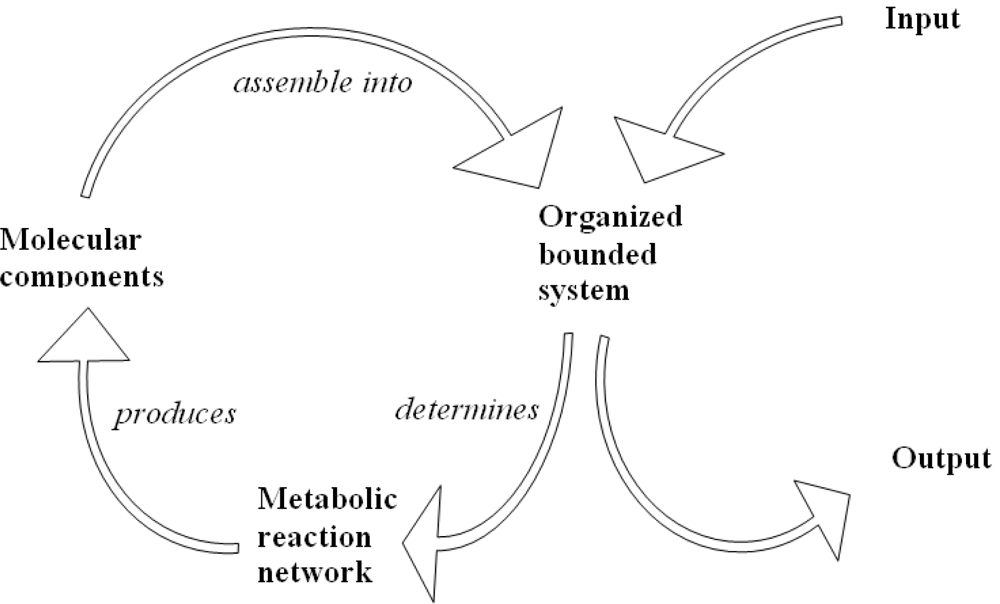
5. Biological Networks and Quantum Statistics
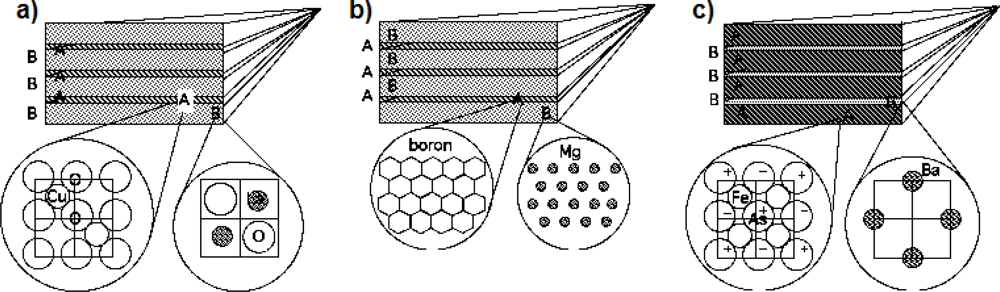
6. The Multigap BCS Superconductivity
7. Feshbach Resonances and Quantum Coherence in Living Matter
8. Conclusions
Acknowledgments
References
- Barabási, AL; Oltvai, ZN. Network biology: understanding the cell’s functional organization. Nature Rev. Genet 2004, 5, 101–113. [Google Scholar]
- Ho, MW. The rainbow and the worm The physics of organism, 3rd Ed ed; World Scientific: Singapore, 2008. [Google Scholar]
- Frölich, H. Coherent excitations in active biological systems. In Modern Bioelectrochemistry; Gutmann, F, Keyzer, H, Eds.; Plenum: New York, NY, USA, 1986; pp. 241–261. [Google Scholar]
- Del Giudice, E; Doglia, S; Milani, M; Vitiello, G. Collective properties of biological systems:solitons and coherent electric waves in a quantum field theoretical approach. In Modern Bioelectrochemistry; Gutmann, F, Keyzer, H, Eds.; Plenum: New York, NY, USA, 1986; pp. 263–288. [Google Scholar]
- Jaeken, L. Linking physiological mechanism of coherent cellular behaviour with more general physical approaches towards the coherence of life. IUBMB Life 2006, 58, 642–646. [Google Scholar]
- Ling, GN. Life at the cell and below-cell level. The hidden history of a fundamental revolution in biology; Pacific Press: New York, NY, USA, 2001. [Google Scholar]
- Pollack, G. Cells, gels and the engines of life: a new, unifying approach to cell function; Ebner and Sons: Seattle, WA, USA, 2001. [Google Scholar]
- Bianconi, G. A statistical mechanics approach for scale-free networks and finite-scale networks. Chaos 2007, 17, 026114–026116. [Google Scholar]
- Bianconi, G. The entropy of network ensembles. Phys. Rev. E 2009, 79, 036114. [Google Scholar]
- Kauffman, SA. The origins of order: self organization and selection in evolution; Oxford University Press: New York, USA, 1993. [Google Scholar]J. Theor. Bio 1969, 22, 437.Kadanoff, LP. Hip Bone is connected to II Phys. Today 2009, 62, 8–9. [Google Scholar]
- Rupley, JA; Siemankowski, L; Careri, G; Bruni, F. Two-dimensional protonic percolation on lightly hydrated purple membrane. Proc. Natl. Acad. Sci. USA 1988, 85, 9022–9025. [Google Scholar]
- Bianconi, G; Barabasi, AL. Bose-Einstein condensation in complex networks. Phys. Rev. Lett 2001, 86, 5632–5635. [Google Scholar]
- Bianconi, G. Emergence of weight-topology correlations in complex networks. Europhys. Lett 2005, 71, 1029–1035. [Google Scholar]
- Ferretti, L; Bianconi, G. Dynamics of condensation in growing complex networks. Phys. Rev. E 2008, 78, 056102–056107. [Google Scholar]
- Engel, GS; Calhoun, TR; Read, EL; Ahn, TK; Mancal, T; Cheng, YC; Blankenship, RE; Fleming, GR. Evidence for wavelike energy transfer through quantum coherence in photosynthetic systems. Nature 2007, 446, 782–786. [Google Scholar]
- Hagan, S; Hameroff, SR; Tuszyn, JA. Quantum computation in brain microtubules: Decoherence and biological feasibility. Phys. Rev. E 2002, 65, 061901–0619012. [Google Scholar]
- Coleman, P. Frontier at your fingertips. Nature 2007, 446, 379. [Google Scholar]
- Barrow, JD; Davies, PCW; Davies Harper, CL, Jr. Science and ultimate reality quantum theory, cosmology, and complexity; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Davies, PCW. Quantum fluctuations and life, Preprint arXiv:quant-ph/0403017, 2004.
- Newman, M; Barabási, AL; Watt, DJ. The Structure and Dynamics of Networks; Princeton University Press: Princeton, NJ, USA, 2006. [Google Scholar]
- Rasmussen, S; Chen, L; Deamer, D; Krakauer, DC; Packard, NH; Stadler, PF; Bedau, MA. Transitions from Non living to Living Matter. Science 2004, 303, 963–965. [Google Scholar]
- Bianconi, A. Feshbach shape resonance in multiband superconductivity in heterostructures. J. Supercond. Novel Magn 2005, 18, 25–36. [Google Scholar]
- Caivano, R; Fratini, M; Poccia, N; Ricci, A; Puri, A; Ren, ZA; Dong, XL; Yang, J; Lu, W; Zhao, ZX; Barba, L; Bianconi, A. Feshbach resonance and mesoscopic phase separation near a quantum critical point in multiband FeAs-based superconductors. Supercond. Sci. Technol 2009, 22, 014004–014016. [Google Scholar]
- Fratini, M; Poccia, N; Bianconi, A. The Feshbach resonance and nanoscale phase separation in a polaron liquid near the quantum critical point for a polaron Wigner crystal. J. Phys. Conf. Ser 2008, 108, 012036–012049. [Google Scholar]
- Kugel, KI; Rakhmanov, AL; Sboychakov, AO; Poccia, N; Bianconi, A. Model for phase separation controlled by doping and the internal chemical pressure in different cuprate superconductors. Phys. Rev. B 2008, 78, 165124–165131. [Google Scholar]
- Agrestini, S; Saini, NL; Bianconi, G; Bianconi, A. The Strain of CuO2 Lattice: the Second Variable for the Phase Diagram of Cuprate Perovskites. J. Phys. A: Math. Gen 2003, 36, 9133–9142. [Google Scholar]
- Dunkley, J; Spergel, DN; Komatsu, E; Hinshaw, G; Larson, D; Nolta, MR; Odegard, N; Page, L; Bennett, CL; Gold, B; Hill, RS; Jarosik, N; Weiland, JL; Halpern, M; Kogut, A; Limon, M; Meyer, SS; Tucker, GS; Wollack, E; Wright, EL. Five-year Wilkinson Microwave Anisotropy Probe (WMAP) observations: Bayesian estimation of CMB polarization maps. Astrophys J 2008. [Google Scholar]
- Tammann, GA; Reindl, B; Thim, F; Saha, A; Sandage, A. Cepheids, Supernovae, H0, and the age of the Universe. ASP Conf Series 2001. [Google Scholar]
- Partridge, RB. 3 K: the cosmic microwave background radiation; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Lineweaver, CH; Schwartzman, D. Thermal constraints on the origin and evolution of life in the universe. Cosm Thermobiol 2004, 1–14. [Google Scholar]
- Roos, M. Expansion of the Universe – Standard Big Bang Model. Astrophys J 2008. [Google Scholar]
- Kirzhnits, DA; Linde, AD. Relativistic phase transition. Zhur. Eksp. Teoret. Fiz 1974, 67, 1263–1275. [Google Scholar]
- Nambu, Y. Quasi-particles and gauge invariance in the theory of superconductivity. Phys. Rev 1960, 117, 648–663. [Google Scholar]
- Nambu, Y; Jona-Lasinio, G. Dynamical model of elementary particles based on an analogy with superconductivity I. Phys. Rev 1961, 122, 345–358. [Google Scholar]
- Bogolyubov, NN. A new method in the theory of superconductivity. Il Nuovo Cimento 1958, 7, 794–805. [Google Scholar]
- Alford, M; Rajagopal, K; Wilczek, F. QCD at Finite Baryon Density: Nucleon droplets and color superconductivity. Phys. Lett. B 1998, 422, 247–256. [Google Scholar]
- Rapp, R; Schäfer, TE; Shuryak, V; Velkovsky, M. Diquark Bose condensates in high density matter and instantons. Phys. Rev. Lett 1998, 81, 53–56. [Google Scholar]
- Rajagopal, K; Wilczek, F. The condensed matter physics of QCD, 2000; arXiv:hep-ph/0011333v2, preprint.
- Kolb, EW; Turner, MS. The Early Universe; Academic Press: Redwood City, CA, USA, 1990. [Google Scholar]
- Lewis, JS. Physics and Chemistry of the Solar System; Academic Press: New York, NY, USA, 1997; p. p. 116. [Google Scholar]
- Allègre, CJ; Manhès, G; Göpel, C. The age of the Earth. Geochim. Cosmochim. Acta 1995, 59, 1445–1456. [Google Scholar]
- Kashefi, K; Lovley, DR. Extending the upper temperature limit for life. Science 2003, 934, 301. [Google Scholar]
- Hartmann, WK; Ryder, G; Dones, L; Grinspoon, D. The time-dependent intense bombardment of the primordial Earth/Moon system Moon; University of Arizona Press: Tucson, AZ, USA, 2000. [Google Scholar]
- Schopf, JW. The oldest known records of life: Early Archean stromatilites, microfossils, and organic mattter; Columbia University Press: New York, NY, USA, 1994. [Google Scholar]
- Martin, RD. Primate origins: plugging the gaps. Nature 1993, 363, 223–234. [Google Scholar]
- Knauth, LP. Origin and diagenesis of cherts: an isotopic perspective; Springer-Verlag: New York, NY, USA, 1992. [Google Scholar]
- Knauth, LP; Lowe, DR. High Archean climatic temperature inferred from oxygen isotope geochemistry of cherts in the 3.5 Ga Swaziland Supergroup. South Africa Bull. Geol. Soc. Am 2003, 115, 566–580. [Google Scholar]
- Lazcano, A; Miller, SL. How long did it take life to begin and evolve to cyanobacteria. J. Mol. Evol 1994, 39, 546–549. [Google Scholar]
- Davies, PCW; Lineweaver, CH. Finding a Second Sample of Life on Earth. Astrobiology 2005, 5, 154–163. [Google Scholar]
- Oparin, AI. The origin of life; Dover Publications Inc.: Mineola, NY, USA, 1953. [Google Scholar]
- Alm, E; Arkin, AP. Biological networks. Curr. Opin. Struct. Biol 2003, 3, 193–202. [Google Scholar]
- Alon, U. Biological Networks: The tinkerer as an engineer. Science 2003, 301, 1866–1867. [Google Scholar]see also: Alon, U. An Introduction to System Biology: Design principles of Biological Circuits; Chapman and Hall/CRC: Boca Raton, FL, USA, 2007. [Google Scholar]
- Gibson, DG; Benders, GA; Andrews-Pfannkoch, C; Denisova, EA; Baden-Tillson, H; Zaveri, J; Stockwell, TB; Brownley, A; Thomas, DW; Algire, MA; Merryman, C; Young, L; Noskov, VN; Glass, JI; Craig Venter, J; Hutchison, CA; Smith, HO. Complete chemical synthesis, assembly, and cloning of a mycoplasma genitalium. Genome. Science 2008, 319, 1215–1220. [Google Scholar]
- Luisi, PL. The emergence of life; from chemical origins to synthetic biology; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Bianconi, G. Growing Cayley trees described by a Fermi distribution. Phys. Rev. E 2002, 66, 036116–036121. [Google Scholar]
- Bianconi, G. Size of quantum networks. Phys. Rev. E 2003, 67, 056119–056125. [Google Scholar]
- Bianconi, G. Quantum statistics in complex networks. Phys. Rev. E 2002, 66, 05123–056128. [Google Scholar]
- Garlaschelli, D; Loffredo, MI. Generalized bose-fermi statistics and structural correlations in weighted networks. Phys. Rev. Lett 2009, 102, 038701–038705. [Google Scholar]
- Dorogovtsev, SN; Goltsev, AV. Critical phenomena in complex networks. 2007; arXiv:0705.0010v6, preprint. [Google Scholar]
- Goltsev, AV; Dorogovtsev, SN; Mendes, JFF. K-core (bootstrap) percolation on complex networks: critical phenomena and nonlocal effects, Preprint, arXiv:cond-mat\0602611v2, 2006.
- Bianconi, G; Ferretti, L; Franz, S. Non-neutral theory of biodiversity 2009.
- Bianconi, G. Flux distribution of metabolic networks close to optimal biomass production. Phys. Rev. E 2008, 78, 035101–035105. [Google Scholar]
- Bianconi, G; Zecchina, R. Viable flux distribution in metabolic networks. Netw. Heterogeneous Media 2008, 3, 361–369. [Google Scholar]
- Volkov, I; Banavar, JR; Maritan, A. Organization of ecosystems in the vicinity of a novel phase transition. 2004; arxiv:q-bio70406020v1, preprint. [Google Scholar]
- Volkov, I; Banavar, JR; Maritan, A. A novel ensemble in statistical physics. J. Stat. Phys 2006, 123, 167–180. [Google Scholar]
- Bianconi, G. The entropy of randomized network ensembles. Europhys. Lett 2008, 81, 28005–28011. [Google Scholar]
- Careri, G. Order and Disorder Matter; Addison-Wesley: New York, NY, USA, 1984. [Google Scholar]
- Davies, PCW. Quantum theory and the ascent of life. New Sci 2004, 2477. [Google Scholar]
- Abbott, D; Davies, PCW; Pati, AK. Quantum Aspect of Life; Imperial College Press: London, UK, 2008. [Google Scholar]
- Bardeen, J; Cooper, LN; Schrieffer, JR. The microscopic theory of superconductivity. Phys. Rev 1957, 106, 162–164. [Google Scholar]
- Josephson, BD. Possible new effects in superconductive tunnelling. Phys. Lett 1962, 1, 251–253. [Google Scholar]
- Leggett, A. The new physics; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar]
- Muller, KA; Bednorz, JG. Perovskite-type oxides the new approach to high-Tc superconductivity. Rev Mod Phys 1988, 60, 585–600. [Google Scholar]
- Nagamatsu, J; Nakagawa, N; Muranaka, T; Zenitani, Y; Akimitsu, J. Superconductivity at 39 K in magnesium diborides. Nature 2001, 410, 63–64. [Google Scholar]
- Kamihara, Y; Watanabe, T; Hirano, M; Hosono, H. Iron-based layered superconductor LaO1-x Fx FeAs (x = 0.05 – 0.12) with Tc= 26 K. J. Am. Chem. Soc 2008, 130, 3296–3297. [Google Scholar]
- Kristoffel, N; Konsin, P; Ord, T. Two-band model for high-temperature superconductivity. Riv. Nuovo Cim 1994, 17, 1–41. [Google Scholar]
- Kresin, VZ; Wolf, SA. Gaplessness and properties of layered superconductors: Application to high-Tc cuprates. Phys. Rev. B 1995, 51, 1229–1235. [Google Scholar]
- Combescot, R. Multiband model of high-Tc superconductors. Phys. Rev. B 1998, 57, 8632–8641. [Google Scholar]
- Imada, M; Kohno, M. How are spin gap and pairing correlations of doped Mott insulators controlled by the geometry of the lattice structure? Phys. Rev. Lett 2000, 84, 303–307. [Google Scholar]
- Bussmann-Holder, A; Bianconi, A. Raising the diboride superconductor transition temperature using quantum interference effects. Phys. Rev. B 2003, 67, 132509–13213. [Google Scholar]
- Tomonaga, S. On the effect of the field reactions on the interaction of mesotrons and nuclear particles. III. Prog. Theor. Phys. (Kyoto) 1947, 2, 6–24. [Google Scholar]
- Kondo, J. Superconductivity in Transition Metal. Prog. Theor. Phys 1963, 29, 1–9. [Google Scholar]
- Bogolyubov, NN. On a Variational Principle in the Many-Body Problem. Soviet Phys. JETP 1958, 3, 292–296. [Google Scholar]
- Feshbach, H; Porter, CE; Weisskopf, VF. Model for nuclear reactions with neutrons. Phys. Rev 1954, 96, 448–464. [Google Scholar]
- Feshbach, H. Unified theory of nuclear reactions. Rev. Mod. Phys 1964, 36, 1076–1078. [Google Scholar]
- Fano, U. Effects of configuration interaction on intensities and phase shifts. Phys. Rev 1961, 124, 1866–1878. [Google Scholar]
- Tiesinga, E; Verhaar, BJ; Stoof, HTC. Threshold and resonance phenomena in ultracold ground-state collisions. Phys. Rev. A 1993, 47, 4114–4122. [Google Scholar]
- Zwierlein, MW; Stan, CA; Schunck, CH; Raupach, SMF; Gupta, S; Hadzibabic, Z; Ketterle, W. Observation of Bose-Einstein condensation of molecules. Phys. Rev. Lett 2003, 91, 250401–250405. [Google Scholar]
- Vittorini-Orgeas, A; Bianconi, A. From majorana theory of atomic autoionization to feshbach resonances in high temperature superconductors. J Supercond Novel Magn 2009, 52, 1557–1939. [Google Scholar]
- Blatt, JM; Thompson, CJ. Shape resonances in superconducting thin films. Phys. Rev. Lett 1963, 10, 332–334. [Google Scholar]
- Thompson, CJ; Blatt, JM. Shape resonances in superconductors - II simplified theory. Phys. Lett 1963, 5, 6–9. [Google Scholar]
- Blatt, JM. Theory of Superconductivity; Academic Press: New York, NY, USA, 1964. [Google Scholar]
- Bianconi, A; Saini, NL; Lanzara, A; Missori, M; Rossetti, T; Oyanagi, H; Yamaguchi, H; Oka, K; Ito, T. Determination of the local lattice distortions in the CuO2 plane of La1.85Sr0.15 CuO4. Phys. Rev. Lett 1996, 76, 3412–3415. [Google Scholar]
- Saini, NL; Avila, J; Bianconi, A; Lanzara, A; Asenzio, MC; Tajima, S; Gu, GD; Koshizuka, N. Topology of the Pseudogap and Shadow Bands in Bi2Sr2CaCu2O8+δ at Optimum Doping. Phys. Rev. Lett 1997, 79, 3467–3470. [Google Scholar]
- Bianconi, A; Saini, NL; Agrestini, S; Di Castro, D; Bianconi, G. The strain quantum critical point for superstripes in the phase diagram of all cuprate perovskites. Int. J. Mod. Phys. B 2000, 14, 3342–3355. [Google Scholar]
- Bianconi, A; Agrestini, S; Bianconi, G; Di Castro, D; Saini, NL. A Quantum Phase Transition Driven by the Electron Lattice Interaction Gives High Tc Superconductivity. J. Alloys Comp 2001, 317, 537–541. [Google Scholar]
- Poccia, N; Fratini, M. The misfit strain critical point in the 3D phase diagrams of cuprates. J Supercond Novel Magn 2009, 22, 1557–1939. [Google Scholar]
- Bianconi, A. Process of increasing the critical temperature Tc of a bulk superconductor by making metal heterostructures at the atomic limit. U.S. Patent: 6, 265, 019 B1, 2001. [Google Scholar]
- Bianconi, A. On the possibility of new high Tc superconductors by producing metal heterostructures as in the cuprate perovskites. Solid State Commun 1994, 89, 933–936. [Google Scholar]
- Bianconi, A; Valletta, A; Perali, A; Saini, NL. Superconductivity of a striped phase at the atomic limit. Phys. C 1998, 296, 269–280. [Google Scholar]
- Bianconi, A; Valletta, A; Perali, A; Saini, NL. High Tc superconductivity in a superlattice of quantum stripes. Solid State Commun 1997, 102, 369–374. [Google Scholar]
- Boroudjerdi, H; Kim, YW; Naji, A; Netz, RR; Schlagberger, X; Serr, A. Static and dynamics of Strongly Charged Soft Matter, Preprint, arXiv: cond-mat/ 0509007v1, 2005.
- Kusmartsev, FV. Formation of electron strings in narrow band polar semiconductors. Phys. Rev. Lett 2000, 84, 530–533. [Google Scholar]
- Ahn, KH; Lookman, T; Bishop, AR. Strain-induced metal–insulator phase coexistence in perovskite manganites. Nature 2004, 428, 401–404. [Google Scholar]
- Clary, DC. Quantum Dynamics of Chemical Reactions. Science 2008, 321, 789. [Google Scholar]
- Hu, W; Schatz, GC. Theories of reactive scattering. J. Chem. Phys 2006, 125, 13230. [Google Scholar]
- Althorpe, SC; Clary, DC. Quantum scattering calculations on chemical reactions. Annu. Rev. Phys. Chem 2003, 54, 493–529. [Google Scholar]
- Clary, DC. Theoretical studies on bimolecular reaction dynamics. Proc. Nat. Acad. Sci. USA 2008, 105, 12649–12653. [Google Scholar]
- Yang, X. State-to-state dynamics of elementary bimolecular reactions. Annu. Rev. Phys. Chem 2007, 58, 433–459. [Google Scholar]
- Qiu, M; Ren, Z; Li, CH; Dai, D; Harich, SA; Wang, X; Yang, X; Xu, C; Xie, D; Gustafsson, M; Skodje, RT; Sun, Z; Zhang, DH. Observation of Feshbach Resonances in the F + H2 HF + H Reaction. Science 2006, 311, 1440–1443. [Google Scholar]
- Wang, X; Dong, W; Qiu, M; Ren, Z; Che, Li; Dai, D; Wang, X; Yang, X; Sun, Z; Fu, B; Lee, S-Y; Xu, X; Zhang, DH. HF(v= 3) forward scattering in the F + H2 reaction: Shape resonance and slow-down mechanism. Proc. Nat. Acad. Sci. USA 2008, 105, 6227–6231. [Google Scholar]
- Ren, Z; Che, L; Qiu, M; Wang, X; Dong, W; Dai, D; Wang, X; Yang, X; Sun, Z; Fu, B; et al. Probing the resonance potential in the F atom reaction with hydrogen deuteride with spectroscopic accuracy. Proc. Nat. Acad. Sci. USA 2008, 105, 12662–12666. [Google Scholar]
- Garcia-Viloca, M; Gao, J; Karplus, M; Truhlar, DG. How enzyme works: analysis by modern rate theory and computer simulations. Science 2004, 303, 186–195. [Google Scholar]
- Kohen, A. Isotope Effects in Chemical and Biology; Kohen, A, Limbach, HH, Eds.; CRC: Boca Raton, FL, USA, 2006; pp. 743–764. [Google Scholar]
- Dybala-Defratyka, A; Paneth, P; Banerjee, R; Truhlar, DG. Coupling of hydrogenic tunneling to active-site motion in the hydrogen radical transfer catalyzed by a coenzyme B12-dependent mutase. Proc. Nat. Acad. Sci. USA 2007, 104, 10774–10779. [Google Scholar]
- Jeong, H; Mason, SP; Barabási, A-L; Oltvai, ZN. Lethality and centrality in protein networks. Nature 2001, 411, 41–42. [Google Scholar]
- Ravasz, E; Somera, AL; Mongru, DA; Oltvai, ZN; Barabási, A-L. Hierarchical Organization of Modularity in Metabolic Networks. Science 2002, 297, 1551–1555. [Google Scholar]
- Schrödinger, E. What Is Life? Cambridge University Press, 1944. [Google Scholar]

© 2009 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Poccia, N.; Ricci, A.; Innocenti, D.; Bianconi, A. A Possible Mechanism for Evading Temperature Quantum Decoherence in Living Matter by Feshbach Resonance. Int. J. Mol. Sci. 2009, 10, 2084-2106. https://doi.org/10.3390/ijms10052084
Poccia N, Ricci A, Innocenti D, Bianconi A. A Possible Mechanism for Evading Temperature Quantum Decoherence in Living Matter by Feshbach Resonance. International Journal of Molecular Sciences. 2009; 10(5):2084-2106. https://doi.org/10.3390/ijms10052084
Chicago/Turabian StylePoccia, Nicola, Alessandro Ricci, Davide Innocenti, and Antonio Bianconi. 2009. "A Possible Mechanism for Evading Temperature Quantum Decoherence in Living Matter by Feshbach Resonance" International Journal of Molecular Sciences 10, no. 5: 2084-2106. https://doi.org/10.3390/ijms10052084
APA StylePoccia, N., Ricci, A., Innocenti, D., & Bianconi, A. (2009). A Possible Mechanism for Evading Temperature Quantum Decoherence in Living Matter by Feshbach Resonance. International Journal of Molecular Sciences, 10(5), 2084-2106. https://doi.org/10.3390/ijms10052084




