Elastic Properties of Alloyed Cementite M3X (M = Fe, Cr; X = C, B) Phases from First-Principle Calculations and CALPHAD Model
Abstract
1. Introduction
2. Computational Methods
2.1. Elastic Properties at 0 K
2.2. CALPHAD Modeling of Elastic Constants
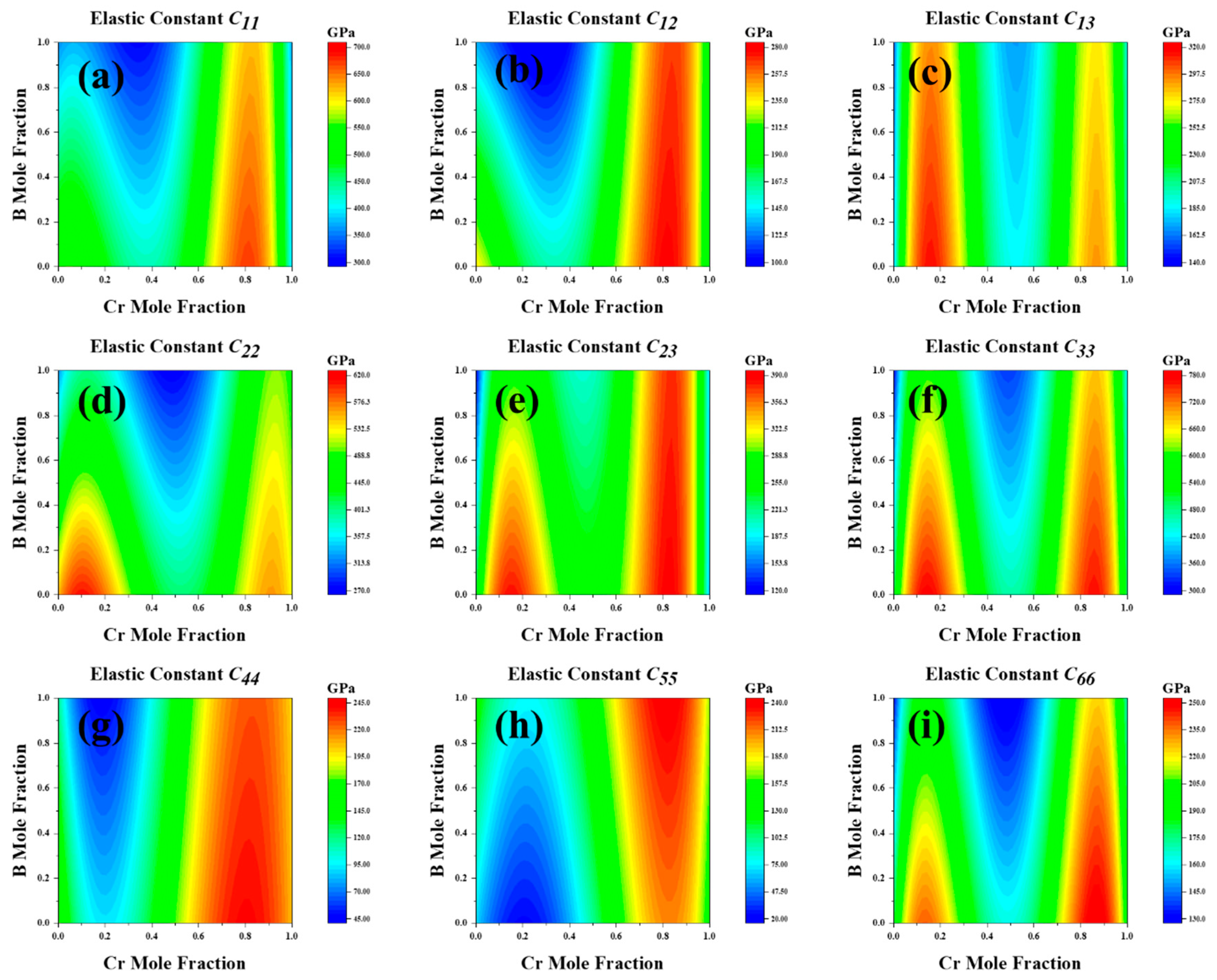
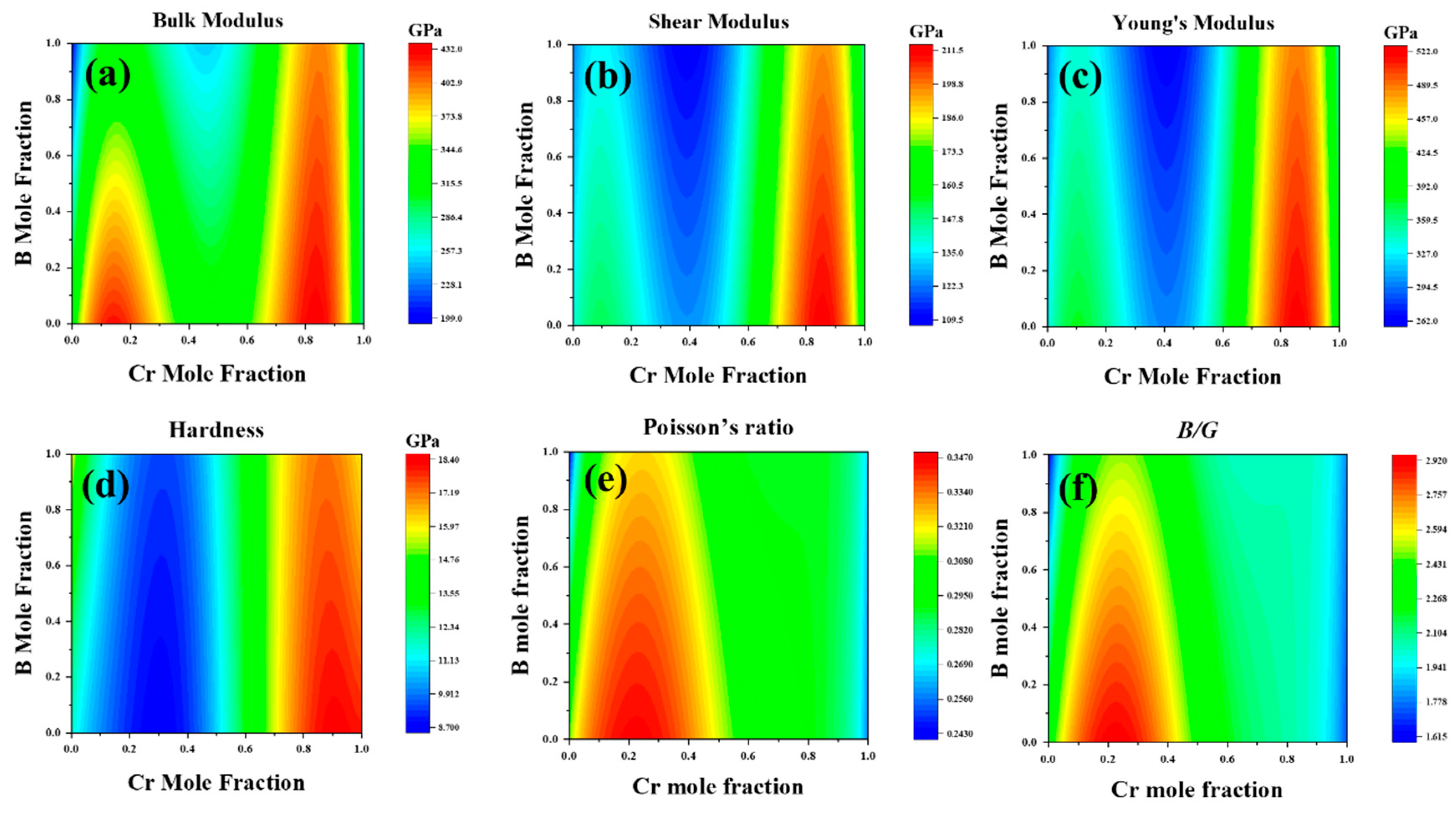
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lin, Y.; Chong, X.; Ding, Y. irst-Principles Calculations of Thermal and Electrical Transport Properties of bcc and fcc Dilute Fe–X (X = Al, Co, Cr, Mn, Mo, Nb, Ni, Ti, V, and W) Binary Alloys. Metals 2021, 11, 1988. [Google Scholar] [CrossRef]
- Ande, C.K.; Sluiter, M.H.F. First-principles prediction of partitioning of alloying elements between cementite and ferrite. Acta Mater. 2010, 58, 6276–6281. [Google Scholar] [CrossRef]
- Chong, X.Y.; Jiang, Y.H.; Zhou, R. Elastic properties and electronic structures of CrxBy as superhard compounds. J. Alloys Compd. 2014, 610, 684–694. [Google Scholar] [CrossRef]
- Chong, X.; Jiang, Y.; Feng, J. Exploring the intrinsic ductile metastable Fe-C compounds: Complex chemical bonds, anisotropic elasticity and variable thermal expansion. J. Alloys Compd. 2018, 745, 196–211. [Google Scholar] [CrossRef]
- Chong, X.; Guan, P.-W.; Hu, M. Exploring accurate structure, composition and thermophysical properties of η carbides in 17.90 wt% W-4.15 wt% Cr-1.10 wt% V-0.69 wt% C steel. Scr. Mater. 2018, 154, 149–153. [Google Scholar] [CrossRef]
- Wang, G.; Jiang, Y.; Li, Z. Balance between strength and ductility of dilute Fe2B by high-throughput first-principles calculations. Ceram. Int. 2021, 47, 4758–4768. [Google Scholar] [CrossRef]
- Zhang, W.H.; Lv, Z.Q.; Shi, Z.P. Electronic, magnetic and elastic properties of ε-phases Fe3X(X = B, C, N) from density-functional theory calculations. J. Magn. Magn. Mater. 2012, 324, 2271–2276. [Google Scholar] [CrossRef]
- Wei, L.I. Progress of Wear-resistant Steel and Iron Technology. Foundry 2006, 55, 1105–1109. [Google Scholar]
- Li, H.N.; Zhang, B.H.; Qiao, G.Y. Refinement effect of NbC particle additions on the microstructure of high-speed steel rolls. J. Mater. Res. Technol. 2023, 26, 1697–1711. [Google Scholar] [CrossRef]
- Chong, X.; Hu, M.; Wu, P. Tailoring the anisotropic mechanical properties of hexagonal M7X3 (M = Fe, Cr, W, Mo; X = C, B) by multialloying. Acta Mater. 2019, 169, 193–208. [Google Scholar] [CrossRef]
- Lentz, J.; Röttgera, A.; Theisen, W. Hardness and modulus of Fe2B, Fe3(C,B), and Fe23(C,B)6 borides and carboborides in the Fe-C-B system. Mater. Charact. 2018, 135, 192–202. [Google Scholar] [CrossRef]
- Shein, I.; Medvedeva, N.; Ivanovskii, A. Electronic and structural properties of cementite-type M3X (M = Fe, Co, Ni; X = C or B) by first principles calculations. Phys. B Condens. Matter 2006, 371, 126–132. [Google Scholar] [CrossRef]
- Konyaeva, M.; Medvedeva, N. Electronic Structure, Magnetic Properties, and Stability of the Binary and Ternary Carbides (Fe,Cr)3C and (Fe,Cr)7C3. Phys. Solid State 2009, 51, 2084–2089. [Google Scholar] [CrossRef]
- Matthews, S.; Hyland, M.; James, B. Microhardness variation in relation to carbide development in heat treated Cr3C2–NiCr thermal spray coatings. Acta Mater. 2003, 51, 4267–4277. [Google Scholar] [CrossRef]
- Zhou, C.T.; Xiao, B.; Feng, J. First principles study on the elastic properties and electronic structures of (Fe, Cr)3C. Comput. Mater. Sci. 2009, 45, 986–992. [Google Scholar] [CrossRef]
- Razumovskiy, V.I.; Ghosh, G. A first-principles study of cementite (Fe3C) and its alloyed counterparts: Structural properties, stability, and electronic structure. Comput. Mater. Sci. 2015, 110, 169–181. [Google Scholar] [CrossRef]
- Lv, Z.Q.; Fu, W.T.; Sun, S.H. First-principles study on the electronic structure, magnetic properties and phase stability of alloyed cementite with Cr or Mn. J. Magn. Magn. Mater. 2011, 323, 915–919. [Google Scholar] [CrossRef]
- Lv, Z.Q.; Zhang, F.C.; Sun, S.H. First-principles study on the mechanical, electronic and magnetic properties of Fe3C. Comput. Mater. Sci. 2008, 44, 690–694. [Google Scholar] [CrossRef]
- Taylor, K.A.; Hansen, S.S. The boron hardenability effect in thermomechanically processed, direct-quenched 0.2 Pct C steels. Metall. Trans. A 1990, 21, 1697–1708. [Google Scholar] [CrossRef]
- Jiang, D.; Xiao, W.; Liu, D. Structural stability, electronic structures, mechanical properties and debye temperature of W-Re alloys: A first-principles study. Fusion Eng. Des. 2021, 162, 112081. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Xiao, B.; Xing, J.; Feng, J. A comparative study of Cr7C3, Fe3C and Fe2B in cast iron both from ab initio calculations and experiments. J. Phys. D Appl. Phys. 2009, 42, 115415. [Google Scholar] [CrossRef]
- Katsura, T.; Tange, Y. A Simple Derivation of the Birch–Murnaghan Equations of State (EOSs) and Comparison with EOSs Derived from Other Definitions of Finite Strain. Minerals 2019, 9, 745. [Google Scholar] [CrossRef]
- Shang, S.; Kim, D.; Zacherl, C. Effects of alloying elements and temperature on the elastic properties of dilute Ni-base superalloys from first-principles calculations. J. Appl. Phys. 2012, 112, 053515. [Google Scholar] [CrossRef]
- Liu, Y.; Jiang, Y.; Xing, J. Mechanical properties and electronic structures of M23C6 (M = Fe, Cr, Mn)-type multicomponent carbides. J. Alloys Compd. 2015, 648, 874–880. [Google Scholar] [CrossRef]
- Nikolussi, M.; Shang, S.; Gressmann, T. Extreme elastic anisotropy of cementite, Fe3C: First-principles calculations and experimental evidence. Scr. Mater. 2008, 59, 814–817. [Google Scholar] [CrossRef]
- Hill, R. The Elastic Behaviour of a Crystalline Aggregate. Proc. Phys. Soc. Sect. A 1952, 65, 349–354. [Google Scholar] [CrossRef]
- Tian, Y.; Xu, B.; Zhao, Z. Microscopic theory of hardness and design of novel superhard crystals. Int. J. Refract. Met. Hard Mater. 2012, 33, 93–106. [Google Scholar] [CrossRef]
- Wang, D.T.; Zhang, X.Z.; Nagaumi, H. Exploring the Relationship between the Structural Characteristics and Mechanical Behavior of Multicomponent Fe-Containing Phases: Experimental Studies and First-Principles Calculations. Molecules 2023, 28, 7141. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Zhou, Y.; Yang, J. Optimization on mechanical properties of Fe7−xCrxC3 carbides by first-principles investigation. J. Alloys Compd. 2013, 560, 49–53. [Google Scholar] [CrossRef]
- Chong, X.; Jiang, Y.; Zhou, R. First principles study the stability, mechanical and electronic properties of manganese carbides. Comput. Mater. Sci. 2014, 87, 19–25. [Google Scholar] [CrossRef]
- Liu, Z.K.; Zhang, H.; Ganeshan, S. Computational modeling of effects of alloying elements on elastic coefficients. Scr. Mater. 2010, 63, 686–691. [Google Scholar] [CrossRef]
- Fruchart, D.; Chaudouet, P.; Fruchart, R. Etudes structurales de composés de type cémentite: Effet de l’hydrogène sur Fe3C suivi par diffraction neutronique. Spectrométrie Mössbauer sur FeCo2B et Co3B dopés au57Fe. J. Solid State Chem. 1984, 51, 246–252. [Google Scholar] [CrossRef]
- Scott, H.P.; Williams, Q.; Knittle, E. Stability and equation of state of Fe3C to 73 GPa: Implications for carbon in the Earth’s core. Geophys. Res. Lett. 2001, 28, 1875–1878. [Google Scholar] [CrossRef]
- Ghosh, G. A first-principles study of cementite (Fe3C) and its alloyed counterparts: Elastic constants, elastic anisotropies, and isotropic elastic moduli. AIP Adv. 2015, 5, 169–181. [Google Scholar] [CrossRef]
- Villars, P.; Cenzual, K.; Pearson, W.B. Pearson’s Crystal Data: Crystal Structure Database for Inorganic Compounds; ASM International: Almere, The Netherlands, 2007. [Google Scholar]
- Li, Y.; Gao, Y.; Xiao, B. The electronic, mechanical properties and theoretical hardness of chromium carbides by first-principles calculations. J. Alloys Compd. 2011, 509, 5242–5249. [Google Scholar] [CrossRef]
- Wang, X.; Liu, L.B.; Wang, M.F. Computational modeling of elastic constants as a function of temperature and composition in Zr–Nb alloys. Calphad 2015, 48, 89–94. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, L.J. Composition-dependent hardness and Young’s modulus in fcc Ni-X (X = Rh, Ta, W, Re, Os, and Ir) alloys: Experimental measurements and CALPHAD modeling. J. Mater. Res. 2019, 34, 3104–3115. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, L.; Guo, Z. Study of low-modulus biomedical β Ti–Nb–Zr alloys based on single-crystal elastic constants modeling. J. Mech. Behav. Biomed. Mater. 2016, 62, 310–318. [Google Scholar] [CrossRef]
- Liang, J.S.; Liu, L.B.; Xu, G.L.; Wang, X.; Zhang, L.G.; Shi, X.; Tao, X.M. Compositional screening of Zr-Nb-Mo alloys with CALPHAD-type model for promising bio-medical implants. Calphad 2017, 56, 196–206. [Google Scholar] [CrossRef]
- Zeng, W.-J.; Hu, K.; Liu, H.-S. Crystal structures and elastic properties of Ti(Cu,Pt)2 and Ti(Cu,Pt)3 phases. Trans. Nonferrous Met. Soc. China 2020, 30, 1839–1848. [Google Scholar] [CrossRef]
- Liu, Z.K. Computational thermodynamics and its applications. Acta Mater. 2020, 200, 745–792. [Google Scholar] [CrossRef]
- Liao, M.Q.; Liu, Y.; Cui, P.C. Modeling of alloying effect on elastic properties in BCC Nb-Ti-V-Zr solid solution: From unary to quaternary. Comput. Mater. Sci. 2020, 172, 109289. [Google Scholar] [CrossRef]
- Marker, C.; Shang, S.L.; Zhao, J.C. Effects of alloying elements on the elastic properties of bcc Ti-X alloys from first-principles calculations. Comput. Mater. Sci. 2018, 142, 215–226. [Google Scholar] [CrossRef]
- Hill, R. A self-consistent mechanics of composite materials. J. Mech. Phys. Solids 1965, 13, 213–222. [Google Scholar] [CrossRef]
- Dodd, S.; Saunders, G.; Cankurtaran, M. Ultrasonic study of the temperature and hydrostatic-pressure dependences of the elastic properties of polycrystalline cementite (Fe3C). Phys. Status Solidi (A) 2003, 198, 272–281. [Google Scholar] [CrossRef]
- Umemoto, M.; Todaka, Y.; Takahashi, T. Characterization of bulk cementite produced by mechanical alloying and spark plasma sintering. J. Metastable Nanocryst. Mater. 2003, 15, 607–614. [Google Scholar] [CrossRef]
- Guo, X.; Li, L.; Liu, Z. Hardness of covalent compounds: Roles of metallic component and d valence electrons. J. Appl. Phys. 2008, 104, 023503. [Google Scholar] [CrossRef]
- Gueddouh, A.; Bentria, B.; Lefkaier, I.K. Effect of spin polarization on the structural properties and bond hardness of FexB (x = 1, 2, 3) compounds first-principles study. Bull. Mater. Sci. 2016, 39, 1427–1434. [Google Scholar] [CrossRef]
- Jiang, C. First-principles study of structural, elastic, and electronic properties of chromium carbides. Appl. Phys. Lett. 2008, 92, 889. [Google Scholar] [CrossRef]
- Ledbetter, H. Polycrystalline elastic constants of in situ cementite (Fe3C). Mater. Sci. Eng. A 2010, 527, 2657–2661. [Google Scholar] [CrossRef]
- Umemoto, M.; Liu, Z.G.; Masuyama, K. Influence of alloy additions on production and properties of bulk cementite. Scr. Mater. 2001, 45, 391–397. [Google Scholar] [CrossRef]

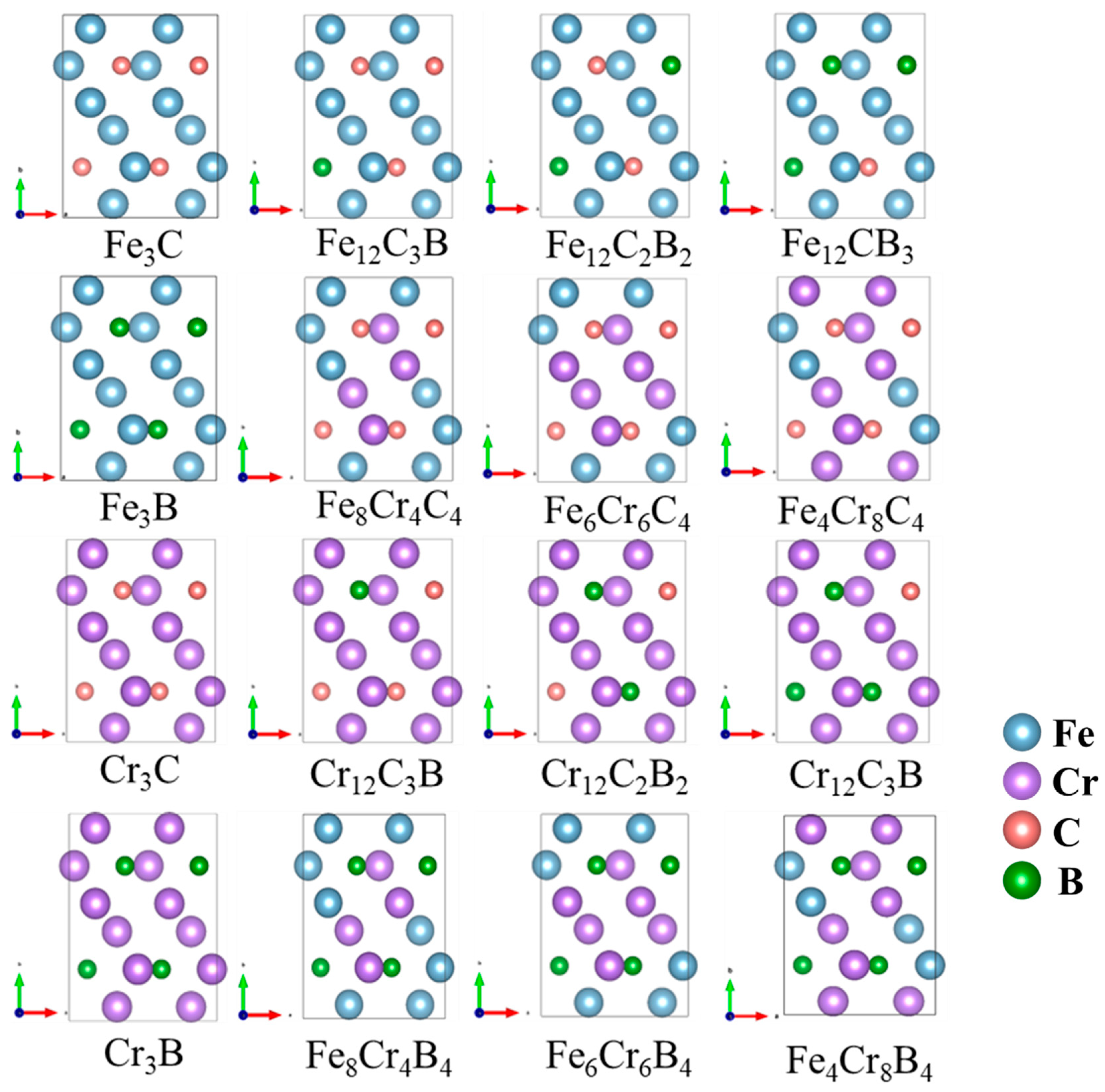
| Phases | Lattice Constants (Å) | V/Å3 | E0 (eV/Cell) | B0 (GPa) | |||
|---|---|---|---|---|---|---|---|
| a | b | c | |||||
| Fe3C | 4.811 | 6.521 | 4.31 | 154.258 | −11,013.761 | 1.984 | 4.602 |
| Fe3C a | 5.092 | 6.748 | 4.520 | ||||
| Fe3C b | 5.062 | 6.748 | 4.533 | ||||
| Fe8Cr4C4 | 4.884 | 6.543 | 4.373 | 139.612 | −17,413.326 | 1.573 | 3.803 |
| Fe6Cr6C4 | 4.967 | 6.746 | 4.44 | 148.753 | −20,618.562 | 2.008 | 3.203 |
| Fe4Cr8C4 | 5.119 | 6.573 | 4.467 | 150.277 | −23,823.442 | 1.903 | 4.945 |
| Cr3C | 5.191 | 6.661 | 4.516 | 160.851 | −30,234.414 | 1.675 | 3.923 |
| Cr3C c | 5.009 | 6.707 | 4.456 | ||||
| Cr3C d | 5.120 | 6.800 | 4.580 | ||||
| Fe12C3B | 5.273 | 6.498 | 4.256 | 145.852 | −10,926.393 | 1.805 | 3.827 |
| Fe12CB3 | 5.045 | 6.759 | 4.504 | 153.581 | −10,771.459 | 1.983 | 4.653 |
| Fe3B | 5.473 | 6.711 | 4.387 | 160.055 | −10,706.463 | 1.425 | 4.252 |
| Fe3B e | 5.397 | 6.648 | 4.380 | ||||
| Cr12C3B | 5.198 | 6.675 | 4.536 | 157.377 | −30,155.826 | 1.774 | 4.269 |
| Cr12C2B2 | 5.216 | 6.697 | 4.551 | 158.964 | −30,078.382 | 1.734 | 4.318 |
| Cr12CB3 | 5.234 | 6.721 | 4.567 | 160.686 | −30,000.972 | 1.696 | 4.146 |
| Cr3B | 5.253 | 6.744 | 4.583 | 162.381 | −29,923.457 | 1.675 | 3.924 |
| Fe8Cr4B4 | 5.011 | 6.714 | 4.474 | 150.521 | −23,823.636 | 1.9141 | 4.905 |
| Fe6Cr6B4 | 5.352 | 6.595 | 4.32 | 152.474 | −20,309.882 | 1.800 | 4.499 |
| Phases | C11 | C22 | C33 | C44 | C55 | C66 | C12 | C13 | C23 |
|---|---|---|---|---|---|---|---|---|---|
| Fe3C | 383.89 | 553.09 | 495.72 | 174.78 | 69.11 | 180.73 | 236.57 | 179.14 | 235.47 |
| Fe3B | 358.02 | 323.17 | 302.63 | 177.70 | 132.41 | 131.72 | 132.07 | 155.72 | 120.11 |
| Cr3B | 363.43 | 478.06 | 415.49 | 199.81 | 167.33 | 167.33 | 184.81 | 190.09 | 175.17 |
| Cr3C | 552.84 | 523.00 | 484.03 | 205.90 | 141.68 | 192.73 | 176.02 | 203.86 | 167.68 |
| Cr3C a | 518.7 | 445.6 | 401.6 | 193.9 | 148 | 202.3 | 195.3 | 208.4 | 212.2 |
| Cr12CB3 | 365.67 | 507.48 | 440.30 | 199.06 | 156.98 | 161.40 | 163.76 | 197.80 | 167.40 |
| Cr12C2B2 | 334.73 | 494.08 | 434.24 | 171.43 | 124.81 | 167.14 | 185.09 | 195.06 | 195.06 |
| Cr12C3B | 385.06 | 506.36 | 467.07 | 204.31 | 146.90 | 186.74 | 178.32 | 200.36 | 173.13 |
| Fe4Cr8C4 | 517.04 | 480.47 | 464.22 | 186.61 | 129.33 | 185.14 | 225.15 | 197.31 | 209.36 |
| Fe6Cr6C4 | 483.26 | 534.05 | 416.59 | 188.57 | 91.67 | 205.01 | 206.17 | 195.43 | 196.12 |
| Fe12CB3 | 522.47 | 397.16 | 493.26 | 183.40 | 137.31 | 157.18 | 212.92 | 188.03 | 242.54 |
| Fe12C2B2 | 345.52 | 343.59 | 370.37 | 175.13 | 118.42 | 143.31 | 115.48 | 154.30 | 141.29 |
| Fe12C3B | 373.59 | 356.57 | 345.10 | 135.29 | 83.880 | 130.90 | 143.94 | 108.47 | 149.03 |
| Fe8Cr4B4 | 394.02 | 441.99 | 452.59 | 199.04 | 123.98 | 138.24 | 138.24 | 183.50 | 209.86 |
| Fe6Cr6B4 | 489.09 | 367.81 | 451.08 | 206.99 | 170.29 | 123.45 | 201.51 | 181.61 | 223.84 |
| Fe4Cr8B4 | 418.64 | 401.33 | 406.39 | 237.73 | 195.15 | 161.14 | 189.51 | 181.71 | 212.32 |
| Fe8Cr4C4 | 573.47 | 565.26 | 587.48 | 223.45 | 68.11 | 226.46 | 250.64 | 287.25 | 288.58 |
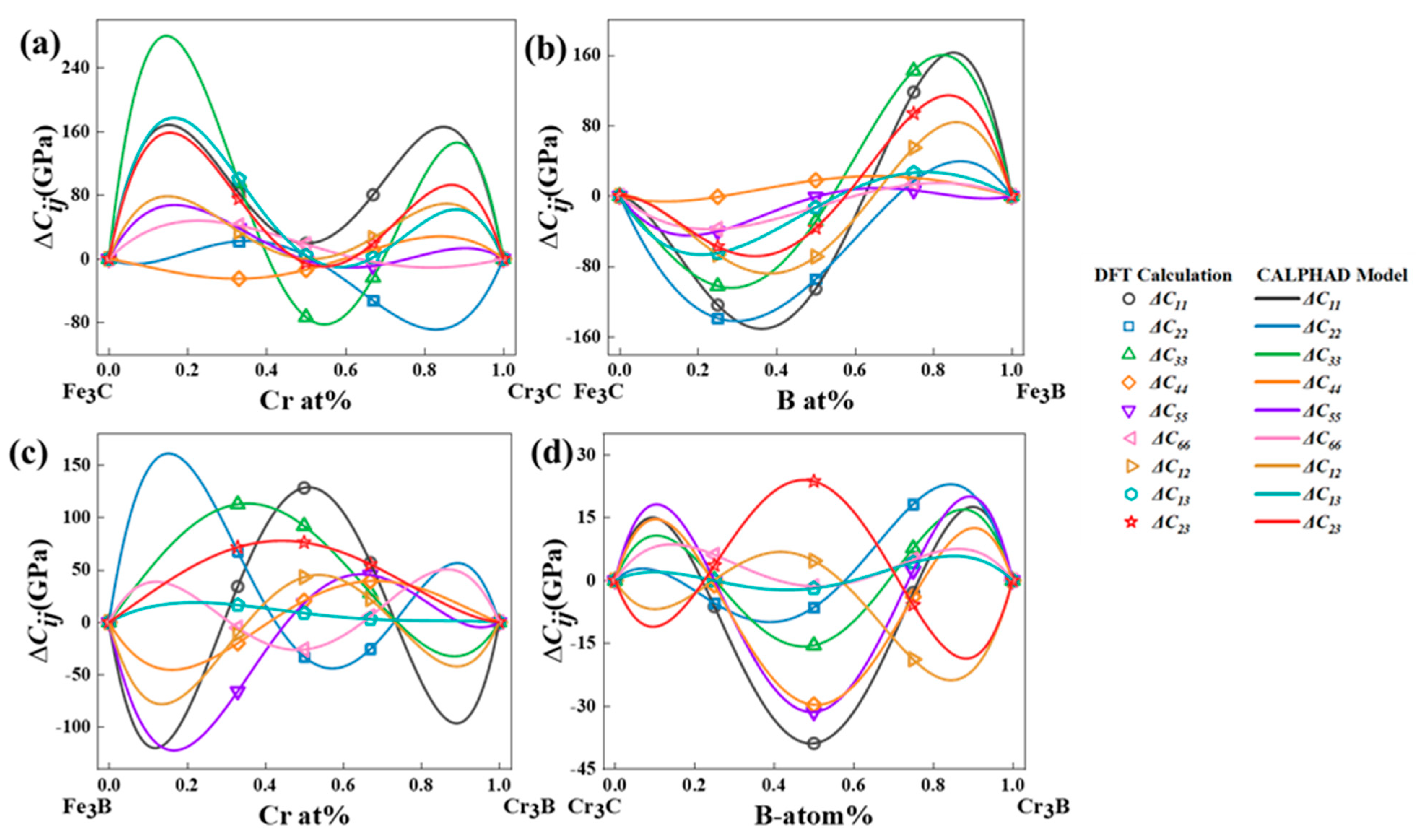
| Phases | ΔC11 | ΔC22 | ΔC33 | ΔC44 | ΔC55 | ΔC66 | ΔC12 | ΔC13 | ΔC23 | ΔG | ΔB | ΔE | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| (Fe,Cr)3B | 0L | −156 | −26 | −62 | −125 | −118 | −6 | 19 | −8 | 94.5 | −31 | −67 | −150 |
| 1L | 18 | 126 | 40 | 13 | −15 | −6 | −101 | 23 | −51 | −20 | 9 | 15 | |
| 2L | 525 | 239 | 332 | 526 | 526 | 140 | −274 | 75 | −403 | 110 | 312 | 693 | |
| (Fe,Cr)3C | 0L | 78 | 16 | −293 | −7 | −55 | 73 | −1 | 16 | −22 | −29 | −12 | −27 |
| 1L | −13 | −496 | −793 | −315 | 243 | −302 | −53 | −645 | −373 | −381 | −18 | −91 | |
| 2L | 2513 | −732 | 3943 | 636 | 214 | 113 | 1183 | 1851 | 2043 | 1855 | 165 | 657 | |
| Fe3(C,B) | 0L | −422 | −378 | −115 | −4 | 71 | −51 | −275 | −53 | −146 | −45 | −11 | −62 |
| 1L | 1290 | 830 | 1305 | 249 | 116 | 271 | 646 | 487 | 806 | 807 | 203 | 599 | |
| 2L | 1625 | 206 | 888 | −343 | −73 | −54 | 976 | −199 | 968 | 51 | −26 | 100 | |
| (Fe,Cr)3B | 0L | 513 | −131 | 368 | 73 | 92 | −104 | 172 | 35 | 305 | 197 | 26 | 98 |
| 1L | 152 | −621 | −563 | 739 | 394 | 72 | 222 | −90 | −108 | −201 | 73 | 115 | |
| 2L | −265 | 1959 | −428 | −107 | −327 | 909 | −126 | 79 | −154 | −116 | 168 | 335 |
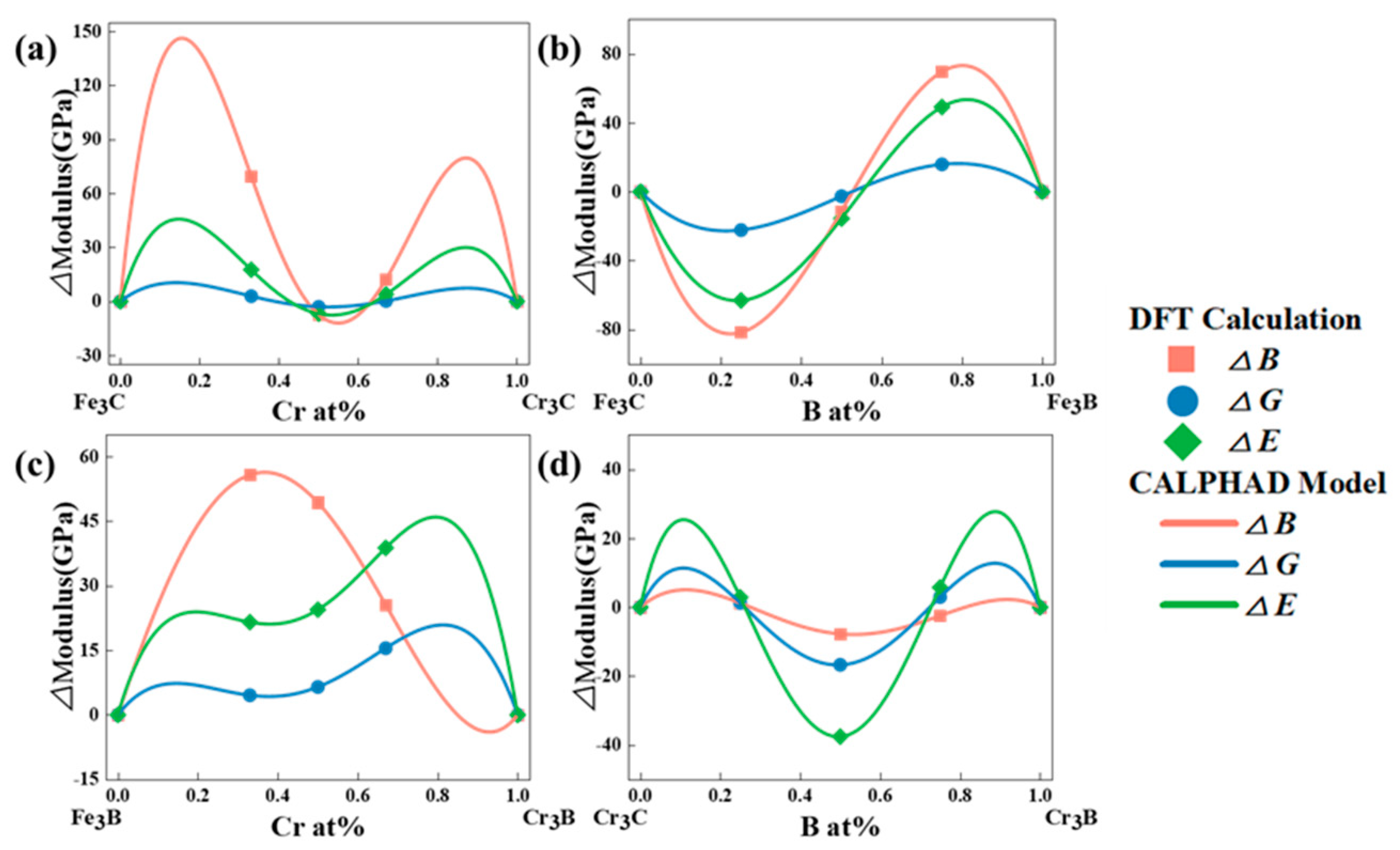
| Phases | B (GPa) | G (GPa) | E (GPa) | ν (GPa) | Hv (GPa) | Aᵁ | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Voigt | Reuss | Hill | Voigt | Reuss | Hill | |||||
| Fe3C | 321.6 | 319.0 | 320.3 | 147.6 | 129.3 | 138.5 | 363.2 | 0.311 | 11.643 | 0.71 |
| Fe3C a | 237 | 74 | ||||||||
| Fe3C b | 105 | 70 | ||||||||
| Fe3C c | 217 | 69 | ||||||||
| Fe3C d | 10.1 | |||||||||
| Fe3B | 199.9 | 198.1 | 199.0 | 126.7 | 119.2 | 123.0 | 305.9 | 0.244 | 16.064 | 0.32 |
| Fe3B e | 16.2 | |||||||||
| Cr3B | 261.9 | 259.3 | 260.6 | 149.4 | 149.4 | 145. | 367.2 | 0.265 | 16.037 | 0.01 |
| Cr3C | 276.2 | 273.1 | 274.6 | 164.2 | 155.5 | 159.9 | 401.7 | 0.256 | 18.076 | 0.29 |
| Cr3C f | 291.8 | 145.6 | ||||||||
| Cr12CB3 | 263.4 | 260.3 | 261.9 | 155.7 | 147.7 | 151.7 | 381.5 | 0.257 | 17.321 | 0.28 |
| Cr12C2B2 | 262.4 | 257.3 | 259.8 | 140.2 | 131.3 | 135.7 | 346.9 | 0.278 | 14.231 | 0.36 |
| Cr12C3B | 273.5 | 271.1 | 272.3 | 161.3 | 153.5 | 157.4 | 396.0 | 0.258 | 17.730 | 0.26 |
| Fe4Cr8C4 | 302.8 | 301.3 | 302.0 | 155.5 | 150.6 | 153.1 | 392.9 | 0.283 | 14.970 | 0.16 |
| Fe4Cr8C4 g | 297.1 | 139.8 | ||||||||
| Fe6Cr6C4 | 292.1 | 288.3 | 290.2 | 152.7 | 152.7 | 146.2 | 375.6 | 0.284 | 14.397 | 0.03 |
| Fe12CB3 | 299.9 | 298.0 | 299.0 | 146.8 | 138.6 | 142.7 | 369.6 | 0.294 | 13.310 | 0.30 |
| Fe12C2B2 | 209.0 | 207.8 | 208.4 | 130.6 | 125.6 | 128.1 | 319.0 | 0.245 | 14.437 | 0.20 |
| Fe12C3B | 208.6 | 207.9 | 208.3 | 114.9 | 109.8 | 112.4 | 285.8 | 0.271 | 12.913 | 0.23 |
| Fe3(C,B)-0.2B h | 11.18 ± 0.9 | |||||||||
| Fe8Cr4B4 | 275.9 | 274.2 | 275.1 | 138.3 | 131.3 | 134.8 | 347.7 | 0.289 | 13.171 | 0.27 |
| Fe6Cr6B4 | 280.2 | 278.1 | 279.1 | 146.8 | 134.1 | 140.5 | 361.0 | 0.284 | 13.980 | 0.48 |
| Fe4Cr8B4 | 265.9 | 265.7 | 265.8 | 161.6 | 144.9 | 153.3 | 385.7 | 0.258 | 17.352 | 0.57 |
| Fe8Cr4C4 | 375. | 374.32 | 374.89 | 163.58 | 133.44 | 148.5 | 393.5 | 0.325 | 11.070 | 1.13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.; Lin, Y.; Wang, G.; Jiang, Y.; Chong, X. Elastic Properties of Alloyed Cementite M3X (M = Fe, Cr; X = C, B) Phases from First-Principle Calculations and CALPHAD Model. Molecules 2024, 29, 1022. https://doi.org/10.3390/molecules29051022
Huang Y, Lin Y, Wang G, Jiang Y, Chong X. Elastic Properties of Alloyed Cementite M3X (M = Fe, Cr; X = C, B) Phases from First-Principle Calculations and CALPHAD Model. Molecules. 2024; 29(5):1022. https://doi.org/10.3390/molecules29051022
Chicago/Turabian StyleHuang, Yongxing, Yang Lin, Guangchi Wang, Yehua Jiang, and Xiaoyu Chong. 2024. "Elastic Properties of Alloyed Cementite M3X (M = Fe, Cr; X = C, B) Phases from First-Principle Calculations and CALPHAD Model" Molecules 29, no. 5: 1022. https://doi.org/10.3390/molecules29051022
APA StyleHuang, Y., Lin, Y., Wang, G., Jiang, Y., & Chong, X. (2024). Elastic Properties of Alloyed Cementite M3X (M = Fe, Cr; X = C, B) Phases from First-Principle Calculations and CALPHAD Model. Molecules, 29(5), 1022. https://doi.org/10.3390/molecules29051022







