Adsorption Properties and Wettability of Ethoxy- and Propoxy- Derivatives of 2-Ethylhexanol as Sterically Specific Surfactant Structures
Abstract
1. Introduction
2. Results and Discussion
2.1. Sterically Specific Surfactants
2.2. Adsorption Properties
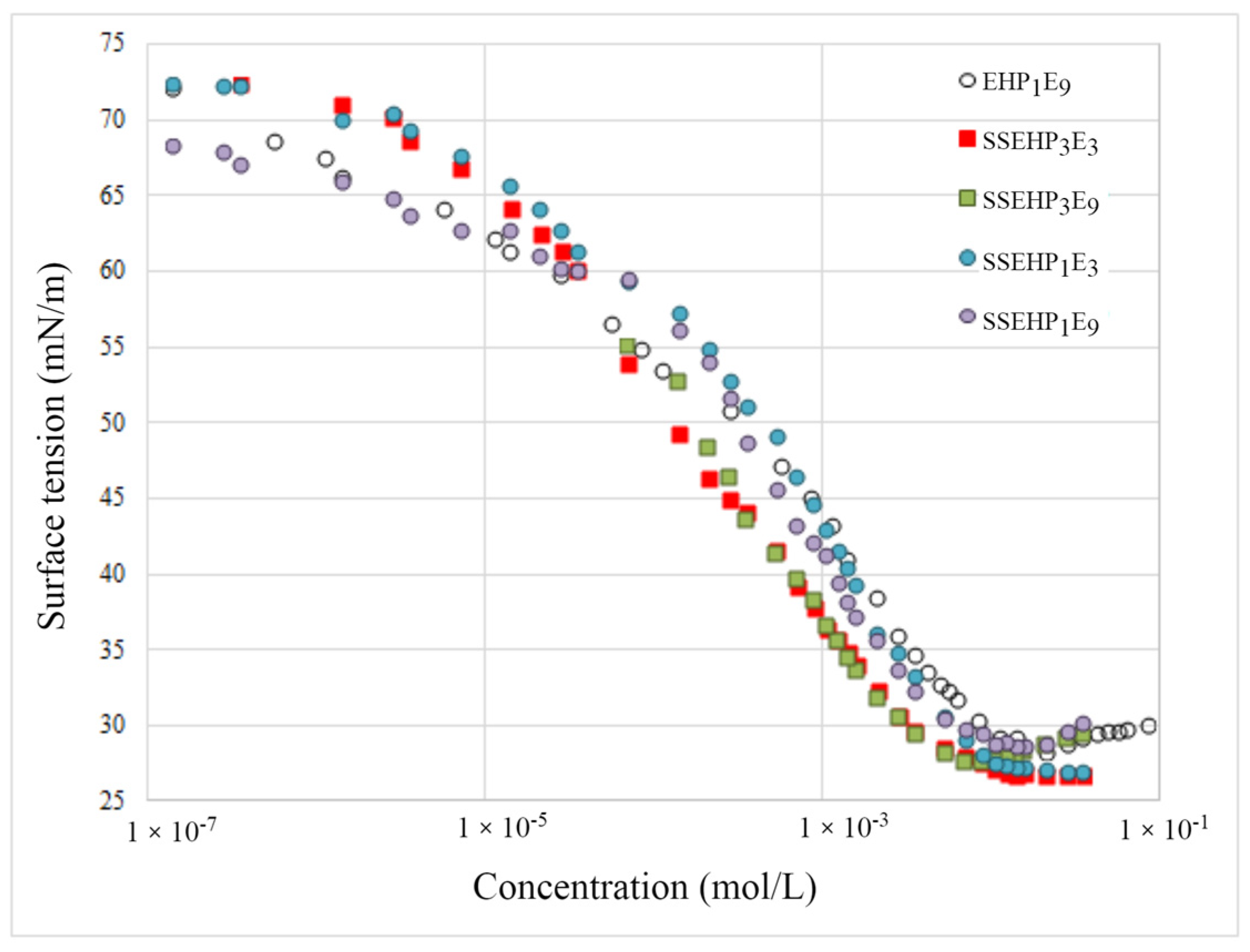
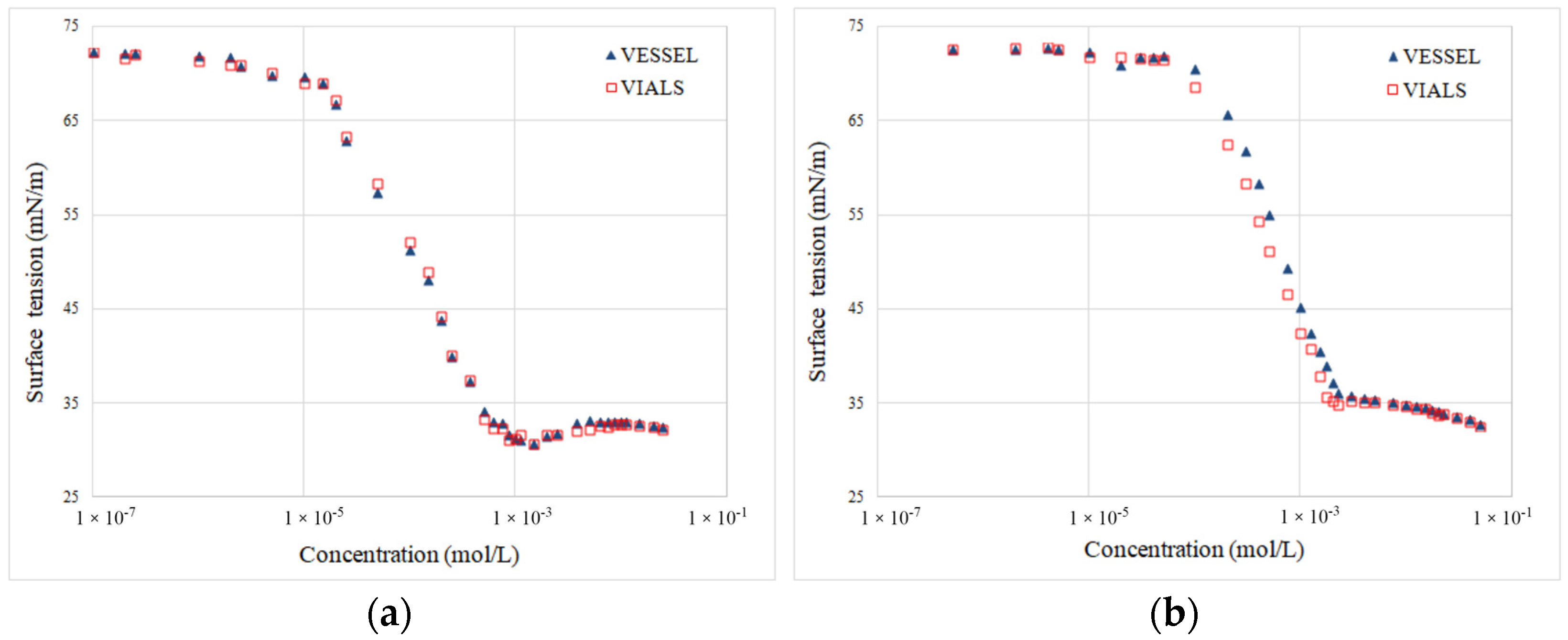
2.2.1. Equilibrium Surface Tension Isotherms
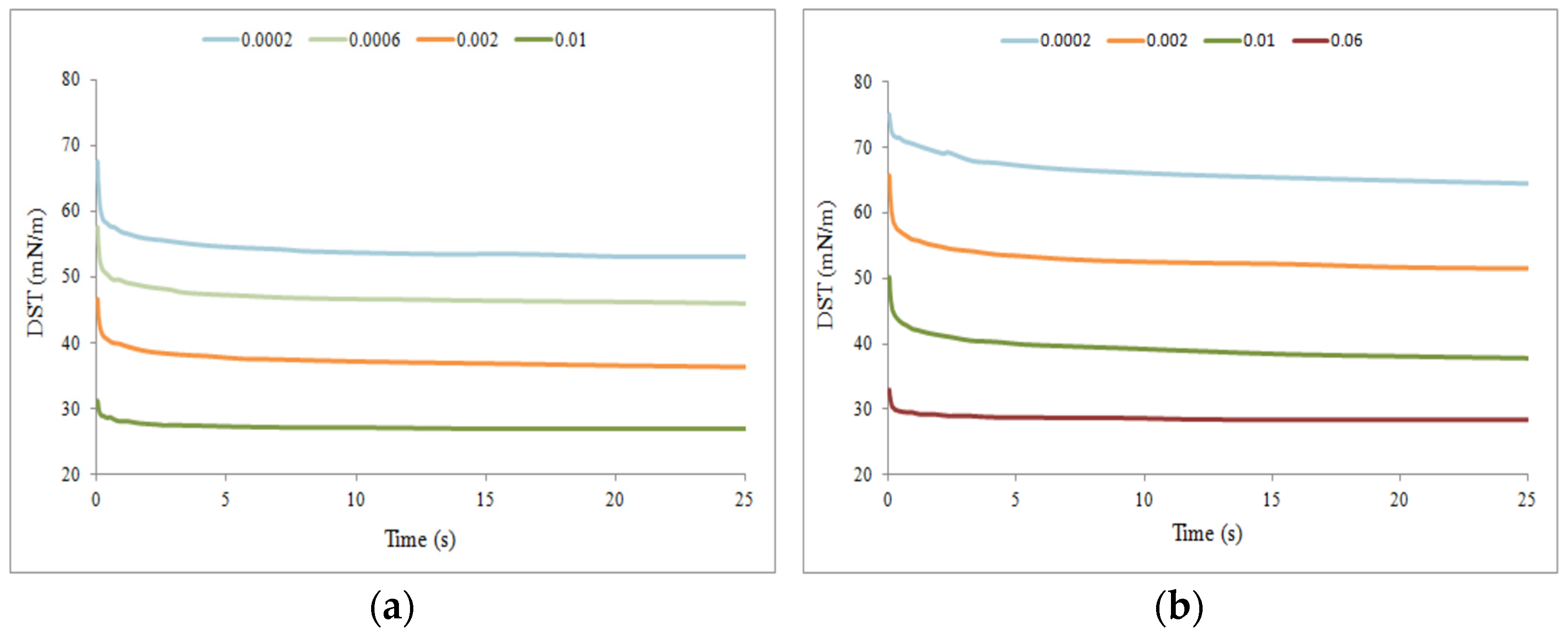
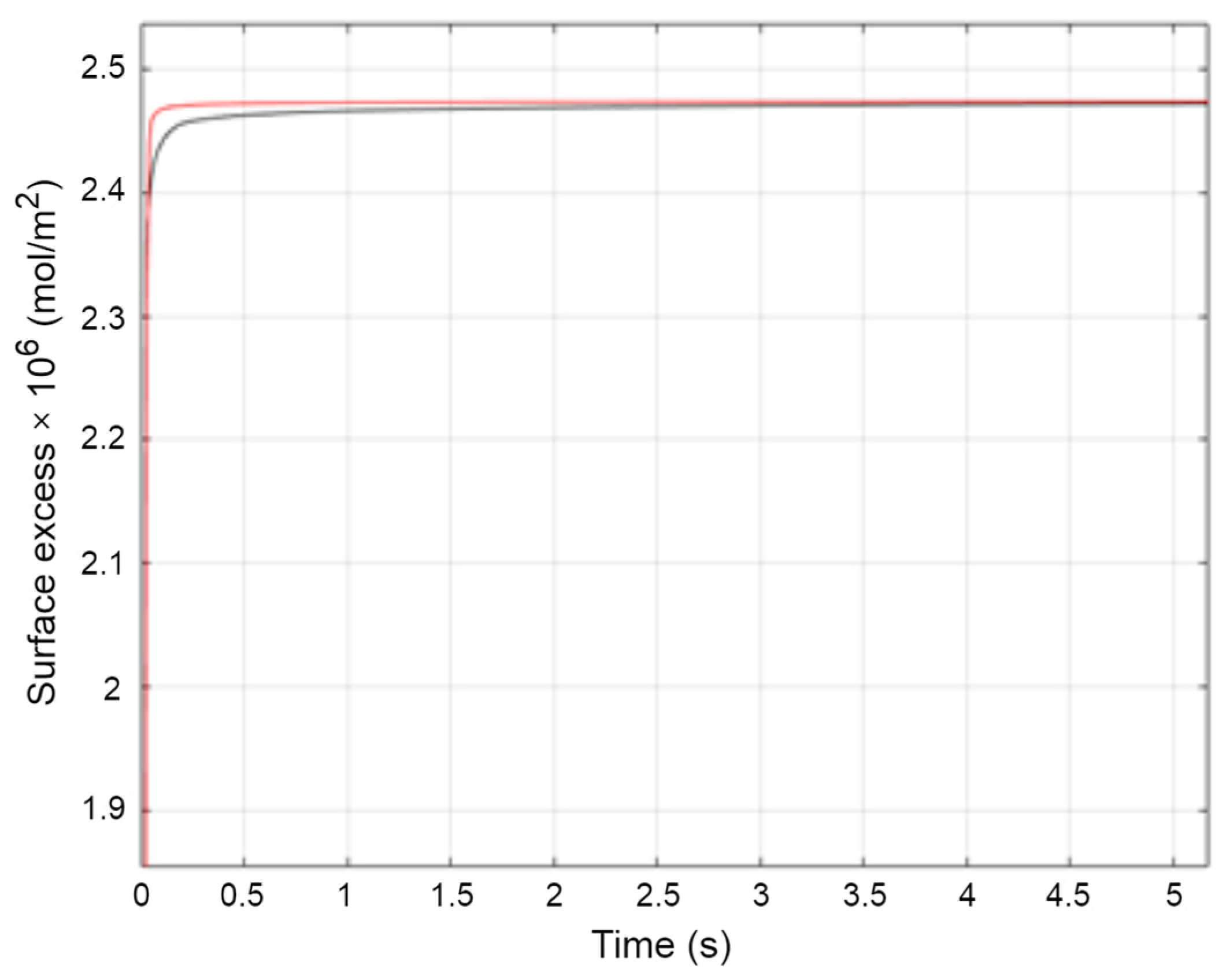
2.2.2. Study of Dynamics of Adsorption
Micelle Stability
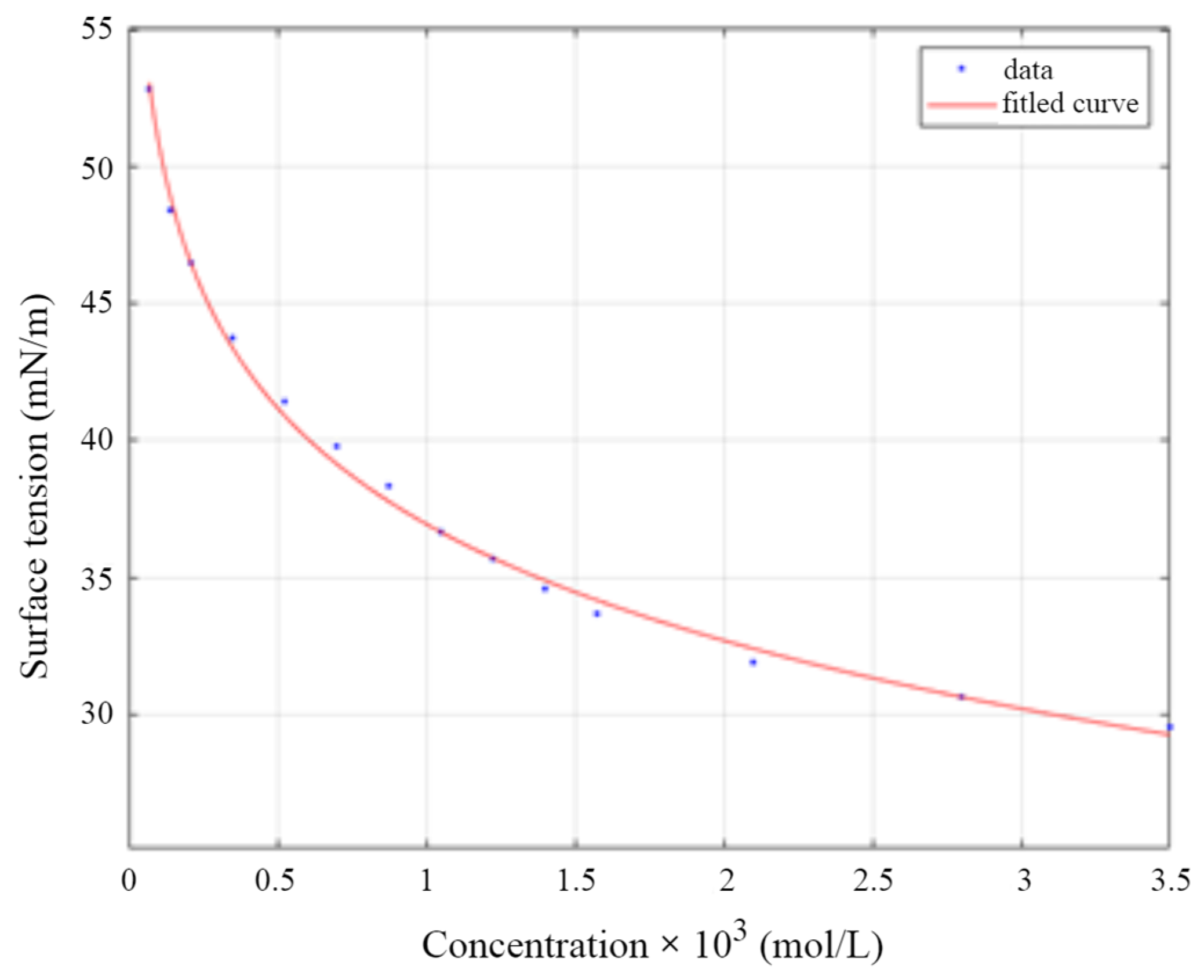
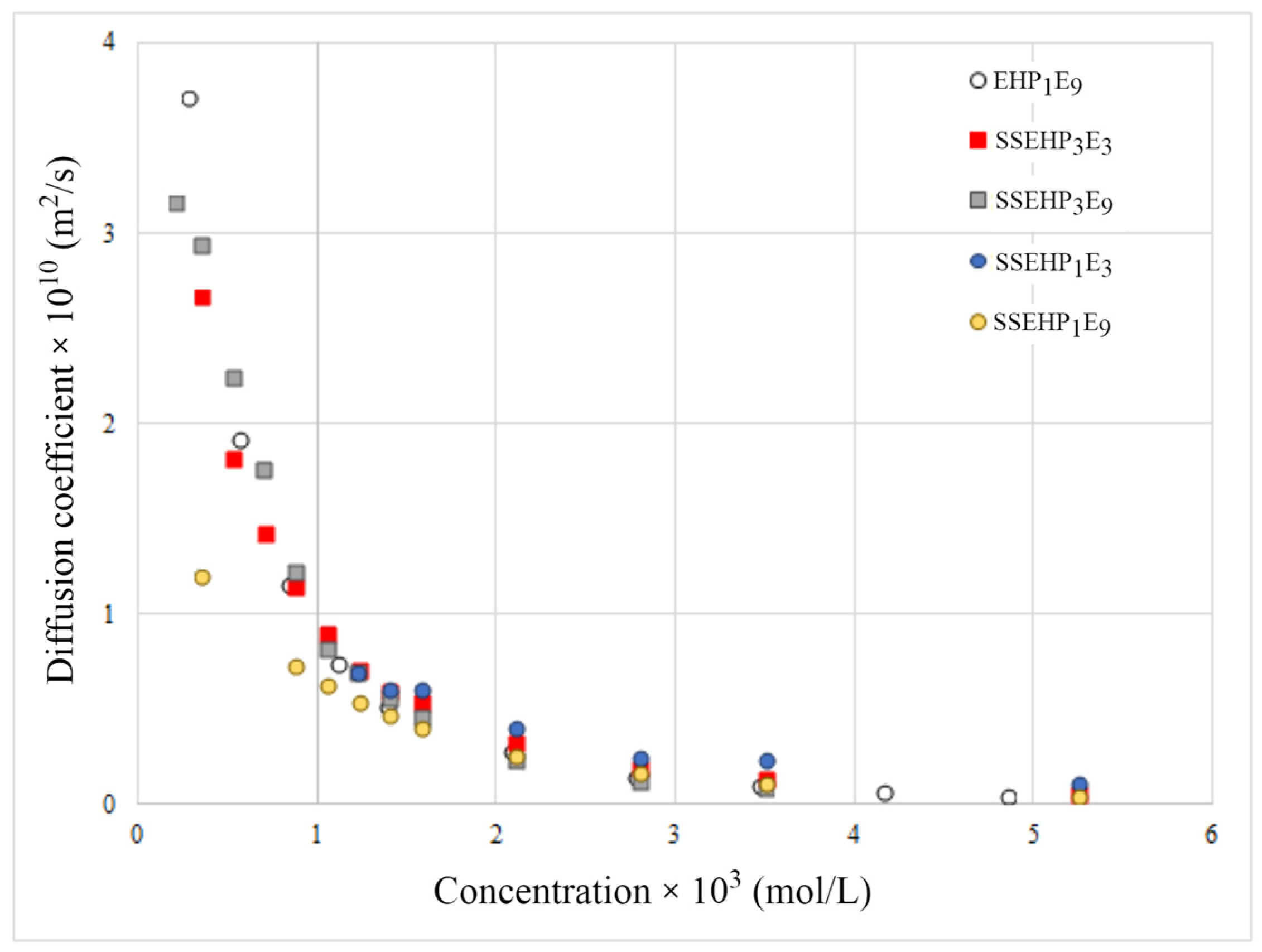
2.2.3. Diffusion Coefficients
2.2.4. Wettability Properties
3. Materials and Experimental Methods
3.1. Materials
- -
- 2-ethylhexanol of purity 99.4% m/m, manufacturer GA ZAK Kędzierzyn-Koźle; before alkoxylation, the alcohol was dehydrated under nitrogen purge to a water content of 0.03% m/m.
- -
- DMC catalyst, manufacturer MEXEO company, Kędzierzyn-Koźle, Poland.
- -
- Maleic anhydride of purity 99.8% m/m, GA ZAK Kędzierzyn-Koźle, Poland.
- -
- Sodium sulfite anhydrous with a purity of 94% m/m, from CHEMPUR, Piekary Śląskie, Poland.
3.2. Method of Synthesis
3.2.1. Description of the Synthesis of the Studied Surfactants
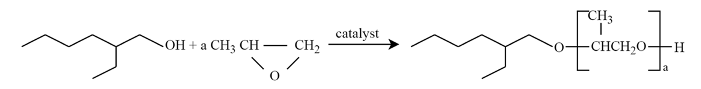



3.2.2. Chromatographic Analysis
- ▪
- Shodex GPC KF-802.5, 8 × 300 mm column (number of theoretical shelves ≥ 18,000; filling grain diameter 6μm; molecular weight range 300–8000 Da);
- ▪
- Shodex GPC KF-801, 8 × 300 mm column (number of theoretical shelves ≥ 18,000; filling grain diameter 6μm; molecular weight range 100–700 Da).
- Additionally:
- Pre-column KF-G4A, Chromeleon 7.2. + GPC/SEC software, and the mobile phase Tetrahydrofuran, BAKER ANALYZED HPLC, J.T. Baker, were applied;
- The sample solvent was THF, as above.
- Calibration procedure: The molecular weight calibration was performed using the internal standard method based on the identified peaks of individual homologs of 2-ethylhexanol ethoxylates, whose GPC signals showed high quality in terms of resolution and thus detection and quantitative measurement of the surface area.
3.3. Experimental Methods and Calculations of Physicochemical Parameters
3.3.1. Surface Tension
3.3.2. Measurement of Wettability
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hreczuch, W.; Dąbrowska, K.; Chruściel, A.; Sznajdrowska, A.; Materna, K. 2-Ethylhexanol Derivatives as Nonionic Surfactants: Synthesis and Properties. J. Surf. Deterg. 2016, 19, 155–164. [Google Scholar] [CrossRef] [PubMed]
- Hreczuch, W.; Dąbrowska, K.; Chruściel, A.; Materna, K.; Sznajdrowska, A.; Czaja, K.; Wang, W.; Qiuxiao, L.; Yongqiang, S. Investigation of Propoxylation and Ethoxylation of 2-Ethylhexanol in the Presence of an Alkaline and DMC Type Catalyst at Initial Stages of The Synteses. China Deterg. Cosmet. 2016, 1, 49–58. [Google Scholar]
- Hreczuch, W.; Chruściel, A.; Dąbrowska, K.; Di Serio, M.; Yongqiang, S. Characteristics of Block Copolymers of Methyl Oxirane and Oxirane Derivatives of 2-Ethylhexanol as Obtained with KOH and Dimetalcyanide Type Catalyst. Tens. Surf. Deterg. 2016, 53, 259–264. [Google Scholar] [CrossRef]
- Chruściel, A.; Hreczuch, W.; Dąbrowska, K.; Materna, K.; Sznajdrowska, A. Interfacial activity of 2-etylheksanol based surfactants in quasi-ternary systems. J. Surf. Deterg. 2017, 20, 83–101. [Google Scholar] [CrossRef]
- Fiutkowska, A.; Piontek, W.; Janik, J.; Materna, K. Aktywność powierzchniowa i właściwości zwilżające polioksyalkilenowanych pochodnych 2-etyloheksanolu. Przem. Chem. 2018, 97, 2010–2013. [Google Scholar] [CrossRef]
- Jańczuk, B.; Zdziennicka, A.; Szymczyk, K.; González-Martín, M.L. Prediction of Aqueous Solution Surface Tension of Some Surfactant Mixtures and Composition of Their Monolayers at the Solution-Air Interface. Coll. Interfaces 2021, 5, 53. [Google Scholar] [CrossRef]
- Seddon, D.; Müller, E.A.; Cabral, J.T. Machine learning hybrid approach for the prediction of surface tension profiles of hydrocarbon surfactants in aqueous solution. J. Coll. Interface Sci. 2022, 625, 328–339. [Google Scholar] [CrossRef] [PubMed]
- Mabrouk, M.M.; Hamed, N.A.; Mansour, F.R. Physicochemical and electrochemical methods for determination of critical micelle concentrations of surfactants: A comprehensive review. Monatsh. Chem. 2022, 153, 125–138. [Google Scholar] [CrossRef]
- Bergfreund, J.; Siegenthaler, S.; Lutz-Bueno, V.; Bertsch, P.; Fischer, P. Surfactant Adsorption to Different Fluid Interfaces. Langmuir 2021, 37, 6722–6727. [Google Scholar] [CrossRef]
- Bratovcic, A.; Nazdrajic, S.; Odobasic, A.; Sestan, I. The Influence of Type of Surfactant on Physicochemical Properties of Liquid Soap. Int. J. Mater. Chem. 2018, 8, 31–37. [Google Scholar]
- Rosen, M.J.; Kunjappu, J.T. Surfactants and Interfacial Phenomena; John Wiley & Sons Ltd.: New York, NY, USA, 2012. [Google Scholar]
- Gaudin, T.; Rotureau, P.; Pezron, I.; Fayet, G. Estimating the Adsorption Efficiency of Sugar-Based Surfactants from QSPR Models. Int. J. Quant. Struct. Prop. Relatsh. 2019, 4, 28–51. [Google Scholar] [CrossRef]
- Kumar, A.; Banjare, M.K.; Sinha, S.; Yadav, T.; Sahu, R.; Satnami, M.L.; Ghosh, K.K. Imidazolium-Based Ionic Liquid as Modulator of Physicochemical Properties of Cationic, Anionic, Nonionic, and Gemini Surfactants. J. Surfactants Deterg. 2018, 21, 355–366. [Google Scholar] [CrossRef]
- El-Dossoki, F.I.; Gomaa, E.A.; Hamza, O.K. Solvation thermodynamic parameters for sodium dodecyl sulfate (SDS) and sodium lauryl ether sulfate (SLES) surfactants in aqueous and alcoholic-aqueous solvents. SN Appl. Sci. 2019, 1, 933. [Google Scholar] [CrossRef]
- Al Sabagh, A.M.; Abdel-Hamid, T.M.; Abdel-Salam, F.H.; Noor El-Din, M.R.; Mohamed, A. Surface activity and thermodynamic properties of some green surfactants from wastes in formation water at reservoir conditions. J. Dispers. Sci. Technol. 2020, 43, 385–398. [Google Scholar] [CrossRef]
- Malik, N.A.; Farooq, U. Effect of caffeine on the micellization and related thermodynamic parameters of sodium dodecyl sulphate, hexadecyltrimethylammonium bromide and triton x-100: A physicochemical study. Phys. Chem. Liq. 2021, 60, 265–274. [Google Scholar] [CrossRef]
- Goddard, E.D. Interactions of Surfactants with Polymers and Proteins; CRC Press: Boca Raton, FL, USA, 1993; p. 32. [Google Scholar]
- Parshad, B.; Prasad, S.; Bhatia, S.; Mittal, A.; Pan, Y. Non-ionic small amphiphile based nanostructures for biomedical applications. RSC Adv. 2020, 10, 42098. [Google Scholar] [CrossRef]
- Holmberg, K.; Jönsson, B.; Kronberg, B.; Lindman, B. Surfactants and Polymers in Aqueous Solution; John Wiley & Sons: London, UK, 2002. [Google Scholar]
- Qazi, M.J.; Schlegel, S.J.; Backus, E.H.G.; Bonn, M.; Bonn, D.; Shahidzadeh, N. Dynamic surface tension of surfactants in the presence of high salt concentrations. Langmuir 2020, 36, 7956–7964. [Google Scholar] [CrossRef] [PubMed]
- Tian, C.; Feng, J.; Prud’homme, R.K. Adsorption dynamics of polymeric nanoparticles at an air-water interface with addition of surfactants. J. Colloid Interface Sci. 2020, 575, 416–424. [Google Scholar] [CrossRef]
- Tripathi, S.; Tan, S.N.; Bhattacharya, A.; Tabor, R.F. Measuring and modelling the adsorption kinetics of polydisperse PiBSA-based emulsifiers using dynamic interfacial tension measurements. Colloids Surf. A Physicochem. Eng. Asp. 2021, 624, 126728. [Google Scholar] [CrossRef]
- Kalli, M.; Chagot, L.; Angeli, P. Comparison of surfactant mass transfer with drop formation times from dynamic interfacial tension measurements in microchannels. J. Colloid Interface Sci. 2022, 605, 204–213. [Google Scholar] [CrossRef]
- Shen, A.Q.; Gleason, B.; McKinley, G.H.; Stone, H.A. Fiber coating with surfactant solutions. Phys. Fluids 2002, 14, 4055–4068. [Google Scholar] [CrossRef]
- Patist, A.; Kanicky, J.R.; Shukla, P.K.; Shah, D.O. Importance of Micellar Kinetics in Relation to Technological Processes. J. Colloid Interface Sci. 2002, 245, 1–15. [Google Scholar] [CrossRef]
- Chattoraj, D.K.; Birdi, K.S. Adsorption and the Gibbs Surface Excess; Plenum Press: New York, NY, USA; London, UK, 1984. [Google Scholar]
- Martínez-Balbuenaa, L.; Arteaga-Jiménezb, A.; Hernández-Zapatac, E.; Márquez-Beltrán, C. Applicability of the Gibbs Adsorption Isotherm to the analysis of experimental surface-tension data for ionic and nonionic surfactants. Adv. Colloid Interface Sci. 2017, 247, 178–184. [Google Scholar] [CrossRef]
- Crank, J. The Mathematics of Diffusion; Clarendon Press: Oxford, UK, 1975. [Google Scholar]
- Fainermana, V.B.; Kazakova, V.N.; Lylyka, S.V.; Makievskib, A.V.; Miller, R. Dynamic surface tension measurements of surfactant solutions using the maximum bubble pressure method—Limits of applicability. Colloids Surf. A Physicochem. Eng. Asp. 2004, 250, 97–102. [Google Scholar] [CrossRef]
- Hines, A.; Maddox, R. Mass Transfer, Fundamentals and Applications; Prentice Hall PTR: New Jersey, NJ, USA, 1985. [Google Scholar]
- Bird, R.; Stewart, W.; Lightfood, E. Transport Phenomena; John Wiley and Sons: New York, NY, USA, 2007. [Google Scholar]
- Ward, A.F.H.; Tordai, L. Time-dependence of boundary tensions of solutions I. The role of diffusion in time-effects. J. Chem. Phys. 1946, 14, 453–461. [Google Scholar] [CrossRef]
- Mucic, N.; Javadi, A.; Karbaschi, M.; Sharipova, A.; Fainerman, V.B.; Aksenenko, E.V.; Kovalchuk, N.M.; Miller, R. Surfactant Adsorption Kinetics. In Encyclopedia of Colloid and Interface Science; Tadros, T., Ed.; Springer-Verlag: Berlin/Heidelberg, Germany, 2013; pp. 1090–1125. [Google Scholar]
- Sutherland, K.L. The kinetics of adsorption at liquidsurfaces. Aust. J. Chem. 1952, 5, 683–696. [Google Scholar] [CrossRef]
- Scher, Y.; Bonomo, O.L.; Pal, A.; Reuveni, S. Microscopic Theory of Adsorption Kinetics. J. Chem. Phys. 2023, 158, 094107. [Google Scholar] [CrossRef] [PubMed]
- Mohammadi, M.; Zirrahi, M.; Hassanzadeh, H. An Analytical Model for Estimation of the Self-Diffusion Coefficient and Adsorption Kinetics of Surfactants Using Dynamic Interfacial Tension Measurements. J. Phys. Chem. B 2020, 124, 3206–3213. [Google Scholar] [CrossRef] [PubMed]
- Miller, R.; Aksenenko, E.V.; Fainerman, V.B. Dynamic interfacial tension of surfactant solutions. Adv. Colloid Interface Sci. 2017, 247, 115–129. [Google Scholar] [CrossRef] [PubMed]
- Delves, L.M.; Mohamed, J.L. Computational Methods for Integral Equations; Cambridge University Press: New York, NY, USA, 1992. [Google Scholar]
- Nele, M. An analytically solvable numerical approximation to Ward-Tordai equation applied to Langmuir adsorption. Colloids Surf. A Physicochem. Eng. Asp. 2022, 648, 129186. [Google Scholar] [CrossRef]
- Atkinson, K. The Numerical Solution of Integral Equations of the Second Kind; Cambridge University Press: New York, NY, USA, 1997. [Google Scholar]
- Chruściel, A.; Hreczuch, W.; Janik, J.; Czaja, K.; Dziubek, K.; Flisak, Z.; Swinarew, A. Charakterization of a double metal cyanide (DMC)—Type catalyst in the polyoxypropylation process: Effects of catalyst concentration. Ind. Eng. Chem. Res. 2014, 53, 6636–6643. [Google Scholar] [CrossRef]
- Prochaska, K.; Konował, E.; Sulej-Chojnacka, J.; Lewandowicz, G. Physicochemical properties of cross-linked and acetylated starches and products of their hydrolysis in continuous recycle membrane reactor. Colloids Surf. B Biointerfaces 2009, 74, 238–243. [Google Scholar] [CrossRef] [PubMed]
- Available online: http://www.thomascat.info/thomascat/Scientific/AdSo/AdSo.htm (accessed on 1 December 2019).
- Frese, C.; Ruppert, S.; Schmidt-Lewerkühne, H.; Wittern, K.P.; Eggers, R.; Fainerman, V.B.; Miller, R. Adsorption dynamics of micellar solutions of a mixed anionic–cationic surfactant system. Colloids Surf. A Physicochem. Eng. Asp. 2004, 239, 33–40. [Google Scholar] [CrossRef]

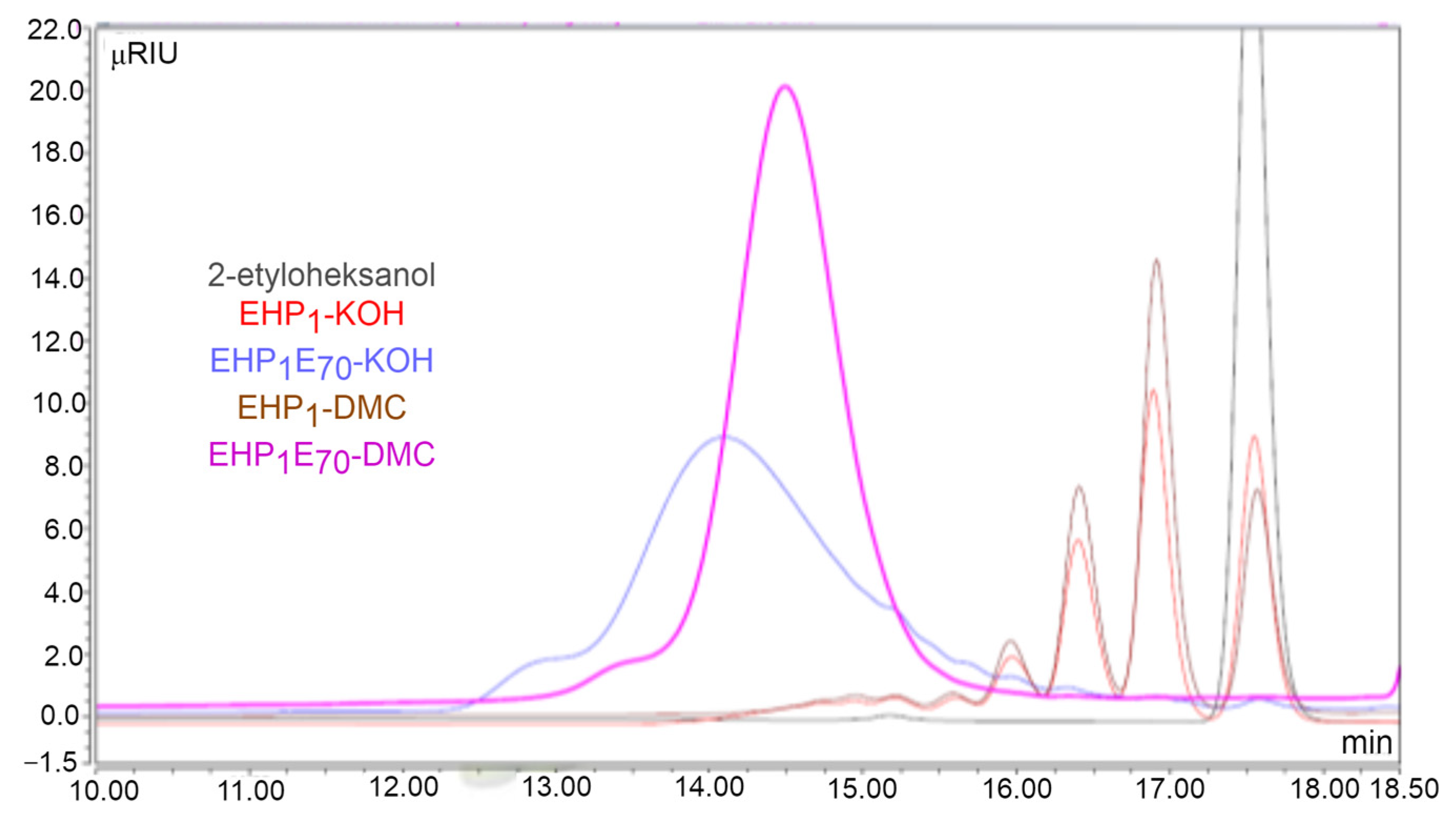
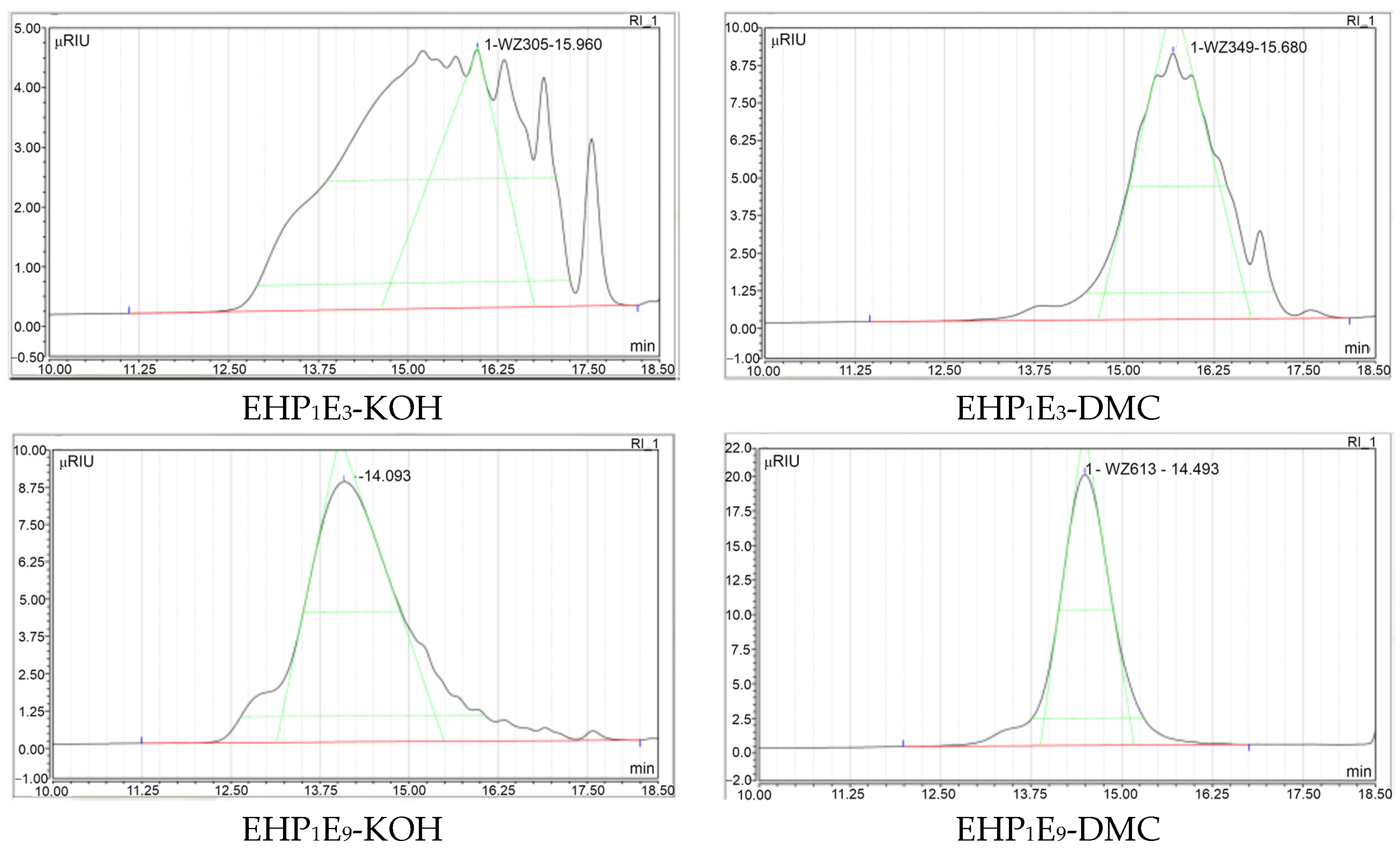





| Analyzed Surfactant, Average Molar Ratio/ Catalyst Type | EH-P1E3-/KOH | EH-P1E3-/DMC | EH-P1E9-/KOH | EH-P1E9-/DMC |
|---|---|---|---|---|
| Mw—weight balance | 320 Da | 320 Da | 584 Da | 584 Da |
| Analyzed surfactant, synthesis mass balance | EH = 40.6% P1 = 18.1% E3 = 41.3% | EH = 22.3% P1 = 9.9% E9 = 67.8% | ||
| Mw at 1/2 peak height—start (Retention time) | 822 Da (13.87 min) | 487 Da (15.06 min) | 956 Da (13.50 min) | 740 Da (14.12 min) |
| Mw at 1/2 peak height—end (Retention time) | 180 Da (17.05 min) | 249 Da (16.44 min) | 541 Da (14.83 min) | 532 Da (14.87 min) |
| Polydispersity, MWD | 1.34 | 1.12 | 1.16 | 1.05 |
| Surfactant | CMC | pC20 | ΠCMC | C | k2 | τ2 |
|---|---|---|---|---|---|---|
| (mmol/L) | (—) | (mN/m) | (~1CMC) | (s−1) | (ms) | |
| EHP1E9 | 9.40 | 3.96 | 41.24 | 11.08 | 0.58 | 1736.20 |
| SSEHP1E9 | 4.81 | 3.55 | 41.11 | 5.25 | 0.84 | 1191.19 |
| SSEHP1E3 | 5.62 | 3.55 | 41.94 | 8.75 | 0.50 | 2000.19 |
| SSEHP3E9 | 3.40 | 3.85 | 43.16 | 5.25 | 0.91 | 1095.96 |
| SSEHP3E3 | 4.39 | 3.85 | 42.92 | 5.25 | 1.66 | 602.19 |
| Triton X100 | 0.34 | 4.70 | 42.40 | 0.40 | 1.61 | 622.64 |
| SDS | 8.09 | 2.60 | 35.17 | 8.50 | 2.99 | 334.33 |
| ABSNa | 2.00 | 3.30 | 37.64 | 3.00 | 1.24 | 803.90 |
| SLES25 | 0.75 | 4.00 | 39.97 | 0.80 | 2.55 | 392.70 |
| Surfactant | Asz∙106 (L/mol) | Bsz (-) | −ΔGads (kJ/mol) | Γ∞ × 106 (mol/m2) | Amin (nm2) |
|---|---|---|---|---|---|
| ABSNa | 2.43 × 10−4 | 0.24 | 20.34 | 7.17 | 0.23 |
| SLES25 | 2.71 × 10−5 | 0.17 | 25.84 | 5.12 | 0.32 |
| EHP1E9 | 7.53 × 10−7 | 0.06 | 34.46 | 1.88 | 0.88 |
| SSEHP1E9 | 2.94 × 10−6 | 0.07 | 31.13 | 2.18 | 0.76 |
| SSEHP3E3 | 5.41 × 10−6 | 0.09 | 29.64 | 2.78 | 0.59 |
| SSEHP1E3 | 1.14 × 10−5 | 0.09 | 27.83 | 2.72 | 0.61 |
| Surfactant Symbol | Description |
|---|---|
| Nonionic and anionic, a-propoxy, n-ethoxy 2-EH—surfactant: | |
| EHP1E9 | Mono-propoxylated 2-ethylhexanol polyethoxylate of average addition degree n = 9, DMC catalyst |
| Anionic, sulfosuccinic surfactants: | |
| SSEHP1E9 | Mono-propoxylated 2-ethylhexanol polyethoxylate of average addition degree n = 9, DMC catalyst, sulfosuccinate sodium salt |
| SSEHP1E3 | Mono-propoxylated 2-ethylhexanol polyethoxylate of average addition degree n = 3, DMC catalyst, sulfosuccinate sodium salt |
| SSEHP3E9 | Three-propoxylated 2-ethylhexanol polyethoxylate of average addition degree n = 9, DMC catalyst, sulfosuccinete sodium salt |
| SSEHP3E3 | Three-propoxylated 2-ethylhexanol polyethoxylate of average addition degree n = 3, DMC catalyst, sulfosuccinete sodium salt |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hreczuch, W.; Konopczyńska, B.; Stasiak, M.; Andrzejewski, A.; Prochaska, K. Adsorption Properties and Wettability of Ethoxy- and Propoxy- Derivatives of 2-Ethylhexanol as Sterically Specific Surfactant Structures. Molecules 2024, 29, 690. https://doi.org/10.3390/molecules29030690
Hreczuch W, Konopczyńska B, Stasiak M, Andrzejewski A, Prochaska K. Adsorption Properties and Wettability of Ethoxy- and Propoxy- Derivatives of 2-Ethylhexanol as Sterically Specific Surfactant Structures. Molecules. 2024; 29(3):690. https://doi.org/10.3390/molecules29030690
Chicago/Turabian StyleHreczuch, Wiesław, Beata Konopczyńska, Marcin Stasiak, Adam Andrzejewski, and Krystyna Prochaska. 2024. "Adsorption Properties and Wettability of Ethoxy- and Propoxy- Derivatives of 2-Ethylhexanol as Sterically Specific Surfactant Structures" Molecules 29, no. 3: 690. https://doi.org/10.3390/molecules29030690
APA StyleHreczuch, W., Konopczyńska, B., Stasiak, M., Andrzejewski, A., & Prochaska, K. (2024). Adsorption Properties and Wettability of Ethoxy- and Propoxy- Derivatives of 2-Ethylhexanol as Sterically Specific Surfactant Structures. Molecules, 29(3), 690. https://doi.org/10.3390/molecules29030690







