Molecular Dynamics Simulation Study on the Occurrence of Shale Oil in Hybrid Nanopores
Abstract
1. Introduction
2. Models and Methods
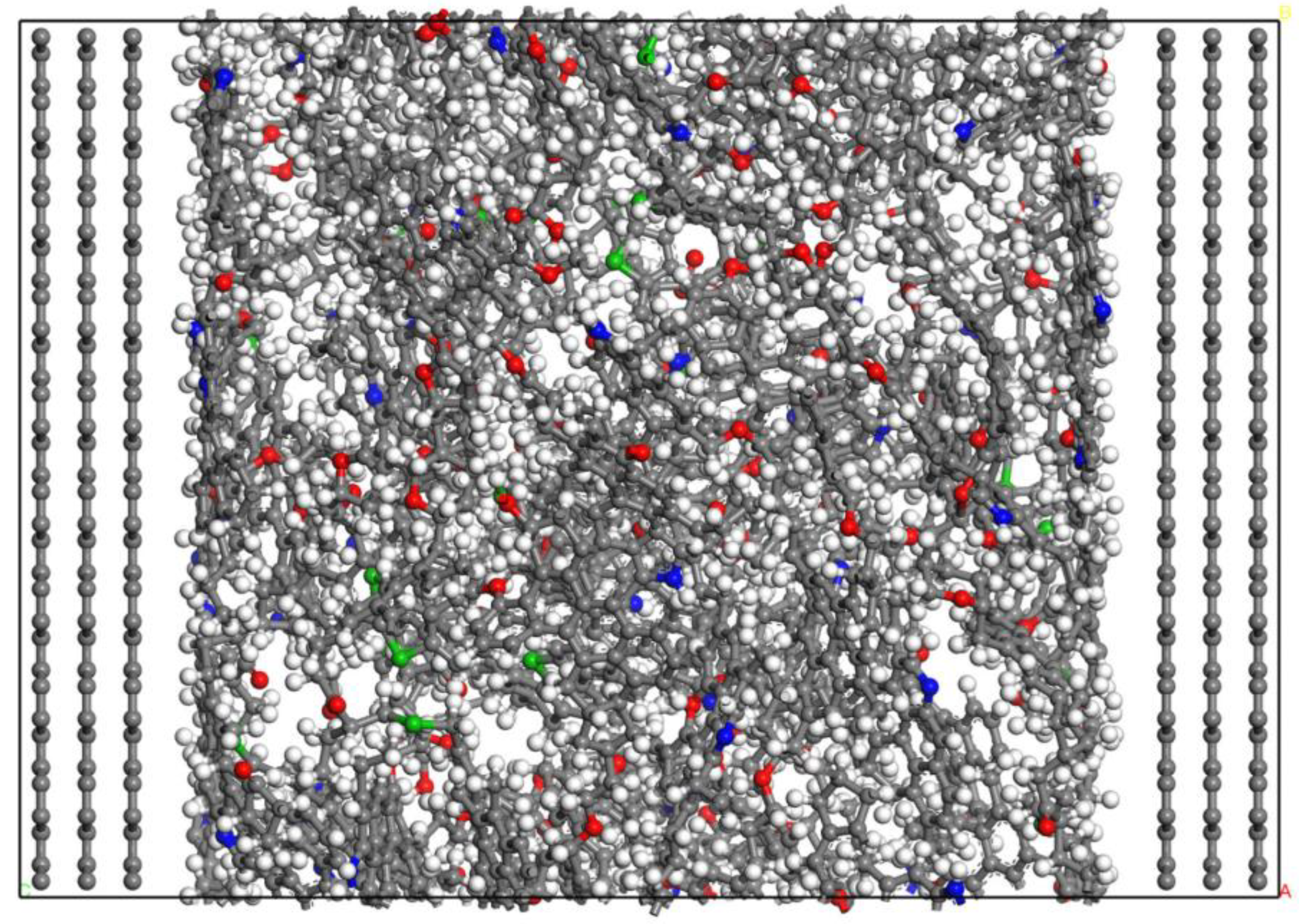
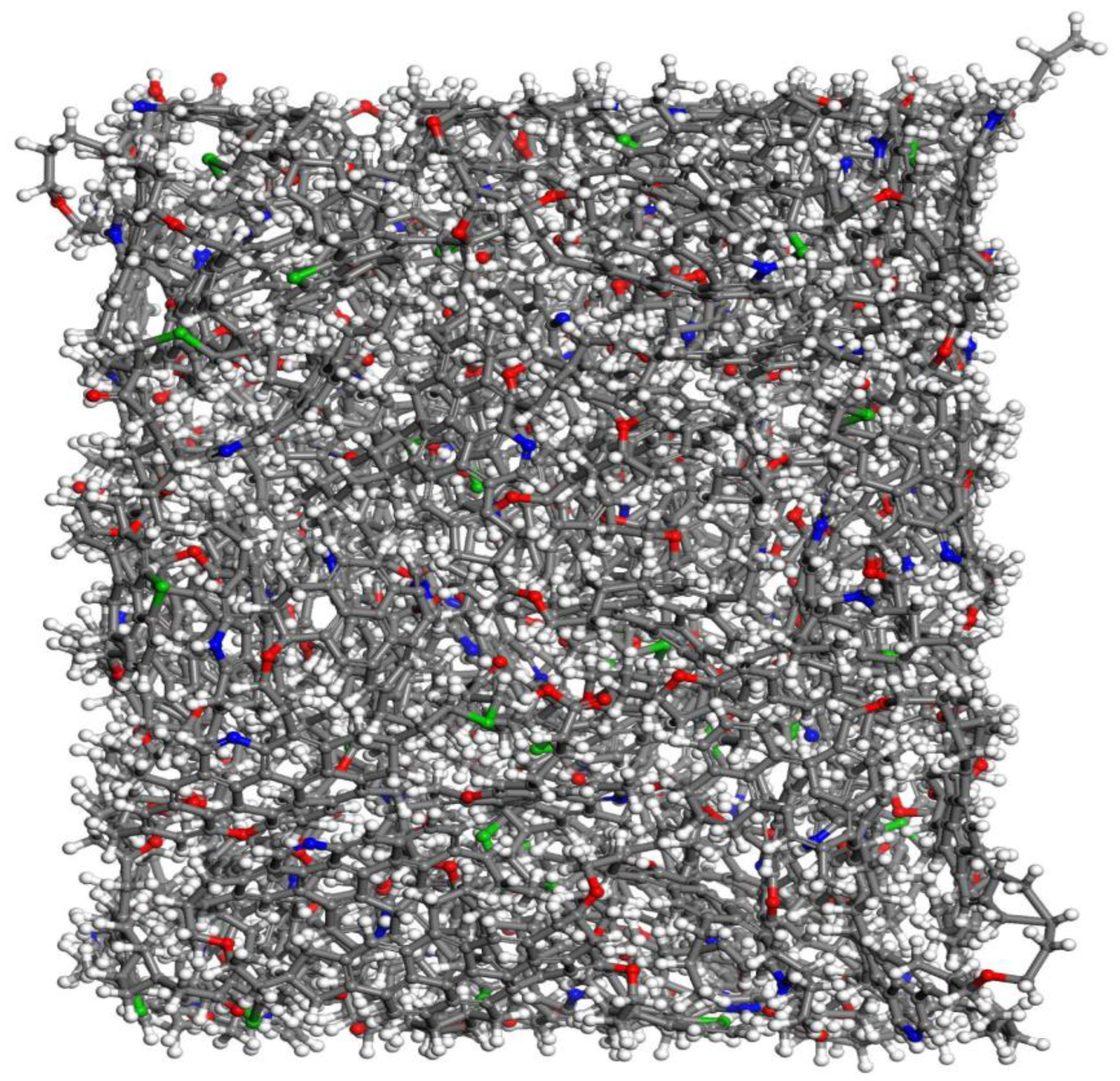
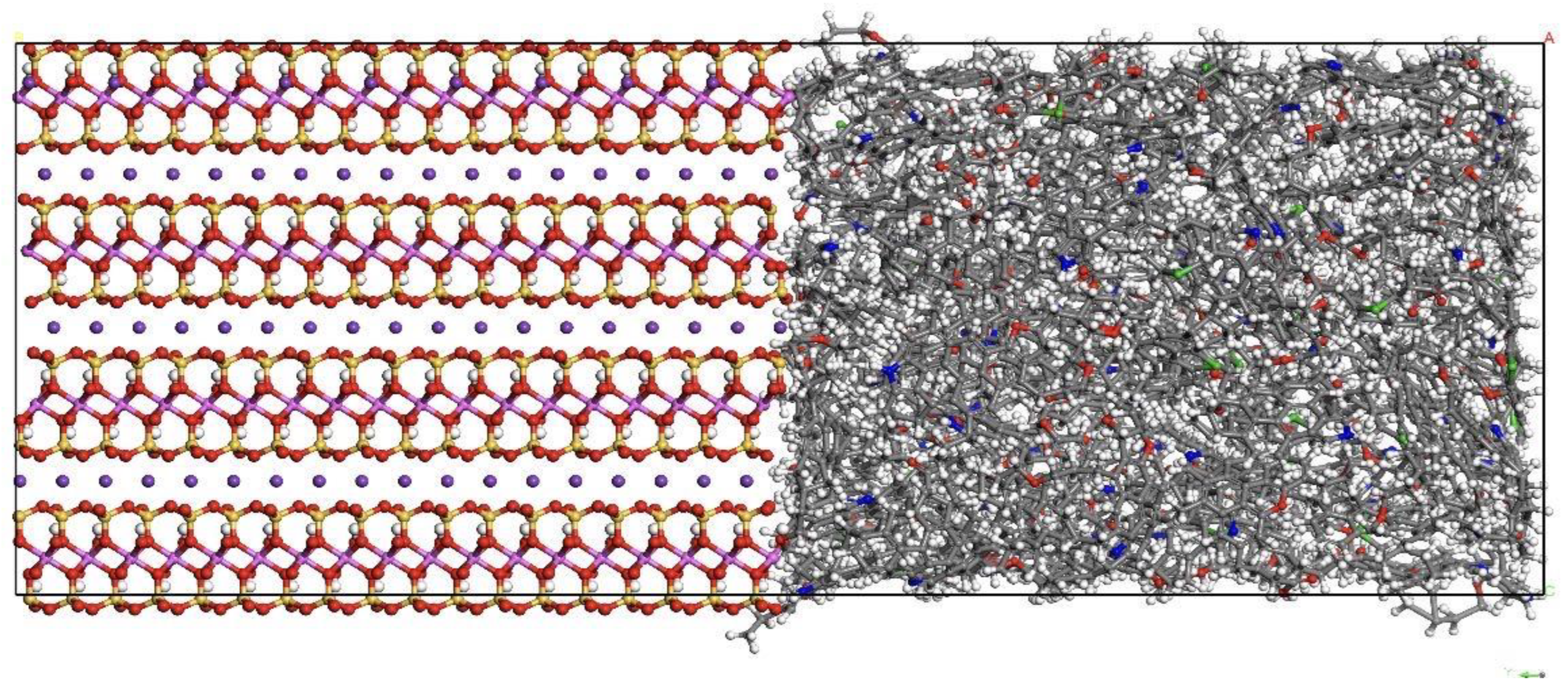
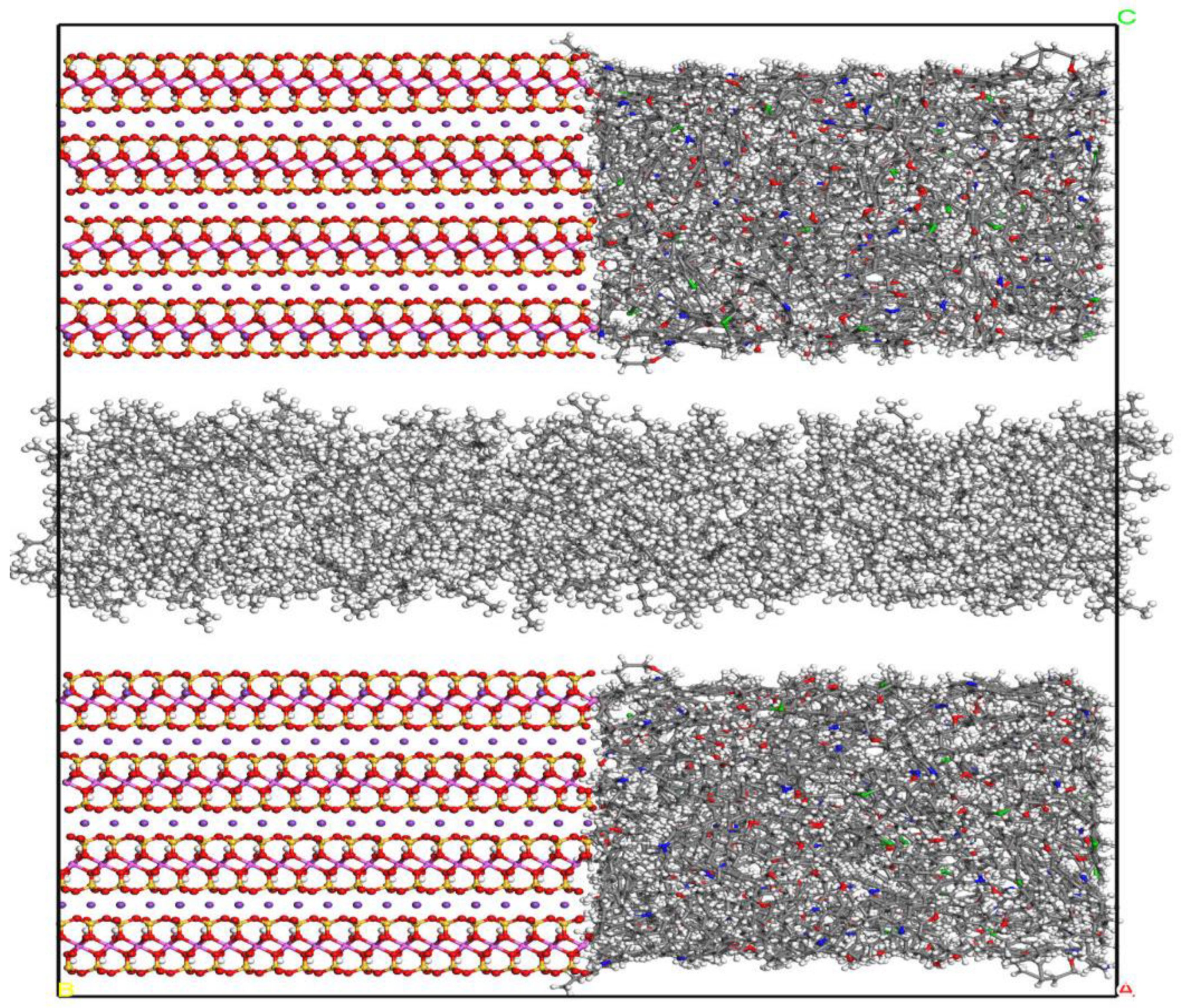
2.1. Fluid Model under Composite Pores
2.2. Simulation Method
3. Occurrence Law of Shale Oil in Composite Pores
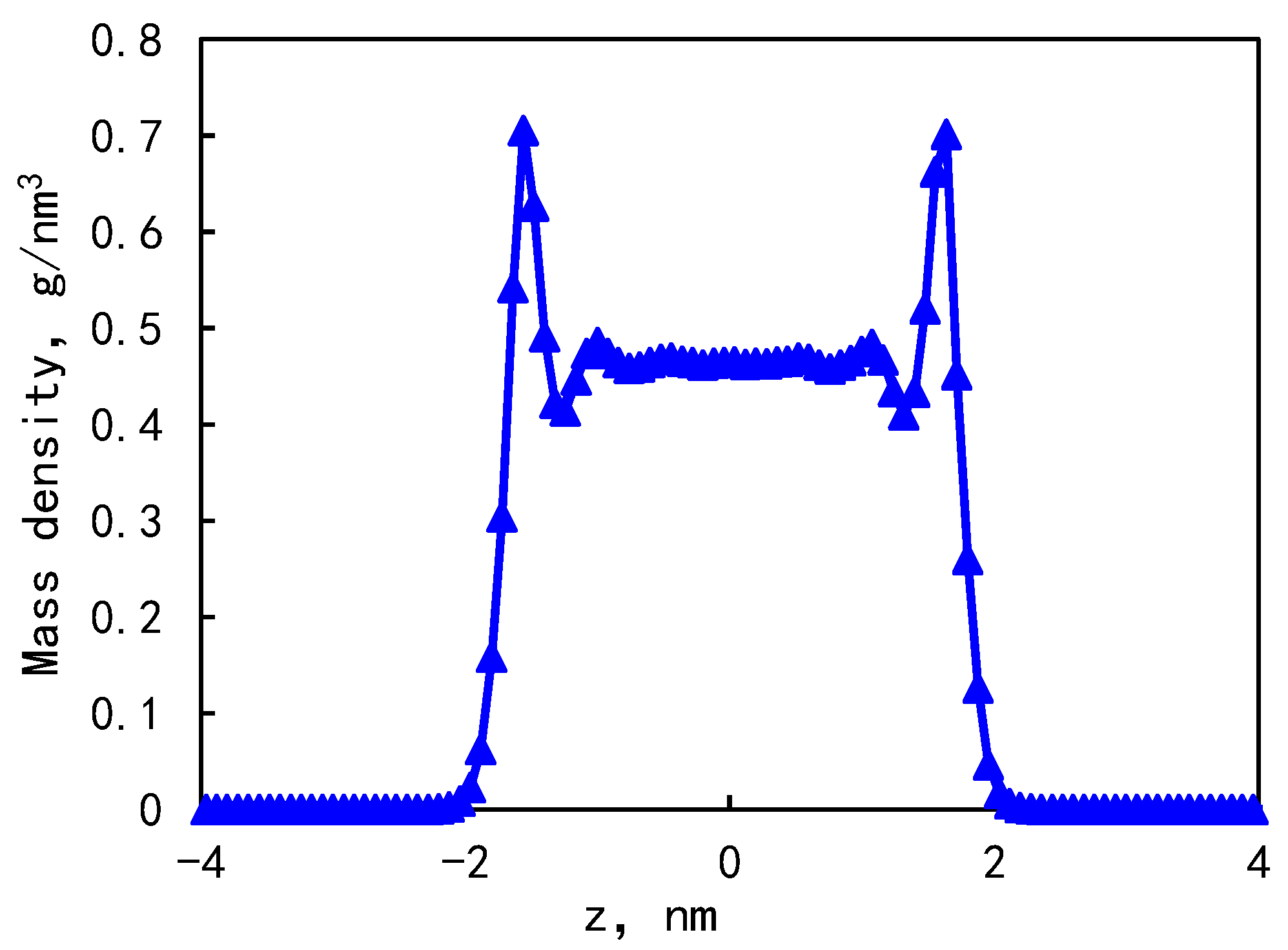
3.1. Occurrence Law of Shale Oil in Composite Pores
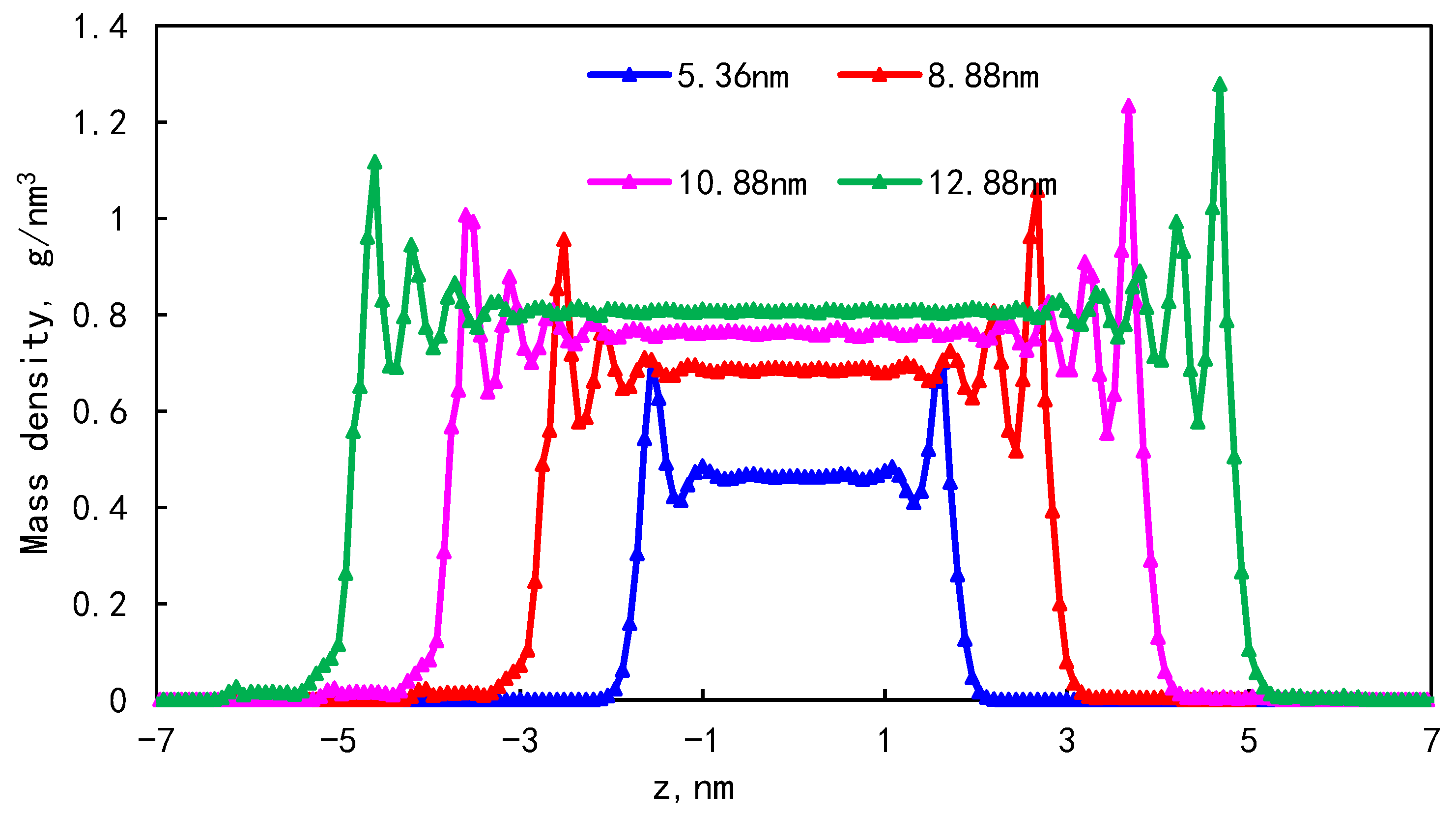
3.2. Effect of Pore Size on Occurrence Characteristics
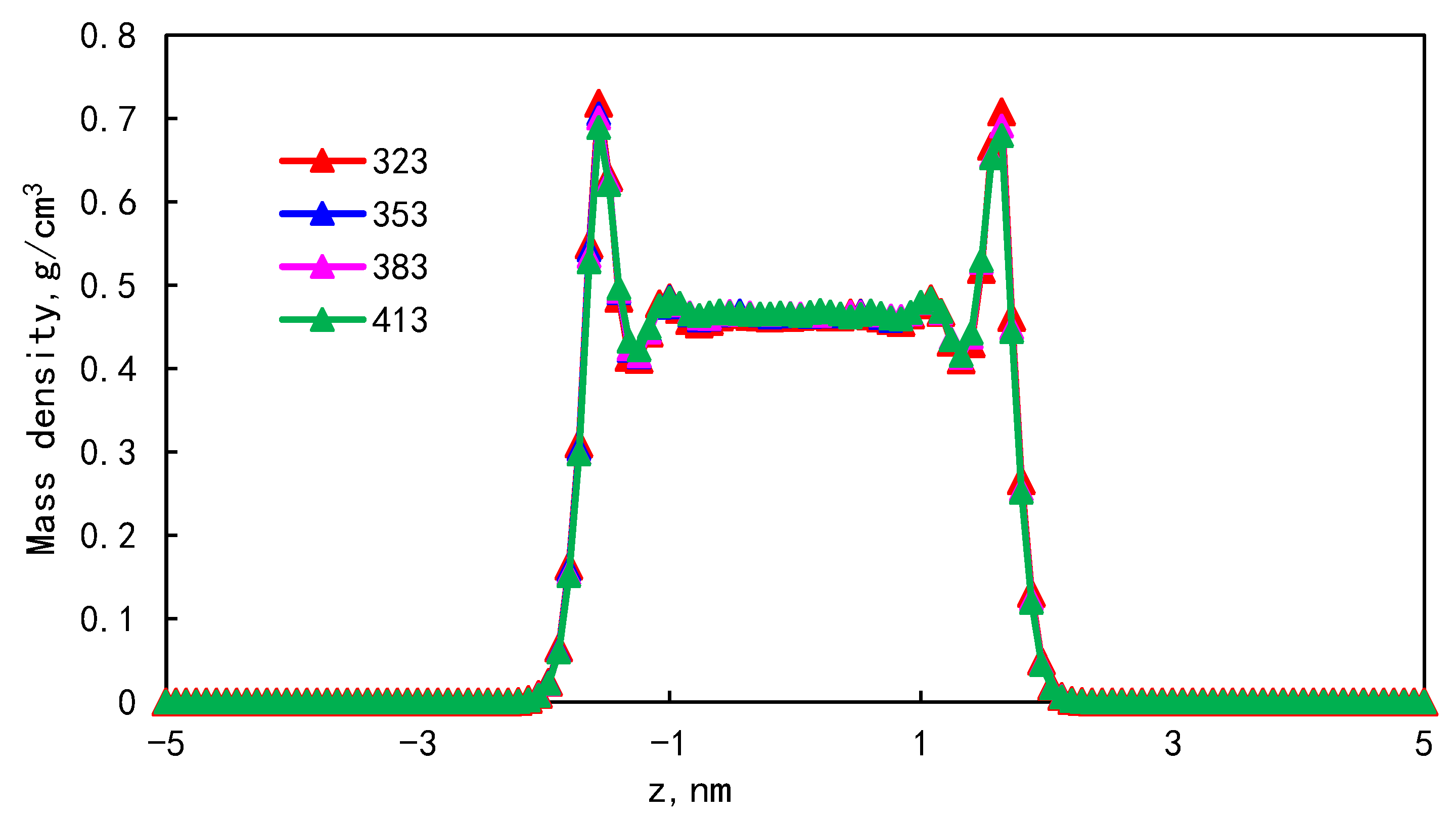
3.3. Effect of Temperature on Occurrence Characteristics
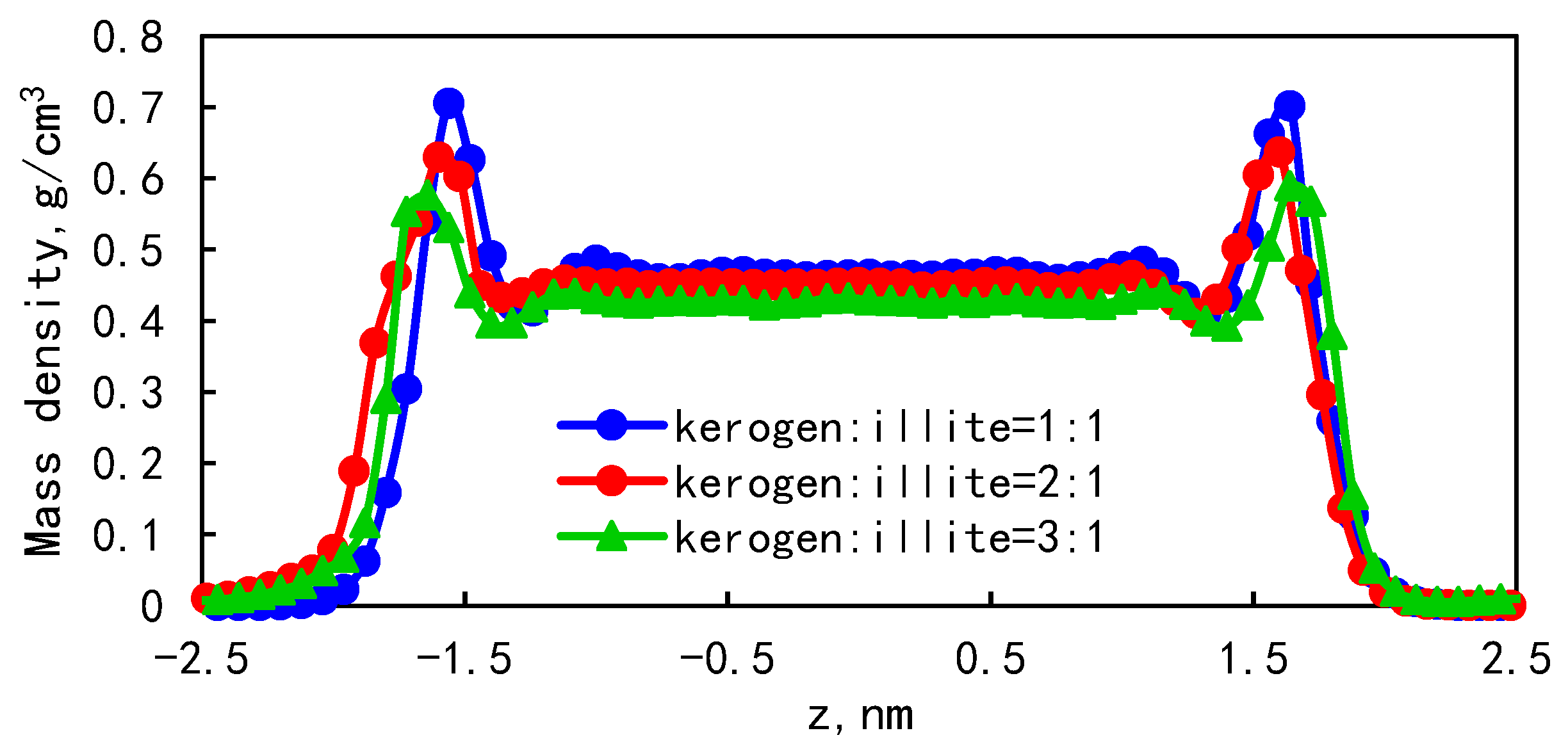
3.4. The Effect of Wall Component Ratio on Occurrence
4. Conclusions
- (1)
- The mass density distribution of the composite wall was analyzed. The results show that the density distribution of octane is not uniform, showing multi-layer adsorption. The thickness of the two adsorption layers is 0.5–0.6 nm, which is the reason for the asymmetric distribution of the adsorption layer when adsorbed on the composite pore surface. The mean square displacement of shale oil molecules parallel to the wall is significantly higher than those perpendicular to the wall, showing that shale oil molecules diffuse mainly in a way that is parallel to the wall.
- (2)
- As the pore size increases, the bulk phase area and pore volume increase but the adsorption capacity tends to remain unchanged, causing a downward trend in the proportion of adsorbed oil. In addition, the proportion of the first adsorption layer to the total adsorption amount decreases rapidly, from 88.88% to 34.19%, and the phenomenon of the “solid-like layer” of shale oil in small pores is more obvious.
- (3)
- The higher the proportion of organic matter in the wall, the greater the adsorption capacity. Because kerogen is more lipophilic, shale oil is more easily retained and the adsorption capacity is larger. The peak of adsorption density is positively correlated with the flatness of the wall. When the ratio of kerogen to illite in the model wall is 1:1, 2:1, and 3:1, the diffusion coefficients were 1.84 × 10−10 m2/s, 1.28 × 10−10 m2/s, and 1.03 × 10−10 m2/s, respectively. The combination of solid–liquid interaction force and friction force makes shale oil more likely to occur in the wall with a high proportion of organic matter.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, Y.; Lun, Z.M.; Pan, Z.J.; Wang, H.T.; Zhou, X.; Zhao, C.P.; Zhang, D.F. Occurrence space and state of shale oil: A review. J. Pet. Sci. Eng. 2022, 211, 110183. [Google Scholar] [CrossRef]
- Taheri Shakib, J.; Kantzas, A. A comprehensive review of microwave application on the oil shale: Prospects for shale oil production. Fuel 2021, 305, 121519. [Google Scholar] [CrossRef]
- Liu, J.; Yang, Y.Y.; Sun, S.S.; Yao, J.; Kou, J.L. Flow behaviors of shale oil in kerogen slit by molecular dynamics simulation. Chem. Eng. J. 2022, 434, 134682-2–134682-10. [Google Scholar] [CrossRef]
- Lu, S.F.; Xue, H.T.; Wang, M.; Xiao, D.S.; Huang, W.B.; Li, J.Q.; Xie, L.J.; Tian, S.X.; Wang, S.; Li, J.J.; et al. Several key issues and research trends in evaluation of shale oil. Acta Pet. Sin. 2016, 37, 1309–1321. [Google Scholar]
- Li, Z.; Zou, Y.R.; Xu, X.Y.; Sun, J.N.; Li, M.W.; Peng, P.A. Adsorption of mudstone source rock for shale oil-experiments, model and a case study. Org. Geochem. 2016, 92, 55–62. [Google Scholar] [CrossRef]
- Wang, S.; Feng, Q.; Javadpour, F.; Xia, T.; Li, Z. Oil adsorption in shale nanopores and its effect on recoverable oil-in-place. Int. J. Coal Geol. 2015, 147, 9–24. [Google Scholar] [CrossRef]
- Lu, X.C.; Li, F.C.; Watson, A.T. Adsorption measurements in Devonian shales. Fuel 1995, 74, 599–603. [Google Scholar] [CrossRef]
- Yang, Y.F.; Liu, J.; Yao, J.; Kou, J.L.; Li, Z.; Wu, T.H.; Zhang, K.; Zhang, L.; Sun, H. Adsorption behaviors of shale oil in kerogen slit by molecular simulation. Chem. Eng. J. 2020, 387, 124054. [Google Scholar] [CrossRef]
- Pawar, G.; Meakin, P.; Huang, H. Reactive molecular dynamics simulation of kerogen thermal maturation and crosslinking pathways. Energy Fuels 2017, 31, 11601–11614. [Google Scholar] [CrossRef]
- Fertl, W.H. Kerogen, Insoluble organic matter from sedimentary rocks. Earth Sci. Rev. 1982, 18, 87–88. [Google Scholar] [CrossRef]
- Huang, L.; Zhou, W.; Xu, H.; Wang, L.; Zou, J.; Zhou, Q.M. Dynamic fluid states in organic-inorganic nanocomposite: Implications for shale gas recovery and CO2 sequestration. Chem. Eng. J. 2021, 411, 128423. [Google Scholar] [CrossRef]
- Wang, S.B.; Song, Z.G.; Cao, T.T.; Song, X. The methane sorption capacity of Paleozoic shales from the Sichuan Basin, China. Mar. Pet. Geol. 2013, 44, 112–119. [Google Scholar] [CrossRef]
- Humayun, R.; Tomasko, D.L. High-resolution adsorption isotherms of supercritical carbon dioxide on activated carbon. AIChE J. 2000, 46, 2065–2075. [Google Scholar] [CrossRef]
- Chang, J.Q.; Fan, X.D.; Jiang, Z.X.; Wang, X.M.; Chen, L.; Li, J.T.; Zhu, L.; Wan, C.X.; Chen, Z.X. Differential impact of clay minerals and organic matter on pore structure and its fractal characteristics of marine and continental shales in China. Appl. Clay Sci. 2022, 216, 106334. [Google Scholar] [CrossRef]
- Liu, X.Y.; Zhang, D.X. A review of phase behavior simulation of hydrocarbons in confined space: Implications for shale oil and shale gas. J. Nat. Gas Sci. Eng. 2019, 68, 102901. [Google Scholar] [CrossRef]
- Hunt, J.M. Oil and organic matter in source rocks of petroleum. Tulsa Geol. Soc. Dig. 1956, 24, 192–197. [Google Scholar]
- Ungerer, P.; Collell, J.; Yiannourakou, M. Molecular modeling of the volumetric and thermodynamic properties of kerogen: Influence of organic type and maturity. Energy Fuels 2015, 29, 91–105. [Google Scholar] [CrossRef]
- Liu, J.; Zhao, Y.; Yang, Y.Y.; Mei, Q.Y.; Yang, S.; Wang, C.C. Multicomponent shale oil flow in real kerogen structures via molecular dynamic simulation. Energies 2020, 13, 3815. [Google Scholar] [CrossRef]
- Collell, J.; Ungerer, P.; Galliero, G.; Yiannourakou, M.; Montel, F.; Pujol, M. Molecular Simulation of Bulk Organic Matter in Type II Shales in the Middle of the Oil Formation Window. Energy Fuels 2014, 28, 7457–7466. [Google Scholar] [CrossRef]
- Ambrose, R.J.; Hartman, R.C.; Diaz-Campos, M.; Akkutlu, I.Y.; Sondergeld, C.H. Shale gas-in-place calculations part I: New pore-scale considerations. SPE J. 2012, 17, 219–229. [Google Scholar] [CrossRef]
- Jorgensen, W.L.; Maxwell, D.S.; Tirado-Rives, J. Development and testing of the OPLS all-atom force field on conformational energetics and properties of organic liquids. J. Am. Chem. Soc. 1996, 118, 11225–11236. [Google Scholar] [CrossRef]
- Perez, F.; Devegowda, D. Spatial distribution of reservoir fluids in mature kerogen using molecular simulations. Fuel 2019, 235, 448–459. [Google Scholar] [CrossRef]
- Dauber-Osguthorpe, P.; Roberts, V.A.; Osguthorpe, D.J.; Wolff, J.; Genest, M.; Hagler, A.T. Structure and energetics of ligand binding to proteins: Escherichia coli dihydrofolate reductase-trimethoprim, a drug-receptor system. Proteins Struct. Funct. Bioinform. 1988, 4, 31–47. [Google Scholar] [CrossRef] [PubMed]
- Drits, V.A.; Weber, L.F.; Salyn, A.L.; Tsipursky, S.I. X-Ray identification of one-layer illite varieties: Application to the study of illites around uranium deposits of Canada. Clays Clay Miner. 1993, 41, 389–398. [Google Scholar] [CrossRef]
- Drits, V.A.; Zviagina, B.B. Trans-vacant and cis-vacant 2:1 Layer Silicates: Structural Features, Identification, and Occurrence. Clays Clay Miner. 2009, 57, 405–415. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1993, 117, 1–19. [Google Scholar] [CrossRef]
- Hockney, R.W.; Eastwood, J.W. Computer Simulation Using Particles; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar]
- Sha, M.L.; Zhang, F.C.; Wu, G.Z.; Fang, H.P.; Hu, J. Ordering layers of [bmim][PF6] ionic liquid on graphite surfaces: Molecular dynamics simulation. J. Chem. Phys. 2008, 128, 134504. [Google Scholar]




| Atomic Type | Molar Mass/ g/mol | σ /Å | /kcal/mol | Electric Charge /e | |
|---|---|---|---|---|---|
| Non-bonded potential energy | C3, C2 | 12.0112 | 3.50 | 0.066 | −0.18, –CH3 −0.12, –CH2 |
| H | 1.0080 | 2.50 | 0.030 | 0.06 |
| Bond Type | , kcal/(mol·Å2) | , Å | |
|---|---|---|---|
| Bond stretching | C2,3–C2,3 | 268.00 | 1.529 |
| C2,3–H | 340.00 | 1.090 |
| Key Angle Type | , kcal/(mol·radian2) | , Radian | |
|---|---|---|---|
| Angle bending | C2,3–C2,3–H | 37.50 | 1.932 |
| H–C2,3–H | 33.00 | 1.881 | |
| C2,3–C2,3–C2,3 | 58.35 | 1.967 |
| Dihedral Angle Type | , kcal/mol | , kcal/mol | , kcal/mol | , kcal/mol | |
|---|---|---|---|---|---|
| Dihedral angle | H–C–C–C | 0.0 | 0.0 | 0.366 | 0.0 |
| H–C–C–H | 0.0 | 0.0 | 0.318 | 0.0 | |
| C–C–C–C | 1.740 | 0.159 | 0.279 | 0.0 |
| Pore size/nm | 5.36 | 8.88 | 10.88 | 12.88 |
| Proportion of adsorption phase/% | 35.19 | 34.30 | 32.99 | 31.53 |
| The proportion of the first adsorption layer/% | 31.25 | 18.72 | 13.55 | 10.78 |
| The ratio of the first adsorption layer to the total adsorption amount/% | 88.80 | 54.58 | 41.07 | 34.19 |
| Pore size/nm | 323 | 353 | 383 | 413 |
| Proportion of adsorption phase/% | 35.36 | 35.19 | 35.04 | 34.97 |
| The proportion of the first adsorption layer/% | 31.45 | 31.25 | 31.10 | 30.97 |
| The ratio of the first adsorption layer to the total adsorption amount/% | 88.92 | 88.79 | 88.75 | 88.56 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, Y.; Li, Z.; Yang, E.; Sha, M.; Song, S. Molecular Dynamics Simulation Study on the Occurrence of Shale Oil in Hybrid Nanopores. Molecules 2024, 29, 312. https://doi.org/10.3390/molecules29020312
Fang Y, Li Z, Yang E, Sha M, Song S. Molecular Dynamics Simulation Study on the Occurrence of Shale Oil in Hybrid Nanopores. Molecules. 2024; 29(2):312. https://doi.org/10.3390/molecules29020312
Chicago/Turabian StyleFang, Yujia, Zhongxiao Li, Erlong Yang, Mingyu Sha, and Shuling Song. 2024. "Molecular Dynamics Simulation Study on the Occurrence of Shale Oil in Hybrid Nanopores" Molecules 29, no. 2: 312. https://doi.org/10.3390/molecules29020312
APA StyleFang, Y., Li, Z., Yang, E., Sha, M., & Song, S. (2024). Molecular Dynamics Simulation Study on the Occurrence of Shale Oil in Hybrid Nanopores. Molecules, 29(2), 312. https://doi.org/10.3390/molecules29020312





