A Density Functional Theory Study of the Physico-Chemical Properties of Alkali Metal Titanate Perovskites for Solar Cell Applications
Abstract
1. Introduction
2. Methodology
3. Results
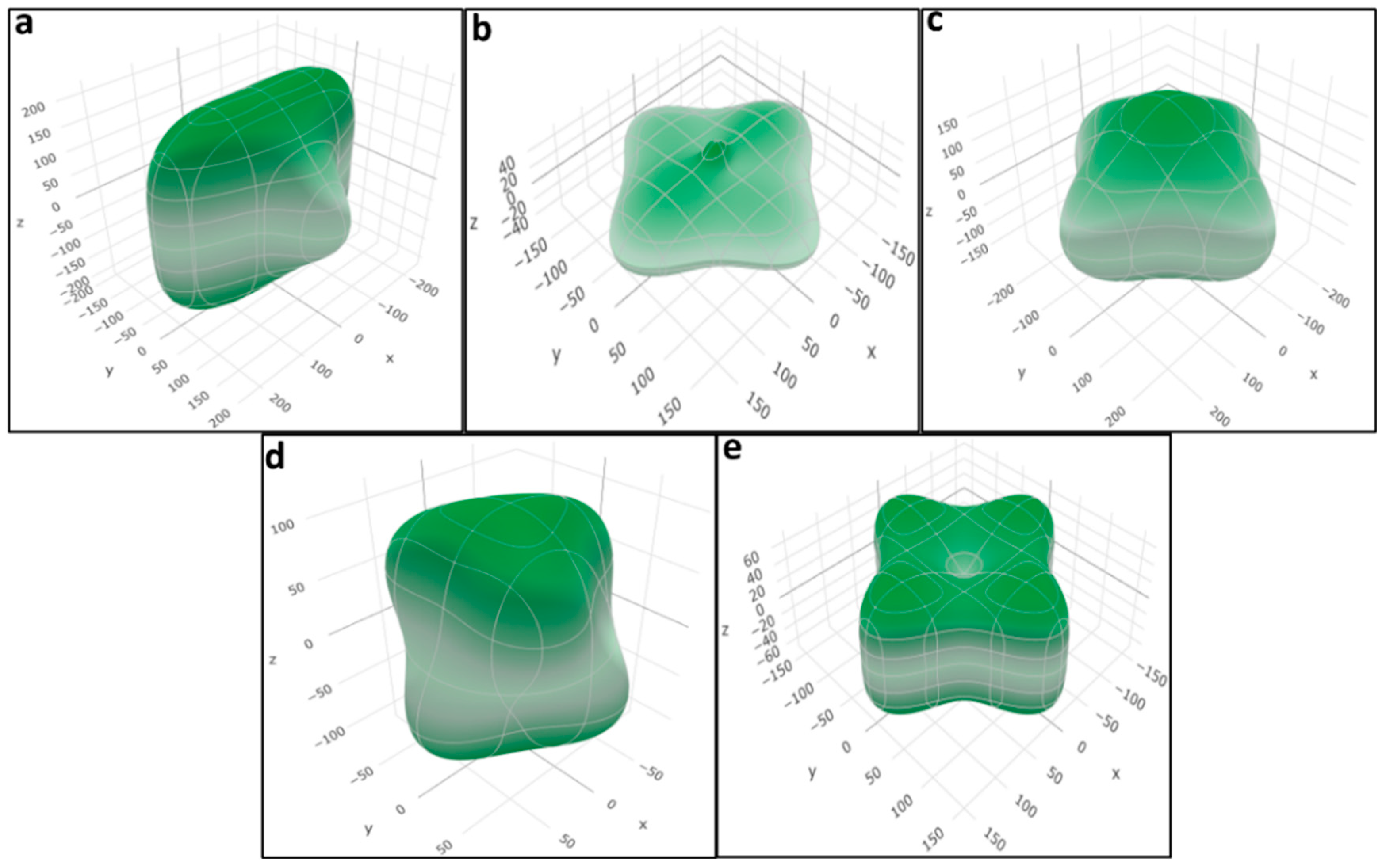
3.1. Structural Properties
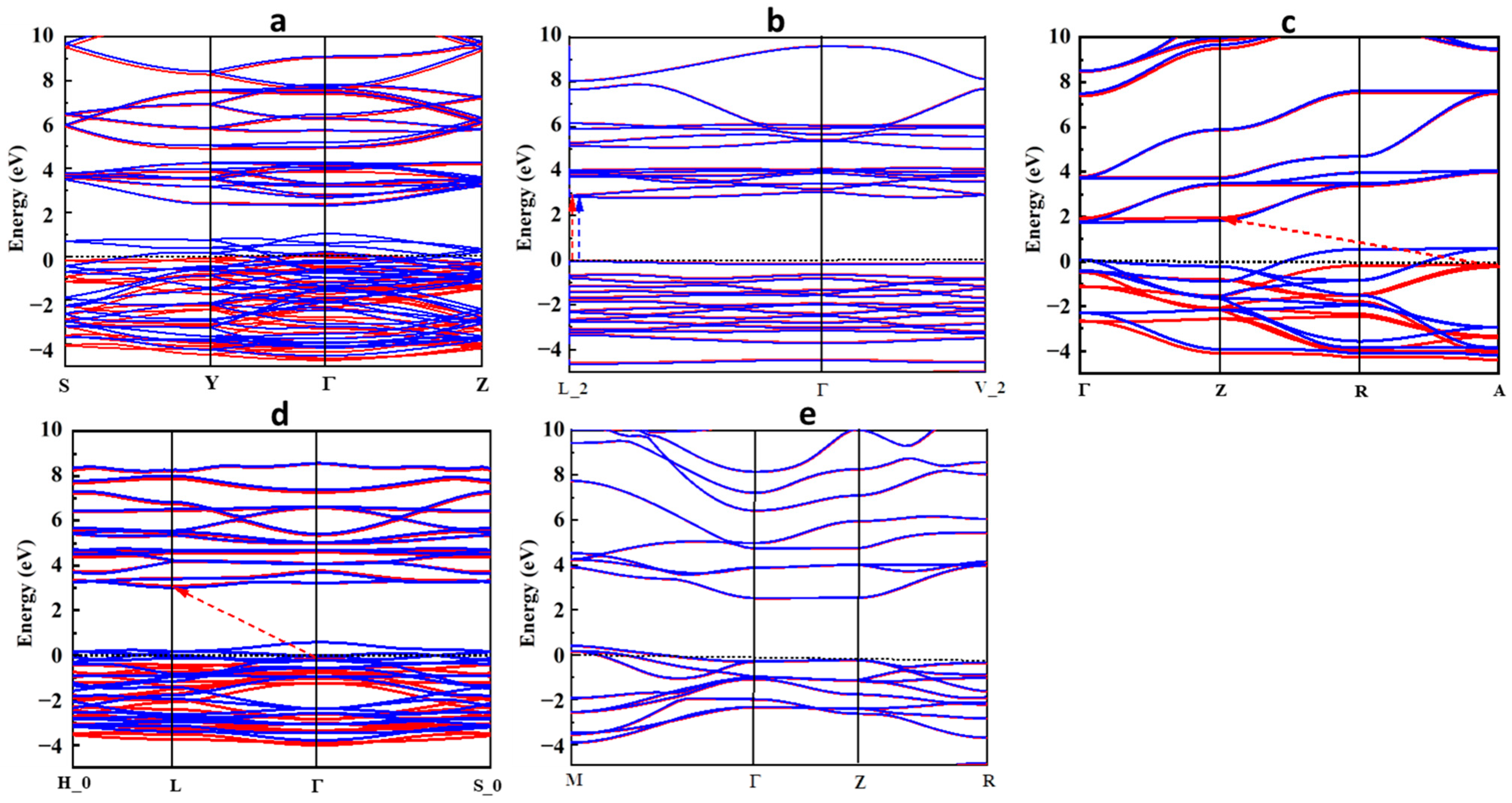
3.2. Electronic and Magnetic Properties
Electronic and Magnetic Properties of ATiO3 (A=Li, Na, K, Rb, and Cs)
3.3. Optical Properties
3.3.1. Dielectric Function
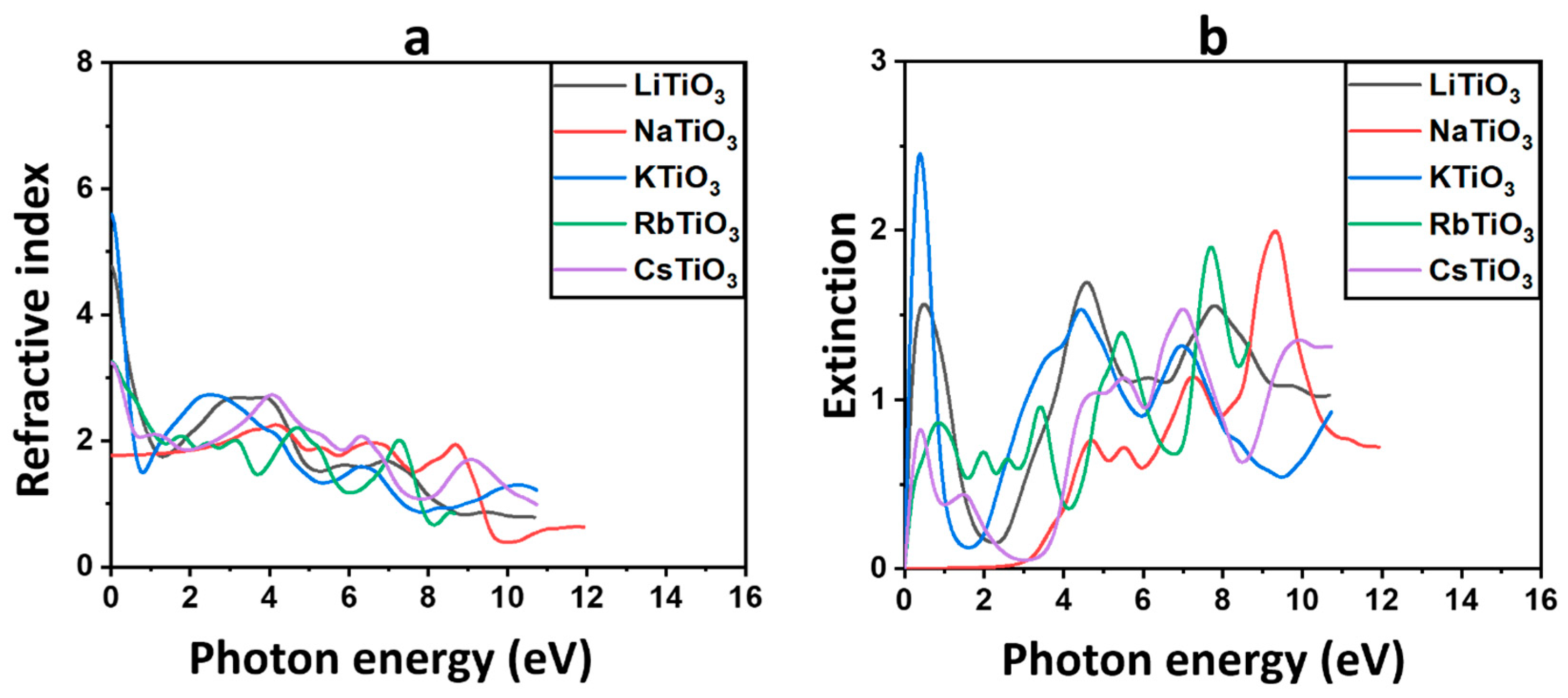
3.3.2. Refractive Index and Extinction Coefficient
3.3.3. Absorption Coefficient
3.3.4. Optical Conductivity
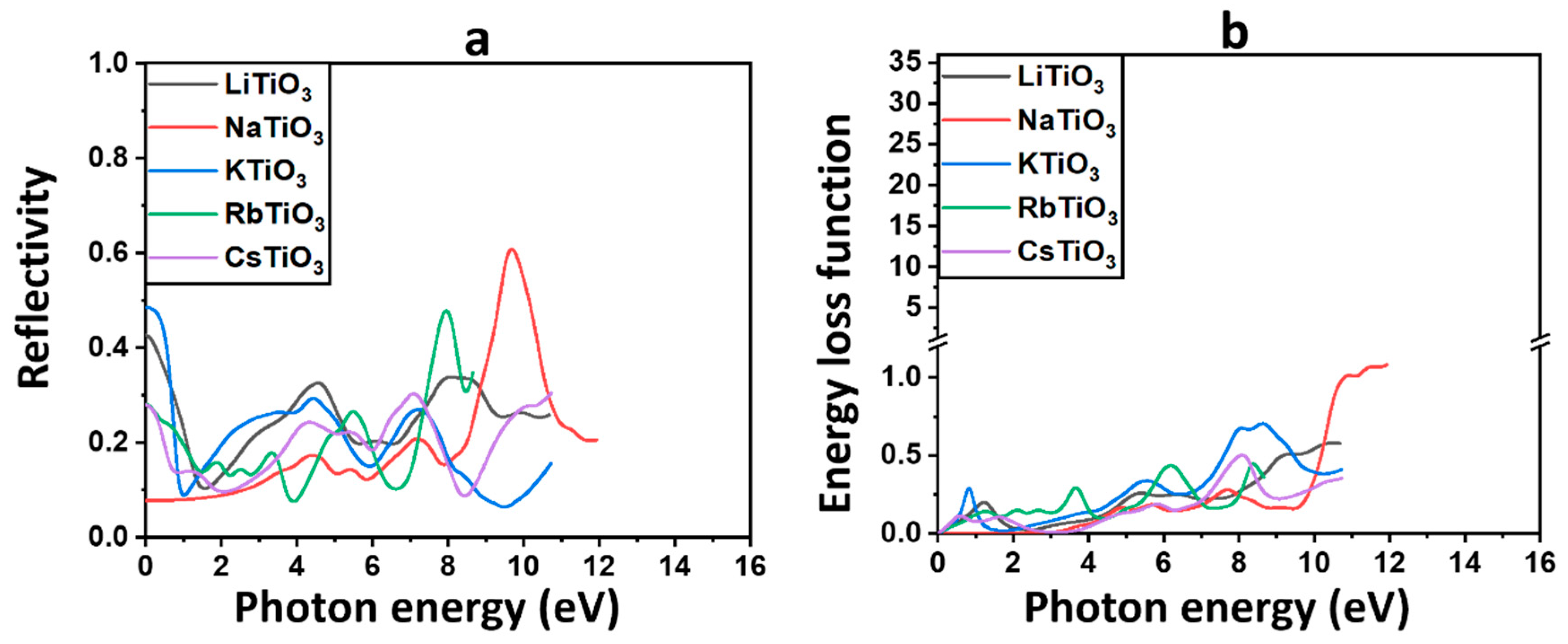
3.3.5. Reflectivity
3.3.6. Energy Loss Function
3.4. Elastic and Thermal Properties
Elastic and Thermal Properties of Alkali-Based Titanates
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shakiba, M.; Pourmadadi, M.; Hosseini, S.; Bigham, A.; Rahmani, E.; Sheikhi, M.; Pahnavar, Z.; Foroozandeh, S.A.; Tajiki, A.; Jouybar, S.; et al. A bi-functional nanofibrous composite membrane for wound healing applications. Arch. Der Pharm. 2024, 7, e202400001. [Google Scholar] [CrossRef] [PubMed]
- Absike, H.; Baaalla, N.; Attou, L.; Labrim, H.; Hartiti, B.; Ez-zahraouy, H. Theoretical investigations of structural, electronic, optical and thermoelectric properties of oxide halide perovskite ACoO3 (A=Nd, Pr or La). Solid State Commun. 2022, 345, 114684. [Google Scholar] [CrossRef]
- Zhang, F.; Xu, J.; Guo, X.; Yuan, B.; Huang, H.; Li, L. Theoretical study on structural properties of silicon-doped benzothiazole/SnO2 (1 0 0): A novel molecular design for solar cells. Appl. Surf. Sci. 2020, 501, 144054. [Google Scholar] [CrossRef]
- Foroozandeh, A.; SalarAmoli, H.; Abdouss, M.; Pourmadadi, M. Development of a labeled-free and labeled electrochemical aptasensor for the detection of cancer antigen 125 by using magnetic g-C3N4/MoS2 nanocomposite. Sens. Actuators Rep. 2024, 7, 100195. [Google Scholar] [CrossRef]
- Gershon, T. Metal oxide applications in organic-based photovoltaics. Mater. Sci. Technol. 2011, 27, 1357–1371. [Google Scholar] [CrossRef]
- Foroozandeh, A.; Hatefirad, P.; Safaei Mahmoudabadi, Z.; Tavasoli, A. Catalytic Activity of Synthesized NiMo Catalysts on Walnut Shell Activated Carbon for Heavy Naphtha Hydrotreating. Iran. J. Chem. Chem. Eng. 2023, 42, 38–50. [Google Scholar] [CrossRef]
- Hosseini, S.M.; Mazinani, S.; Abdouss, M.; Kalhor, H.; Kalantari, K.; Amiri, I.S.; Ramezani, Z. Designing chitosan nanoparticles embedded into graphene oxide as a drug delivery system. Polym. Bull. 2022, 79, 541–554. [Google Scholar] [CrossRef]
- Mahapatra, A.; Prochowicz, D.; Tavakoli, M.M.; Trivedi, S.; Kumar, P.; Yadav, P. A review of aspects of additive engineering in perovskite solar cells. J. Mater. Chem. A 2020, 8, 27–54. [Google Scholar] [CrossRef]
- Marinova, N.; Valero, S.; Delgado, J.L. Organic and perovskite solar cells: Working principles, materials and interfaces. J. Colloid Interface Sci. 2017, 488, 373–389. [Google Scholar] [CrossRef] [PubMed]
- Zhu, K.; Chen, Y.; Wang, Y.; Feng, M.; Zhao, Y. Progress of Solution-Processed Metal Oxides as Charge Transport Layers towards Efficient and Stable Perovskite Solar Cells and Modules. Mater. Today Nano 2022, 20, 100252. [Google Scholar] [CrossRef]
- Manders, J.R.; Tsang, S.W.; Hartel, M.J.; Lai, T.H.; Chen, S.; Amb, C.M.; Reynolds, J.R.; So, F. Solution-processed nickel oxide hole transport layers in high efficiency polymer photovoltaic cells. Adv. Funct. Mater. 2013, 23, 2993–3001. [Google Scholar] [CrossRef]
- Anrango-Camacho, C.; Pavón-Ipiales, K.; Frontana-Uribe, B.A.; Palma-Cando, A. Recent advances in hole-transporting layers for organic solar cells. Nanomaterials 2022, 12, 443. [Google Scholar] [CrossRef]
- De Bruyn, P.; Moet, D.; Blom, P. A facile route to inverted polymer solar cells using a precursor based zinc oxide electron transport layer. Org. Electron. 2010, 11, 1419–1422. [Google Scholar] [CrossRef]
- Zheng, D.; Wang, G.; Huang, W.; Wang, B.; Ke, W.; Logsdon, J.L.; Wang, H.; Wang, Z.; Zhu, W.; Yu, J. Combustion synthesized zinc oxide electron-transport layers for efficient and stable perovskite solar cells. Adv. Funct. Mater. 2019, 29, 1900265. [Google Scholar] [CrossRef]
- Völker, S.; Collavini, S.; Delgado, J. Organic Charge Carriers for Perovskite Solar Cells (ChemSusChem 18/2015). ChemSusChem 2015, 8, 3012–3028. [Google Scholar] [CrossRef]
- Ameen, S.; Akhtar, M.S.; Shin, H.-S.; Nazeeruddin, M.K. Charge-transporting materials for perovskite solar cells. In Advances in Inorganic Chemistry; Elsevier: Amsterdam, The Netherlands, 2018; Volume 72, pp. 185–246. [Google Scholar]
- Fan, X. Doping and Design of Flexible Transparent Electrodes for High-Performance Flexible Organic Solar Cells: Recent Advances and Perspectives. Adv. Funct. Mater. 2021, 31, 2009399. [Google Scholar] [CrossRef]
- Zhang, S.; Guo, R.; Zeng, H.; Zhao, Y.; Liu, X.; You, S.; Li, M.; Luo, L.; Lira-Cantu, M.; Li, L. Improved performance and stability of perovskite solar modules by interface modulating with graphene oxide crosslinked CsPbBr3 quantum dots. Energy Environ. Sci. 2022, 15, 244–253. [Google Scholar] [CrossRef]
- Palilis, L.C.; Vasilopoulou, M.; Verykios, A.; Soultati, A.; Polydorou, E.; Argitis, P.; Davazoglou, D.; Mohd Yusoff, A.R.b.; Nazeeruddin, M.K. Inorganic and hybrid interfacial materials for organic and perovskite solar cells. Adv. Energy Mater. 2020, 10, 2000910. [Google Scholar] [CrossRef]
- Wang, J.; Liu, Y.; Chen, X.; Chen, C.; Chen, P.; Wang, Z.; Duan, Y. Functional metal oxides in perovskite solar cells. ChemPhysChem 2019, 20, 2580–2586. [Google Scholar] [CrossRef]
- Jouybar, S.; Yazdani, F. Synthesis of Magnetite Nanoparticles Under UV/IR Irradiation: Investigation of Effects on the Properties. Nanosci. Nanotechnol. Asia 2017, 8, 289–296. [Google Scholar] [CrossRef]
- Wang, J.; Fu, W.; Jariwala, S.; Sinha, I.; Jen, A.K.-Y.; Ginger, D.S. Reducing surface recombination velocities at the electrical contacts will improve perovskite photovoltaics. ACS Energy Lett. 2018, 4, 222–227. [Google Scholar] [CrossRef]
- Haque, M.A.; Sheikh, A.D.; Guan, X.; Wu, T. Metal oxides as efficient charge transporters in perovskite solar cells. Adv. Energy Mater. 2017, 7, 1602803. [Google Scholar] [CrossRef]
- Yin, Z.; Wei, J.; Zheng, Q. Interfacial materials for organic solar cells: Recent advances and perspectives. Adv. Sci. 2016, 3, 1500362. [Google Scholar] [CrossRef]
- Shin, S.; Lee, S.; Seok, S.I. Exploring wide bandgap metal oxides for perovskite solar cells. APL Mater. 2019, 7, 022401. [Google Scholar] [CrossRef]
- Rizwan, M.; Khadija, Z.; Mahmood, T.; Gillani, S.; Khan, M.I. Alteration impact of electronic properties of c-SrTiO
3 on optical response due to Ca inclusion: A DFT study. Phys. B Condens. Matter 2021, 602, 412553. [Google Scholar] [CrossRef] - Cheng, Z.; Lin, J. Layered organic–inorganic hybrid perovskites: Structure, optical properties, film preparation, patterning and templating engineering. CrystEngComm 2010, 12, 2646–2662. [Google Scholar] [CrossRef]
- Boubchir, M.; Aourag, H. Materials genome project: Mining the ionic conductivity in oxide perovskites. Mater. Sci. Eng. B 2021, 267, 114984. [Google Scholar] [CrossRef]
- Guo, Y.; Ma, L.; Mao, K.; Ju, M.; Bai, Y.; Zhao, J.; Zeng, X.C. Eighteen functional monolayer metal oxides: Wide bandgap semiconductors with superior oxidation resistance and ultrahigh carrier mobility. Nanoscale Horiz. 2019, 4, 592–600. [Google Scholar] [CrossRef]
- Rajamanickam, N.; Soundarrajan, P.; Jayakumar, K.; Ramachandran, K. Improve the power conversion efficiency of perovskite BaSnO3 nanostructures based dye-sensitized solar cells by Fe doping. Sol. Energy Mater. Sol. Cells 2017, 166, 69–77. [Google Scholar] [CrossRef]
- Neophytou, M.; De Bastiani, M.; Gasparini, N.; Aydin, E.; Ugur, E.; Seitkhan, A.; Moruzzi, F.; Choaie, Y.; Ramadan, A.J.; Troughton, J.R. Enhancing the charge extraction and stability of perovskite solar cells using strontium titanate (SrTiO3) electron transport layer. ACS Appl. Energy Mater. 2019, 2, 8090–8097. [Google Scholar] [CrossRef]
- Gholamrezaei, S.; Salavati Niasari, M.; Dadkhah, M.; Sarkhosh, B. New modified sol–gel method for preparation SrTiO3 nanostructures and their application in dye-sensitized solar cells. J. Mater. Sci. Mater. Electron. 2016, 27, 118–125. [Google Scholar] [CrossRef]
- Shang, Q.; Yu, J.; Hu, R.; Liu, Z.; Cheng, J.; Li, Y.; Shai, X.; Huo, M.-m.; Yang, X.; Li, L. Enhanced charge transport in conventional polymer solar cells with a perovskite-type LaNiO3 layer. ACS Appl. Mater. Interfaces 2020, 12, 13051–13060. [Google Scholar] [CrossRef]
- Jouybar, S.; Naji, L.; Mozaffari, S.A.; Sarabadani Tafreshi, S.; de Leeuw, N.H. Electrochemically Engineered Lanthanum Nickelate as a Promising Transparent Hole-Transport Layer for Bulk Heterojunction Polymer Solar Cells: An Experimental and DFT Study. ACS Appl. Energy Mater. 2024, 7, 1647–1665. [Google Scholar] [CrossRef]
- Jouybar, S.; Naji, L.; Mozaffari, S.A.; Sarabadani Tafreshi, S. In Situ Electrochemical Cobalt Doping in Perovskite-Structured Lanthanum Nickelate Thin Film Toward Energy Conversion Enhancement of Polymer Solar Cells. ACS Appl. Mater. Interfaces 2024, 16, 32857–32873. [Google Scholar] [CrossRef]
- Kesavan, A.V.; Rao, A.D.; Ramamurthy, P.C. Tailoring optoelectronic properties of CH3NH3PbI3 perovskite photovoltaics using al nanoparticle modified PC61BM layer. Sol. Energy 2020, 201, 621–627. [Google Scholar] [CrossRef]
- Zhang, C.; Li, Z.; Deng, X.; Yan, B.; Wang, Z.; Chen, X.; Sun, Z.; Huang, S. Enhancing photovoltaic performance of perovskite solar cells utilizing germanium nanoparticles. Sol. Energy 2019, 188, 839–848. [Google Scholar] [CrossRef]
- Piralaee, M.; Ebrahimpour, Z.; Asgari, A. The improved performance of BHJ organic solar cells by random dispersed metal nanoparticles through the active layer. Curr. Appl. Phys. 2020, 20, 531–537. [Google Scholar] [CrossRef]
- Shabani, L.; Mohammadi, A.; Jalali, T. Numerical study of plasmonic effects of Ag nanoparticles embedded in the active layer on performance polymer organic solar cells. Plasmonics 2022, 17, 491–504. [Google Scholar] [CrossRef]
- Ike, J.N.; Hamed, M.S.; Mola, G.T. Effective energy harvesting in thin film organic solar cells using Ni: Zn as bimetallic nanoparticles. J. Phys. Chem. Solids 2022, 161, 110405. [Google Scholar] [CrossRef]
- Lamrani, A.F. Ferromagnetic alloy for high-efficiency photovoltaic conversion in solar cells: First-principles insights when doping SnO2 rutile with coupled Eu–Gd. RSC Adv. 2021, 11, 7096–7106. [Google Scholar] [CrossRef]
- Adewale, A.A.; Chik, A.; Adam, T.; Yusuff, O.K.; Ayinde, S.A.; Sanusi, Y.K. First principles calculations of structural, electronic, mechanical and thermoelectric properties of cubic ATiO3 (A=Be, Mg, Ca, Sr and Ba) perovskite oxide. Comput. Condens. Matter 2021, 28, e00562. [Google Scholar] [CrossRef]
- Nazir, S.; Noor, N.; Afzal, Q.; Mahmood, A. Pressure-Induced Thermodynamic and Opto-Electronic Behavior of BeTiO3 Perovskite: A DFT Investigation. J. Electron. Mater. 2020, 49, 3072–3079. [Google Scholar] [CrossRef]
- Djellabi, R.; Ordonez, M.F.; Conte, F.; Falletta, E.; Bianchi, C.L.; Rossetti, I. A review of advances in multifunctional XTiO3 perovskite-type oxides as piezo-photocatalysts for environmental remediation and energy production. J. Hazard. Mater. 2022, 421, 126792. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Niu, S.; Wang, J.; Zhou, W.; Wang, Y.; Han, K.; Lu, C. Mesoporous SrTiO3 perovskite as a heterogeneous catalyst for biodiesel production: Experimental and DFT studies. Renew. Energy 2022, 184, 164–175. [Google Scholar] [CrossRef]
- Jameel, M.H.; Xu, T.; Jiang, Z.-Y.; Agam, M.A.B.; Roslan, M.S.; Farhina, A.; Hamzah, M.Q.; Rafique, F. First principal calculations of electronic, optical and magnetic properties of cubic K1− xYxNbO3 (Y = Fe, Ni). Phys. Scr. 2021, 96, 125839. [Google Scholar] [CrossRef]
- Pramchu, S.; Jaroenjittichai, A.P.; Laosiritaworn, Y. First-principles calculations of ferroelectricity and structural stability in Bi-and Alkali-Metal-Modified BaTiO3 for PTC thermistor applications. Ceram. Int. 2018, 44, S19–S21. [Google Scholar] [CrossRef]
- Hosseini, S.M.; Abdouss, M.; Mazinani, S.; Soltanabadi, A.; Kalaee, M. Modified nanofiber containing chitosan and graphene oxide-magnetite nanoparticles as effective materials for smart wound dressing. Compos. Part B Eng. 2022, 231, 109557. [Google Scholar] [CrossRef]
- Li, X.; Li, P.; Wu, Z.; Luo, D.; Yu, H.-Y.; Lu, Z.-H. Review and perspective of materials for flexible solar cells. Mater. Rep. Energy 2021, 1, 100001. [Google Scholar] [CrossRef]
- Xu, Y.; Lin, Z.; Wei, W.; Hao, Y.; Liu, S.; Ouyang, J.; Chang, J. Recent Progress of Electrode Materials for Flexible Perovskite Solar Cells. Nano-Micro Lett. 2022, 14, 117. [Google Scholar] [CrossRef]
- Rizwan, M.; Shahid, A.; Mahmood, T.; Zafar, A.A.; Aslam, I.; Adnan, N.; Hussain, T.; Jin, H.; Cao, C. Effect of magnesium on structural and optical properties of CaTiO3: A DFT study. Phys. B Condens. Matter 2019, 568, 88–91. [Google Scholar] [CrossRef]
- Fan, Q.; Yang, J.; Deng, C.; Zhang, J.; Cao, J. Electronic structure and optical properties of CaTiO3: An ab initio study. In Proceedings of the 6th International Conference on Electronics and Information Engineering, Dalian, China, 26–27 September 2015; pp. 485–490. [Google Scholar]
- Tariq, S.; Ahmed, A.; Saad, S.; Tariq, S. Structural, electronic and elastic properties of the cubic CaTiO3 under pressure: A DFT study. Aip Adv. 2015, 5, 077111. [Google Scholar] [CrossRef]
- Wang, Y.; Lian, W.; Liu, Y. Electronic structure and optical properties in alkaline-earth metals (A=Mg, Ca, Ba) and Ir co-doped SrTiO3: A DFT + U investigation. Optik 2021, 228, 166128. [Google Scholar] [CrossRef]
- Rizwan, M.; Ali, A.; Usman, Z.; Khalid, N.; Jin, H.; Cao, C. Structural, electronic and optical properties of copper-doped SrTiO3 perovskite: A DFT study. Phys. B Condens. Matter 2019, 552, 52–57. [Google Scholar] [CrossRef]
- Sokolov, M.; Eglitis, R.; Piskunov, S.; Zhukovskii, Y.F. Ab initio hybrid DFT calculations of BaTiO3 bulk and BaO-terminated (001) surface F-centers. Int. J. Mod. Phys. B 2017, 31, 1750251. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Abbasi, A.; Sardroodi, J.J. A novel nitrogen dioxide gas sensor based on TiO2-supported Au nanoparticles: A van der Waals corrected DFT study. J. Nanostruct. Chem. 2017, 7, 121–132. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 2011, 32, 1456–1465. [Google Scholar] [CrossRef]
- Demir, S.; Torkashvand, M.; Jouybar, S.; Nikfarjam, Z.; Zargari, F.; Tafreshi, S.S.; Tekin, A. Hydrogen Storage in Trimetallic Borohydrides: A Crystal Structure Prediction and Ab Initio Molecular Dynamics Simulations Study. J. Phys. Chem. C 2023, 127, 19344–19355. [Google Scholar] [CrossRef]
- Hill, R. The Elastic Behaviour of a Crystalline Aggregate. Proc. Phys. Society. Sect. A 1952, 65, 349–354. [Google Scholar] [CrossRef]
- Reuss, A. Berechnung der Fließgrenze von Mischkristallen auf Grund der Plastizitätsbedingung für Einkristalle. ZAMM J. Appl. Math. Mech. Z. Für Angew. Math. Und Mech. 1929, 9, 49–58. [Google Scholar] [CrossRef]
- Voigt, W. Lehrbuch Der Kristallphysik; Johnson Reprint Corporation: London, UK, 1928. [Google Scholar]
- Liu, Y.; Liu, B.; Xiang, H.; Zhou, Y.; Nian, H.; Chen, H.; Yang, G.; Gao, Y. Theoretical investigation of anisotropic mechanical and thermal properties of ABO3 (A=Sr, Ba; B = Ti, Zr, Hf) perovskites. J. Am. Ceram. Soc. 2018, 101, 3527–3540. [Google Scholar] [CrossRef]
- Liu, B.; Wang, J.; Zhou, Y.; Liao, T.; Li, F. Theoretical elastic stiffness, structure stability and thermal conductivity of La2Zr2O7 pyrochlore. Acta Mater. 2007, 55, 2949–2957. [Google Scholar] [CrossRef]
- Liu, B.; Wang, J.Y.; Li, F.Z.; Zhou, Y.C. Theoretical elastic stiffness, structural stability and thermal conductivity of La2T2O7 (T = Ge, Ti, Sn, Zr, Hf) pyrochlore. Acta Mater. 2010, 58, 4369–4377. [Google Scholar] [CrossRef]
- Zhou, Y.; Liu, B. Theoretical investigation of mechanical and thermal properties of MPO4 (M=Al, Ga). J. Eur. Ceram. Soc. 2013, 33, 2817–2821. [Google Scholar] [CrossRef]
- Zhan, X.; Li, Z.; Liu, B.; Wang, J.; Zhou, Y.; Hu, Z. Theoretical Prediction of Elastic Stiffness and Minimum Lattice Thermal Conductivity of Y3Al5O12, YAlO3 and Y4Al2O9. J. Am. Ceram. Soc. 2012, 95, 1429–1434. [Google Scholar] [CrossRef]
- Xiang, H.; Feng, Z.; Zhou, Y. Ab initio computations of electronic, mechanical, lattice dynamical and thermal properties of ZrP2O7. J. Eur. Ceram. Soc. 2014, 34, 1809–1818. [Google Scholar] [CrossRef]
- Clarke, D.R. Materials selection guidelines for low thermal conductivity thermal barrier coatings. Surf. Coat. Technol. 2003, 163, 67–74. [Google Scholar] [CrossRef]
- Anderson, O.L. A simplified method for calculating the debye temperature from elastic constants. J. Phys. Chem. Solids 1963, 24, 909–917. [Google Scholar] [CrossRef]
- Wang, V.; Xu, N.; Liu, J.-C.; Tang, G.; Geng, W.-T. VASPKIT: A user-friendly interface facilitating high-throughput computing and analysis using VASP code. Comput. Phys. Commun. 2021, 267, 108033. [Google Scholar] [CrossRef]
- Gaillac, R.; Pullumbi, P.; Coudert, F.-X. ELATE: An open-source online application for analysis and visualization of elastic tensors. J. Phys. Condens. Matter 2016, 28, 275201. [Google Scholar] [CrossRef] [PubMed]
- Kirklin, S.; Saal, J.E.; Meredig, B.; Thompson, A.; Doak, J.W.; Aykol, M.; Rühl, S.; Wolverton, C. The Open Quantum Materials Database (OQMD): Assessing the accuracy of DFT formation energies. npj Comput. Mater. 2015, 1, 15010. [Google Scholar] [CrossRef]
- Saal, J.E.; Kirklin, S.; Aykol, M.; Meredig, B.; Wolverton, C. Materials Design and Discovery with High-Throughput Density Functional Theory: The Open Quantum Materials Database (OQMD). JOM 2013, 65, 1501–1509. [Google Scholar] [CrossRef]
- Goodenough, J.B. Electronic and ionic transport properties and other physical aspects of perovskites. Rep. Prog. Phys. 2004, 67, 1915–1993. [Google Scholar] [CrossRef]
- Travis, W.; Glover, E.; Bronstein, H.; Scanlon, D.; Palgrave, R. On the application of the tolerance factor to inorganic and hybrid halide perovskites: A revised system. Chem. Sci. 2016, 7, 4548–4556. [Google Scholar] [CrossRef] [PubMed]
- Maddah, H.A.; Berry, V.; Behura, S.K. Cuboctahedral stability in Titanium halide perovskites via machine learning. Comput. Mater. Sci. 2020, 173, 109415. [Google Scholar] [CrossRef]
- Noh, M.F.M.; Teh, C.H.; Daik, R.; Lim, E.L.; Yap, C.C.; Ibrahim, M.A.; Ludin, N.A.; bin Mohd Yusoff, A.R.; Jang, J.; Teridi, M.A.M. The architecture of the electron transport layer for a perovskite solar cell. J. Mater. Chem. C 2018, 6, 682–712. [Google Scholar]
- Tang, Q.; Zhou, Z.; Chen, Z. Innovation and discovery of graphene-like materials via density-functional theory computations. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2015, 5, 360–379. [Google Scholar] [CrossRef]
- Park, J.; Wu, Y.-N.; Saidi, W.A.; Chorpening, B.; Duan, Y. First-principles exploration of oxygen vacancy impact on electronic and optical properties of ABO3−δ (A=La, Sr; B = Cr, Mn) perovskites. Phys. Chem. Chem. Phys. 2020, 22, 27163–27172. [Google Scholar] [CrossRef] [PubMed]
- Hosseini, S.M.; Soltanabadi, A.; Abdouss, M.; Mazinani, S. Investigating the structure of the product of graphene oxide reaction with folic acid and chitosan: Density functional theory calculations. J. Biomol. Struct. Dyn. 2022, 40, 14146–14159. [Google Scholar] [CrossRef] [PubMed]
- Hussain, M.I.; Khalil, R.M.A.; Hussain, F.; Rana, A.M. Ab initio prediction of the structural, electronic and optical behavior of novel combinations of ternary perovskite oxides ATiO3 (A=Rb, Cs, Fr) using the Hubbard ‘U’ correction for optoelectronic devices. J. Comput. Electron. 2020, 19, 1380–1388. [Google Scholar] [CrossRef]
- Sakhya, A.P.; Dutta, A.; Sinha, T.P. Dielectric relaxation of samarium aluminate. Appl. Phys. A 2014, 114, 1097–1104. [Google Scholar] [CrossRef]
- Sandeep; Rai, D.P.; Shankar, A.; Ghimire, M.P.; Khenata, R.; Thapa, R.K. Study of electronic and magnetic properties in 4felectron based cubic EuAlO3: A first-principles calculation. Phys. Scr. 2015, 90, 065803. [Google Scholar] [CrossRef]
- El Amine Monir, M.; Baltach, H.; El Haj Hassan, F.; Bahnes, A.; Bahnes, Z. Study of Structural, Electronic, and Magnetic Properties of Cubic Lanthanide Based on Oxide Perovskite-Type NdGaO3. J. Supercond. Nov. Magn. 2019, 32, 2149–2154. [Google Scholar] [CrossRef]
- Butt, M.K.; Yaseen, M.; Bhatti, I.A.; Iqbal, J.; Misbah; Murtaza, A.; Iqbal, M.; Al-Anazy, M.m.; Alhossainy, M.H.; Laref, A. A DFT study of structural, magnetic, elastic and optoelectronic properties of lanthanide based XAlO3 (X = Nd, Gd) compounds. J. Mater. Res. Technol. 2020, 9, 16488–16496. [Google Scholar] [CrossRef]
- Kobayashi, K.I.; Kimura, T.; Sawada, H.; Terakura, K.; Tokura, Y. Room-temperature magnetoresistance in an oxide material with an ordered double-perovskite structure. Nature 1998, 395, 677–680. [Google Scholar] [CrossRef]
- Mir, S.A.; Gupta, D.C. Exploration of uranium double perovskites Ba2MUO6 (M = Co, Ni) for magnetism, spintronic and thermoelectric applications. J. Magn. Magn. Mater. 2020, 493, 165722. [Google Scholar] [CrossRef]
- Mir, S.A.; Gupta, D.C. Analysis of Cage Structured Halide Double Perovskites Cs2NaMCl6 (M = Ti, V) by Spin Polarized Calculations. J. Alloys Compd. 2021, 854, 156000. [Google Scholar] [CrossRef]
- Park, J.H.; Vescovo, E.; Kim, H.J.; Kwon, C.; Ramesh, R.; Venkatesan, T. Direct evidence for a half-metallic ferromagnet. Nature 1998, 392, 794–796. [Google Scholar] [CrossRef]
- Soulen, R.J.; Byers, J.M.; Osofsky, M.S.; Nadgorny, B.; Ambrose, T.; Cheng, S.F.; Broussard, P.R.; Tanaka, C.T.; Nowak, J.; Moodera, J.S.; et al. Measuring the Spin Polarization of a Metal with a Superconducting Point Contact. Science 1998, 282, 85–88. [Google Scholar] [CrossRef]
- Jedema, F.J.; Filip, A.T.; van Wees, B.J. Electrical spin injection and accumulation at room temperature in an all-metal mesoscopic spin valve. Nature 2001, 410, 345–348. [Google Scholar] [CrossRef]
- Schwarz, K. CrO2 predicted as a half-metallic ferromagnet. J. Phys. F Met. Phys. 1986, 16, L211–L215. [Google Scholar] [CrossRef]
- Sherkar, T.S.; Momblona, C.; Gil-Escrig, L.; Avila, J.; Sessolo, M.; Bolink, H.J.; Koster, L.J.A. Recombination in perovskite solar cells: Significance of grain boundaries, interface traps, and defect ions. ACS Energy Lett. 2017, 2, 1214–1222. [Google Scholar] [CrossRef]
- Cheng, Y.; Chen, C.; Chen, X.; Jin, J.; Li, H.; Song, H.; Dai, Q. Considerably enhanced perovskite solar cells via the introduction of metallic nanostructures. J. Mater. Chem. A 2017, 5, 6515–6521. [Google Scholar] [CrossRef]
- Mircholi, F.; Moghadam, H.G. Study of electronic and optical properties of Bi0.5Na0.5TiO3, BiTiO3, NaTiO3 crystals using full potential linear argumented plane wave method. Optik 2015, 126, 1505–1509. [Google Scholar]
- Hilal, M.; Rashid, B.; Khan, S.H.; Khan, A. Investigation of electro-optical properties of InSb under the influence of spin-orbit interaction at room temperature. Mater. Chem. Phys. 2016, 184, 41–48. [Google Scholar] [CrossRef]
- Nishat, S.S.; Hossain, M.J.; Mullick, F.E.; Kabir, A.; Chowdhury, S.; Islam, S.; Hossain, M. Performance Analysis of Perovskite Solar Cells Using DFT-Extracted Parameters of Metal-Doped TiO2 Electron Transport Layer. J. Phys. Chem. C 2021, 125, 13158–13166. [Google Scholar] [CrossRef]
- Azri, F.; Meftah, A.; Sengouga, N.; Meftah, A. Electron and hole transport layers optimization by numerical simulation of a perovskite solar cell. Sol. Energy 2019, 181, 372–378. [Google Scholar] [CrossRef]
- Kittel, C. Introduction to Solid State Physics; Wiley: New York, NY, USA, 1966. [Google Scholar]
- Shen, T.; Hu, C.; Dai, H.L.; Yang, W.L.; Liu, H.C.; Tan, C.L.; Wei, X.L. First principles calculations of magnetic, electronic and optical properties of (Mn–Fe) co-doped SrTiO3. Opt. Int. J. Light Electron Opt. 2016, 127, 3055–3058. [Google Scholar] [CrossRef]
- Rizwan, M.; Zeba, I.; Shakil, M.; Gillani, S.; Usman, Z. Electronic, structural and optical properties of BaTiO3 doped with lanthanum (La): Insight from DFT calculation. Optik 2020, 211, 164611. [Google Scholar] [CrossRef]
- Gillani, S.; Ahmad, R.; Rizwan, M.; Shakil, M.; Rafique, M.; Murtaza, G.; Jin, H. First-principles investigation of structural, electronic, optical and thermal properties of Zinc doped SrTiO3. Optik 2020, 201, 163481. [Google Scholar] [CrossRef]
- El Sayed, A.M. Aspects of structural, optical properties, and relaxation in (BiFeO3 or NaTiO3)–PMMA: Hybrid films for dielectric applications. J. Phys. Chem. Solids 2021, 148, 109767. [Google Scholar] [CrossRef]
- Aktary, M.; Kamruzzaman, M.; Afrose, R. A comparative study of the mechanical stability, electronic, optical and photocatalytic properties of CsPbX3 (X = Cl, Br, I) by DFT calculations for optoelectronic applications. RSC Adv. 2022, 12, 23704–23717. [Google Scholar] [CrossRef]
- El-Ghtami, H.; Laref, A.; Laref, S. Electronic and optical behaviors of methylammonium and formamidinium lead trihalide perovskite materials. J. Mater. Sci. Mater. Electron. 2019, 30, 711–720. [Google Scholar] [CrossRef]
- Xiong, K.; Robertson, J.; Clark, S.J. Defect states in the high-dielectric-constant gate oxide LaAlO3. Appl. Phys. Lett. 2006, 89, 022907. [Google Scholar] [CrossRef]
- Dey, A.; Sharma, R.; Dar, S.A.; Wani, I.H. Cubic PbGeO3 perovskite oxide: A compound with striking electronic, thermoelectric and optical properties, explored using DFT studies. Comput. Condens. Matter 2021, 26, e00532. [Google Scholar] [CrossRef]
- Boudali, A.; Abada, A.; Khodja, M.D.; Amrani, B.; Amara, K.; Khodja, F.D.; Elias, A. Calculation of structural, elastic, electronic, and thermal properties of orthorhombic CaTiO3. Phys. B Condens. Matter 2010, 405, 3879–3884. [Google Scholar] [CrossRef]
- Berri, S. Ab initio study of fundamental properties of XAlO3 (X = Cs, Rb and K) compounds. J. Sci. Adv. Mater. Devices 2018, 3, 254–261. [Google Scholar] [CrossRef]
- Hussain Reshak, A.; Charifi, Z.; Baaziz, H. Ab-initio calculation of structural, electronic, and optical characterizations of the intermetallic trialuminides ScAl3 compound. J. Solid State Chem. 2010, 183, 1290–1296. [Google Scholar] [CrossRef]
- Palik, E.D. (Ed.) Handbook of Optical Constants of Solids; Academic Press: Boston, MA, USA, 1998. [Google Scholar]
- Mao, Y.; Yao, Z.; Yuan, J.; Chang, X. Two-dimensional germanene-based Janus material Ge8HnX8−n (n = 0–8, X = F, Cl, Br, I) for photovoltaic and photocatalytic applications. Appl. Surf. Sci. 2022, 598, 153633. [Google Scholar] [CrossRef]
- Dar, S.A.; Sharma, R.; Srivastava, V.; Sakalle, U.K. Investigation on the electronic structure, optical, elastic, mechanical, thermodynamic and thermoelectric properties of wide band gap semiconductor double perovskite Ba2InTaO6. RSC Adv. 2019, 9, 9522–9532. [Google Scholar] [CrossRef] [PubMed]
- Dey, A.; Sharma, R.; Dar, S.A. An extensive investigation of structural, electronic, thermoelectric and optical properties of bi-based half-Huesler alloys by first principles calculations. Mater. Today Commun. 2020, 25, 101647. [Google Scholar] [CrossRef]
- Dey, A. A First-Principles Study of TiX2 (X = S, Se, and Te) Compounds Optical Properties under the Effect of Externally Applied Electric Field and Strain. Phys. Solid State 2020, 62, 1905–1915. [Google Scholar] [CrossRef]
- Pankove, J.I. Optical Processes in Semiconductors; Prentice-Hall: Hoboken, NJ, USA; The University of Michigan: Ann Arbor, MI, USA, 1971. [Google Scholar]
- Eya, H.I.; Dzade, N.Y. Density Functional Theory Insights into the Structural, Electronic, Optical, Surface, and Band Alignment Properties of BaZrS3 Chalcogenide Perovskite for Photovoltaics. ACS Appl. Energy Mater. 2023, 6, 5729–5738. [Google Scholar] [CrossRef]
- Hofer, F.; Schmidt, F.-P.; Grogger, W.; Kothleitner, G. Fundamentals of electron energy-loss spectroscopy. IOP Conf. Ser. Mater. Sci. Eng. 2016, 109, 012007. [Google Scholar]
- Arévalo-López, A.M.; Castillo-Martinez, E.; Alario-Franco, M.A. Electron energy loss spectroscopy in ACrO3 (A=Ca, Sr and Pb) perovskites. J. Phys. Condens. Matter 2008, 20, 505207. [Google Scholar] [CrossRef][Green Version]
- Bera, A.; Wu, K.; Sheikh, A.; Alarousu, E.; Mohammed, O.F.; Wu, T. Perovskite Oxide SrTiO3 as an Efficient Electron Transporter for Hybrid Perovskite Solar Cells. J. Phys. Chem. C 2014, 118, 28494–28501. [Google Scholar] [CrossRef]
- Okamoto, Y.; Suzuki, Y. Perovskite-type SrTiO3, CaTiO3 and BaTiO3 porous film electrodes for dye-sensitized solar cells. J. Ceram. Soc. Jpn. 2014, 122, 728–731. [Google Scholar] [CrossRef]
- Tapa, A.R.; Xiang, W.; Wu, S.; Li, B.; Liu, Q.; Zhang, M.; Ghadamyari, M.; Verpoort, F.; Wang, J.; Trokourey, A.; et al. Enhanced Performance of Carbon–Selenide Composite with La0.9Ce0.1NiO3 Perovskite Oxide for Outstanding Counter Electrodes in Platinum-Free Dye-Sensitized Solar Cells. Nanomaterials 2022, 12, 961. [Google Scholar] [CrossRef]
- Roose, B.; Wang, Q.; Abate, A. The role of charge selective contacts in perovskite solar cell stability. Adv. Energy Mater. 2019, 9, 1803140. [Google Scholar] [CrossRef]
- Wang, Y.; Djurišić, A.B.; Chen, W.; Liu, F.; Cheng, R.; Feng, S.P.; Ng, A.M.C.; He, Z. Metal oxide charge transport layers in perovskite solar cells—Optimising low temperature processing and improving the interfaces towards low temperature processed, efficient and stable devices. J. Phys. Energy 2020, 3, 012004. [Google Scholar] [CrossRef]
- Feng, R.; Kim, G.; Yu, D.; Chen, Y.; Chen, W.; Liaw, P.K.; An, K. Elastic behavior of binary and ternary refractory multi-principal-element alloys. Mater. Des. 2022, 219, 110820. [Google Scholar] [CrossRef]
- Mazumder, J.T.; Mayengbam, R.; Tripathy, S. Theoretical investigation on structural, electronic, optical and elastic properties of TiO2, SnO2, ZrO2 and HfO2 using SCAN meta-GGA functional: A DFT study. Mater. Chem. Phys. 2020, 254, 123474. [Google Scholar] [CrossRef]
- Jayan, K.D.; Sebastian, V. A review on computational modelling of individual device components and interfaces of perovskite solar cells using DFT. AIP Conf. Proc. 2019, 2162, 020036. [Google Scholar]
- Wang, T.; Wu, C.; Ji, X.; Cui, D. Direct Synthesis of Functional Thermoplastic Elastomer with Excellent Mechanical Properties by Scandium-Catalyzed Copolymerization of Ethylene and Fluorostyrenes. Angew. Chem. 2021, 133, 25939–25944. [Google Scholar] [CrossRef]
- Hasan, M.M.; Kabir, A.; Kamruzzaman, M. The structural, elastic, electronic, magnetic and optical properties of SrNiO3 perovskite: A DFT and DFT + U study. Results Phys. 2022, 41, 105920. [Google Scholar] [CrossRef]
- Ayub, S.; Siddique, A.; Khalil, A.; Shaheen, R.; Tahir, M.B.; Ullah, Z.; Hannan, A. An extensive investigation of structural, electronic, optical, magnetic, and mechanical properties of YGaO3 for photovoltaic and optoelectronic applications: First-principles approach. Inorg. Chem. Commun. 2023, 153, 110754. [Google Scholar] [CrossRef]
- Khandy, S.A.; Islam, I.; Gupta, D.C.; Laref, A. Electronic structure, mechanical and thermodynamic properties of BaPaO3 under pressure. J. Mol. Model. 2018, 24, 131. [Google Scholar] [CrossRef]
- Ugural, A.C.; Fenster, S.K. Advanced Strength and Applied Elasticity; Pearson Education: London, UK, 2003. [Google Scholar]
- Yu, X.; Zhou, J.; Liang, H.; Jiang, Z.; Wu, L. Mechanical metamaterials associated with stiffness, rigidity and compressibility: A brief review. Prog. Mater. Sci. 2018, 94, 114–173. [Google Scholar] [CrossRef]
- Razbin, M.; Bagherzadeh, R. Predicting the longitudinal young’s modulus of helical auxetic yarn reinforced unidirectional composite. J. Text. Inst. 2022, 114, 273–281. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, B.; Chen, L.; Qin, F.; Hou, Y. Measurement of Elastic Constants of Additive Manufactured Ti-6Al-4V by Non-contact Multi-mode Laser Ultrasonic System. J. Mater. Eng. Perform. 2022, 31, 7328–7336. [Google Scholar] [CrossRef]
- Musembi, R.; Mbilo, M. Ab initio study of structural, electronic, elastic, mechanical, and optical properties of K4XP2 (X = Zn, Cd) compounds for optoelectronic applications. Materialia 2022, 26, 101587. [Google Scholar] [CrossRef]
- Hwayyin, R.N.; Ameed, A.S. The Time Dependent Poisson’s Ratio of Nonlinear Thermoviscoelastic Behavior of Glass/Polyester Composite. Jordan J. Mech. Ind. Eng. 2022, 16, 515–528. [Google Scholar]
- Antonio, J.; Rosas-Huerta, J.; Cervantes, J.; León-Flores, J.; Romero, M.; Carvajal, E.; Escamilla, R. Li/Na atoms’ substitution effects on the structural, electronic, and mechanical properties of the CaSnO3 perovskite for battery applications. Comput. Mater. Sci. 2023, 219, 112006. [Google Scholar] [CrossRef]
- Agne, M.T.; Hanus, R.; Snyder, G.J. Minimum thermal conductivity in the context of diffuson-mediated thermal transport. Energy Environ. Sci. 2018, 11, 609–616. [Google Scholar] [CrossRef]
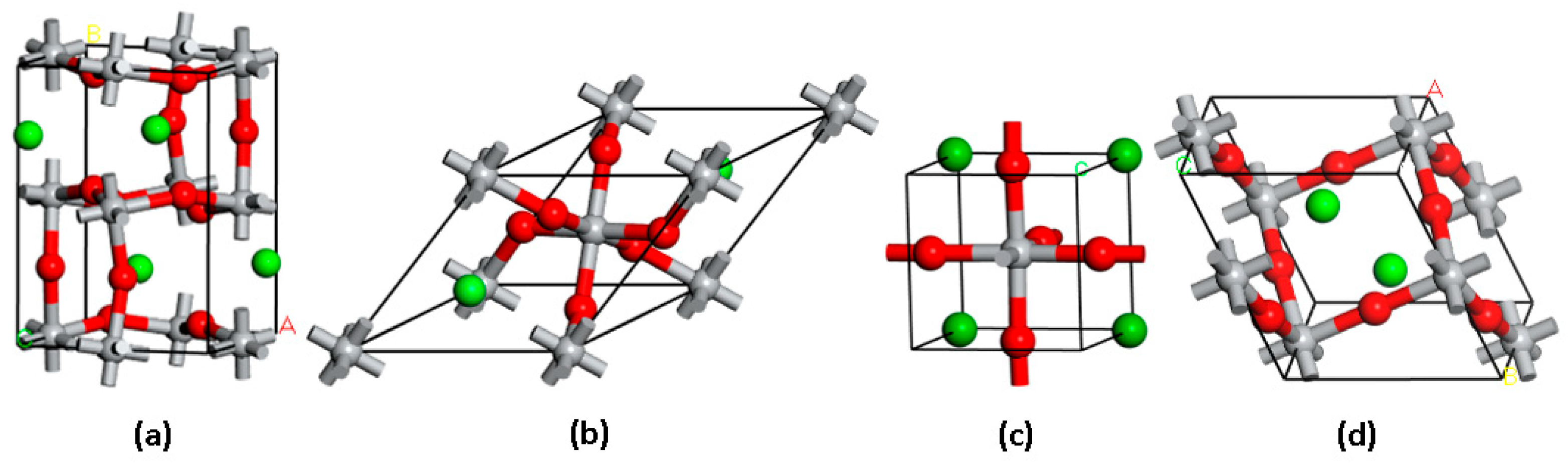



| Bulk Unit Cell Formula | Crystal Structures (Space Group) | Volume (Å3) | a (Å) | b (Å) | c (Å) | α (°) | β (°) | γ (°) | ΔHf [77,78] (eV/Atom) | t-Factor |
|---|---|---|---|---|---|---|---|---|---|---|
| (LiTiO3)4 | Orthorhombic (Pnma) | 199.587 | 5.550 | 7.001 | 5.136 | 90.00 | 90.00 | 90.00 | −2.454 | 0.962 |
| (NaTiO3)4 | Monoclinic (C2/m) | 135.444 | 5.647 | 5.647 | 5.492 | 100.67 | 100.67 | 122.97 | −2.510 | 0.855 |
| KTiO3 | Tetragonal (P4mm) | 62.569 | 3.969 | 3.969 | 3.972 | 90.00 | 90.00 | 90.00 | −2.443 | 1.000 |
| (RbTiO3)2 | Trigonal (R-3) | 160.963 | 6.966 | 6.966 | 6.966 | 46.25 | 46.25 | 46.25 | −2.386 | 1.041 |
| CsTiO3 | Tetragonal (P4mm) | 76.328 | 3.901 | 3.901 | 5.016 | 90.00 | 90.00 | 90.00 | −2.307 | 1.005 |
| mA (µB) | mTi (µB) | m3O (µB) | mtot (µB/f.u.) | |
|---|---|---|---|---|
| A=Li | −0.004 | −0.062 | 0.902 | 0.836 |
| A=Na | 0 | 0 | 0 | 0 |
| A=K | −0.012 | −0.164 | 0.957 | 0.781 |
| A=Rb | 0.006 | −0.1 | 0.914 | 0.820 |
| A=Cs | 0 | 0 | 0 | 0 |
| Eg Spin-Up | EVBM Spin-Up | ECBM Spin-Up | Eg Spin-Down | EVBM Spin-Down | ECBM Spin-Down | Electronic Nature | |
|---|---|---|---|---|---|---|---|
| LiTiO3 | 0 | 0.063 | 2.317 | 0 | 1.005 | 2.283 | metallic |
| NaTiO3 | 2.771 | −0.021 | 2.750 | 2.771 | −0.021 | 2.750 | semiconductor |
| KTiO3 | 2.333 | −0.090 | 2.243 | 0 | 0.687 | 2.095 | half-metallic |
| RbTiO3 | 3.177 | −0.122 | 3.055 | 0 | 0.547 | 2.964 | half-metallic |
| CsTiO3 | 0 | 0.410 | 2.456 | 0 | 0.410 | 2.456 | metallic |
| Name | (104/cm) | (1016/s) | ||||
|---|---|---|---|---|---|---|
| LiTiO3 | 22.68 | 4.76 | 0.43 | 123.53 | 15.88 | 0.58 |
| NaTiO3 | 3.14 | 1.77 | 0.08 | 188.80 | 25.76 | 1.08 |
| KTiO3 | 31.34 | 5.60 | 0.49 | 100.63 | 12.29 | 0.70 |
| RbTiO3 | 10.60 | 3.26 | 0.28 | 148.60 | 21.66 | 0.45 |
| CsTiO3 | 10.51 | 3.24 | 0.28 | 143.03 | 17.74 | 0.50 |
| Elastic Parameters | LiTiO3 | NaTiO3 | KTiO3 | RbTiO3 | CsTiO3 |
|---|---|---|---|---|---|
| c11 | 287.71 | 205.61 | 310.72 | 149.23 | 210.18 |
| c12 | 77.64 | 61.32 | 76.09 | 95.27 | 77.44 |
| c13 | 104.48 | 18.79 | 67.85 | 85.52 | 45.29 |
| c14 | ⸺ | ⸺ | ⸺ | 0.533 | ⸺ |
| c15 | ⸺ | 0.40 | ⸺ | -6.49 | ⸺ |
| c22 | 226.00 | 199.69 | ⸺ | ⸺ | ⸺ |
| c23 | 76.41 | 22.81 | ⸺ | ⸺ | ⸺ |
| c25 | ⸺ | 0.93 | ⸺ | ⸺ | ⸺ |
| c33 | 255.03 | 51.94 | 210.30 | 169.40 | 43.86 |
| c35 | ⸺ | 2.25 | ⸺ | ⸺ | |
| c44 | 60.61 | 11.52 | 72.27 | 40.05 | 50.17 |
| c46 | ⸺ | 2.06 | ⸺ | ⸺ | ⸺ |
| c55 | 107.38 | 16.98 | ⸺ | ⸺ | ⸺ |
| c66 | 44.82 | 47.61 | 71.92 | 26.98 | 35.50 |
| Bulk modulus (BH) | 141.59 | 59.28 | 137.03 | 111.11 | 66.38 |
| Young’s modulus (EH) | 186.99 | 79.10 | 206.81 | 91.71 | 101.18 |
| Shear modulus (GH) | 73.05 | 30.96 | 82.83 | 33.66 | 40.61 |
| Poisson’s ratio (νH) | 0.28 | 0.28 | 0.25 | 0.36 | 0.25 |
| Pugh’s ratio | 1.94 | 1.91 | 1.65 | 3.30 | 1.63 |
| Cauchy’s pressure | 17.03 | 49.80 | 4.17 | 55.22 | 27.27 |
| Anisotropy factor | 0.57 | 0.16 | 0.61 | 1.48 | 0.75 |
| Minimum thermal conductivity (κmin) | 0.24 | 0.11 | 0.12 | 0.16 | 0.13 |
| Debye temperature (θD) | 711.90 | 453.40 | 684.50 | 398.30 | 380.40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jouybar, S.; Naji, L.; Sarabadani Tafreshi, S.; de Leeuw, N.H. A Density Functional Theory Study of the Physico-Chemical Properties of Alkali Metal Titanate Perovskites for Solar Cell Applications. Molecules 2024, 29, 3355. https://doi.org/10.3390/molecules29143355
Jouybar S, Naji L, Sarabadani Tafreshi S, de Leeuw NH. A Density Functional Theory Study of the Physico-Chemical Properties of Alkali Metal Titanate Perovskites for Solar Cell Applications. Molecules. 2024; 29(14):3355. https://doi.org/10.3390/molecules29143355
Chicago/Turabian StyleJouybar, Shirzad, Leila Naji, Saeedeh Sarabadani Tafreshi, and Nora H. de Leeuw. 2024. "A Density Functional Theory Study of the Physico-Chemical Properties of Alkali Metal Titanate Perovskites for Solar Cell Applications" Molecules 29, no. 14: 3355. https://doi.org/10.3390/molecules29143355
APA StyleJouybar, S., Naji, L., Sarabadani Tafreshi, S., & de Leeuw, N. H. (2024). A Density Functional Theory Study of the Physico-Chemical Properties of Alkali Metal Titanate Perovskites for Solar Cell Applications. Molecules, 29(14), 3355. https://doi.org/10.3390/molecules29143355







