Mechanism of Methyl Transfer Reaction between CH3Co(dmgBF2)2py and PPh3Ni(Triphos)
Abstract
1. Introduction
2. Computational Method
3. Results
3.1. BP86 Calculations without Dispersion
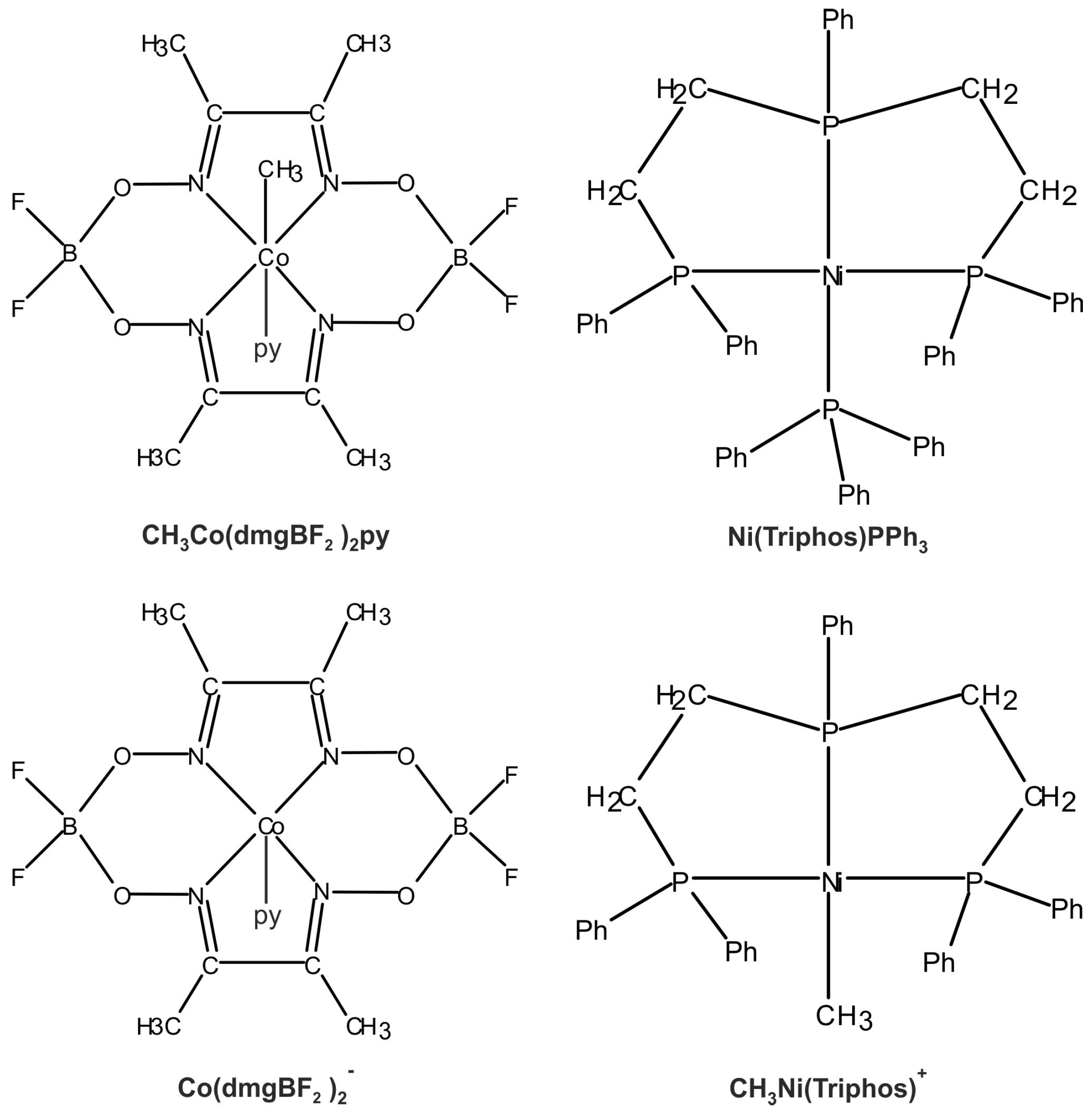
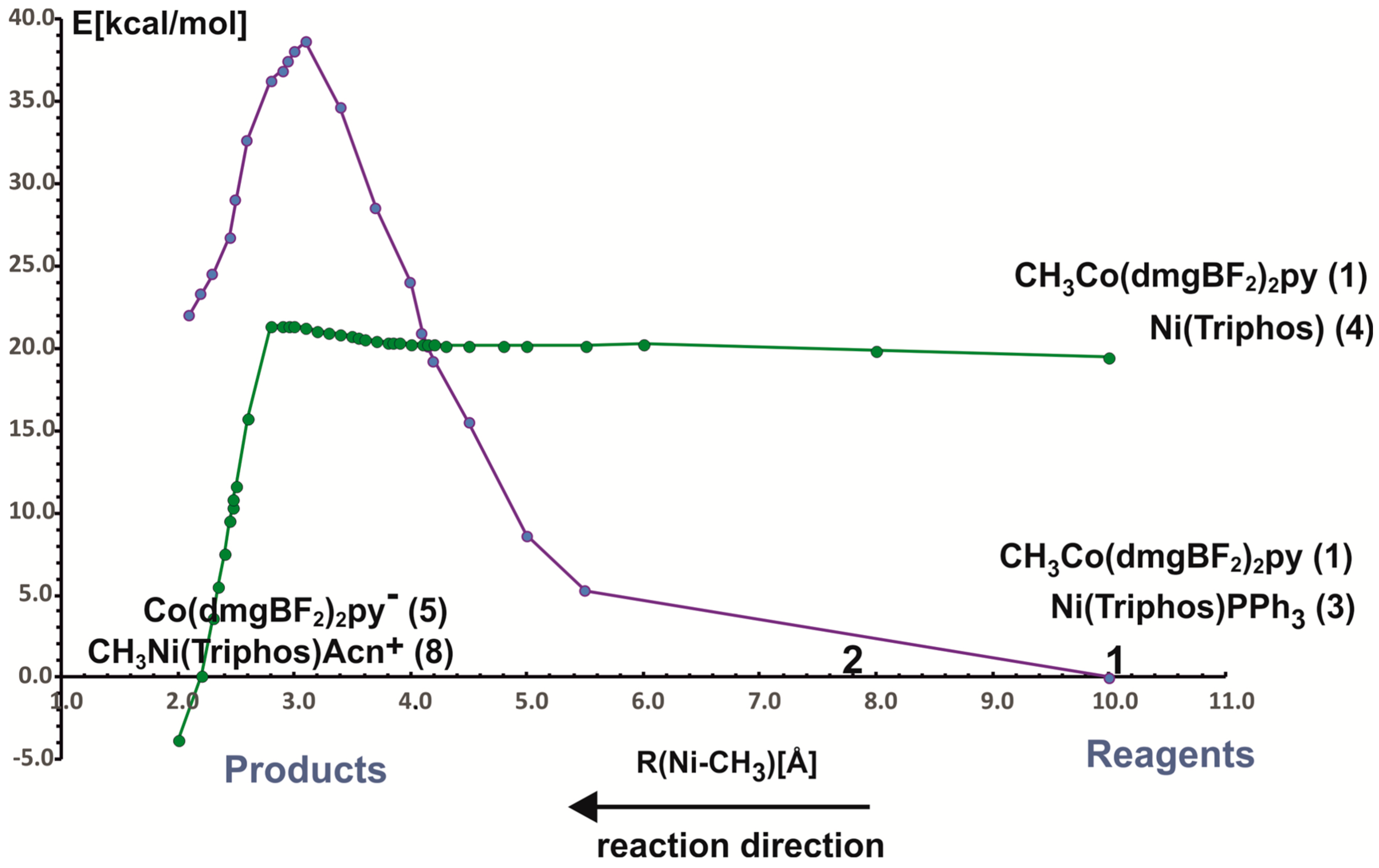
3.1.1. Geometry of Species and Energetics of Reaction Path
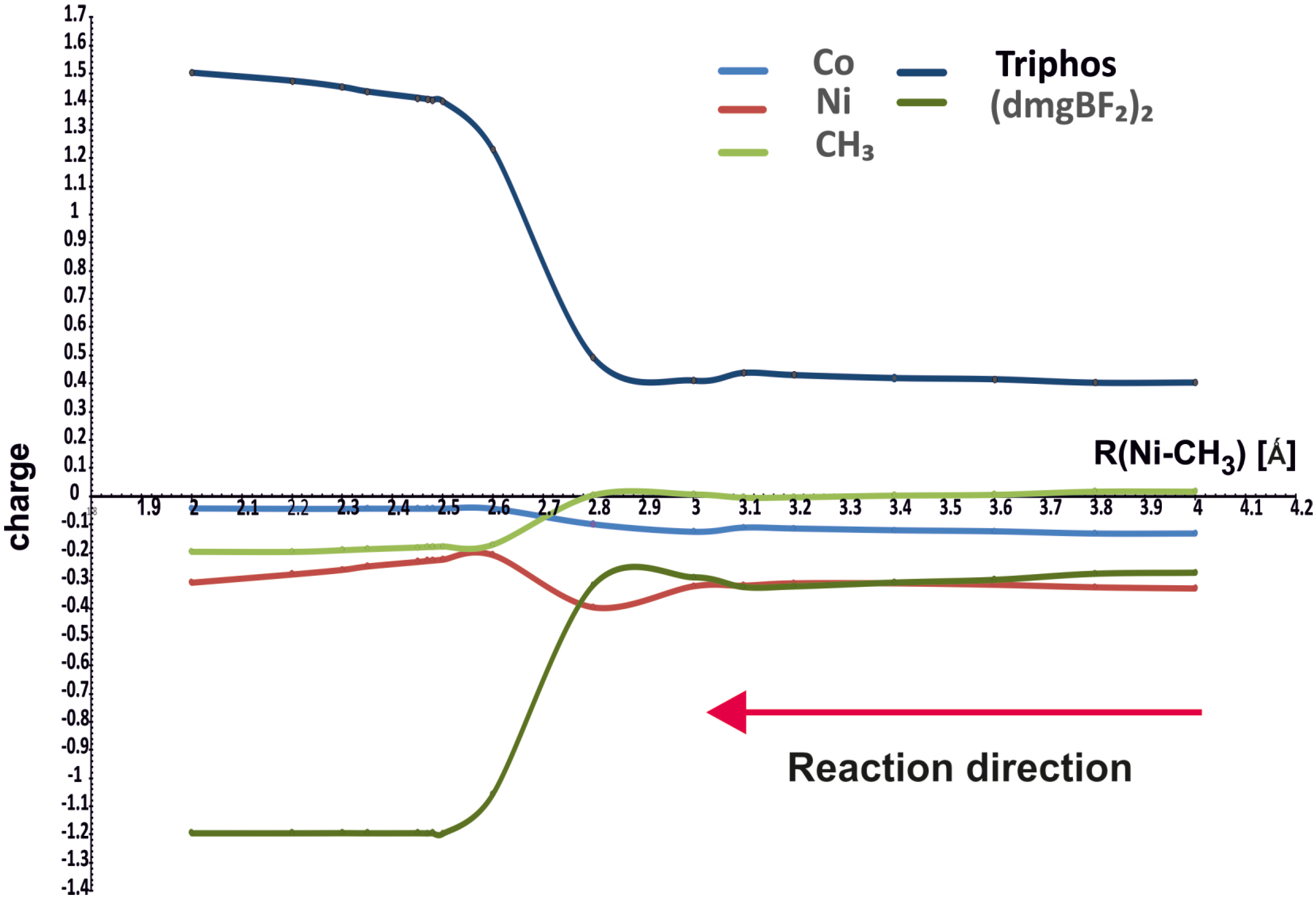
3.1.2. Charges
3.2. DFT with Dispersion Corrections
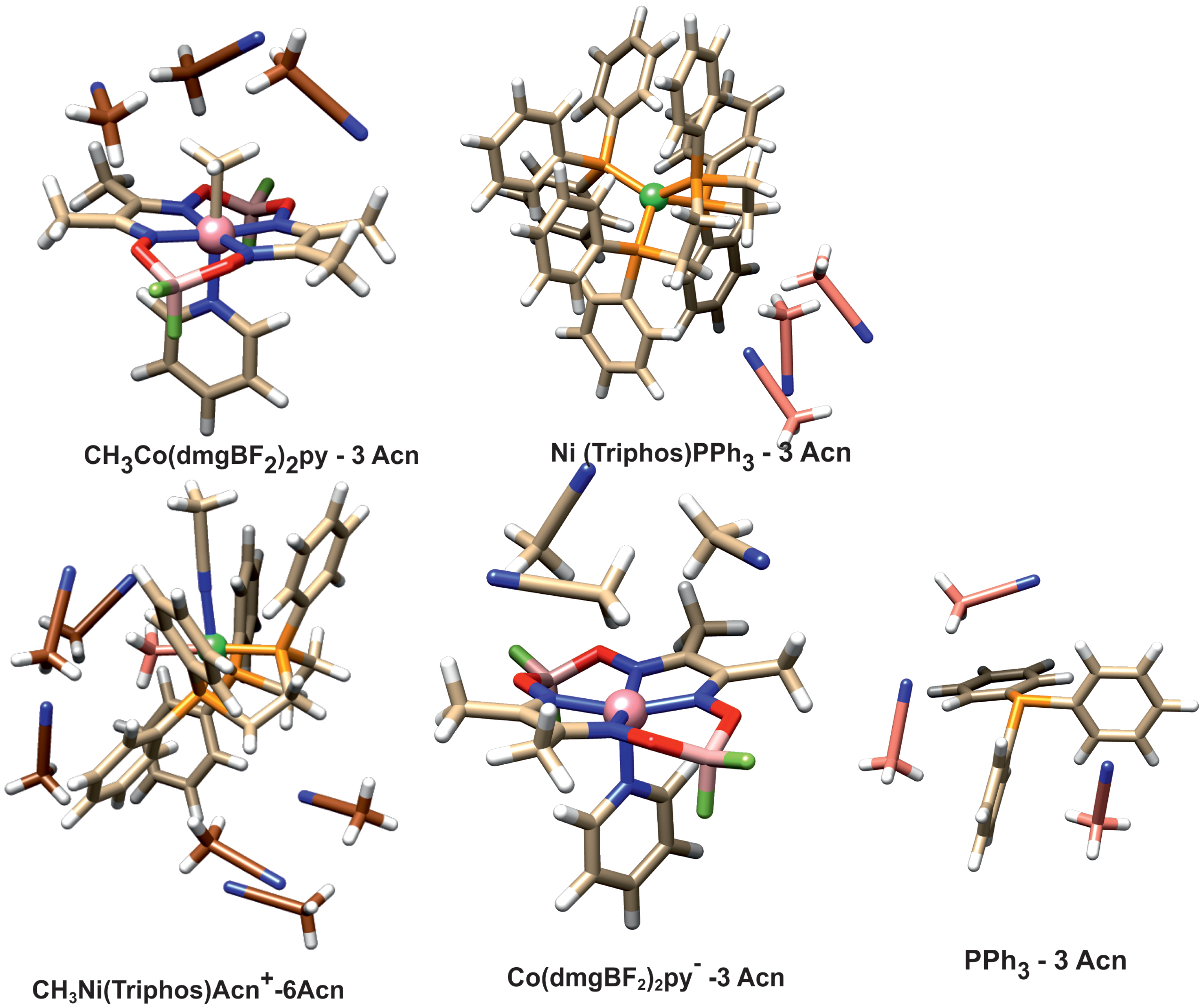
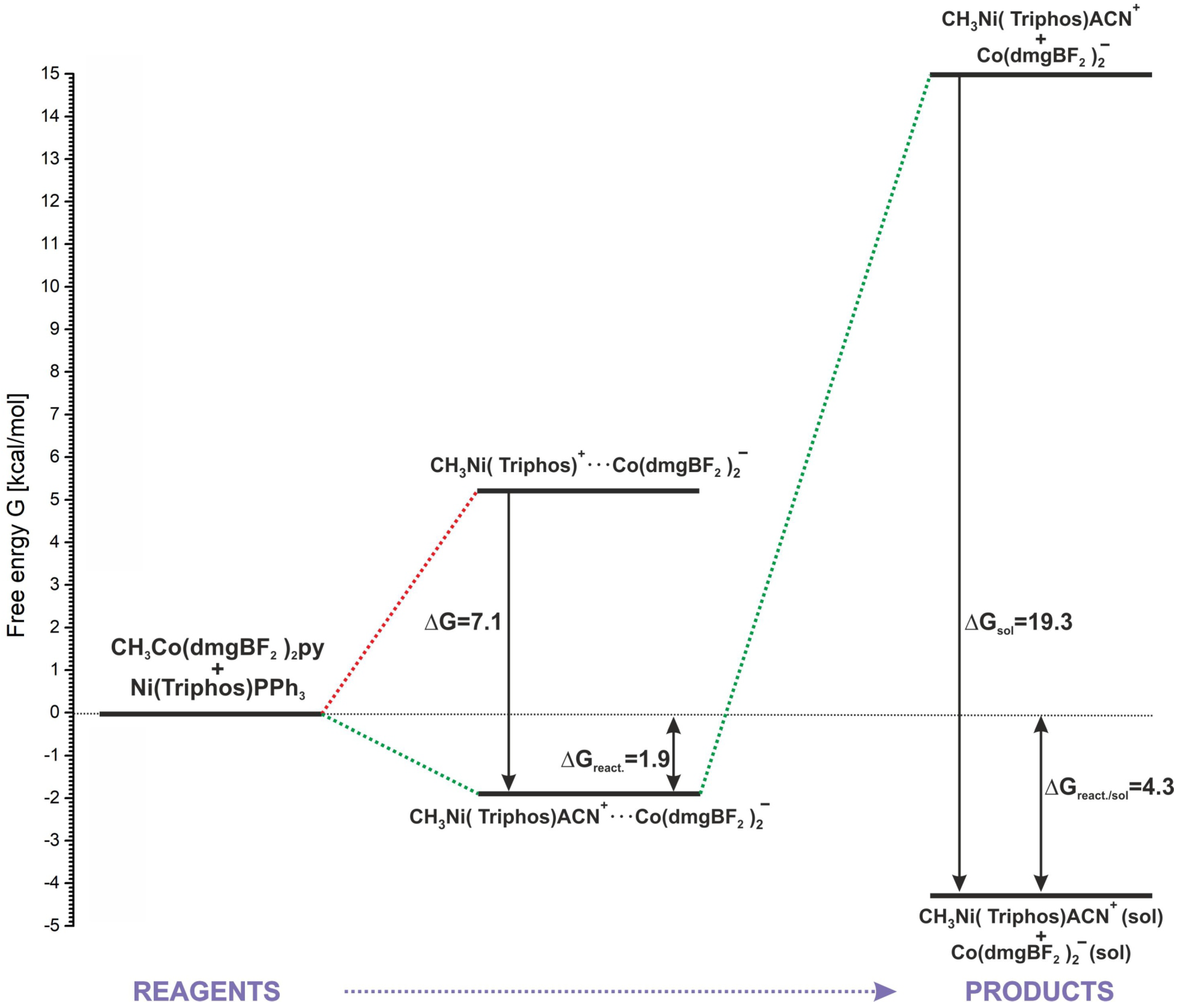
3.3. Solvation of Reagents and Products
4. Discussion
Analysis of Energy of the Methyl Transfer Reaction
5. Conclusions
- The analysis of reaction energy reveals that acetonitrile solvent participates actively in the course of the reaction by coordination with cobalt and nickel centers.
- The dispersion corrections influence energetics and the mechanism of the reaction by forming the associated product complex and impeding the dissociation of the phospine ligand.
- The methyl group binding energy is larger with nickel than with cobalt, which is in favor of product formation. However, the high binding energy of the phosphine group in the starting nickel complex has an unfavorable impact on the course of the reaction.
- The calculated energy shows that the reaction is basically endothermic. However, the solvation strongly stabilizes the reaction products.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| py | pyridine |
| acn | acetonitrile |
| dmg | dimethylglioxyme |
| Triphos | Bis(diphenylphosphinoethyl)phenylphosphine |
| PEC | Potential Energy Curve |
| NBO | Natural Bond Orbital |
References
- Matthews, R.; Koutmos, M.; Datta, S. Cobalamin–dependent and cobamide–dependent methyltransferases. Curr. Opin. Struct. Biol. 2008, 18, 658–666. [Google Scholar] [CrossRef]
- Matthews, R.G. Cobalamin- and corrinoid-dependent enzymes. Met. Ions Life Sci. 2009, 6, 53–114. [Google Scholar]
- Jensen, K.P.; Ryde, U. Cobalamins uncovered by modern electronic structure calculations. Coord. Chem. Rev. 2009, 253, 769–778. [Google Scholar] [CrossRef]
- Goetzl, S.; Jeoung, J.H.; Hennig, S.; Dobbek, H. Structural basis for electron and methyl-group transfer in a methyltransferase system operating in the reductive acetyl-CoA pathway. J. Mol. Biol. 2011, 411, 96–109. [Google Scholar] [CrossRef] [PubMed]
- Schrapers, P.; Mebs, S.; Goetzl, S.; Hennig, S.; Dau, H.; Dobbek, H.; Haumann, M. Axial ligation and redox changes at the cobalt ion in cobalamin bound to Corrinoid Iron-Sulfur Protein (CoFeSP) or in solution characterized by XAS and DFT. PLoS ONE 2016, 11, e0158681. [Google Scholar] [CrossRef] [PubMed]
- Svetlitchnaia, T.; Svetlitchnyi, V.; Meyer, O.; Dobbek, H. Structural insights into methyltransfer reactions of a corrinoid iron-sulfur protein involved in acetyl-CoA synthesis. Proc. Natl. Acad. Sci. USA 2006, 103, 14331–14336. [Google Scholar] [CrossRef]
- Ragsdale, S.W.; Pierce, E. Acetogenesis and the Wood-Ljungdahl pathway of CO2 fixation. Biochim. Biophys. Acta 2008, 1784, 1873–1898. [Google Scholar] [CrossRef] [PubMed]
- Ragsdale, S. Life with Carbon Monoxide. Crit. Rev. Biochem. Mol. Biol. 2004, 39, 165–195. [Google Scholar] [CrossRef]
- Drennan, C.L.; Doukov, T.I.; Ragsdale, S.W. The metalloclusters of carbon monoxide dehydrogenase/Acetyl-CoA synthase: A story in pictures. J. Biol. Inorg. Chem. 2004, 9, 511–515. [Google Scholar] [CrossRef]
- Lindahl, P.A. Acetyl-coenzyme A synthase: The case for a Nip(0)—Based mechanism of catalysis. J. Biol. Inorg. Chem. 2004, 9, 516–524. [Google Scholar] [CrossRef]
- Gencic, S.; Grahame, D. Two separate one–electron steps in the reductive activation of the A cluster in subunit beta of the ACDS complex in Methanosarcina thermophila. Biochemistry 2008, 47, 5544–5555. [Google Scholar] [CrossRef] [PubMed]
- Hsiao, Y.M.; Chojnacki, S.S.; Hinton, P.; Reibenspies, J.H.; Darensbourg, M.Y. Organometallic chemistry of Sulfur/Phosphorus donor ligand complexes of Nickel(II) and Nickel(0). Organometallics 1993, 12, 870–875. [Google Scholar] [CrossRef]
- Tan, X.; Surovtsev, I.V.; Lindahl, P.A. Kinetics of CO insertion and acetyl group transfer steps, and a model of the acetyl–CoA synthase catalytic mechanism. J. Am. Chem. Soc. 2006, 128, 12331–12338. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Seravalli, J.; Kumar, M.; Ragsdale, S.W. Rapid kinetic studies of Acetyl-CoA synthesis: Evidence supporting the catalytic intermediacy of a paramagnetic NiFeC species in the autotrophic Wood-Ljungdahl pathway. Biochemistry 2002, 41, 1807–1819. [Google Scholar] [CrossRef] [PubMed]
- Seravalli, J.; Ragsdale, S.W. Pulse–chase studies of the synthesis of acetyl—CoA by carbon monoxide dehydrogenase/acetyl–CoA synthase: Evidence for a random mechanism of methyl and carbonyl addition. J. Biol. Chem. 2008, 283, 8384–8394. [Google Scholar] [CrossRef] [PubMed]
- Ito, M.; Kotera, M.; Matsumoto, T.; Tatsumi, K. Dinuclear nickel complexes modeling the structure and function of the acetyl CoA synthase active site. Proc. Natl. Acad. Sci. USA 2009, 106, 11862–11866. [Google Scholar] [CrossRef] [PubMed]
- Rigo, P.; Bressan, M.; Basato, M. Nickel(0) complexes with the hybrid bidentate ligand 1-(thioethyl)-2-(diphenylphosphino)ethane. Synthesis and catalytic properties of the related nickel hydride derivative. Inorg. Chem. 1979, 18, 860–863. [Google Scholar] [CrossRef]
- Stavropoulos, P.; Muetterties, M.C.; Carrie, M.; Holm, R.H. Structural and reaction chemistry of nickel complexes in relation to carbon monoxide dehydrogenase: A reaction system simulating acetyl–coenzyme A synthase activity. J. Am. Chem. Soc. 1991, 113, 8485–8492. [Google Scholar] [CrossRef]
- Kitto, H.J.; Rae, A.D.; Webster, R.D.; Willis, A.C.; Wild, S.B. Synthesis, structure, and electrochemistry of di- and zerovalent Nickel, Palladium, and Platinum monomers and dimers derived from an Enantiopure (S,S)-tetra(tertiary phosphine). Inorg. Chem. 2007, 46, 8059–8070. [Google Scholar] [CrossRef]
- Matsumoto, T.; Ito, M.; Kotera, M.; Tatsumi, K. A dinuclear nickel complex modeling of the Nid(ii)–Nip(i) state of the active site of acetyl CoA synthase. Dalton Trans. 2010, 39, 2995–2997. [Google Scholar] [CrossRef]
- Ram, M.S.; Riordan, C.G.; Yap, G.P.A.; Liable-Sands, L.; Rheingold, A.L.; Marchaj, A.; Norton, J.R. Kinetics and mechanism of alkyl transfer from organocobalt(III) to nickel(I): Implications for the synthesis of acetyl coenzyme A by CO dehydrogenase. J. Am. Chem. Soc. 1997, 119, 1648–1655. [Google Scholar] [CrossRef]
- Selvan, D.; Chakraborty, S. A De Novo Designed Trimeric Metalloprotein as a Nip Model of the Acetyl-CoA Synthase. Int. J. Mol. Sci. 2023, 24, 317. [Google Scholar] [CrossRef] [PubMed]
- Golden, M.L.; Whaley, C.M.; Rampersad, M.V.; Reibenspies, J.H.; Hancock, R.D.; Darensbourg, M.Y. N2S2Ni metallodithiolate complexes as ligands: Structural and aqueous solutionquantitative studies of the ability of metal ions to form M-S-Ni bridges to Mercapto groups coordinated to Nickel(II). Implications for Acetyl Coenzyme A synthase. Inorg. Chem. 2005, 44, 875–883. [Google Scholar] [CrossRef] [PubMed]
- Webster, C.E.; Darensbourg, M.Y.; Lindahl, P.A.; Hall, M.B. Structures and energetics of models for the active site of acetyl–Coenzyme A synthase: Role of distal and proximal metals in catalysis. J. Am. Chem. Soc. 2004, 126, 3410–3411. [Google Scholar] [CrossRef] [PubMed]
- Harrop, T.C.; Olmstead, M.M.; Mascharak, P.K. Synthetic analogues of the active site of the A-cluster of Acetyl Coenzyme A synthase/CO dehydrogenase: Syntheses, structures, and reactions with CO. Inorg. Cdrohem. 2006, 45, 3424–3436. [Google Scholar] [CrossRef] [PubMed]
- Dougherty, W.G.; Rangan, K.; O’Hagan, M.J.; Yap, G.P.; Riordan, C.G. Binuclear complexes containing a methylnickel moiety: Relevance to organonickel intermediates in acetyl–coenzyme A synthase catalysis. J. Am. Chem. Soc. 2008, 130, 13510–13511. [Google Scholar] [CrossRef] [PubMed]
- Krishnan, R.; Riordan, C.G. Cys-Gly-Cys Tripeptide complexes of Nickel: Binuclear analogues for the catalytic site in Acetyl Coenzyme A synthase. J. Am. Chem. Soc. 2004, 126, 4484–4485. [Google Scholar] [CrossRef] [PubMed]
- Green, K.N.; Brothers, S.M.; Jenkins, R.M.; Carson, C.E.; Grapperhaus, C.A.; Darensbourg, M.Y. An experimental and computational study of Sulfur–modified nucleophilicity in a dianionic NiN2S2 complex. Inorg. Chem. 2007, 46, 7536–7544. [Google Scholar] [CrossRef] [PubMed]
- Schenker, R.; Mock, M.T.; Kieber-Emmons, M.T.; Riordan, C.G.; Brunold, T.C. Spectroscopic and computational studies on [Ni(tmc)CH3]OTf: Implications for Ni–methyl bonding in the A cluster of acetyl–CoA synthase. Inorg. Chem. 2005, 14, 3605–3617. [Google Scholar] [CrossRef]
- Schenker, R.; Kieber-Emmons, M.T.; Riordan, C.G.; Brunold, T.C. Spectroscopic and computational studies on the trans-Mu-1,2-peroxo-bridged dinickel(II) species [Ni(tmc)2(O2)](OTf)2: Nature of end-on peroxo-nickel(II) bonding and comparison with peroxo-copper(II) bonding. Inorg. Chem. 2005, 44, 1752–1762. [Google Scholar] [CrossRef]
- Eckert, N.A.; Dougherty, W.G.; Yap, G.P.A.; Riordan, C.G. Methyl transfer from methylcobaloxime to (Triphos)Ni(PPh3): Relevance to the mechanism of acetyl–coenzyme A synthase. J. Am. Chem. Soc. 2007, 129, 9286–9287. [Google Scholar] [CrossRef] [PubMed]
- Zhou, D.L.; Walder, P.; Scheffold, R.; Walder, L. SN2 or electron transfer? A new technique discriminates the mechanisms of oxidative addition of alkyl halides to corrinato- and porphyrinatocobalt(I). Helv. Chim. Acta 1992, 75, 995–1011. [Google Scholar] [CrossRef]
- Banerjee, S.; Ragsdale, S.W. The many faces of Vitamin B12: Catalysis by cobalamin-dependent enzymes. Annu. Rev. Biochem. 2003, 72, 209–247. [Google Scholar] [CrossRef] [PubMed]
- Sitek, P.; Chmielowska, A.; Jaworska, M.; Lodowski, P.; Szczepańska, M. Theoretical study of cobalt and nickel complexes involved in methyl transfer reactions: Structures, redox potentials and methyl binding energies. Struct. Chem. 2019, 30, 1957–1970. [Google Scholar] [CrossRef]
- Sitek, P.; Jaworska, M.; Lodowski, P.; Chmielowska, A. Methyl transfer reaction between MeI and Ni(PPh2CH2CH2SEt2) complex. A DFT study. Inorg. Chem. Commun. 2013, 29, 65–69. [Google Scholar] [CrossRef]
- Chen, S.L.; Siegbahn, P.E.M. Insights into the Chemical Reactivity in Acetyl–CoA Synthase. Inorg. Chem. 2020, 59, 15167–15179. [Google Scholar] [CrossRef] [PubMed]
- Amara, P.; Volbeda, A.; Fontecilla-Camps, J.C.; Field, M.J. A Quantum Chemical Study of the Reaction Mechanism of Acetyl–Coenzyme A Synthase. J. Am. Chem. Soc. 2005, 127, 2776–2784. [Google Scholar] [CrossRef]
- Siegbahn, P.E.M.; Chen, S.L.; Liao, R.Z. Theoretical Studies of Nickel–Dependent Enzymes. Inorganics 2019, 7, 95. [Google Scholar] [CrossRef]
- Jaworska, M.; Lodowski, P. Theoretical Studies of Acetyl–CoA Synthase Catalytic Mechanism. Catalysts 2022, 12, 195. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian˜16 Revision C.01; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Neese, F. The ORCA program system. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Neese, F. Software update: The ORCA program system—Version 5.0. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2022, 12, e1606. [Google Scholar] [CrossRef]
- Neese, F. Software update: The ORCA program system, version 4.0. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2018, 8, e1327. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P. Density-functional approximation for the correlation energy of the inhomogeneous electron gas. Phys. Rev. B 1986, 33, 8822–8824. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, A.; Huber, C.; Ahlrichs, R. Fully optimized contracted Gaussian basis sets of triple zeta valence quality for atoms Li to Kr. J. Chem. Phys. 1994, 100, 5829–5835. [Google Scholar] [CrossRef]
- Jensen, K.P.; Ryde, U. Theoretical Prediction of the Co–C Bond Strength in Cobalamins. J. Phys. Chem. A 2003, 107, 7539–7545. [Google Scholar] [CrossRef]
- Dölker, N.; Maseras, F.; Lledös, A. A density functional study on the effect of the trans axial ligand of cobalamin on the homolytic cleavage of the Co—C bond. J. Phys. Chem. B 2001, 105, 7564–7571. [Google Scholar] [CrossRef]
- Jensen, K.P.; Ryde, U. The axial N–base has minor influence on Co—C bond cleavage in Cobalamins. J. Mol. Struct. 2002, 585, 239–255. [Google Scholar] [CrossRef]
- Emelyanova, N.S.; Sanina, N.A.; Shestakov, A.F. DFT calculations of the redox potentials for the nitrosyl complexes [Fe2(l-RS)2(NO)4] with R 5 alkyl. Int. J. Quantum Chem. 2013, 113, 740–744. [Google Scholar] [CrossRef]
- Emelyanova, N.; Sanina, N.; Krivenko, A.; Manzhos, R.; Bozhenko, K.; Aldoshin, S. Comparison of pure and hybrid DFT functionals for geometry optimization and calculation of redox potentials for iron nitrosyl complexes with l–SCN bridging ligands. Theor. Chem. Accounts 2013, 132, 1316–1318. [Google Scholar] [CrossRef]
- Castro, L.; Buühl, M. Calculations of one–electron redox potentials of oxoiron(IV) porphyrin complexes. J. Chem. Theory Comput. 2014, 10, 243–251. [Google Scholar] [CrossRef]
- Cossi, M.; Rega, N.; Scalmani, G.; Barone, V. Energies, structures, and electronic properties of molecules in solution with the C-PCM solvation model. J. Comput. Chem. 2003, 24, 669–681. [Google Scholar] [CrossRef]
- Tomasi, J.; Mennucci, B.; Cammi, R. Quantum mechanical continuum solvation models. Chem. Rev. 2005, 105, 2999–3073. [Google Scholar] [CrossRef]
- Himo, F.; Noodleman, L.; Blomberg, M.R.A.; Siegbahn, P.E.M. Relative Acidities of Ortho–Substituted Phenols, as Models for Modified Tyrosines in Proteins. J. Phys. Chem. A 2002, 106, 8757–8761. [Google Scholar] [CrossRef]
- Ehrlich, S.; Moellmann, J.; Reckien, W.; Bredow, T.; Grimme, S. System-dependent dispersion coefficients for the DFT-D3 treatment of adsorption processes on ionic surfaces. ChemPhysChem 2011, 12, 3414–3420. [Google Scholar] [CrossRef] [PubMed]
- Schröder, H.; Creon, A.; Schwabe, T. Reformulation of the D3(Becke-Johnson) Dispersion Correction without Resorting to Higher than C6 Dispersion Coefficients. J. Chem. Theory Comput. 2015, 11, 3163–3170. [Google Scholar] [CrossRef]
- Caldeweyher, E.; Mewes, J.M.; Ehlert, S.; Grimme, S. Extension and evaluation of the D4 London-dispersion model for periodic systems. Phys. Chem. Chem. Phys. 2020, 22, 8499–8512. [Google Scholar] [CrossRef]
- Vydrov, O.A.; Van Voorhis, T. Benchmark assessment of the accuracy of several Van der Waals density functionals. J. Chem. Theory Comput. 2012, 8, 1929–1934. [Google Scholar] [CrossRef] [PubMed]
- Hujo, W.; Grimme, S. Performance of the van der waals density functional VV10 and (hybrid)GGA variants for thermochemistry and noncovalent interactions. J. Chem. Theory Comput. 2011, 7, 3866–3871. [Google Scholar] [CrossRef]
- Reed, A.E.; Curtiss, L.A.; Weinhold, F. Intermolecular interactions from a natural bond orbital, donor-acceptor viewpoint. Chem. Rev. 1988, 88, 899–926. [Google Scholar] [CrossRef]
- Plata, R.E.; Singleton, D.A. A case study of the mechanism of alcohol-mediated morita baylis-hillman reactions. the importance of experimental observations. J. Am. Chem. Soc. 2015, 137, 3811–3826. [Google Scholar] [CrossRef] [PubMed]
- Kua, J.; Krizner, H.E.; De Haan, D.O. Thermodynamics and kinetics of imidazole formation from glyoxal, methylamine, and formaldehyde: A computational study. J. Phys. Chem. A 2011, 115, 1667–1675. [Google Scholar] [CrossRef] [PubMed]
- Tantillo, D.J. Portable Models for Entropy Effects on Kinetic Selectivity. J. Am. Chem. Soc. 2022, 144, 13996–14004. [Google Scholar] [CrossRef] [PubMed]
- Besora, M.; Vidossich, P.; Lledós, A.; Ujaque, G.; Maseras, F. Calculation of Reaction Free Energies in Solution: A Comparison of Current Approaches. J. Phys. Chem. A 2018, 122, 1392–1399. [Google Scholar] [CrossRef] [PubMed]
- Barone, V.; Bencini, A.; Cossi, M.; Di Matteo, A.; Mattesini, M.; Totti, F. Assessment of a Combined QM/MM Approach for the Study of Large Nitroxide Systems in Vacuo and in Condensed Phases. J. Am. Chem. Soc. 1998, 120, 7069–7078. [Google Scholar] [CrossRef]
- Garza, A.J. Solvation Entropy Made Simple. J. Chem. Theory Comput. 2019, 15, 3204–3214. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.C.; Zhu, X.R.; Liu, D.Y.; Fang, D.C. DFT calculations in solution systems: Solvation energy, dispersion energy and entropy. Phys. Chem. Chem. Phys. 2023, 25, 913–931. [Google Scholar] [CrossRef] [PubMed]
- Shin, W.; Yang, Z.J. Computational Strategies for Entropy Modeling in Chemical Processes. Chem. Asian J. 2023, 18, e202300117. [Google Scholar] [CrossRef] [PubMed]
- Oszajca, M.; Drabik, G.; Rado n, M.; Franke, A.; van Eldik, R.; Stochel, G. Experimental and Computational Insight into the Mechanism of NO Binding to Ferric Microperoxidase. The Likely Role of Tautomerization to Account for the pH Dependence. Inorg. Chem. 2021, 60, 15948–15967. [Google Scholar] [CrossRef]
- Wertz, D.H. Relationship between the gas-phase entropies of molecules and their entropies of solvation in water and 1-octanol. J. Am. Chem. Soc. 1980, 102, 5316–5322. [Google Scholar] [CrossRef]



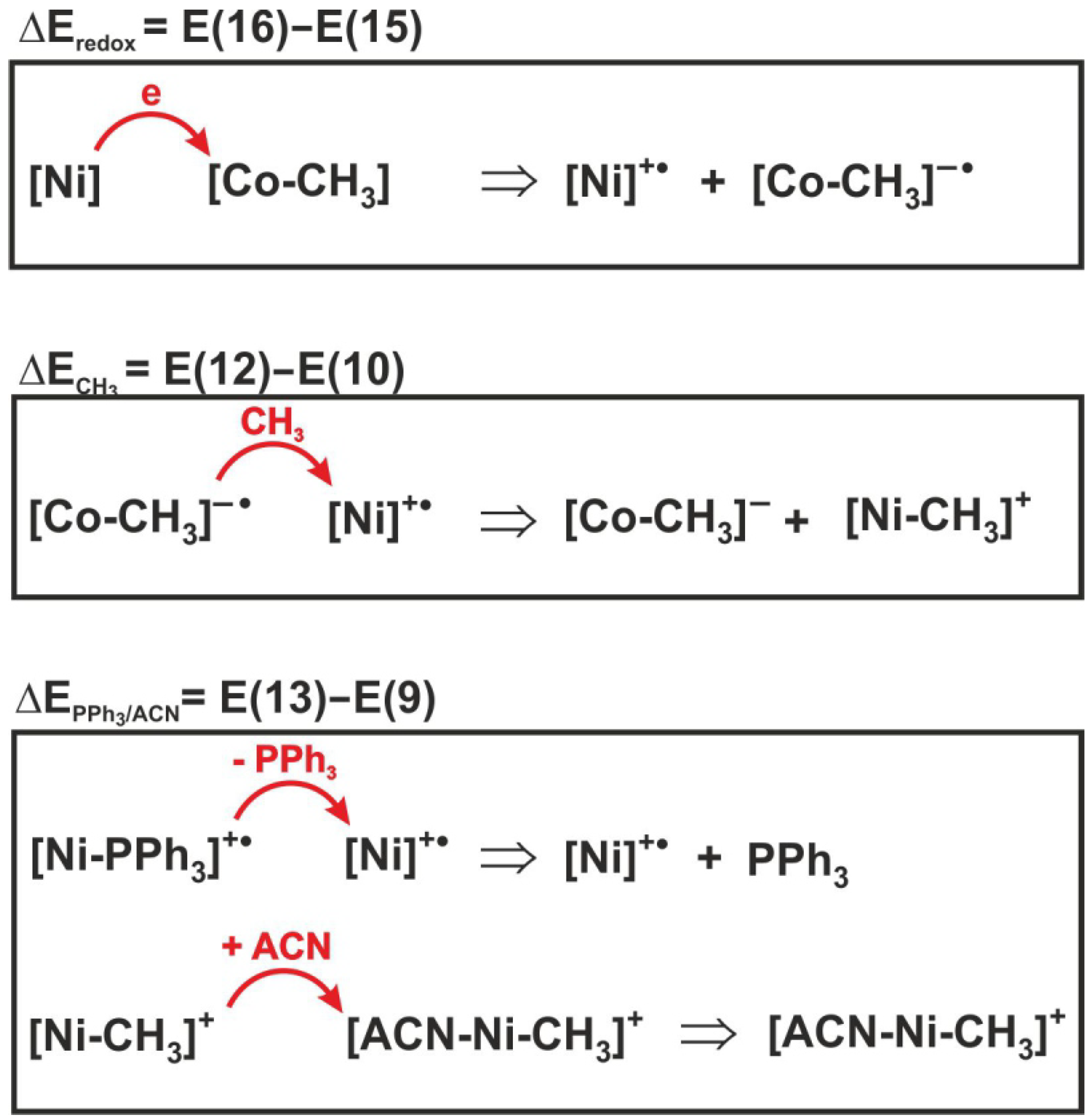
| Reaction | BP86 | PBE/D3BJ | PBE/D3BJ |
|---|---|---|---|
| ΔE | ΔE | ΔG | |
| (4) | 0.4 | −30.7 | −22.88 |
| (5) | – | −12.2 | −7.08 |
| (6) | 3.8 | −17.7 | −15.01 |
| (7) | – | 1.1 | 1.85 |
| Solvation | ||
|---|---|---|
| Energy | ||
| Products | PPh3 − 3 Acn | 8.40 |
| Co(dmgBF2)2py− − 3Acn | 9.71 | |
| CH3Ni(Triphos)Acn+ − 6Acn | 19.52 | |
| Reagents | CH3Co(dmgBF2)2py + − 3Acn | 6.13 |
| Ni(Triphos)PPh3 − 3Acn | 4.21 | |
| Solvation | ||
| Stabilization | 27.29 | |
| energy |
| BP86 | PBE-D3BJ | ||
|---|---|---|---|
| Reaction | ΔE | ΔE | ΔG |
| Total reaction energy | |||
| (18) | −1.5 | −27.7 | −22.94 |
| (19) | – | −8.9 | −6.08 |
| Elementary reaction energy | |||
| (8) | 19.4 | 50.9 | 42.54 |
| (9) | 11.6 | 44.4 | 36.82 |
| (10) | 19.6 | 26.6 | 19.68 |
| (11) | 39.3 | 47.0 | 36.46 |
| (12) | 47.9 | 56.6 | 48.07 |
| (13) | 3.4 | 13.0 | 7.86 |
| (14) | 5.3 | 10.0 | 7.93 |
| (15) (a) | −0.3 | −0.5 | −0.42 |
| (16) (b) | −1.0 | −1.2 | −1.04 |
| E(6) | |||||
|---|---|---|---|---|---|
| eV (kcal/mol) | |||||
| BP86 | E | 3.8 | −0.7 (−16.1) | 28.3 | −8.2 |
| BP86-D3BJ | E | −25.1 | −0.59 (−13.6) | 32.0 | −44.4 |
| PBE | E | −0.4 | −0.7 (−16.1) | 28.5 | −12.4 |
| PBE-D3BJ | E | −17.7 | −0.7 (−16.1) | 30.0 | −31.4 |
| PBE-D3BJ | G | −15.0 | −0.62 (−14.3) | 28.39 | −28.96 |
| PBe-D4 | E | −17.6 | −0.7 (−16.1) | 32.1 | −34.1 |
| PBE-SCNL | E | −16.6 | −0.7 (−16.1) | 31.6 | −31.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sitek, P.; Lodowski, P.; Jaworska, M. Mechanism of Methyl Transfer Reaction between CH3Co(dmgBF2)2py and PPh3Ni(Triphos). Molecules 2024, 29, 3335. https://doi.org/10.3390/molecules29143335
Sitek P, Lodowski P, Jaworska M. Mechanism of Methyl Transfer Reaction between CH3Co(dmgBF2)2py and PPh3Ni(Triphos). Molecules. 2024; 29(14):3335. https://doi.org/10.3390/molecules29143335
Chicago/Turabian StyleSitek, Patrycja, Piotr Lodowski, and Maria Jaworska. 2024. "Mechanism of Methyl Transfer Reaction between CH3Co(dmgBF2)2py and PPh3Ni(Triphos)" Molecules 29, no. 14: 3335. https://doi.org/10.3390/molecules29143335
APA StyleSitek, P., Lodowski, P., & Jaworska, M. (2024). Mechanism of Methyl Transfer Reaction between CH3Co(dmgBF2)2py and PPh3Ni(Triphos). Molecules, 29(14), 3335. https://doi.org/10.3390/molecules29143335









