Screening the Optimal Probe by Expounding the ESIPT Mechanism and Photophysical Properties in Bis-HBX with Multimodal Substitutions

Abstract

DFT and TD-DFT were used in this article to investigate the effects of different substitutions at multiple sites on the photophysical mechanism of bis-HBX in the gas phase. Four different substitution modes were selected, denoted as A₁ (X=Me, Y=S), A₂ (X=OMe, Y=S), B₁ (X=Me, Y=NH), and C₁ (X=Me, Y=O). The geometric parameters proved that the IHBs enhanced after photoexcitation, which was conducive to promote the ESIPT process. Combining the analysis of the PECs, it was revealed that the bis-HBX molecule underwent the ESIPT process, and the ease of the ESIPT process was in the order of A₁ > A₂> B₁ > C₁. In particular, the TICT process in A₁ and B₁ promoted the occurrence of the ESIPT process. In addition, the IC process was identified, particularly in C₁. Meanwhile, the calculation of fluorescence lifetime and fluorescence rate further confirmed that A₁ was the most effective fluorescent probe molecule. This theoretical research provides an innovative theoretical reference for regulating ESIPT reactions and optimizing fluorescent probe molecules.

1. Introduction

The excited-state intramolecular proton transfer (ESIPT) process is a photo-induced enol-keto tautomerization process [1,2,3] accompanied by strong intramolecular hydrogen bonds (IHBs) [4,5], which allows proton H from specific amino or hydroxyl atoms to transfer to carbonyl oxygen or azo nitrogen atoms after photoexcitation. As shown in Scheme 1, the integral ESIPT reaction is essentially a four-level photo-cycle process. Firstly, the enol form in the ground state is photoexcited to the Franck–Condon point of the S₁ state, generating the enol* form simultaneously. Secondly, the enol* form undergoes the ESIPT process to produce a tautomer keto (keto*) form. Thirdly, the keto* form relaxes back to the ground-state keto structure via releasing the fluorescence or non-radiative transition. Eventually, the keto form is converted to the initial thermodynamically stable enol form achieved by the reverse proton transfer (RPT) process. Generally speaking, the ESIPT system tends to exhibit a large Stokes shift and dual fluorescence [6,7,8]. In the 1950s, Weller first observed the double fluorescence phenomenon during an experimental study on the dynamic characteristics of methyl salicylate and attributed it to the ESIPT reaction [9]. Since then, the mechanism of the ESIPT reaction has attracted the attention of extensive researchers, most of whom have studied it experimentally and theoretically [10,11,12,13].

2-(2′-hydroxyphenyl) benzazole (HBX) derivatives with ESIPT properties are typically characterized by high yield and low synthesis complexity [14]. This distinctive nature makes them widely used in laser dyes [15], chemical sensors [16,17], molecular switches [18], light-emitting diode devices [19], and fluorescent probes for use in biological systems [20,21]. Previous reports have shown that modifying the molecular structure can effectively regulate the ESIPT process of HBX derivatives. In 2017, Manojai et al. [22] investigated the effect of heteroatom substitution (X=NH, O, and S) on the photophysical properties of HBX derivatives. They proved that NH substitution increased the probability of the ESIPT process in HBT, resulting in redshift emission fluorescence. In 2020, yang et al. [23] probed the effects of -NH₂ group substitutions at different positions on the ESIPT mechanism. They revealed that the para-substitution of the -NH₂ group promoted the occurrence of the ESIPT process. In 2022, Zhang et al. [24] designed four molecules (BPN, BPNS, BPS, and BPSN) to explore the effects of different electron groups on the ESIPT process in depth. They believed that introducing electron-withdrawing groups accelerates the ESIPT process, while the electron-donating groups do the opposite. It should not be ignored that in previous studies on HBX derivatives, researchers mainly focused on the effects of substitutions on the mono-HBX [22,23,24,25]. Recently, Lee et al. [26] designed and synthesized bis 2-(2′-hydroxyphenyl) benzazole (bis-HBX) fluorescent probe molecules based on two HBX moieties. The bis-HBX had extra proton-binding sites and was more effective for the detection of alkaline pH than mono-HBX [26,27]. However, the internal mechanism of the effects of different substitutions at multiple sites on the fluorescence properties of bis-HBX was still deficient, and the fluorescence probe molecules with the strongest luminous efficiency were screened, which merits further attention. Therefore, a thorough theoretical investigation was urgently needed to better understand the ESIPT process and the effects of different substitutions at multiple sites on the fluorescence properties of bis-HBX.

In this study, we simultaneously adjusted two key parameters, namely the extension group of central phenol (X: Me or OMe) and the properties of heteroatoms (Y: S, O, N) (as shown in Scheme 2). Using DFT and TD-DFT methods, the effects of different substitutions at multiple sites on the photophysical mechanism and the ESIPT process in bis-HBX were rationally researched. Our research not only provides a new idea and method for improving the efficiency of the ESIPT process but also has an important reference value for designing and optimizing fluorescent probe molecules with high fluorescence efficiency.

2. Results and Discussion

2.1. Geometric Parameters of Bis-HBX

All the geometric configurations of different states of bis-HBX (see Figure 1 and Figure S1) were optimized completely by the B3LYP-D3/6-31G+(d,p) method without any restrictions on bond lengths or bond angles. In the excited state, we labeled the enol* structure as the S₁ state and the keto* structure as the S₁′ state. Some of the significant geometric parameters are displayed in

Table 1. Calculated bond lengths (Å), bond angles (°), and dihedral angles (°) of bis-HBX in different states in the gas phase.

		O1–H1	H1–N1	δ (O1–H1–N1)	δ (C1–C2–C3–N2)
A1	S0	0.994	1.720	147.14	−47.02
	S1	1.058	1.501	153.08	−0.02
	S1′	1.768	1.036	134.02	13.29
A2	S0	0.992	1.727	147.08	46.28
	S1	1.050	1.522	152.73	19.42
	S1′	1.723	1.043	135.84	18.12
B1	S0	0.995	1.712	147.53	−47.60
	S1	1.041	1.549	151.92	−21.94
	S1′	1.883	1.024	127.12	−22.91
C1	S0	0.990	1.764	146.80	0.12
	S1	1.026	1.611	150.90	0.01
	S1′	1.869	1.028	127.24	−0.02

, including bond lengths and bond angles.

The intramolecular hydrogen bonding (IHB) parameters of bis-HBX from the S₀ to the S₁ state followed the similar varying tendency recorded in Table 1. We chose A₁ as a representative for ease of expounding and fully discussing the changing trend in IHB intensity upon photoexcitation. The bond length (O₁–H₁) of the A₁ molecule was elongated from 0.994 Å (S₀) to 1.058 Å (S₁), and N₁-H₁ was decreased from 1.720 Å (S₀) to 1.501 Å (S₁). Furthermore, the bond angle δ (O₁-H₁-N₁) was increased from 147.14° to 153.08°. It has been validated that the IHB (O₁-H₁…N₁) intensity is enhanced in the S₁ state, which is conducive to the ESIPT process [28,29,30]. In addition, in the S₁′ state, we obtained tautomer configuration, and a new IHB (N₁-H₁…O₁) was formed with a bond length of 1.036 Å. The bis-HBX molecule underwent the ESIPT process, which was proved by the above-calculated results.

It is noteworthy that, in contrast to C₁, the dihedral angle δ (C₁–C₂–C₃–N₂) of A₁, A_2, and B₁ existed a torsion between the central benzene ring and the right five-membered ring. The δ (C₁–C₂–C₃–N₂) of A₁, called the dihedral angle, enlarged from 47.02°(S₀) to 13.29° (S₁′), with a torsion of 60.31°. Similarly, both the A₂ and B₁ molecules underwent torsions of 28.16° and 24.69°, respectively. All the alterations indicated that A₁, A₂, and B₁ underwent the ESIPT process with distortions in molecular configuration.

2.2. Infrared (IR) Vibrational Spectra

Due to the IR vibrational spectra providing direct evidence for the formation and fracture of hydrogen bonds (HBs) during the ESIPT process [31], we simulated the IR vibrational spectra of four probes in different electronic states.

From the simulated IR spectra (Figure 2), we found that the stretching vibration frequencies of O₁-H₁ in A₁, A₂, B₁, and C₁ were separately redshifted from 3244 cm⁻¹, 3279 cm⁻¹, 3193 cm⁻¹, and 3322 cm⁻¹ in the S₀ state to 2148 cm⁻¹, 2266 cm⁻¹, 2359 cm⁻¹, and 2654 cm⁻¹ in the S₁ state, accompanied with redshifts of 1096 cm⁻¹, 1013 cm⁻¹, 798 cm⁻¹, and 668 cm⁻¹, respectively. The above data indicated that the redshift value followed the order: A₁ > A₂ > B₁ > C₁, as we all know the weakening and strengthening of the IHB can be explained by the blue- and redshifts in the IR vibrational frequencies of the O-H bond [32,33,34], respectively, so we can infer that the ESIPT process was more prone to occur in A₁. Meanwhile, in the S₁′ state, a new vibrational peak was observed, corresponding to the stretching vibration frequency of N₁–H₁, which was located around 3225 cm⁻¹ (A₁), 3125 cm⁻¹ (A₂), 3425 cm⁻¹ (B₁), and 3374 cm⁻¹ (C₁). The above discussion confirmed that the four probe molecules underwent the ESIPT process after photoexcitation.

2.3. RDG Scatter Plot and Topological Analyses

Considering that using the scatter plot of RDG versus Sign(I₂)r can effectively reveal IHB interactions in real space [35], and Lu et al. recently proposed the IRI isosurface based on RDG versus Sign(I₂)r, the expression of RDG function was as shown in Equation (1) [36,37]:

$$RDG\left(r\right)=\frac{1\left|\nabla \rho \left(r\right)\right|}{2{\left(3{\pi }^2\right)}^{1/3}\rho {\left(r\right)}^{\frac{4}{3}}}$$

(1)

The obtained electron density matrix equation was Equation (2):

$$\mathsf{\Omega }\left(r\right)=sign\left(I_2\left(r\right)\right)\rho \left(r\right)$$

(2)

As clarified in Figures S2 and S3, the blue section of the color level corresponds to the HB effect, and the intensity of HB gradually increased with the deepening of the color. In addition, the spatial interactions and van der Waals forces were represented by the red and green areas, respectively. Through analyzing the RDG versus Sign(I₂)r scatter plot, the relationship of IHB intensity in A₁, A₂, B₁, and C₁ was clear-cut and unambiguous. As shown in Figure 3, with the substitution modes of C₁, B₁, A₂, and A₁, the Sign(I₂)r values became more and more negative, unveiling that the strength of IHB in different surroundings obeys the order of A₁ > A₂ > B₁ > C₁. In the S₀ state, the prong peaks of A₁, A₂, B₁, and C₁ were located at −0.050, −0.049, −0.050, and −0.044, respectively. After photoexcitation, the prong peaks of A₁, A₂, B₁, and C₁ shifted to the more negative region (−0.087, −0.081, −0.075, and −0.063, respectively). The interaction type was judged to be an IHB interaction, corresponding to O₁–H₁…N₁. The IHB intensity of the four compounds was enhanced after photoexcitation, as indicated by the above-mentioned data, which is conducive to the ESIPT process. Moreover, comparison of the left-shifted peak positions in the four compounds revealed that the strength of IHB followed the order of A₁ > A₂ > B₁ > C₁ in the S₁ state. This was consistent with our previous analysis conclusion, revealing that different substitutions at multiple sites did affect the ESIPT process.

Topological analysis, first proposed by Bader [38], is also one of the most common methods for measuring the strength of HBs [39]. The obvious bond paths and bond critical points (BCPs) [40] in the IHB region are captured in Figure S4, and we have listed the relevant parameters in

Table 2. Calculated BCP parameters of bis-HBX. a: density of all electrons; b: potential energy density; c: Laplacian of electron density; d: Lagrangian kinetic energy; e: energy density; f: hydrogen bond energy $E$_HB = V(r)/2.

		ρ(r) a (a.u.)	ν(r) b (a.u.)	∇2(ρ) c (a.u.)	G(r) d (a.u.)	H(r) e (a.u.)	$\mathit{E}$HBf (kcal/mol)
A1	BCP1	0.050	−0.038	0.121	0.034	−0.004	−11.986
	BCP2	0.086	−0.085	0.105	0.056	−0.029	−26.795
A2	BCP3	0.049	−0.037	0.120	0.034	−0.004	−11.735
	BCP4	0.081	−0.078	0.115	0.053	−0.025	−24.473
B1	BCP5	0.050	−0.039	0.121	0.034	−0.004	−12.111
	BCP6	0.075	−0.069	0.123	0.050	−0.019	−21.712
C1	BCP7	0.044	−0.033	0.112	0.030	−0.002	−10.291
	BCP8	0.044	−0.033	0.112	0.030	−0.002	−10.291

. As is known to all, ρ(r) and ν(r) can gauge the intensity of IHBs with advantages, while the larger ρ(r) is and the more negative ν(r) is, the stronger IHB intensity will be. It could clearly be seen that the IHBs of the four molecules were all enhanced in the S₁ state. Moreover, a horizontal comparison of the absolute values of E_HB in bis-HBX showed that the E_HB values followed the order of E_HB(BCP2) > E_HB(BCP4) > E_HB(BCP6) > E_HB(BCP8), indicating that the IHB intensity of A₁ was the strongest of the four molecules in the S₁ state. It is well known that the stronger the IHB intensity is, the more favorable the ESIPT process is. Therefore, through the analysis of the above methods, we believe that the stronger the IHBs interaction, the easier the ESIPT process.

2.4. Fluorescence Attribution and Mechanism Analysis

We performed electron–hole analysis on bis-HBX molecules in the S₁ state, as shown in Figure 4. Furthermore, they were intuitively analyzed by utilizing the inter-fragment charge transfer (IFCT) method. During the emission process, from the right side to the central benzene ring direction, 0.776 electrons, 0.691 electrons, 0.675 electrons, and 0.188 electrons were transmitted, respectively. Moreover, the characteristics of hole and electron distribution indexes are included in

Table 3. Indexes illustrating the distribution of holes and electrons in bis-HBX. Sr: the extent of hole-and-electron overlap; t: the separation of electrons and holes.

	A1–S1	A2–S1	B1–S1	C1–S1
Sr	0.554	0.609	0.556	0.587
D (Å)	3.551	2.420	3.775	2.627
H (Å)	3.423	3.361	3.443	3.538
t (Å)	0.795	−0.182	0.945	−0.340

. It was well known that for analogs, the lower the Sr index and the more positive the t index, the more complete the separation of electrons and holes. In the light of the t and Sr indexes, a significant separation of holes and electrons was implied through the S₀→S₁ transition in A₁ and B₁, which proved that A₁ and B₁ had significant ICT (intramolecular charge transfer) characteristics. Furthermore, combined with the configuration of bis-HBX, the dihedral angles δ (C₁–C₂–C₃–N₂) of A₁ and B₁ had abnormal twists of 47 degrees and 24.69 degrees, respectively, during photoexcitation. Perspicuously, it can be confirmed that the typical twisted intramolecular charge transfer (TICT) [41,42] process existed in A₁ and B₁. From the above analysis results, it can be seen that A₁ and B₁ first underwent the TICT process in the S₁ state, and then underwent the ESIPT process. Thus, we know that the TICT process in A₁ and B₁ facilitated the ESIPT process.

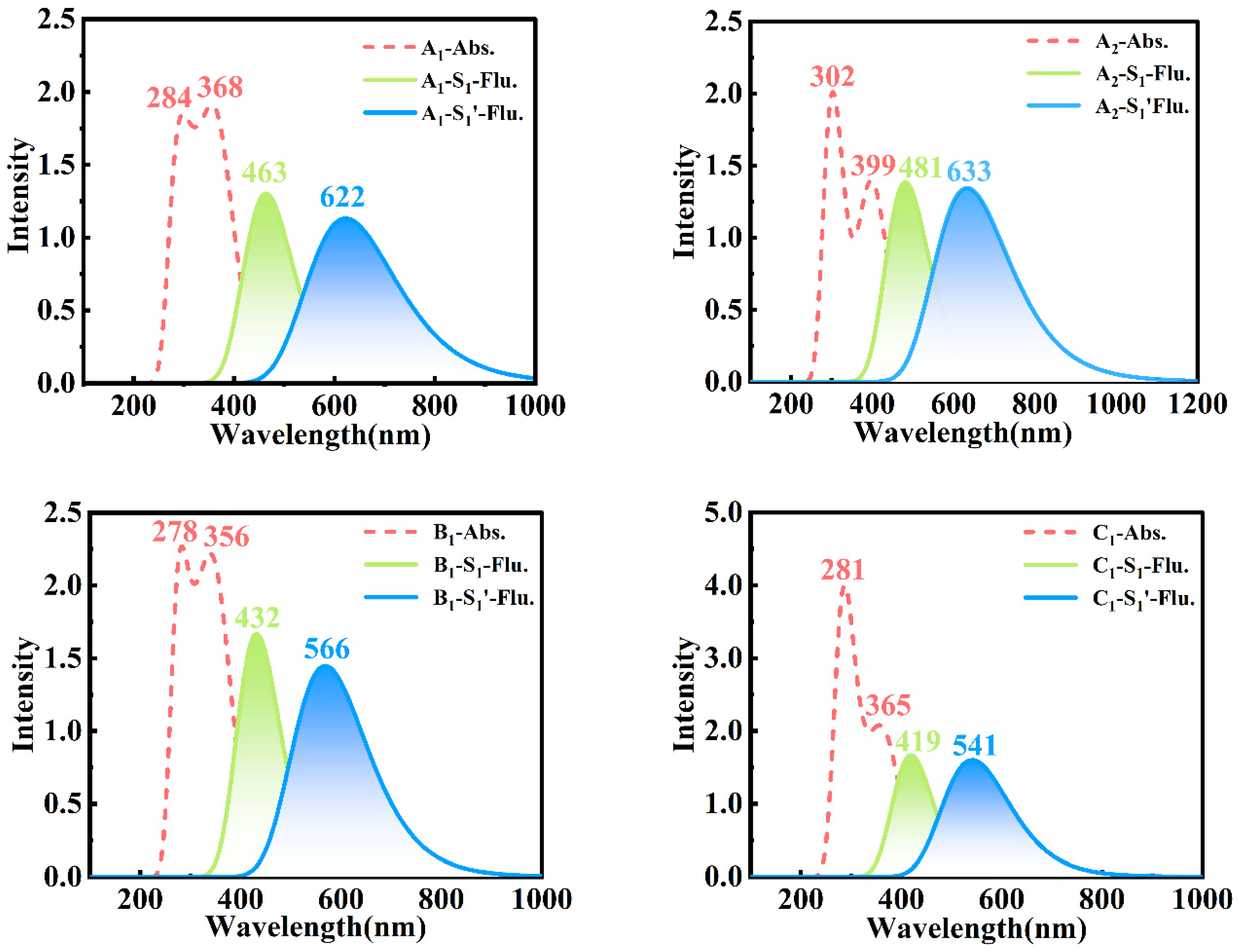
In order to gain a deeper understanding of the effects of different substitutions at multiple sites on photophysical properties in bis-HBX, we simulated the absorption and fluorescence spectra of bis-HBX (see Figure 5), which were obtained based on the optimized S₀, S₁, and S₁′ configurations. As shown in

Table 4. Calculated absorption peak (λ), oscillator intensity (ƒ), and orbital contribution (%) of bis-HBX in the gas phase.

	Transition	${\mathit{\lambda }}_{\mathit{a}\mathit{b}\mathit{s}}$	ƒ	Composition	CI (%)
A1	S0→S1	368	0.3971	H→L	69.51%
	S0→S2	323	0.0761	H-2→L	49.83%
	S0→S3	320	0.1709	H-2→L	49.07%
	S0→S4	298	0.0101	H-3→L	56.49%
	S0→S5	295	0.0623	H→L+1	59.81%
	S0→S6	284	0.2971	H-4→L	60.31%
A2	S0→S1	399	0.3376	H→L	70.02%
	S0→S2	324	0.0475	H-2→L	50.33%
	S0→S3	321	0.1545	H-2→L	47.17%
	S0→S4	317	0.0404	H→L+1	64.43%
	S0→S5	302	0.0522	H-3→L	56.66%
	S0→S6	292	0.3057	H-4→L	55.06%
B1	S0→S1	356	0.4091	H→L	69.77%
	S0→S2	319	0.1957	H-1→L	62.17%
	S0→S3	312	0.1080	H-2→L	62.75%
	S0→S4	283	0.0101	H-3→L	59.64%
	S0→S5	278	0.3668	H-4→L	52.07%
	S0→S6	277	0.1199	H→L+1	63.10%
C1	S0→S1	365	0.4634	H→L	69.14%
	S0→S2	313	0.2566	H-1→L	68.58%
	S0→S3	296	0.0475	H-2→L	64.08%
	S0→S4	288	0.3273	H→L+1	62.92%
	S0→S5	281	0.4930	H-1→L+1	60.23%
	S0→S6	272	0.0478	H-3→L	52.42%

, the relevant photophysical parameters were recorded, and the absorption peak, oscillator strength, and corresponding orbital transition contributions were also included.

The bis-HBX had double absorption peaks that could be distinctly observed from the data listed in Table S1, in which the calculated maximum absorption peak was very consistent with the experimental value, proving the feasibility of our method [26]. In addition, as shown in Figure 5, the short-wavelength emission peaks of A₂, A₁, B₁, and C₁ were 481 nm, 463 nm, 432 nm, and 419 nm, showing a blueshift from A₂ to C₁, respectively. The proton transfer tautomers in A₂, A₁, B₁, and C₁ corresponding to the longer wavelength fluorescence peaks were 633 nm, 622 nm, 566 nm, and 541 nm, respectively. Obviously, with the introduction of different substitution forms, the significant redshift was produced in the longer wavelength emission peaks of A₂, A₁, B₁, and C₁, and the degree of redshift followed the order of A₁ > A₂ > B₁ > C₁. However, only one short-wavelength fluorescence was observed in A₁ in accord with the emission peak in the experiment; thus, we attributed it to the S₁ (TICT) state.

Moreover, in order to study the fluorescence properties in bis-HBX more comprehensively, we used Equations (3) and (4) [36,37]:

$$\tau =\frac{1.499}{f_1{E}^2}\times {10}^{-5}$$

(3)

$$f_2=\frac{1}{\tau }$$

(4)

We calculated the fluorescence lifetime and rate in the bis-HBX and listed them in

Table 5. Photophysical parameters of bis-HBX. $\tau$ represents fluorescence lifetime (ns); $f_2$ represents fluorescence rate (×10⁻⁸).

	Transition	${\mathit{\lambda }}_{\mathit{a}\mathit{b}\mathit{s}}$	ƒ1	Composition	CI (%)	$\mathit{\tau }$	${\mathit{f}}_2$
A1	S0→S1	368	0.3971	H→L	69.51%	5.1121	0.1956
A2	S0→S1	399	0.3376	H→L	70.02%	5.1027	0.1960
B1	S0→S1	356	0.4091	H→L	69.77%	4.6438	0.2240
C1	S0→S5	281	0.4930	H-1→L+1	60.23%	2.4009	0.4165

. In the formula, $f_1$ represents the oscillator strength and $E$ delegates the wavenumber. It can be observed that A₁ exhibited the greatest fluorescence lifetime, which may be due to its lower oscillation intensity. Similarly, a larger fluorescence lifetime resulted in a lower fluorescence rate, with A₁ exhibiting the lowest fluorescence rate. All the above analyses indicated that A₁ was the fluorescence probe molecule with the strongest luminous efficiently in bis-HBX, and we were also able to confirm that different substitutions at multiple sites did affect the spectral characteristics of bis-HBX.

Finally, we conducted a detailed analysis of the excited-state decay process in bis-HBX. We have provided a diagrammatic sketch of the excitation and emission processes in bis-HBX (Figure 6) according to Table 4. Perspicuously, from the oscillator strength in Table 3, the excited states of A₁, A₂, and B₁ were separated from each other. It was interesting that the excited states of S₁ (0.4634) and S₅ (0.4930) in C₁ were coupled together and formed an intersection point where the internal conversion (IC) took place. The vertical transition S₁→S₀ that occurred at the Franck–Condon region of A₁, A₂, B₁, and C₁ was accompanied by shorter wavelength fluorescence measurements of 463 nm, 481 nm, 432 nm, and 419 nm, respectively. Subsequently, in the S₁ state, from the higher vibration level to the lowest vibration level, a vibration relaxation process occurred in which A₁, A₂, B₁, and C₁ recovered from the tautomer structure to the S₀ state, while at 622 nm, 633 nm, 566 nm, and 541 nm, respectively, they emitted long-wavelength fluorescence.

2.5. Frontier Molecular Orbitals and NBO Population

It is particularly acknowledged that frontier molecular orbitals (FMOs) are an effective means to reflect charge distribution and recombination [43]. Since the first single transition of the target compound is mainly related to the highest occupied orbital (HOMO) and the lowest unoccupied orbital (LUMO), Figure 7 only renders these two orbitals. During the photoexcitation process, the electronic clouds on the O₁ and N₁ atoms were drastically reduced and increased, respectively. This result indicated that there was a strong binding ability between the N₁ atom and the H₁ proton, which provided the driving force for the ESIPT process. For the purpose of studying the changes in electron density distribution on O₁ and N₁ quantitatively, the NBO charge distributions of bis-HBX are recorded in

Table 6. NBO charge allotments (a. u.) of the O₁ and N₁ in bis-HBX in different states.

	A1		A2		B1		C1
	S0	S1	S0	S1	S0	S1	S0	S1
O1	−0.693	−0.677	−0.707	−0.685	−0.693	−0.684	−0.696	−0.676
N1	−0.515	−0.523	−0.514	−0.519	−0.543	−0.545	−0.540	−0.547

. The negative charges on the O₁ shrank from −0.693 a. u., −0.707 a. u., −0.693 a. u., and −0.696 a. u. in the ground state to −0.677 a. u., −0.685 a. u., −0.684 a. u., and −0.676 a. u. in the excited state, while that of the N₁ atom was enlarged during the photo-absorption process, which was confirmed by the analytical results of the FMOs.

In addition, we used the Hirshfeld method to calculate the electron density components in Fragment 1 and Fragment 2 in bis-HBX (see Figure 7). In Fragment 1, the electron density components of A₁, A_2, and B₁ increased from 80.897%, 88.180%, 53.063%, and 57.988% in HOMO to 93.816%, 93.110%, 92.774%, and 86.433% in LUMO, respectively. Regarding Fragment 2, the electron density components were 19.103%, 11.820%, 46.937%, and 42.012% in HOMO, while they separately decreased to 6.184%, 6.890%, 7.226%, and 13.562% in LUMO. Meanwhile, as mentioned in the geometric parameters, the obtained dihedral angles δ (C₁–C₂–C₃–N₂) of A₁, A₂, B_1, and C₁ underwent torsions of 47°, 28.16°, 24.69°, and 0°, respectively, which demonstrated that A₁ has obvious TICT characteristics.

2.6. Potential Energy Curves (PECs)

With the aim of uncovering the mechanism of the ESIPT process with different substitutions at multiple sites more intuitively, the potential energy curves (PECs) in bis-HBX were scanned via prolonging the O₁–H₁ bond length from 0.9 Å to 1.9 Å in increments of 0.1 Å based on the optimized structures in the gas phase [44].

As shown in Figure 8, the PECs of the four probe molecules showed an ascending trend in the S₀ state. This demonstrates that it was difficult for the proton transfer (PT) process to occur. After photoexcitation, the energy barriers in the four probe molecules were diminished to 0.24 kcal/mol (A₁), 0.55 kcal/mol (A₂), 0.67 kcal/mol (B₁), and 1.15 kcal/mol (C₁), respectively. It is obvious that it was more possible for the four probe molecules to undergo the PT process in the S₁ state. It is a remarkable fact that the order of energy barriers in bis-HBX followed the order of A₁ < A₂ < B₁ < C₁, which verified that A₁ can occur the ESIPT process more easily. Although the B3LYP/6-31+G (d,p) can provide qualitative descriptions of the hypersurface, it may underestimate some of the energy differences and energy barriers. We recalculated the PECs using Cam-B3LYP/TZVP [45,46] theory (see Figure 8; the blue font is the corrected energy barrier), taking the corrected results as the reference. It was very clear and concise that the energy barriers calculated using Cam-B3LYP/TZVP theory were consistent with the order of A₁ < A₂ < B₁ < C₁. In addition, to further confirm the ESIPT process mechanism, we also performed transition-state (TS) calculations and obtained the intrinsic reaction coordinate (IRC) to validate the reasonableness and accuracy. Meanwhile, we have listed the ESIPT barriers via the IRC paths for bis-HBX in

Table 7. Theoretical PT barriers (kcal/mol) calculated from the PECs (B3LYP and Cam-B3LYP) and from IRCs (S₁ state) for bis-HBX.

	A1	A2	B1	C1
B3LYP	0.24	0.55	0.67	1.15
Cam-B3LYP	0.32	0.68	0.69	3.40
IRC	0.35	0.67	0.69	3.42

, which is consistent with the simulation results of the PECs. We further confirmed that A₁ can cause the ESIPT process to occur more easily. To date, we have explained the effects of different substitutions at multiple sites in the ESIPT process of bis-HBX molecules and reasonably elucidated the whole kinetic process of the studied molecules. This provides important reference values for the application of fluorescent probe molecules.

3. Calculation Details

In the gas phase, the geometric structures of different electronic states of bis-HBX were fully optimized by DFT [46,47,48,49,50,51] and TD-DFT [52,53,54,55] based on B3LYP-D3/6-31+g (d, p) [47,48] levels. We chose six different functionals to calculate the absorption spectra of bis-HBX, and the results are listed in

Table 8. Using six functionals, we calculated absorption peaks (nm) in bis-HBX. (Exp. was the experimental value.)

Exp.	B3LYP [63]	B3LYP-D3 [47]	Cam-B3LYP [64]	M06-2X [65]	MPW1PW91 [66]
290	295	295	251	250	284
363	370	368	321	320	357

. The calculated values of B3LYP-D3 (295 nm and 368 nm) were compatible with the experiment values (290 nm and 363 nm) [26]. Therefore, it was reasonable to use the B3LYP-D3 functional to evaluate the excited-state properties of the system. Based on the optimized geometric configurations, we calculated the infrared (IR) [56] vibration spectra and confirmed that all the local minima had no virtual frequency. For the purpose of probing into the study of the ESIPT mechanism in bis-HBX, the PECs (potential energy curves) of bis-HBX were scanned by setting O₁-H₁ from 0.9 Å to 1.9 Å in steps of 0.1 Å. The transition-state (TS) structure was located by the Berny algorithm to obtain a more accurate barrier height [57]. In addition, the RDG scatter plot [35], electron–hole, and frontier molecular orbitals (FMOs) [58,59] were analyzed by using the Multiwfn program and visualized by the VMD 1.9.4 software [60,61]. All calculation details of the system were carried out by the Gaussian program [62].

4. Conclusions

In summary, the DFT/TDDFT theoretical method was used to systematically investigate the effects of different substitutions at multiple sites on the photophysical properties of bis-HBX. Relying on the results of IR vibration spectra, RDG scatter plots, and topological structure, it was demonstrated that the IHBs of bis-HBX were enhanced in the S₁ state and underwent the ESIPT process in the S₁′ state. Significantly, upon analyzing the energy barrier height of bis-HBX, we noticed that the ease of the ESIPT process followed the order of A₁ > A₂ > B₁ > C₁. Furthermore, combining molecular configuration and electron–hole analysis, it was demonstrated that A₁ and B₁ underwent severe twisting (TICT state), which promoted the ESIPT process. Meanwhile, the internal conversion process was verified in C₁. Finally, it was verified that A₁ is the most suitable fluorescence probe molecule for detecting alkaline pH values when combined with the calculated fluorescence lifetime and fluorescence rate. Herein, the above research not only proposed the photophysical mechanism of bis-HBX, but also provided the effects of different substitutions at multiple sites on the ESIPT process in bis-HBX. Our work has provided strong support for the experiment, and in the meantime, we are sincerely anxious for this work to shed some light on regulating the photophysical behaviors and ESIPT reactions of developing and designing fluorescence probe molecules at the theoretical level.