Next Article in Journal
The Effects of Essential Oils on the Nervous System: A Scoping Review
Previous Article in Journal
Thermodynamic and Kinetic Stabilities of Al(III) Complexes with N2O3 Pentadentate Ligands
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 28
Issue 9
10.3390/molecules28093768
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

A Computational Evaluation of the Steric and Electronic Contributions in Stereoselective Olefin Polymerization with Pyridylamido-Type Catalysts

by
Olga D’Anania
1,
Claudio De Rosa
2 and
Giovanni Talarico
2,*
1
Scuola Superiore Meridionale, Largo San Marcellino, 80138 Napoli, Italy
2
Dipartimento di Scienze Chimiche, Università degli Studi di Napoli Federico II, 80124 Napoli, Italy
*
Author to whom correspondence should be addressed.
Molecules 2023, 28(9), 3768; https://doi.org/10.3390/molecules28093768
Submission received: 10 February 2023 / Revised: 21 April 2023 / Accepted: 25 April 2023 / Published: 27 April 2023
(This article belongs to the Section Inorganic Chemistry)
Browse Figures
Review Reports Versions Notes

Abstract

:
A density functional theory (DFT) study combined with the steric maps of buried volume (%VBur) as molecular descriptors and an energy decomposition analysis through the ASM (activation strain model)–NEDA (natural energy decomposition analysis) approach were applied to investigate the origins of stereoselectivity for propene polymerization promoted by pyridylamido-type nonmetallocene systems. The relationships between the fine tuning of the ligand and the propene stereoregularity were rationalized (e.g., the metallacycle size, chemical nature of the bridge, and substituents at the ortho-position on the aniline moieties). The DFT calculations and %VBur steric maps reproduced the experimental trend: substituents on the bridge and on the ortho-positions of aniline fragments enhance the stereoselectivity. The ASM–NEDA analysis enabled the separation of the steric and electronic effects and revealed how subtle ligand modification may affect the stereoselectivity of the process.

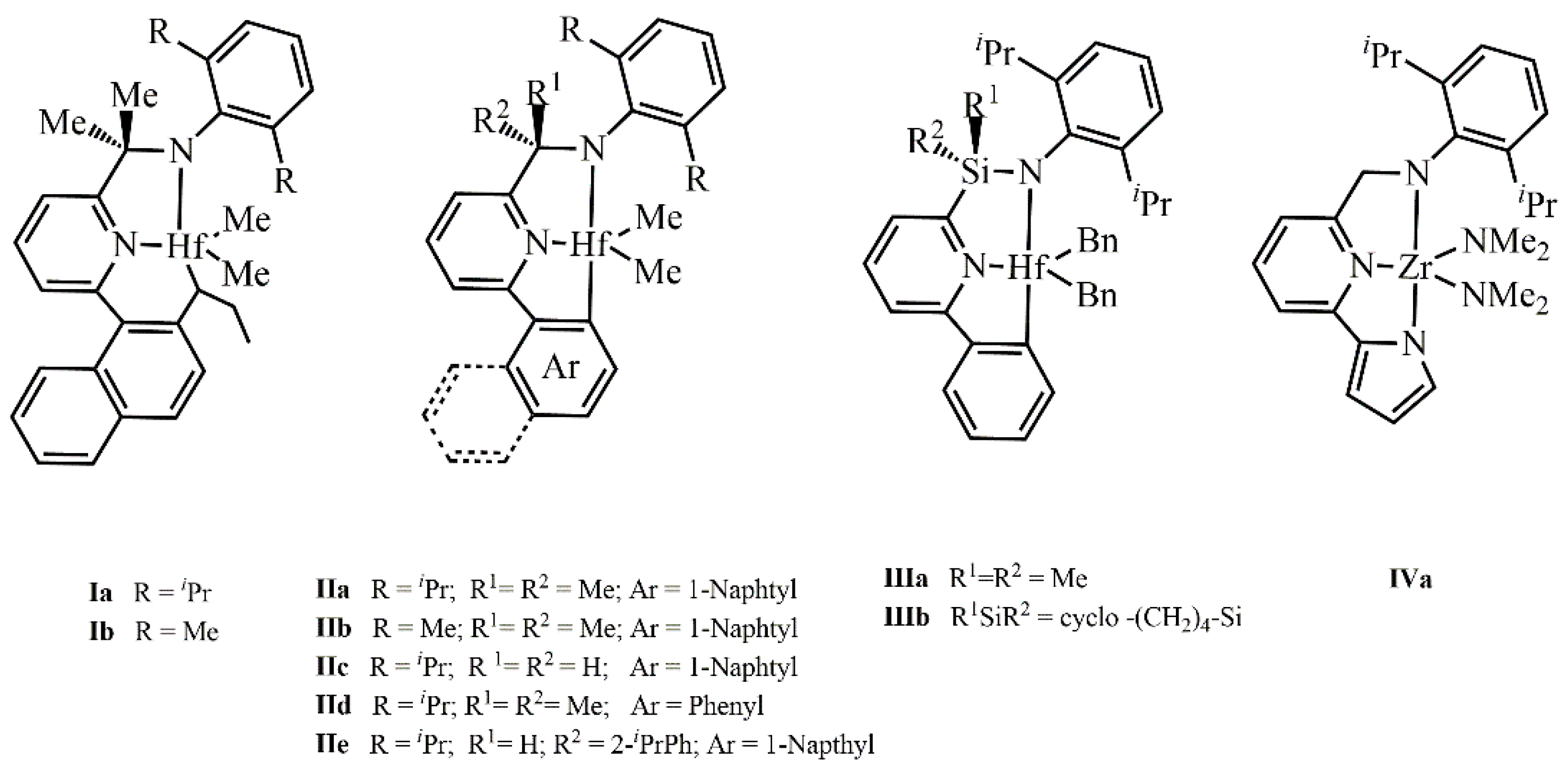
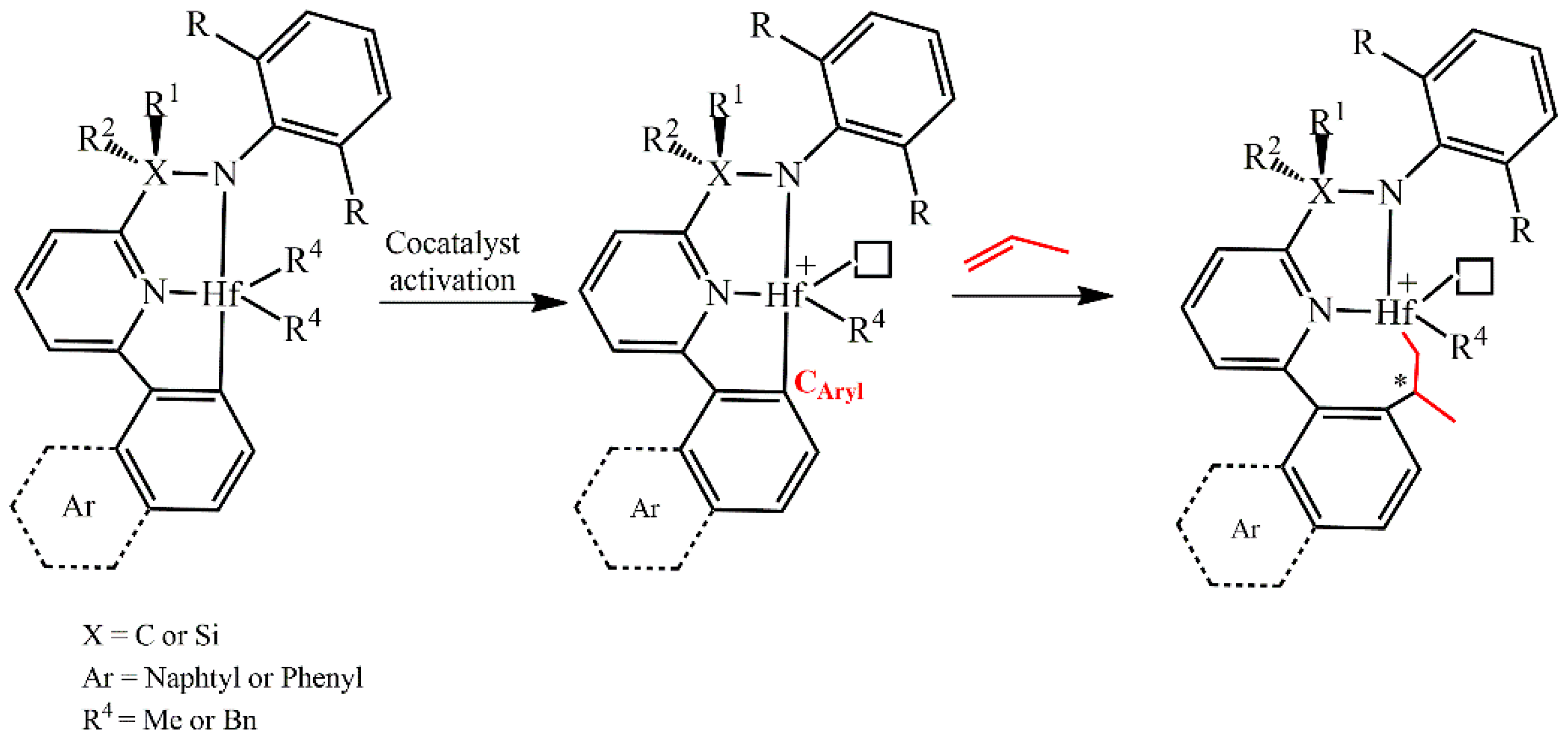
1. Introduction

The advent of single-site α-olefin polymerization catalysts has revolutionized the world of polyolefins, as they enable the fine tuning of polymer microstructures in terms of stereoregularity [1], regioregularity [2,3] molecular mass [4,5,6], and polymer properties [7]. Increasing interest in the design of novel nonmetallocene complexes [8] has also opened up a way to further investigate the factors that determine the origin of the stereocontrol of α-olefin polymerization promoted by homogeneous systems. Some interesting examples of this are the pyridylamido-Hf catalyst model compounds developed by Coates et al. [9] (Ia, Ib, Chart 1) and the modification [10] (IIa–IId, Chart 1) of Dow Chemical systems [11] obtained via high-throughput technologies (IIe, Chart 1). Besides propylene, systems IIc and IIe (Chart 1) have been also employed to polymerize higher α-olefins such as 1-hexene and 1-octene [12], as well as to incorporate functional comonomers [13]. Moreover, block copolymers can be obtained through “chain shuttling” processes, which involve IIe in combination with a second catalyst [14,15,16,17]. Density functional theory (DFT) calculations have revealed that IIa-IIe produce isotactic polypropylenes (iPP), despite the C1 or Cs symmetry of the catalyst precursors, through a combination of peculiar aspects that distinguish this class of complexes, which are briefly summarized here. The cationic species deriving from the cocatalyst activation show both the Hf-CAryl and Hf-CAlkyl bonds into which the first olefin insertion may occur, and it has been demonstrated that the initial insertion takes place at the Hf-CAryl bond, thus generating the monoinserted active species (Scheme 1) [18,19,20,21].
The DFT calculations also found a substantial preference for propene insertion at one of the two diastereotopic sites thus generated (Scheme 1) [22], leading to a chain stationary (CS) mechanism (or site epimerization) [23] different from the usual chain migratory (CM) mechanism (the switching of the olefin and the growing chain at each insertion step) [24]. Very recently [25,26], we identified the stereo-electronic factors that induce a CS mechanism by using a combined approach based on DFT calculations, a sterical descriptor (the percentage of occupied volume (%VBur) [27,28]), and an activation strain model (ASM) with a natural energy decomposition analysis (NEDA) scheme [29,30], which were applied to polymerization catalysis [31,32].
This family of catalysts deviates from the classical “chiral growing chain orientation” mechanism of stereocontrol [1,33] operating on ansa-metallocene [1] and heterogeneous Ziegler–Natta (ZN) systems [33,34], because its stereoselectivity is indeed based on a direct monomer–ligand interaction mechanism [22]. The experimental propene polymerization data (Table S1) show that the ortho-substituents on the aniline moiety (R in Chart 1) play a crucial role in the stereoselectivity, with the catalyst performance also being influenced by the R1 and R2 substituents on the bridge linking the pyridine and the N-aryl fragment (Chart 1). Although quite far from the active sites, they do affect the enantioselectivity of the complex, pushing the N-aryl ring, and consequently its ortho-substituent, closer to the active site thus, enhancing the stereoselectivity of the catalyst through a “buttressing effect” [10]. Furthermore, Hagadorn et al. [35] claimed that a substitution of the C-bridge with a Si-bridge (IIIa, IIIb, Chart 1) seems to increase the stereoselectivity of propene polymerization. Finally, a replacement of the aryl group (e.g., phenyl or naphthyl) with heteroaryls [36,37] has also been reported. For instance, Pellecchia et al. synthesized a Cs-symmetric Zr(IV) complex which bears a tridentate pyrrolidepyridine ligand (IVa, Chart 1) and affords iPP when combined with AliBu2H and methylalumoxane [38]. The presence of Al-H alkyl species is necessary for making the complex stereoselective, and the DFT calculations suggest [39] that Al coordinates with the Npyrrolic and that H interacts with the central metal, leading to a sort of “ligand modification”, similar to what happens for pyridylamido Hf catalysts. The original catalyst symmetry is thus altered by the AliBu2H coordination, shifting from Cs to C1 symmetry. The two diastereotopic active sites, one which is better described by a pyramidal square geometry (site 1) and the other by a trigonal bypiramidal geometry (site 2), select the same propene enantioface, with the unprecedented combination of a “direct ligand–monomer” interaction for one site and a “chiral growing chain orientation” model for the other [39].
In this work, we decided to investigate the propene stereoselectivity promoted by the systems of Chart 1 to achieve a unified picture, with respect to the (large) spread of the experimental data (Table S1). We used a combined approach based on DFT calculations, a %VBur analysis, and an ASM–NEDA model to assess the steric and electronic contributions to the propene stereoselectivity for the fine tuning of: (a) the metallacycle size, characterized by a six-membered (Ia-Ib) and seven-membered (IIa-IIe, IIIa, IIIb) ring, respectively; (b) the chemical nature of the R, R1, and R2 substituents located on the aniline ring and the bridge linking the pyridine and the aniline fragments; (c) the central atom on the bridge by replacing the C with Si atoms (IIIa, IIIb); and (d) the pyridylamido framework by replacing the aryl with heteroaryl groups (IVa).

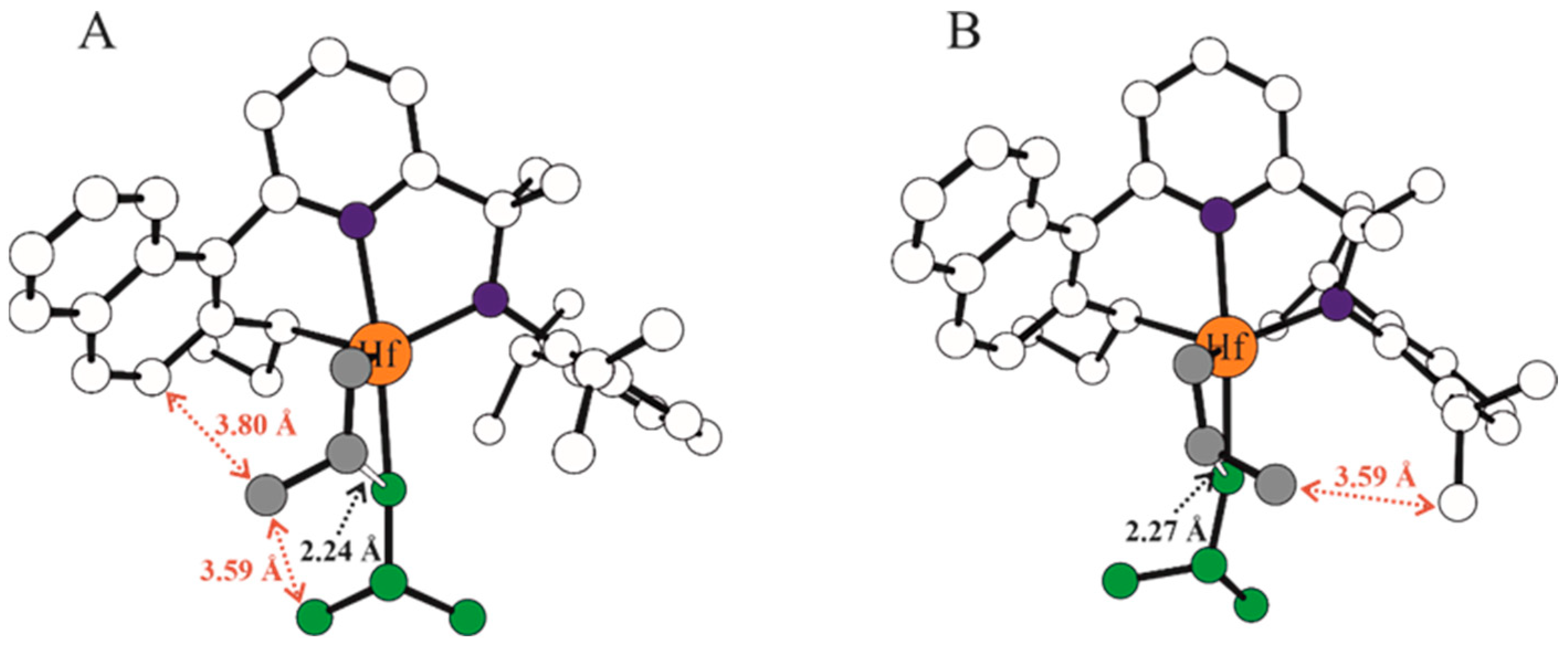
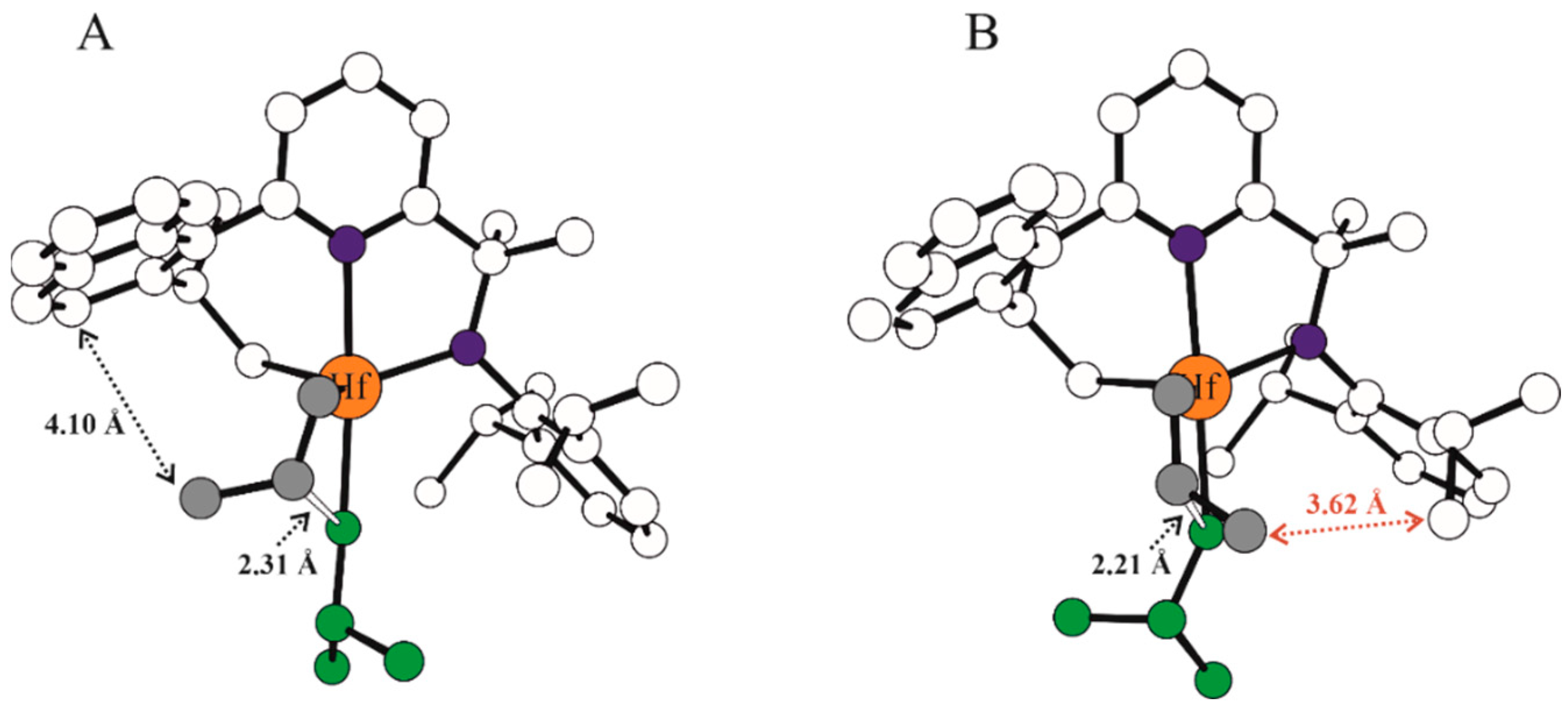
2. Results and Discussion

The DFT values calculated for the stereoselectivity of the propene polymerization promoted by the systems of Chart 1 are summarized in Table 1. They are reported as the differences in the electronic energies (free energies) between the lower 1,2 si and 1,2 re propene enantioface insertion transition states (TSs), which were calculated in the presence of a solvent contribution (first column, PCM model, see Section 3). Since the ASM–NEDA analysis employs electronic energies in the gas phase (ΔE), for the sake of consistency, we also report the differences in the DFT electronic energies (free energies) values in the gas phase (Table 1, second column). Given the findings about the CS mechanism disclosed for the pyridylamido-Hf complexes [22], only the results for the propene insertion at the preferred site are reported for Ia-IIIb, whereas the energetics for the monomer insertion at both diastereotopic sites are reported for IVa. The partitioning of ΔETot into its contributions obtained through the ASM–NEDA analysis is reported in Table 2 and the details about the decomposition of the ΔEInt into all its terms can be found in Table S2. To simplify the discussion, we also added into Table 2 the ΔΔE between the 1,2 re and 1,2 si enantiofaces (kcal/mol) obtained by the ASM–NEDA analysis. The effect of the dispersion corrections on the DFT electronic energies is reported in Table S3, whereas the values of the energetic terms obtained through the ASM–NEDA scheme, without including the dispersion corrections, are illustrated in Table S4.
Looking at the ASM–NEDA results in Table 2, we noted that the ΔEStrain is, indeed, the main factor for the origin of the stereoselectivity promoted by the analyzed systems. The clear preference for the 1,2 re enantioface is only partially compensated by the ΔEInt contribution, which instead stabilizes the si enantioface (Ia-Ib being the only exceptions). The further decomposition of the ΔEStrain into the two components (ΔEStrain(Mon) and ΔEStrain(Cat)) is highly indicative; systems II and III, characterized by the formation of seven-membered metallacycles, show the propene deformation between the si and re insertions (ΔΔEStrain(Mon)), which outweighs that of the catalyst (ΔΔEStrain(Cat)), thus playing the primary role in the ΔEStrain variation, although the two components become similar for IIe and IIIa (Table 2).
On the contrary, systems I and IV, which cannot undergo a ligand modification in situ, deviate from this trend, and the ΔΔEStrain(Cat) is the dominant term for the ΔEStrain variation (Table 2). This difference may be rationalized by examining the orientation of the growing polymer chain obtained by the DFT calculations, taking system Ia as an example. In Figure 1, the optimized geometries for the TSs of the right (Figure 1A) and wrong propene enantioface insertions (Figure 1B) promoted by Ia are reported. For such a system, characterized by a six-member metallacycle, there is not enough room to accommodate the bent growing chain [40]; therefore, the catalyst structure distorts and ΔΔEStrain(Cat.) becomes the fundamental contribution to the ΔΔEStrain. For the other pyridylamido catalysts (see, e.g., IIa TS structures reported in Figure 2), the first C-C bond of the iBu group simulating the polymeryl chain is perfectly in anti with respect to the methyl group of propene, and is thus bent towards the aryl group. It appears that the metallacycle size does affect the iPP stereoselectivity and the results reported in Table 1 and Table 2 show that IIa (ΔE(ΔG)Stereo = 4.0 (3.1) kcal/mol) is more stereoselective than Ia (ΔE(ΔG)Stereo = 2.4 (1.0) kcal/mol), even if they bear the same R, R1, and R2 substituents, in agreement with the experimental data [9,10] (Table S1). As already mentioned, the ASM–NEDA analysis suggests that the ΔΔEStereo for Ia is mainly due to the ΔΔEStrain(Cat) contribution, rather than that of the ΔΔEStrain(Mon) (Table 2). As a matter of fact, the ΔEStrain(Mon) term favors the wrong propene enantioface insertion (1,2 si) rather than the right one (1,2 re) and the greater distortion of the latter may be attributable to the presence of an additional disfavoring interaction between the propene and naphthyl moiety (Figure 1A).
Removing this “penalty” with a larger metallacycle (a seven-member metallacycle shown by IIa, Figure 2) forces the naphthyl group to stay further from the olefin and the ΔEStrain(Mon) becomes the main factor in the stereoselectivity (Table 2).
The variation in the steric hindrance moving from Ia to the IIa active sites may be visualized by the steric maps of the corresponding neutral mono-inserted species (Figure 3A,B). The northeast (NE) quadrant is effective for the direct monomer enantioface selection, since it contains the N-aryl ring with its ortho-substituents that interact with the “wrong” propene enantioface (1,2 si). At the same time, the southeast (SE) quadrant may be responsible for adding the penalty for the “right” propene enantioface (1,2 re), whereas the southwest (SW) quadrant contains the metallacycles and the naphtyl group.
The computed %VBur are consistent with the ASM–NEDA results. In fact, the ΔΔEStrain(Mon) term is the main ΔΔEStrain contribution to the system showing the higher %VBur in the NE quadrant (IIa). Furthermore, although the SW quadrants of the maps for Ia and IIa have comparable buried volumes (81.2% for Ia and 82% for IIa), the SE quadrant for Ia has a significantly higher %VBur (38.7%) than IIa (33.1%). The greater steric hindrance in the SE quadrant for system Ia is due to the closer proximity of the naphtyl ring to the active site, caused by the smaller metallacycle size. Consequently, the aryl fragment occupies the SE quadrant along with the SW quadrant, thus adding a small penalty to the “right” propene enantioface insertion in the case of catalyst Ia (Figure 3A). Instead, such a penalty is absent for complex IIa, where the naphtyl moiety occupies only the SW quadrant (Figure 3B); therefore, it does not interact sterically with the re propene enantioface.
The %VBur steric maps separated by quadrants allow us also to visualize the effect of the substituents R1 and R2 on the CCN bridge by comparing IIa and IIc (Figure 3B,C). Through the DFT calculations, we found that the ΔE(ΔG)Stereo for system IIa is higher than that for IIc (Table 1), and the ASM–NEDA analysis reveals that the main reason for this energetic difference is the ΔΔEStrain, whereas the ΔΔEInt is quite similar (Table 2). In particular, the ΔΔEStrain(Mon) contribution is larger than the ΔΔEStrain(Cat) term and it increases from IIc to IIa. Indeed, as already reported by Coates et al., bulky substituents at the C-bridge enhance the stereoselectivity through a Thorpe–Ingold-like “buttressing effect”, as they interact with ortho-substituents on the aniline ring, forcing them closer to the olefin [10]. This explains the greater distortion of the monomer for IIa rather than IIc, but also the longer distance between the propene and iPr group on the aniline moiety in the 1,2 si insertion TS for IIc (Figure S1) with respect to IIa (Figure 2). The influence of the “buttressing effect” becomes evident when the north quadrants of the steric maps are examined. In fact, the presence of different substituents at the bridge not only affects the %VBur of the northwest (NW) quadrant, but also the buried volume in the NE quadrant, which contains the aniline moiety. Both the NW and NE quadrants of the IIc map (Figure 3C) have a lower buried volume (73.8% and 45.3%) than the analogous of IIa (75.7% and 50.5%) (Figure 3B). Furthermore, the substitution of the R substituents with smaller groups (from iPr to Me) clearly decreases the stereoselectivity of the propene insertion for both the Ib and IIb catalysts (Table 1). The corresponding DFT-optimized geometries showing less effective ligand–monomer interactions are reported in Figures S2 and S3 and the %VBur steric maps are reported in Figure S4A,B.
An interesting modification of the chemical nature of the bridge (replacing the central C atom with a Si one) has been recently published in the literature [35]. Our calculated stereoselectivity for system IIIa was not particularly encouraging with respect to the analogous system IId being the energetic (free energies) difference between the two TSs, with facial selectivities of 2.5 (2.2) kcal/mol and 3.8 (3.1) kcal/mol, respectively. The smaller CCN angle in complex IId (Figure 4A,B) forced the iPr groups on the N-aryl ring closer to the active site, increasing the steric ligand–monomer interactions (3.61 Å) with respect to the Si-bridged system (Figure 4C,D) (3.88 Å). The preferred configuration of the growing chain was similar for IId and IIIa, and in both cases, we noted the absence of the chiral configuration of the growing chain [41]. The ASM–NEDA analysis (Table 2) clarified these findings, especially the ΔΔEStrain(Mon), which represents the principal contribution to the ΔΔEStrain and is higher for system IId (4.7 kcal/mol) compared to system IIIa (3.5 kcal/mol). However, it has been claimed that the suitable modification of silicon-bridged pyridylamido-hafnium complexes may effectively reach a higher stereoselectivity, and when we performed DFT calculations on the cyclo-(CH2)4Si bridged complex (IIIa) employed by Hagadorn [35], we found that IIIb was more stereoselective than IIIa (Table 1). The presence of a cyclic substituent on the Si-bridge rather than two methyl groups must be regarded as the reason why IIIb produces a more stereoregular iPP than IIIa. As a matter of fact, the –(CH2)4- substituent on the bridge makes the complex more constrained, reducing the C-Si-N angle, thereby forcing the N-aryl fragment closer to the monomer (Figure 5).
In agreement with such statements, the %VBur steric map analysis revealed that there were no notable differences between systems IId and IIIa (Figure 3D,E), whereas IIIb (Figure 3F) showed larger %VBur values in the NW and NE quadrants, in agreement with a higher stereoselectivity. The ASM–NEDA results (Table 2) confirmed that the enhanced stereoselectivity of this complex was due to the larger variation in the ΔEStrain(Mon) between the 1,2 si and 1,2 re insertions, and a quick visualization of the monomer deformation is reported in Figure S5.
Interestingly, an even higher isotacticity is predicted for the polymerization promoted by the Dow system IIe (Table 1); the unsymmetrical substitution of R1 and R2 on the C bridge increases the ΔΔEStrain(Cat) contribution (with respect to IIa), although with a slight decrease in the ΔΔEStrain(Mon) (Table 2). The DFT-optimized geometries for the IIe TSs are reported in Figure S6 and the %VBur steric map is reported in Figure S4C.
As a final step, we focused on the role of the CAryl bond (Scheme 1) in the pyridylamido framework, replacing the aryl with heteroaryl groups (IVa). The presence of a heteroatom in place of the CAryl bond avoids the ligand modification in situ and the experimental propene isotacticity promoted by IVa can be explained by the formation of diastereotopic active sites following the AliBu2H coordination (Figure S7). Interestingly, site 1 and site 2 select the same propene enantioface and the stereoselectivity is dictated by the “direct ligand–monomer” for site 1 (Figure S7A,B) and the “chiral growing chain orientation” model for site 2 (Figure S7C,D) [39]. The ASM–NEDA results for IVa, although unraveling the subtle differences for the ΔEInt contribution at each site, are substantially similar to the ones discussed for Ia (Table 2). This demonstrates the relevance of the ΔΔEStrain(Cat) as the main term for the ΔEStrain variation and as the origin of isotactic propagation. The loss of the additional contribution of the ΔΔEStrain(Mon), is, in the end, the main reason for the lower stereoselectivity calculated for IVa with respect to IIa, IIe, IIIa, and IIIb (Table 1), and the way to increase this stereoselectivity is an unsymmetrical substitution with bulky substituents on the bridging methylene atom [37] analogously to system IIe.

3. Methodology

The DFT calculations were performed by using Gaussian16 programs [42]. B3LYP hybrid functional [43,44] was employed in conjunction with a polarized split-valence basis set (SVP) for H, C, N, Si, O [45] and LANL2DZ basis and pseudopotential for the metal [46]. The stationary points were characterized using vibrational analyses and these analyses were also used to calculate zero-point energies and thermal (enthalpy and entropy) corrections (298.15 K, 1 bar). Improved electronic energies were obtained from single-point calculations using the TZVP basis set for H, C, N, Si, O [47] and the SDD basis set and pseudopotentials [48] for Hf and Zr (an f function with an exponent of 0.5 was added). The dispersion corrections (EmpiricalDispersion=D3 in the Gaussian package) [49] and solvation contribution (PCM model [50], toluene) were evaluated, thus obtaining ΔG (B3/D3/TZVP/PCM) values. With the ASM model proposed by Bickelhaupt [29,30], we partitioned the ΔETot into the ΔEStrain and ΔEInt components, where the former was the energy associated with the reactant deformation required to achieve the geometries necessary for a reaction, and the latter was the energy associated with the strength of their reciprocal interactions. Furthermore, the ΔEStrain contribution was additionally expressed as the sum of the strain of the cationic active species bearing the growing polymer chain (simulated by an iBu group), ΔEStrain(Cat), and the propene monomer, ΔEStrain(Mon), calculated with respect to the optimized geometries of each species. By using the NEDA [51] approach, we also decomposed the ΔEInt into its terms, which are: the classical electrostatic interaction (ES), polarization interaction (POL), charge transfer (CT), exchange correlation interaction (XC), and deformation (DEF), which represents the energy required to deform the wavefunction of a fragment in the presence of all the other fragments. The ΔETot partitioning into its contributions through the ASM–NEDA model is reported in Scheme 2.
The NBO version 7 software, coupled with Gaussian16 (in conjunction with the TZVP basis set for H, C, N, O, and Si and the SDD basis and pseudopotentials for Hf), was used to perform the NEDA calculations. The steric maps and the percentage of buried volume for each quadrant (%VBur) were computed employing a modified version of the SambVca package [27,28] and the whole computational approach has already been tested on the olefin polymerization catalysis [52]. The steric maps for the neutral mono-inserted species (IIa-IIe and IIIa-IIIb) or the neutral catalytic precursors (Ia, Ib and IVa) were created by setting the transition metal as the center of the sphere, whose radius was set to 3.5 Å.

4. Conclusions

In conclusion, in this work, we analyzed the origin of the stereoselectivity for propene polymerization promoted by pyridylamido-type catalysts. This catalyst class have emerged within the nonmetallocene family because it allows the formation of block-copolymers [53,54,55,56,57] via living and chain shuttling processes [14], and such molecular architectures are not accessible to ansa-metallocenes [58] and heterogeneous ZN systems [59,60]. The mechanism of stereocontrol in olefin polymerization has been proven to be different from the one proposed for ansa-metallocenes [1,33], as well as for octahedral nonmetallocene ligands [33,61]. We used a combined DFT/%VBur/ASM–NEDA approach for the computational assessment of the steric and electronic contributions to rationalize the ligand structure/polymer microstructure. By using this methodology, we clarified the effect of the metallacycle size, the chemical nature of the bridge linking the pyridine moiety to the N-aryl fragment, the R, R1, and R2 substituent effects, and, finally, the role of aryl and/or heteroaryl groups. It is worth stressing that the DFT calculations were consistent with the experimental data: hindered substituents at the bridge and the ortho-positions on the aniline fragments may increase the stereoselectivity of propene polymerization, as well as moving from a carbon- to silicon-bridge. Analogously, the %VBur steric maps enabled an easy visualization of the ligand steric hindrance, still reproducing the experimental trend. However, a better understanding of the interplay of these steric and electronic contributions was achieved by the ASM–NEDA analysis and its ΔE(Tot) energy decomposition into the ΔEStrain and ΔEInt contributions. The former term is more relevant than the latter for the origin of stereocontrol, and the additional partitioning of the ΔEStrain into the ΔEStrain(Cat) and ΔEStrain(Mon) revealed how subtle ligand modification (see, e.g., the metallacycle size and the asymmetric R1 and R2 substitution on X atom, Scheme 1) increases the propene stereoselectivity. Overall, the complete DFT/%VBur/ASM–NEDA approach, is, in our opinion, a powerful tool for the fine tuning of catalyst design/polymer properties [62].

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules28093768/s1, Table S1: experimental data set. Table S2: NEDA decomposition for ΔEInt. term. Table S3: Effect of dispersion corrections on DFT electronic energies. Table S4: Effect of dispersion corrections on NEDA energetic terms. Figures S1–S3: propene insertion TSs for system IIc, Ib, IIb. Figure S4: steric maps for systems Ib, IIb, IIe and IVa. Figure S5: propene deformation for systems Ia, IIa, IIIb. Figures S6 and S7: propene insertion TSs for system IIe, IVa.

Author Contributions

Investigation, O.D.; Supervision, C.D.R. and G.T. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by University of Naples Federico II, grant number “Ricerca di Ateneo 2017, DR_409_2017”. O.D. thanks the Scuola Superiore Meridionale for a PhD Grant.

Conflicts of Interest

The authors declare no conflict of interest.

Sample Availability

Samples of the compounds are not available from the authors.

References

  1. Resconi, L.; Cavallo, L.; Fait, A.; Piemontesi, F. Selectivity in Propene Polymerization with Metallocene Catalysts. Chem. Rev. 2000, 100, 1253–1346. [Google Scholar] [CrossRef] [PubMed]
  2. Correa, A.; Talarico, G.; Cavallo, L. Regiochemistry of propene insertion with group 4 polymerization catalysts from a theoretical perspective. J. Organomet. Chem. 2007, 692, 4519–4527. [Google Scholar] [CrossRef]
  3. Busico, V.; Cipullo, R.; Talarico, G.; Segre, A.L.; Caporaso, L. High-Field 13C NMR Characterization of Ethene-1-13C/Propene Copolymers Prepared with Cs-Symmetric ansa-Metallocene Catalysts: A Deeper Insight into the Regio- and Stereoselectivity of Syndiotactic Propene Polymerization. Macromolecules 1998, 31, 8720–8724. [Google Scholar] [CrossRef]
  4. Talarico, G.; Budzelaar, P.H.M. Variability of Chain Transfer to Monomer Step in Olefin Polymerization. Organometallics 2008, 27, 4098–4107. [Google Scholar] [CrossRef]
  5. Talarico, G.; Budzelaar, P.H.M. A Second Transition State for Chain Transfer to Monomer in Olefin Polymerization Promoted by Group 4 Metal Catalysts. J. Am. Chem. Soc. 2006, 128, 4524–4525. [Google Scholar] [CrossRef]
  6. Cavallo, L.; Guerra, G. A Density Functional and Molecular Mechanics Study of β-Hydrogen Transfer in Homogeneous Ziegler–Natta Catalysis. Macromolecules 1996, 29, 2729–2737. [Google Scholar] [CrossRef]
  7. De Rosa, C.; Auriemma, F. Single site metallorganic polymerization catalysis as a method to probe the properties of polyolefins. Polym. Chem. 2011, 2, 2155. [Google Scholar] [CrossRef]
  8. Baier, M.C.; Zuideveld, M.A.; Mecking, S. Post-Metallocenes in the Industrial Production of Polyolefins. Angew. Chem. Int. Ed. 2014, 53, 9722–9744. [Google Scholar] [CrossRef]
  9. Domski, G.J.; Edson, J.B.; Keresztes, I.; Lobkovsky, E.B.; Coates, G.W. Synthesis of a new olefin polymerization catalyst supported by an sp3-C donor via insertion of a ligand-appended alkene into the Hf–C bond of a neutral pyridylamidohafnium trimethyl complex. Chem. Commun. 2008, 46, 6137–6139. [Google Scholar] [CrossRef]
  10. Domski, G.J.; Eagan, J.M.; De Rosa, C.; Di Girolamo, R.; LaPointe, A.M.; Lobkovsky, E.B.; Talarico, G.; Coates, G.W. Combined Experimental and Theoretical Approach for Living and Isoselective Propylene Polymerization. ACS Catal. 2017, 7, 6930–6937. [Google Scholar] [CrossRef]
  11. Boussie, T.R.; Diamond, G.M.; Goh, C.; Hall, K.A.; LaPointe, A.M.; Leclerc, M.K.; Murphy, V.; Shoemaker, J.A.W.; Turner, H.; Rosen, R.K.; et al. Nonconventional Catalysts for Isotactic Propene Polymerization in Solution Developed by Using High-Throughput-Screening Technologies. Angew. Chem. Int. Ed. 2006, 45, 3278–3283. [Google Scholar] [CrossRef] [PubMed]
  12. Cueny, E.S.; Johnson, H.C.; Anding, B.J.; Landis, C.R. Mechanistic Studies of Hafnium-Pyridyl Amido-Catalyzed 1-Octene Polymerization and Chain Transfer Using Quench-Labeling Methods. J. Am. Chem. Soc. 2017, 139, 11903–11912. [Google Scholar] [CrossRef] [PubMed]
  13. Shang, R.; Gao, H.; Luo, F.; Li, Y.; Wang, B.; Ma, Z.; Pan, L.; Li, Y. Functional Isotactic Polypropylenes via Efficient Direct Copolymerizations of Propylene with Various Amino-Functionalized α-Olefins. Macromolecules 2019, 52, 9280–9290. [Google Scholar] [CrossRef]
  14. Arriola, D.J.; Carnahan, E.M.; Hustad, P.D.; Kuhlman, R.L.; Wenzel, T.T. Catalytic Production of Olefin Block Copolymers via Chain Shuttling Polymerization. Science 2006, 312, 714–719. [Google Scholar] [CrossRef]
  15. Rocchigiani, L.; Busico, V.; Pastore, A.; Talarico, G.; Macchioni, A. Unusual Hafnium–Pyridylamido/ERn Heterobimetallic Adducts (ERn=ZnR2 or AlR3). Angew. Chem. Int. Ed. 2014, 53, 2157–2161. [Google Scholar] [CrossRef] [PubMed]
  16. Auriemma, F.; De Rosa, C.; Scoti, M.; Di Girolamo, R.; Malafronte, A.; Talarico, G.; Carnahan, E. Unveiling the molecular structure of ethylene/1-octene multi-block copolymers from chain shuttling technology. Polymer 2018, 154, 298–304. [Google Scholar] [CrossRef]
  17. Urciuoli, G.; Vittoria, A.; Talarico, G.; Luise, D.; De Rosa, C.; Busico, V.; Cipullo, R.; Ruiz de Ballesteros, O.; Auriemma, F. In-Depth Analysis of the Nonuniform Chain Microstructure of Multiblock Copolymers from Chain-Shuttling Polymerization. Macromolecules 2021, 54, 10891–10902. [Google Scholar] [CrossRef]
  18. Froese, R.D.J.; Hustad, P.D.; Kuhlman, R.L.; Wenzel, T.T. Mechanism of activation of a hafnium pyridyl–amide olefin polymerization catalyst: Ligand modification by monomer. J. Am. Chem. Soc. 2007, 129, 7831–7840. [Google Scholar] [CrossRef]
  19. Zuccaccia, C.; Busico, V.; Cipullo, R.; Talarico, G.; Froese, R.D.; Vosejpka, P.C.; Hustad, P.D.; Macchioni, A. On the first insertion of α-olefins in hafnium pyridyl-amido polymerization catalysts. Organometallics 2009, 28, 5445–5458. [Google Scholar] [CrossRef]
  20. Zuccaccia, C.; Macchioni, A.; Busico, V.; Cipullo, R.; Talarico, G.; Alfano, F.; Boone, H.W.; Frazier, K.A.; Hustad, P.D.; Stevens, J.C.; et al. Intra- and Intermolecular NMR Studies on the Activation of Arylcyclometallated Hafnium Pyridyl-Amido Olefin Polymerization Precatalysts. J. Am. Chem. Soc. 2008, 130, 10354–10368. [Google Scholar] [CrossRef]
  21. Busico, V.; Cipullo, R.; Pellecchia, R.; Rongo, L.; Talarico, G.; Macchioni, A.; Zuccaccia, C.; Froese, R.D.J.; Hustad, P.D. “Uni et Trini”: In Situ Diversification of (Pyridylamide)hafnium(IV) Catalysts. Macromolecules 2009, 42, 4369–4373. [Google Scholar] [CrossRef]
  22. De Rosa, C.; Di Girolamo, R.; Talarico, G. Expanding the Origin of Stereocontrol in Propene Polymerization Catalysis. ACS Catal. 2016, 6, 3767–3770. [Google Scholar] [CrossRef]
  23. Miller, S.A.; Bercaw, J.E. Mechanism of Isotactic Polypropylene Formation with C1-Symmetric Metallocene Catalysts. Organometallics 2006, 25, 3576–3592. [Google Scholar] [CrossRef]
  24. Cossee, P. Ziegler-Natta catalysis I. Mechanism of polymerization of α-olefins with Ziegler-Natta catalysts. J. Catal. 1964, 3, 80–88. [Google Scholar] [CrossRef]
  25. Cicolella, A.; Romano, E.; Barone, V.; De Rosa, C.; Talarico, G. Metallocenes and Beyond for Propene Polymerization: Energy Decomposition of Density Functional Computations Unravels the Different Interplay of Stereoelectronic Effects. Organometallics 2022, 41, 3872–3883. [Google Scholar] [CrossRef]
  26. Romano, E.; Budzelaar, P.H.M.; De Rosa, C.; Talarico, G. Unconventional Stereoerror Formation Mechanisms in Nonmetallocene Propene Polymerization Systems Revealed by DFT Calculations. J. Phys. Chem. A 2022, 126, 6203–6209. [Google Scholar] [CrossRef] [PubMed]
  27. Falivene, L.; Cao, Z.; Petta, A.; Serra, L.; Poater, A.; Oliva, R.; Scarano, V.; Cavallo, L. Towards the online computer-aided design of catalytic pockets. Nat. Chem. 2019, 11, 872–879. [Google Scholar] [CrossRef]
  28. Falivene, L.; Cavallo, L.; Talarico, G. Buried Volume Analysis for Propene Polymerization Catalysis Promoted by Group 4 Metals: A Tool for Molecular Mass Prediction. ACS Catal. 2015, 5, 6815–6822. [Google Scholar] [CrossRef]
  29. Vermeeren, P.; van der Lubbe, S.C.C.; Fonseca Guerra, C.; Bickelhaupt, F.M.; Hamlin, T.A. Understanding chemical reactivity using the activation strain model. Nat. Protoc. 2020, 15, 649–667. [Google Scholar] [CrossRef]
  30. Bickelhaupt, F.M.; Houk, K.N. Analyzing Reaction Rates with the Distortion/Interaction-Activation Strain Model. Angew. Chem. Int. Ed. 2017, 56, 10070–10086. [Google Scholar] [CrossRef]
  31. Zhao, Y.; Xu, X.; Wang, Y.; Liu, T.; Li, H.; Zhang, Y.; Wang, L.; Wang, X.; Zhao, S.; Luo, Y. Ancillary ligand effects on α-olefin polymerization catalyzed by zirconium metallocene: A computational study. RSC Adv. 2022, 12, 21111–21121. [Google Scholar] [CrossRef] [PubMed]
  32. Falivene, L.; Kozlov, S.M.; Cavallo, L. Constructing Bridges between Computational Tools in Heterogeneous and Homogeneous Catalysis. ACS Catal. 2018, 8, 5637–5656. [Google Scholar] [CrossRef]
  33. Corradini, P.; Guerra, G.; Cavallo, L. Do New Century Catalysts Unravel the Mechanism of Stereocontrol of old Ziegler–Natta Catalysts? Acc. Chem. Res. 2004, 37, 231–241. [Google Scholar] [CrossRef]
  34. Bahri-Laleh, N.; Hanifpour, A.; Mirmohammadi, S.A.; Poater, A.; Nekoomanesh-Haghighi, M.; Talarico, G.; Cavallo, L. Computational modeling of heterogeneous Ziegler-Natta catalysts for olefins polymerization. Prog. Polym. Sci. 2018, 84, 89–114. [Google Scholar] [CrossRef]
  35. Kulyabin, P.S.; Uborsky, D.V.; Voskoboynikov, A.Z.; Canich, J.A.M.; Hagadorn, J.R. Pyridylamido hafnium complexes with a silylene bridge: Synthesis and olefin polymerization. Dalton Trans. 2020, 49, 6693–6702. [Google Scholar] [CrossRef] [PubMed]
  36. Luconi, L.; Rossin, A.; Tuci, G.; Tritto, I.; Boggioni, L.; Klosin, J.J.; Theriault, C.N.; Giambastiani, G. Facing unexpected reactivity paths with ZrIV–pyridylamido polymerization catalysts. Chem. Eur. J. 2012, 18, 671–687. [Google Scholar] [CrossRef] [PubMed]
  37. Li, G.; Zuccaccia, C.; Tedesco, C.; D’Auria, I.; Macchioni, A.; Pellecchia, C. NMR Spectroscopy and X-Ray Characterisation of Cationic N-Heteroaryl-Pyridylamido Zr(IV) Complexes: A Further Level of Complexity for the Elusive Active Species of Pyridylamido Olefin Polymerisation Catalysts. Chem. Eur. J. 2014, 20, 232–244. [Google Scholar] [CrossRef]
  38. Annunziata, L.; Pappalardo, D.; Tedesco, C.; Pellecchia, C. Isotactic-specific polymerization of propene by a Cs-symmetric zirconium (IV) complex bearing a dianionic tridentate [−NNN−] amidomethylpyrrolidepyridine ligand. Macromolecules 2009, 42, 5572–5578. [Google Scholar] [CrossRef]
  39. De Rosa, C.; Di Girolamo, R.; Muñoz-García, A.B.; Pavone, M.; Talarico, G. Breaking Symmetry Rules Enhance the Options for Stereoselective Propene Polymerization Catalysis. Macromolecules 2020, 53, 2959–2964. [Google Scholar] [CrossRef]
  40. Talarico, G.; Budzelaar, P.H.M. Analysis of Stereochemistry Control in Homogeneous Olefin Polymerization Catalysis. Organometallics 2014, 33, 5974–5982. [Google Scholar] [CrossRef]
  41. Falivene, L.; Barone, V.; Talarico, G. Unraveling the role of entropy in tuning unimolecular vs. bimolecular reaction rates: The case of olefin polymerization catalyzed by transition metals. Mol. Catal. 2018, 452, 138–144. [Google Scholar] [CrossRef]
  42. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision A.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
  43. Becke, A. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
  44. Perdew, J.P. Density-functional approximation for the correlation energy of the inhomogeneous electron gas. Phys. Rev. B 1986, 33, 8822–8824, Erratum in Phys. Rev. B 1986, 34, 7406–7406. [Google Scholar]
  45. Schäfer, A.; Horn, H.; Ahlrichs, R. Fully optimized contracted Gaussian basis sets for atoms lithium to krypton. J. Chem. Phys. 1992, 97, 2571–2577. [Google Scholar] [CrossRef]
  46. Hay, P.J.; Wadt, W.R. Ab initio effective core potentials for molecular calculations. Potentials for K to Au including the outermost core orbitals. J. Chem. Phys. 1985, 82, 299–310. [Google Scholar] [CrossRef]
  47. Weigend, F.; Furche, F.; Ahlrichs, R. Gaussian basis sets of quadruple zeta valence quality for atoms H–Kr. J. Chem. Phys. 2003, 119, 12753–12762. [Google Scholar] [CrossRef]
  48. Wadt, W.R.; Hay, P.J. Ab initio effective core potentials for molecular calculations. Potentials for main group elements Na to Bi. J. Chem. Phys. 1985, 82, 284–298. [Google Scholar] [CrossRef]
  49. Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
  50. Barone, V.; Cossi, M. Quantum Calculation of Molecular Energies and Energy Gradients in Solution by a Conductor Solvent Model. J. Phys. Chem. A 1998, 102, 1995–2001. [Google Scholar] [CrossRef]
  51. Glendening, E.D. Natural Energy Decomposition Analysis:  Explicit Evaluation of Electrostatic and Polarization Effects with Application to Aqueous Clusters of Alkali Metal Cations and Neutrals. J. Am. Chem. Soc. 1996, 118, 2473–2482. [Google Scholar] [CrossRef]
  52. Talarico, G.; Budzelaar, P.H.M. α-Agostic Interactions and Growing Chain Orientation for Olefin Polymerization Catalysts. Organometallics 2016, 35, 47–54. [Google Scholar] [CrossRef]
  53. Eagan, J.M.; Xu, J.; Di Girolamo, R.; Thurber, C.M.; Macosko, C.W.; LaPointe, A.M.; Bates, F.S.; Coates, G.W. Combining polyethylene and polypropylene: Enhanced performance with PE/iPP multiblock polymers. Science 2017, 355, 814–816. [Google Scholar] [CrossRef] [PubMed]
  54. De Rosa, C.; Di Girolamo, R.; Auriemma, F.; D’Avino, M.; Talarico, G.; Cioce, C.; Scoti, M.; Coates, G.W.; Lotz, B. Oriented Microstructures of Crystalline-Crystalline Block Copolymers Induced by Epitaxy and Competitive and Confined Crystallization. Macromolecules 2016, 49, 5576–5586. [Google Scholar] [CrossRef]
  55. De Rosa, C.; Di Girolamo, R.; Malafronte, A.; Scoti, M.; Talarico, G.; Auriemma, F.; Ruiz de Ballesteros, O. Polyolefins based crystalline block copolymers: Ordered nanostructures from control of crystallization. Polymer 2020, 196, 122423. [Google Scholar] [CrossRef]
  56. Di Girolamo, R.; Santillo, C.; Malafronte, A.; Scoti, M.; De Stefano, F.; Talarico, G.; Coates, G.W.; De Rosa, C. Structure and morphology of isotactic polypropylene–polyethylene block copolymers prepared with living and stereoselective catalyst. Polym. Chem. 2022, 13, 2950–2963. [Google Scholar] [CrossRef]
  57. De Rosa, C.; Di Girolamo, R.; Auriemma, F.; Talarico, G.; Malafronte, A.; Scarica, C.; Scoti, M. Controlling Size and Orientation of Lamellar Microdomains in Crystalline Block Copolymers. ACS Appl. Mater. Interfaces 2017, 9, 31252–31259. [Google Scholar] [CrossRef]
  58. Domski, G.J.; Rose, J.M.; Coates, G.W.; Bolig, A.D.; Brookhart, M. Living alkene polymerization: New methods for the precision synthesis of polyolefins. Prog. Polym. Sci. 2007, 32, 30–92. [Google Scholar] [CrossRef]
  59. De Rosa, C.; Ruiz de Ballesteros, O.; Auriemma, F.; Talarico, G.; Scoti, M.; Di Girolamo, R.; Malafronte, A.; Piemontesi, F.; Liguori, D.; Camurati, I.; et al. Crystallization Behavior of Copolymers of Isotactic Poly(1-butene) with Ethylene from Ziegler–Natta Catalyst: Evidence of the Blocky Molecular Structure. Macromolecules 2019, 52, 9114–9127. [Google Scholar] [CrossRef]
  60. De Rosa, C.; Ruiz de Ballesteros, O.; Di Girolamo, R.; Malafronte, A.; Auriemma, F.; Talarico, G.; Scoti, M. The blocky structure of Ziegler–Natta “random” copolymers: Myths and experimental evidence. Polym. Chem. 2020, 11, 34–38. [Google Scholar] [CrossRef]
  61. Cipullo, R.; Busico, V.; Fraldi, N.; Pellecchia, R.; Talarico, G. Improving the Behavior of Bis(phenoxyamine) Group 4 Metal Catalysts for Controlled Alkene Polymerization. Macromolecules 2009, 42, 3869–3872. [Google Scholar] [CrossRef]
  62. Han, J.W.; Hollmann, F.; Luque, R.; Song, I.K.; Talarico, G.; Tatsumi, T.; Yan, N. Molecular Catalysis for the Chemistry of the future: A perspective. Mol. Catal. 2022, 522, 112233. [Google Scholar] [CrossRef]
Molecules 28 03768 ch001 550
Chart 1. Systems analyzed in this study.
Chart 1. Systems analyzed in this study.
Molecules 28 03768 ch001
Molecules 28 03768 sch001 550
Scheme 1. Ligand modification with the monomer via α-olefin insertion into Hf-aryl bond [18,19,20,21].
Scheme 1. Ligand modification with the monomer via α-olefin insertion into Hf-aryl bond [18,19,20,21].
Molecules 28 03768 sch001
Molecules 28 03768 g001 550
Figure 1. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re (A), and si enantioface (B) for system Ia. Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the main sterical interactions and H atoms are omitted for clarity.
Figure 1. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re (A), and si enantioface (B) for system Ia. Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the main sterical interactions and H atoms are omitted for clarity.
Molecules 28 03768 g001
Molecules 28 03768 g002 550
Figure 2. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re (A) and si enantioface (B) for system IIa. Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the ligand-monomer interactions and H atoms are omitted for clarity.
Figure 2. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re (A) and si enantioface (B) for system IIa. Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the ligand-monomer interactions and H atoms are omitted for clarity.
Molecules 28 03768 g002
Molecules 28 03768 g003 550
Figure 3. Steric maps of neutral mono-inserted species for systems Ia (A), IIa (B), IIc (C), IId (D), IIIa (E), and IIIb (F) with the %VBur values obtained for each quadrant. Propene drawing is reported in yellow. The steric hindrance of the ligand framework is described through a color scale (on the right), which ranges from red to blue colors, indicating the more- and less-hindered zones, respectively.
Figure 3. Steric maps of neutral mono-inserted species for systems Ia (A), IIa (B), IIc (C), IId (D), IIIa (E), and IIIb (F) with the %VBur values obtained for each quadrant. Propene drawing is reported in yellow. The steric hindrance of the ligand framework is described through a color scale (on the right), which ranges from red to blue colors, indicating the more- and less-hindered zones, respectively.
Molecules 28 03768 g003
Molecules 28 03768 g004 550
Figure 4. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re and si enantiofaces for systems IId (A,B), and IIIa (C,D). Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the ligand-monomer interactions and H atoms are omitted for clarity.
Figure 4. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re and si enantiofaces for systems IId (A,B), and IIIa (C,D). Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the ligand-monomer interactions and H atoms are omitted for clarity.
Molecules 28 03768 g004
Molecules 28 03768 g005 550
Figure 5. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re (A) and si enantioface (B) for system IIIb. Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the ligand-monomer interactions and H atoms are omitted for clarity.
Figure 5. DFT-optimized geometries for 1,2 propene insertion TS into the growing polymer chain with re (A) and si enantioface (B) for system IIIb. Propene is shown in grey and the growing polymer chain (represented by iBu group) in green. The dashed red arrows show the ligand-monomer interactions and H atoms are omitted for clarity.
Molecules 28 03768 g005
Molecules 28 03768 sch002 550
Scheme 2. ΔETot partitioning through ASM-NEDA model.
Scheme 2. ΔETot partitioning through ASM-NEDA model.
Molecules 28 03768 sch002
Table
Table 1. DFT electronic energies (Gibbs energies) in kcal/mol for the propene stereoselectivity at the preferred site (Ia–IIIb) and at both the diastereotopic active sites (IVa).
Table 1. DFT electronic energies (Gibbs energies) in kcal/mol for the propene stereoselectivity at the preferred site (Ia–IIIb) and at both the diastereotopic active sites (IVa).
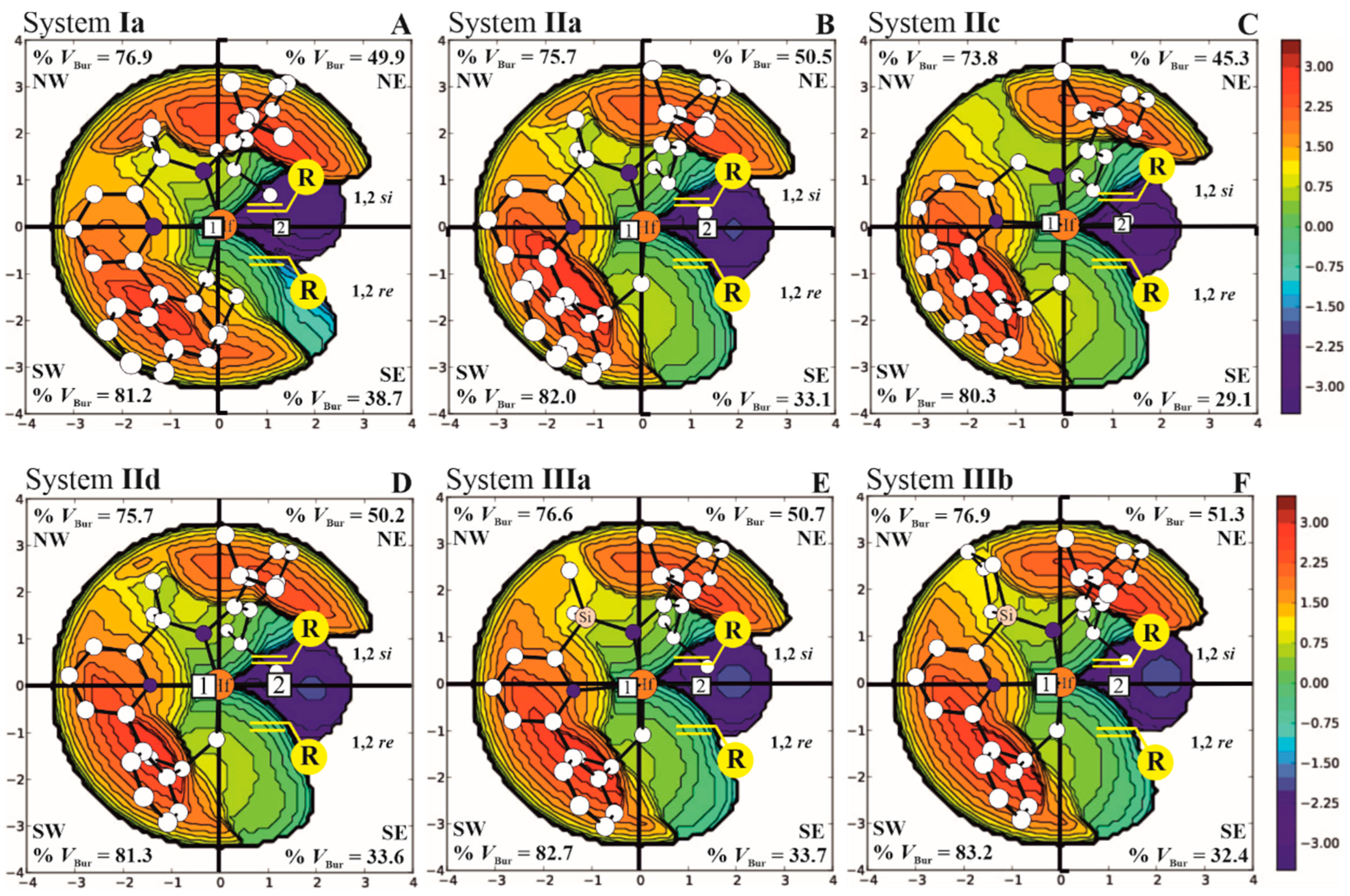
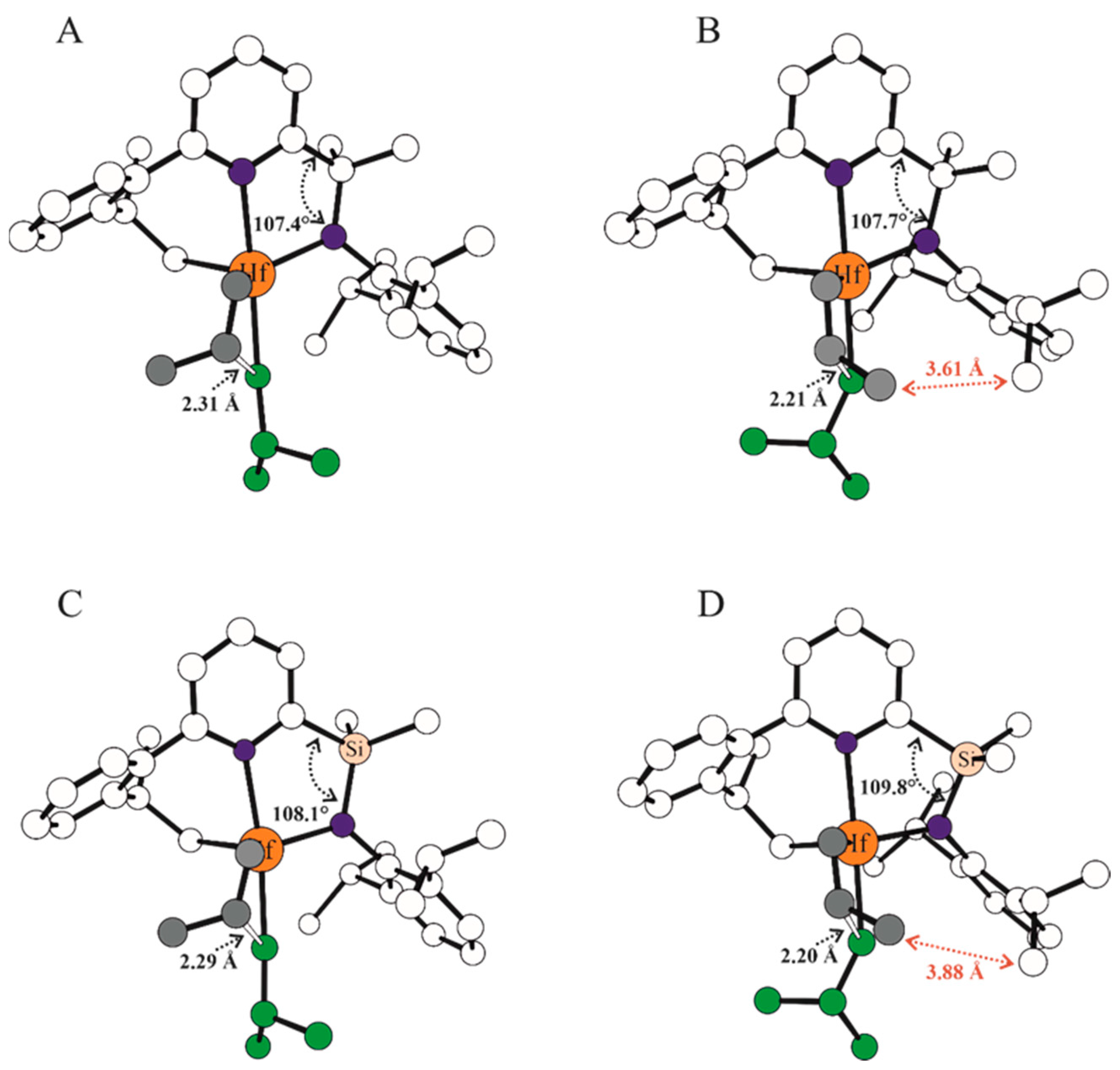
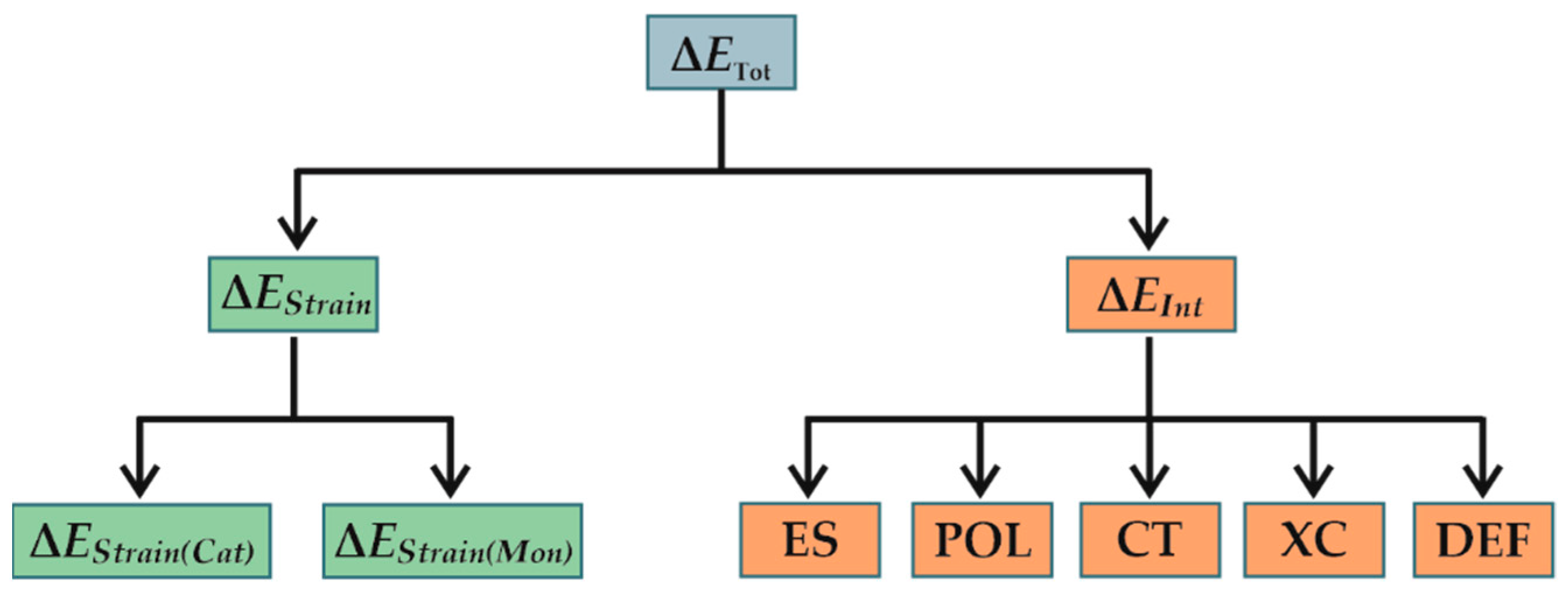
SystemsΔE(ΔG)Stereo a)ΔE(ΔG)Stereo b)
Ia2.4 (1.4)2.8 (1.4)
Ib−0.6 (−0.2)−0.5 (−0.1)
IIa4.0 (3.1)3.6 (2.8)
IIb0.8 (0.5)0.5 (0.2)
IIc1.2 (1.4)1.3 (2.0)
IId3.8 (3.1)3.2 (2.5)
IIe3.8 (4.4)3.8 (4.1)
IIIa2.2 (2.5)2.2 (2.5)
IIIb4.6 (4.1)4.6 (4.1)
IVa (site 1)1.8 (2.4)2.2 (2.4)
IVa (site 2)3.1 (2.4)3.6 (2.9)
a) DFT electronic energies (free energies) for the stereoselectivity at preferred site including dispersion and solvent corrections (PCM model) Differences are calculated with respect to the favored propene enantioface insertion TS (1,2 re). b) DFT electronic energies (free energies) for the stereoselectivity at preferred site including dispersion corrections. Differences are calculated with respect to the favored propene enantioface insertion TS (1,2 re).
Table
Table 2. ASM–NEDA results for 1,2 re and 1,2 si propene enantioface insertion TSs calculated at the preferred site for pyridylamido-Hf systems (Ia-IIIb) and at both diastereotopic sites for system IVa.
Table 2. ASM–NEDA results for 1,2 re and 1,2 si propene enantioface insertion TSs calculated at the preferred site for pyridylamido-Hf systems (Ia-IIIb) and at both diastereotopic sites for system IVa.
1,2 re Insertion1,2 si Insertion1,2 (si-re) Insertion a)
ΔETotΔEIntΔEStrainΔEStrain(Cat)ΔEStrain(Mon)ΔETotΔEIntΔEStrainΔEStrain(Cat)ΔEStrain(Mon)ΔΔEIntΔΔEStrainΔΔEStrain(Cat)ΔΔEStrain(Mon)
Ia−12.0−46.934.915.819.1−9.2−45.736.518.817.71.21.63.0−1.4
Ib−8.5−45.837.318.718.6−9.0−45.536.517.818.70.3−0.8−0.90.1
IIa−2.8−41.939.124.714.40.8−45.846.627.419.2−3.97.52.74.8
IIb−1.4−42.240.925.315.6−0.9−45.144.225.119.2−2.93.4−0.23.5
IIc−3.7−43.039.323.316.0−2.5−46.443.923.720.2−3.44.60.44.2
IId−4.0−40.936.922.214.7−0.8−44.944.124.719.4−4.07.22.54.7
IIe−5.2−41.936.721.415.3−1.4−45.343.925.318.6−3.47.23.93.3
IIIa−0.6−40.539.924.115.81.5−45.947.428.119.3−5.47.54.03.5
IIIb−1.3−40.439.123.016.13.3−43.646.724.422.3−3.27.61.46.2
IVa (site1)−0.8−44.739.922.721.21.4−45.046.424.921.5−0.32.52.20.3
IVa (site2)−2.3−47.845.525.220.31.3−46.547.927.720.21.32.42.5−0.1
a) ΔΔE values correspond to the difference between each ΔE term calculated for the 1,2 si insertion and 1,2 re insertion. The 1,2 re insertion ΔE values are the reference points. Values are reported in kcal/mol.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

D’Anania, O.; De Rosa, C.; Talarico, G. A Computational Evaluation of the Steric and Electronic Contributions in Stereoselective Olefin Polymerization with Pyridylamido-Type Catalysts. Molecules 2023, 28, 3768. https://doi.org/10.3390/molecules28093768

AMA Style

D’Anania O, De Rosa C, Talarico G. A Computational Evaluation of the Steric and Electronic Contributions in Stereoselective Olefin Polymerization with Pyridylamido-Type Catalysts. Molecules. 2023; 28(9):3768. https://doi.org/10.3390/molecules28093768

Chicago/Turabian Style

D’Anania, Olga, Claudio De Rosa, and Giovanni Talarico. 2023. "A Computational Evaluation of the Steric and Electronic Contributions in Stereoselective Olefin Polymerization with Pyridylamido-Type Catalysts" Molecules 28, no. 9: 3768. https://doi.org/10.3390/molecules28093768

APA Style

D’Anania, O., De Rosa, C., & Talarico, G. (2023). A Computational Evaluation of the Steric and Electronic Contributions in Stereoselective Olefin Polymerization with Pyridylamido-Type Catalysts. Molecules, 28(9), 3768. https://doi.org/10.3390/molecules28093768

Article Metrics

Back to TopTop