The Relationship between Gelation Behavior and the Amount of Polymer Dose per Silica Surface Area of “Shake-Gels” Consisting of Silica Nanoparticles and Poly(Ethylene Oxide)
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of Shake-Gel Samples
2.3. Observation of Flowability Change by End-over-End Rotation
2.4. Viscosity Measurements
3. Results
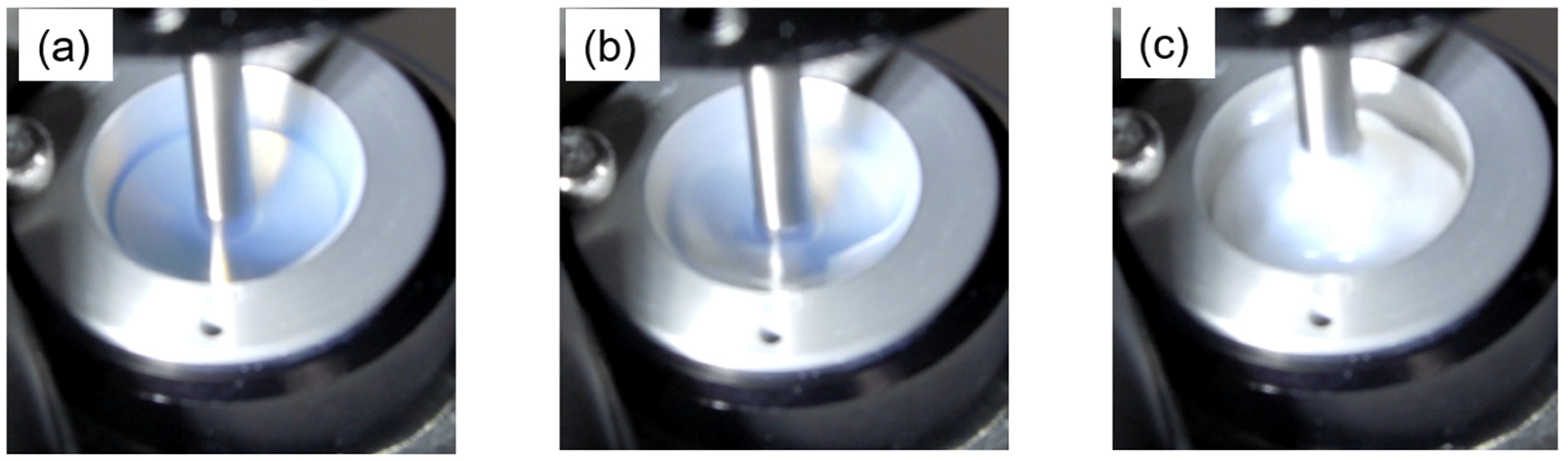
3.1. On Visual Changes of Prepared Suspension
3.2. Observation of Flowability Change by End-over-End Rotation
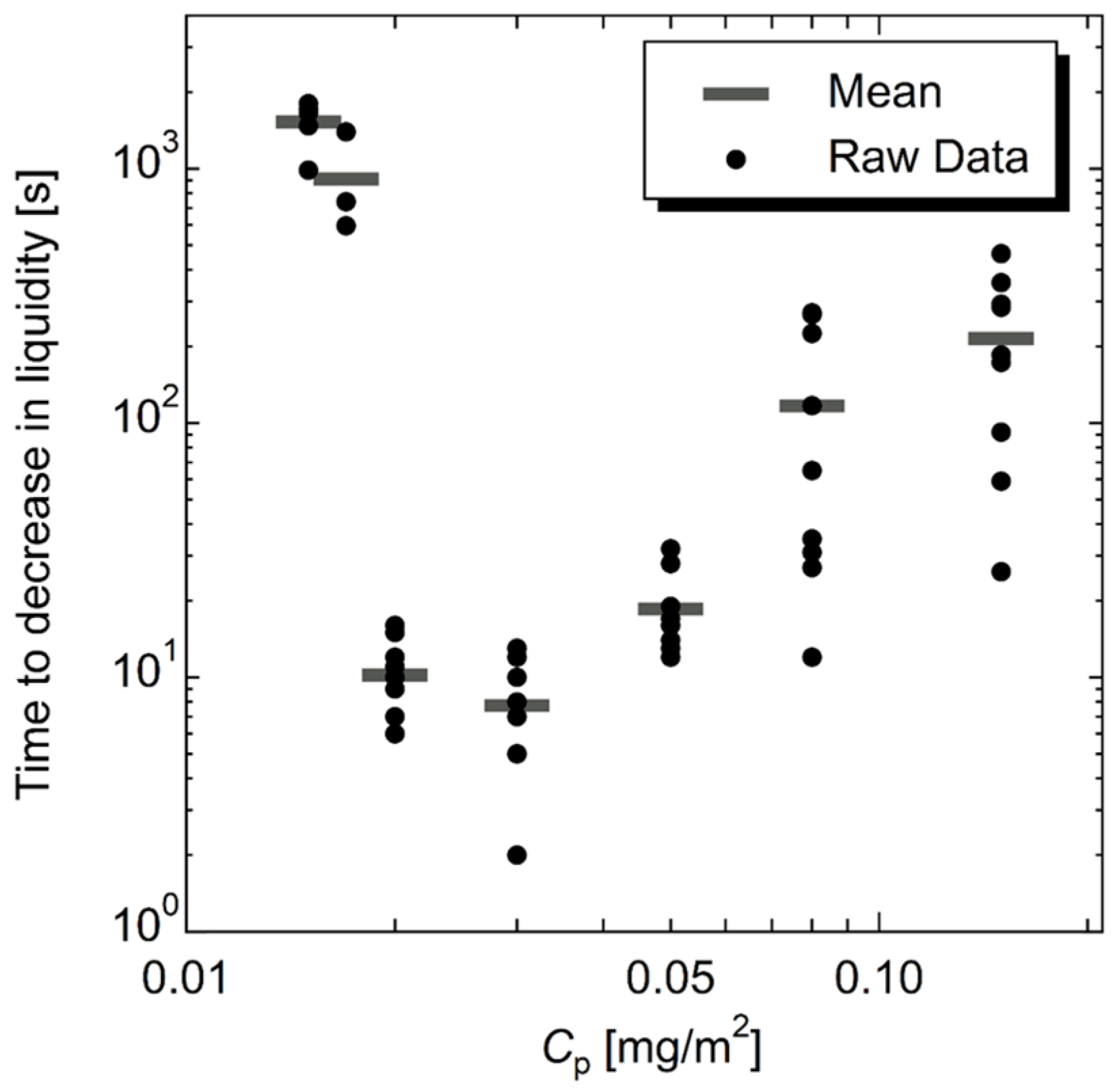
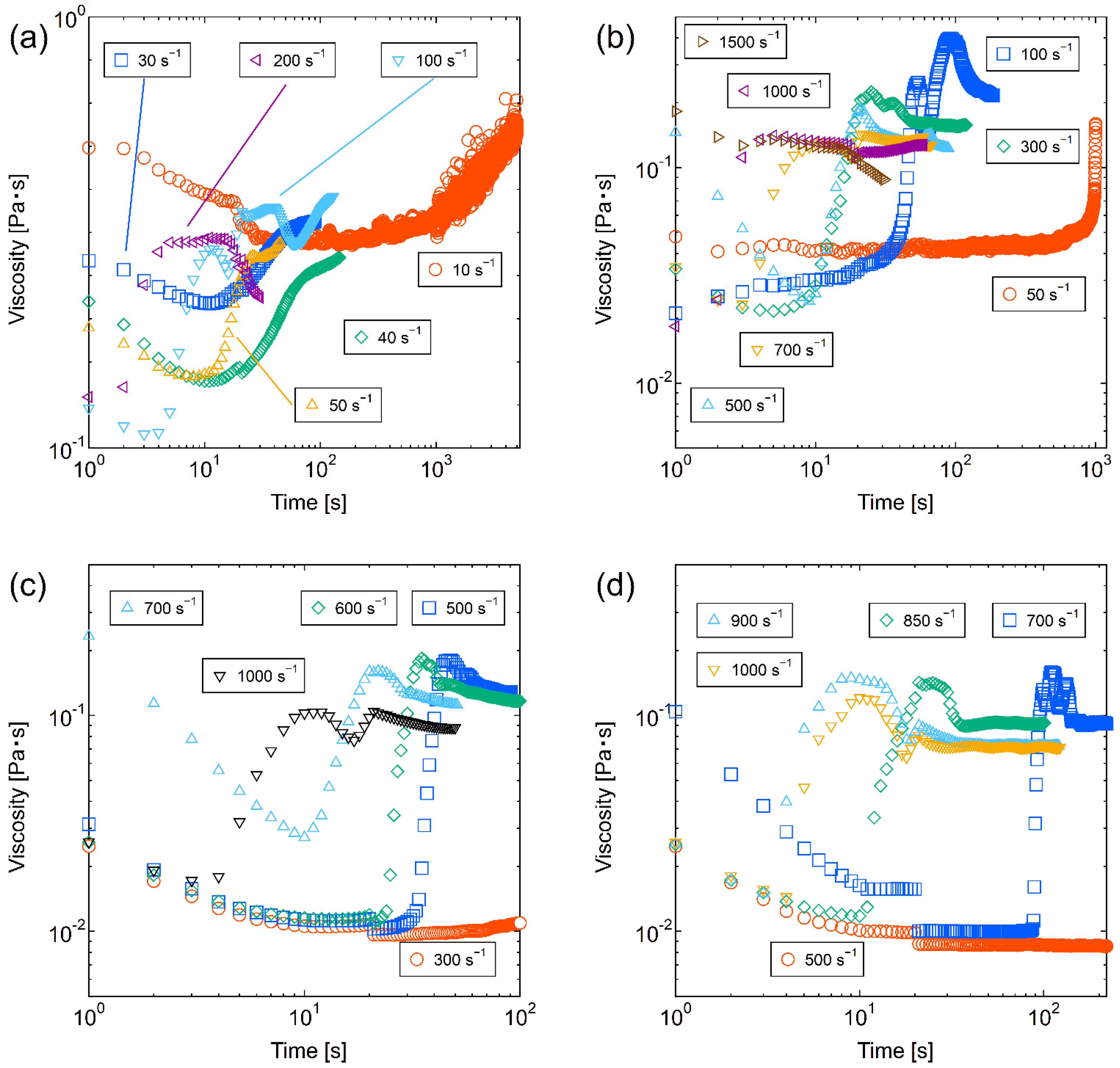
3.3. Critical Shear Rate
3.4. Gelation Time by Applying Constant Shear Flow
4. Discussion
4.1. Effect of the Value of Cp
4.2. Effect of the Different Flow Types
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Luckham, P.F.; Rossi, S. The Colloidal and Rheological Properties of Bentonite Suspensions. Adv. Colloid Interface Sci. 1999, 82, 43–92. [Google Scholar] [CrossRef]
- Nakamura, H.; Makino, S.; Ishii, M. Effects of Electrostatic Interaction on Rheological Behavior and Microstructure of Concentrated Colloidal Suspensions. Colloids Surf. A Physicochem. Eng. Asp. 2021, 623, 126576. [Google Scholar] [CrossRef]
- Tsujimoto, Y.; Kobayashi, M.; Adachi, Y. Viscosity of Dilute Na-Montmorillonite Suspensions in Electrostatically Stable Condition under Low Shear Stress. Colloids Surf. A Physicochem. Eng. Asp. 2014, 440, 20–26. [Google Scholar] [CrossRef]
- Kobayashi, M.; Adachi, Y.; Ooi, S. On the Steady Shear Viscosity of Coagulated Suspensions. Nihon Reoroji Gakkaishi 2000, 28, 143–144. [Google Scholar] [CrossRef]
- Mewis, J.; Wagner, N.J. Colloidal Suspension Rheology; Cambridge University Press: Cambridge, UK, 2011; Volume 9780521515993. [Google Scholar]
- Lee, Y.S.; Wetzel, E.D.; Wagner, N.J. The Ballistic Impact Characteristics of Kevlar® Woven Fabrics Impregnated with a Colloidal Shear Thickening Fluid. J. Mater. Sci. 2003, 38, 2825–2833. [Google Scholar] [CrossRef]
- Zaman, A.A.; Delorme, N. Effect of Polymer Bridging on Rheological Properties of Dispersions of Charged Silica Particles in the Presence of Low-Molecular-Weight Physically Adsorbed Poly(Ethylene Oxide). Rheol. Acta 2002, 41, 408–417. [Google Scholar] [CrossRef]
- Saito, Y.; Hirose, Y.; Otsubo, Y. Effect of Poly(Ethylene Oxide) on the Rheological Behavior of Silica Suspensions. Rheol. Acta 2011, 50, 291–301. [Google Scholar] [CrossRef]
- Paul, C.; Hiemenz, R.R. Principles of Colloid and Surface Chemistry, Revised and Expanded, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2016; ISBN 9781315274287. [Google Scholar]
- Otsubo, Y.; Watanabe, K. Rheological Studies on Bridging Flocculation. Colloids Surf. 1990, 50, 341–352. [Google Scholar] [CrossRef]
- Pozzo, D.C.; Hollabaugh, K.R.; Walker, L.M. Rheology and Phase Behavior of Copolymer-Templated Nanocomposite Materials. J. Rheol. 2007, 51, 585. [Google Scholar] [CrossRef]
- Geonzon, L.C.; Kobayashi, M.; Sugimoto, T.; Adachi, Y. Study on the Kinetics of Adsorption of Poly ( Ethylene Oxide ) onto a Silica Particle Using Optical Tweezers and Microfluidics. Colloids Surf. A Physicochem. Eng. Asp. 2022, 642, 128691. [Google Scholar] [CrossRef]
- Liu, S.F.; Lafuma, F.; Audebert, R. Rheological Behavior of Moderately Concentrated Silica Suspensions in the Presence of Adsorbed Poly(Ethylene Oxide). Colloid Polym. Sci. 1994, 272, 196–203. [Google Scholar] [CrossRef]
- Cabane, B.; Wong, K.; Lindner, P.; Lafuma, F. Shear Induced Gelation of Colloidal Dispersions. J. Rheol. 1998, 41, 531. [Google Scholar] [CrossRef]
- Zebrowski, J.; Prasad, V.; Zhang, W.; Walker, L.M.; Weitz, D.A. Shake-Gels: Shear-Induced Gelation of Laponite–PEO Mixtures. Colloids Surf. A Physicochem. Eng. Asp. 2003, 213, 189–197. [Google Scholar] [CrossRef]
- Kamibayashi, M.; Ogura, H.; Otsubo, Y. Shear-Thickening Flow of Nanoparticle Suspensions Flocculated by Polymer Bridging. J. Colloid Interface Sci. 2008, 321, 294–301. [Google Scholar] [CrossRef]
- Saito, Y.; Hirose, Y.; Otsubo, Y. Shear-Induced Reversible Gelation of Nanoparticle Suspensions Flocculated by Poly(Ethylene Oxide). Colloids Surf. A Physicochem. Eng. Asp. 2011, 384, 40–46. [Google Scholar] [CrossRef]
- Mar Ramos-Tejada, M.; Luckham, P.F. Shaken but Not Stirred: The Formation of Reversible Particle—Polymer Gels under Shear. Colloids Surf. A Physicochem. Eng. Asp. 2015, 471, 164–169. [Google Scholar] [CrossRef]
- Kawasaki, S.; Kobayashi, M. Affirmation of the Effect of PH on Shake-Gel and Shear Thickening of a Mixed Suspension of Polyethylene Oxide and Silica Nanoparticles. Colloids Surf. A Physicochem. Eng. Asp. 2018, 537, 236–242. [Google Scholar] [CrossRef]
- Collini, H.; Mohr, M.; Luckham, P.; Shan, J.; Russell, A. The Effects of Polymer Concentration, Shear Rate and Temperature on the Gelation Time of Aqueous Silica-Poly(Ethylene-Oxide) “Shake-Gels. ” J. Colloid Interface Sci. 2018, 517, 1–8. [Google Scholar] [CrossRef]
- Huang, Y.; Kobayashi, M. Direct Observation of Relaxation of Aqueous Shake-Gel Consisting of Silica Nanoparticles and Polyethylene Oxide. Polymers 2020, 12, 1141. [Google Scholar] [CrossRef]
- Tian, Q.; Sun, J.; Julian, M.; Huang, X.; Li, N. Quantitative Analysis of the Structural Relaxation of Silica-PEO Shake Gel by X-Ray and Light Scattering. Polym. Test. 2021, 104, 107391. [Google Scholar] [CrossRef]
- Huang, Y.; Sato, S.; Kobayashi, M. Conditions for Shake-Gel Formation: The Relationship between the Size of Poly(Ethylene Oxide) and the Distance between Silica Particles. Molecules 2022, 27, 7770. [Google Scholar] [CrossRef]
- Akada, K.; Okubo, S.; Yamada, T.; Tokuda, K.; Yamaguchi, K.; Uemura, S.; Onoki, T.; Tejima, S.; Kobayashi, M.; Fujita, J.-I. Anisotropic Flocculation in Shear Thickening Colloid-Polymer Suspension via Simultaneous Observation of Rheology and X-Ray Scattering. Colloids Surf. A Physicochem. Eng. Asp. 2023, 658, 130727. [Google Scholar] [CrossRef]
- Yamagata, Y.; Miyamoto, K. Gel Formation and Its Relaxation Mechanism of Shear-Induced Aqueous Suspensions Comprised of Bentonite and Heptaethylene Oleyl Ether. Colloids Surf. A Physicochem. Eng. Asp. 2021, 624, 126786. [Google Scholar] [CrossRef]
- Pozzo, D.C.; Walker, L.M. Reversible Shear Gelation of Polymer-Clay Dispersions. Colloids Surf. A Physicochem. Eng. Asp. 2004, 1–3, 187–198. [Google Scholar] [CrossRef]
- Takeda, M.; Matsunaga, T.; Nishida, T.; Endo, H.; Takahashi, T.; Shibayama, M. Rheo-SANS Studies on Shear Thickening in Clay-Poly(Ethylene Oxide) Mixed Solutions. Macromolecules 2010, 43, 7793–7799. [Google Scholar] [CrossRef]
- Can, V.; Okay, O. Shake Gels Based on Laponite–PEO Mixtures: Effect of Polymer Molecular Weight. Des. Monomers Polym. 2012, 8, 453–462. [Google Scholar] [CrossRef]
- Koga, T.; Li, C. Shear-Induced Network Formation in Colloid/Polymer Mixtures: A Molecular Dynamics Study. Nihon Reoroji Gakkaishi 2014, 42, 123–127. [Google Scholar] [CrossRef]
- Lattuada, M.; Zaccone, A.; Wu, H.; Morbidelli, M. Population-Balance Description of Shear-Induced Clustering, Gelation and Suspension Viscosity in Sheared DLVO Colloids. Soft Matter 2016, 12, 5313–5324. [Google Scholar] [CrossRef]
- Bergna, H.E.; Roberts, W.O. Colloidal Silica: Fundamentals and Applications; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar] [CrossRef]
- Kobayashi, M.; Juillerat, F.; Galletto, P.; Bowen, P.; Borkovec, M. Aggregation and Charging of Colloidal Silica Particles: Effect of Particle Size. Langmuir 2005, 21, 5761–5769. [Google Scholar] [CrossRef]
- Kobayashi, M.; Skarba, M.; Galletto, P.; Cakara, D.; Borkovec, M. Effects of Heat Treatment on the Aggregation and Charging of Stöber-Type Silica. J. Colloid Interface Sci. 2005, 292, 139–147. [Google Scholar] [CrossRef]
- Kawaguchi, S.; Imai, G.; Suzuki, J.; Miyahara, A.; Kitano, T.; Ito, K. Aqueous Solution Properties of Oligo- and Poly(Ethylene Oxide) by Static Light Scattering and Intrinsic Viscosity. Polymer 1997, 38, 2885–2891. [Google Scholar] [CrossRef]
- Rauschkolb, J.C.; Ribeiro, B.C.; Feiden, T.; Fischer, B.; Weschenfelder, T.A.; Cansian, R.L.; Junges, A. Parameter Estimation of Mark-Houwink Equation of Polyethylene Glycol (PEG) Using Molecular Mass and Intrinsic Viscosity in Water. Biointerface Res. Appl. Chem. 2021, 12, 1778–1790. [Google Scholar]
- Sugimoto, T.; Kobayashi, M.; Adachi, Y. The Effect of Double Layer Repulsion on the Rate of Turbulent and Brownian Aggregation: Experimental Consideration. Colloids Surf. A Physicochem. Eng. Asp. 2014, 443, 418–424. [Google Scholar] [CrossRef]
- Sugimoto, T.; Kobayashi, M.; Adachi, Y. Orthokinetic Aggregation of Charged Colloidal Particles in the Presence of Repulsive Double Layer Force: A Trajectory Analysis with the Solution of Non-Linear Poisson–Boltzmann Equation. Colloids Surf. A Physicochem. Eng. Asp. 2015, 483, 321–327. [Google Scholar] [CrossRef]
- Gao, J.; Sugimoto, T.; Kobayashi, M. Effects of Ionic Valence on Aggregation Kinetics of Colloidal Particles with and without a Mixing Flow. J. Colloid Interface Sci. 2023, 638, 733–742. [Google Scholar] [CrossRef]
- Adachi, Y.; Stuart, M.A.C.; Fokkink, R. Kinetics of Turbulent Coagulation Studied by Means of End-over-End Rotation. J. Colloid Interface Sci. 1994, 165, 310–317. [Google Scholar] [CrossRef]
- Dhont, J.K.G.; Briels, W.J. Gradient and Vorticity Banding. Rheol. Acta 2008, 47, 257–281. [Google Scholar] [CrossRef]
- Doi, M. Soft Matter Physics; Oxford University Press: Oxford, UK, 2013; ISBN 9780191774942. [Google Scholar]
- Fielding, S.M.; Olmsted, P.D. Spatiotemporal Oscillations and Rheochaos in a Simple Model of Shear Banding. Phys. Rev. Lett. 2004, 92, 084502. [Google Scholar] [CrossRef]
- Gentile, L.; Silva, B.F.B.; Lages, S.; Mortensen, K.; Kohlbrecher, J.; Olsson, U. Rheochaos and Flow Instability Phenomena in a Nonionic Lamellar Phase. Soft Matter 2012, 9, 1133–1140. [Google Scholar] [CrossRef]
- Fardin, M.A.; Perge, C.; Taberlet, N. “The Hydrogen Atom of Fluid Dynamics”--Introduction to the Taylor-Couette Flow for Soft Matter Scientists. Soft Matter 2014, 10, 3523–3535. [Google Scholar] [CrossRef]


| Mw (kg/mol) | <S2>z1/2 (nm) | C* (10−3 g/cm3) | (cm3/g) |
|---|---|---|---|
| 600 | 45 | 26 | 0.32 |
| 1000 | 61 | 17 | 0.45 |
| 4000 | 136 | 6.3 | 1.12 |
| Cp (mg/m2) | k (-) | a (-) | R2 |
|---|---|---|---|
| 0.03 | 7 × 104 | −2.07 | 0.993 |
| 0.05 | 4 × 105 | −1.74 | 0.938 |
| 0.08 | 3 × 109 | −2.95 | 0.967 |
| 0.15 | 1 × 1027 | −8.88 | 0.995 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sato, S.; Kobayashi, M. The Relationship between Gelation Behavior and the Amount of Polymer Dose per Silica Surface Area of “Shake-Gels” Consisting of Silica Nanoparticles and Poly(Ethylene Oxide). Molecules 2023, 28, 3555. https://doi.org/10.3390/molecules28083555
Sato S, Kobayashi M. The Relationship between Gelation Behavior and the Amount of Polymer Dose per Silica Surface Area of “Shake-Gels” Consisting of Silica Nanoparticles and Poly(Ethylene Oxide). Molecules. 2023; 28(8):3555. https://doi.org/10.3390/molecules28083555
Chicago/Turabian StyleSato, Shunsuke, and Motoyoshi Kobayashi. 2023. "The Relationship between Gelation Behavior and the Amount of Polymer Dose per Silica Surface Area of “Shake-Gels” Consisting of Silica Nanoparticles and Poly(Ethylene Oxide)" Molecules 28, no. 8: 3555. https://doi.org/10.3390/molecules28083555
APA StyleSato, S., & Kobayashi, M. (2023). The Relationship between Gelation Behavior and the Amount of Polymer Dose per Silica Surface Area of “Shake-Gels” Consisting of Silica Nanoparticles and Poly(Ethylene Oxide). Molecules, 28(8), 3555. https://doi.org/10.3390/molecules28083555






