3D Active Brownian Motion of Single Dust Particles Induced by a Laser in a DC Glow Discharge
Abstract
1. Introduction
2. Data Analysis and Discussion
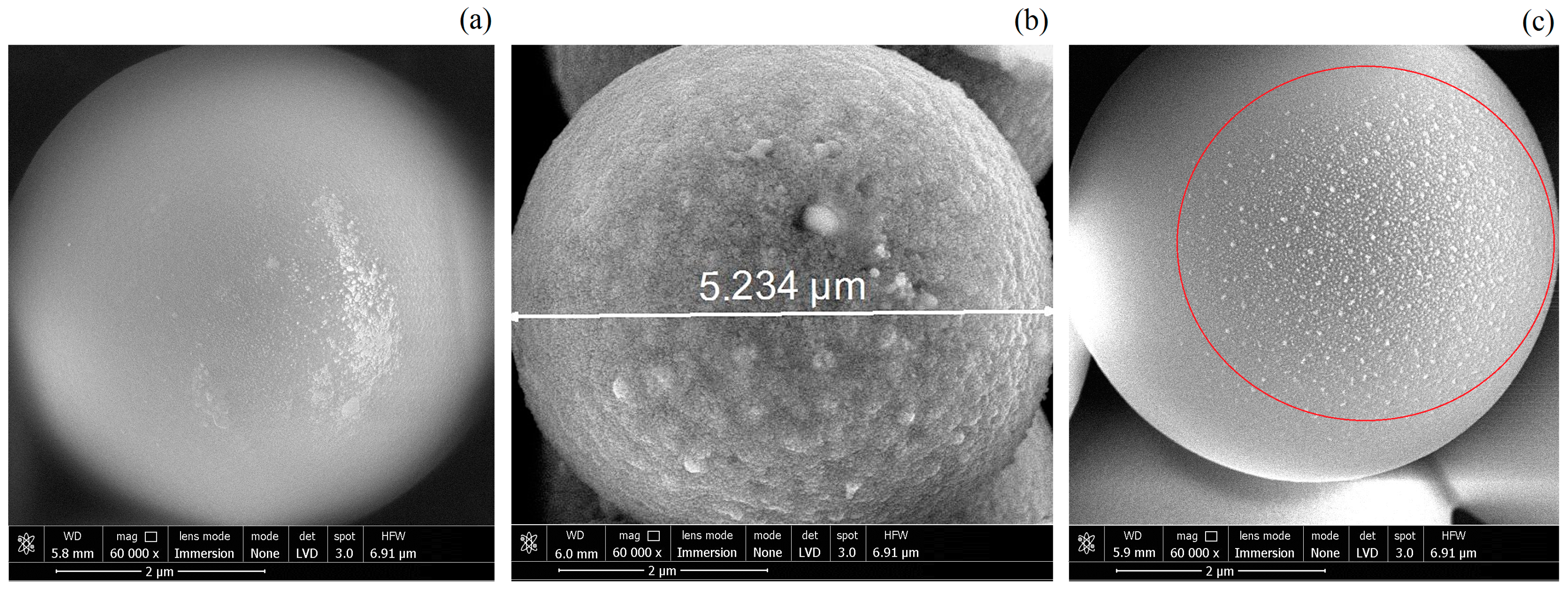
2.1. Materials
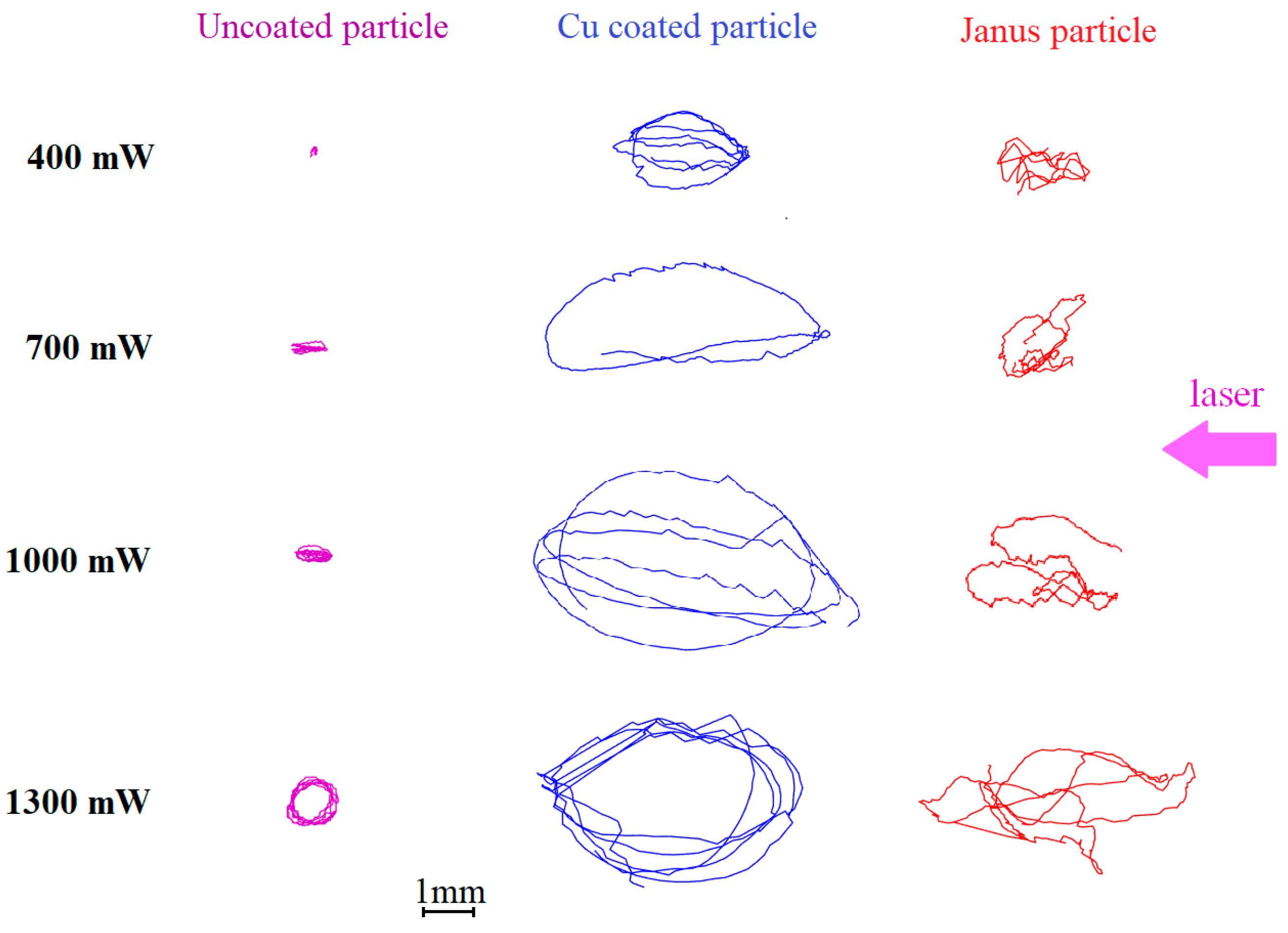
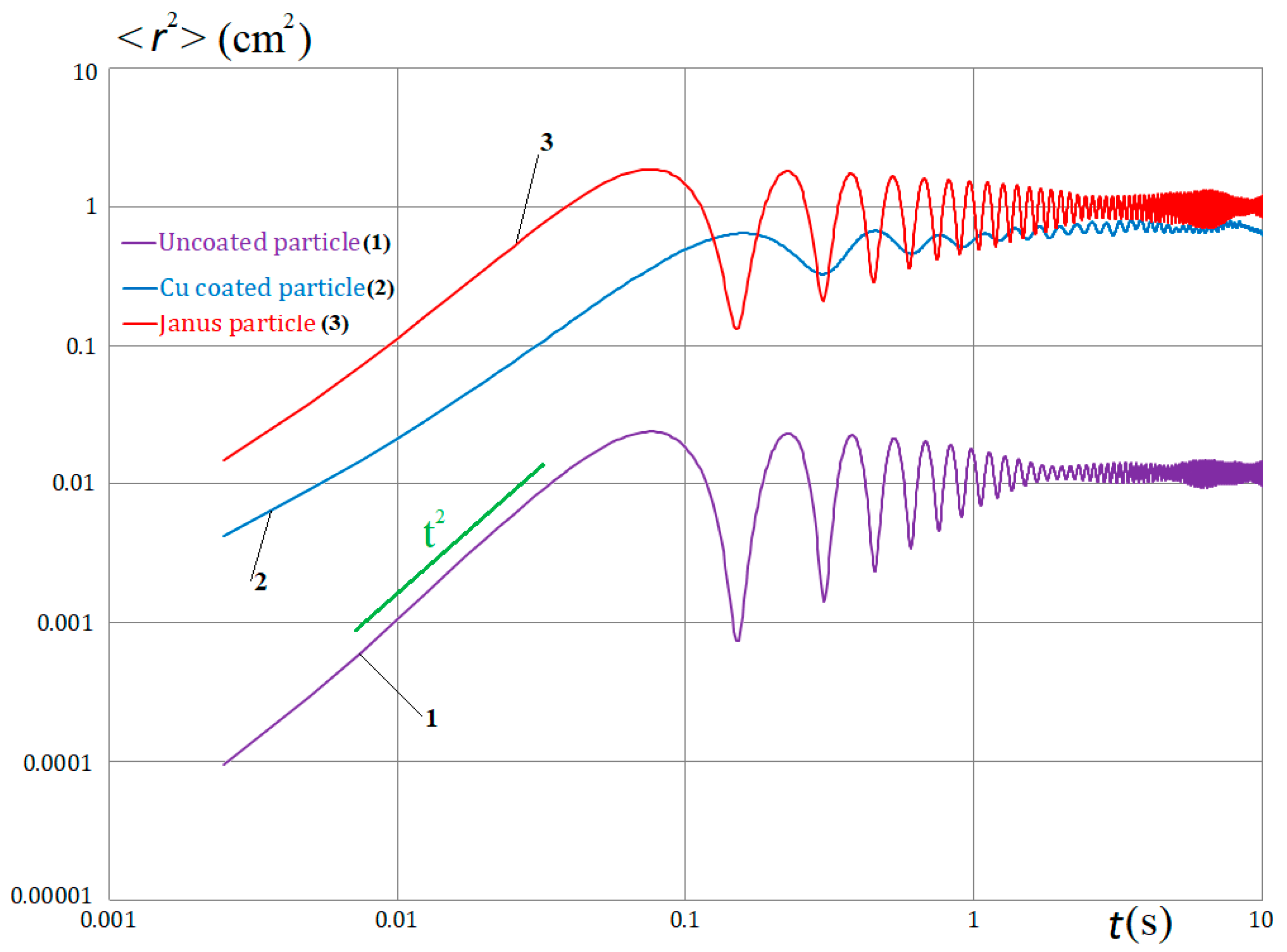
2.2. Analysis of Dust Particle Trajectories
- Photophoresis due to a temperature gradient on the dust particle surface, that is an asymmetric neutral drag force caused by a temperature difference (for all dust particles);
- Photophoresis due to different accommodation coefficients, that is a neutral drag force caused by different accommodation coefficients of MF and copper (for Janus particles).
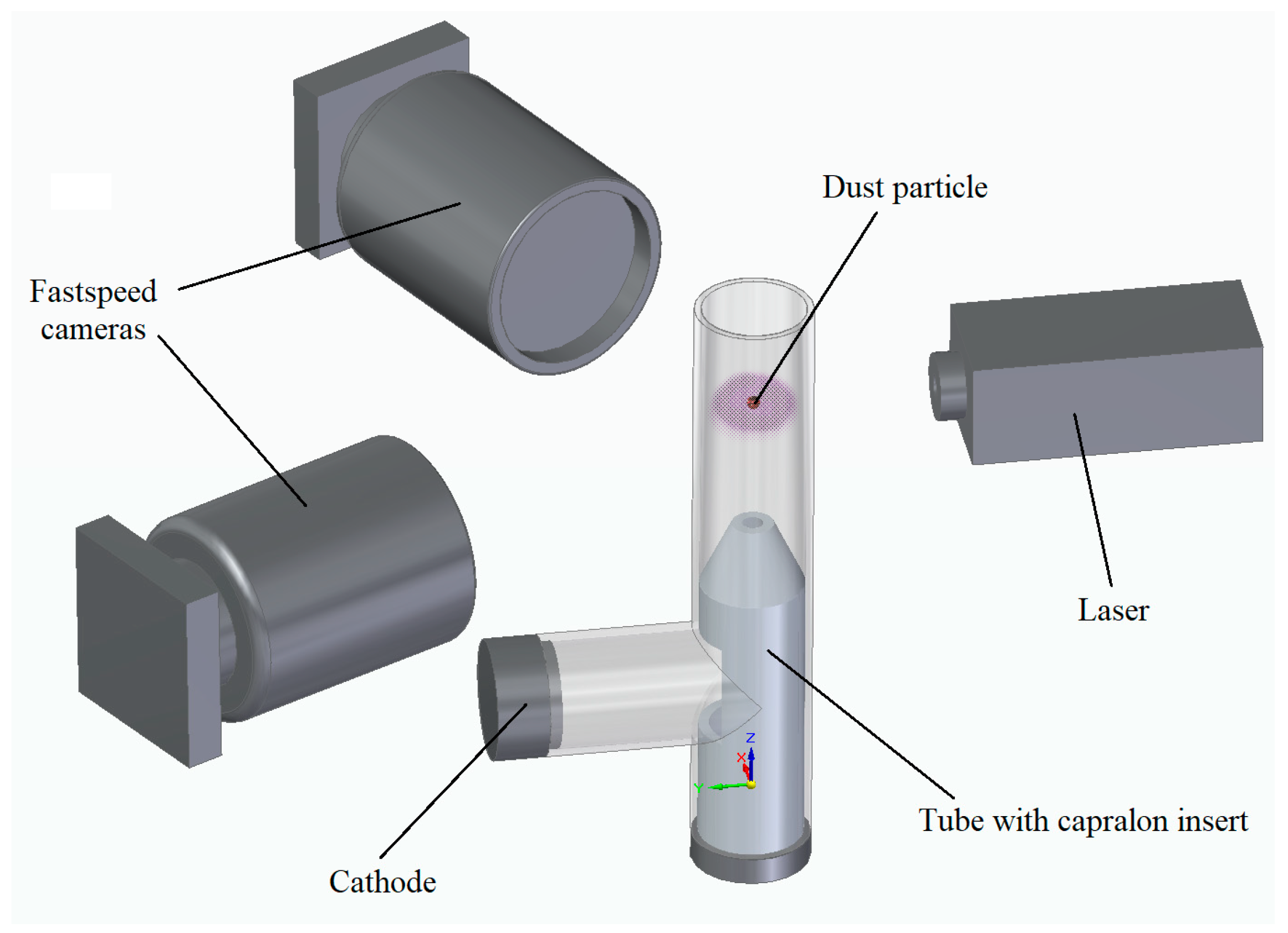
3. Experimental Setup
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Feynman, R. The Brownian Movement. In The Feynman Lectures of Physics, Volume I.; Perseus Book Group: New York, NY, USA, 1964. [Google Scholar]
- Einstein, A. Über die von der molekular kinetischen Theorie der Wärmege forderte Bewegung von in ruhenden Flüssigkeitensuspendierten Teilchen. Annalen der Physik 1905, 322, 549. [Google Scholar] [CrossRef]
- Aranson, I.S. Active colloids. Phys. Usp. 2013, 56, 79–92. [Google Scholar] [CrossRef]
- Bechinger, C.; DiLeonardo, R.; Löwen, H.; Reichhardt, C.; Volpe, G. Active particles in complex and crowded environments. Rev. Mod. Phys. 2016, 88, 045006. [Google Scholar] [CrossRef]
- Selmeczi, D.; Li, L.; Pedersen, L.I.; Nrrelykke, S.F.; Hagedorn, P.H.; Mosler, S.; Larsen, N.B.; Cox, E.C.; Flyvbjerg, H. Cell motility as random motion: A review. Eur. Phys. J. Spec. Top. 2008, 157, 1–15. [Google Scholar] [CrossRef]
- Berg, H.C. E. coli in Motion; Springer: New York, NY, USA, 2008; p. 134. [Google Scholar]
- Cates, M.E. Diffusive transport without detailed balance in motile bacteria: Does microbiology need statistical physics. Rep. Prog. Phys. 2012, 75, 042601. [Google Scholar] [CrossRef]
- Sanchez, S.; Ananth, A.N.; Fomin, V.M. Superfast motion of catalytic microjet engines at physiological temperature. J. Am. Chem. Soc. 2011, 133, 14860–14863. [Google Scholar] [CrossRef]
- Paxton, W.F.; Kistler, K.C.; Olmeda, C.C. Catalytic nanomotors: Autonomous movement of striped nanorods. J. Am. Chem. Soc. 2004, 126, 13424–13431. [Google Scholar] [CrossRef]
- Howse, J.R.; Jones, R.A.; Ryan, A.J.; Gough, T.; Vafabakhsh, R.; Golestanian, R. Self-Motile colloidal particles: From directed propulsion to randomwalk. Phys. Rev. Lett. 2007, 99, 048102. [Google Scholar] [CrossRef]
- Li, J.; Singh, V.V.; Sattayasamitsathit, S. Water-driven micromotors for rapid photocatalytic degradation of biological and chemical warfare agents. ACS Nano 2014, 8, 11118–11125. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Sudakov, V.; Gubernov, V.; Kolobov, A.; Korshunova, E.; Kiverin, A. Oscillating motion of oil droplets in the emulsion near the air–water interface. J. Phys. Chem. B 2021, 125, 10373. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Sudakov, V.; Petrov, O.; Gubernov, V.; Korshunova, E.; Kolobov, A.; Kiverin, A. Magnetic nanomotors in emulsions for locomotion of microdroplets. ACS Appl. Mater. Interfaces 2022, 14, 10976. [Google Scholar]
- Nosenko, V.; Luoni, F.; Kaouk, A.; Rubin-Zuzic, M.; Thomas, H. Active Janus particles in a complex plasma. Phys. Rev. Res. 2020, 2, 033226. [Google Scholar] [CrossRef]
- Arkar, K.; Vasiliev, M.M.; Petrov, O.F.; Kononov, E.A.; Trukhachev, F.M. Dynamics of active Brownian particles in plasma. Molecules 2021, 26, 561. [Google Scholar] [CrossRef] [PubMed]
- Fairushin, I.I.; Vasiliev, M.M.; Petrov, O.F. Effect of laser radiation on the dynamics of active Brownian macroparticles in an extended plasma-dust monolayer. Molecules 2021, 26, 6974. [Google Scholar] [CrossRef] [PubMed]
- Koss, X.G.; Kononov, E.A.; Lisina, I.I.; Vasiliev, M.M.; Petrov, O.F. Dynamic entropy of two-dimensional active Brownian systems in colloidal plasmas. Molecules 2022, 27, 1614. [Google Scholar] [CrossRef] [PubMed]
- Petrov, O.F.; Statsenko, K.B.; Vasiliev, M.M. Active Brownian motion of strongly coupled charged grains driven by laser radiation in plasma. Sci. Rep. 2022, 12, 8618. [Google Scholar] [CrossRef]
- Ismagilov, R.F.; Schwartz, A.; Bowden, N. Autonomous movement and self-assembly. Angew. Chem. Int. Ed. Engl. 2002, 41, 652–654. [Google Scholar] [CrossRef]
- Perro, A.; Reculusa, S.; Ravaine, S.; Bourgeat-Lamic, E.; Duguet, E. Design and synthesis of Janus micro- and nanoparticles. J. Mater. Chem. 2005, 15, 3745–3760. [Google Scholar] [CrossRef]
- Kononov, E.A.; Vasiliev, M.M.; Vasilieva, E.V.; Petrov, O.F. Particle surface modification in the near-electrode region of an RF discharge. Nanomaterials 2021, 11, 2931. [Google Scholar]
- Shukla, P.K.; Mamun, A.A. Introduction to dusty plasma physics. Plasma Phys. Control Fusion 2002, 44, 395. [Google Scholar] [CrossRef]
- Allen, J.E. Probe theory—The orbital motion approach. Phys. Scr. 1992, 45, 497–503. [Google Scholar] [CrossRef]
- Eymeren, J.; Wurm, G. The implications of particle rotation on the effect of photophoresis. Mon. Not. R. Astron. Soc. 2012, 420, 183–186. [Google Scholar] [CrossRef]
- Vasiliev, M.M.; Petrov, O.F.; Alekseevskaya, A.A.; Ivanov, A.S.; Vasilieva, E.V. Dynamic effects of laser action on quasi-two-dimensional dusty plasma systems of charged particles. Molecules 2020, 25, 3375. [Google Scholar] [CrossRef] [PubMed]
- Jahanshahi, S.; Lowen, H.; Hagen, B. Brownian motion of a circle swimmer in a harmonic trap. Phys. Rev. E 2017, 95, 022606. [Google Scholar]
- Qian, H.; Sheetz, M.P.; Elson, E.L. Single particle tracking. Analysis of diffusion and flow in two-dimensional systems. Biophys. J. 1991, 60, 910–921. [Google Scholar] [CrossRef] [PubMed]
- Löwen, H. Twenty years of confined colloids: From confinement-induced freezing to giant breathing. J. Phys. Condens. Matter 2009, 21, 474203. [Google Scholar] [CrossRef]
- Golubovskii, Y.B.; Nisimov, S.U. 2-Dimensional character of strates in low-pressure discharges in inert-gases. 2. J. Tech. Phys. 1995, 65, 46–54. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Svetlov, A.S.; Vasiliev, M.M.; Kononov, E.A.; Petrov, O.F.; Trukhachev, F.M. 3D Active Brownian Motion of Single Dust Particles Induced by a Laser in a DC Glow Discharge. Molecules 2023, 28, 1790. https://doi.org/10.3390/molecules28041790
Svetlov AS, Vasiliev MM, Kononov EA, Petrov OF, Trukhachev FM. 3D Active Brownian Motion of Single Dust Particles Induced by a Laser in a DC Glow Discharge. Molecules. 2023; 28(4):1790. https://doi.org/10.3390/molecules28041790
Chicago/Turabian StyleSvetlov, Anton S., Mikhail M. Vasiliev, Evgeniy A. Kononov, Oleg F. Petrov, and Fedor M. Trukhachev. 2023. "3D Active Brownian Motion of Single Dust Particles Induced by a Laser in a DC Glow Discharge" Molecules 28, no. 4: 1790. https://doi.org/10.3390/molecules28041790
APA StyleSvetlov, A. S., Vasiliev, M. M., Kononov, E. A., Petrov, O. F., & Trukhachev, F. M. (2023). 3D Active Brownian Motion of Single Dust Particles Induced by a Laser in a DC Glow Discharge. Molecules, 28(4), 1790. https://doi.org/10.3390/molecules28041790



.jpg)


