Interlayer Chemical Modulation of Phase Transitions in Two-Dimensional Metal Chalcogenides
Abstract
1. Introduction
2. Interlayer Chemical Modulation of Electronic Phase Transitions
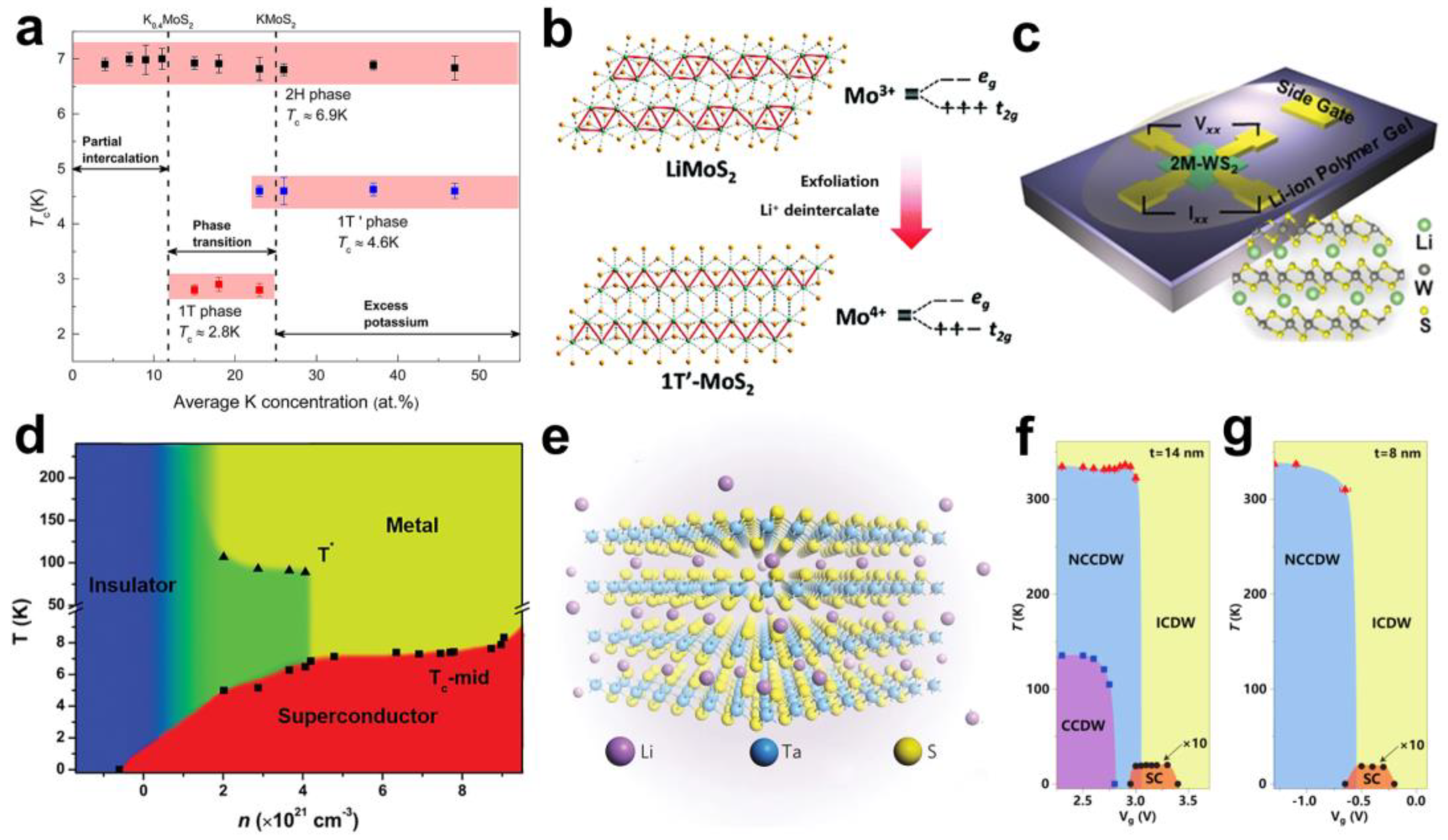
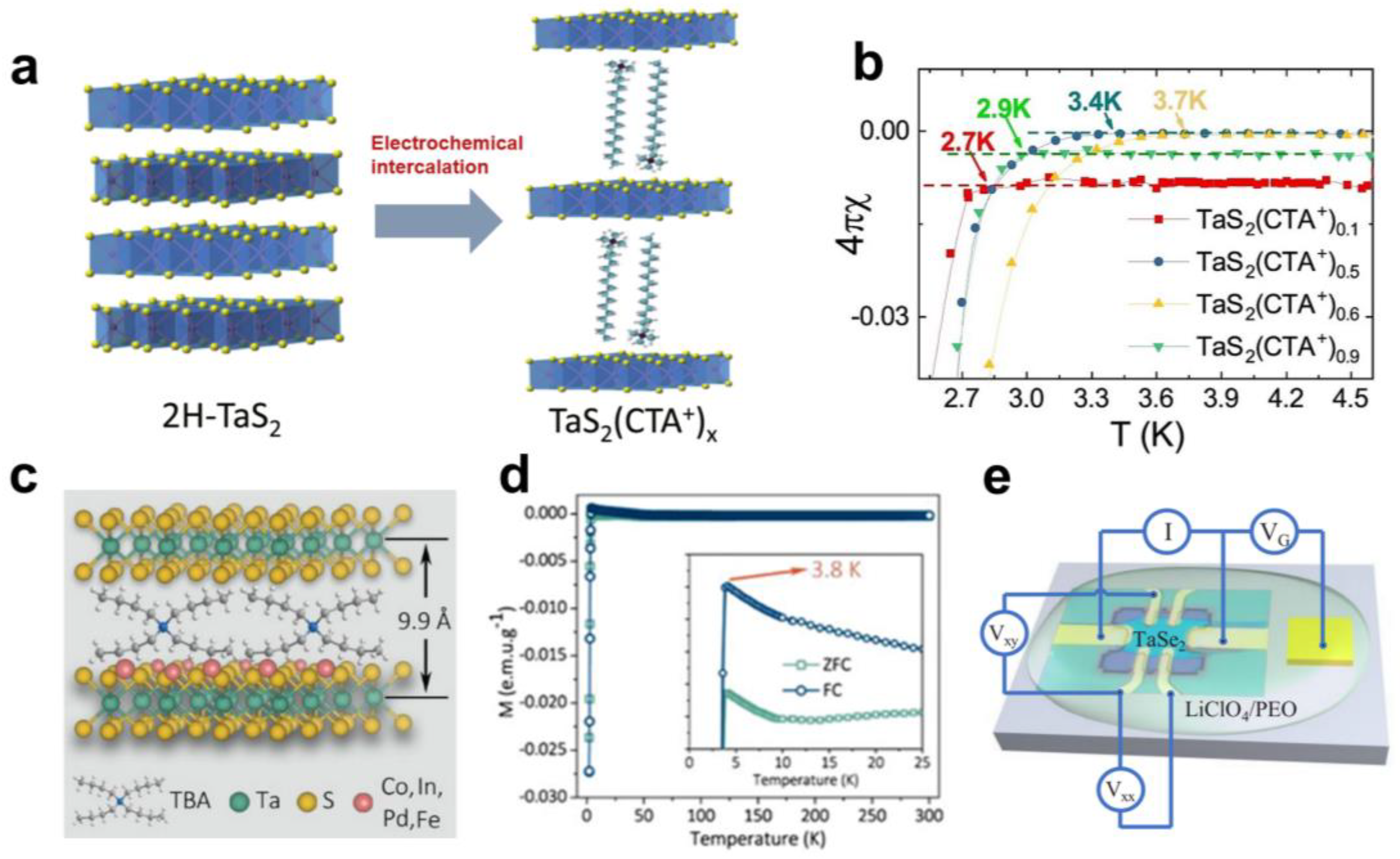
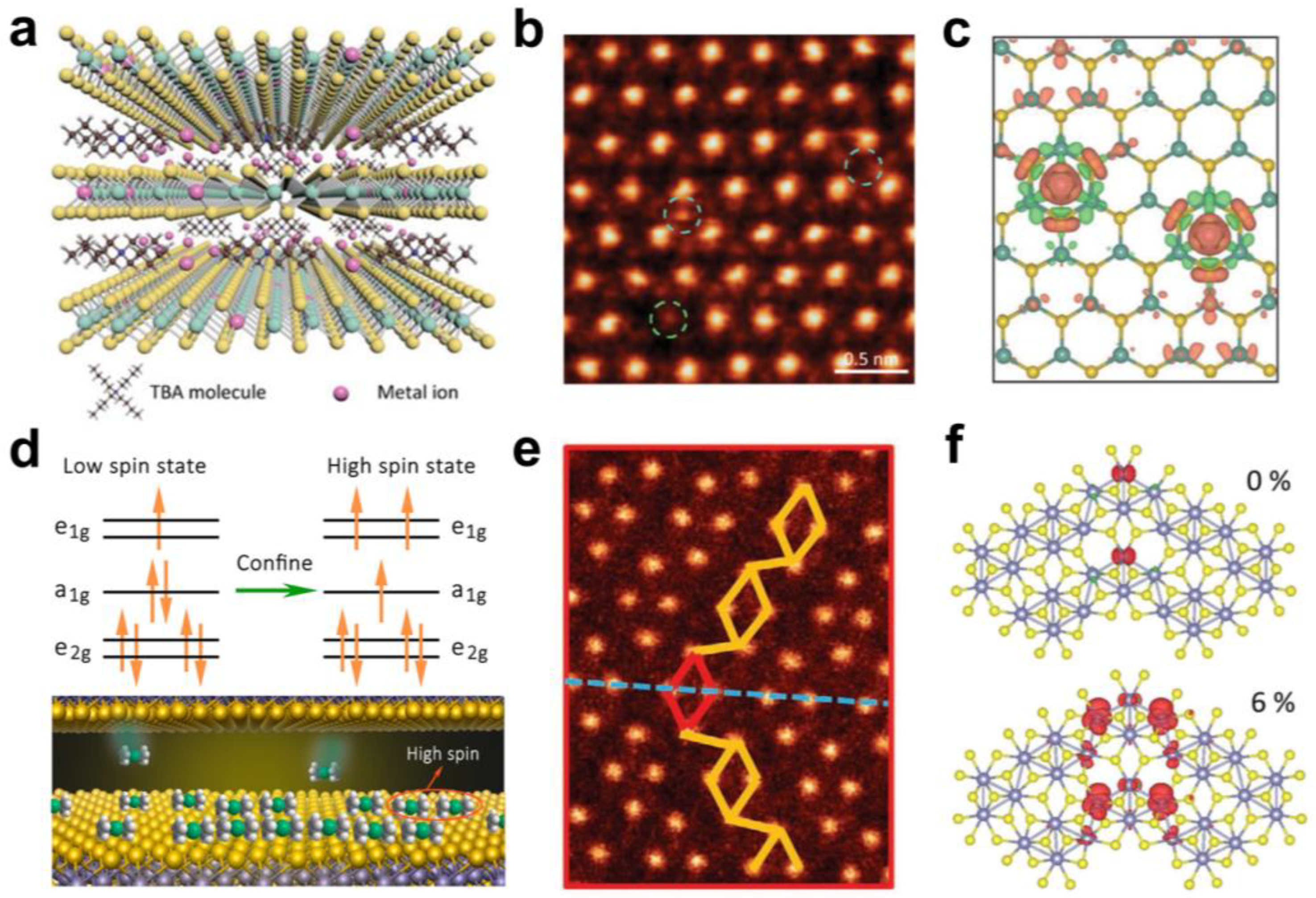
2.1. Superconducting Transition
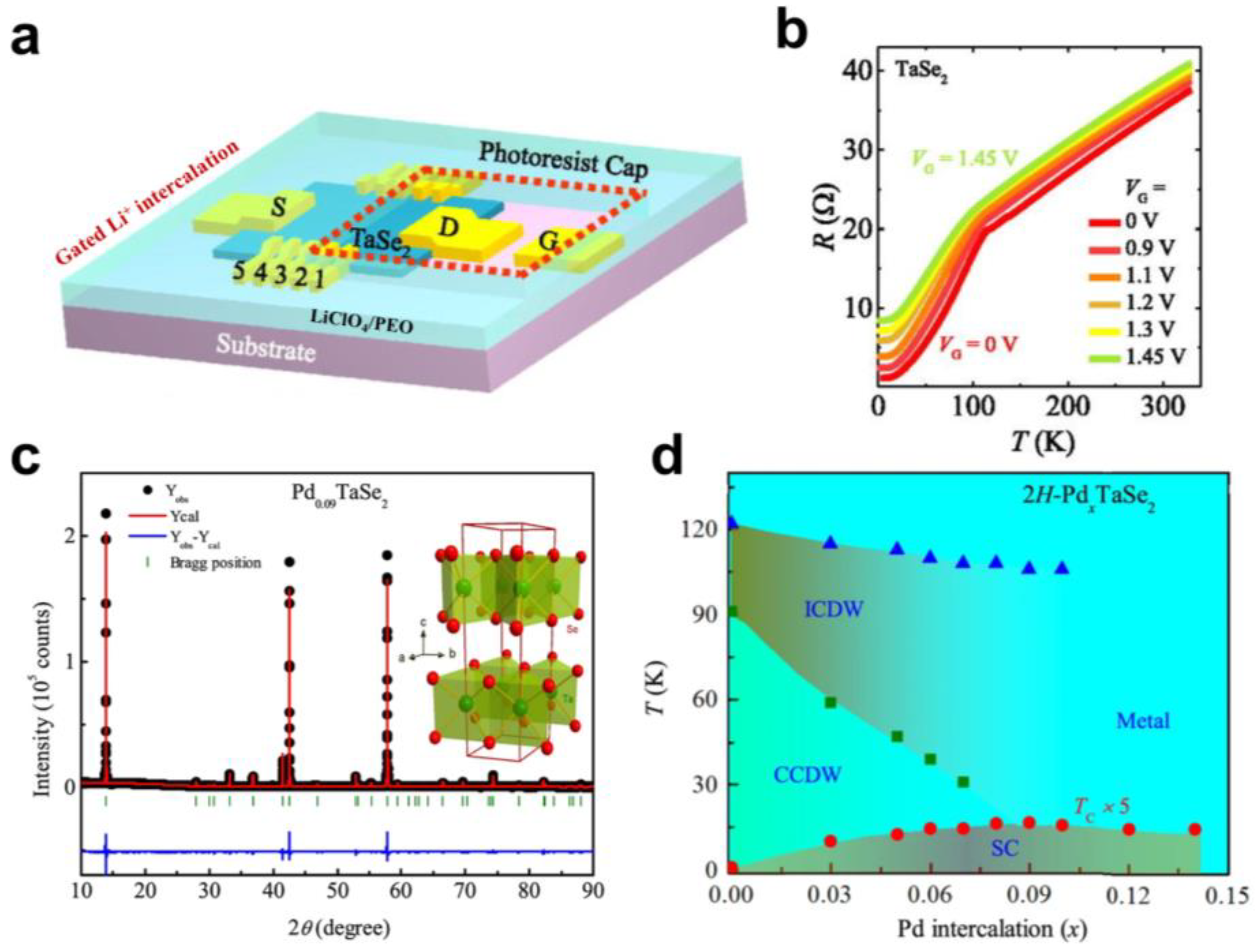
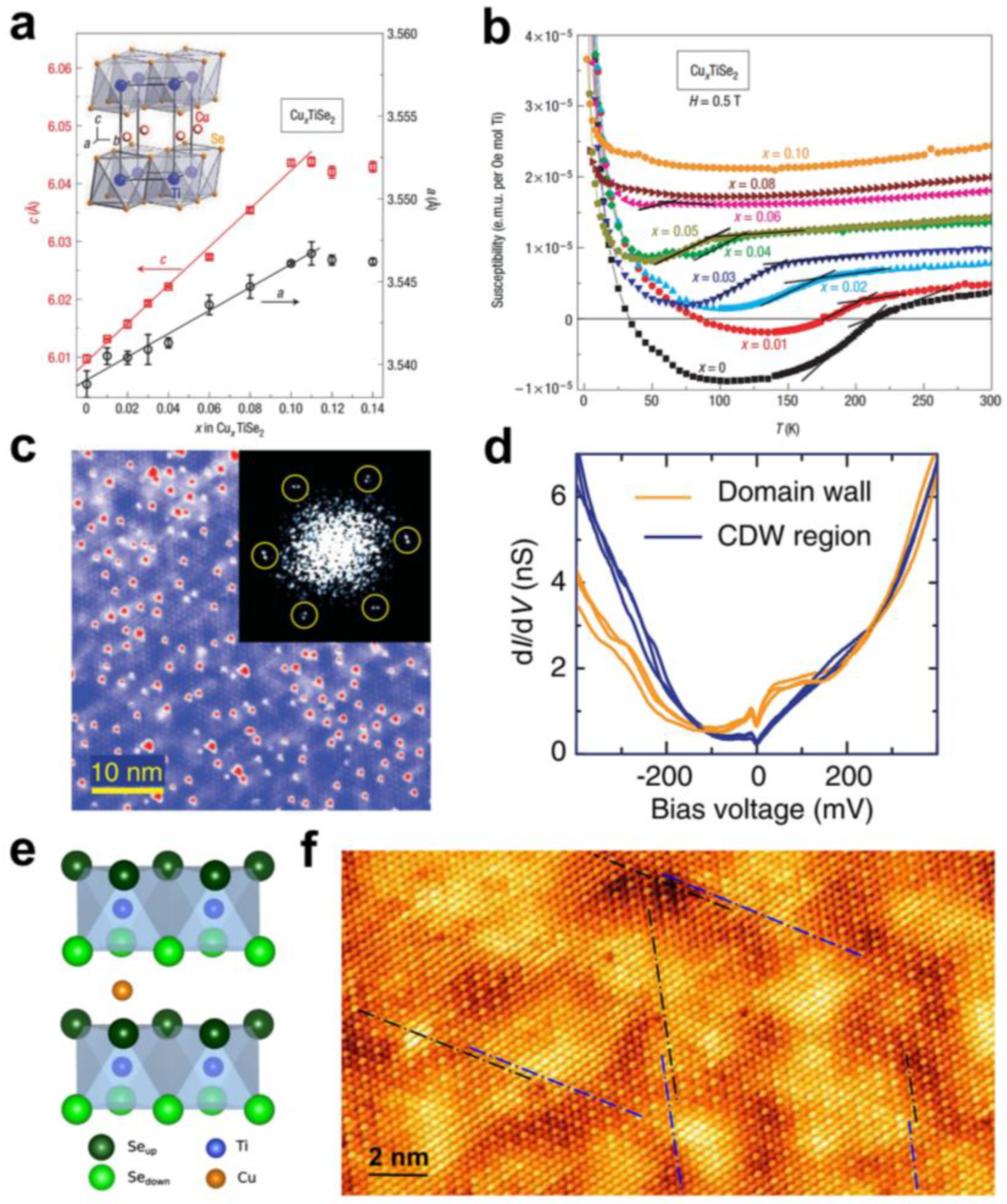
2.2. Charge Density Wave Transition
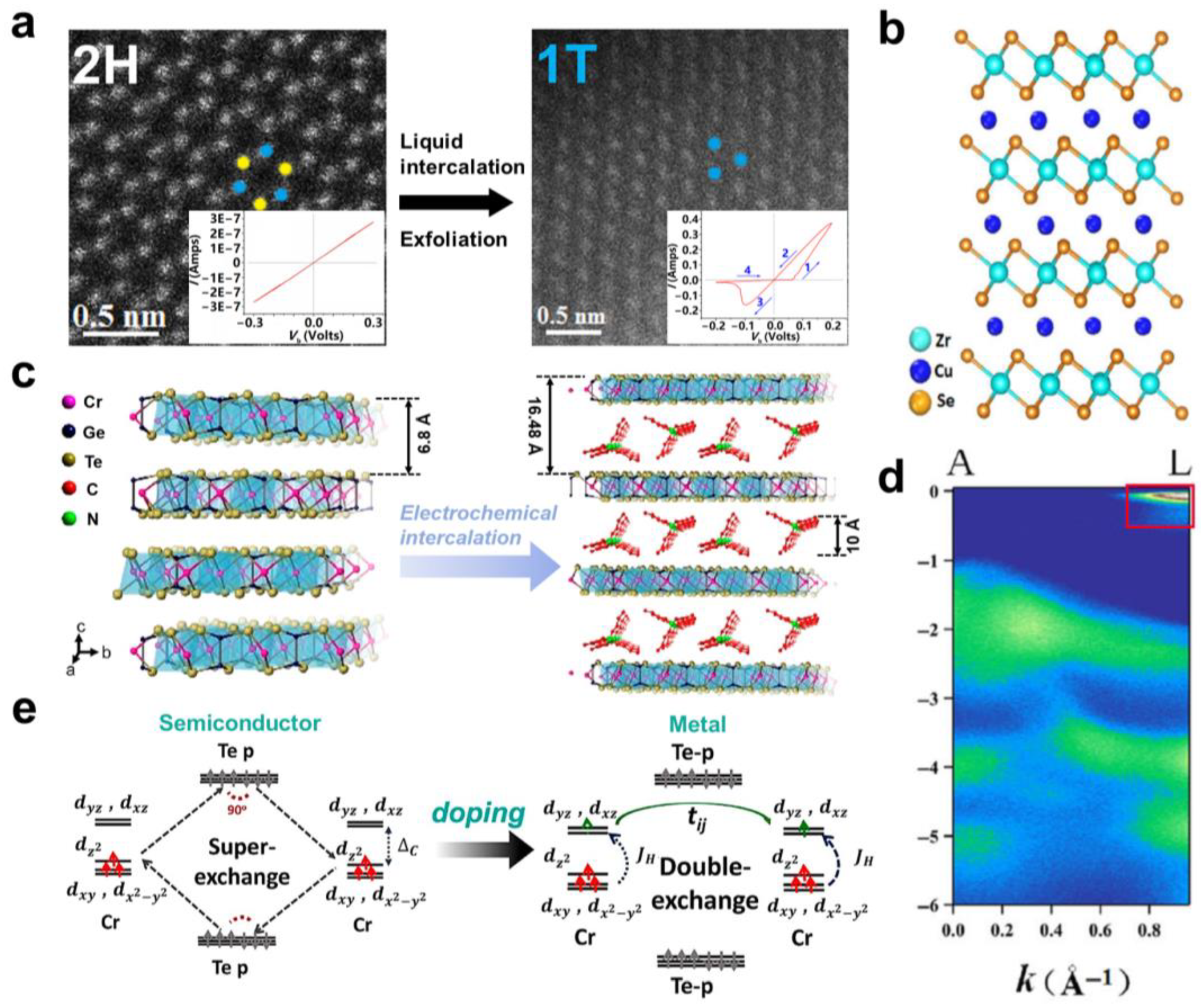
2.3. Semiconductor-to-Metal Transition
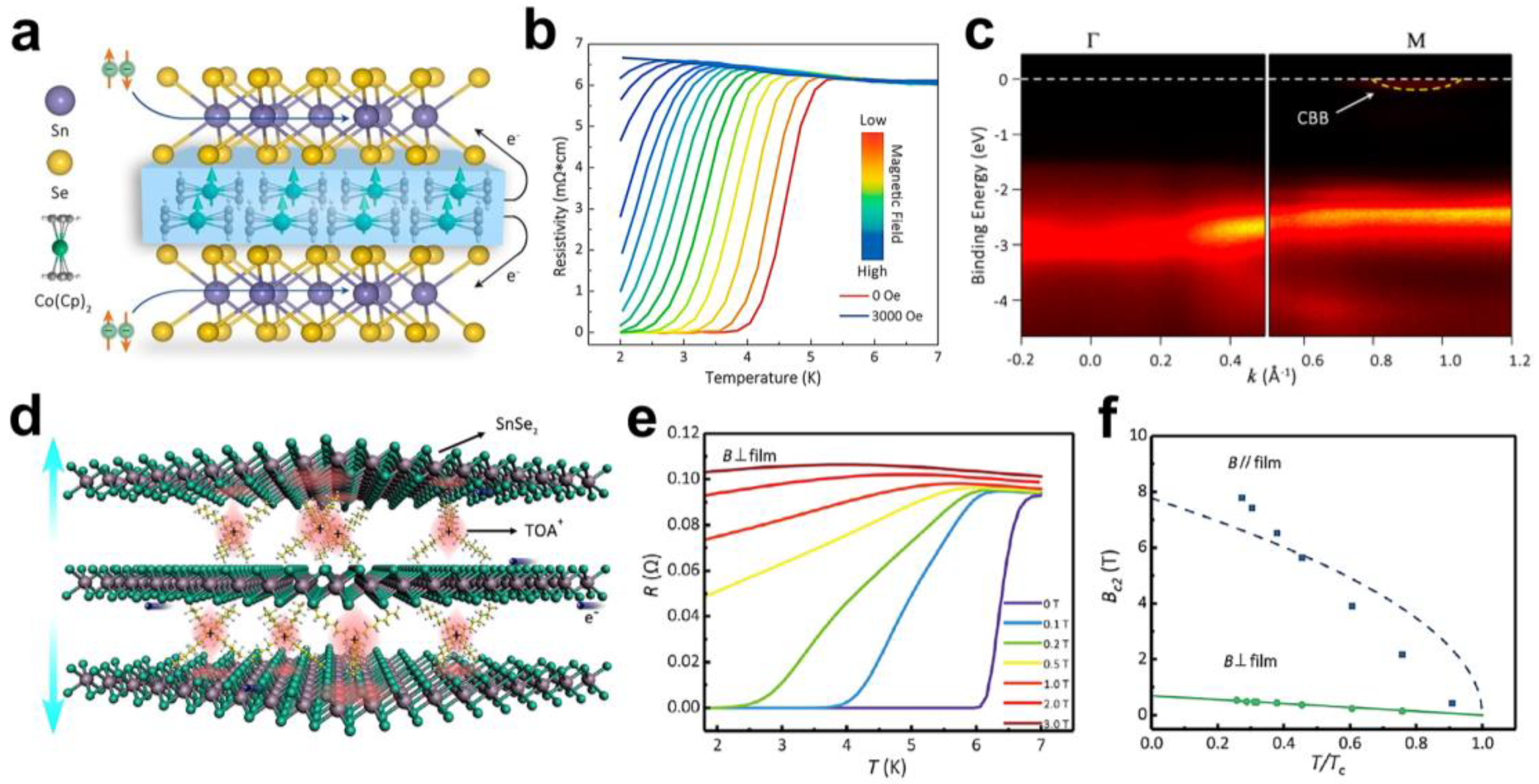
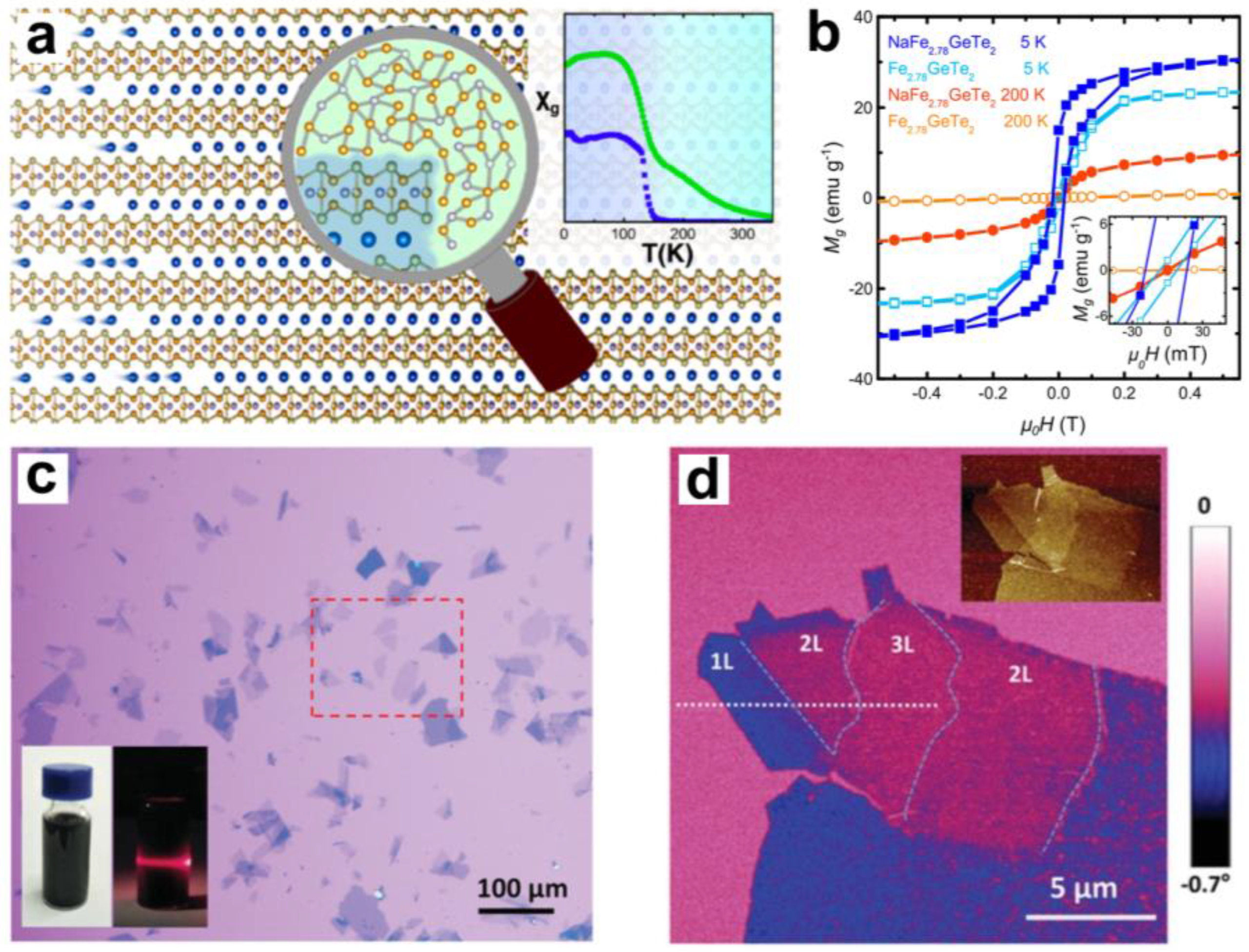
3. Interlayer Chemical Modulation of Magnetic Phase Transitions
4. Interlayer Chemical Modulation of Lattice Phase Transitions

5. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Butler, S.Z.; Hollen, S.M.; Cao, L.; Cui, Y.; Gupta, J.A.; Gutiérrez, H.R.; Heinz, T.F.; Hong, S.S.; Huang, J.; Ismach, A.F.; et al. Progress, Challenges, and Opportunities in Two-Dimensional Materials Beyond Graphene. ACS Nano 2013, 7, 2898–2926. [Google Scholar] [CrossRef] [PubMed]
- Mounet, N.; Gibertini, M.; Schwaller, P.; Campi, D.; Merkys, A.; Marrazzo, A.; Sohier, T.; Castelli, I.E.; Cepellotti, A.; Pizzi, G.; et al. Two-dimensional materials from high-throughput computational exfoliation of experimentally known compounds. Nat. Nanotechnol. 2018, 13, 246–252. [Google Scholar] [CrossRef] [PubMed]
- Tan, C.; Cao, X.; Wu, X.-J.; He, Q.; Yang, J.; Zhang, X.; Chen, J.; Zhao, W.; Han, S.; Nam, G.-H.; et al. Recent Advances in Ultrathin Two-Dimensional Nanomaterials. Chem. Rev. 2017, 117, 6225–6331. [Google Scholar] [CrossRef] [PubMed]
- Chhowalla, M.; Jena, D.; Zhang, H. Two-dimensional semiconductors for transistors. Nat. Rev. Mater. 2016, 1, 16052. [Google Scholar] [CrossRef]
- Tong, L.; Wan, J.; Xiao, K.; Liu, J.; Ma, J.; Guo, X.; Zhou, L.; Chen, X.; Xia, Y.; Dai, S.; et al. Heterogeneous complementary field-effect transistors based on silicon and molybdenum disulfide. Nat. Electron. 2022. [Google Scholar] [CrossRef]
- Shen, Y.; Dong, Z.; Sun, Y.; Guo, H.; Wu, F.; Li, X.; Tang, J.; Liu, J.; Wu, X.; Tian, H.; et al. The Trend of 2D Transistors toward Integrated Circuits: Scaling Down and New Mechanisms. Adv. Mater. 2022, 34, 2201916. [Google Scholar] [CrossRef]
- Yang, L.; Majumdar, K.; Liu, H.; Du, Y.; Wu, H.; Hatzistergos, M.; Hung, P.Y.; Tieckelmann, R.; Tsai, W.; Hobbs, C.; et al. Chloride Molecular Doping Technique on 2D Materials: WS2 and MoS2. Nano Lett. 2014, 14, 6275–6280. [Google Scholar] [CrossRef]
- Moody, M.J.; Henning, A.; Jurca, T.; Shang, J.Y.; Bergeron, H.; Balla, I.; Olding, J.N.; Weiss, E.A.; Hersam, M.C.; Lohr, T.L.; et al. Atomic Layer Deposition of Molybdenum Oxides with Tunable Stoichiometry Enables Controllable Doping of MoS2. Chem. Mater. 2018, 30, 3628–3632. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Z.; Xi, S.; Zhao, X.; Sun, T.; Li, J.; Yu, W.; Xu, H.; Herng, T.S.; Hai, X.; et al. Tuning the Spin Density of Cobalt Single-Atom Catalysts for Efficient Oxygen Evolution. ACS Nano 2021, 15, 7105–7113. [Google Scholar] [CrossRef]
- Zhou, H.; Hu, X.; Fang, W.-H.; Su, N.Q. Revealing intrinsic spin coupling in transition metal-doped graphene. Phys. Chem. Chem. Phys. 2022, 24, 16300–16309. [Google Scholar] [CrossRef]
- Bolar, S.; Shit, S.; Murmu, N.C.; Samanta, P.; Kuila, T. Activation Strategy of MoS2 as HER Electrocatalyst through Doping-Induced Lattice Strain, Band Gap Engineering, and Active Crystal Plane Design. ACS Appl. Mater. Interfaces 2021, 13, 765–780. [Google Scholar] [CrossRef] [PubMed]
- Qin, Y.; Yu, T.; Deng, S.; Zhou, X.-Y.; Lin, D.; Zhang, Q.; Jin, Z.; Zhang, D.; He, Y.-B.; Qiu, H.-J.; et al. RuO2 electronic structure and lattice strain dual engineering for enhanced acidic oxygen evolution reaction performance. Nat. Commun. 2022, 13, 3784. [Google Scholar] [CrossRef] [PubMed]
- Wan, J.; Lacey, S.D.; Dai, J.; Bao, W.; Fuhrer, M.S.; Hu, L. Tuning two-dimensional nanomaterials by intercalation: Materials, properties and applications. Chem. Soc. Rev. 2016, 45, 6742–6765. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Li, D.; Wu, C.-L.; Hwang, H.Y.; Cui, Y. Electrostatic gating and intercalation in 2D materials. Nat. Rev. Mater. 2023, 8, 41–53. [Google Scholar] [CrossRef]
- Zeng, J.; Liu, E.; Fu, Y.; Chen, Z.; Pan, C.; Wang, C.; Wang, M.; Wang, Y.; Xu, K.; Cai, S.; et al. Gate-Induced Interfacial Superconductivity in 1T-SnSe2. Nano Lett. 2018, 18, 1410–1415. [Google Scholar] [CrossRef]
- Marini, G.; Calandra, M. Light-Tunable Charge Density Wave Orders in MoTe2 and WTe2 Single Layers. Phys. Rev. Lett. 2021, 127, 257401. [Google Scholar] [CrossRef]
- Ahn, S.; Das Sarma, S. Disorder-induced two-dimensional metal-insulator transition in moiré transition metal dichalcogenide multilayers. Phys. Rev. B 2022, 105, 115114. [Google Scholar] [CrossRef]
- Xia, B.; Gao, D.; Xue, D. Ferromagnetism of two-dimensional transition metal chalcogenides: Both theoretical and experimental investigations. Nanoscale 2021, 13, 12772–12787. [Google Scholar] [CrossRef]
- Chen, C.-W.; Choe, J.; Morosan, E. Charge density waves in strongly correlated electron systems. Rep. Prog. Phys. 2016, 79, 84505. [Google Scholar] [CrossRef]
- Zhao, B.; Shen, D.; Zhang, Z.; Lu, P.; Hossain, M.; Li, J.; Li, B.; Duan, X. 2D Metallic Transition-Metal Dichalcogenides: Structures, Synthesis, Properties, and Applications. Adv. Funct. Mater. 2021, 31, 2105132. [Google Scholar] [CrossRef]
- Morosan, E.; Natelson, D.; Nevidomskyy, A.H.; Si, Q. Strongly Correlated Materials. Adv. Mater. 2012, 24, 4896–4923. [Google Scholar] [CrossRef] [PubMed]
- Sun, L.; Yan, X.; Zheng, J.; Yu, H.; Lu, Z.; Gao, S.-p.; Liu, L.; Pan, X.; Wang, D.; Wang, Z.; et al. Layer-Dependent Chemically Induced Phase Transition of Two-Dimensional MoS2. Nano Lett. 2018, 18, 3435–3440. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Qian, X.; Li, J. Phase transitions in 2D materials. Nat. Rev. Mater. 2021, 6, 829–846. [Google Scholar] [CrossRef]
- Klemm, R.A. Pristine and intercalated transition metal dichalcogenide superconductors. Phys. C Supercond. Its Appl. 2015, 514, 86–94. [Google Scholar] [CrossRef]
- Wang, N.Z.; Shi, M.Z.; Shang, C.; Meng, F.B.; Ma, L.K.; Luo, X.G.; Chen, X.H. Tunable superconductivity by electrochemical intercalation in TaS2. New J. Phys. 2018, 20, 23014. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, X.; Zhao, X.; Li, J.; Herng, T.S.; Xu, H.; Lin, F.; Lyu, P.; Peng, X.; Yu, W.; et al. Imprinting Ferromagnetism and Superconductivity in Single Atomic Layers of Molecular Superlattices. Adv. Mater. 2020, 32, 1907645. [Google Scholar] [CrossRef]
- Peng, J.; Yu, Z.; Wu, J.; Zhou, Y.; Guo, Y.; Li, Z.; Zhao, J.; Wu, C.; Xie, Y. Disorder Enhanced Superconductivity toward TaS2 Monolayer. ACS Nano 2018, 12, 9461–9466. [Google Scholar] [CrossRef]
- Wu, Y.; He, J.; Liu, J.; Xing, H.; Mao, Z.; Liu, Y. Dimensional reduction and ionic gating induced enhancement of superconductivity in atomically thin crystals of 2H-TaSe2. Nanotechnology 2019, 30, 35702. [Google Scholar] [CrossRef]
- Wu, Y.; Xing, H.; Lian, C.-S.; Lian, H.; He, J.; Duan, W.; Liu, J.; Mao, Z.; Liu, Y. Ion intercalation engineering of electronic properties of two-dimensional crystals of 2H-TaSe2. Phys. Rev. Mater. 2019, 3, 104003. [Google Scholar] [CrossRef]
- Li, Z.; Zhao, Y.; Mu, K.; Shan, H.; Guo, Y.; Wu, J.; Su, Y.; Wu, Q.; Sun, Z.; Zhao, A.; et al. Molecule-Confined Engineering toward Superconductivity and Ferromagnetism in Two-Dimensional Superlattice. J. Am. Chem. Soc. 2017, 139, 16398–16404. [Google Scholar] [CrossRef]
- Zhao, Y.; Su, Y.; Guo, Y.; Peng, J.; Zhao, J.; Wang, C.; Wang, L.; Wu, C.; Xie, Y. Quantum Griffiths Singularity in a Layered Superconducting Organic–Inorganic Hybrid Superlattice. ACS Mater. Lett. 2021, 3, 210–216. [Google Scholar] [CrossRef]
- Zhang, R.; Tsai, I.L.; Chapman, J.; Khestanova, E.; Waters, J.; Grigorieva, I.V. Superconductivity in Potassium-Doped Metallic Polymorphs of MoS2. Nano Lett. 2016, 16, 629–636. [Google Scholar] [CrossRef] [PubMed]
- Somoano, R.B.; Hadek, V.; Rembaum, A. Alkali metal intercalates of molybdenum disulfide. J. Chem. Phys. 1973, 58, 697–701. [Google Scholar] [CrossRef]
- Guo, C.; Pan, J.; Li, H.; Lin, T.; Liu, P.; Song, C.; Wang, D.; Mu, G.; Lai, X.; Zhang, H.; et al. Observation of superconductivity in 1T’-MoS2 nanosheets. J. Mater. Chem. C 2017, 5, 10855–10860. [Google Scholar] [CrossRef]
- Fang, Y.; Pan, J.; He, J.; Luo, R.; Wang, D.; Che, X.; Bu, K.; Zhao, W.; Liu, P.; Mu, G.; et al. Structure Re-determination and Superconductivity Observation of Bulk 1T MoS2. Angew. Chem. Int. Ed. 2018, 57, 1232–1235. [Google Scholar] [CrossRef]
- Fang, Y.; Pan, J.; Zhang, D.; Wang, D.; Hirose, H.T.; Terashima, T.; Uji, S.; Yuan, Y.; Li, W.; Tian, Z.; et al. Discovery of Superconductivity in 2M WS2 with Possible Topological Surface States. Adv. Mater. 2019, 31, 1901942. [Google Scholar] [CrossRef]
- Che, X.; Deng, Y.; Fang, Y.; Pan, J.; Yu, Y.; Huang, F. Gate-Tunable Electrical Transport in Thin 2M-WS2 Flakes. Adv. Electron. Mater. 2019, 5, 1900462. [Google Scholar] [CrossRef]
- Mollah, S.; Biswas, B.; Haldar, S.; Ghosh, A.K. Carrier concentration induced transformations and existence of pseudogap in NdBa2Cu3O7-δ. Phys. C Supercond. Its Appl. 2017, 539, 40–43. [Google Scholar] [CrossRef]
- Chmaissem, O.; Jorgensen, J.D.; Short, S.; Knizhnik, A.; Eckstein, Y.; Shaked, H. Scaling of transition temperature and CuO2 plane buckling in a high-temperature superconductor. Nature 1999, 397, 45–48. [Google Scholar] [CrossRef]
- Yu, Y.; Yang, F.; Lu, X.F.; Yan, Y.J.; Cho, Y.-H.; Ma, L.; Niu, X.; Kim, S.; Son, Y.-W.; Feng, D.; et al. Gate-tunable phase transitions in thin flakes of 1T-TaS2. Nat. Nanotechnol. 2015, 10, 270–276. [Google Scholar] [CrossRef]
- Zhang, R.; Waters, J.; Geim, A.K.; Grigorieva, I.V. Intercalant-independent transition temperature in superconducting black phosphorus. Nat. Commun. 2017, 8, 15036. [Google Scholar] [CrossRef] [PubMed]
- Ugeda, M.M.; Bradley, A.J.; Zhang, Y.; Onishi, S.; Chen, Y.; Ruan, W.; Ojeda-Aristizabal, C.; Ryu, H.; Edmonds, M.T.; Tsai, H.-Z.; et al. Characterization of collective ground states in single-layer NbSe2. Nat. Phys. 2016, 12, 92–97. [Google Scholar] [CrossRef]
- Sipos, B.; Kusmartseva, A.F.; Akrap, A.; Berger, H.; Forró, L.; Tutiš, E. From Mott state to superconductivity in 1T-TaS2. Nat. Mater. 2008, 7, 960–965. [Google Scholar] [CrossRef]
- Wu, C.-L.; Yuan, H.; Li, Y.; Gong, Y.; Hwang, H.Y.; Cui, Y. Gate-Induced Metal–Insulator Transition in MoS2 by Solid Superionic Conductor LaF3. Nano Lett. 2018, 18, 2387–2392. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Lian, H.; He, J.; Liu, J.; Wang, S.; Xing, H.; Mao, Z.; Liu, Y. Lithium ion intercalation in thin crystals of hexagonal TaSe2 gated by a polymer electrolyte. Appl. Phys. Lett. 2018, 112, 023502. [Google Scholar] [CrossRef]
- Strocov, V.N.; Shi, M.; Kobayashi, M.; Monney, C.; Wang, X.; Krempasky, J.; Schmitt, T.; Patthey, L.; Berger, H.; Blaha, P. Three-Dimensional Electron Realm in VSe2 by Soft-X-Ray Photoelectron Spectroscopy: Origin of Charge-Density Waves. Phys. Rev. Lett. 2012, 109, 86401. [Google Scholar] [CrossRef]
- Fang, L.; Wang, Y.; Zou, P.Y.; Tang, L.; Xu, Z.; Chen, H.; Dong, C.; Shan, L.; Wen, H.H. Fabrication and superconductivity of NaxTaS2 crystals. Phys. Rev. B 2005, 72, 14534. [Google Scholar] [CrossRef]
- Yokota, K.-I.; Kurata, G.; Matsui, T.; Fukuyama, H. Superconductivity in the quasi-two-dimensional conductor 2H-TaSe2. Phys. B Condens. Matter 2000, 284–288, 551–552. [Google Scholar] [CrossRef]
- Bhoi, D.; Khim, S.; Nam, W.; Lee, B.S.; Kim, C.; Jeon, B.G.; Min, B.H.; Park, S.; Kim, K.H. Interplay of charge density wave and multiband superconductivity in 2H-PdxTaSe2. Sci. Rep. 2016, 6, 24068. [Google Scholar] [CrossRef]
- Di Salvo, F.J.; Moncton, D.E.; Waszczak, J.V. Electronic properties and superlattice formation in the semimetal TiSe2. Phys. Rev. B 1976, 14, 4321–4328. [Google Scholar] [CrossRef]
- Morosan, E.; Zandbergen, H.W.; Dennis, B.S.; Bos, J.W.G.; Onose, Y.; Klimczuk, T.; Ramirez, A.P.; Ong, N.P.; Cava, R.J. Superconductivity in CuxTiSe2. Nat. Phys. 2006, 2, 544–550. [Google Scholar] [CrossRef]
- Wu, G.; Yang, H.X.; Zhao, L.; Luo, X.G.; Wu, T.; Wang, G.Y.; Chen, X.H. Transport properties of single-crystalline CuxTiSe2 (0.015 ≤ x ≤ 0.110). Phys. Rev. B 2007, 76, 24513. [Google Scholar] [CrossRef]
- Zhao, J.F.; Ou, H.W.; Wu, G.; Xie, B.P.; Zhang, Y.; Shen, D.W.; Wei, J.; Yang, L.X.; Dong, J.K.; Arita, M.; et al. Evolution of the Electronic Structure of 1T-CuxTiSe2. Phys. Rev. Lett. 2007, 99, 146401. [Google Scholar] [CrossRef]
- Cercellier, H.; Monney, C.; Clerc, F.; Battaglia, C.; Despont, L.; Garnier, M.G.; Beck, H.; Aebi, P.; Patthey, L.; Berger, H.; et al. Evidence for an Excitonic Insulator Phase in 1T-TiSe2. Phys. Rev. Lett. 2007, 99, 146403. [Google Scholar] [CrossRef] [PubMed]
- Hildebrand, B.; Jaouen, T.; Didiot, C.; Razzoli, E.; Monney, G.; Mottas, M.L.; Ubaldini, A.; Berger, H.; Barreteau, C.; Beck, H.; et al. Short-range phase coherence and origin of the 1T-TiSe2 charge density wave. Phys. Rev. B 2016, 93, 125140. [Google Scholar] [CrossRef]
- Rossnagel, K. On the origin of charge-density waves in select layered transition-metal dichalcogenides. J. Phys. Condens. Matter 2011, 23, 213001. [Google Scholar] [CrossRef]
- Porer, M.; Leierseder, U.; Ménard, J.M.; Dachraoui, H.; Mouchliadis, L.; Perakis, I.E.; Heinzmann, U.; Demsar, J.; Rossnagel, K.; Huber, R. Non-thermal separation of electronic and structural orders in a persisting charge density wave. Nat. Mater. 2014, 13, 857–861. [Google Scholar] [CrossRef]
- Li, L.J.; O’Farrell, E.C.T.; Loh, K.P.; Eda, G.; Özyilmaz, B.; Castro Neto, A.H. Controlling many-body states by the electric-field effect in a two-dimensional material. Nature 2016, 529, 185–189. [Google Scholar] [CrossRef]
- Yan, S.; Iaia, D.; Morosan, E.; Fradkin, E.; Abbamonte, P.; Madhavan, V. Influence of Domain Walls in the Incommensurate Charge Density Wave State of Cu Intercalated 1T-TiSe2. Phys. Rev. Lett. 2017, 118, 106405. [Google Scholar] [CrossRef]
- Novello, A.M.; Spera, M.; Scarfato, A.; Ubaldini, A.; Giannini, E.; Bowler, D.R.; Renner, C. Stripe and Short Range Order in the Charge Density Wave of 1T-CuxTiSe2. Phys. Rev. Lett. 2017, 118, 17002. [Google Scholar] [CrossRef]
- Cheng, Y.; Zong, A.; Li, J.; Xia, W.; Duan, S.; Zhao, W.; Li, Y.; Qi, F.; Wu, J.; Zhao, L.; et al. Light-induced dimension crossover dictated by excitonic correlations. Nat. Commun. 2022, 13, 963. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Zhang, X.; Bao, S.; Zhang, Z.; Fei, H.; Wu, Z. Phase engineering of a multiphasic 1T/2H MoS2 catalyst for highly efficient hydrogen evolution. J. Mater. Chem. A 2017, 5, 2681–2688. [Google Scholar] [CrossRef]
- Radisavljevic, B.; Kis, A. Mobility engineering and a metal–insulator transition in monolayer MoS2. Nat. Mater. 2013, 12, 815–820. [Google Scholar] [CrossRef] [PubMed]
- Friedman, A.L.; Hanbicki, A.T.; Perkins, F.K.; Jernigan, G.G.; Culbertson, J.C.; Campbell, P.M. Evidence for Chemical Vapor Induced 2H to 1T Phase Transition in MoX2 (X = Se, S) Transition Metal Dichalcogenide Films. Sci. Rep. 2017, 7, 3836. [Google Scholar] [CrossRef] [PubMed]
- Kang, Y.; Najmaei, S.; Liu, Z.; Bao, Y.; Wang, Y.; Zhu, X.; Halas, N.J.; Nordlander, P.; Ajayan, P.M.; Lou, J.; et al. Plasmonic Hot Electron Induced Structural Phase Transition in a MoS2 Monolayer. Adv. Mater. 2014, 26, 6467–6471. [Google Scholar] [CrossRef]
- Cheng, P.; Sun, K.; Hu, Y.H. Memristive Behavior and Ideal Memristor of 1T Phase MoS2 Nanosheets. Nano Lett. 2016, 16, 572–576. [Google Scholar] [CrossRef]
- Muhammad, Z.; Mu, K.; Lv, H.; Wu, C.; ur Rehman, Z.; Habib, M.; Sun, Z.; Wu, X.; Song, L. Electron doping induced semiconductor to metal transitions in ZrSe2 layers via copper atomic intercalation. Nano Res. 2018, 11, 4914–4922. [Google Scholar] [CrossRef]
- Wang, N.; Tang, H.; Shi, M.; Zhang, H.; Zhuo, W.; Liu, D.; Meng, F.; Ma, L.; Ying, J.; Zou, L.; et al. Transition from Ferromagnetic Semiconductor to Ferromagnetic Metal with Enhanced Curie Temperature in Cr2Ge2Te6 via Organic Ion Intercalation. J. Am. Chem. Soc. 2019, 141, 17166–17173. [Google Scholar] [CrossRef]
- Huang, B.; Clark, G.; Navarro-Moratalla, E.; Klein, D.R.; Cheng, R.; Seyler, K.L.; Zhong, D.; Schmidgall, E.; McGuire, M.A.; Cobden, D.H.; et al. Layer-dependent ferromagnetism in a van der Waals crystal down to the monolayer limit. Nature 2017, 546, 270–273. [Google Scholar] [CrossRef]
- Gong, C.; Li, L.; Li, Z.; Ji, H.; Stern, A.; Xia, Y.; Cao, T.; Bao, W.; Wang, C.; Wang, Y.; et al. Discovery of intrinsic ferromagnetism in two-dimensional van der Waals crystals. Nature 2017, 546, 265–269. [Google Scholar] [CrossRef]
- Torelli, D.; Moustafa, H.; Jacobsen, K.W.; Olsen, T. High-throughput computational screening for two-dimensional magnetic materials based on experimental databases of three-dimensional compounds. Npj Comput. Mater. 2020, 6, 158. [Google Scholar] [CrossRef]
- Yu, W.; Wang, Z.; Zhao, X.; Wang, J.; Herng, T.S.; Ma, T.; Zhu, Z.; Ding, J.; Eda, G.; Pennycook, S.J.; et al. Domain Engineering in ReS2 by Coupling Strain during Electrochemical Exfoliation. Adv. Funct. Mater. 2020, 30, 2003057. [Google Scholar] [CrossRef]
- Liu, F.; Zheng, S.; He, X.; Chaturvedi, A.; He, J.; Chow, W.L.; Mion, T.R.; Wang, X.; Zhou, J.; Fu, Q.; et al. Highly Sensitive Detection of Polarized Light Using Anisotropic 2D ReS2. Adv. Funct. Mater. 2016, 26, 1169–1177. [Google Scholar] [CrossRef]
- Jiang, S.; Hong, M.; Wei, W.; Zhao, L.; Zhang, N.; Zhang, Z.; Yang, P.; Gao, N.; Zhou, X.; Xie, C.; et al. Direct synthesis and in situ characterization of monolayer parallelogrammic rhenium diselenide on gold foil. Commun. Chem. 2018, 1, 17. [Google Scholar] [CrossRef]
- Sun, X.; Li, W.; Wang, X.; Sui, Q.; Zhang, T.; Wang, Z.; Liu, L.; Li, D.; Feng, S.; Zhong, S.; et al. Room temperature ferromagnetism in ultra-thin van der Waals crystals of 1T-CrTe2. Nano Res. 2020, 13, 3358–3363. [Google Scholar] [CrossRef]
- Seo, J.; Kim, D.Y.; An, E.S.; Kim, K.; Kim, G.-Y.; Hwang, S.-Y.; Kim, D.W.; Jang, B.G.; Kim, H.; Eom, G.; et al. Nearly room temperature ferromagnetism in a magnetic metal-rich van der Waals metal. Sci. Adv. 2020, 6, eaay8912. [Google Scholar] [CrossRef]
- Huang, M.; Ma, Z.; Wang, S.; Li, S.; Li, M.; Xiang, J.; Liu, P.; Hu, G.; Zhang, Z.; Sun, Z.; et al. Significant perpendicular magnetic anisotropy in room-temperature layered ferromagnet of Cr-intercalated CrTe2. 2D Mater. 2021, 8, 31003. [Google Scholar] [CrossRef]
- May, A.F.; Calder, S.; Cantoni, C.; Cao, H.; McGuire, M.A. Magnetic structure and phase stability of the van der Waals bonded ferromagnet Fe3-xGeTe2. Phys. Rev. B 2016, 93, 14411. [Google Scholar] [CrossRef]
- Deng, Y.; Yu, Y.; Song, Y.; Zhang, J.; Wang, N.Z.; Sun, Z.; Yi, Y.; Wu, Y.Z.; Wu, S.; Zhu, J.; et al. Gate-tunable room-temperature ferromagnetism in two-dimensional Fe3GeTe2. Nature 2018, 563, 94–99. [Google Scholar] [CrossRef]
- Weber, D.; Trout, A.H.; McComb, D.W.; Goldberger, J.E. Decomposition-Induced Room-Temperature Magnetism of the Na-Intercalated Layered Ferromagnet Fe3–xGeTe2. Nano Lett. 2019, 19, 5031–5035. [Google Scholar] [CrossRef]
- Yu, W.; Li, J.; Herng, T.S.; Wang, Z.; Zhao, X.; Chi, X.; Fu, W.; Abdelwahab, I.; Zhou, J.; Dan, J.; et al. Chemically Exfoliated VSe2 Monolayers with Room-Temperature Ferromagnetism. Adv. Mater. 2019, 31, 1903779. [Google Scholar] [CrossRef] [PubMed]
- Bonilla, M.; Kolekar, S.; Ma, Y.; Diaz, H.C.; Kalappattil, V.; Das, R.; Eggers, T.; Gutierrez, H.R.; Phan, M.-H.; Batzill, M. Strong room-temperature ferromagnetism in VSe2 monolayers on van der Waals substrates. Nat. Nanotechnol. 2018, 13, 289–293. [Google Scholar] [CrossRef] [PubMed]
- Duvjir, G.; Choi, B.K.; Jang, I.; Ulstrup, S.; Kang, S.; Thi Ly, T.; Kim, S.; Choi, Y.H.; Jozwiak, C.; Bostwick, A.; et al. Emergence of a Metal–Insulator Transition and High-Temperature Charge-Density Waves in VSe2 at the Monolayer Limit. Nano Lett. 2018, 18, 5432–5438. [Google Scholar] [CrossRef]
- Coelho, P.M.; Nguyen Cong, K.; Bonilla, M.; Kolekar, S.; Phan, M.-H.; Avila, J.; Asensio, M.C.; Oleynik, I.I.; Batzill, M. Charge Density Wave State Suppresses Ferromagnetic Ordering in VSe2 Monolayers. J. Phys. Chem. C 2019, 123, 14089–14096. [Google Scholar] [CrossRef]
- Guo, Y.; Sun, D.; Ouyang, B.; Raja, A.; Song, J.; Heinz, T.F.; Brus, L.E. Probing the Dynamics of the Metallic-to-Semiconducting Structural Phase Transformation in MoS2 Crystals. Nano Lett. 2015, 15, 5081–5088. [Google Scholar] [CrossRef]
- Leng, K.; Chen, Z.; Zhao, X.; Tang, W.; Tian, B.; Nai, C.T.; Zhou, W.; Loh, K.P. Phase Restructuring in Transition Metal Dichalcogenides for Highly Stable Energy Storage. ACS Nano 2016, 10, 9208–9215. [Google Scholar] [CrossRef]
- Ng, H.K.; Abutaha, A.; Voiry, D.; Verzhbitskiy, I.; Cai, Y.; Zhang, G.; Liu, Y.; Wu, J.; Chhowalla, M.; Eda, G.; et al. Effects Of Structural Phase Transition On Thermoelectric Performance in Lithium-Intercalated Molybdenum Disulfide (LixMoS2). ACS Appl. Mater. Interfaces 2019, 11, 12184–12189. [Google Scholar] [CrossRef]
- Huang, H.; Cui, Y.; Li, Q.; Dun, C.; Zhou, W.; Huang, W.; Chen, L.; Hewitt, C.A.; Carroll, D.L. Metallic 1T phase MoS2 nanosheets for high-performance thermoelectric energy harvesting. Nano Energy 2016, 26, 172–179. [Google Scholar] [CrossRef]
- Hippalgaonkar, K.; Wang, Y.; Ye, Y.; Qiu, D.Y.; Zhu, H.; Wang, Y.; Moore, J.; Louie, S.G.; Zhang, X. High thermoelectric power factor in two-dimensional crystals of MoS2. Phys. Rev. B 2017, 95, 115407. [Google Scholar] [CrossRef]
- Zhu, X.; Li, D.; Liang, X.; Lu, W.D. Ionic modulation and ionic coupling effects in MoS2 devices for neuromorphic computing. Nat. Mater. 2019, 18, 141–148. [Google Scholar] [CrossRef]
- Li, Y.; Wu, D.; Zhou, Z.; Cabrera, C.R.; Chen, Z. Enhanced Li Adsorption and Diffusion on MoS2 Zigzag Nanoribbons by Edge Effects: A Computational Study. J. Phys. Chem. Lett. 2012, 3, 2221–2227. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Li, Z.; Su, Y.; Wu, C.; Xie, Y. Ultralow In-Plane Thermal Conductivity in 2D Magnetic Mosaic Superlattices for Enhanced Thermoelectric Performance. ACS Nano 2022, 16, 11152–11160. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Shi, X.; Xu, F.; Zhang, L.; Zhang, W.; Chen, L.; Li, Q.; Uher, C.; Day, T.; Snyder, G.J. Copper ion liquid-like thermoelectrics. Nat. Mater. 2012, 11, 422–425. [Google Scholar] [CrossRef] [PubMed]
- Zhao, W.; Liu, Z.; Sun, Z.; Zhang, Q.; Wei, P.; Mu, X.; Zhou, H.; Li, C.; Ma, S.; He, D.; et al. Superparamagnetic enhancement of thermoelectric performance. Nature 2017, 549, 247–251. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Wang, Y.; Zhao, Z.; Song, W.; Zhou, X.; Li, Z. Interlayer Chemical Modulation of Phase Transitions in Two-Dimensional Metal Chalcogenides. Molecules 2023, 28, 959. https://doi.org/10.3390/molecules28030959
Zhang Z, Wang Y, Zhao Z, Song W, Zhou X, Li Z. Interlayer Chemical Modulation of Phase Transitions in Two-Dimensional Metal Chalcogenides. Molecules. 2023; 28(3):959. https://doi.org/10.3390/molecules28030959
Chicago/Turabian StyleZhang, Zhi, Yi Wang, Zelin Zhao, Weijing Song, Xiaoli Zhou, and Zejun Li. 2023. "Interlayer Chemical Modulation of Phase Transitions in Two-Dimensional Metal Chalcogenides" Molecules 28, no. 3: 959. https://doi.org/10.3390/molecules28030959
APA StyleZhang, Z., Wang, Y., Zhao, Z., Song, W., Zhou, X., & Li, Z. (2023). Interlayer Chemical Modulation of Phase Transitions in Two-Dimensional Metal Chalcogenides. Molecules, 28(3), 959. https://doi.org/10.3390/molecules28030959




