Two-Dimensional Transition Metal Boride TMB₁₂ (TM = V, Cr, Mn, and Fe) Monolayers: Robust Antiferromagnetic Semiconductors with Large Magnetic Anisotropy

Abstract

Currently, two-dimensional (2D) materials with intrinsic antiferromagnetism have stimulated research interest due to their insensitivity to external magnetic fields and absence of stray fields. Here, we predict a family of stable transition metal (TM) borides, TMB₁₂ (TM = V, Cr, Mn, Fe) monolayers, by combining TM atoms and B₁₂ icosahedra based on first-principles calculations. Our results show that the four TMB₁₂ monolayers have stable antiferromagnetic (AFM) ground states with large magnetic anisotropic energy. Among them, three TMB₁₂ (TM=V, Cr, Mn) monolayers display an in-plane easy magnetization axis, while the FeB₁₂ monolayer has an out-of-plane easy magnetization axis. Among them, the CrB₁₂ and the FeB₁₂ monolayers are AFM semiconductors with band gaps of 0.13 eV and 0.35 eV, respectively. In particular, the AFM FeB₁₂ monolayer is a spin-polarized AFM material with a Néel temperature of 125 K. Moreover, the electronic and magnetic properties of the CrB₁₂ and the FeB₁₂ monolayers can be modulated by imposing external biaxial strains. Our findings show that the TMB₁₂ monolayers are candidates for designing 2D AFM materials, with potential applications in electronic devices.

1. Introduction

With the development of spintronics, the search for magnetic semiconductors has become a prominent topic in both scientific and industrial communities due to their great potential to act as next-generation information storage devices. Compared to three-dimensional (3D) magnetic materials, two-dimensional (2D) magnetic materials hold appealing properties such as high crystallinity and flexibility, high optical transparency, large carrier mobility, etc., which are promising in low-power and ultra-compact spintronics at nanoscale [1,2,3,4,5,6,7,8]. Spintronics can combine standard microelectronics with spin-dependent effects, in which the electron spin carries information and provides opportunities for a new platform for designing devices, arising from the interaction between the spin of the carrier and the magnetic properties of the materials. Unfortunately, many 2D materials synthesized in experiments are nonmagnetic, such as graphene [9], silicene [10], phosphorus [11], etc.

To date, various approaches have been proposed to regulate the spin configurations of 2D materials, such as applying an electric field, imposing external strains, doping, building heterostructures, and so on. However, finding 2D materials with intrinsic magnetism is still a prominent topic and many efforts have been devoted to it. A milestone finding of 2D magnetic materials research is the successful fabrication of an atomically thick CrI₃ monolayer and a Cr₂Ge₂Te₆ bilayer by exfoliation from their van der Waals bulks, illustrated in 2017 by Gong et al. [12] and Huang et al. [13], respectively [12,13], which highly accelerated the development of 2D magnetic materials. To date, numerous 2D magnetic materials have been reported theoretically or experimentally, such as transition metal (TM) trihalide monolayers (TMX₃, X = F, Cl, Br, I) [14,15,16,17], TM dichalcogenides or dihalides (TMX₂, TM = V, Mn, Fe, Co, Ni; X = S, Se, Cl, Br, I) [18,19,20], and ternary materials like Fe₃GeTe₂ [21,22,23], TMPX₃ (X = S, Se, Te) [24,25], TMSiTe₃ [26,27], CrSnTe₃ [28], CrGaSe₃ [29], Cr_2x3S₃ (X = Br, I) [30], CrSBr [31,32], EuSn₂X₂ (X = P, As) [33], TMInX₃ (X = Te, Se) [34,35], etc., all of which have been widely studied in terms of magnetoelectric coupling, magnetoresistance, and proximity effects. However, the transition temperatures of most of the known 2D magnetic materials are significantly lower than room temperature, which largely hinders their practical applications. For example, the Curie temperature for the ferromagnetic (FM) bilayer Cr₂Ge₂Te₆ [12], the monolayer CrX₃ (X = Cl, Br, I) [13,14,15,16,17], and Fe₃GeTe₂ [21] are 40 K, 45 K (X = I)/17 K (X = Cl)/34 K (X = Br) and 130 K, respectively. Moreover, compared to FM semiconductors, antiferromagnetic (AFM) semiconductors have become a promising research field due to their robustness against magnetic field perturbation, no stray fields, high-frequency dynamics, etc., which enable them to potentially replace ferromagnets as the active components of spintronic devices [36].

Among the discovered 2D magnetic materials, 2D TM borides composed of TM atoms and boron (B) atoms have attracted great attention in recent years and have become potential candidates for designing 2D magnetic materials. As the valence states of the B atom are unsaturated, it can easily hybridize with exotic atoms and display distinct electronic properties. Currently, a number of 2D TM borides with rich stoichiometries and geometric configurations have been predicted in theory and synthesized in experiments, such as TMB_x (x = 1-6, 8, 9, 12) [37,38,39,40,41,42,43,44,45,46,47], TM_nB_n (n = 1, 2) [37,38,48], TM_nB_m (n = 2, m = 3, 6, 12) [49,50,51], etc., and robust magnetic properties have been found in these TM borides. For example, Ozdemir et al. found that an Fe₂B₂ monolayer with rectangular lattice and Fe atoms in different atomic planes has a stable columnar AFM ground state, which can change to an FM order with double hysteresis behavior [48]. Zhang et al. [39] found that the hexagonal FeB₂ monolayer is a Dirac material with a Fermi velocity comparable to that of graphene. Our group [40] suggested that an FeB₃ monolayer with a stripe arrangement of Fe atoms is a robust FM metal with a high Curie temperature of 367 K. Zhang et al. [42] predicted the graphene-like FeB₆ monolayer is an indirect bandgap semiconductor with a band gap of 1.89 eV. In another study, we predicted a type of stable sandwiched B-Fe-B compound, known as the Fe₂B₆ monolayer, in which the Curie temperature is as high as 420 K [50]. Recently, we found that the monoatom-thickness FeB₁₂ monolayer, composed of a TM©B₈ wheel and B₄ cluster within the space group of Pmmm, is a stable AFM metal [52]. Therefore, TM borides provide a playground for studying 2D magnetic materials.

Here, based on density functional theory calculations, we report a new class of 2D AFM TM borides, TMB₁₂ (TM = V, Cr, Mn, and Fe) monolayers composed of TM atoms and B₁₂ icosahedra. Such structures are different from the TMB₁₂ monolayer, with the same chemical formula as we predicted previously [52]. On the basis of the results of lattice dynamic calculations and thermodynamic studies, we show that 2D TMB₁₂ structures are stable AFM semiconductors or metals. The antiferromagnetism mainly arises from the super-exchange interaction between the B-p orbitals and TM-3d orbitals. It is found that the VB₁₂ monolayer and the MnB₁₂ monolayer are AFM metals, while the CrB₁₂ monolayer and the FeB₁₂ monolayer are AFM semiconductors with band gaps of 0.13 eV and 0.35 eV, respectively. In addition, all four TMB₁₂ monolayers have large magnetic anisotropic energy, and three TMB₁₂ (TM = V, Cr, Mn) monolayers display an in-plane easy magnetization axis, while the FeB₁₂ monolayer has an out-of-plane easy magnetization axis. More interestingly, the AFM semiconducting CrB₁₂ monolayer and FeB₁₂ monolayer can be modulated into an FM half metal or an FM metal under biaxial strains, and the magnetic transition temperature and band gap can also be adjusted. Our findings provide a new strategy for designing 2D TM borides with potential applications in electronic and spintronic devices, though such TMB₁₂ monolayers have not yet been synthesized in experiment.

2. Results and Discussions

The top and side views of the TMB₁₂ (TM = V, Cr, Mn, and Fe) monolayer are shown in Figure 1a, which consists of TM atoms and B₁₂ icosahedra, and the TM atoms bind with four nearby B₁₂ icosahedras. The TMB₁₂ monolayers have space groups of C2/m. Moreover, both phases have the potential to be fabricated by using different precursors in different experimental conditions. As summarized in

Table 1. The lattice constant (L, Å), monoclinic angle (θ, degree), TM-B bond length (d_TM-B, Å), formation energy (E_f, eV), energy difference between the ground AFM state and the FM state (ΔE = E_FM − E_AFM, eV), local magnetic moment on TM atoms (LMM, μ_B), electrons transferred from TM atoms to B atoms (Δe, e), exchange integral (J₁, J₂, meV), and Néel temperatures (T_N, K) for TMB₁₂ monolayers (TM = V, Cr, Mn, and Fe).

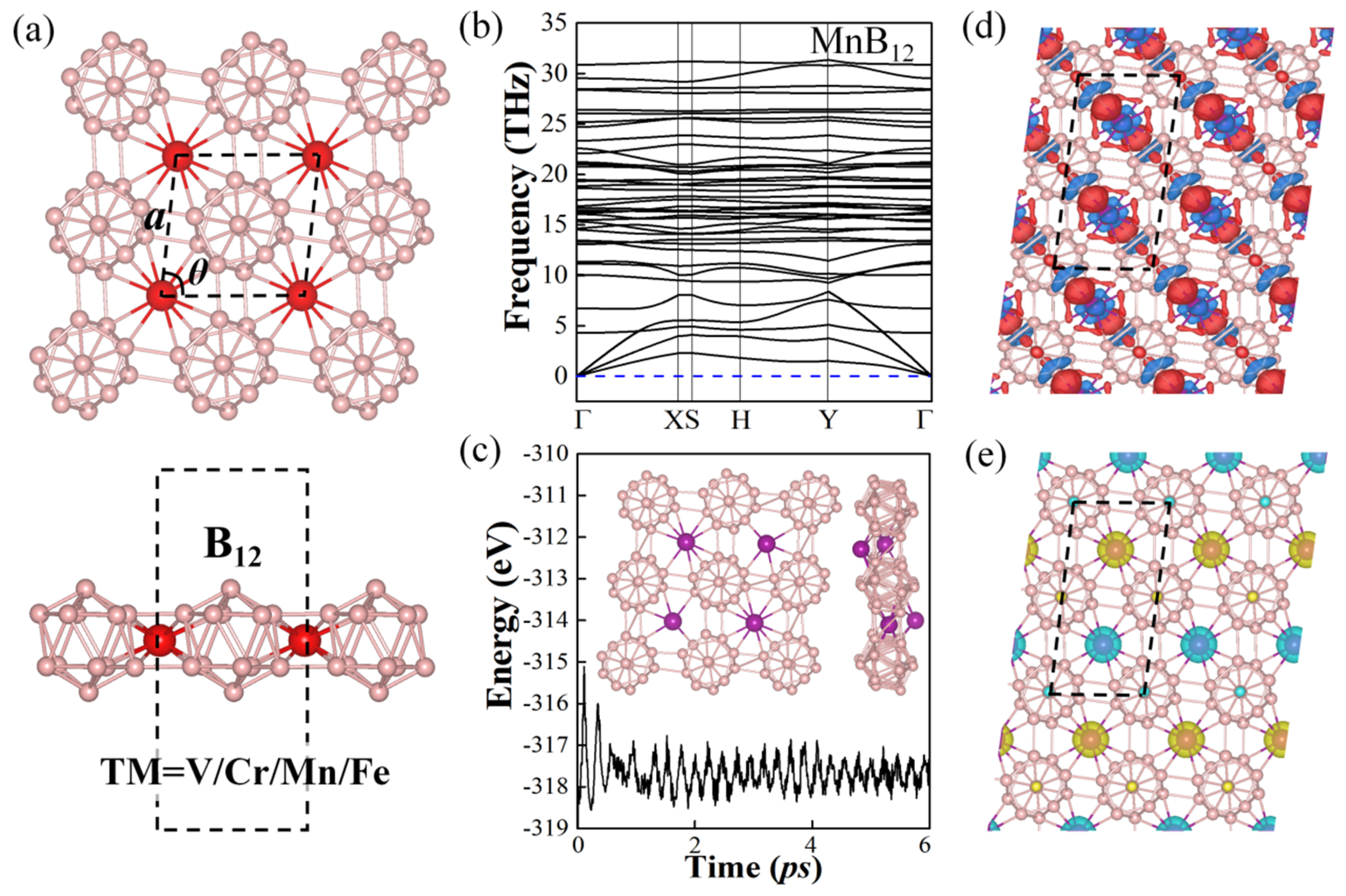
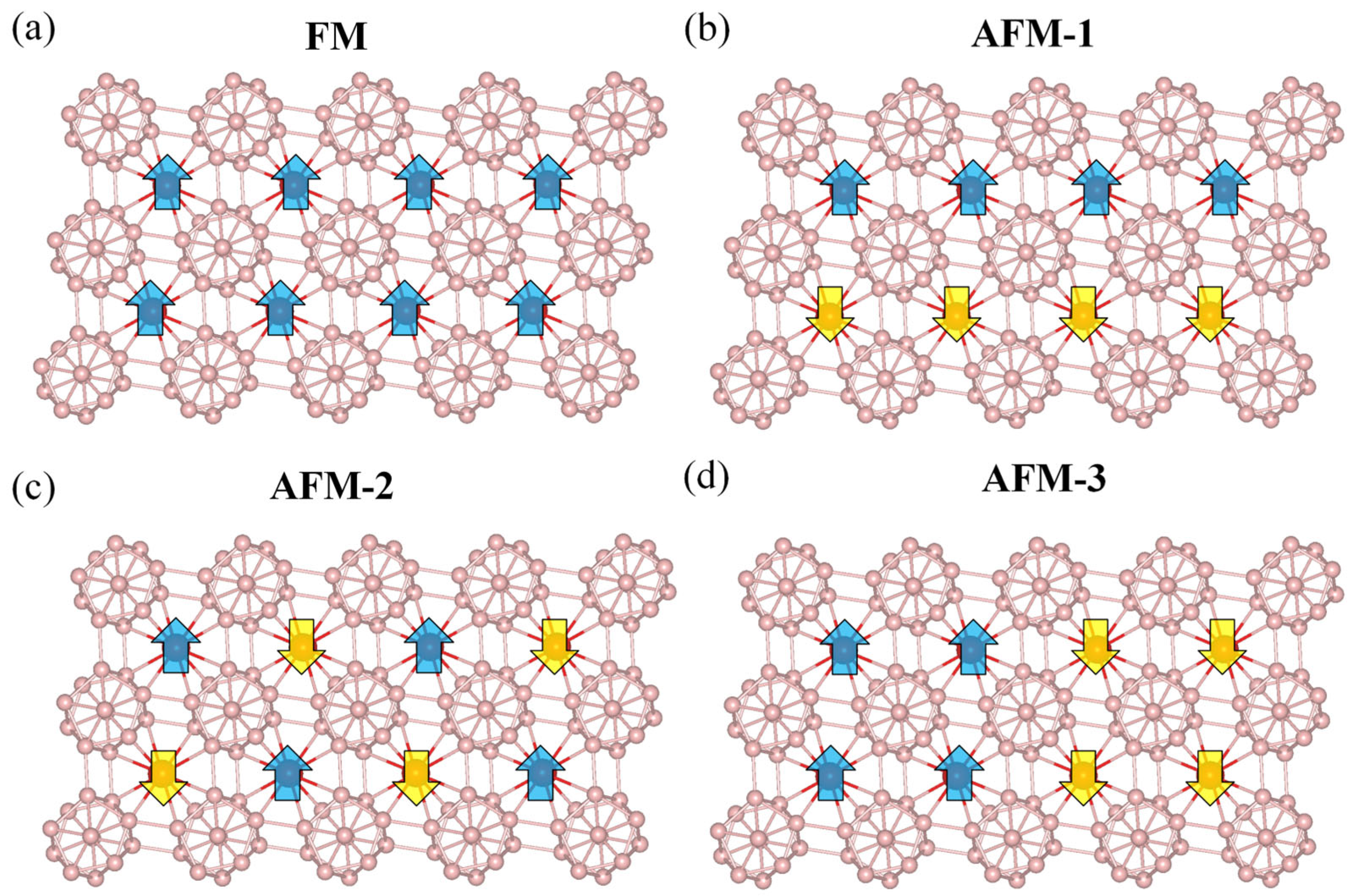
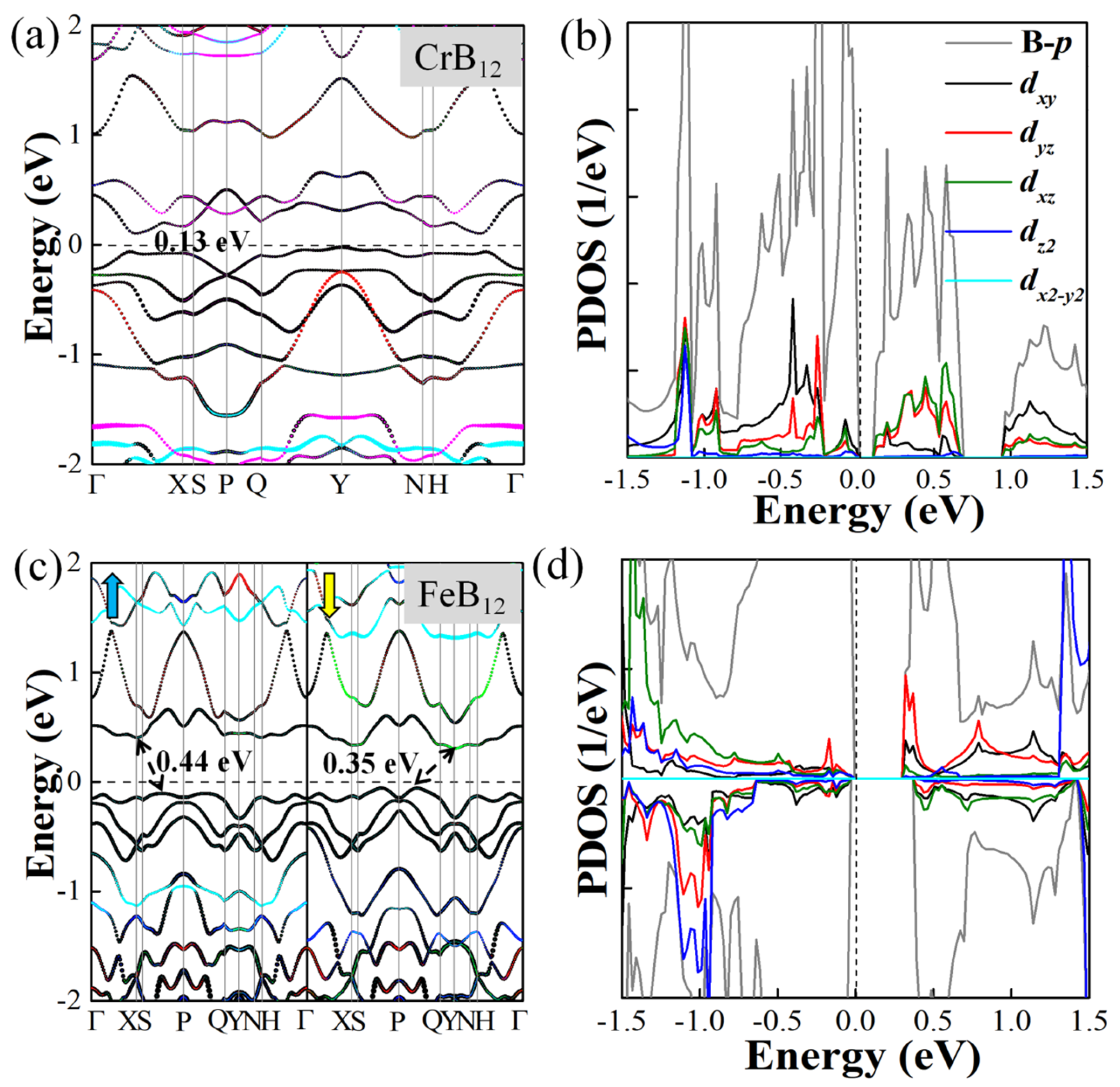
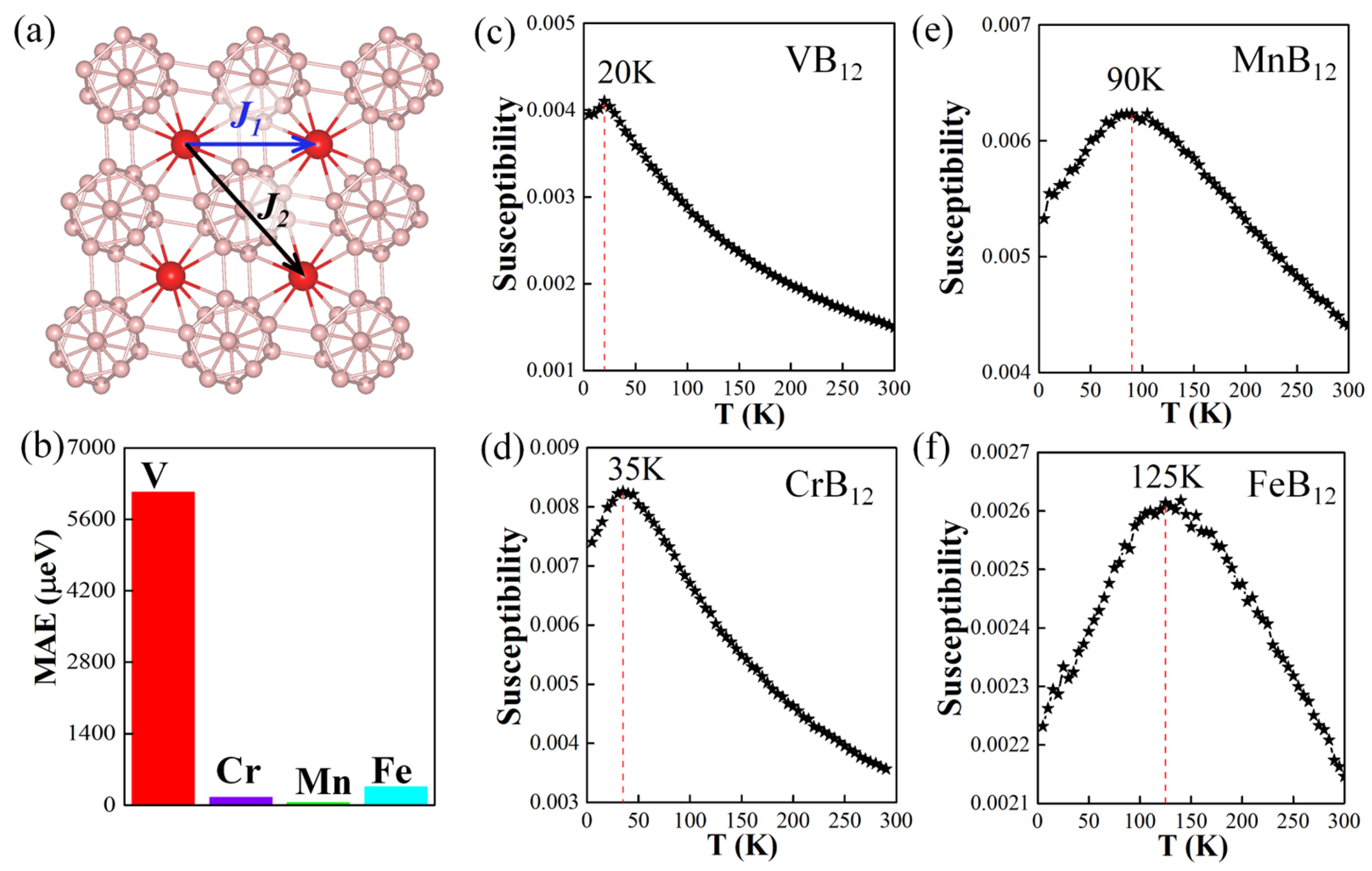
Sys	L	θ	dTM-B	E	ΔE	LMM	Δe	J1	J2	TN
VB12	4.78	83.39	2.05–2.30	−0.68	0.07	2.05	1.05	−3.88	−4.81	20
CrB12	4.78	83.95	2.06–2.32	−0.63	0.08	3.18	0.93	−2.09	−6.93	35
MnB12	4.86	82.52	2.01–2.35	−0.74	0.34	3.86	0.93	−2.80	−16.03	90
FeB12	4.81	81.76	1.97–2.34	−0.58	0.18	2.79	0.66	−0.85	−22.58	125

, the schematic of lattice constants (a) and monoclinic angles (θ) are shown in the top view of TMB₁₂ monolayers in Figure 1a. For TM = V, Cr, Mn, and Fe, the lattice constants are 4.78 Å, 4.78 Å, 4.86 Å, and 4.81 Å, respectively, and the monoclinic angles are in the range of 81.76~83.95°. The TM-B bond lengths for the TMB₁₂s are in the range of 1.97~2.35 Å, and the detailed structure parameters of TMB₁₂ monolayers are listed in Table 1.

To determine the stability of these TMB₁₂ monolayers, phonon spectra and ab initio molecular dynamics (AIMD) calculation curves are calculated. As shown in the phonon dispersion spectra plot in Figure 1b and Figure S1a–c, there are no imaginary frequencies along the high symmetry points path in the whole Brillouin zone, indicating that all the TMB₁₂ monolayers are dynamically stable. In addition, the thermodynamical stabilities of the TMB₁₂ monolayers were evaluated using AIMD simulations. The total energy profiles of the TMB₁₂ monolayer are shown in Figure 1c and Figure S1d–f, and the structures of these TMB₁₂ monolayers at the end of 6 ps under 300 K are shown in the insets. It is clearly shown that all the TMB₁₂ monolayers could retain their main framework, indicating that they are thermodynamically stable at room temperature. In addition, the formation energy ($E_f$) of the TMB₁₂ monolayers are calculated using the following Equation (1):

$$E_f=(E_{TMB12}-12{\mu }_B-{\mu }_{TM})/n$$

(1)

where $E_{TMB12}$, ${\mu }_B$, and ${\mu }_{TM}$ are the energies of the TMB₁₂ monolayer, the chemical potential of the B atom in the B₁₂ icosahedron, and the chemical potential of the TM atom in its bulk, respectively, and n is the atom number in the unit cell. The $E_f$ values for TM = V, Cr, Mn, and Fe are −0.68, −0.63, −0.74, and −0.58 eV/atom, respectively, implying that the formation of the TMB₁₂ monolayers is possible and energetically favorable. It is proposed that the TMB₁₂ monolayer may be synthesized by reducing the dimension from bulk to 2D nanosheets [53,54] or by doping the TM atoms in 2D γ-boron film with a certain concentration by the chemical vapor deposition method [55].

The stability of the TMB₁₂ monolayers can be explained by the electron transfer between the TM atoms and B₁₂ icosahedra. The charge density difference (CDD) plots defined as $\Delta \rho ={\rho }_{TMB12}-{\rho }_{TM}-{\rho }_{B12}$ are calculated and plotted in Figure 1d, where ${\rho }_{TMB12}$, ${\rho }_{TM}$, and ${\rho }_{B12}$ are the electron densities of the TMB₁₂ monolayer, the TM atom, and the B₁₂ unit, respectively. It is shown that the red regions are distributed around the bonds between the B₁₂ icosahedra and the TM atoms, as well as the area near the B atoms on top of the B₁₂ icosahedra, while the blue-color regions are mainly around the TM atoms, suggesting that the electrons are depleted from the TM atoms and accumulated on the TM-B bonds and the B atoms of the B₁₂ icosahedra, which indicates that the bonding in such complexes is the coexistence of ionic and covalent bonding. Moreover, the electrons transferred between the B₁₂ icosahedra and the TM atoms are analyzed by calculating the Bader charge (see Table 1). It is found that about 1.05 e, 0.93 e, 0.93 e, and 0.66 e are transferred from the TM atoms to the B atoms at TM = V, Cr, Mn, and Fe, respectively, which is consist with the charge density difference results in Figure 1d.

To ascertain the magnetic ground state of the TMB₁₂ monolayers, four different magnetic configurations, including one FM state and three AFM states, labeled AFM-1, AFM-2, and AFM-3, are considered, as displayed in Figure 2. (i) AFM-1 configuration: the TM atoms with opposite spins are arranged in a stripy distribution (Figure 2b); (ii) AFM-2 configuration: magnetization with up and down directions are in zigzag distribution (Figure 2c); (iii) AFM-3 configuration: two rows of TM with the same spins are in a stripy arrangement (Figure 2d). According to the energies of these magnetic configurations, it is shown that all the TMB₁₂ monolayers favor an AFM ground state, namely, the VB₁₂ monolayer has an AFM-2 ground state, which is 0.016 eV/u.c., 0.001 eV/u.c., and 0.011 eV/u.c. lower than those of FM, AFM-1, and AFM-3 states, respectively. The TMB₁₂ monolayers with TM = Cr, Mn, and Fe have an AFM-1 ground state, which are lower than the FM, AFM-2, and AFM-3 states by about 0.019~0.086 eV/u.c., 0.001~0.046 eV/u.c., and 0.033~0.093 eV/u.c., respectively. Detailed energy information is summarized in Table S1 in SI. The spin density plots of the TMB₁₂ monolayers with different AFM ground states are shown in Figure 1e and Figure S2. The distances of the TM-TM atoms are around 4.78 Å to 4.86 Å, which are much larger than the covalent radius, indicating that the direct exchange interactions are weak. By analyzing the orbital components and the bond angles of the TM-B-TM, it is shown that the antiferromagnetism of the TMB₁₂ monolayers mainly arises from the super-exchange interaction, which comes from the p-d hybridization between the B-p orbitals and the TM-3d orbitals. According to the Goodenough–Kanamori–Anderson (GKA) rule [56,57,58], systems with TM-B-TM angles close to 90° favor an FM magnetic ordering. As shown in Figure S3 in Supplementary Materials, the two TM-B-TM bond angles are around 119.29–122.16° and 125.73–127.61°, which are much larger than 90°; therefore, antiferromagnetic ordering is preferred through a super-exchange interaction.

The projected band structures of these TMB₁₂ monolayers are plotted in Figure 3 and Figure S4. It is shown that the 2D VB₁₂ monolayer and the MnB₁₂ monolayer are AFM metals with bands crossing the Fermi level. The according projected density of states (PDOS) are also explored, as shown in Figure S4 in Supplementary Materials. For the VB₁₂ monolayer, the states around the Fermi level are mainly contributed by the B-p and V-d_z2 orbitals, while for the MnB₁₂ monolayer, the contributions near the Fermi level are mainly from the B-p orbitals, and the Mn-d_yz, d_xz, and d_xy orbitals also have a slight contribution. The CrB₁₂ monolayer and the FeB₁₂ monolayer are AFM semiconductors with band gaps of 0.13 eV and 0.35 eV, respectively. The projected density of states of the semiconducting CrB₁₂ monolayer and the FeB₁₂ monolayer are plotted in Figure 3b,d. It is indicated that for the CrB₁₂ monolayer, the valence band maximum (VBM) is located in the Γ-X path, which is dominated by B-p orbitals, while the conduction band minimum (CBM) is located in the Γ-X path, which primarily comes from the hybridization of B-p, Cr-d_xz, and Cr-d_yz orbitals. As for the FeB₁₂ monolayer (Figure 3d), the CBM located at the X/Y point is dominated by the hybridization of the B-p, Fe-d_xy, and Fe-d_yz orbitals, while the VBM located at the S-P/P-Q path comes from the B-p orbitals. The magnetic moments of these TMB₁₂ monolayers are mainly contributed by the d orbitals of TM atoms, while a few are contributed by p orbitals of the B atoms. The local magnetic moments on the TM atoms are 2.05 μ_B, 3.18 μ_B, 3.86 μ_B, and 2.79 μ_B for TM = V, Cr, Mn, and Fe, respectively.

The magnetic anisotropy energy (MAE) is an important parameter of 2D magnets in terms of thermal stability, which is defined as the larger energy difference between the out-of-plane z direction and in-plane x/y directions: MAE = |E_z − E_x/y|. By considering spin–orbit coupling (SOC) effects (the MAE values of the TMB₁₂ monolayers are displayed in Figure 4b) it is shown that the easy magnetization axes of the VB₁₂ monolayer, the CrB₁₂ monolayer, and the MnB₁₂ monolayer are in-plane and along the x direction, and their MAE values are 6.133 meV, 0.157 meV, and 0.054 meV, respectively. As for the FeB₁₂ monolayer, the easy magnetization axis is out-of-plane and along the z direction with an MAE of 0.357 meV, which is highly desired for information storage. Furthermore, Monte Carlo (MC) simulations were performed to calculate the T_N with the Hamiltonian based on the 2D Heisenberg model written as Equation (2):

$$H=-{\displaystyle \sum _{\mathrm{i},j}{J}_1{S}_i\cdot S_j}-{\displaystyle \sum _{\mathrm{i},k}{J}_2{S}_i\cdot S_k}$$

(2)

where J₁ and J₂ are the nearest and the second-nearest exchange coupling parameters as shown in Figure 4a, and S_i is the spin vector of TM atom on site i. Two exchange parameters (J₁ and J₂) are derived from the energy mapping results of different magnetic configurations as listed in Equation (3):

$$\begin{array}{l}{E}_{FM}=-(8J_1+4J_2)\times S^2\\ E_{AFM-1}=4J_2\times S^2\\ E_{AFM-2}=-(-8J_1+4J_3)\times S^2\\ E_{AFM-3}=-4J_1\times S^2\end{array}$$

(3)

The exchange parameters (J₁ and J₂) can be derived with Equation (4):

$$\begin{array}{l}{J}_1=\frac{E_2-E_0}{16S^2}\\ J_2=\frac{2\times (E_1-E_3)-(E_2-E_1)}{16S^2}\end{array}$$

(4)

The calculated J₁ and J₂ values of the TMB₁₂ monolayers according to the energies of different magnetic configurations are listed in Table 1. It is shown that all the J₁ and J₂ values are negative, indicating the AFM coupling between the TM atoms. The susceptibility as a function of temperature for TMB₁₂ monolayers are shown in Figure 4c–f. The T_Ns can be obtained by locating the peak of the susceptibility, which are 20 K, 35 K, 90 K, and 135 K for the VB₁₂ monolayer, the CrB₁₂ monolayer, the MnB₁₂ monolayer, and the FeB₁₂ monolayer, respectively. Such magnetic transition temperatures are comparable with the reported Cr₂B₁₂ (145 K) and Mn₂B₁₂ (135 K) monolayers [51]. On the other hand, we should state that the magnetic transition temperatures of the predicted systems are much lower than room temperature, which blocks their applications in spintronic devices.

Next, the effects of biaxial strains from −5% to +5% on the electronic and magnetic properties of the semiconducting CrB₁₂ monolayer and the FeB₁₂ monolayer are investigated. The strain intensity is defined as ε = (a − a₀)/a₀, where a₀ and a are the lattice constant before and after imposing biaxial strains. It is proposed that such strains with a uniform strain gradient may be imposed on the TMB₁₂ monolayers by applying a displacement-controlled stretch to the monolayers that can be shaped by focused ion beams (FIB) in experiments. [59] The energy difference (ΔE = E_FM − E_AFM) between the FM and the lowest-energy AFM state as a function of strain intensity are shown in Figure 5a. It is shown that the CrB₁₂ monolayer and the FeB₁₂ monolayer retain the AFM-1 ground states under biaxial compressive strains, which are transformed to FM ground states when the biaxial tensile strains are up to 3% and 1%, respectively. The band structures of the CrB₁₂ monolayer and the FeB₁₂ monolayer under biaxial strains are shown in Figures S5 and S6 in Supplementary Materials. It is shown that for the CrB₁₂ monolayer, it is turned metallic under tensile strains; that is, it is maintained as an AFM-1 metal under 1% and 2% biaxial tensile strains, but turned to an FM half metal under larger (3~5%) tensile strains with the spin band gap of 0.49 eV, 0.60 eV, and 0.69 eV for ε = 3%, 4%, and 5% (Figure S5c–e in Supplementary Materials), respectively. When exerting biaxial compressive strains, the CrB₁₂ monolayer retains the AFM-1 semiconductor property till −4%, with the band gap decreasing with the increasing of the biaxial compressive strains, as shown in Figure 5b and Figure S5f–i. Under −5% biaxial compressive strain, the CrB₁₂ monolayer is transformed into an AFM metal (Figure S5j in Supplementary Materials). As shown in Figure S6 in Supplementary Materials, the semiconducting FeB₁₂ monolayer transforms into an FM metal under biaxial tensile strain within 4%, and it turns into an FM half metal under 5% tensile strain. When biaxial compressive strains are imposed, it maintains the AFM-1 semiconductor properties, in which the band gap decreases with the increasing of compressive strain as shown in Figure 5b. Moreover, the influence of the magnetic transition temperature of the CrB₁₂ and FeB₁₂ monolayers under biaxial strains is explored. As shown in Figure 5c,d, Figures S7 and S8, the T_C of the FM CrB₁₂ monolayer increases with the increasing of the tensile strain from 3% to 5%, and the T_N of the AFM CrB₁₂ monolayer increases with the increasing of the compressive strain from −2% to −5%. There is a sharp increase in T_N at the −2% compressive strain, which is due to the large variation in J₂ as shown in Figure 5e. For the FeB₁₂ monolayer, the T_C of the FM FeB₁₂ monolayer decreases with the increasing of the tensile strains, in which the J₁ and J₂ values are positive. The T_N for the AFM semiconductors or metal are oscillating in the range of 75–120 K, which is due to the different changing trends in J₁ and J₂ as shown in Figure 5f.

3. Materials and Methods

All the first-principles calculations were performed within the Vienna Ab-initio Simulation Package (VASP) [60,61] with projector-augmented wave (PAW) potentials [62]. The exchange–correlation interaction is described by the general gradient approximation of the Perdew–Burke–Ernzerholf (PBE) functional [63], where the generalized gradient approximation (GGA) was employed. The energy cutoff for the plane–wave basis set was set to 500 eV, and the vacuum spaces were larger than 15 Å to eliminate the physical interactions caused by periodic boundary conditions. For transition metal atoms V, Cr, Mn, and Fe, a GGA + U method with U_eff = 4.0 eV was adopted to treat the Coulomb and exchange interactions of the 3d-electron according to previous studies [38,51,64,65]. The k mesh was set as 9 × 9 × 1, 5 × 9 × 1, and 5 × 5 × 1 for FM, AFM-1, and AFM-2 geometry optimization, and a denser k-mesh of 27 × 27 × 1, 15 × 27 × 1, and 15 × 15 × 1 was adopted for electronic structure calculations. Conjugated gradient (CG) atomic optimization was performed with a criterion of convergence for energy and atom force set to 10^-5 eV and 0.01 eV/Å, respectively. During the optimization, both the cell shape and the position of the atoms was fully relaxed. The Néel temperature (T_N) and the Curie temperature (T_C) of the TMB₁₂ monolayers were calculated by using the EspinS package, [66] in which 20 × 20 ×1 lattices were adopted in the Monte Carlo (MC) simulations, and the spins randomly rotated in the space. Phonon dispersions were calculated using 2 × 2 × 1 supercells to reduce the lattice translational constraints using the Phonopy code on the basis of DFPT [67] to identify the dynamic stability, with the threshold for the convergence on energy and force selected as 10⁻⁸ eV and 10⁻⁴ eV/Å. The ab initio molecular dynamics (AIMD) simulations in the NVT ensemble were employed to evaluate the thermal stability using 2 × 2 × 1 supercells with a temperature of 300 K for 6 ps using a Nose–Hoover thermostat method [68].

4. Conclusions

In summary, we predicted a type of 2D transition metal boride, TMB₁₂ (TM = V, Cr, Mn, and Fe) monolayers consisting of transition metal atoms and B₁₂ icosahedras, using first-principles calculations. Our results show that all the TMB₁₂ monolayers are stable antiferromagnets with large magnetic anisotropy, and the Néel temperatures for the TMB₁₂ monolayers range from 20 K to 125 K. Specifically, the CrB₁₂ monolayer and the FeB₁₂ monolayer are antiferromagnetic semiconductors with band gaps of 0.13 eV and 0.35 eV, respectively. In addition, the electronic and magnetic properties of the semiconducting CrB₁₂ monolayer and the FeB₁₂ monolayer could be effectively modulated by exerting biaxial strains due to the variation in exchange parameters. In particular, the FeB₁₂ monolayer is a spin-polarized antiferromagnet with a Néel temperature of 125 K. By imposing tensile strains, the FeB₁₂ monolayer can be altered to be an FM metal with a magnetic transition temperature of up to 210 K. Our findings provide a way to design candidates that are flexible for electronic and spintronic applications.