Benchmark Study of the Electronic States of the LiRb Molecule: Ab Initio Calculations with the Fock Space Coupled Cluster Approach
Abstract
1. Introduction
2. Results and Discussion
2.1. Atomic Energies at the Dissociation Limit
2.2. Dipole Moment
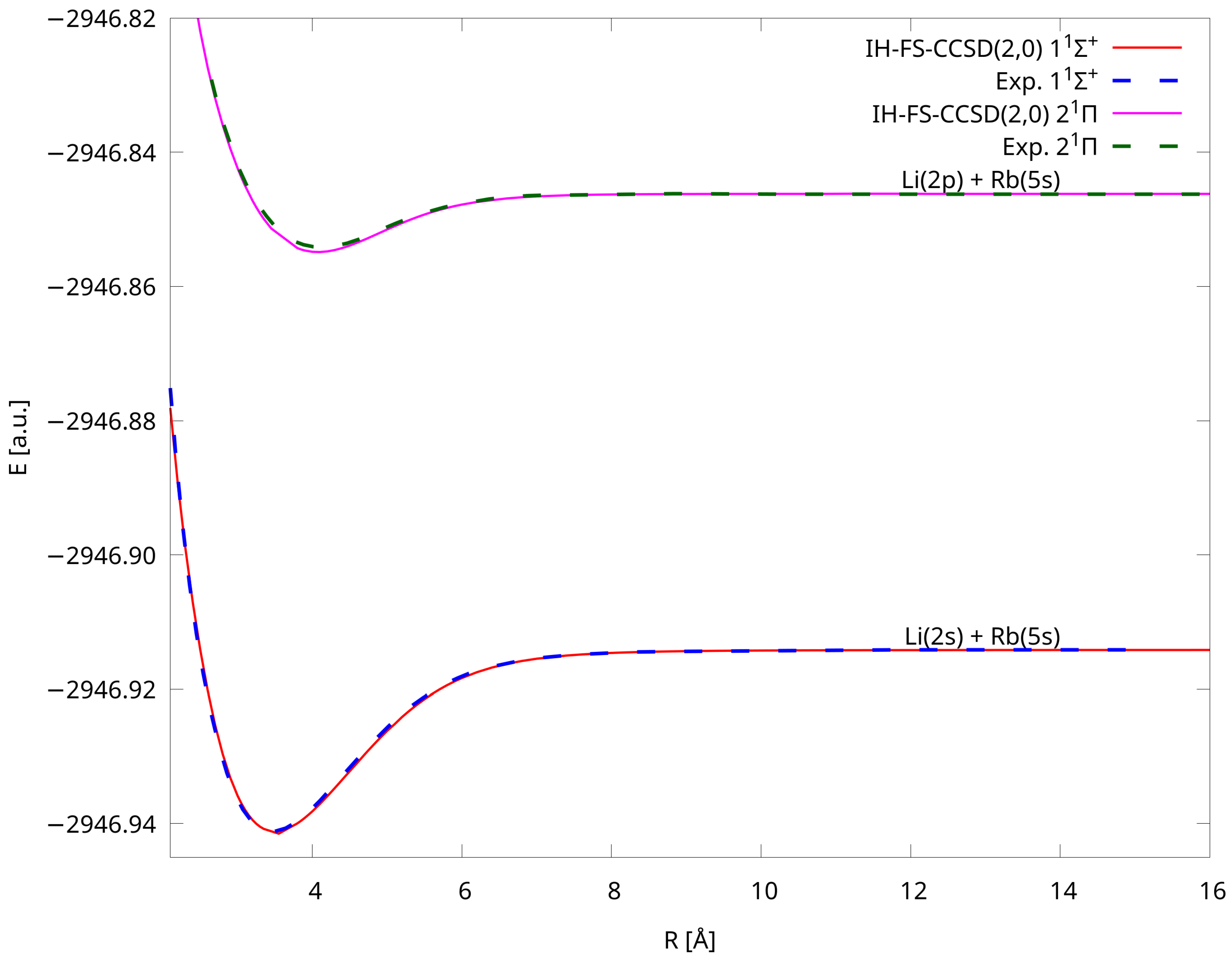
2.3. Potential Energy Curves
2.4. Spectroscopic Constants
3. Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Żuchowski, P.S.; Kosicki, M.; Kodrycka, M.; Soldán, P. Van der Waals coefficients for systems with ultracold polar alkali-metal molecules. Phys. Rev. A 2013, 87, 022706. [Google Scholar] [CrossRef]
- Pazyuk, E.A.; Zaitsevskii, A.V.; Stolyarov, A.V.; Tamanis, M.; Ferber, R. Laser synthesis of ultracold alkali metal dimers: Optimization and control. Russ. Chem. Rev. 2015, 84, 1001–1020. [Google Scholar] [CrossRef]
- Vexiau, R.; Borsalino, D.; Lepers, M.; Orbán, A.; Aymar, M.; Dulieu, O.; Bouloufa-Maafa, N. Dynamic dipole polarizabilities of heteronuclear alkali dimers: Optical response, trapping and control of ultracold molecules. Inv. Rev. Phys. Chem. 2017, 36, 709–750. [Google Scholar] [CrossRef]
- Li, H.; Li, M.; Makrides, C.; Petrov, A.; Kotochigova, S. Universal Scattering of Ultracold Atoms and Molecules in Optical Potentials. Atoms 2019, 7, 36. [Google Scholar] [CrossRef]
- Frye, M.D.; Hutson, J.M. Complexes formed in collisions between ultracold alkali-metal diatomic molecules and atoms. New J. Phys. 2021, 23, 125008. [Google Scholar] [CrossRef]
- Pupillo, G.; Griessner, A.; Micheli, A.; Ortner, M.; Wang, D.W.; Zoller, P. Cold Atoms and Molecules in Self-Assembled Dipolar Lattices. Phys. Rev. Lett. 2008, 100, 050402. [Google Scholar] [CrossRef] [PubMed]
- Aymar, M.; Dulieu, O. Calculation of accurate permanent dipole moments of the lowest 1,3Σ+ states of heteronuclear alkali dimers using extended basis sets. J. Chem. Phys. 2005, 122, 204302. [Google Scholar] [CrossRef] [PubMed]
- Herrera, F.; Litinskaya, M.; Krems, R.V. Tunable disorder in a crystal of cold polar molecules. Phys. Rev. A 2010, 82, 033428. [Google Scholar] [CrossRef]
- Kuznetsova, E.; Yelin, S.F.; Côté, R. An atom-molecule platform for quantum computing. Quantum. Inf. Process. 2011, 10, 821. [Google Scholar] [CrossRef]
- Büchler, H.P.; Micheli, A.; Zoller, P. Three-body interactions with cold polar molecules. Nature Phys 2007, 3, 726–731. [Google Scholar] [CrossRef]
- Cui, Y.; Deng, M.; You, L.; Gao, B.; Tey, M.K. Broad Feshbach resonances in ultracold alkali-metal systems. Phys. Rev. A 2018, 98, 042708. [Google Scholar] [CrossRef]
- Ospelkaus, S.; Ni, K.K.; Wang, D.; de Miranda, M.H.G.; Neyenhuis, B.; Quéméner, G.; Julienne, P.S.; Bohn, J.L.; Jin, D.S.; Ye, J. Quantum-State Controlled Chemical Reactions of Ultracold Potassium-Rubidium Molecules. Science 2010, 327, 853–857. [Google Scholar] [CrossRef] [PubMed]
- Pires, R.; Ulmanis, J.; Häfner, S.; Repp, M.; Arias, A.; Kuhnle, E.D.; Weidemüller, M. Observation of Efimov Resonances in a Mixture with Extreme Mass Imbalance. Phys. Rev. Lett. 2014, 112, 250404. [Google Scholar] [CrossRef] [PubMed]
- Musial, M. Multi-reference Fock space coupled-cluster method in standard an intermediate Hamiltonian formulation for the (2,0) sector. J. Chem. Phys. 2012, 136, 134111. [Google Scholar] [CrossRef] [PubMed]
- Ivanova, M.; Stein, A.; Pashov, A.; Knöckel, H.; Tiemann, E. The X1Σ+ state of LiRb studied by Fourier-transform spectroscopy. J. Chem. Phys. 2011, 134, 024321. [Google Scholar] [CrossRef]
- Ivanova, M.; Stein, A.; Pashov, A.; Knöckel, H.; Tiemann, E. The B1Π and D1Π states of LiRb. J. Chem. Phys. 2013, 138, 094315. [Google Scholar] [CrossRef] [PubMed]
- Dutta, S.; Altaf, A.; Elliott, D.S.; Chen, Y.P. Laser spectroscopy of the X1Σ+ and B1Π states of the LiRb molecule. Chem. Phys. Lett. 2011, 511, 7–11. [Google Scholar] [CrossRef]
- Altaf, A.; Dutta, S.; Lorenz, J.; Pérez-Ríos, J.; Chen, Y.P.; Elliott, D.S. Formation of ultracold 7Li85Rb molecules in the lowest triplet electronic state by photoassociation and their detection by ionization spectroscopy. J. Chem. Phys. 2015, 142, 114310. [Google Scholar] [CrossRef]
- Stevenson, I.C.; Blasing, D.B.; Chen, Y.P.; Elliott, D.S. C1Σ+, A1Σ+, and b3Π0+ states of LiRb. Phys. Rev. A 2016, 94, 062503. [Google Scholar] [CrossRef]
- Stevenson, I.C.; Blasing, D.B.; Altaf, A.; Chen, Y.P.; Elliott, D.S. The d3Π state of LiRb. J. Chem. Phys. 2016, 145, 224301. [Google Scholar] [CrossRef]
- Stevenson, I.C.; Blasing, D.B.; Chen, Y.P.; Elliott, D.S. Production of ultracold ground-state LiRb molecules by photoassociation through a resonantly coupled state. Phys. Rev. A 2016, 94, 062510. [Google Scholar] [CrossRef]
- Urban, M.; Sadlej, A.J. Electronic structure and electric properties of the alkali metal dimers. J. Chem. Phys. 1995, 103, 9692–9704. [Google Scholar] [CrossRef]
- Deiglmayr, J.; Aymar, M.; Wester, R.; Weidemüller, M.; Dulieu, O. Calculations of static dipole polarizabilities of alkali dimers: Prospects for alignment of ultracold molecules. J. Chem. Phys. 2008, 129, 064309. [Google Scholar] [CrossRef]
- Bormotova, E.A.; Kozlov, S.V.; Pazyuk, E.A.; Stolyarov, A.V. Long-range behavior of the transition dipole moments of heteronuclear dimers XY (X, Y = Li, Na, K, Rb) based on ab initio calculations. Phys. Chem. Chem. Phys. 2018, 20, 1889–1896. [Google Scholar] [CrossRef] [PubMed]
- Dyall, K.G.; Tecmer, P.; Sunaga, A. Diffuse Basis Functions for Relativistic s and d Block Gaussian Basis Sets. J. Chem. Theory Comput. 2023, 19, 198–210. [Google Scholar] [CrossRef] [PubMed]
- Bussery, B.; Achkar, Y.; Aubert-Frécon, M. Long-range molecular states dissociating to the three or four lowest asymptotes for the ten heteronuclear diatomic alkali molecules. Chem. Phys. 1987, 116, 319–338. [Google Scholar] [CrossRef]
- Quéméner, G.; Bohn, J.L.; Petrov, A.; Kotochigova, S. Universalities in ultracold reactions of alkali-metal polar molecules. Phys. Rev. A 2011, 84, 062703. [Google Scholar] [CrossRef]
- Byrd, J.N.; Montgomery, J.A.; Côté, R. Long-range forces between polar alkali-metal diatoms aligned by external electric fields. Phys. Rev. A 2012, 86, 032711. [Google Scholar] [CrossRef]
- Olaya, V.; Pérez-Ríos, J.; Herrera, F. C6 coefficients for interacting Rydberg atoms and alkali-metal dimers. Phys. Rev. A 2020, 101, 032705. [Google Scholar] [CrossRef]
- Gáspár, R.; Tamássy-Lentei, I. Molecular pseudopotential calculations III. Acta. Phys. Hung. 1976, 40, 283–291. [Google Scholar] [CrossRef]
- Igel-Mann, G.; Wedig, U.; Fuentealba, P.; Stoll, H. Ground-state properties of alkali dimers XY (X, Y=Li to Cs). J. Chem. Phys. 1986, 84, 5007–5012. [Google Scholar] [CrossRef]
- Korek, M.; Allouche, A.R.; Kobeissi, M.; Chaalan, A.; Dagher, M.; Fakherddin, K.; Aubert-Frécon, M. Theoretical study of the electronic structure of the LiRb and NaRb molecules. Chem. Phys. 2000, 256, 1–6. [Google Scholar] [CrossRef]
- Smirnov, A.D. Calculation of spectroscopic constants for the ground electronic states of CsK, CsLi, and RbLi molecules. J. Struct. Chem. 2007, 48, 21–27. [Google Scholar] [CrossRef]
- Korek, M.; Younes, G.; Al-Shawa, S. Theoretical calculation of the electronic structure of the molecule LiRb including the spin-orbit interaction. J. Mol. Struc. THEOCHEM 2009, 899, 25–31. [Google Scholar] [CrossRef]
- Dardouri, R.; Issa, K.; Oujia, B.; Gadéa, F.X. Theoretical study of the electronic structure of LiX and NaX (X = Rb, Cs) molecules: Electronic Structure of LiX and NaX Molecules. Int. J. Quantum Chem. 2012, 112, 2724–2734. [Google Scholar] [CrossRef]
- Dardouri, R.; Habli, H.; Oujia, B.; Gadéa, F.X. Ab Initio Diabatic energies and dipole moments of the electronic states of RbLi molecule. J. Comput. Chem. 2013, 34, 2091–2099. [Google Scholar] [CrossRef] [PubMed]
- Jendoubi, I.; Berriche, H.; Ben Ouada, H.; Gadéa, F.X. Radial coupling and adiabatic correction for the LiRb molecule. In Advances in the Theory of Quantum Systems in Chemistry and Physics; Hoggan, P., Brändas, E.J., Maruani, J., Piecuch, P., Delgado-Barrio, G., Eds.; Springer: Dordrecht, The Netherlands, 2012; pp. 405–430. [Google Scholar]
- Jendoubi, I.; Berriche, H.; Ben Ouada, H.; Gadéa, F.X. Structural and Spectroscopic Study of the LiRb Molecule beyond the Born-Oppenheimer Approximation. J. Phys. Chem. A 2012, 116, 2945–2960. [Google Scholar] [CrossRef]
- Bellayouni, S.; Jendoubi, I.; Mabrouk, N.; Berriche, H. Systematic Study of the Electronic Properties and Trends in the LiX (X=Na, K, Rb, Cs and Fr) Molecules. In Advances in Quantum Chemistry; Hoggan, P., Ed.; Academic Press: Cambridge, MA, USA, 2014; Volume 68, pp. 203–238. [Google Scholar]
- Fedorov, D.A.; Derevianko, A.; Varganov, S.A. Accurate potential energy, dipole moment curves, and lifetimes of vibrational states of heteronuclear alkali dimers. J. Chem. Phys. 2014, 140, 184315. [Google Scholar] [CrossRef]
- You, Y.; Yang, C.L.; Wang, M.S.; Ma, X.G.; Liu, W.W.; Wang, L.Z. Analytic functions for potential energy curves, dipole moments, and transition dipole moments of LiRb molecule. Spectrochim. Acta A 2016, 153, 488–495. [Google Scholar] [CrossRef]
- You, Y.; Yang, C.L.; Zhang, Q.Q.; Wang, M.S.; Ma, X.G.; Liu, W.W. Ab initio studies on the spin-forbidden cooling transitions of the LiRb molecule. Phys. Chem. Chem. Phys. 2016, 18, 19838–19846. [Google Scholar] [CrossRef]
- Kozlov, S.V.; Bormotova, E.A.; Medvedev, A.A.; Pazyuk, E.A.; Stolyarov, A.V.; Zaitsevskii, A. A first principles study of the spin-orbit coupling effect in LiM (M = Na, K, Rb, Cs) molecules. Phys. Chem. Chem. Phys. 2020, 22, 2295–2306. [Google Scholar] [CrossRef] [PubMed]
- Benichou, E.; Allouche, A.R.; Antoine, R.; Aubert-Frecon, M.; Bourgoin, M.; Broyer, M.; Dugourd, P.; Hadinger, G.; Rayane, D. Non perturbative approach for a polar and polarizable linear molecule in an inhomogeneous electric field: Application to molecular beam deviation experiments. Eur. Phys. J. D 2000, 10, 233–242. [Google Scholar] [CrossRef][Green Version]
- Ouerdane, H.; Jamieson, M.J. Scattering parameters for cold Li-Rb and Na-Rb collisions derived from variable phase theory. Phys. Rev. A 2004, 70, 022712. [Google Scholar] [CrossRef]
- Pérez-Ríos, J.; Dutta, S.; Chen, Y.P.; Greene, C.H. Quantum defect theory description of weakly bound levels and Feshbach resonances in LiRb. New J. Phys. 2015, 17, 045021. [Google Scholar] [CrossRef]
- Li, X.; Dutta, S. Extracting molecular potentials from incomplete spectroscopic information. Molecular Physics 2015, 113, 3854–3858. [Google Scholar] [CrossRef][Green Version]
- Lutz, J.J.; Hutson, J.M. Deviations from Born-Oppenheimer mass scaling in spectroscopy and ultracold molecular physics. J. Mol. Spectrosc. 2016, 33, 43–56. [Google Scholar] [CrossRef]
- Bormotova, E.A.; Kozlov, S.V.; Pazyuk, E.A.; Stolyarov, A.V.; Skomorowski, W.; Majewska, I.; Moszynski, R. Ab initio and analytical studies of the spin-orbit coupling in heteronuclear alkali-metal dimers AB (A, B = Li, Na, K, Rb) at long ranges. Phys. Rev. A 2019, 99, 012507. [Google Scholar] [CrossRef]
- Bormotova, E.A.; Stolyarov, A.V.; Skripnikov, L.V.; Titov, A.V. Ab initio study of R-dependent behavior of the hyperfine structure parameters for the (1)1,3Σ+ states of LiRb and LiCs. Chem. Phys. Lett. 2020, 760, 137998. [Google Scholar] [CrossRef]
- Bormotova, E.A.; Kozlov, S.V.; Pazyuk, E.A.; Stolyarov, A.V.; Majewska, I.; Moszynski, R. Theoretical study of the Coriolis effect in LiNa, LiK, and LiRb molecules. Phys. Chem. Chem. Phys. 2021, 23, 5187–5198. [Google Scholar] [CrossRef]
- Lamb, H.D.L.; McCann, J.F.; McLaughlin, B.M.; Goold, J.; Wells, N.; Lane, I. Structure and interactions of ultracold Yb ions and Rb atoms. Phys. Rev. A 2012, 86, 022716. [Google Scholar] [CrossRef]
- ElOualhazi, R.; Berriche, H. Electronic Structure and Spectra of the MgLi+ Ionic Molecule. J. Phys. Chem. A 2016, 120, 452–465. [Google Scholar] [CrossRef] [PubMed]
- Hou, X.-W.; Dong, S.-H.; Ma, Z.-Q. Algebraic Model Applied to Vibrations in the Electronic Ground State of NO2*. Commun. Theor. Phys. 1998, 30, 355–360. [Google Scholar]
- Hou, X.-W.; Xie, M.; Dong, S.-H.; Ma, Z.-Q. Overtone Spectra and Intensities of Tetrahedral Molecules in Boson-Realization Models. Ann. Phys. 1998, 263, 340–352. [Google Scholar] [CrossRef][Green Version]
- Frank, A.; Lemus, R.; Iachello, F. Algebraic model for molecular electronic spectra. In Symmetries in Science V; Gruber, B., Biedenharn, L.C., Doebner, H.D., Eds.; Plenum Press: New York, NY, USA, 1991; pp. 173–186. [Google Scholar]
- Lemus, R. Potential Energy Surfaces Using Algebraic Methods Based on Unitary Groups. Adv. Phys. Chem. 2011, 593872. [Google Scholar] [CrossRef]
- Stanton, J.F.; Bartlett, R.J. The equation of motion coupled-cluster method: A systematic biorthogonal approach to molecular excitation energies, transition probabilities, and excited state properties. J. Chem. Phys. 1993, 98, 7029–7039. [Google Scholar] [CrossRef]
- Kucharski, S.A.; Włoch, M.; Musial, M.; Bartlett, R.J. Coupled-cluster theory for excited electronic states: The full equation-of-motion coupled-cluster single, double, and triple excitation method. J. Chem. Phys. 2001, 115, 8263–8266. [Google Scholar] [CrossRef]
- Kowalski, K.; Piecuch, P. The active-space equation-of-motion coupled-cluster methods for excited electronic states: Full EOMCCSDt. J. Chem. Phys. 2001, 115, 643–651. [Google Scholar] [CrossRef]
- Hirata, S. Higher-order equation-of-motion coupled-cluster methods. J. Chem. Phys. 2004, 121, 51–59. [Google Scholar] [CrossRef]
- Krylov, A.I. Equation-of-Motion Coupled-Cluster Methods for Open-Shell and Electronically Excited Species: The Hitchhiker’s Guide to Fock Space. Annu Rev. Phys. Chem. 2008, 59, 433. [Google Scholar] [CrossRef]
- Bala, R.; Nataraj, H.S.; Abe, M.; Kajita, M. Accurate ab initio calculations of spectroscopic constants and properties of BeLi+. J. Mol. Spec. 2018, 349, 1–9. [Google Scholar] [CrossRef]
- Bala, R.; Nataraj, H.S.; Abe, M.; Kajita, M. Calculations of electronic properties and vibrational parameters of alkaline-earth lithides: MgLi+ and CaLi+. Mol. Phys. 2019, 117, 712–725. [Google Scholar] [CrossRef]
- Musial, M.; Bartlett, R.J. Charge-transfer separability and size-extensivity in the equation-of-motion coupled cluster method: EOM-CCx. J. Chem. Phys. 2011, 134, 034106. [Google Scholar] [CrossRef] [PubMed]
- Musial, M.; Kucharski, S.A. First principle calculations of the potential energy curves for electronic states of the lithium dimer. J. Chem. Theory Comput. 2014, 10, 1200. [Google Scholar] [CrossRef] [PubMed]
- Musial, M.; Bewicz, A.; Kucharski, S.A. Potential energy curves for electronic states of the sodium dimer with multireference coupled cluster calculations. Mol. Phys. 2023, 121, 1–13. [Google Scholar] [CrossRef]
- Musial, M.; Kucharski, S.A.; Bewicz, A.; Skupin, P.; Tomanek, M. Electronic states of NaLi molecule: Benchmark results with Fock space coupled cluster approach. J. Chem. Phys. 2021, 154, 054109. [Google Scholar] [CrossRef]
- Tomza, M.; Goerz, M.H.; Musial, M.; Moszynski, R.; Koch, C.P. Optimized production of ultracold ground-state molecules: Stabilization employing potentials with ion-pair character and strong spin-orbit coupling. Phys. Rev. A 2012, 86, 043424. [Google Scholar] [CrossRef]
- Salomonsen, S.; Lindgren, I. Martensson, A.-M. Numerical Many-Body Perturbation Calculations on Be-like Systems Using a Multi-Configurational Model Space. Phys. Scr. 1980, 21, 351–355. [Google Scholar] [CrossRef]
- Kaldor, U. Intruder states and incomplete model spaces in multireference coupled-cluster theory: The 2p2 states of Be. Phys. Rev. A 1988, 38, 6013–6016. [Google Scholar] [CrossRef]
- Nakajima, T.; Hirao, K. The higher-order Douglas-Kroll transformation. J. Chem. Phys. 2000, 113, 7786–7789. [Google Scholar] [CrossRef]
- Stanton, J.F.; Gauss, J.; Watts, J.D.; Nooijen, M.; Oliphant, N.; Perera, S.A.; Szalay, P.G.; Lauderdale, W.J.; Kucharski, S.A.; Gwaltney, S.R.; et al. Integral Packages Included Are VMOL (Almlof, J.; Taylor, P.); VPROPS (Taylor, P.R.); A Modified Version of ABACUS Integral Derivative Package (Helgaker, T.U.; Jensen, J.J.A.; Olsen, J.; Joergensen, P.; Taylor, P.R.); ACES II Program is a Product of the Quantum Theory Project; University of Florida: Gainesville, FL, USA, 2005. [Google Scholar]
- Barca, G.M.J.; Bertoni, C.; Carrington, L.; Datta, D.; DeSilva, N.; Deustua, J.E.; Fedorov, D.G.; Gour, J.R.; Gunina, A.O.; Guidez, E.; et al. Recent developments in the general atomic and molecular electronic structure system. J. Chem. Phys. 2020, 152, 154102. [Google Scholar] [CrossRef]
- LeRoy, R.J. LEVEL: A computer program for solving the radial Schrödinger equation for bound and quasibound levels. J. Quant. Spectrosc. Ra. 2017, 186, 167–178. [Google Scholar] [CrossRef]
- Roos, B.O.; Veryazov, V.; Widmark, P.O. Relativistic atomic natural orbital type basis sets for the alkaline and alkaline-earth atoms applied to the ground-state potentials for the corresponding dimers. Theor. Chem. Acc. 2004, 111, 345–351. [Google Scholar] [CrossRef]
- Noro, T.; Sekiya, M.; Koga, T. Segmented contracted basis sets for atoms H through Xe: Sapporo-(DK)-nZP sets (n=D, T, Q). Theor. Chem. Acc. 2012, 131, 1124. [Google Scholar] [CrossRef]
- Skupin, P.; Musial, M.; Kucharski, S.A. Potential Energy Curves for the Low-Lying Electronic States of K2+ from ab Initio Calculations with All Electrons Correlated. J. Phys. Chem. A 2017, 121, 1480–1486. [Google Scholar] [CrossRef] [PubMed]
- Kurtz, H.A.; Stewart, J.J.P.; Dieter, K.M. Calculation of the nonlinear optical properties of molecules. J. Comput. Chem. 1990, 11, 82–87. [Google Scholar] [CrossRef]
- Galván, I.F.; Vacher, M.; Alavi, A.; Angeli, C.; Aquilante, F.; Autschbach, J.; Bao, J.J.; Bokarev, S.I.; Bogdanov, N.A.; Carlson, R.K.; et al. OpenMolcas: From Source Code to Insight. J. Chem. Theory Comput. 2019, 15, 5925–5964. [Google Scholar] [CrossRef] [PubMed]
- Tarnovsky, V.; Bunimovicz, M.; Vušković, L.; Stumpf, B.; Bederson, B. Measurements of the dc electric dipole polarizabilities of the alkali dimer molecules, homonuclear and heteronuclear. J. Chem. Phys. 1993, 98, 3894–3904. [Google Scholar] [CrossRef]
- Marzok, C.; Deh, B.; Zimmermann, C.; Courteille, W.; Tiemann, E.; Vanne, Y.V.; Saenz, A. Feshbach resonances in an ultracold 7Li and 87Rb mixture. Phys. Rev. A 2009, 79, 012717. [Google Scholar] [CrossRef]
- Čížek, J. On the Correlation Problem in Atomic and Molecular Systems. Calculation of Wavefunction Components in Ursell-Type Expansion Using Quantum-Field Theoretical Methods. J. Chem. Phys. 1966, 45, 4256–4266. [Google Scholar] [CrossRef]
- Bartlett, R.J.; Musial, M. Coupled-Cluster theory in Quantum Chemistry. Rev. Mod. Phys. 2007, 79, 291–352. [Google Scholar] [CrossRef]
- Jeziorski, B.; Monkhorst, H.J. Coupled-cluster method for multideterminantal reference states Phys. Rev. A 1981, 24, 1668–1681. [Google Scholar] [CrossRef]
- Jeziorski, B.; Paldus, J. Spin-adapted multireference coupled-cluster approach: Linear approximation for two closed-shell-type reference configurations. J. Chem. Phys. 1988, 88, 5673–5687. [Google Scholar] [CrossRef]
- Meissner, L.; Jankowski, K.; Wasilewski, J.A. A coupled-cluster method for quasidegenerate states. Int. J. Quantum Chem. 1988, 34, 535–557. [Google Scholar] [CrossRef]
- Berkovic, S.; Kaldor, U. Hilbert space coupled-cluster method in an incomplete model space. Chem. Phys. Lett. 1992, 199, 42–46. [Google Scholar] [CrossRef]
- Mukherjee, D.; Moitra, R.K.; Mukhopadhay, A. Applications of a non-perturbative many-body formalism to general open-shell atomic and molecular problems: Calculation of the ground and the lowest π-π* singlet and triplet energies and the first ionization potential of trans-butadiene. Mol. Phys. 1977, 33, 955–969. [Google Scholar] [CrossRef]
- Mukherjee, D. On the hierarchy equations of the wave-operator for open-shell systems. Pramana 1979, 12, 203–225. [Google Scholar] [CrossRef]
- Mukhopadhay, A.; Moitra, R.K.; Mukherjee, D. A non-perturbative open-shell theory for ionisation potential and excitation energies using HF ground state as the vacuum. J. Phys. B 1979, 12, 1–18. [Google Scholar] [CrossRef]
- Lindgren, I. A coupled-cluster approach to the many-body perturbation theory for open-shell systems. Int. J. Quantum Chem. Symp. 1978, 12, 33–58. [Google Scholar] [CrossRef]
- Stolarczyk, L.Z.; Monkhorst, H.J. Coupled-cluster method in Fock space. I. General formalism. Phys. Rev. A 1985, 32, 725–742. [Google Scholar] [CrossRef]
- Jeziorski, B.; Paldus, J. Valence universal exponential ansatz and the cluster structure of multireference configuration interaction wave function. J. Chem. Phys. 1989, 90, 2714–2731. [Google Scholar] [CrossRef]
- Mukherjee, D.; Pal, S. Use of cluster-expansion methods in the open-shell correlation-problem. Adv. Quantum Chem. 1989, 20, 291–373. [Google Scholar]
- Haque, M.A.; Kaldor, U. Open-shell coupled-cluster theory applied to atomic and molecular systems. Chem. Phys. Lett. 1985, 17, 347–351. [Google Scholar] [CrossRef]
- Meissner, L. A Fock-space coupled-cluster method fully utilizing valence universal strategy. J. Chem. Phys. 1995, 103, 8014–8021. [Google Scholar] [CrossRef]
- Meissner, L.; Bartlett, R.J. A Dressing for the matrix elements of the singles and doubles equation-of-motion coupled-cluster method that recovers additive separability of excitation energies. J. Chem. Phys. 1995, 102, 7490–7498. [Google Scholar] [CrossRef]
- Meissner, L. Fock-space coupled-cluster method in the intermediate Hamiltonian formulation: Model with singles and doubles. J. Chem. Phys. 1998, 108, 9227–9235. [Google Scholar] [CrossRef]
- Musial, M.; Bartlett, R.J. Multireference Fock-space coupled-cluster and Equation-of-Motion coupled-cluster theories: The detailed interconnections. J. Chem. Phys. 2008, 129, 134105. [Google Scholar] [CrossRef] [PubMed]
- Lyakh, D.I.; Musial, M.; Lotrich, V.; Bartlett, R.J. Multireference nature of chemistry: The coupled-cluster view. Chem. Rev. 2012, 112, 182–243. [Google Scholar] [CrossRef]
- Oleynichenko, A.V.; Zaitsevskii, A.; Skripnikov, L.V.; Eliav, E. Relativistic Fock Space Coupled Cluster Method for Many-Electron Systems: Non-Perturbative Account for Connected Triple Excitations. Symmetry 2020, 12, 1101. [Google Scholar] [CrossRef]
- Nooijen, M.; Bartlett, R.J. Equation of motion coupled cluster method for electron attachment. J. Chem. Phys. 1995, 102, 3629–3647. [Google Scholar] [CrossRef]
- Musial, M.; Bartlett, R.J. Equation-of-motion coupled cluster method with full inclusion of the connected triple excitations for electron attached states: EA-EOM-CCSDT. J. Chem. Phys. 2003, 119, 1901–1908. [Google Scholar] [CrossRef]
- Kamiya, M.; Hirata, S. Higher-order equation-of-motion coupled-cluster methods for electron attachment. J. Chem. Phys. 2007, 126, 134112. [Google Scholar] [CrossRef]
- Gour, J.R.; Piecuch, P.; Włoch, M. Active-Space Equation-of-Motion Coupled-Cluster Methods for Excited States of Radicals and Other Open-Shell Systems: EA-EOMCCSDt and IP-EOMCCSDt. J. Chem. Phys. 2005, 123, 134113. [Google Scholar] [CrossRef]



| Dissociation Limit | Li | Rb | Li + Rb | LiRb R = ∞ | ||
|---|---|---|---|---|---|---|
| Config. | E (a.u.) | Config. | E (a.u.) | E (a.u.) | E (a.u.) | |
| Li(2s) + Rb(5s) | [He] 2s | −7.473553 | [Kr] 5s | −2939.440615 | −2946.914167 | −2946.914167 |
| Li(2s) + Rb(5p) | [He] 2s | −7.473553 | [Kr] 5p | −2939.385063 | −2946.858616 | −2946.858616 |
| Li(2p) + Rb(5s) | [He] 2p | −7.405597 | [Kr] 5s | −2939.440615 | −2946.846212 | −2946.846212 |
| Li(2s) + Rb(4d) | [He] 2s | −7.473553 | [Kr] 4d | −2939.355029 | −2946.828582 | −2946.828582 |
| Li(2s) + Rb(6s) | [He] 2s | −7.473553 | [Kr] 6s | −2939.350976 | −2946.824529 | −2946.824529 |
| Li(2s) + Rb(6p) | [He] 2s | −7.473553 | [Kr] 6p | −2939.334975 | −2946.808528 | −2946.808528 |
| Sym. | (Debye) | Method |
|---|---|---|
| 4.01 (−0.38) | This work | |
| 4.05 | Exp. [81] | |
| 4.1 | Exp. [21] | |
| 4.84 | CCSD [22] | |
| 4.42 | MBPT4 [22] | |
| 4.66 | CCSD(T) [22] | |
| 4.34 | CCSD(T) + rel. [22] | |
| 4.14 | CCSD(T) [28] | |
| 3.99 | CCSD(T) [1] | |
| 4.046 | CCSDT [27] | |
| 4.06 | CCSDT [40] | |
| 4.58 | MRCI [41] | |
| 4.168 | Pseudopotential/basis A [7] | |
| 4.142 | Pseudopotential/basis B [7] | |
| 4.13 | Pseudopotential [31] | |
| 4.78 | Pseudopotential [39] | |
| 4.46 | Pseudopotential [34] | |
| 4.165 | Pseudopotential [23] | |
| 4.78 | Pseudopotential [38] | |
| 4.40 | DFT/PW91 [44] |
| State | Position (Å) | Energy (a.u.) | Energy (cm) |
|---|---|---|---|
| Min. | 4.18 | −2946.834640 | |
| Max. | 4.75 | −2946.833272 | |
| Diff. | 0.001368 | 300 | |
| Min. | 4.02 | −2946.839545 | |
| Max. | 8.05 | −2946.828999 | |
| Diff. | 0.010546 | 2314 | |
| Min. | 4.08 | −2946.827538 | |
| Max. | 6.20 | −2946.817448 | |
| Diff. | 0.010090 | 2214 | |
| Min. | 4.40 | −2946.824168 | |
| Max. | 8.00 | −2946.810238 | |
| Diff. | 0.013930 | 3057 |
| State | Position in This Work (Å) | Position in [38] (Å) |
|---|---|---|
| / | 8.61 | N/A |
| / | 13.21 | 13.12 |
| / | 14.24 | N/A |
| / | 4.75 | 4.71 |
| / | 3.26 | 3.12 |
| 3.13 | 2.94 | |
| / | 3.88 | 3.84 |
| 7.96 | 8.07 |
| Sym. | De | Te | Re | e | exe | Be | Source |
|---|---|---|---|---|---|---|---|
| Li(2s) + Rb(5s) | |||||||
| 5886(−43) | 3.466(−0.034) | 194.53(2.60) | 1.21(0.16) | 0.216(0.004) | This work | ||
| 5968 | 3.428 | 196.02 | 1.44 | 0.223 | [38] | ||
| 5917.0 | 3.490 | 195.3 | 1.31 | 0.216 | [41] | ||
| 5922.5 | 3.508 | 194.0 | 1.240 | 0.213 | [42] | ||
| 5921 | 3.466 | 195.18 | Exp. [15] | ||||
| 290(−15) | 5592(−32) | 4.993(0.014) | 43.11(−0.93) | 1.84(0.00) | 0.104(−0.001) | This work | |
| 276 | 5693 | 5.126 | 40.13 | 17.46 | 0.098 | [38] | |
| 282.4 | 5.141 | 39.1 | 0.85 | 0.096 | [41] | ||
| 277.2 | 5650.5 | 5.140 | 40.548 | Exp. [15] | |||
| Li(2s) + Rb(5s) | |||||||
| 7003(170) | 11,594(305) | 4.166(−0.034) | 120.03(2.70) | 0.54(0.04) | 0.150(0.002) | This work | |
| 7053 | 11,654 | 4.137 | 118.78 | 1.04 | 0.153 | [38] | |
| 7039.6 | 4.201 | 116.5 | 0.56 | 0.147 | [41] | ||
| 11,614 | 4.16 | [43] e | |||||
| 117.3 | 0.36 | Exp. [19] | |||||
| 1529(70) | 17,069(407) | 3.905(−0.057) | 117.44(4.78) | 3.05(0.23) | 0.171(0.005) | This work | |
| 1461 | 17,245 | 3.873 | 116.12 | 2.92 | 0.175 | [38] | |
| 1415.9 | 17,578.4 | 3.969 | 113.8 | 2.750 | 0.1651 | [42] | |
| 1634 | 17,110.406 | 3.8751 | 122.2 | Exp. [16] | |||
| 3940(152) | 14,657(323) | 4.107(−0.020) | 133.64(0.83) | 1.42(−0.04) | 0.154(0.001) | This work | |
| 3969 | 14,737 | 4.058 | 128.63 | 1.09 | 0.159 | [38] | |
| 3997.2 | 4.133 | 128.7 | 0.97 | 0.152 | [41] | ||
| 8359(202) | 10,237(273) | 3.382(−0.016) | 190.19(1.87) | 0.74(0.08) | 0.227(0.002) | This work | |
| 8457 | 10,249 | 3.338 | 192.0 | 0.860 | 0.235 | [38] | |
| 10,232 | 3.44 | [43] e | |||||
| Li(2s) + Rb(5s) | |||||||
| 3588(−155) | 17,212(111) | 4.228(0.021) | 115.16(−3.35) | 0.66(−0.05) | 0.146(−0.001) | This work | |
| 3494 | 17,382 | 4.243 | 114.24 | 1.22 | 0.145 | [38] | |
| 3601 | 17,230.571 | 4.2834 | 113.8 | Exp. [16] | |||
| 115.4 | 0.36 | Exp. [19] | |||||
| 1774(−145) | 19,026(101) | 4.091(0.017) | 122.53(−2.10) | 1.26(0.02) | 0.156(−0.001) | This work | |
| 1639 | 19,235 | 4.084 | 122.08 | 1.83 | 0.157 | [38] | |
| 1743 | 19,089.88 | 4.115 | 120.5 | Exp. [16] | |||
| 409(−506) | 20,390(462) | 3.932(−0.022) | 136.51(1.87) | 1.68(0.09) | 0.168(0.002) | This work | |
| 362 | 20,513 | 3.904 | 136.61 | 1.82 | 0.171 | [38] | |
| 1583(−476) | 19,217(432) | 4.128(−0.016) | 104.46(−0.63) | 1.61(0.23) | 0.152(0.001) | This work | |
| 1411 | 19,484 | 4.100 | 103.3 | 1.82 | 0.156 | [38] | |
| 195.1 | 0.84 | Exp. [19] | |||||
| Li(2s) + Rb(5s) | |||||||
| 4090(200) | 21,255(431) | 7.635(0.380) | 33.92(−1.00) | 1.16(0.36) | 0.045(−0.004) | This work | |
| 3998 | 21,326 | 7.671 | 41.27 | 0.10 | 0.044 | [38] | |
| 1190(66) | 24,155(566) | 4.573(−0.060) | 82.82(2.90) | 1.28(0.05) | 0.124(0.003) | This work | |
| 989 | 24,335 | 4.570 | 81 | 1.34 | 0.125 | [38] | |
| 3209(156) | 22,135(475) | 3.709(−0.022) | 142.39(2.26) | 1.08(−0.04) | 0.189(0.002) | This work | |
| 3063 | 22,277 | 3.714 | 143.2 | 1.6 | 0.19 | [38] | |
| 1653(324) | 23,692(308) | 4.165(−0.016) | 134.98(7.92) | 8.91(2.78) | 0.150(0.001) | This work | |
| 1st min. | 1527 | 23,797 | 4.137 | 117.85 | 1.74 | 0.153 | [38] |
| 2130(331) | 23,215(301) | 5.457(−0.193) | 109.29(16.09) | 2.81(1.06) | 0.087(0.006) | This work | |
| 2nd min. | 1983 | 23,340 | 5.433 | 109.58 | 1.74 | 0.088 | [38] |
| 106.3 | 2.4 | Exp. [18] | |||||
| 2172(361) | 23,174(272) | 5.651(−0.205) | 70.32(4.62) | 0.09(−0.03) | 0.081(0.005) | This work | |
| 2055 | 23,269 | 5.661 | 74.29 | 1.09 | 0.081 | [38] | |
| 2413(145) | 22,932(487) | 3.932(−0.031) | 135.37(3.51) | 1.41(−0.04) | 0.168(0.002) | This work | |
| 2294 | 23,046 | 3.878 | 133.5 | 1.67 | 0.174 | [38] | |
| Li(2s) + Rb(5s) | |||||||
| 3382(86) | 22,583(276) | 4.006(−0.018) | 201.98(14.73) | 4.86(0.48) | 0.162(0.001) | This work | |
| 1st min. | 3360 | 22,702 | 3.962 | 211.81 | 7.07 | 0.167 | [38] |
| 480(−237) | 25,483(598) | 11.524(0.160) | 12.65(−4.37) | 0.70(0.24) | 0.019(−0.001) | This work | |
| 2nd min. | 598 | 25,473 | 11.650 | 13.10 | 7.07 | 0.019 | [38] |
| 1935(46) | 24,028(315) | 4.724(−0.057) | 210.11(−9.16) | 12.40(−1.35) | 0.117(0.003) | This work | |
| 1964 | 24,106 | 4.740 | 215.11 | 43.55 | 0.116 | [38] | |
| Li(2s) + Rb(5s) | |||||||
| 4471(184) | 25,130(302) | 4.055(−0.022) | 132.59(1.01) | 0.79(−0.07) | 0.158(0.001) | This work | |
| 1st min. | 4438 | 25,330 | 4.021 | 132.28 | 0.86 | 0.162 | [38] |
| 3420(92) | 26,179(392) | 12.847(−0.128) | 21.61(2.30) | −0.04(0.05) | 0.016(0.001) | This work | |
| 2nd min. | 3502 | 26,266 | 12.666 | 22.38 | 0.86 | 0.016 | [38] |
| 3902(136) | 25,697(348) | 3.968(−0.030) | 129.77(1.99) | 1.21(−0.02) | 0.165(0.002) | This work | |
| 3918 | 25,850 | 3.936 | 127.0 | 1.52 | 0.169 | [38] | |
| 3514(81) | 26,085(403) | 4.378(−0.032) | 169.63(0.59) | 3.14(−0.15) | 0.136(0.002) | This work | |
| 1st min. | 3598 | 26,170 | 4.343 | 175.31 | 6.02 | 0.139 | [38] |
| 420(−11) | 29,179(496) | 9.264(0.054) | 22.51(−0.04) | 0.028(−0.01) | 0.030(−0.001) | This work | |
| 2nd min. | 444 | 29,324 | 9.560 | 19.35 | 6.02 | 0.028 | [38] |
| 4437(129) | 25,162(355) | 3.953(−0.038) | 182.95(−2.55) | 3.75(−0.73) | 0.166(0.003) | This work | |
| 4483 | 25,285 | 3.941 | 198.74 | 14.44 | 0.168 | [38] | |
| MAE | 39 | 46 | 0.051 | 13.46 | 0.42 | − | This work |
| 86 | 118 | 0.025 | 13.24 | 0.80 | − | [38] | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Skrzyński, G.; Musial, M. Benchmark Study of the Electronic States of the LiRb Molecule: Ab Initio Calculations with the Fock Space Coupled Cluster Approach. Molecules 2023, 28, 7645. https://doi.org/10.3390/molecules28227645
Skrzyński G, Musial M. Benchmark Study of the Electronic States of the LiRb Molecule: Ab Initio Calculations with the Fock Space Coupled Cluster Approach. Molecules. 2023; 28(22):7645. https://doi.org/10.3390/molecules28227645
Chicago/Turabian StyleSkrzyński, Grzegorz, and Monika Musial. 2023. "Benchmark Study of the Electronic States of the LiRb Molecule: Ab Initio Calculations with the Fock Space Coupled Cluster Approach" Molecules 28, no. 22: 7645. https://doi.org/10.3390/molecules28227645
APA StyleSkrzyński, G., & Musial, M. (2023). Benchmark Study of the Electronic States of the LiRb Molecule: Ab Initio Calculations with the Fock Space Coupled Cluster Approach. Molecules, 28(22), 7645. https://doi.org/10.3390/molecules28227645






