ISIDORE, a Probe for In Situ Trace Metal Speciation Based on the Donnan Membrane Technique and Electrochemical Detection Part 2: Cd and Pb Measurements during the Accumulation Time of the Donnan Membrane Technique
Abstract
1. Introduction
2. Donnan Membrane Technique (DMT)
3. Results and Discussion
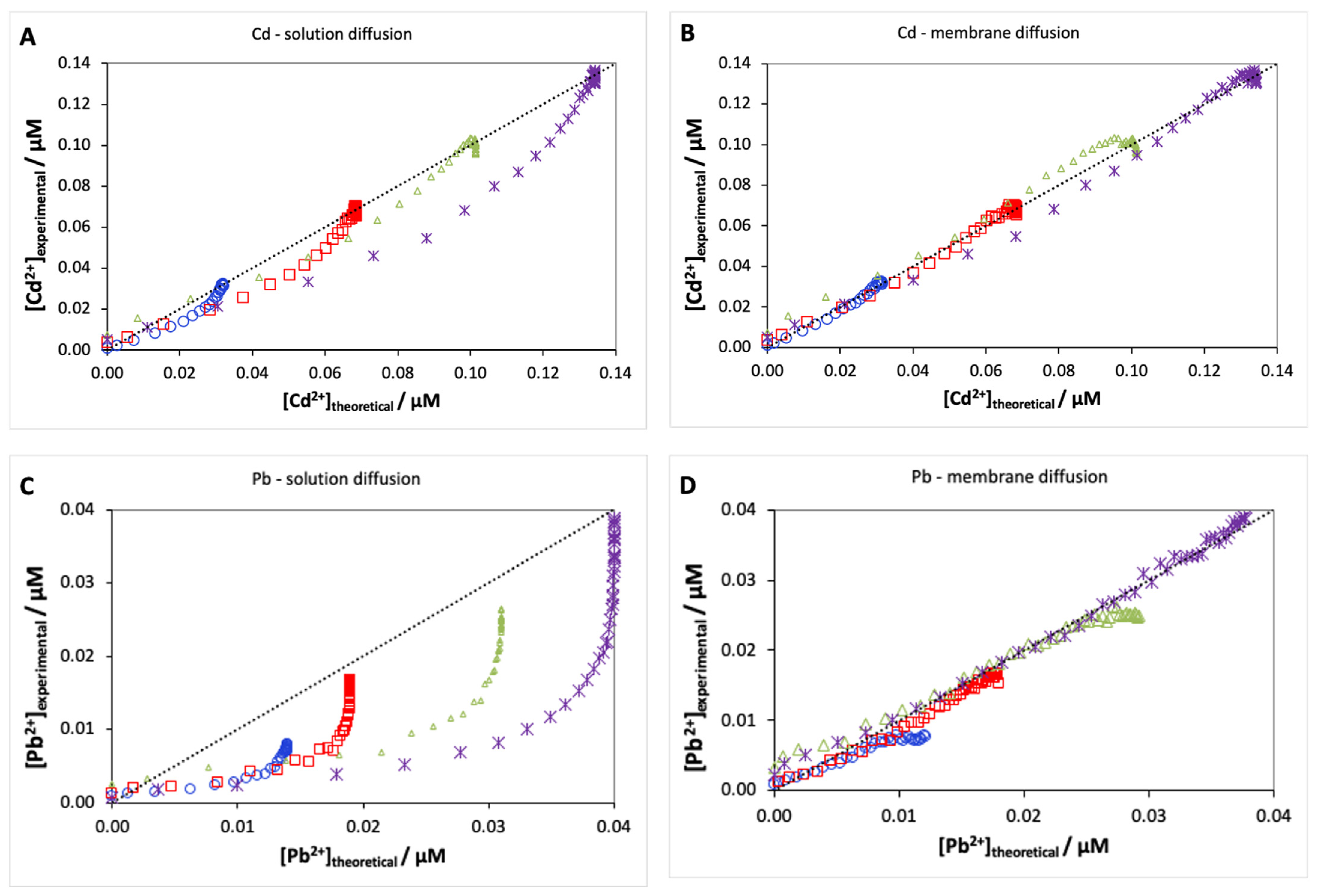
3.1. Non-Equilibrium ISIDORE Probe Calibration
3.2. Behaviour of Cd and Pb in the Membrane
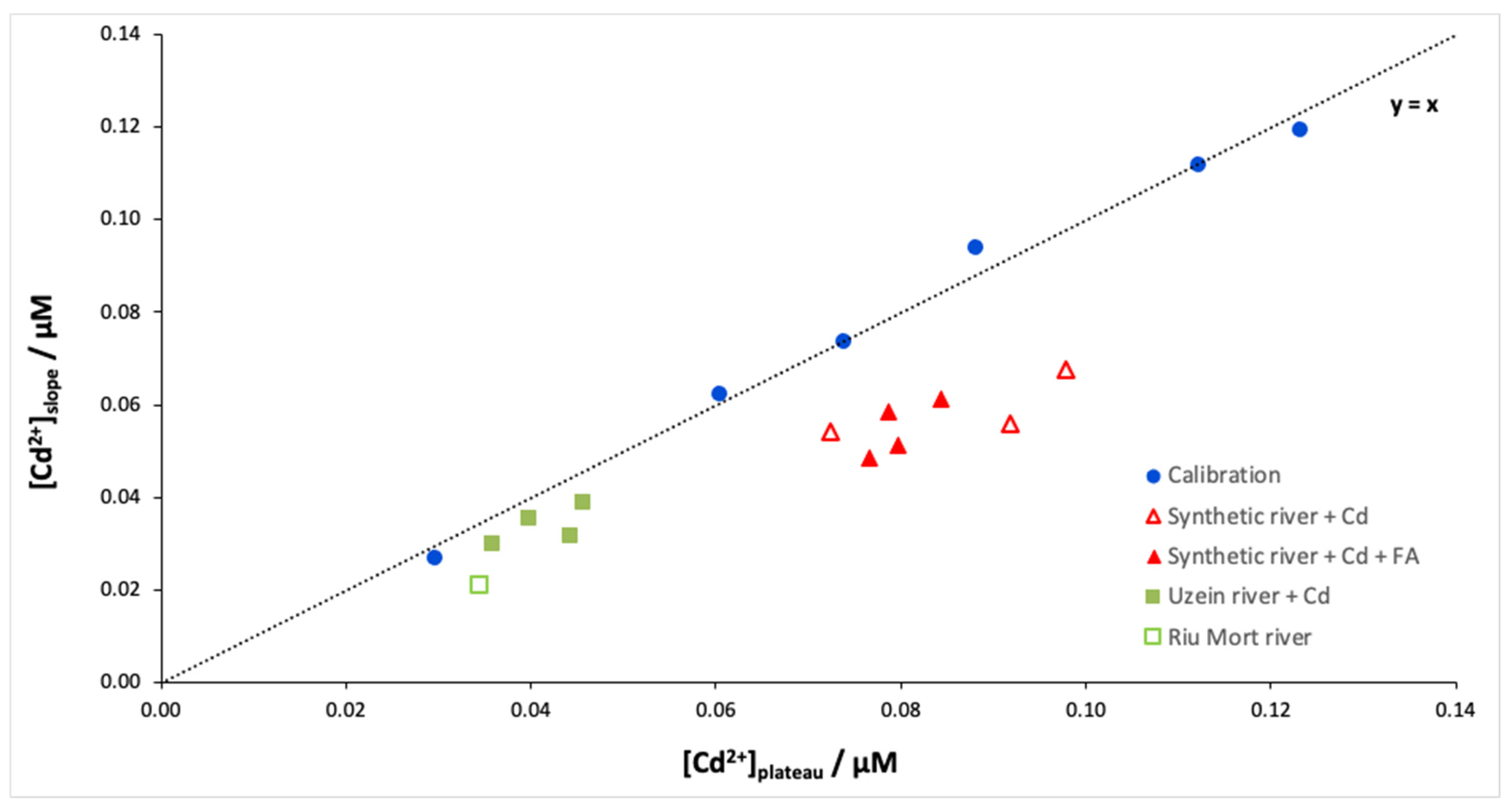
3.3. Analysis of Synthetic and Natural Samples
4. Materials and Methods
4.1. Reagents
4.2. Equipment
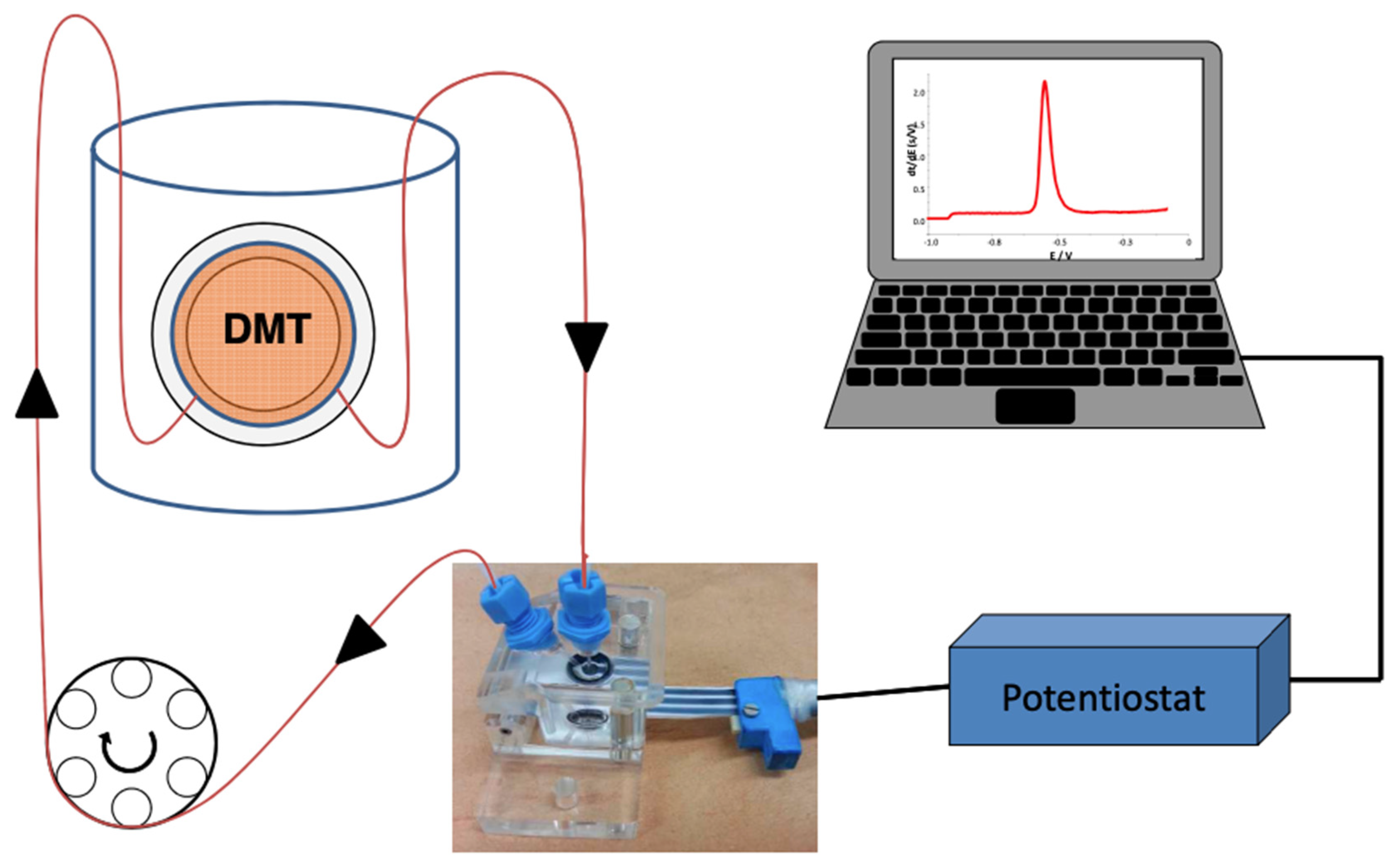
4.3. ISIDORE Probe
4.4. Natural Samples
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Buffle, J. Complexation Reactions in Aquatic Systems: An Analytical Approach; Ellis Horwood Limited: Chichester, UK, 1988; p. 692. [Google Scholar]
- Davison, W.; Zhang, H. In situ speciation measurements of trace components in natural waters using thin-film gels. Nature 1994, 367, 546–548. [Google Scholar] [CrossRef]
- Tomaszewski, L.; Buffle, J.; Galceran, J. Theoretical and Analytical Characterization of a Flow-Through Permeation Liquid Membrane with Controlled Flux for Metal Speciation Measurements. Anal. Chem. 2003, 75, 883–900. [Google Scholar] [CrossRef] [PubMed]
- Temminghoff, E.J.M.; Plette, A.C.C.; Van Eck, R.; Van Riemsdijk, W.H. Determination of the chemical speciation of trace metals in aqueous systems by the Wageningen Donnan Membrane Technique. Anal. Chim. Acta 2000, 417, 149–157. [Google Scholar] [CrossRef]
- Belmont-Hébert, C.; Tercier, M.-L.; Buffle, J.; Fiaccabrino, G.C.; de Rooij, N.F.; Koudelka-Hep, M. Gel-integrated microelectrode arrays for direct voltammetric measurements of heavy metals in natural waters and other complex media. Anal. Chem. 1998, 70, 2949–2956. [Google Scholar] [CrossRef]
- Tercier-Waeber, M.L.; Buffle, J. Submersible Online Oxygen Removal System Coupled to an in Situ Voltammetric Probe for Trace Element Monitoring in Freshwater. Environ. Sci. Technol. 2000, 34, 4018–4024. [Google Scholar] [CrossRef]
- Salaun, P.; Buffle, J. Integrated microanalytical system coupling permeation liquid membrane and voltammetry for trace metal speciation. Theory and application. Anal. Chem. 2004, 76, 31–39. [Google Scholar] [CrossRef]
- Han, H.; Pan, D. Voltammetric methods for speciation analysis of trace metals in natural waters. Trends Environ. Anal. Chem. 2021, 29, e00119. [Google Scholar] [CrossRef]
- Galceran, J.; Huidobro, C.; Companys, E.; Alberti, G. AGNES: A technique for determining the concentration of free metal ions. The case of Zn(II) in coastal Mediterranean seawater. Talanta 2007, 71, 1795–1803. [Google Scholar] [CrossRef]
- Chito, D.; Weng, L.; Galceran, J.; Companys, E.; Puy, J.; Van Riemsdijk, W.H.; Van Leeuwen, H.P. Determination of free Zn2+ concentration in synthetic and natural samples with AGNES (Absence of Gradients and Nernstian Equilibrium Stripping) and DMT (Donnan Membrane Technique). Sci. Total Environ. 2012, 421, 238–244. [Google Scholar] [CrossRef]
- Parat, C.; Authier, L.; Castetbon, A.; Aguilar, D.; Companys, E.; Puy, J.; Galceran, J.; Potin-Gautier, M. Free Zn2+ determination in natural freshwater of the Pyrenees: Towards on-site measurements with AGNES. Environ. Chem. 2015, 12, 329–337. [Google Scholar] [CrossRef]
- van Leeuwen, H.P.; Town, R.M. Kinetic Limitations in Measuring Stabilities of Metal Complexes by Competitive Ligand Exchange-Adsorptive Stripping Voltammetry (CLE-AdSV). Environ. Sci. Technol. 2005, 39, 7217–7225. [Google Scholar] [CrossRef] [PubMed]
- Mongin, S.; Uribe, R.; Puy, J.; Cecilia, J.; Galceran, J.; Zhang, H.; Davison, W. Key Role of the Resin Layer Thickness in the Lability of Complexes Measured by DGT. Environ. Sci. Technol. 2011, 45, 4869–4875. [Google Scholar] [CrossRef] [PubMed]
- Puy, J.; Galceran, J.; Rey-Castro, C. Interpreting the DGT Measurement. In Diffusive Gradients in Thin-Films for Environmental Measurements; Cambridge University Press: Cambridge, UK, 2016; pp. 93–122. [Google Scholar]
- Gao, L.; Gao, B.; Xu, D.; Liu, L. DGT: A promising technology for in-situ measurement of metal speciation in the environment. Sci. Total Environ. 2020, 715, 136810. [Google Scholar] [CrossRef] [PubMed]
- Pommier, A.L.; Buzier, R.; Simon, S.; Guibaud, G. Impact of low ionic strength on DGT sampling with standard APA gels: Effect of pH and analyte. Talanta 2021, 222, 121413. [Google Scholar] [CrossRef]
- van der Veeken, P.L.R.; Pinheiro, J.P.; van Leeuwen, H.P. Metal Speciation by DGT/DET in Colloidal Complex Systems. Environ. Sci. Technol. 2008, 42, 8835–8840. [Google Scholar] [CrossRef]
- Domingos, R.F.; Benedetti, M.F.; Pinheiro, J.P. Application of Permeation Liquid Membrane (PLM) and Scanned Stripping Chronopotentiometry (SSCP) to metal speciation analysis of colloidal complexes. Anal. Chim. Acta 2007, 589, 261–268. [Google Scholar] [CrossRef]
- Kalis, E.J.J.; Weng, L.; Dousma, F.; Temminghoff, E.J.M.; Van Riemsdijk, W.H. Measuring Free Metal Ion Concentrations in Situ in Natural Waters Using the Donnan Membrane Technique. Environ. Sci. Technol. 2006, 40, 955–961. [Google Scholar] [CrossRef] [PubMed]
- Nolan, A.L.; McLaughlin, M.J.; Mason, S.D. Chemical Speciation of Zn, Cd, Cu, and Pb in Pore Waters of Agricultural and Contaminated Soils Using Donnan Dialysis. Environ. Sci. Technol. 2003, 37, 90–98. [Google Scholar] [CrossRef] [PubMed]
- Pan, Y.; Koopmans, G.F.; Bonten, L.T.C.; Song, J.; Luo, Y.; Temminghoff, E.J.M.; Comans, R.N.J. In-situ measurement of free trace metal concentrations in a flooded paddy soil using the Donnan Membrane Technique. Geoderma 2015, 241–242, 59–67. [Google Scholar] [CrossRef]
- Ren, Z.-L.; Tella, M.; Bravin, M.N.; Comans, R.N.J.; Dai, J.; Garnier, J.-M.; Sivry, Y.; Doelsch, E.; Straathof, A.; Benedetti, M.F. Effect of dissolved organic matter composition on metal speciation in soil solutions. Chem. Geol. 2015, 398, 61–69. [Google Scholar] [CrossRef]
- Vega, F.A.; Weng, L. Speciation of heavy metals in River Rhine. Water Res. 2013, 47, 363–372. [Google Scholar] [CrossRef]
- Weng, L.; Temminghoff, E.J.M.; Van Riemsdijk, W.H. Aluminum speciation in natural waters: Measurement using Donnan membrane technique and modeling using NICA-Donnan. Water Res. 2002, 36, 4215–4226. [Google Scholar] [CrossRef] [PubMed]
- Janot, N.; Groenenberg, J.E.; Otero-Fariña, A.; Pinheiro, J.P. Free Eu(III) Determination by Donnan Membrane Technique with Electrochemical Detection: Implementation and Evaluation. Aquat. Geochem. 2021, 27, 127–140. [Google Scholar] [CrossRef]
- Asante-Sackey, D.; Rathilal, S.; Kweinor Tetteh, E.; Ezugbe, E.O.; Pillay, L.V. Donnan Membrane Process for the Selective Recovery and Removal of Target Metal Ions-A Mini Review. Membranes 2021, 11, 358. [Google Scholar] [CrossRef]
- Parat, C.; Pinheiro, J.P. ISIDORE, a probe for in situ trace metal speciation based on Donnan membrane technique with related electrochemical detection part 1: Equilibrium measurements. Anal. Chim. Acta 2015, 896, 1–10. [Google Scholar] [CrossRef]
- Liberti, I.; Helfferich, F.G. Mass Transfer and Kinetics of Ion Exchange; Liberti, I., Helfferich, F.G., Eds.; Springer: Dordrecht, The Netherlands, 1983; p. 459. [Google Scholar]
- Marang, L.; Reiller, P.; Pepe, M.; Benedetti, M.F. Donnan membrane approach: From equilibrium to dynamic speciation. Environ. Sci. Technol. 2006, 40, 5496–5501. [Google Scholar] [CrossRef] [PubMed]
- Weng, L.; Van Riemsdijk, W.H.; Temminghoff, E.J.M. Kinetic aspects of Donnan membrane technique for measuring free trace cation concentration. Anal. Chem. 2005, 77, 2852–2861. [Google Scholar] [CrossRef]
- Weng, L.; Alonso Vega, F.; Van Riemsdijk, W.H. Strategies in the application of the Donnan membrane technique. Environ. Chem. 2011, 8, 466–474. [Google Scholar] [CrossRef]
- Rocha, L.S.; Botero, W.G.; Alves, N.G.; Moreira, J.A.; da Costa, A.M.R.; Pinheiro, J.P. Ligand Size Polydispersity Effect on Sscp Signal Interpretation. Electrochim. Acta 2015, 166, 395–402. [Google Scholar] [CrossRef]
- Weng, L.; Van Riemsdijk, W.H.; Temminghoff, E.J.M. Effects of lability of metal complex on free ion measurement using DMT. Environ. Sci. Technol. 2010, 44, 2529–2534. [Google Scholar] [CrossRef]
- Aguilar, D.; Galceran, J.; Companys, E.; Puy, J.; Parat, C.; Authier, L.; Potin, M. Non-purged voltammetry explored with AGNES. Phys. Chem. Chem. Phys. 2013, 15, 17510–17521. [Google Scholar] [CrossRef] [PubMed]
- Audry, S.; Blanc, G.; Schafer, J. Cadmium transport in the Lot-Garonne River system (France)-temporal variability and a model for flux estimation. Sci. Total Environ. 2004, 319, 197–213. [Google Scholar] [CrossRef] [PubMed]



| Synthetic River | Natural Samples | |||
|---|---|---|---|---|
| Cd | Cd + FA | Uzein + Cd | Riu Mort | |
| Slope | 0.059 ± 0.007 | 0.055 ± 0.006 | 0.034 ± 0.004 | 0.034 |
| Plateau | 0.087 ± 0.013 | 0.080 ± 0.003 | 0.041 ± 0.004 | 0.021 |
| Plateau-corrected | 0.049 ± 0.011 | 0.052 ± 0.015 | 0.031 ± 0.007 | 0.021 |
| Riou Mort | Uzein River | |
|---|---|---|
| pH | 7.5 | 7.44 ± 0.04 |
| Conductivity (μS/cm) | 354 | 285 ± 13 |
| DOC (mg/L) | nd ** | 1.5 |
| Ca2+ (mM) * | 3.7 | 3.2 ± 0.3 |
| Cd (nM) | 37 | nd ** |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ricard, E.; Pinheiro, J.-P.; Le Hécho, I.; Parat, C. ISIDORE, a Probe for In Situ Trace Metal Speciation Based on the Donnan Membrane Technique and Electrochemical Detection Part 2: Cd and Pb Measurements during the Accumulation Time of the Donnan Membrane Technique. Molecules 2023, 28, 846. https://doi.org/10.3390/molecules28020846
Ricard E, Pinheiro J-P, Le Hécho I, Parat C. ISIDORE, a Probe for In Situ Trace Metal Speciation Based on the Donnan Membrane Technique and Electrochemical Detection Part 2: Cd and Pb Measurements during the Accumulation Time of the Donnan Membrane Technique. Molecules. 2023; 28(2):846. https://doi.org/10.3390/molecules28020846
Chicago/Turabian StyleRicard, Estelle, Jose-Paulo Pinheiro, Isabelle Le Hécho, and Corinne Parat. 2023. "ISIDORE, a Probe for In Situ Trace Metal Speciation Based on the Donnan Membrane Technique and Electrochemical Detection Part 2: Cd and Pb Measurements during the Accumulation Time of the Donnan Membrane Technique" Molecules 28, no. 2: 846. https://doi.org/10.3390/molecules28020846
APA StyleRicard, E., Pinheiro, J.-P., Le Hécho, I., & Parat, C. (2023). ISIDORE, a Probe for In Situ Trace Metal Speciation Based on the Donnan Membrane Technique and Electrochemical Detection Part 2: Cd and Pb Measurements during the Accumulation Time of the Donnan Membrane Technique. Molecules, 28(2), 846. https://doi.org/10.3390/molecules28020846






