Next Article in Journal
Aldiminium Cations as Countercations to Discrete Main Group Fluoroanions
Next Article in Special Issue
Influence of Co3O4 Nanostructure Morphology on the Catalytic Degradation of p-Nitrophenol
Previous Article in Journal
Detection of Polonium-210 in Environmental, Biological and Food Samples: A Review
Previous Article in Special Issue
Mass Spectra Fitting as Diagnostic Tool for Magnetron Plasmas Generated in Ar and Ar/H2 Gases with Tungsten Targets
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 28
Issue 17
10.3390/molecules28176269
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

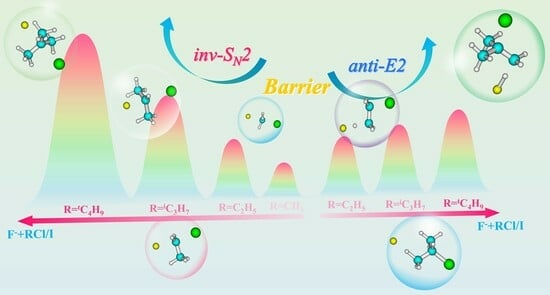
Effects of Methyl Substitution and Leaving Group on E2/SN2 Competition for Reactions of F− with RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I)

by
Wenqing Zhen
,
Siwei Zhao
,
Gang Fu
,
Hongyi Wang
,
Jianmin Sun
,
Li Yang
* and
Jiaxu Zhang
*
State Key Laboratory of Urban Water Resource and Environment, School of Chemistry and Chemical Engineering, Harbin Institute of Technology, Harbin 150001, China
*
Authors to whom correspondence should be addressed.
Molecules 2023, 28(17), 6269; https://doi.org/10.3390/molecules28176269
Submission received: 3 August 2023 / Revised: 22 August 2023 / Accepted: 24 August 2023 / Published: 27 August 2023
(This article belongs to the Special Issue Exclusive Feature Papers in Physical Chemistry)
Browse Figures
Versions Notes

Abstract

:
The competition between base-induced elimination (E2) and bimolecular nucleophilic substitution (SN2) is of significant importance in organic chemistry and is influenced by many factors. The electronic structure calculations for the gas-phase reactions of F− + RY (R = CH3, C2H5, iC3H7, tC4H9, and Y = Cl, I) are executed at the MP2 level with aug-cc-pVDZ or ECP/d basis set to investigate the α-methyl substitution effect. The variation in barrier height, reaction enthalpy, and competition of SN2/E2 as a function of methyl-substitution and leaving group ability has been emphasized. And the nature of these rules has been explored. As the degree of methyl substitution on α-carbon increases, the E2 channel becomes more competitive and dominant with R varying from C2H5, iC3H7, to tC4H9. Energy decomposition analysis offers new insights into the competition between E2 and SN2 processes, which suggests that the drop in interaction energy with an increasing degree of substitution cannot compensate for the rapid growth of preparation energy, leading to a rapid increase in the SN2 energy barrier. By altering the leaving group from Cl to I, the barriers of both SN2 and E2 monotonically decrease, and, with the increased number of substituents, they reduce more dramatically, which is attributed to the looser transition state structures with the stronger leaving group ability. Interestingly, ∆E0‡ exhibits a positive linear correlation with reaction enthalpy (∆H) and halogen electronegativity. With the added number of substituents, the differences in ∆E0‡ and ∆H between Y = Cl and I likewise exhibit good linearity.

Graphical Abstract

1. Introduction

Two basic organic reactions in the development of modern physical organic chemistry, base-induced bimolecular elimination (E2) and bimolecular nucleophilic substitution (SN2) reactions, usually compete with each other in many cases. In the past few decades, E2 and SN2 reactions have been widely researched in the gas and condensed phase, both experimentally [1,2,3] and theoretically [4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19]. Since SN2 and E2 pathways generate the same ionic product, it is a serious challenge to distinguish between the two processes using conventional spectrometric techniques [20]. Kinetic isotope effects could qualitatively tell E2 and SN2 pathways apart, as it is observed that E2 reactions have normal KIEs (kH/kD > 1), whereas SN2 reactions have inverse KIEs (kH/kD < 1) [1]. In this regard, theoretical approaches have played an important role in probing the mechanisms. Extensive theoretical investigations have mainly focused on the reactions of the type of X− + CH3CH2Y (X = F, Cl, OH, ClO, et al.; Y = F, Cl, Br, I) over the years [13,14,16,17,21,22,23,24,25,26,27,28,29]. The competition between E2 and SN2 reaction pathways caused by multiple factors, such as nucleophile, leaving groups, substrate characteristics, and environment, has been investigated in detail [10,16,17,25,28,29,30,31]. Mugnai et al. [10] analyzed the influence of temperature on the competition between E2 and SN2 in F− + CH3CH2Cl reaction by ab initio molecular dynamics, and found that SN2 and E2 reaction mechanisms were favored at high and low temperatures, respectively. In addition, the ion-imaging experiments and quasi-classical trajectory simulations of this system conducted by Wester et al. [31] also showed that the SN2 mechanism becomes more relevant as the collision energy is increased. Bickelhaupt and colleagues [22], founded on an activation-strain analysis, reported that F− could participate in a more stabilizing orbital interaction with CH3CH2Cl than PH2−, owing to its augmented proton affinity, leading to the observed preference for E2. Shaik et al. [24]. from the VB perspective, revealed that essentially SN2 was the preferred reaction pathway but the E2 pathway prevailed in many cases on account of the greater resonance stabilization in its transition-state (TS) region.
Among these factors, substituent effects have revealed an important function in studying the mechanisms and the competition between the E2 and SN2 [32,33,34,35,36,37]. Gronert [28,29] measured fluoride reacting with CH3Cl with a reaction efficiency of 0.56, and with the increased degree of substitution to ethyl, isopropyl, and tert-butyl chloride, the efficiency improved from 0.79 to 0.93 due to the presence of an E2 pathway, which was entropically favored [36]. Further theoretical calculations at the MP2/6-31 + G** level for the reaction of F− with CH3CHClCH3 pointed out methyl substitution at the α-carbon elevated the barrier of the SN2 pathway due to the crowded environment while stabilizing the transition state in E2 reactions [38]. Accordingly, Bierbaum et al. [1] explored the competition between E2 and SN2 pathways for BrO− and ClO− with RCl (R = CH3, C2H5, iC3H7, tC4H9) by using deuterium kinetic isotope effect (KIE) means. For each anion series, the trend that KIEs became increasingly more normal (kH/kD > 1) as the extent of substitution indicated that the E2 pathway became the predominant pathway and steric effects inhibited the SN2 pathway. A method calculating “steric hindrance (SH)” was proposed by Pendás and co-workers [39,40], which was consistent with the chemical intuition that the SH of the complex methylated alkyl system SN2 was greater than E2.
Recently, Wester et al. [41] presented a series of reactions X− + RY (X = F, Cl, R = CH3, C2H5, iC3H7, tC4H9 and Y = Cl, I), which displayed the transition from backward to forward scattering by the substitution of the α-carbon. It is noticed that the changes following increasing methyl substitution are somewhat different for different leaving groups. Compared to the rich experimental studies, systematic and accurate PESs for complex methylated alkyl halides, especially iC3H7Y and tC4H9Y, are still lacking. In this paper, a series of F− + RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I) reactions as shown in Scheme 1 have been investigated with electronic structure calculations. We revisit these systems and try to address the following two issues. (1) The accurate PESs for a series of reactions are established and compared to explore how methyl substitution at the α-carbon affects the E2/SN2 competing mechanisms. (2) It will be of interest to probe the role of the leaving group in E2/SN2 reactions of α-methyl substitution.

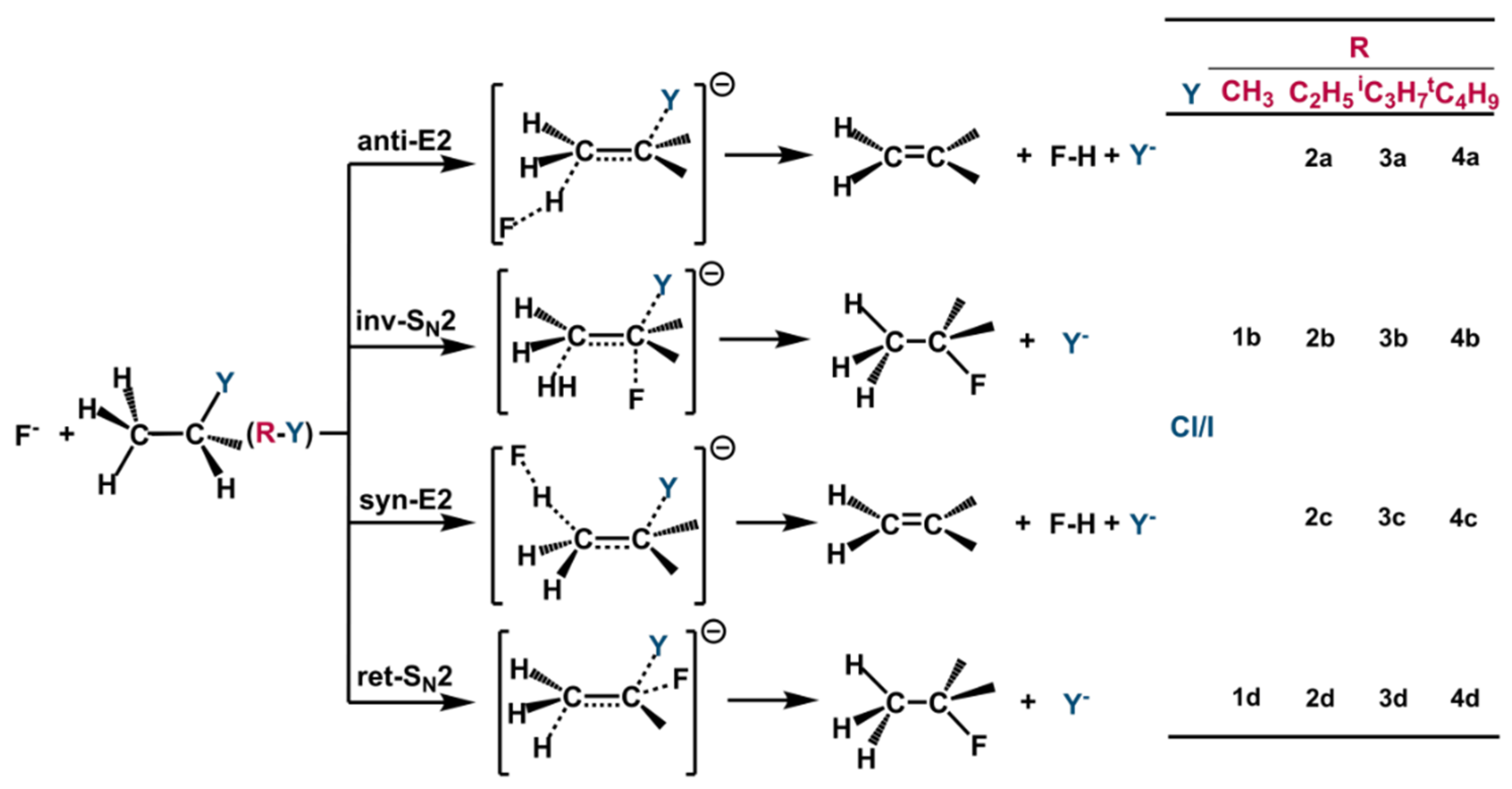
2. Results and Discussion

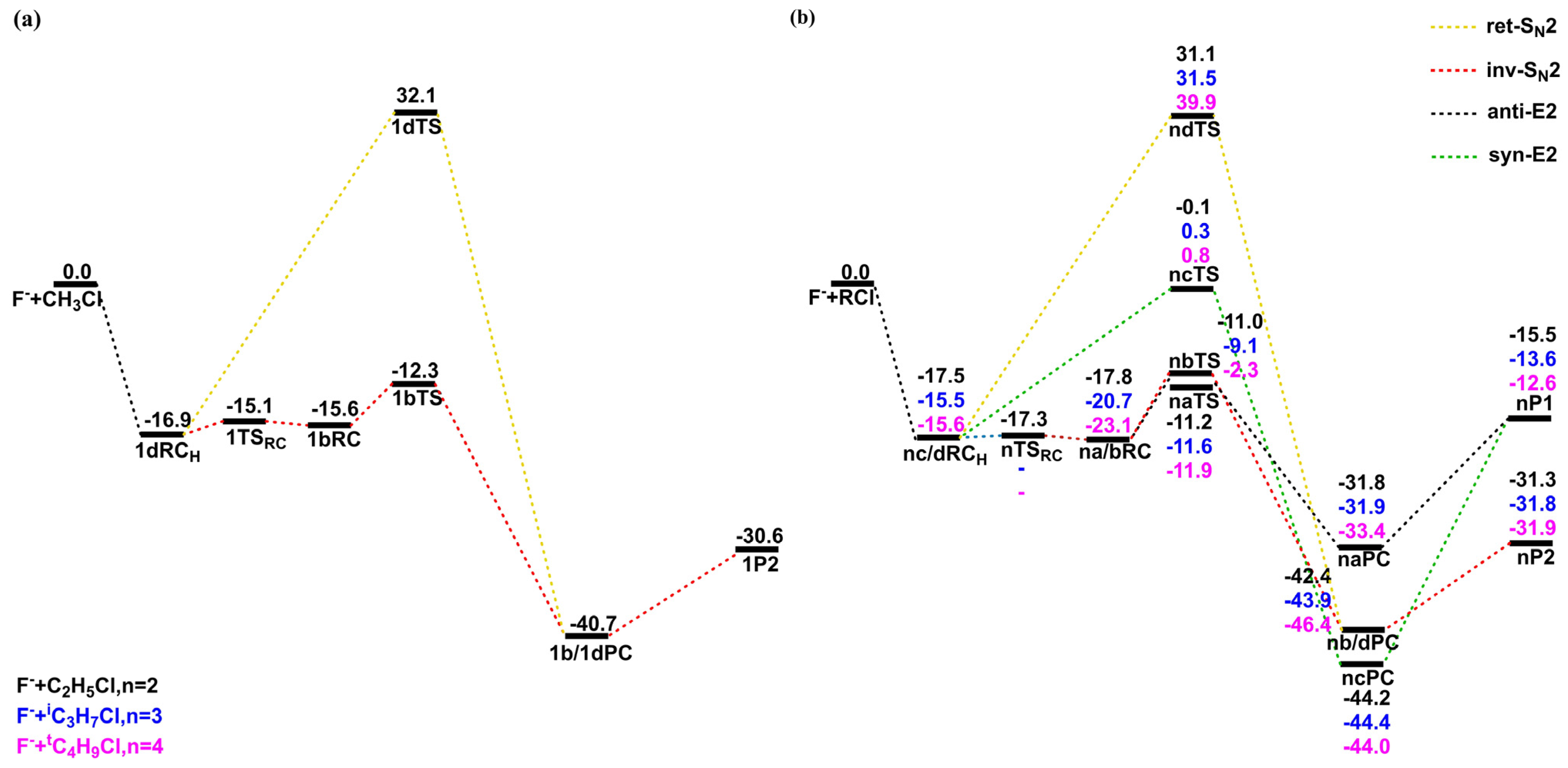
2.1. Potential Energy Surfaces of F− + RY Reactions

The relative energy of stationary points of F− + RCl (R = CH3, C2H5, iC3H7, tC4H9 and Y = Cl, I) calculated by the MP2 method are displayed in Table 1 together with the high-level CCSD(T)-F12b [16,42], CCSD(T) [17] benchmark values, and the available B97-1/aug-cc-pVDZ values [43]. The average deviation from the benchmark is 0.11/0.48, 0.16/0.13, and 0.16/0.35 kcal/mol for CH3Cl/I, C2H5Cl/I, and iC3H7Cl/I, respectively, indicating that the MP2/aug-cc-pVDZ (ECP/d) level is reasonable. The profiles of PES for F− + RY are presented in detail in Figure 1a,b and Figure S1a,b together with the geometrical structures in Figure S2a,d. As shown in Figure 1 and Figure S1, similar to the reaction of F− with ethyl halide, for isopropyl and tert-butyl reactions, four traditional reaction pathways, including base-induced anti and syn elimination (anti-E2 and syn-E2), as well as nucleophilic substitution with inversion and retention of configuration (inv-SN2 and ret-SN2), are predicted by MP2 theory. Inv-SN2 and anti-E2 reactions share the same reactant complex (a/bRC), and ret-SN2 and syn-E2 also utilize a common complex (c/dRC) along the reaction coordinate. With the increased methyl substitution, the difference in PES profiles of the series of reactions lies in the entrance channel. For F− + RY (R = CH3, C2H5, and Y = Cl, I), a hydrogen-bonded F···HCαH2(CH2)Y complex (c/dRCH) is obtained, which can easily convert to an ion-dipole complex F···HβCH2(CH2)Y (a/bRC) via a low-energy TSRC [16,29,42,44,45,46,47,48,49,50,51,52]. In contrast, for F− + RCl (R = iC3H7, tC4H9 and Y = Cl, I) reactions, only an ion-dipole complex (a/bRC) is found, where F− is situated between α-carbon and β-hydrogen of iso-propyl or tert-butyl moiety, so that F− can attack either target atom to form an inv-SN2 or anti-E2 channel. For the reactant complex F···HβH2(CH3)CHY (c/dRC) in syn-E2 and ret-SN2 channels, F− and some hydrogen atoms in β-position, instead of α-hydrogen of alkyl halides, have a mild hydrogen-bond interaction. There is no transition state available for the conversion of these two RCs.
Resulting from the strong steric exclusion between the nucleophile and substrate, the ret- SN2 TS usually gives a much higher overall barrier than other reaction pathways, suggesting it is the least favorable pathway. The barrier for the syn-E2 pathway is usually higher than inv-SN2 and anti-E2 for a similar hindrance effect. Therefore, in the following discussions, the most competitive inv-SN2 and anti-E2 pathways for the series of F− + RY reactions are considered here, and their PES profiles obtained at the MP2 level of theory are characterized in Figure 2 for convenience of comparison. For the F− + CH3Y reaction, F− attacks CH3Y on the back-side via a traditional path along a pre-reaction ion-dipole complex (1bRC), a Walden-inversion transition state (1bTS), and a post-reaction ion-dipole complex FCH3···Y− (1bPC). The E2 pathways appear with the successive addition of the methyl group besides the SN2 pathways and show similar double well potential characters. The initial association of F− and RY can form an ion-dipole complex a/bRC and, after going over a/bTS, the system drops down to the deep potential energy well a/bPC and then decomposes to products P1 (RF + Cl−/I−) and P2 (RCH2 = CH2 + HF + Cl−/I−), respectively.

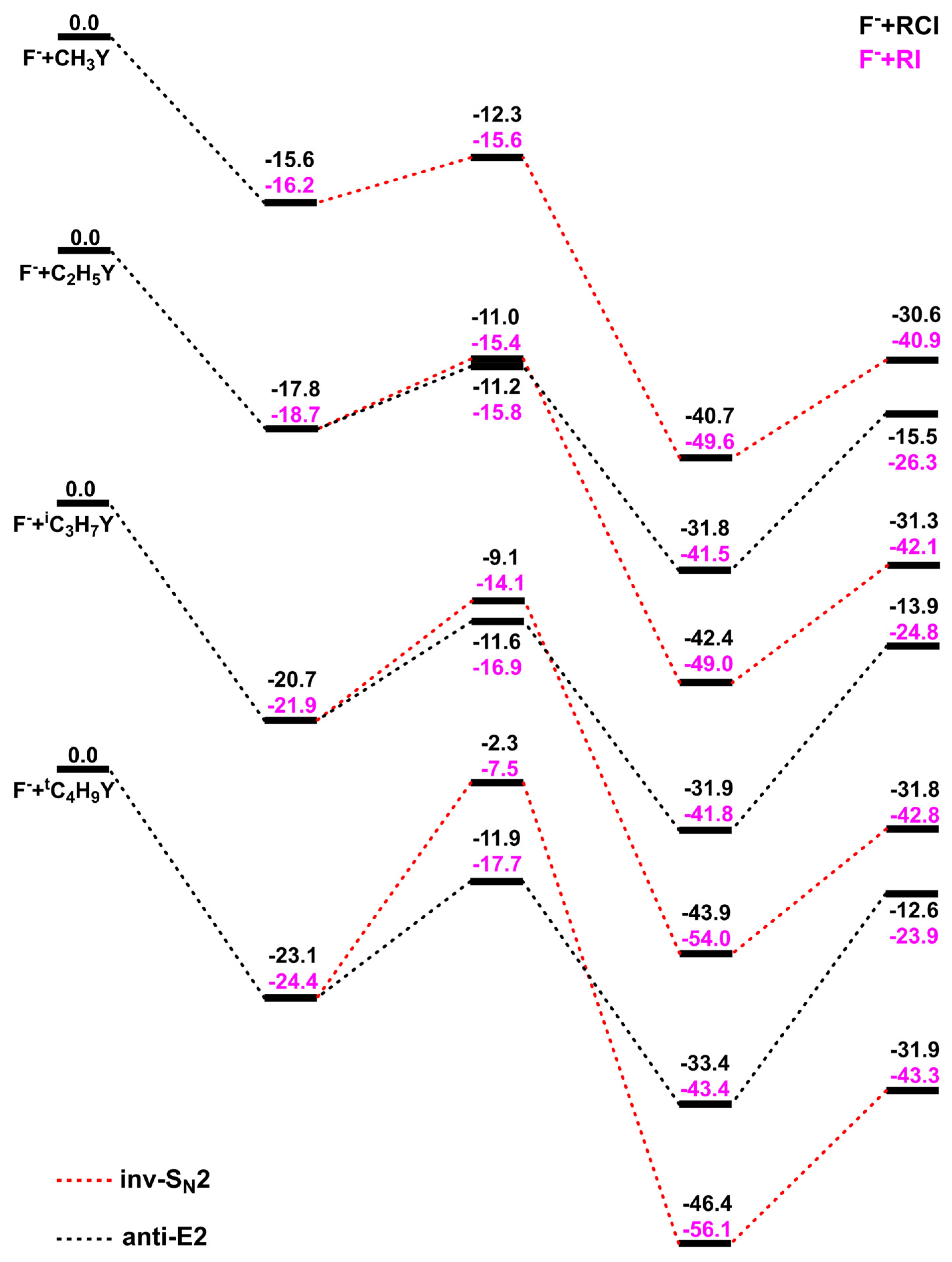
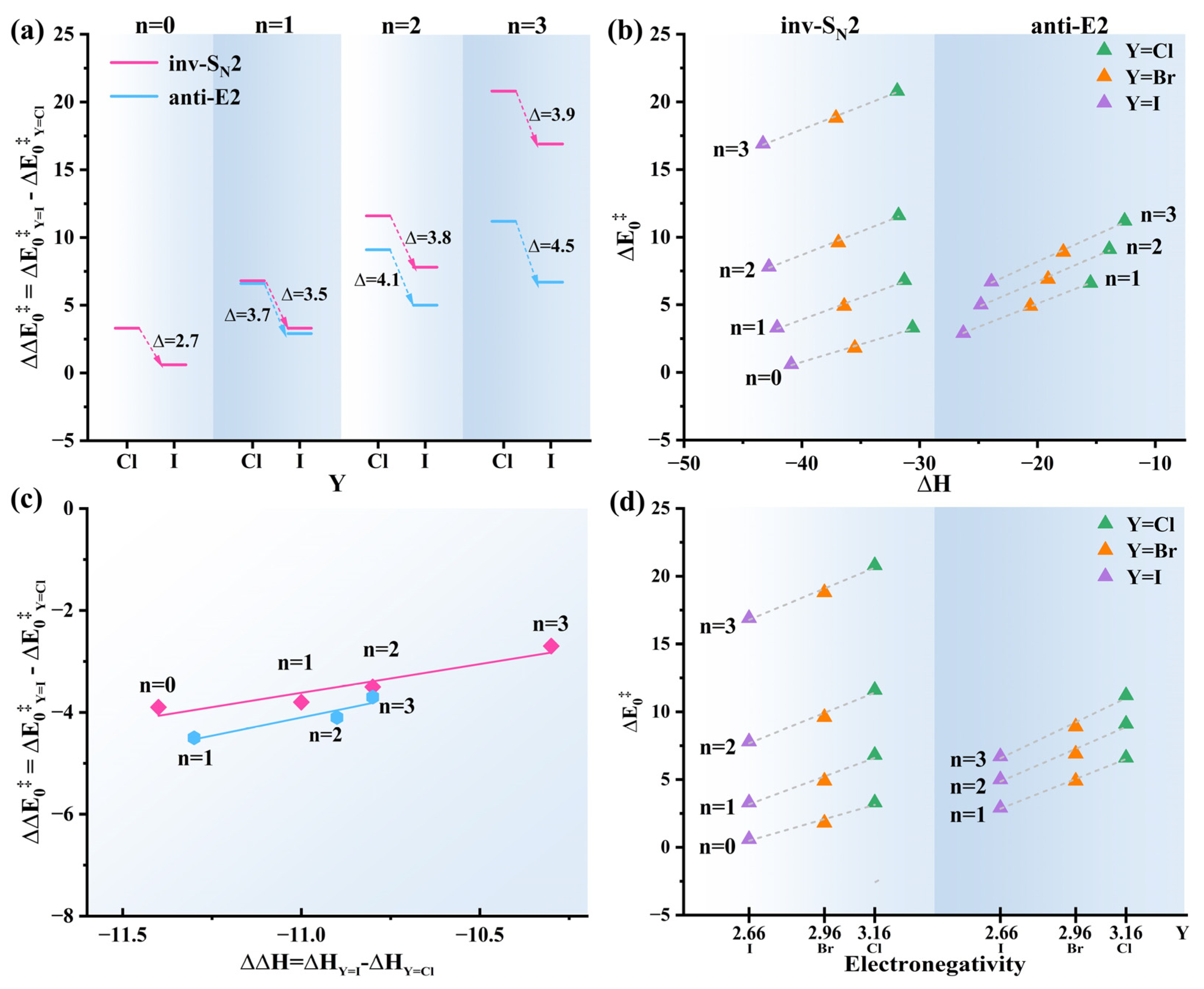
2.2. Effects of α-Methyl Substitution

To explore the effects of the addition of the α-methyl group on the competition of E2 and SN2 mechanisms, the activation (∆E0‡) and the overall barrier (∆E‡) are especially emphasized for discussion as presented in Scheme 2, and the calculated values of the relevant energies are summarized in Table 1. Here, Y = Cl is used as an example.
Exothermicity. As shown in Figure 2, it is clear that all E2 and SN2 paths for the reaction of F− + RY (R = CH3, C2H5, iC3H7, tC4H9, and Y = Cl, I) are highly exothermic, and, for each reaction, the reaction enthalpy ∆H of SN2 pathways is much more negative than that of E2 pathways, suggesting SN2 reactions are more exothermic than E2 reactions owing to the strong combination between the F atom and C atoms in the neutral products. By changing R from the methyl to the tert-butyl group, the ∆H of SN2 pathways slightly drops, while the values of E2 pathways escalate, eventually widening the ∆H gap between E2 and SN2.
Barrier Height. The variation of ∆E0‡, ∆E‡, and the difference in ∆E0‡ between inv-SN2 and anti-E2 along the methylation of the α-carbon is illustrated in Figure 3a–c. The horizontal coordinate is the degree of methyl substitution of Cα, named n, ranging from 0 to 3. As described in Figure 3a, successively adding methyl groups to the Cα dramatically raises the activation barrier (∆E0‡) of inv-SN2 from 3.3 to 6.8 to 11.6 and finally to 20.8 kcal/mol, while the ∆E0‡ of anti-E2 gently escalates from 6.6, 9.1 to 11.2 kcal/mol. Figure 3b depicts the activation barrier difference between the inv-SN2 and anti-E2 pathways (∆∆E0‡ = ∆E0‡inv-SN2 − ∆E0‡anti-E2) as n changes from 1 to 3, which is 0.2 and 2.5 kcal/mol for n = 1 and 2, respectively. The significant augment of ∆∆E0‡ for the F− + tC4H9Cl reaction is observed in doubling the difference to 9.6 kcal/mol. All these results suggest that anti-E2 is becoming more and more competitive. Wester and co-workers [32] have disentangled the dynamics of the competition between anti-E2 and inv-SN2 in the reaction F− + C2H5Cl, indicating that anti-E2 is more advantageous. In addition, Gronert [38] also predicted that in the reaction of F− + iC3H7Cl anti-E2 is completely dominated and substitution should be more competitive with ethyl halides. As the maximum difference between ∆E0‡(SN2) and ∆E0‡(anti-E2) of these three systems, the anti-E2 mechanism will also be the most favorable pathway for F− + tC4H9Cl (I).
For the variation pattern of overall barriers (∆E‡), the α-methyl group slightly changes the ∆E‡ of an anti-E2 pathway with values gradually dropping from −11.2, −11.6 to −11.9 kcal/mol but significantly alters the barrier of inv-SN2 from −12.3 kcal/mol for F− + CH3Cl to −2.3 kcal/mol for F− + tC4H9Cl. Rablen et al. [35] systematically compared the free energies of SN2/E2 transition states for CN− + RCl reactions at the W1 and G4 levels of electronic structure theory in the presence of a simulated acetonitrile solvent. Their results suggest that the barrier to the E2 reaction reduces by the same magnitude as the barrier to SN2 raises when methylation of the α-carbon increases. Connor and Gronert [34] studied the impact of α- and β-methylation on E2 and SN2 reactions between a series of alkyl bromides and nucleophiles in the gas phase using both mass spectrometer experiment and computational methods. They found the reduction in SN2 rate constant and the mounting in E2 rate constant when adding a methyl group to the α-carbon position, which is in line with our findings.
To understand why the increasing alkyl substitution strikingly enhances the ∆E‡ of the SN2 reaction and slightly lowers the E2 barrier, we decompose the transition state energy by referring to the activation strain analyses proposed by Bickelhaupt et al. [28,53,54] as shown in Figure 3b. Energy decomposition contributes to a quantitative understanding of how methyl substitution affects the inv-SN2/anti-E2 reaction barrier. The total energy ∆E‡, that is, the difference between reactants and transition states, can be decomposed into preparation energy (∆Eprep) and interaction energy (∆Eint) based on the formula ∆E‡ = ∆Eprep + ∆Eint. The ∆Eprep is the energy that is needed to overcome the deformation of individual reactants from their equilibrium structure into the geometries of the transition structure. And the interaction energy ∆Eint is considered as the energy difference between the individual fragments of transition states’ geometries and the transition states. For F− + RCl reactions, the inv-SN2 goes with less preparation energy than anti-E2, which can be attributed to the different mechanisms. One bond breaking occurs in the inv-SN2 mechanism, whereas two bond breakings and one C-C bond shrinking occur along the anti-E2 pathway. Hence, the destabilizing distortion characteristic for the anti-E2 reaction pathway is by definition higher than the inv-SN2 reaction pathway. As previously proposed by Bickelhaupt [53], the Cα-Y bond extension of inv-SN2 reduces the antibonding orbital overlap between C2p and Y2p orbitals, which makes the LUMO of the substrate more stable. Obviously, due to the antibonding orbital overlap of both the Cα-Y and Cβ-H bonds being diminished, this stabilization of the LUMO is more significant in the E2 reaction. For inv-SN2 transition structures, when the degree of CH3 varies from 0 to 3, ∆Eprep increases gradually, indicating the fragments in the transition structures distorted more violently, whereas ∆Eint decreases, suggesting the interaction of two parts is stronger. The decline in ∆Eint cannot pay for the rapid growth of ∆Eprep, resulting in the overall rise in ∆E‡. Especially in the F− + tC4H9Cl reaction, the increase in SN2 ∆E‡ is particularly significant. In comparison, the ∆Eprep of the anti-E2 transition structure is higher but elevates more slowly than that of the inv-SN2. Accordingly, the results obtained suggest that, compared to the anti-E2 reaction, the inv-SN2 reaction is more sensitive to structural changes in the substrate. Therefore, the barrier of inv-SN2 increases with the increased degree of substitution, resulting in less competitiveness compared to anti-E2, which is in agreement with previous research by Pendás et al. [39]. The ∆Eint is further broken down into the steric term ∆Esteric (∆Esteric = ∆Eels + ∆EXC + ∆EPauli) and the orbital interaction term ∆Eorb in order to ascertain the primary contributor to the fluctuation in ∆Eint as shown in Figure S3 [55]. Obviously, the orbital interaction term ∆Eorb is responsible for the interaction energy. The orbital interactions of both SN2 and E2 decrease as the number of substituents increases. It is noteworthy that the stronger orbital interaction favors the E2 reaction to lower ∆Eint compared to its SN2 analog. This is consistent with the finding by Bickelhaupt et al. [22] for the F− + CH3CH2Cl reaction.
These results suggest that, with the addition of α-methyl substituent, the anti-E2 reaction is completely dominant considering the energetics from ethyl to butyl reactions in the gas phase. This is consistent with the scattering experimental phenomenon [41] that the character of forward scattering becomes more and more obvious with the increased size of residue R, where scattering into the forward hemisphere is a mechanistic fingerprint of E2 reactions. Further dynamics simulations are desired for revealing the variation in an aspect of dynamical factors.

2.3. Effects of Leaving Group

It is of interest to explore the effect of the leaving group along the α-methyl substitution. Figure 4a compares the activation barrier heights of F− + RCl and F− + RI; it can be seen that, as the enhanced leaving group ability varies from Cl to I, both SN2 and E2 reaction barriers are dropped by similar amounts. More significantly, with the increased number of substituents of n = 0–3 the barrier heights decrease more dramatically from 3.5/3.7, 3.8/4.1, to 3.9/4.5 kcal/mol for the SN2/E2 reaction pathways along Y = Cl to I. Their key TS structural character could be closely related to this change. Variation in the leaving group from Cl to I causes the elongation of the Cα-Y bond ∆L (LCα-I − LCα-Cl) ranging from 0.26, 0.29, 0.32 to 0.38Å with n going from 0 to 3 for the TS structure of inv-SN2. Similarly, the bond elongation ∆L of Cα-Y for anti-E2 TS also grows along with methyl substitution degree n. The looser transition states typically align with the reduced barrier heights, resulting in heightened reactivity [20,56,57].
Along the leaving group ability changing from Cl, Br to I, a good linear relationship is found between ∆E0‡ and ∆H for both inv-SN2 and anti-E2 pathways of each F− + RY reaction as presented in Figure 4b. Obviously, the F− + RY reaction set obeys the expression ∆E0‡ = a∆H + C, which connects the barrier with the reaction enthalpy, following the Bell–Evans–Polanyi principle, which is a long-standing chemical theory [58]. Furthermore, good relevance is also found between activation barrier difference ∆∆E0‡ and enthalpy difference ∆∆H for Y = Cl and I with the increased degree of substitution of the corresponding SN2 and E2 pathways as shown in Figure 4c. The above results indicate that, as the leaving group changes from Cl to I, the reaction becomes more exothermic and the barrier drops. Barriers of both inv-SN2 and anti-E2 reactions are found to exhibit good linear dependences with halogen electronegativity increasing in the order of I (2.66) < Br (2.96) < Cl (3.16) as shown in Figure 4d. The above results suggest the decreased electronegativity of Y is expected to lead to a looser TS structure (elongation of C-Y bond), and further a higher reaction reactivity.

3. Computational Methods

The stationary points for a series of F− + RY (R = CH3, C2H5, iC3H7, tC4H9 and Y = Cl, I) reactions are studied by second-order Møller–Plesset perturbation MP2 [59,60] with the frozen core (FC) method. The atoms of H, C, F, and Cl are based on Dunning and Woons aug-cc-pVDZ [61,62] basis set. For I, the core electrons use the Wadt and Hay ECP [63] and the valence electrons use a 3s, 3p basis, plus a d-polarization function with a 0.262 exponent, and s, p, and diffuse functions with exponents of 0.034, 0.039, and 0.0873, respectively.
According to previous work, aug-cc-pVDZ basis set has the lowest systematic errors, while G** and aug-cc-pVTZ tend to overestimate and underestimate the single-point energies [64]. Aug-cc-pVDZ basis set also showed good agreement with the experiment in previous research on similar reactions [17,30,42]. Vibrational analysis is used to determine each stationary point under the harmonic oscillator mode in which balanced structures have no imaginary frequencies and transition states have one normal mode with an imaginary frequency. Furthermore, each transition state is calculated with the internal reaction coordinate (IRC) to make sure that it connects the assumed pre- and post-reaction complexes. The coupled cluster theory with triple excitations treated perturbatively CCSD(T) is often used as a benchmark due to its good accuracy [16,17,25,65]. Hence, the relative energies of the stationary points are compared with the high-level CCSD(T)-F12b [16,42] and CCSD(T) [17] benchmark values, and the available B97-1 values [43] for methyl, ethyl, and tert-butyl halide systems, to rationalize the dependability of the computing method. Since there is no report available previously for isopropyl halide reactions, the high-level CCSD(T)/aug-cc-pVTZ or CCSD(T)/ECP/d energy corrections based on MP2 geometries are performed. Gaussian 09 package [66] was used for all computations.

4. Conclusions

In this paper, F− ions with a series of α-substituted alkyl chlorides and alkyl iodides in the gas phase are studied by using MP2/aug-cc-pVDZ or MP2/ECP/d methods, and the effect of methyl substituents and leaving group ability on the competition of E2 and SN2 pathways is investigated. As the degree of methyl substitution increases, the preference for anti-E2 over inv-SN2 enlarges, and, till R = tC4H9, anti-E2 becomes overwhelmingly dominant. The prediction of this reaction trend is consistent with the differential scattering experiment [41]. This can be explained by energy decomposition analysis. With the increased degree of substitution, the drop in the interaction energy between reactants cannot compensate for the rapid growth of preparation energy resulting from the more distorted transition state structure for the inv-SN2 reaction pathway.
In the aspect of the leaving group, the barrier heights for both E2 and SN2 reactions drop more dramatically along Y = Cl to I with the increased number of substituents, which can be attributed to the relaxation of the key transition state structure. Variation in the leaving group from Cl to I results in the larger extension of the Cα-Y for the TS structure of anti-E2 and inv-SN2 with the methyl group going from 0 to 3, and thus the larger reaction activity. Along the leaving group ability changing from Cl, Br to I, a notable linear relationship is found between ∆E0‡ and ∆H for both the inv-SN2 and anti-E2 channels of each F− + RY reaction, indicating the smaller the reaction barrier, the more negative the reaction enthalpy. These results correspond to the decreasing halogen electronegativity from Cl to I, which results in the more relaxed structural characters of TS and thus the larger reaction probability. This work lays the foundation for our subsequent dynamic simulations. Since it is known that the dynamics of the reaction may deviate from the potential energy surface, dynamics simulations are able to intensify our understanding of the effects of the substitution and leaving group on the competition mechanism between E2 and SN2 reactions.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules28176269/s1, Figure S1: Potential energy curves and stationary points at MP2/ ECP/d level for F- + RI reactions. The energy (in kcal/mol) is relative to F- + CH3I (a) and F- + RI (b) reactants at 0K, and does not include ZPE. For Figure S1b, the black, blue, and pink numbers represent F- + C2H5I, F- + iC3H7I, and F- + tC4H9I reactions, respectively; Figure S2: Stationary point structures of E2 and SN2 pathways for (a) F-+ CH3Y, (b) F-+ C2H5Y, (c) F-+ iC3H7Y, (d) F-+ tC4H9Y(Y = Cl, I) reaction optimized at the MP2/aug-cc-pVDZ(ECP/d) theoretical level. Bond distances are in Å, and black/pink line represents Y = Cl/I.; Figure S3: Interaction energy decomposition according to formula ∆Eint = (∆Eels + ∆EXC + ∆EPauli) + ∆Eorb = ∆Esteric + ∆Eorb. ∆Eels is electrostatic interacton term, ∆EXC is the change of exchange-correlation energy during complexation process, and ∆EPauli is the Pauli repulsion effect between electrons in occupied orbitals of the fragments and is invariably positive. They combine to form steric term ∆Esteric (Dashed line). ∆Eorb is orbital interaction term and represented by short dotted line. The full line represents ∆Eint.

Author Contributions

Conceptualization, J.Z. and L.Y.; Funding acquisition, J.S., L.Y. and J.Z.; Investigation, W.Z., S.Z., G.F. and H.W.; Resources, L.Y. and J.Z.; Supervision, J.S., J.Z. and L.Y.; Writing—original draft, W.Z.; writing—review and editing, J.Z. and L.Y. All authors have read and agreed to the published version of the manuscript.

Funding

This work is supported by the State Key Lab of Urban Water Resource and Environment of Harbin Institute of Technology (No. ES202303, 2022TS25), the National Natural Science Foundation of China (No. 21972034), and Heilongjian Touyan Team (HITTY-20190033).

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The data presented in this study are available in Supplementary Material.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Villano, S.M.; Eyet, N.; Lineberger, W.C.; Bierbaum, V.M. Reactions of α-Nucleophiles with Alkyl Chlorides: Competition between SN2 and E2 Mechanisms and the Gas-Phase α-Effect. J. Am. Chem. Soc. 2009, 131, 8227–8233. [Google Scholar] [CrossRef] [PubMed]
  2. Garver, J.M.; Fang, Y.R.; Eyet, N.; Villano, S.M.; Bierbaum, V.M.; Westaway, K.C. A Direct Comparison of Reactivity and Mechanism in the Gas Phase and in Solution. J. Am. Chem. Soc. 2010, 132, 3808–3814. [Google Scholar] [CrossRef] [PubMed]
  3. Carrascosa, E.; Meyer, J.; Michaelsen, T.; Stei, M.; Wester, R. Conservation of direct dynamics in sterically hindered SN2/E2 reactions. Chem. Sci. 2018, 9, 693–701. [Google Scholar] [CrossRef] [PubMed]
  4. Gronert, S. Theoretical Studies of Elimination Reactions. 1. Reactions of F− and PH2− with CH3CH2Cl. Competition between SN2 and E2 Mechanisms for First- and Second-Row Nucleophiles. J. Am. Chem. Soc. 1991, 113, 6041–6048. [Google Scholar] [CrossRef]
  5. Haib, J.; Stahl, D. Competition Between Substitution (SN2), Elimination (E2) and Addition Elimination (AE) Reactions in the Gas Phase. Org. Mass. Spectrom. 1992, 27, 377–382. [Google Scholar] [CrossRef]
  6. Hu, W.P.; Truhlar, D.G. Factors Affecting Competitive Ion-Molecule Reactions: ClO− + C2H5Cl and C2D5Cl via E2 and SN2 Channels. J. Am. Chem. Soc. 1996, 118, 860–869. [Google Scholar] [CrossRef]
  7. Glad, S.S.; Jensen, F. Kinetic Isotope Effects and Transition State Geometries. A Theoretical Investigation of E2 Model Systems. J. Org. Chem. 1997, 62, 253–260. [Google Scholar] [CrossRef]
  8. Chung, D.S.; Kim, C.K.; Lee, I. Theoretical Studies of Competitive Gas-Phase SN2 and E2 Reactions of NCCH2CH2Cl with OH− and SH−. J. Phys. Chem. A 1997, 101, 9097–9104. [Google Scholar] [CrossRef]
  9. Bickelhaupt, F.M. Understanding Reactivity with Kohn-Sham Molecular Orbital Theory: E2-SN2 Mechanistic Spectrum and Other Concepts. J. Comput. Chem. 1999, 20, 114–128. [Google Scholar] [CrossRef]
  10. Mugnai, M.; Cardini, G.; Schettino, V. Substitution and Elimination Reaction of F− with C2H5Cl: An ab Initio Molecular Dynamics Study. J. Phys. Chem. A 2003, 107, 2540–2547. [Google Scholar] [CrossRef]
  11. Vayner, G.; Houk, K.N.; Jorgensen, W.L.; Brauman, J.I. Steric Retardation of SN2 Reactions in the Gas Phase and Solution. J. Am. Chem. Soc. 2004, 126, 9054–9058. [Google Scholar] [CrossRef]
  12. Ochran, R.A.; Uggerud, E. SN2 reactions with allylic substrates—Trends in reactivity. Int. J. Mass. Spectrom. 2007, 265, 169–175. [Google Scholar] [CrossRef]
  13. Bento, A.P.; Solà, M.; Bickelhaupt, F.M. E2 and SN2 Reactions of X− + CH3CH2X(X = F, Cl); an ab Initio and DFT Benchmark Study. J. Chem. Theory Comput. 2008, 4, 929–940. [Google Scholar] [CrossRef] [PubMed]
  14. Wu, X.P.; Sun, X.M.; Wei, X.G.; Ren, Y.; Wong, N.B.; Li, W.K. Exploring the Reactivity Trends in the E2 and SN2 Reactions of X− + CH3CH2Cl (X = F, Cl, Br, HO, HS, HSe, NH2, PH2, AsH2,CH3, SiH3, and GeH3). J. Chem. Theory Comput. 2009, 5, 1597–1606. [Google Scholar] [CrossRef]
  15. Wolters, L.P.; Ren, Y.; Bickelhaup, F.M. Understanding E2 versus SN2 Competition under Acidic and Basic Conditions. ChemistryOpen 2014, 3, 29–36. [Google Scholar] [CrossRef]
  16. Tajti, V.; Czakó, G. Benchmark ab Initio Characterization of the Complex Potential Energy Surface of the F− + CH3CH2Cl Reaction. J. Phys. Chem. A 2017, 121, 2847–2854. [Google Scholar] [CrossRef] [PubMed]
  17. Yang, L.; Zhang, J.X.; Xie, J.; Ma, X.Y.; Zhang, L.Y.; Zhao, C.Y.; Hase, W.L. Competing E2 and SN2 Mechanisms for the F− + CH3CH2I Reaction. J. Phys. Chem. A 2017, 121, 1078–1085. [Google Scholar] [CrossRef]
  18. Hamlin, T.A.; Swart, M.; Bickelhaupt, F.M. Nucleophilic Substitution (SN2): Dependence on Nucleophile, Leaving Group, Central Atom, Substituents, and Solvent. Chemphyschem 2018, 19, 1315–1330. [Google Scholar] [CrossRef] [PubMed]
  19. Satpathy, L.; Sahu, P.K.; Behera, P.K.; Mishra, B.K. Solvent Effect on the Potential Energy Surfaces of the F− + CH3CH2Br Reaction. J. Phys. Chem. A 2018, 122, 5861–5869. [Google Scholar] [CrossRef]
  20. Garver, J.M.; Eyet, N.; Villano, S.M.; Yang, Z.; Bierbaum, V.M. Mechanistic investigation of SN2 dominated gas phase alkyl iodide reactions. Int. J. Mass. Spectrom. 2011, 301, 151–158. [Google Scholar] [CrossRef]
  21. Vermeeren, P.; Hansen, T.; Jansen, P.; Swart, M.; Hamlin, T.A.; Bickelhaupt, F.M. A Unified Framework for Understanding Nucleophilicity and Protophilicity in the SN2/E2 Competition. Chem. Eur. J. 2020, 26, 15538–15548. [Google Scholar] [CrossRef] [PubMed]
  22. Vermeeren, P.; Hansen, T.; Grasser, M.; Silva, D.R.; Hamlin, T.A.; Bickelhaupt, F.M. SN2 versus E2 Competition of F− and PH2− Revisited. J. Org. Chem. 2020, 85, 14087–14093. [Google Scholar] [CrossRef] [PubMed]
  23. Hansen, T.; Vermeeren, P.; Bickelhaupt, F.M.; Hamlin, T.A. Origin of the α-Effect in SN2 Reactions. Angew. Chem. Int. Ed. 2021, 60, 20840–20848. [Google Scholar] [CrossRef] [PubMed]
  24. Stuyver, T.; Shaik, S. Resolving Entangled Reactivity Modes through External Electric Fields and Substitution: Application to E2/SN2 Reactions. J. Org. Chem. 2021, 86, 9030–9039. [Google Scholar] [CrossRef] [PubMed]
  25. Tasi, D.A.; Tokaji, C.; Czako, G. A benchmark ab initio study of the complex potential energy surfaces of the OH− + CH3CH2Y [Y = F, Cl, Br, I] reactions. Phys. Chem. Chem. Phys. 2021, 23, 13526–13534. [Google Scholar] [CrossRef]
  26. Hansen, T.; Roozee, J.C.; Bickelhaupt, F.M.; Hamlin, T.A. How Solvation Influences the SN2 versus E2 Competition. J. Org. Chem. 2022, 87, 1805–1813. [Google Scholar] [CrossRef]
  27. Li, Y.; Li, C.; Gao, D.; Wang, D. Atomic-Level Mechanism, Solvent Effect, and Potential of the Mean Force of the F− + CH3CH2Cl SN2 Reaction in Aqueous Solution. J. Phys. Chem. A 2022, 126, 5527–5533. [Google Scholar] [CrossRef]
  28. Wu, X.; Zhang, S.; Xie, J. Investigating the competing E2 and SN2 mechanisms for the microsolvated HO− (H2O)n=0–4 + CH3CH2X (X = Cl, Br, I) reactions. Phys. Chem. Chem. Phys. 2022, 24, 12993–13005. [Google Scholar] [CrossRef]
  29. Zhao, S.; Fu, G.; Zhen, W.; Yang, L.; Sun, J.; Zhang, J. Reaction mechanism conversion induced by the contest of nucleophile and leaving group. Phys. Chem. Chem. Phys. 2022, 24, 24146–24154. [Google Scholar] [CrossRef]
  30. Tajti, V.; Czako, G. Vibrational mode-specific dynamics of the F− + CH3CH2Cl multi-channel reaction. Phys. Chem. Chem. Phys. 2022, 24, 8166–8181. [Google Scholar] [CrossRef]
  31. Meyer, J.; Tajti, V.; Carrascosa, E.; Gyori, T.; Stei, M.; Michaelsen, T.; Bastian, B.; Czako, G.; Wester, R. Atomistic dynamics of elimination and nucleophilic substitution disentangled for the F− + CH3CH2Cl reaction. Nat. Chem. 2021, 13, 977–981. [Google Scholar] [CrossRef]
  32. Nettey, S.; Swift, C.A.; Joviliano, R.; Noin, D.O.; Gronert, S. The impact of substituents on the transition states of SN2 and E2 reactions in aliphatic and vinylic systems: Remarkably facile vinylic eliminations. J. Am. Chem. Soc. 2012, 134, 9303–9310. [Google Scholar] [CrossRef] [PubMed]
  33. Martinez, H.; Rebeyrol, A.; Nelms, T.B.; Dolbier, W.R. Impact of fluorine substituents on the rates of nucleophilic aliphatic substitution and β-elimination. J. Fluor. Chem. 2012, 135, 167–175. [Google Scholar] [CrossRef]
  34. Conner, K.M.; Gronert, S. Impact of alkyl substituents on the gas-phase competition between substitution and elimination. J. Org. Chem. 2013, 78, 8606–8613. [Google Scholar] [CrossRef] [PubMed]
  35. Rablen, P.R.; McLarney, B.D.; Karlow, B.J.; Schneider, J.E. How alkyl halide structure affects E2 and SN2 reaction barriers: E2 reactions are as sensitive as SN2 reactions. J. Org. Chem. 2014, 79, 867–879. [Google Scholar] [CrossRef]
  36. DePuy, C.H.; Gronert, S.; Mullin, A.; Bierbaum, V.M. Gas-Phase SN2 and E2 Reactions of Alkyl Halides. J. Am. Chem. Soc. 1990, 112, 8650–8655. [Google Scholar] [CrossRef]
  37. Gronert, S.; DePuy, C.H.; Bierbaum, V.M. Deuterium Isotope Effects in Gas-Phase Reactions of Alkyl Halides: Distinguishing E2 and SN2 Pathways. J. Am. Chem. Soc. 1991, 113, 4009–4010. [Google Scholar] [CrossRef]
  38. Gronert, S. Theoretical Studies of Elimination Reactions. 3. Gas-Phase Reactions of F− with (CH3)2CHCl and CH3CH2CH2Cl. The Effect of Methyl Substituents. J. Am. Chem. Soc. 1993, 115, 652–659. [Google Scholar] [CrossRef]
  39. Gallegos, M.; Costales, A.; Pendás, Á.M. Does Steric Hindrance Actually Govern the Competition between Bimolecular Substitution and Elimination Reactions? J. Phys. Chem. A 2022, 126, 1871–1880. [Google Scholar] [CrossRef]
  40. Gallegos, M.; Costales, A.; Pendás, Á.M. A real space picture of the role of steric effects in SN2 reactions. J. Comput. Chem. 2022, 43, 785–795. [Google Scholar] [CrossRef]
  41. Carrascosa, E.; Meyer, J.; Zhang, J.; Stei, M.; Michaelsen, T.; Hase, W.L.; Yang, L.; Wester, R. Imaging dynamic fingerprints of competing E2 and SN2 reactions. Nat. Comun. 2017, 8, 25. [Google Scholar] [CrossRef] [PubMed]
  42. Szabo, I.; Czako, G. Revealing a double-inversion mechanism for the F− + CH3Cl SN2 reaction. Nat. Commun. 2015, 6, 5972. [Google Scholar] [CrossRef] [PubMed]
  43. Meyer, J.; Carrascosa, E.; Michaelsen, T.; Bastian, B.; Li, A.; Guo, H.; Wester, R. Unexpected Indirect Dynamics in Base-Induced Elimination. J. Am. Chem. Soc. 2019, 141, 20300–20308. [Google Scholar] [CrossRef] [PubMed]
  44. Zhang, J.; Hase, W.L. Electronic Structure Theory Study of the F− + CH3I → FCH3 + I− Potential Energy Surface. J. Phys. Chem. A 2010, 114, 9635–9643. [Google Scholar] [CrossRef] [PubMed]
  45. Szabó, I.; Császár, A.G.; Czakó, G. Dynamics of the F− + CH3Cl → Cl− + CH3F SN2 reaction on a chemically accurate potential energy surface. Chem. Sci. 2013, 4, 4362. [Google Scholar] [CrossRef]
  46. Stei, M.; Carrascosa, E.; Kainz, M.A.; Kelkar, A.H.; Meyer, J.; Szabo, I.; Czako, G.; Wester, R. Influence of the leaving group on the dynamics of a gas-phase SN2 reaction. Nat. Chem. 2016, 8, 151–156. [Google Scholar] [CrossRef]
  47. Olasz, B.; Szabo, I.; Czako, G. High-level ab initio potential energy surface and dynamics of the F− + CH3I SN2 and proton-transfer reactions. Chem. Sci. 2017, 8, 3164–3170. [Google Scholar] [CrossRef]
  48. Szabo, I.; Czako, G. Benchmark ab Initio Characterization of the Complex Potential Energy Surface of the Cl− + CH3I Reaction. J. Phys. Chem. A 2017, 121, 5748–5757. [Google Scholar] [CrossRef]
  49. Gyori, T.; Olasz, B.; Paragi, G.; Czako, G. Effects of the Level of Electronic Structure Theory on the Dynamics of the F− + CH3I Reaction. J. Phys. Chem. A 2018, 122, 3353–3364. [Google Scholar] [CrossRef]
  50. Tasi, D.A.; Fabian, Z.; Czako, G. Benchmark ab Initio Characterization of the Inversion and Retention Pathways of the OH− + CH3Y [Y = F, Cl, Br, I] SN2 Reactions. J. Phys. Chem. A 2018, 122, 5773–5780. [Google Scholar] [CrossRef]
  51. Tasi, D.A.; Fabian, Z.; Czako, G. Rethinking the X− + CH3Y [X = OH, SH, CN, NH2, PH2; Y = F, Cl, Br, I] SN2 reactions. Phys. Chem. Chem. Phys. 2019, 21, 7924–7931. [Google Scholar] [CrossRef] [PubMed]
  52. Kerekes, Z.; Tasi, D.A.; Czako, G. SN2 Reactions with an Ambident Nucleophile: A Benchmark Ab Initio Study of the CN− + CH3Y [Y = F, Cl, Br, and I] Systems. J. Phys. Chem. A 2022, 126, 889–900. [Google Scholar] [CrossRef] [PubMed]
  53. Vermeeren, P.; Hamlin, T.A.; Bickelhaupt, F.M. Chemical reactivity from an activation strain perspective. Chem. Commun. 2021, 57, 5880–5896. [Google Scholar] [CrossRef] [PubMed]
  54. Zhao, S.; Fu, G.; Zhen, W.; Wang, H.; Liu, M.; Yang, L.; Zhang, J. Nucleophile Effects on the E2/SN2 Competition for the X− + CH3CH2Cl Reactions: A Theoretical Study. J. Phys. Chem. A 2023, 127, 3381–3389. [Google Scholar] [CrossRef]
  55. Lu, T.; Chen, F. Multiwfn: A Multifunctional Wavefunction Analyzer. J. Comput. Chem. 2012, 33, 580–592. [Google Scholar] [CrossRef]
  56. Kim, C.K.; Li, H.G.; Lee, B.-S.; Kim, C.K.; Lee, H.W.; Lee, I. Gas-Phase Identity Nucleophilic Substitution Reactions of Cyclopropenyl Halides. J. Org. Chem. 2002, 67, 1953–1960. [Google Scholar] [CrossRef]
  57. Ren, Y.; Yamataka, H. The α-Effect in Gas-Phase SN2 Reactions: Existence and the Origin of the Effect. J. Org. Chem. 2007, 72, 5660–5667. [Google Scholar] [CrossRef]
  58. Chen, Y.; Chang, K.H.; Meng, F.Y.; Tseng, S.M.; Chou, P.T. Broadening the Horizon of the Bell–Evans–Polanyi Principle towards Optically Triggered Structure Planarization. Angew. Chem. Int. Ed. 2021, 60, 7205–7212. [Google Scholar] [CrossRef]
  59. Head-Gordon, M.; Pople, J.A. MP2 Eenergy Evaluation By Direct Methods. Chem. Phys. Lett. 1988, 153, 503–506. [Google Scholar] [CrossRef]
  60. Frisch, M.J.; Head-Gordon, M.; Pople, J.A. Semi-Direct Algorithms for the MP2 Energy and Gradient. Chem. Phys. Lett. 1990, 166, 281–289. [Google Scholar] [CrossRef]
  61. Dunning, T.H. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 1989, 90, 1007–1023. [Google Scholar] [CrossRef]
  62. Woon, D.E.; Dunning, T.H. Gaussian basis sets for use in correlated molecular calculations. III. The atoms aluminum through argon. J. Chem. Phys. 1993, 98, 1358–1371. [Google Scholar] [CrossRef]
  63. Wadt, W.R.; Hay, P.J. Ab initio effective core potentials for molecular calculations. Potentials for main group elements Na to Bi. J. Chem. Phys. 1985, 82, 284–298. [Google Scholar] [CrossRef]
  64. Zhang, L.; Yang, L.; Zhao, Y.; Zhang, J.; Feng, D.; Sun, S. Effects of Water Molecule on CO Oxidation by OH: Reaction Pathways, Kinetic Barriers, and Rate Constants. J. Phys. Chem. A 2017, 121, 4868–4880. [Google Scholar] [CrossRef]
  65. Szucs, T.; Czako, G. Benchmark ab initio potential energy surface mapping of the F− + CH3NH2 reaction. Phys. Chem. Chem. Phys. 2022, 24, 20249. [Google Scholar] [CrossRef]
  66. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Montgomery, J.A.; Scalmani, G.; Barone, V.; Mennucci, B.; et al. Gaussian 09; Revision A.01; Gaussian Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
Molecules 28 06269 sch001
Scheme 1. Pathways for reactants F− + RY (R= CH3, C2H5, iC3H7, tC4H9,represented in red; Y = Cl, I, represented in blue).
Scheme 1. Pathways for reactants F− + RY (R= CH3, C2H5, iC3H7, tC4H9,represented in red; Y = Cl, I, represented in blue).
Molecules 28 06269 sch001
Molecules 28 06269 g001
Figure 1. Potential energy curves and stationary points at MP2/aug-cc-pVDZ level for F− + RCl reactions. The energy (in kcal/mol) is relative to F− + CH3Cl (a) and F− + RCl (b) reactants at 0 K, and does not include ZPE. In (b), the black, blue, and pink numbers represent F− + C2H5Cl, F− + iC3H7Cl, and F− + tC4H9Cl reactions, respectively.
Figure 1. Potential energy curves and stationary points at MP2/aug-cc-pVDZ level for F− + RCl reactions. The energy (in kcal/mol) is relative to F− + CH3Cl (a) and F− + RCl (b) reactants at 0 K, and does not include ZPE. In (b), the black, blue, and pink numbers represent F− + C2H5Cl, F− + iC3H7Cl, and F− + tC4H9Cl reactions, respectively.
Molecules 28 06269 g001
Molecules 28 06269 g002
Figure 2. Schematic potential energy surface (PES) of the F− + RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I) with stationary points along the anti-E2 (black) and inv-SN2 (red) channels at MP2/aug-cc-pVDZ(ECP/d) level. The reported energies (in kcal/mol) are relative to reactants without ZPE.
Figure 2. Schematic potential energy surface (PES) of the F− + RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I) with stationary points along the anti-E2 (black) and inv-SN2 (red) channels at MP2/aug-cc-pVDZ(ECP/d) level. The reported energies (in kcal/mol) are relative to reactants without ZPE.
Molecules 28 06269 g002
Molecules 28 06269 sch002
Scheme 2. Schematic of activation barrier (∆E0‡), overall barrier (∆E‡), and reaction enthalpy ∆H.
Scheme 2. Schematic of activation barrier (∆E0‡), overall barrier (∆E‡), and reaction enthalpy ∆H.
Molecules 28 06269 sch002
Molecules 28 06269 g003
Figure 3. (a) Activation barrier heights ∆E0‡ (full line) and overall barrier heights (dashed line) of anti-E2 and inv-SN2 transition states as a function of methyl substitution degree n of Cα (n = 0–3). (b) Barrier difference ∆∆E0‡ between anti-E2 and inv-SN2 as a function of n (n = 1–3). Values of Y = Cl are reported. All the energies are in kcal/mol. (c) Energy decomposition analysis of the anti-E2 and inv-SN2 transition structure between F− + (CH3)nCCl (n = 0–3).
Figure 3. (a) Activation barrier heights ∆E0‡ (full line) and overall barrier heights (dashed line) of anti-E2 and inv-SN2 transition states as a function of methyl substitution degree n of Cα (n = 0–3). (b) Barrier difference ∆∆E0‡ between anti-E2 and inv-SN2 as a function of n (n = 1–3). Values of Y = Cl are reported. All the energies are in kcal/mol. (c) Energy decomposition analysis of the anti-E2 and inv-SN2 transition structure between F− + (CH3)nCCl (n = 0–3).
Molecules 28 06269 g003
Molecules 28 06269 g004
Figure 4. (a) Comparison of activation barriers between inv-SN2 and anti-E2 TS in the F−+ RY reaction calculated by MP2 method. The correlation of (b) activation barrier heights ∆E0‡ and reaction enthalpy ∆H, (c) activation barrier difference ∆∆E0‡ and reaction enthalpy ∆∆H between Y = Cl and I, and (d) activation barrier heights and electronegativity of leaving group Y.
Figure 4. (a) Comparison of activation barriers between inv-SN2 and anti-E2 TS in the F−+ RY reaction calculated by MP2 method. The correlation of (b) activation barrier heights ∆E0‡ and reaction enthalpy ∆H, (c) activation barrier difference ∆∆E0‡ and reaction enthalpy ∆∆H between Y = Cl and I, and (d) activation barrier heights and electronegativity of leaving group Y.
Molecules 28 06269 g004
Table 1. The relative energies (kcal/mol) for stationary points of the E2 and SN2 pathways on the F− + RY with different methods.
Table 1. The relative energies (kcal/mol) for stationary points of the E2 and SN2 pathways on the F− + RY with different methods.
ClI
RSpeciesMP2CCSD(T) aExptl cMP2CCSD(T) bExptl c
CH31dRCH16.917.6 −18.9−20.3
1TSRC−15.1−15.8 −15.8−17.6
1bRC−15.6−16.1 −16.2−18.3
1bTS−12.3−12.8 −15.6−18.1
1dTS32.130.7 22.818.3
1dPC−40.7−41.6 −49.6−55.3
1bPC−40.7−41.6 −49.6−55.3
1P2−30.6−31.8 −31.1−40.9−48.0−42.3
C2H52c/2dRCH−17.8−18.0 −18.7−19.6
2a/2bRC−17.5−17.7 −19.4−20.1
2aTS−11.2−11.1 −15.8−16.0
2bTS−11−11.3 −15.4−16.9
2cTS−0.1−6.8 −4.2−4.9
2dTS31.130.0 21.519.2
2aPC−31.9−33.6 −41.5−42.7
2bPC−42.4−44.6 −51.8−50.9
2cPC−44.2−41.6 −49.0−54.0
2dPC−42.4−44.6 −51.8−54.0
2P1−15.5−18.1 −22.5−26.3−26.2−37.8
2P2−31.3−34.0 −32.0−42.1−44.0−48.7
iC3H73a/3bRC−20.7−21.4 −21.9−22.8
3c/3dRC−15.5−16.3 −16.9−17.9
3aTS−11.6−12.4 −16.9−17.5
3bTS−9.1−11.0 −14.1−16.3
3cTS0.3−1.0 −4.3−5.4
3dTS31.528.8 21.4-
3aPC−31.8−33.8 −41.8−44.4
3bPC−43.9−46.9 −54.0−57.9
3cPC−44.4−47.3 −48.8−52.8
3dPC−44.4−47.3 −54.0-
3P1−13.9−16.5 −22.1−24.8−28.4−37.7
3P2−31.8−34.9 −30.3−42.8−46.8−45.9
tC4H94a/4bRC−23.1−23.3 −24.4−25.6
4c/4dRC−15.6−16.1 −17.2−17.8
4aTS−11.9−17.6 −17.7−24.4
4bTS−2.3- −7.5-
4cTS0.8−4.6 −3.9−10.8
4dTS39.9- 28.8-
4aPC−33.4−37.8 −43.4−51.2
4bPC−46.2- −56.1-
4cPC−44.0−49.1 −49.1−57.4
4dPC−46.2- −56.1-
4P1−12.6−18.2 −22.1−23.9−37.6−37.6
4P2−31.9-−29.5−43.3-−37.8
a (1) Energies with respect to the F− + CH3Cl reactants at AE-CCSD(T)/aug-cc-pCVQZ level are from ref. [41]. (2) Energies for F− +C2H5Cl reaction are obtained at the CCSD(T)-F12b/aug-cc-pVQZ + Δcore level of theory from ref. [16]. (3) Energies for F− + i-C3H7Cl reaction are calculated at CCSD(T)/aug-cc-pVTZ//MP2/aug-cc-pVDZ level of theory. (4) Energies For F− + t-C4H9Cl reaction obtained at B97-1/aug-cc-pVDZ level are from ref. [43]. b (1) Energies of F− + CH3I reaction at CCSD(T)-F12b/aug-cc-pVTZ-pp are from ref. [47]. (2) Energies of F− + C2H5I reaction at CCSD(T)/PP/t level are from ref. [17]. (3) Energies for F− + i-C3H7I reaction are calculated at CCSD(T)/pp/t//MP2/ECP/d level of theory. (4) Energies For F− + t-C4H9I reaction obtained at B97-1/aug-cc-pVDZ level are from ref. [43]. c The reaction enthalpies of reaction at 0 K with ZPE calculated from tabulated reaction enthalpies in ref. [41].
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Zhen, W.; Zhao, S.; Fu, G.; Wang, H.; Sun, J.; Yang, L.; Zhang, J. Effects of Methyl Substitution and Leaving Group on E2/SN2 Competition for Reactions of F− with RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I). Molecules 2023, 28, 6269. https://doi.org/10.3390/molecules28176269

AMA Style

Zhen W, Zhao S, Fu G, Wang H, Sun J, Yang L, Zhang J. Effects of Methyl Substitution and Leaving Group on E2/SN2 Competition for Reactions of F− with RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I). Molecules. 2023; 28(17):6269. https://doi.org/10.3390/molecules28176269

Chicago/Turabian Style

Zhen, Wenqing, Siwei Zhao, Gang Fu, Hongyi Wang, Jianmin Sun, Li Yang, and Jiaxu Zhang. 2023. "Effects of Methyl Substitution and Leaving Group on E2/SN2 Competition for Reactions of F− with RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I)" Molecules 28, no. 17: 6269. https://doi.org/10.3390/molecules28176269

APA Style

Zhen, W., Zhao, S., Fu, G., Wang, H., Sun, J., Yang, L., & Zhang, J. (2023). Effects of Methyl Substitution and Leaving Group on E2/SN2 Competition for Reactions of F− with RY (R = CH3, C2H5, iC3H7, tC4H9; Y = Cl, I). Molecules, 28(17), 6269. https://doi.org/10.3390/molecules28176269

Article Metrics

Back to TopTop