Time Evolution of Plasmonic Features in Pentagonal Ag Clusters
Abstract
1. Introduction
2. Theory
2.1. Real-Time Propagation
2.2. Electron Dynamics Descriptors
2.2.1. Time-Dependent TCM
2.2.2. Time-Dependent Induced Density
3. Computational Details
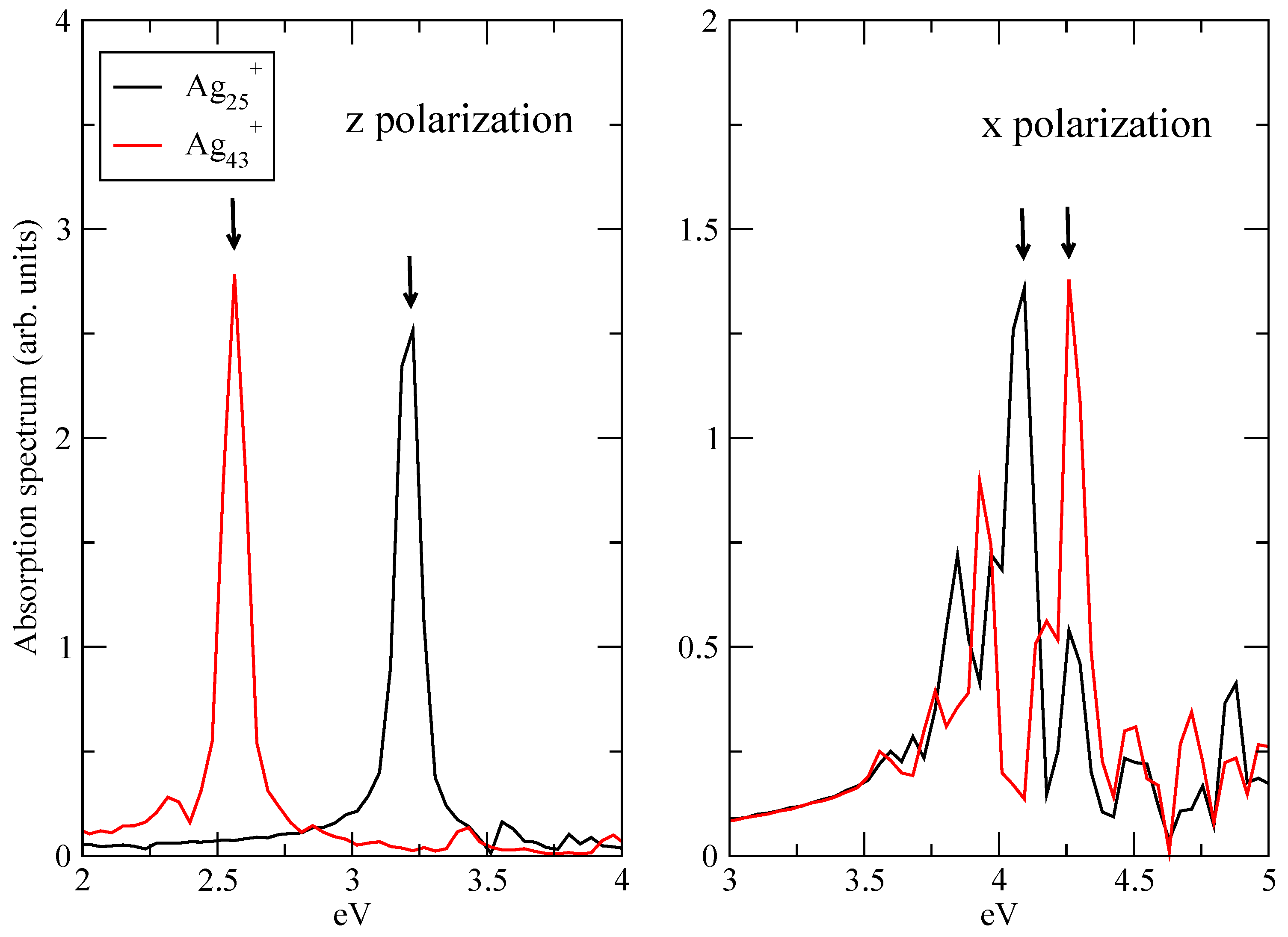
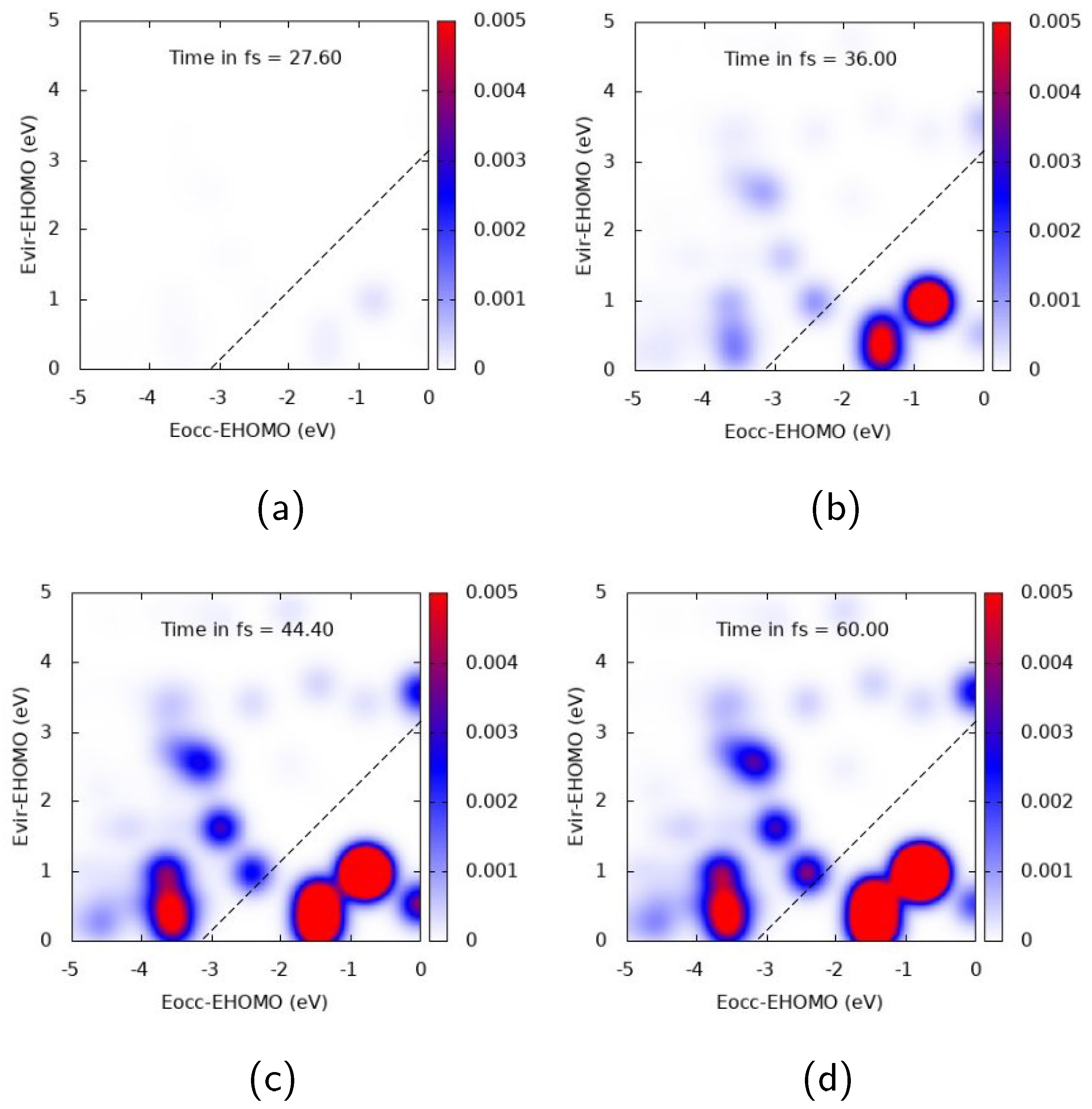
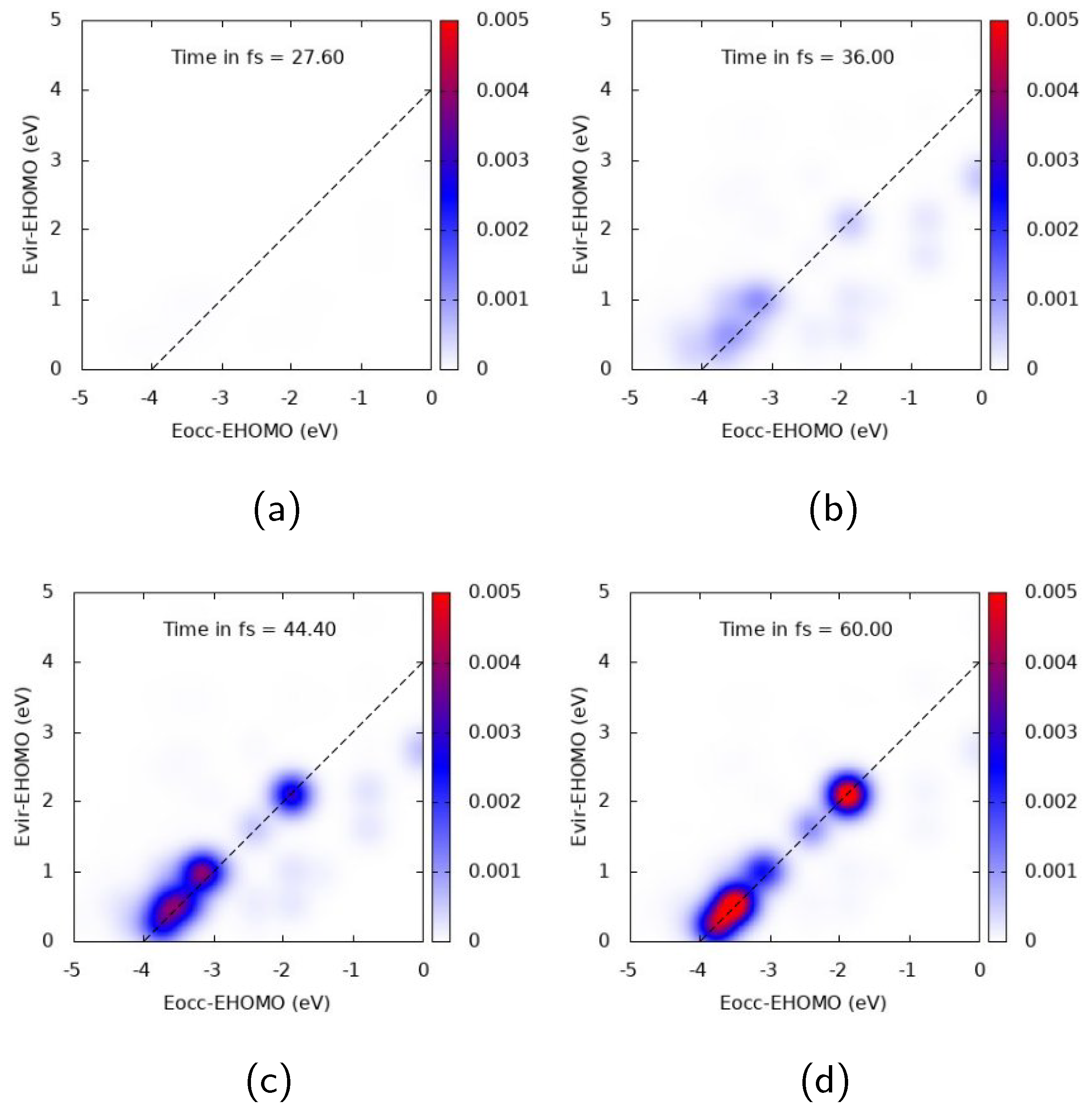
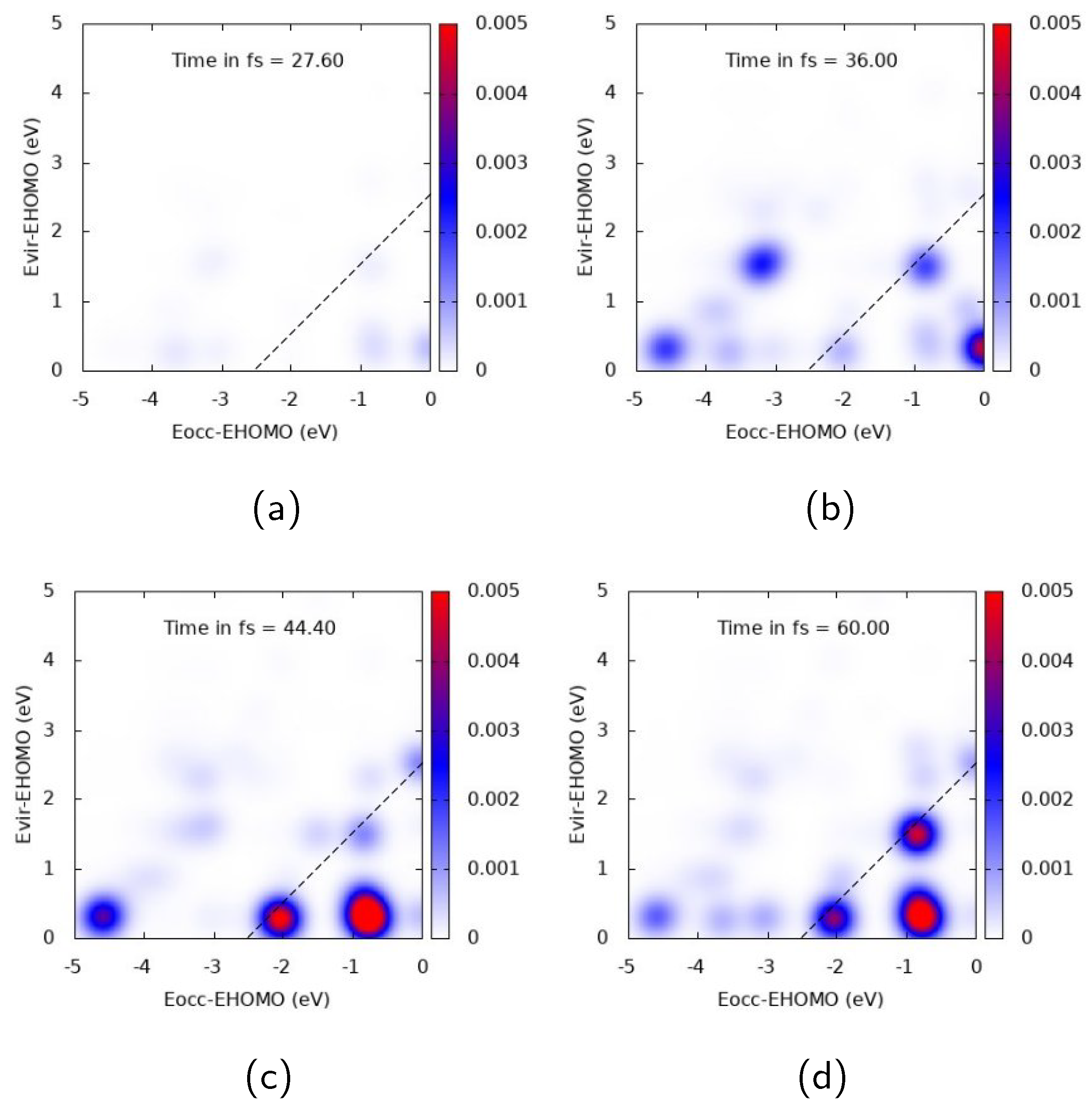
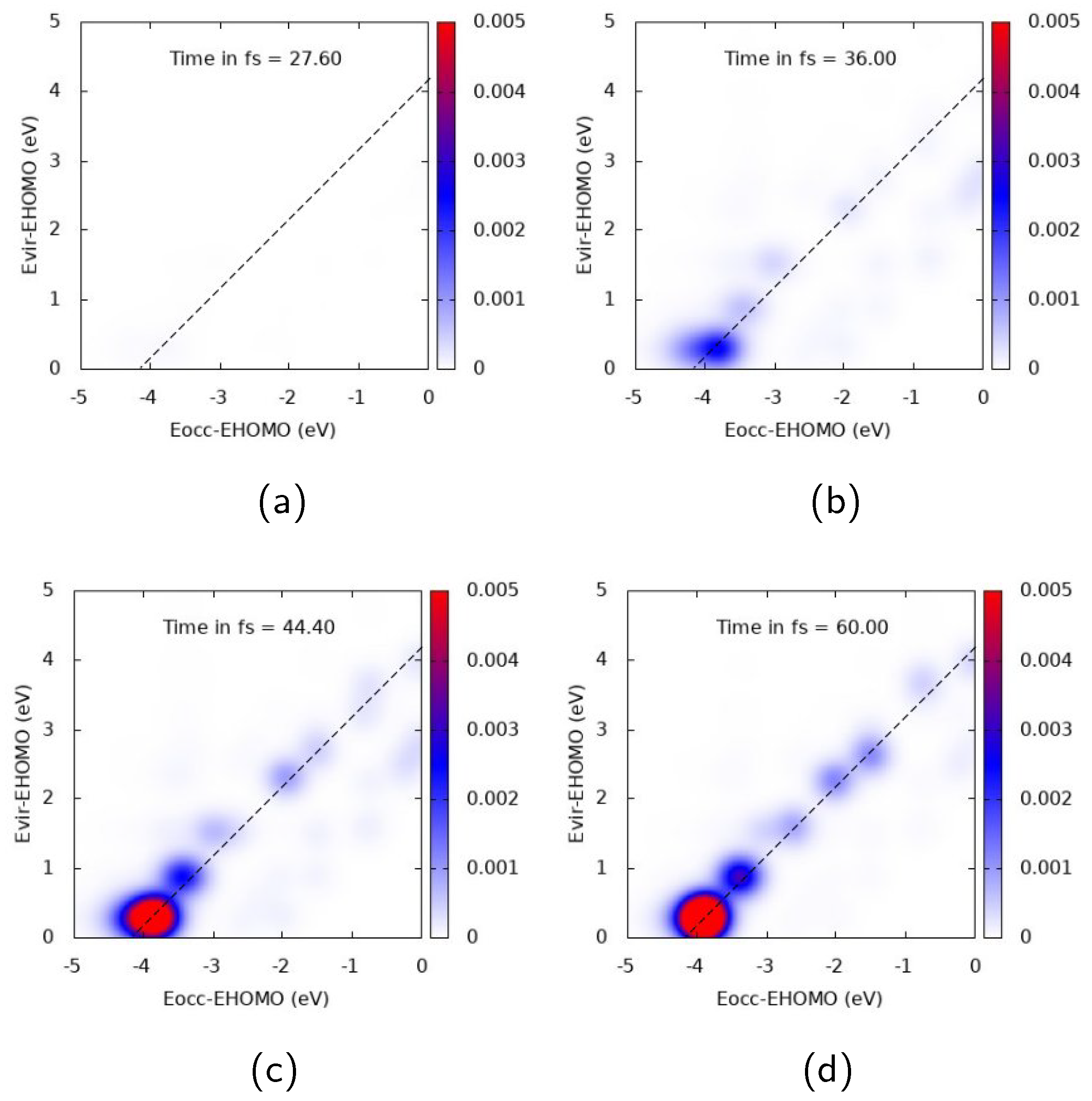
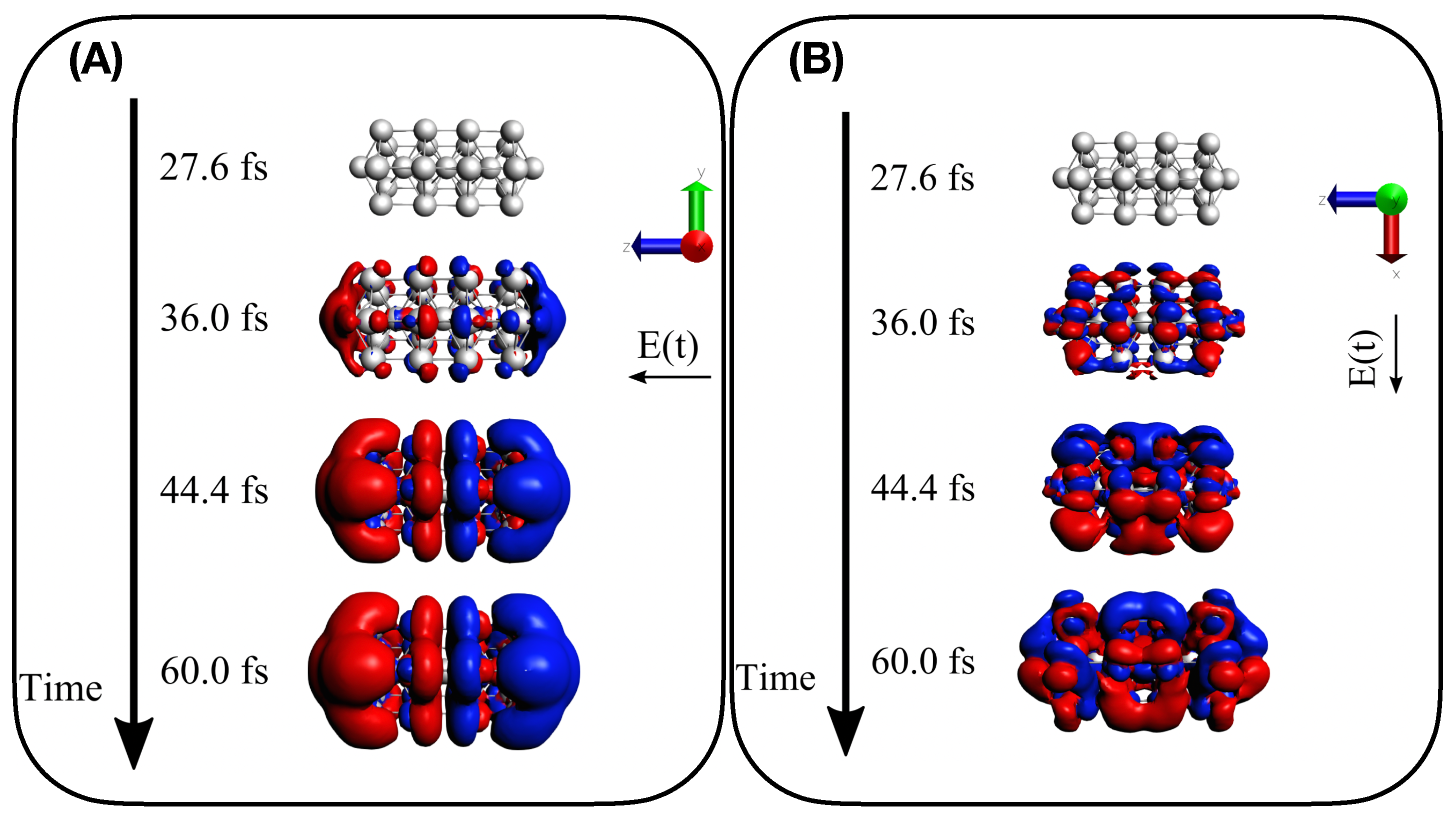
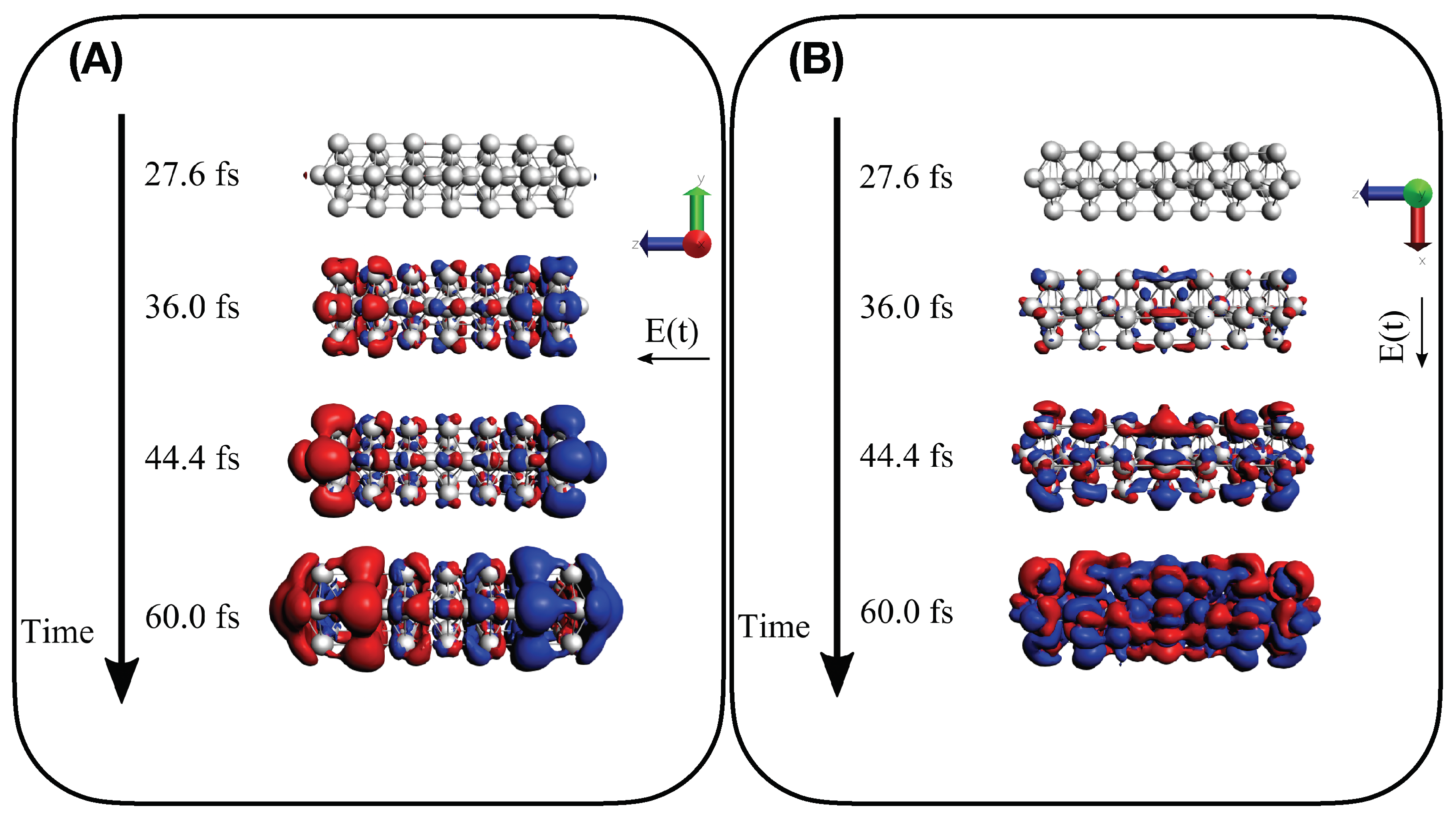
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ma, Y.Y.; Li, W.Y.; Cho, E.C.; Li, Z.Y.; Yu, T.K.; Zeng, J.; Xie, Z.X.; Xia, Y.N. Au@Ag core-shell nanocubes with finely tuned and well-controlled sizes, shell thicknesses, and optical properties. ACS Nano 2010, 4, 6725. [Google Scholar] [CrossRef]
- Del Fatti, N.; Christofilos, D.; Vallée, F. Optical response of a single gold nanoparticle. Gold Bull. 2008, 41, 147. [Google Scholar] [CrossRef][Green Version]
- Chen, H.; McMahon, J.M.; Ratner, M.A.; Schatz, G.C. Classical Electrodynamics Coupled to Quantum Mechanics for Calculation of Molecular Optical Properties: A RT-TDDFT/FDTD Approach. J. Phys. Chem. C 2010, 114, 14384. [Google Scholar] [CrossRef]
- Rycenga, M.; Cobley, C.M.; Zeng, J.; Li, W.; Moran, C.H.; Zhang, Q.; Din, Q.; Xia, Y. Controlling the Synthesis and Assembly of Silver Nanostructures for Plasmonic Applications. Chem. Rev. 2011, 111, 3669. [Google Scholar] [CrossRef]
- Yu, Y.Y.; Chang, S.S.; Lee, C.L.; Wang, C.R.C. Gold Nanorods: Electrochemical Synthesis and Optical Properties. J. Phys. Chem. B 1997, 101, 6661. [Google Scholar] [CrossRef]
- Link, S.; Mohamed, M.B.; El-Sayed, M.A. Simulation of the Optical Absorption Spectra of Gold Nanorods as a Function of Their Aspect Ratio and the Effect of the Medium Dielectric Constant. J. Phys. Chem. B 1999, 103, 3073. [Google Scholar] [CrossRef]
- Kelly, K.L.; Coronado, E.; Zhao, L.L.; Schatz, G.C. The Optical Properties of Metal Nanoparticles: The Influence of Size, Shape, and Dielectric Environment. J. Phys. Chem. B 2002, 107, 668. [Google Scholar] [CrossRef]
- Burda, C.; Chen, X.; Narayanan, R.; El-Sayed, M.A. Chemistry and Properties of Nanocrystals of Different Shapes. Chem. Rev. 2005, 105, 1025. [Google Scholar] [CrossRef]
- Marimuthu, A.; Zhang, J.W.; Linic, S. Tuning Selectivity in Propylene Epoxidation by Plasmon Mediated Photo-Switching of Cu Oxidation State. Science 2013, 339, 1590. [Google Scholar] [CrossRef]
- Zhang, J.Z. Biomedical Applications of Shape-Controlled Plasmonic Nanostructures: A Case Study of Hollow Gold Nanospheres for Photothermal Ablation Therapy of Cancer. J. Phys. Chem. Lett. 2010, 1, 686. [Google Scholar] [CrossRef]
- Heo, M.; Cho, H.; Jung, J.W.; Jeong, J.R.; Park, S.; Kim, J.Y. High-performance organic optoelectronic devices enhanced by surface plasmon resonance. Adv. Mater. 2011, 23, 5689. [Google Scholar] [CrossRef]
- Kreibig, K.; Vollmer, M. Optical Properties of Metal Clusters; Springer: New York, NY, USA, 1995. [Google Scholar]
- Ye, E.; Win, K.Y.; Tan, H.R.; Lin, M.; Teng, C.P.; Mlayah, A.; Han, M.Y. Plasmonic Gold Nanocrosses with Multidirectional Excitation and Strong Photothermal Effect. J. Am. Chem. Soc. 2011, 133, 8506. [Google Scholar] [CrossRef]
- Murray, W.A.; Suckling, J.R.; Barnes, W.L. Overlayers on Silver Nanotriangles: Field Confinement and Spectral Position of Localized Surface Plasmon Resonances. Nano Lett. 2006, 6, 1772. [Google Scholar] [CrossRef]
- Casida, M.E. Time-Dependent Density Functional Response Theory for Molecules. In Recent Advances in Density Functional Methods; Chong, D.P., Ed.; World Scientific: Singapore, 1995; Part I; p. 155. [Google Scholar]
- Aikens, C.M.; Li, S.; Schatz, G.C. From Discrete Electronic States to Plasmons: TDDFT Optical Absorption Properties of Ag n (n = 10, 20, 35, 56, 84, 120) Tetrahedral Clusters. J. Phys. Chem. C 2008, 112, 11272. [Google Scholar] [CrossRef]
- Durante, N.; Fortunelli, A.; Broyer, M.; Stener, M. Optical Properties of Au Nanoclusters from TD-DFT Calculations. J. Phys. Chem. C 2011, 115, 6277. [Google Scholar] [CrossRef]
- Bae, G.T.; Aikens, C.M. Time-Dependent Density Functional Theory Studies of Optical Properties of Ag Nanoparticles: Octahedra, Truncated Octahedra, and Icosahedra. J. Phys. Chem. C 2012, 116, 10356. [Google Scholar] [CrossRef]
- Johnson, H.E.; Aikens, C.M. Electronic Structure and TDDFT Optical Absorption Spectra of Silver Nanorods. J. Phys. Chem. A 2009, 113, 4445. [Google Scholar] [CrossRef]
- Guidez, E.B.; Aikens, C.M. Diameter dependence of the excitation spectra of silver and gold nanorods. J. Phys. Chem. C 2013, 117, 12325. [Google Scholar] [CrossRef]
- Piccini, G.; Havenith, R.W.A.; Broer, R.; Stener, M. Gold nanowires: A time-dependent density functional assessment of plasmonic behavior. J. Phys. Chem. C 2013, 117, 17196. [Google Scholar] [CrossRef]
- Liao, M.-S.; Bonifassi, P.; Leszczynski, J.; Ray, P.; Huang, M.; Watts, J. Structure, bonding, and linear optical properties of a series of silver and gold nanorod clusters: DFT/TDDFT studies. J. Phys. Chem. A 2010, 114, 12701. [Google Scholar]
- Mayer, A.; Gonzales, A.L.; Aikens, C.M.; Schatz, G.C. A charge–dipole interaction model for the frequency-dependent polarizability of silver clusters. Nanotechnology 2009, 20, 195204. [Google Scholar] [CrossRef]
- Barcaro, G.; Sementa, L.; Fortunelli, A.; Stener, M. Optical properties of Ag nanoshells from TDDFT calculations. J. Phys. Chem. C 2014, 118, 12450. [Google Scholar] [CrossRef]
- Baseggio, O.; De Vetta, M.; Fronzoni, G.; Stener, M.; Sementa, L.; Fortunelli, A.; Calzolari, A. Photoabsorption of Icosahedral Noble Metal Clusters: An Efficient TDDFT Approach to Large-Scale Systems. J. Phys. Chem. C 2016, 120, 12773. [Google Scholar] [CrossRef]
- Asadi-Aghbolaghi, N.; Rüger, R.; Jamshidi, Z.; Visscher, L. TD-DFT+TB: An Efficient and Fast Approach for Quantum Plasmonic Excitations. J. Phys. Chem. C 2020, 124, 7946. [Google Scholar] [CrossRef]
- D’Agostino, S.; Della Sala, F.; Andreani, L.C. Dipole-excited surface plasmons in metallic nanoparticles: Engineering decay dynamics within the discrete-dipole approximation. Phys. Rev. B 2013, 87, 205413. [Google Scholar] [CrossRef]
- Andreussi, O.; Corni, S.; Mennucci, B.; Tomasi, J. Radiative and nonradiative decay rates of a molecule close to a metal particle of complex shape. J. Chem. Phys. 2004, 121, 10190. [Google Scholar] [CrossRef]
- Caricato, M.; Andreussi, O.; Corni, S. Semiempirical (ZINDO-PCM) Approach to Predict the Radiative and Nonradiative Decay Rates of a Molecule Close to Metal Particles. J. Phys. Chem. B 2006, 110, 16652. [Google Scholar]
- Vukovic, S.; Corni, S.; Mennucci, B. Fluorescence Enhancement of Chromophores Close to Metal Nanoparticles. Optimal Setup Revealed by the Polarizable Continuum Model. J. Phys. Chem. C 2009, 113, 121. [Google Scholar] [CrossRef]
- Mennucci, B.; Corni, S. Multiscale modelling of photoinduced processes in composite systems. Nat. Rev. Chem. 2019, 3, 315. [Google Scholar] [CrossRef]
- Coccia, E.; Fregoni, J.; Guido, C.A.; Marsili, M.; Pipolo, S.; Corni, S. Hybrid theoretical models for molecular nanoplasmonics. J. Chem. Phys. 2020, 153, 200901. [Google Scholar] [CrossRef]
- Gersten, J.; Nitzan, A. Spectroscopic properties of molecules interacting with small dielectric particles. J. Chem. Phys. 1981, 75, 1139. [Google Scholar] [CrossRef]
- Van Duyne, R.P. Molecular plasmonics. Science 2004, 306, 985. [Google Scholar] [CrossRef]
- Novotny, L.; Hecht, B. Principles of Nano-Optics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Wilson, A.J.; Willets, K.A. Molecular Plasmonics. Annu. Rev. Anal. Chem. 2016, 9, 27. [Google Scholar] [CrossRef]
- Bursi, L.; Calzolari, A.; Corni, S.; Molinari, E. Light-induced field enhancement in nanoscale systems from first-principles: The case of polyacenes. ACS Photonics 2014, 1, 1049. [Google Scholar] [CrossRef]
- Adam Lauchner and Andrea, E. Schlather and Alejandro Manjavacas and Yao Cui and Michael J. McClain and Grant J. Stec and García de Abajo, F. Javier and Peter Nordlander and Naomi J. Halas. Molecular Plasmonics. Nano Lett. 2015, 15, 6208. [Google Scholar]
- Baseggio, O.; De Vetta, M.; Fronzoni, G.; Stener, M.; Fortunelli, A. A new time-dependent density-functional method for molecular plasmonics: Formalism, implementation, and the Au144(SH)60 case study. Int. J. Quant. Chem. 2016, 116, 1603. [Google Scholar] [CrossRef]
- Zhang, R.; Bursi, L.; Cox, J.D.; Cui, Y.; Krauter, C.M.; Alabastri, A.; Manjavacas, A.; Calzolari, A.; Corni, S.; Molinari, E. How to identify plasmons from the optical response of nanostructures. ACS Nano 2017, 11, 7321. [Google Scholar] [CrossRef]
- D’Agostino, S.; Rinaldi, R.; Cuniberti, G.; Della Sala, F. Density Functional Tight Binding for Quantum Plasmonics. J. Phys. Chem. C 2018, 122, 19756. [Google Scholar] [CrossRef]
- Guerrini, M.; Calzolari, A.; Varsano, D.; Corni, S. Quantifying the Plasmonic Character of Optical Excitations in a Molecular J-Aggregate. J. Chem. Theory Comput. 2019, 15, 3197. [Google Scholar] [CrossRef]
- Müller, M.M.; Kosik, M.; Pelc, M.; Bryant, G.W.; Ayuela, A.; Rockstuhl, C.; Słowik, K. Energy-Based Plasmonicity Index to Characterize Optical Resonances in Nanostructures. J. Phys. Chem. C 2020, 124, 24331. [Google Scholar] [CrossRef]
- Langford, J.; Xu, X.; Yang, Y. Plasmon Character Index: An Accurate and Efficient Metric for Identifying and Quantifying Plasmons in Molecules. J. Phys. Chem. Lett. 2021, 12, 9391. [Google Scholar] [CrossRef]
- Yabana, K.; Bertsch, G.F. Time-dependent local-density approximation in real time. Phys. Rev. B 1996, 54, 4484. [Google Scholar] [CrossRef]
- Rossi, T.P.; Kuisma, M.; Puska, M.J.; Nieminen, R.M.; Erhart, P. Kohn-Sham Decomposition in Real-Time Time-Dependent Density-Functional Theory: An Efficient Tool for Analyzing Plasmonic Excitations. J. Chem. Theory Comput. 2017, 13, 4779. [Google Scholar] [CrossRef]
- Rossi, T.P.; Erhart, P.; Kuisma, M. Hot-Carrier Generation in Plasmonic Nanoparticles: The Importance of Atomic Structure. ACS Nano 2020, 14, 9963. [Google Scholar] [CrossRef]
- Provorse, M.R.; Isborn, C.M. Electron dynamics with real-time time-dependent density functional theory. Int. J. Quantum Chem. 2016, 739, 116. [Google Scholar] [CrossRef]
- Li, X.; Govind, N.; Isborn, C.; DePrince III, A.E.; Lopata*, K. Real-Time Time-Dependent Electronic Structure Theory. Chem. Rev. 2020, 120, 9951. [Google Scholar] [CrossRef]
- Makkonen, E.; Rossi, T.P.; Larsen, A.H.; Lopez-Acevedo, O.; Rinke, P.; Kuisma, M.; Chen, X. Real-time time-dependent density functional theory implementation of electronic circular dichroism applied to nanoscale metal–organic clusters. J. Chem. Phys. 2021, 154, 114102. [Google Scholar] [CrossRef]
- Sanchez, C.G.; Berdakin, M. Plasmon-Induced Hot Carriers: An Atomistic Perspective of the First Tens of Femtoseconds. J. Phys. Chem. C 2022, 126, 10015. [Google Scholar] [CrossRef]
- Ilawe, N.V.; Oviedo, M.B.; Wong, B.M. Real-Time Quantum Dynamics of Long-Range Electronic Excitation Transfer in Plasmonic Nanoantennas. J. Chem. Theory Comput. 2017, 13, 3442. [Google Scholar] [CrossRef]
- Liu, Z.; Oviedo, M.B.; Wong, B.M.; Aikens, C.M. Plasmon-induced excitation energy transfer in silver nanoparticle dimers: A real-time TDDFTB investigation. J. Chem. Phys. 2022, 156. [Google Scholar] [CrossRef]
- Witt, W.C.; del Rio, B.G.; Dieterich, J.M.; Carter, E.A. Orbital-free density functional theory for materials research. J. Mater. Res. 2018, 33, 777–795. [Google Scholar] [CrossRef]
- Della Sala, F. Orbital-free methods for plasmonics: Linear response. J. Chem. Phys. 2022, 157, 104101. [Google Scholar] [CrossRef] [PubMed]
- Grobas Illobre, P.; Marsili, M.; Corni, S.; Stener, M.; Toffoli, D.; Coccia, E. Time-resolved excited-state analysis of molecular electron dynamics by TDDFT and Bethe-Salpeter equation formalisms. J. Chem. Theory Comput. 2021, 17, 6314. [Google Scholar] [CrossRef] [PubMed]
- Lu, T.; Chen, F. Multiwfn: A multifunctional wavefunction analyzer. J. Comput. Chem. 2012, 33, 580. [Google Scholar] [CrossRef] [PubMed]
- Guido, C.A.; Cortona, P.; Mennucci, B.; Adamo, C. On the Metric of Charge Transfer Molecular Excitations: A Simple Chemical Descriptor. J. Chem. Theory Comput. 2013, 9, 3118. [Google Scholar] [CrossRef] [PubMed]
- Guido, C.A.; Cortona, P.; Adamo, C. Effective electron displacements: A tool for time-dependent density functional theory computational spectroscopy. J. Chem. Phys. 2014, 140, 104101. [Google Scholar] [CrossRef]
- Pohl, V.; Hermann, G.; Tremblay, J.C. An open-source framework for analyzing N-electron dynamics. I. Multideterminantal wave functions. J. Comput. Chem. 2017, 38, 1515. [Google Scholar] [CrossRef]
- Hermann, G.; Pohl, V.; Tremblay, J.C. An open-source framework for analyzing N-electron dynamics. II. Hybrid density functional theory/configuration interaction methodology. J. Comput. Chem. 2017, 38, 2378. [Google Scholar] [CrossRef]
- Lu, T.; Chen, Q. Ultrastrong Regulation Effect of the Electric Field on the All-Carboatomic Ring Cyclo[18]Carbon. ChemPhysChem 2020, 22, 386. [Google Scholar] [CrossRef]
- Liu, Z.; Lu, T.; Chen, Q. Intermolecular interaction characteristics of the all-carboatomic ring, cyclo[18]carbon: Focusing on molecular adsorption and stacking. Carbon 2021, 171, 514. [Google Scholar] [CrossRef]
- Shang, C.; Cao, Y.; Sun, C.; Zhao, H. Theoretical study on an intriguing excited-state proton transfer process induced by weakened intramolecular hydrogen bonds. Phys. Chem. Chem. Phys. 2022, 24, 8453. [Google Scholar] [CrossRef]
- Shang, C.; Sun, C. Substituent effects on photophysical properties of ESIPT-based fluorophores bearing the 4-diethylaminosalicylaldehyde core. J. Mol. Liq. 2022, 367, 120477. [Google Scholar] [CrossRef]
- Zhang, Y.; Shang, C.; Cao, Y.; Sun, C. Quantum mechanics/molecular mechanics studies on the photoprotection mechanisms of three chalcones. J. Mol. Liq. 2023, 372, 121165. [Google Scholar] [CrossRef]
- Kuisma, M.; Sakko, A.; Rossi, T.P.; Larsen, A.H.; Enkovaara, J.; Lehtovaara, L.; Rantala, T.T. Localized surface plasmon resonance in silver nanoparticles: Atomistic first-principles time-dependent density-functional theory calculations. Phys. Rev. B-Condens. Matter Mater. Phys. 2015, 91, 1–8. [Google Scholar] [CrossRef]
- Kumar, P.V.; Rossi, T.P.; Kuisma, M.; Erhart, P.; Norris, D.J. Direct hot-carrier transfer in plasmonic catalysis. Faraday Discuss. 2019, 214, 189. [Google Scholar] [CrossRef] [PubMed]
- Pipolo, S.; Corni, S.; Cammi, R. The cavity electromagnetic field within the polarizable continuum model of solvation: An application to the real-time time dependent density functional theory. Comput. Theor. Chem. 2014, 1040–1041, 112–119. [Google Scholar] [CrossRef]
- Pipolo, S.; Corni, S. Real-Time Description of the Electronic Dynamics for a Molecule Close to a Plasmonic Nanoparticle. J. Phys. Chem. C 2016, 120, 28774. [Google Scholar] [CrossRef]
- Dall’Osto, G.; Gil, G.; Pipolo, S.; Corni, S. Real-time dynamics of plasmonic resonances in nanoparticles described by a boundary element method with generic dielectric function. J. Chem. Phys. 2020, 153, 184114. [Google Scholar] [CrossRef]
- Malola, S.; Lehtovaara, L.; Enkovaara, J.; Häkkinen, H. Birth of the Localized Surface Plasmon Resonance in Monolayer-Protected Gold Nanoclusters. ACS Nano 2013, 7, 10263. [Google Scholar] [CrossRef]
- Rüger, R.; Franchini, M.; Trnka, T.; Yakovlev, A.; van Lenthe, E.; Philipsen, P.; van Vuren, T.; Klumpers, B.; Soini, T. AMS 2022.1, SCM, Theoretical Chemistry; Vrije Universiteit: Amsterdam, The Netherlands, 2022. [Google Scholar]
- Ernzerhof, M.; Scuseria, G.E. Assessment of the Perdew–Burke–Ernzerhof exchange-correlation functional. J. Chem. Phys. 1999, 110, 5029–5036. [Google Scholar] [CrossRef]
- Monti, M.; Stener, M.; Coccia, E. Electronic circular dichroism from real-time propagation in state space. J. Chem. Phys. 2023, 158, 084102. [Google Scholar] [CrossRef]
- Theivendran, S.; Chang, L.; Mukherjee, A.; Sementa, L.; Stener, M.; Fortunelli, A.; Dass, A. Principles of Optical Spectroscopy of Aromatic Alloy Nanomolecules: Au36−xAgx(SPh-tBu)24. J. Phys. Chem. C 2018, 122, 4524. [Google Scholar] [CrossRef]
- Ma, J.; Wang, Z.; Wang, L.W. Interplay between plasmon and single-particle excitations in a metal nanocluster. Nat. Commun. 2015, 6, 10107. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, D.; Meng, W.; Li, S.; Meng, S. Plasmon-Induced Water Splitting on Ag-Alloyed Pt Single-Atom Catalysts. Front. Chem. 2021, 9, 742794. [Google Scholar] [CrossRef] [PubMed]
- Kuda-Singappulige, G.U.; Lingerfelt, D.B.; Li, X.; Aikens, C.M. Ultrafast Nonlinear Plasmon Decay Processes in Silver Nanoclusters. J. Phys. Chem. C 2020, 124, 20477. [Google Scholar] [CrossRef]
- Mittal, R.; Glenn, R.; Saytashev, I.; Lozovoy, V.V.; Dantus, M. Femtosecond Nanoplasmonic Dephasing of Individual Silver Nanoparticles and Small Clusters. J. Phys. Chem. Lett. 2015, 6, 1638. [Google Scholar] [CrossRef]
- Coccia, E.; Troiani, F.; Corni, S. Probing quantum coherence in ultrafast molecular processes: An ab initio approach to open quantum systems. J. Chem. Phys. 2018, 148, 204112. [Google Scholar] [CrossRef]
- Coccia, E.; Corni, S. Role of coherence in the plasmonic control of molecular absorption. J. Chem. Phys. 2019, 151, 044703. [Google Scholar] [CrossRef]
- Dall’Osto, G.; Coccia, E.; Guido, C.A.; Corni, S. Investigating ultrafast two-pulse experiments on single DNQDI fluorophores: A stochastic quantum approach. Phys. Chem. Chem. Phys. 2020, 22, 16734. [Google Scholar] [CrossRef] [PubMed]
- Vanzan, M.; Marsili, M.; Corni, S. Study of the Rate-Determining Step of Rh Catalyzed CO2 Reduction: Insight on the Hydrogen Assisted Molecular Dissociation. Catalysts 2021, 11, 538. [Google Scholar] [CrossRef]
- Ezendam, S.; Herran, M.; Nan, L.; Gruber, C.; Kang, Y.; Gröbmeyer, F.; Lin, R.; Gargiulo, J.; Sousa-Castillo, A.; Cortés, E. Hybrid Plasmonic Nanomaterials for Hydrogen Generation and Carbon Dioxide Reduction. ACS Energy Lett. 2022, 7, 778. [Google Scholar] [CrossRef] [PubMed]
- Martirez, J.M.P.; Bao, J.L.; Carter, E.A. First-Principles Insights into Plasmon-Induced Catalysis. Annu. Rev. Phys. Chem. 2021, 72, 99. [Google Scholar] [CrossRef] [PubMed]
- Swearer, D.F.; Zhao, H.; Zhou, L.; Zhang, C.; Robatjazi, H.; Martirez, J.M.P.; Krauter, C.M.; Yazdi, S.; McClain, M.J.; Ringe, E.; et al. Heterometallic antenna-reactor complexes for photocatalysis. Proc. Natl. Acad. Sci. USA 2016, 113, 8916. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Domenis, N.; Grobas Illobre, P.; Marsili, M.; Stener, M.; Toffoli, D.; Coccia, E. Time Evolution of Plasmonic Features in Pentagonal Ag Clusters. Molecules 2023, 28, 5671. https://doi.org/10.3390/molecules28155671
Domenis N, Grobas Illobre P, Marsili M, Stener M, Toffoli D, Coccia E. Time Evolution of Plasmonic Features in Pentagonal Ag Clusters. Molecules. 2023; 28(15):5671. https://doi.org/10.3390/molecules28155671
Chicago/Turabian StyleDomenis, Nicola, Pablo Grobas Illobre, Margherita Marsili, Mauro Stener, Daniele Toffoli, and Emanuele Coccia. 2023. "Time Evolution of Plasmonic Features in Pentagonal Ag Clusters" Molecules 28, no. 15: 5671. https://doi.org/10.3390/molecules28155671
APA StyleDomenis, N., Grobas Illobre, P., Marsili, M., Stener, M., Toffoli, D., & Coccia, E. (2023). Time Evolution of Plasmonic Features in Pentagonal Ag Clusters. Molecules, 28(15), 5671. https://doi.org/10.3390/molecules28155671






