Next Article in Journal
Quercitrin Is a Novel Inhibitor of Salmonella enterica Serovar Typhimurium Type III Secretion System
Previous Article in Journal
Phenolics Extracted from Jasminum sambac Mitigates Diabetic Cardiomyopathy by Modulating Oxidative Stress, Apoptotic Mediators and the Nfr-2/HO-1 Pathway in Alloxan-Induced Diabetic Rats
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 28
Issue 14
10.3390/molecules28145454
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Computational Investigation of Conformational Properties of Short Azapeptides: Insights from DFT Study and NBO Analysis

by
Mouna El Khabchi
1,*,
Mohammed Mcharfi
1,
Mohammed Benzakour
1,
Asmae Fitri
1,
Adil Touimi Benjelloun
1,
Jong-Won Song
2,
Kang-Bong Lee
3 and
Ho-Jin Lee
4,*
1
LIMAS, Department of Chemistry, Faculty of Sciences Dhar El Mahraz, Sidi Mohamed Ben Abdallah University, Fez 30000, Morocco
2
Department of Chemistry Education, Daegu University, Daegudae-ro 201, Gyeongsan-si 38453, Republic of Korea
3
Climate and Environmental Research Institute, Korea Institute of Science & Technology, Hwarang-ro 14-gil 5, Seoul 02792, Republic of Korea
4
Department of Natural Sciences, Southwest Tennessee Community College, Memphis, TN 38134, USA
*
Authors to whom correspondence should be addressed.
Molecules 2023, 28(14), 5454; https://doi.org/10.3390/molecules28145454
Submission received: 9 June 2023 / Revised: 5 July 2023 / Accepted: 12 July 2023 / Published: 17 July 2023
(This article belongs to the Topic Theoretical, Quantum and Computational Chemistry)
Browse Figures
Review Reports Versions Notes

Abstract

:
Azapeptides have gained much attention due to their ability to enhance the stability and bioavailability of peptide drugs. Their structural preferences, essential to understanding their function and potential application in the peptide drug design, remain largely unknown. In this work, we systematically investigated the conformational preferences of three azaamino acid residues in tripeptide models, Ac-azaXaa-Pro-NHMe [Xaa = Asn (4), Asp (5), Ala (6)], using the popular DFT functionals, B3LYP and B3LYP-D3. A solvation model density (SMD) was used to mimic the solvation effect on the conformational behaviors of azapeptides in water. During the calculation, we considered the impact of the amide bond in the azapeptide models on the conformational preferences of models 4–6. We analyzed the effect of the HB between the side-chain main chain and main-chain main-chain on the conformational behaviors of azapeptides 4–6. We found that the predicted lowest energy conformation for the three models differs depending on the calculation methods. In the gas phase, B3LYP functional indicates that the conformers tttANP-1 and tttADP-1 of azapeptides 4 and 5 correspond to the type I of β-turn, the lowest energy conformation with all-trans amide bonds. Considering the dispersion correction, B3LYP-D3 functional predicts the conformers tctANP-2 and tctADP-3 of azapeptide 4 and 5, which contain the cis amide bond preceding the Pro residue, as the lowest energy conformation in the gas phase. The results imply that azaAsx and Pro residues may involve cis-trans isomerization in the gas phase. In water, the predicted lowest energy conformer of azapeptides 4 and 5 differs from the gas phase results and depends on the calculational method. For azapeptide 6, regardless of calculation methods and phases, tttAAP-1 (β-I turn) is predicted as the lowest energy conformer. The results imply that the effect of the side chain that can form HBs on the conformational preferences of azapeptides 4 and 5 may not be negligible. We compared the theoretical results of azaXaa-Pro models with those of Pro-azaXaa models, showing that incorporating azaamino acid residue in peptides at different positions can significantly impact the folding patterns and stability of azapeptides.

Graphical Abstract

1. Introduction

Peptides are promising tools with biological and pharmaceutical applications [1,2]. However, their low metabolic stability because of enzymatic degradation, lack of receptor selectivity, and many other drawbacks made it necessary to search for alternatives with the same function as natural peptides but with better properties [3]. These alternatives are called peptidomimetics, in which some structural modifications involve peptides’ backbone or side chains [3,4]. Among peptidomimetics, we focus on azapeptides, a change in the backbone of a peptide by substituting the α-carbon with a nitrogen atom [5,6,7]. This modification confers azapeptides more resistance to physiological degradation and long duration of action [8]. Many studies revealed the importance of incorporating an azaamino acid in the peptide’s sequence to enhance the stability and bioavailability of drug candidates [9,10,11,12,13,14]. In addition, synthetic methods [5,9,11] and biological studies [5,8,9,12,13,14] of azapeptides have been reported. However, the structural behaviors of azaamino acid residue in peptides remain limited.
The conformational preferences of azaamino acid residues have been studied theoretically [15,16,17,18,19,20,21] and experimentally [22,23,24,25,26,27,28,29,30,31]. The results showed that the preferred dihedral angles of the backbone of these azaamino acid residues are in the range of ϕ = ±90° ± 30°, ψ = 0° or ±180° ± 30°, which is appeared to be the polyproline II (βP), and β-turn motif (αR, αL, δR, or δL conformation) [15,16,17,18,19]. The nomenclature was denoted by Karplus et al. [32]. Our groups and others examined the effect of azaamino acid residue on the structures of peptides [33,34,35,36,37,38]. For example, the azaglycine adopted the polyproline II (βP) in azaGly-Pro-Hyp (hydroxyproline) of the collagen [39]; the δL conformer (ϕ = 90° ± 30°, ψ = 0° ± 30°) in Ac-Phe-azaGly-NH2, forming the βII-turn structure; the αR or αL conformer (ϕ = ±90° ± 30°, ψ = 0°) in Ac-Aib-azaGly-NH2, adopting βI or βI′-turn [40]. Meanwhile, incorporating azaamino acids destabilized the β sheet secondary structure compared to the parent peptide [41]. The results showed that the conformational preference of azaamino acid residue in peptides should be sequence dependence. However, it is still largely unknown of these questions: why azaamino acid residue adopts a specific conformation in the context dependence, the influence of the side chain of azaamino acid residue on its backbone structure, and the neighboring effect on the conformational behaviors of azapeptides. As a part of the efforts to answer these questions, we previously examined the conformational properties of tripeptides, Ac-Pro-azaXaa-NHMe [Xaa = Asn (1), Asp (2), Ala (3)] using the DFT method, showing that the azaamino acid in this sequence, regardless of the side chain, adopts βII (βII′) turn structure [21]. Additionally, we also suggested the importance of the side-chain and main-chain or main-chain and main-chain HBs found in these model peptides on the stability of the azapeptides. In this work, we extended our ongoing efforts in understanding conformational properties of short azapeptides containing three azaamino acid residues, Ac-azaXaa-Pro-NHMe [Xaa = Asn (4), Asp (5), and Ala (6)] (Figure 1). While previous theoretical studies focused on the dipeptide models [14], we wondered about the position effect of azaamino acid residue in tripeptides. We used the popular DFT functionals, B3LYP and B3LYP-D3 [42], to compare our previous work [21] in the gas phase and water. The solvation effect was calculated with the SMD method [43]. We also investigated the influence of the cis-trans isomerization amide bonds on the conformational preferences of model peptides.
Intramolecular HB interactions found in the molecules in the gas phase (Figure 2) were also inspected using Natural Bond Orbital (NBO) theory at the two levels of theory. The strength of the HB in azapeptides models was estimated using the second-order perturbation analysis (E(2) value) [44].

2. Results and Discussion

The backbone (ϕ and ψ) dihedral angles of the resulting structures for the three azapeptides 4–6 are denoted by the nomenclature given by Karplus [32] (Supporting Information Tables S1–S12). Further, the side chain of azaAsn and azaAsp are marked g+(gauche+), g−(gauche−), s+(skew+), and s−(skew−) based on the values of χ1′ [45]. Relative energies of the most stable conformations of the three model compounds 4–6 calculated at the B3LYP/6-311++G(d, p) and B3LYP-D3/6-311++G(d, p) levels of theory in the gas phase and water are listed in Table 1 and Table 2.

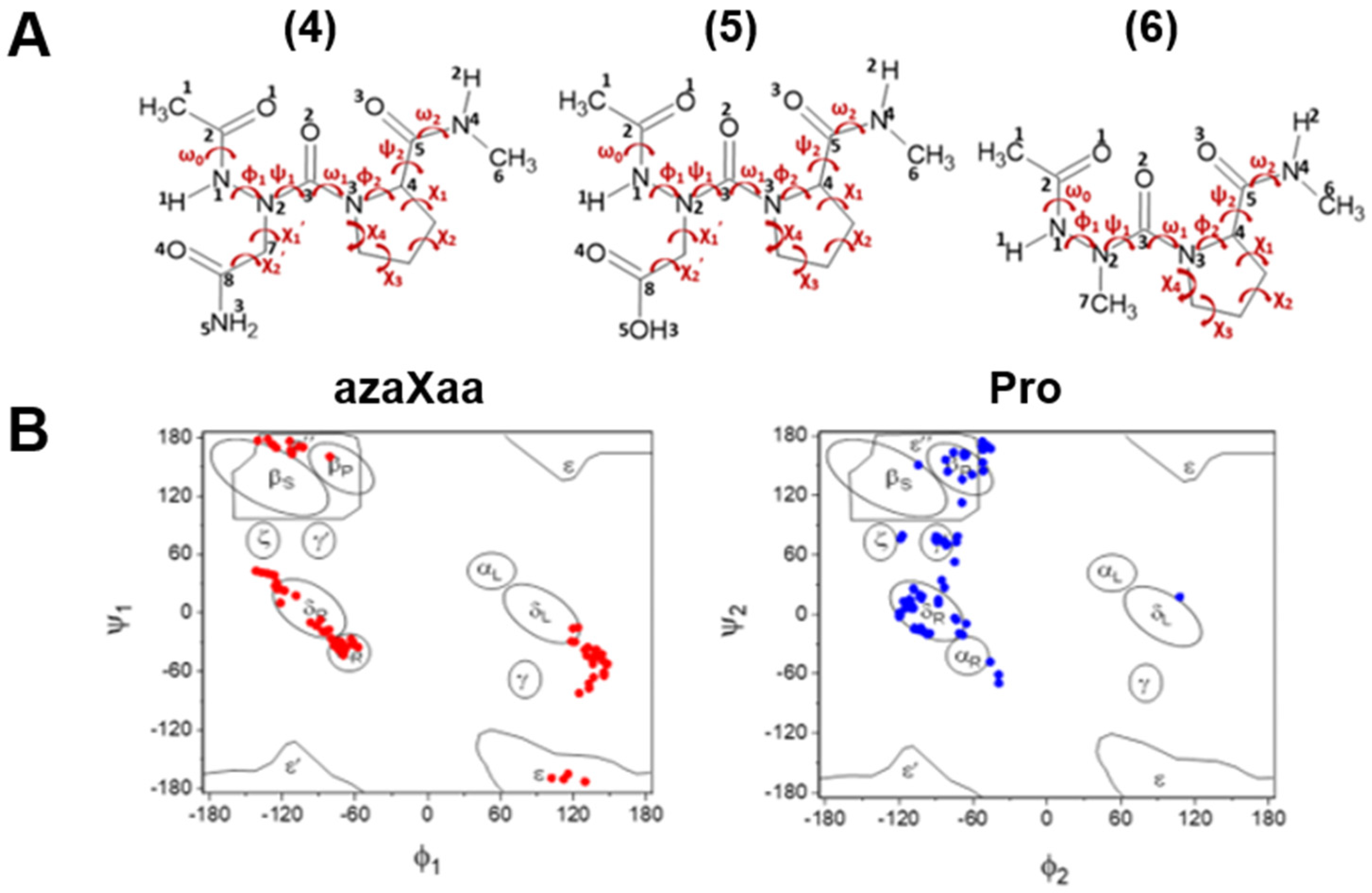
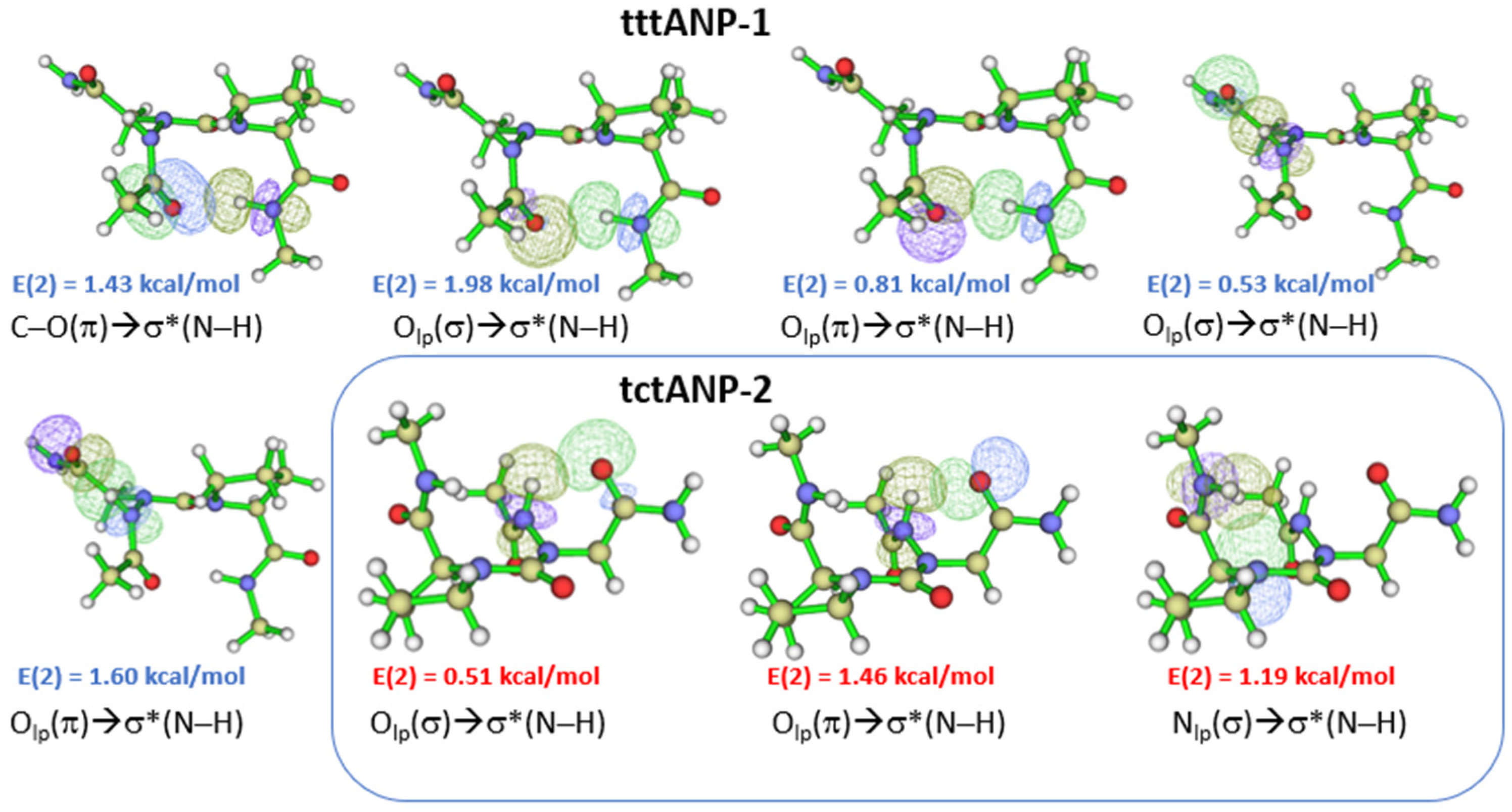
2.1. Cis-Trans Isomerization for Minimum Energy Conformations

Ac-azaAsn-Pro-NHMe (4): The conformer tttANP-1 (αRδR(g+)), corresponding to the type I of β-turn, and has a trans-trans orientation of the amide bond preceding both residues, was found as the lowest energy minimum for azaAsn model in the gas phase calculated with the B3LYP method. However, the same conformation is the second energy minimum at the B3LYP-D3 functional with a relative energy of 0.94 kcal/mol. Interestingly, the lowest energy minimum conformer of azapeptide 4 calculated at the B3LYP functional is found in the X-ray structure containing azaAsn-Pro sequence, showing the presence of a β-Ⅰ turn with all-trans amide bonds [46,47]. In inclusion of dispersion correction, B3LYP-D3 functional predicts tctANP-2 as the lowest energy minimum containing a cis amide bond between azaAsn-Pro sequence, which is also predicted to be the most stable conformer in water (Table 2). The second lowest energy minimum in solution calculated with B3LYP functional is tttANP-12 (δLβP(s+)) with a relative energy of 0.94 kcal/mol. This relative energy value was also found for the second energy minimum calculated with B3LYP-D3 functional. Another thing to highlight is the trans amide bond preceding both Pro and azaAsn found for the five lowest energy minima calculated with the B3LYP functional in the isolated state, except for the second energy minimum in which the amide bond preceding azaAsn is cis-oriented (Table 1). However, most of the lowest energy minimum conformers calculated with the B3LYP-D3 functional in the gas phase are cis-oriented amide bonds preceding either the two residues or at least one. In the solution state, the five lowest energy minima with B3LYP-D3 functional are all trans amide bonds except for the first and the fifth conformers, which have a cis amide bond preceding azaAsn residue. The theoretical results suggest that the azaAsn-Pro sequence may also be involved in cis-trans isomerization in water.
Ac-azaAsp-Pro-NHMe (5): The results of Ac-azaAsp-Pro-NHMe are similar to those of the AzaAsn model. The B3LYP functional predicted tttADP-1 (αRδR(g+)) with all-trans amide bonds as the lowest energy minimum in the gas phase. The second most stable conformation, tttADP-2 (αRγ’(g+)) (ΔE = 1.00 kcal/mol), is also oriented to all-trans amide bonds. However, B3LYP-D3 functional predicts the conformer tctADP-3 as the lowest energy minimum and the conformer tctADP-4 as the second energy minimum. The results imply that the dispersion interaction may be essential in stabilizing the cis amide bond in the azaAsp-Pro sequence. Contrary to the isolated state, both SMD/B3LYP and SMD/B3LYP-D3 functionals predict the conformer tttADP-9 (δLβP(s+)) as the lowest energy conformer (Table 2). Similarly, the second most stable conformation in water with the two methods is tttADP-7, with all-trans amide bonds. However, the third lowest energy minimum tctADP-3 (βδR(g+)) (ΔE = 1.07 kcal/mol) has a cis orientation of amide bond preceding Pro residue. The results also imply that the azaAsp-Pro sequence may be involved in cis-trans isomerization in water.
Ac-azaAla-Pro-NHMe (6): The results of azapeptide 6 significantly differ from those of azapeptides 4 and 5. Both B3LYP and B3LYP-D3 functionals predict that the conformer tttAAP-1 (αRδR), related to the β-Ⅰ turn structure, is the lowest energy minimum in the gas and water. In addition, the B3LYP function predicts that the four most stable conformers are trans-trans amide bonds in the gas phase. However, the third and the fourth energy minimum have trans-cis amide bonds with B3LYP-D3 functional. The two most stable conformations calculated by SMD/B3LYP in water have all trans amide bonds.
In contrast, the most stable conformation calculated by SMD/B3LYP-D3 was found to be all trans amide bonds followed by two trans-cis-trans or cis-trans-trans conformers (Table 2). The results imply that the side chain of azaAsn and azaAsp may play a vital role in cis-trans isomer.

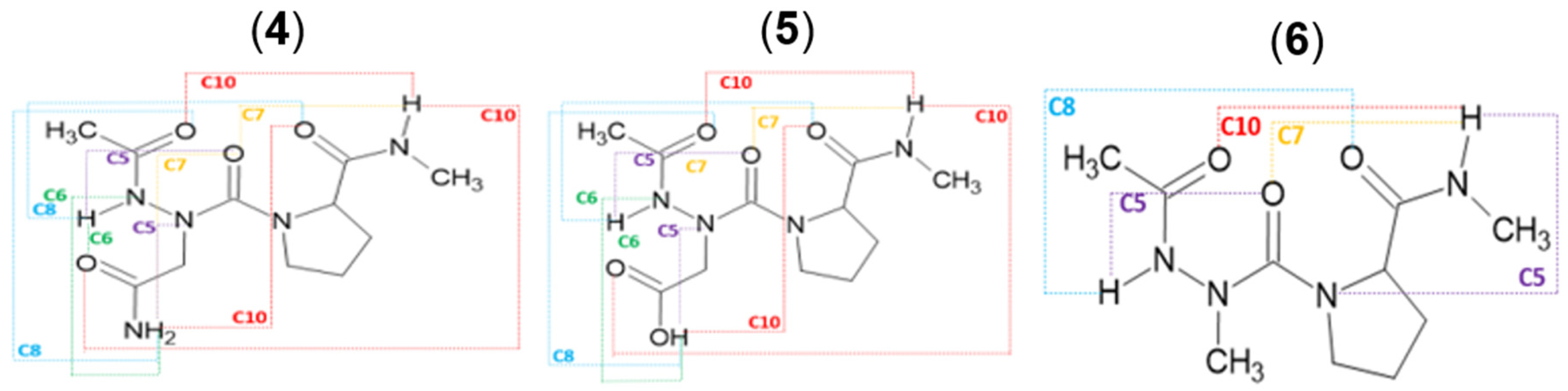
2.2. Hydrogen Bonds in Ac-azaXaa-Pro-NHMe

Optimized structures of Ac-azaXaa-Pro-NHMe are presented with the denotation of HBs (Supporting Information Tables S1–S12 and Figures S1–S12). NBO orbital interactions corresponding to HBs are depicted in the Supporting Information Tables S13–S17, calculated with the B3LYP method. We used the E(2) value to describe the strength of the HB [44].
The most stable conformation in the gas phase at the B3LYP method is tttANP-1, which is stabilized with two intramolecular HBs closing C10 and C6 pseudo cycles between carbonyl and N–H groups C2=O1…H2–N4 and C8=O4…H1-N1, respectively (Figure 3). The C10 pseudo cycle is an HB interaction related to the β-Ⅰ turn, which is found in the X-ray structure of Z-azaAsn(Me)-Pro-NHiPr [47]. The HB is made up of three interactions as follows: πCO → σ*NH of 1.43 kcal/mol, nO(σ) → σ*NH of 1.98 kcal/mol, and n′O(π) → σ*NH of 0.81 kcal/mol (Figure 3). The sum ΣEHB of the individual E(2) stabilization energies of the three HB interactions is 4.22 kcal/mol. It is not the strongest interaction, yet tttANP-1 is the most stable conformation. Moreover, the strongest interaction is that related to the C8 pseudo cycle in tctANP-7 conformation in which the sum of the individual E(2) stabilization energies, associated with πCO, nO, and n′O/σ*NH overlaps involved in the C.O…HN HB is 9.34 kcal/mol. The same result was found with the B3LYP-D3 method (Supporting Information Table S16) since the tctANP-7 conformation has the highest value of the sum of E(2) (9.98 kcal/mol) for the C8 HB pseudocycle. However, the most stable conformation, tttANP-1, has a sum value of 5.39 kcal/mol related to the C10 pseudocycle. We notice a slight increase in the values of the sum of the individual E(2) energies calculated with B3LYP-D3 compared to those found with B3LYP.
The opposite conclusions are drawn in water; the SMD/B3LYP method predicted the tttANP-12 (δLβP) conformer to be the lowest energy minimum in water. This conformer is stabilized with one HB closing a C8 pseudocycle (Supporting Information Figure S7) between the C=O of the main chain and the N-H of the azaAsn side chain. The length of the HB is calculated to be 1.92 Å. Unlike the gas phase, results in water showed that the second most stable conformation is the conformer tttANP-1, stabilized with an HB interaction closing a C10 pseudocycle, and the HB length is equal to 2.30 Å. We observed the absence of the C6 pseudocycle found in the gas phase for the tttANP-1 conformer. Additionally, the HBs length becomes longer than that in the gas phase.
Unlike the B3LYP functional, the B3LYP-D3 functional predicted the conformer tctANP-2 to be the lowest energy minimum in gas and solution states. This conformer is stabilized with two intramolecular HBs in the gas phase. One is a backbone-side chain HB closing a C6 pseudocycle, and a backbone HB closing a C5 ring, with the length of HBs estimated to be 2.26 Å for both interactions. The C6 ring was not observed in the solution for this same conformer. However, the length of the C5 HB was slightly larger than that found in the gas phase (2.33 Å). Our result indicates that the side chain of azaAsn in solution may be involved in an intermolecular interaction with water.
The results of compound (5) showed few similarities with those of compound (4). The most stable conformer in the gas phase with the B3LYP function is tttADP-1, with two HBs interactions closing C10 and C6 rings. The C10 HB is related to the βⅠ turn motif for which the sum of the individual E(2) stabilization energies (Supporting Information Table S14), associated with πCO, nO, and n′O/σ*NH overlaps (Figure 4) involved in the CO…HN HB is 3.71 kcal/mol. This turn motif was also found in the X-ray structure of Z-azaAsp(OEt)-Pro-NhiPr [47]. Comparing these results with those observed with B3LYP-D3 functional, we see clearly that the dispersion-corrected B3LYP-D3 functional might not predict the lowest energy minimum; since it is tctADP-3 conformer with one HB closing a C5 pseudocycle (Supporting Information Figure S5) with a sum of E(2) is 1.22 kcal/mol related to the n′N → σ*NH NBO overlap of the NH……N backbone HB [48] (Figure 4).
The tttADP-9 conformer was found as the most stable conformation in water with SMD/B3LYP and SMD/B3LYP-D3 methods. This conformer is stabilized with one HB interaction, a C=O…H–O backbone-side chain HB. We also notice that the length of this type of HB is slightly larger with the dispersion-corrected functional (1.75 Å) than that found with the traditional B3LYP functional (1.64 Å).
The most stable conformation of compound (3) in the gas phase with and without dispersion functional is tttAAP-1, which is stabilized with an H.B. closing a C10 pseudocycle related to βⅠ turn (Supporting Information Figures S3 and S6). The length of such bond is predicted to be 2.16 and 2.08 Å with B3LYP and B3LYP-D3 functional, respectively. The HB length has slightly decreased from B3LYP to B3LYP-D3, contrary to azaAsn and azaAsp models. The NBO overlaps of the C10 HB are shown in Figure 5. The sum of E(2) associated with πCO, nO, and n′O/σ*NH overlaps calculated with B3LYP functional is 3.62 kcal/mol (Supporting Information Table S15). While that calculated with B3LYP-D3 functional is 4.15 kcal/mol (Supporting Information Table S18), which is only made of two πCO and nO/σ*NH overlaps.
Results of the azaAla model in the water show significant similarities with those of the gas phase. The tttAAP-1 conformer is the lowest energy minimum and stabilized with a C10 backbone C=O…H–N HB. The length of this HB is found to be 2.32 Å with B3LYP functional and 2.14 Å with B3LYP-D3 functional, which is the same finding as in the gas phase.

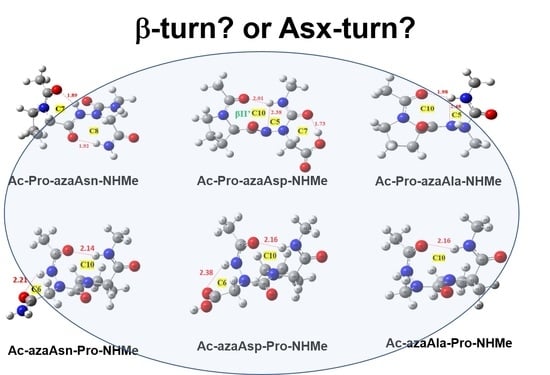
2.3. Asx Turn vs. βⅠ-Tun in Ac-azaAsx-Pro-NHMe (x = n or p)

Hydrogen-bonded Asx turns are similar to β turns since both have a C10 pseudocycle. However, in Asx turns, it is the side chain’s carbonyl group of Asn or Asp at the i + 1 position, which is hydrogen bonded to NH of the backbone of residue at the i + 3 position. Thus, the nomenclature of Asx-turns is the same as β-turns. Four categories were found: type-I, type-II, type-I′, and type-II′ Asx turn (Table 3). This nomenclature is based on the dihedral angles ϕ and ψ of residues i + 1 and i + 2. Since Asx turns are mimicry of β turns, the dihedral angles ϕe and ψe of Asx turn (Figure 6) should resemble those of i + 1 in β turns, while ϕ and ψ of residue i + 1 of Asx turns should resemble those of residue i + 2 in β turns [49,50].
Furthermore, the azaAsx-Pro chain contains another C10 pseudocycle similar to an Asx turn, which is related to the HB involving the NδH or Oδ–H of the side chain of Asn or Asp, respectively, and carbonyl group two residues ahead which will be noted (C10SC). Results of the azaAsn model compound in the gas phase calculated with the B3LYP functional showed that all three conformations adopting the βⅠ-turn motif are more stable than those adopting the Asx turn. However, the most stable Asx turn conformation tttANP-9 is only 2.89 kcal/mol higher in energy, which means there is a competition between Asx and βⅠ turn secondary structures. The C10 C=O…H–N backbone side chain HB with the sum of E(2) being 5.76 kcal/mol which is associated with nO and n′O/σ*NH overlaps (Supporting Information Table S13). On the other hand, the most stable Asx turn conformation with B3LYP-D3 functional is cctANP-17, which has a sum of E(2) value of 1.88 kcal/mol related to nO and n′O/σ*NH overlaps (Supporting Information Table S16). Moreover, the majority of βI-turn conformations are more stable than the Asx turn, and the cctANP-16 conformation related to the C10SC interaction is more stable than the Asx turn. The sum of the E(2) value (3.05 kcal/mol) related to the C10 HB of this conformer is higher than that of cccANP-17, which means a stronger HB bond interaction. The Asx turn was not reported in the azaAsn-Pro sequence yet. Instead, the Nδ–H of the side chain of azaAsn is bounded to Nα of the same residue [46,47]. Moreover, based on the dihedral angles of a typical Asx turn (Table 3), the most stable Asx turn conformation tttANP-9 (ΔE = 2.89 kcal/mol for B3LYP; ΔE = 4.52 kcal/mol for B3LYP-D3) is of type-I Asx turn. This Asx turn was also found in Asn-Pro studied with NMR and IR spectroscopies [49]. On the contrary, a study of a database of 500 proteins found that the frequency of occurrence of Asx turn is type-II′, while the most common type is βI turn [50]. These findings were also supported by a recent study of Asx turn in Asn-Ala and Asn-Gly in crystallized protein structures in the PDB [51], revealing that the most common Asx turn in these two sequences are type-II′ and type-II, respectively. For comparison, we calculated the conformational properties of Ac-Asn-Pro-NHMe (7, NP) and Ac-Asp-Pro-NHMe (8, DP) at the same levels of theories used here in the gas phase (Supporting Information Tables S19–S22). The most favorable Asn turn of model 7 is tttNP-02 (ΔE = 1.54 kcal/mol for B3LYP; ΔE = 0.58 kcal/mol for B3LYP-D3), likely type-I Asx-turn. Note that the βI-turn conformer tttNP-16 (ΔE = 5.60 kcal/mol for B3LYP; ΔE = 3.3 kcal/mol for B3LYP-D3) is predicted to have higher energy than type-I Asx turn conformer tttNP-02. We also found that the most favorable Asp turn of model 8 is tctDP-01 (ΔE = 0.00 for B3LYP and B3LYP-D3). For model 8, βII′-turn conformer tttDP-09 (ΔE = 2.51 kcal/mol for B3LYP; ΔE = 4.58 kcal/mol for B3LYP-D3) has higher energy than Asx-turn conformation tctDP-01 (Supporting Information Figures S13 and S14).
In sum, our results suggest that azaAsn and azaAsp residues in models 4 and 5 could have different folding patterns than natural peptides, and they could also enhance the formation of a type-I Asx turn instead of type-II′ mostly found in peptides. Even though the Asx turn is more stable than the C10SC, which is similar to the Asx turn, most conformers with C10SC are more stable than those adopting the Asx turn, especially with B3LYP-D3 functional. The results are comparable to models Ac-Pro-azaAsn-NHMe (1) and Ac-Pro-Asp-NHMe (2). The results revealed that the most favorable β-turn structure of azapeptide 1 is the βII(II′) turn, and that of azapeptide 2 is the βII′ turn stabilized by an HB closing a C10 cycle and two other HB cycles, C7 and C5 with B3LYP functional [15]. The results show that depending on the position of Asx residue in the sequence could induce different folding patterns.
In solution, results showed few similarities regarding the most stable Asx turn conformation with B3LYP functional, which was found to be the conformer tttANP-9. However, the tctANP-14 conformer related to the C10SC turn also has the same relative energy as tttANP-9 (∆E = 2.95 kcal/mol). The C10SC turn was found to be more stable than Asx turn in solution with the B3LYP-D3 functional since the tctANP-14 conformer adopting the C10SC turn has a relative energy of 2.07 kcal/mol and the tttANP-9 conformer related to the Asx turn is 4.20 kcal/mol energy. The result indicates that the side chain of azaAsn in the azaAsn-Pro sequence acts as a proton donor in water.
Similarly, the βI turn structure in the azaAsp-Pro sequence with B3LYP functional is more stable than the Asx turn in the isolated state. This is observed when comparing tttADP-1 and tttADP-8 conformers. The latter conformer related to the type-I Asx turn is 3.70 kcal/mol higher in energy than the first one, which adopts the βI turn. It is also noticed that the Asx turn in this sequence is less stable than that found for the azaAsn-Pro sequence. In addition, conformers cctADP-15 and tctADP-16 adopt an undefined type of Asx turn stabilized with the C10 cycle. Like the azaAsn model compound, the Asx turn in the azaAsp model compound is more stable than the C10SC motif as we compare the most stable Asx turn conformer tttADP-8 and the most stable C10SC conformer cctADP-19 (ΔE = 7.40 Kcal/mol). Additionally, most Asx turn conformations are energetically more favorable than C10SC conformations. Like the B3LYP functional, the B3LYP-D3 functional predicted that the Asx turn is more stable than the C10SC turn in the isolated state. The B3LYP-D3 functionality showed that the tctADP-16 conformer is the most stable Asx turn with a relative energy of 3.83 kcal/mol, and the sum of E(2) value is equal to 2.47 kcal/mol and associated with nO and n′O/σ*NH overlaps.
Similar results were found in the solution state since the βI turn is more stable than C10SC and Asx turn conformations. However, the C10SC is more stable than Asx turn with B3LYP functional, conformation tctADP-22 is the most stable C10SC turn conformation with a relative energy value of 5.15 kcal/mol while conformation cctADP-15 adopting the Asx turn has a relative energy value of 9.29 kcal/mol. The B3LYP-D3 functional reveals that the C10SC turn is more stable than the Asx turn, with the conformer tctADP-22 being the most stable C10SC conformer in water with a relative energy of 3.45 kcal/mol.

3. Materials and Methods

All calculations were performed using the Gaussian 09/16 programs package. The starting geometries of the three compounds were selected from the previous work [21] and were fully optimized in the gas phase at the B3LYP/6-311++G(d, p) level of theory. These geometries combine all possible intramolecular hydrogen bonds formed in the molecule between acceptor groups C=O or Nα and the N–H donor group. The orientation of amide bonds in model azapeptides, which is either cis (ω = 0°) or trans (ω = ±180°) amide bond, was also assessed. All conformations were found to be true local minima, as was indicated by the absence of imaginary frequencies. These conformations were then reoptimized using the B3LYP-D3 [42] in the gas phase using the same basis set. The solvent effect was examined using the solvation model density (SMD) [43] with and without the dispersion-correction B3LYP functional in water. For comparison, the conformational preferences of peptide models, Ac-Asn-Pro-NHMe (7, NP) and Ac-Asp-Pro-NHMe (8, DP), were investigated using the B3LYP and B3LYP-D3 functionals. The initial structures for peptides 7 and 8 were generated by conformation search implemented in Hyperchem 8.1 [52] with the molecular mechanism. The local minima with ΔE less than 10 kcal/mol were selected and then fully optimized at the B3LYP-D3/3-21G level of theory. The local minima were fully optimized at the B3LYP and B3LYP-D3 functionals with the 6-311++G(d, p) basis set. Intramolecular hydrogen bond interactions found in the molecules in the gas phase were also inspected using natural bond orbital (NBO) theory at the two levels of theory using NBO 3.1 program implemented in Gaussian 16 package [53]. The strength of the hydrogen bond in azapeptides models was estimated using the second-order perturbation analysis (E(2) value) [26]. The NBO overlaps were drawn using the Multiwfn program [54].

4. Conclusions

In summary, conformational preferences of three azapeptides models Ac-azaXaa-Pro-NHMe [Xaa = Asn, Asp, Ala] were studied using the B3LYP/6-311++G(d, p) and B3LYP-D3/6-311++G(d, p) levels of theory in isolated and water to examine the effect of side chain’s nature and position effect on the folding patterns of azapeptides. Our result demonstrates that the position of azaamino acid residue largely affects the folding patterns of these compounds. Another finding is the orientation of amide bonds preceding azaamino acid residue and Pro. The most stable conformations of all three compounds in gas and solution states calculated with B3LYP functional are all trans amide bonds. Moreover, the stability order of the three secondary structures stabilized with a 10-membered HB in azaAsn and azaAsp models is βI ˃ C10S ˃ Asx for azaAsn-Pro and azaAsp-Pro sequences in water calculated by SMD/B3LYP and SMD/B3LYP-D3, and for the zaAsp-Pro model in the gas phase also calculated by two methods. In contrast, the stability order for the azaAsn-Pro in the gas phase calculated with the two functionals is βI ˃ Asx ˃ C10SC. Note the hat azaXaa-Pro sequence prefers the βI-turn, whereas the Pro-azaXaa sequence prefers the βII-turn structure [15]. Our computational investigation of the conformational properties of short azapeptides demonstrates that the nature of the side chain of azapeptides could largely influence the folding patterns of these components.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules28145454/s1.

Author Contributions

Conceptualization, M.E.K.; data curation, M.E.K. and H.-J.L.; writing original draft, M.E.K.; writing-review and editing, M.E.K., J.-W.S., K.-B.L. and H.-J.L.; supervision, M.M. and M.B.; validation, A.T.B., A.F. and H.-J.L.; project administration and visualization, H.-J.L.; funding acquisition, J.-W.S. and K.-B.L. All authors have read and agreed to the published version of the manuscript.

Funding

J.-W.S. would like to acknowledge the partial financial support from the Korea Ministry of Science and ICT (2020R1A2C1102741) and a Daegu University Research Grant, 2023-0314. This research was supported by the Korea Institute of Science and Technology with project 2E32432 (K.-B.L.).

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The data supporting reported results can be found from one of the authors (M.E.K).

Acknowledgments

The numerical calculations were partly conducted on the Hokusai supercomputing facility of RIKEN Information Systems Division (J.-W.S.).

Conflicts of Interest

The authors declare no conflict of interest.

Sample Availability

Not applicable.

References

  1. Barman, P.; Joshi, S.; Sharma, S.; Preet, S.; Sharma, S.; Saini, A. Strategic Approaches to Improvise Peptide Drugs as Next Generation Therapeutics. Int. J. Pept. Res. Ther. 2023, 29, 61. [Google Scholar] [CrossRef]
  2. Wang, L.; Wang, N.; Zhang, W.; Cheng, X.; Yan, Z.; Shao, G.; Wang, X.; Wang, R.; Fu, C. Therapeutic peptides: Current applications and future directions. Signal Transduct. Target. Ther. 2022, 7, 48. [Google Scholar] [CrossRef]
  3. Wątły, J.; Miller, A.; Kozłowski, H.; Rowińska-Żyrek, M. Peptidomimetics–An infinite reservoir of metal binding motifs in metabolically stable and biologically active molecules. J. Inorg. Biochem. 2021, 217, 111386. [Google Scholar] [CrossRef] [PubMed]
  4. Avan, I.; Hall, C.D.; Katritzky, A.R. Peptidomimetics via modifications of amino acids and peptide bonds. Chem. Soc. Rev. 2014, 43, 3575–3594. [Google Scholar] [CrossRef] [PubMed]
  5. Cheng, K.F.; VanPatten, S.; He, M.; Al-Abed, Y. Azapeptides -A History of Synthetic Milestones and Key Examples. Curr. Med. Chem. 2022, 29, 6336–6358. [Google Scholar] [CrossRef]
  6. Zega, A. Azapeptides as pharmacological agents. Curr. Med. Chem. 2005, 12, 589–597. [Google Scholar] [CrossRef]
  7. Arujõe, M.; Järv, J.; Mastitski, A.; Ploom, A.; Troska, A. Aza-peptides: Expectations and reality. Proc. Est. Acad. Sci. 2022, 71, 241–254. [Google Scholar] [CrossRef]
  8. Härk, H.H.; Troska, A.; Arujõe, M.; Burk, P.; Järv, J.; Ploom, A. Kinetic study of aza-amino acid incorporation into peptide chains: Influence of the steric effect of the side chain. Tetrahedron 2022, 129, 133161. [Google Scholar] [CrossRef]
  9. Proulx, C.; Zhang, J.; Sabatino, D.; Chemtob, S.; Ong, H.; Lubell, W.D. Synthesis and Biomedical Potential of Azapeptide Modulators of the Cluster of Differentiation 36 Receptor (CD36). Biomedicines 2020, 8, 241. [Google Scholar] [CrossRef] [PubMed]
  10. Bourguet, C.B.; Boulay, P.L.; Claing, A.; Lubell, W.D. Design and synthesis of novel azapeptide activators of apoptosis mediated by caspase-9 in cancer cells. Bioorg. Med. Chem. Lett. 2014, 24, 3361–3365. [Google Scholar] [CrossRef]
  11. Mhidia, R.; Melnyk, O. Selective cleavage of an azaGly peptide bond by copper(II). Long-range effect of histidine residue. J. Pept. Sci. 2010, 16, 141–147. [Google Scholar] [CrossRef] [PubMed]
  12. Ludwig, C.; Desmoulins, P.O.; Driancourt, M.A.; Goericke-Pesch, S.; Hoffmann, B. Reversible downregulation of endocrine and germinative testicular function (hormonal castration) in the dog with the GnRH-agonist azagly-nafarelin as a removable implant “Gonazon”; a preclinical trial. Theriogenology 2009, 71, 1037–1045. [Google Scholar] [CrossRef] [PubMed]
  13. Ho, T.L.; Nestor, J.J., Jr.; McCrae, G.I.; Vickery, B.H. Hydrophobic, aza-glycine analogues of luteinizing hormone-releasing hormone. Int. J. Pept. Protein Res. 1984, 24, 79–84. [Google Scholar] [CrossRef]
  14. Dutta, A.S.; Furr, B.J.; Giles, M.B.; Valcaccia, B.; Walpole, A.L. Potent agonist and antagonist analogues of luliberin containing an azaglycine residue in position 10. Biochem. Biophys. Res. Commun. 1978, 81, 382–390. [Google Scholar] [CrossRef] [PubMed]
  15. Lee, H.-J.; Kim, J.H.; Jung, H.J.; Kim, K.-Y.; Kim, E.-J.; Choi, Y.-S.; Yoon, C.-J. Computational study of conformational preferences of thioamide-containing azaglycine peptides. J. Comput. Chem. 2004, 25, 169–178. [Google Scholar] [CrossRef]
  16. Lee, H.-J.; Jung, H.J.; Kim, J.H.; Park, H.-M.; Lee, K.-B. Conformational preference of azaglycine-containing dipeptides studied by PCM and IPCM methods. Chem. Phys. 2003, 294, 201–210. [Google Scholar] [CrossRef]
  17. Lee, H.; Song, J.; Choi, Y.; Ro, S.; Yoon, C. The energetically favorable cis peptide bond for the azaglycine-containing peptide: For-AzGly-NH2 model. Phys. Chem. Chem. Phys. 2001, 3, 1693–1698. [Google Scholar] [CrossRef]
  18. Kang, Y.K.; Byun, B.J. Conformational preferences and cis-trans isomerization of azaproline residue. J. Phys. Chem. B 2007, 111, 5377–5385. [Google Scholar] [CrossRef]
  19. Lee, H.J.; Song, J.W.; Choi, Y.S.; Park, H.M.; Lee, K.B. A theoretical study of conformational properties of N-methyl azapeptide derivatives. J. Am. Chem. Soc. 2002, 124, 11881–11893. [Google Scholar] [CrossRef]
  20. Lee, H.J.; Park, H.M.; Lee, K.B. The beta-turn scaffold of tripeptide containing an azaphenylalanine residue. Biophys. Chem. 2007, 125, 117–126. [Google Scholar] [CrossRef]
  21. El Khabchi, M.; Lahlou, H.; El Adnani, Z.; McHarfi, M.; Benzakour, M.; Fitri, A.; Benjelloun, A.T. Conformational preferences of Ac-Pro-azaXaa-NHMe (Xaa = Asn, Asp, Ala) and the effect of intramolecular hydrogen bonds on their stability in gas phase and solution. J. Mol. Model 2021, 27, 368. [Google Scholar] [CrossRef] [PubMed]
  22. Bowles, M.; Proulx, C. Solid phase submonomer azapeptide synthesis. Methods Enzym. 2021, 656, 169–190. [Google Scholar] [CrossRef]
  23. Dai, C.; Ma, J.; Li, M.; Wu, W.; Xia, X.; Zhang, J. Diversity-oriented submonomer synthesis of azapeptides mediated by the Mitsunobu reaction. Org. Chem. Front. 2019, 6, 2529–2533. [Google Scholar] [CrossRef]
  24. Chingle, R.M.; Proulx, C.; Lubell, W.D. Azapeptide Synthesis Methods for Expanding Side-Chain Diversity for Biomedical Applications. Acc. Chem. Res. 2017, 50, 1541–1556. [Google Scholar] [CrossRef]
  25. Doan, N.D.; Zhang, J.; Traoré, M.; Kamdem, W.; Lubell, W.D. Solid-phase synthesis of C-terminal azapeptides. J. Pept. Sci. 2015, 21, 387–391. [Google Scholar] [CrossRef]
  26. Ollivier, N.; Besret, S.; Blanpain, A.; Melnyk, O. Silver-catalyzed azaGly ligation. Application to the synthesis of azapeptides and of lipid-peptide conjugates. Bioconjug. Chem. 2009, 20, 1397–1403. [Google Scholar] [CrossRef]
  27. Bowles, M.; Proulx, C. Late-Stage N-Alkylation of Azapeptides. Org. Lett. 2022, 24, 1768–1773. [Google Scholar] [CrossRef]
  28. Luo, Z.; Xu, L.; Tang, X.; Zhao, X.; He, T.; Lubell, W.D.; Zhang, J. Synthesis and biological evaluation of novel all-hydrocarbon cross-linked aza-stapled peptides. Org. Biomol. Chem. 2022, 20, 7963–7971. [Google Scholar] [CrossRef]
  29. Singh, S.; Shrivastava, R.; Singh, G.; Ali, R.; Sankar Ampapathi, R.; Bhadhuria, S.; Haq, W. AzaGly-Appended Peptidomimetics Structurally Related to PTR6154 as Potential PKB/Akt Inhibitors. Chembiochem 2017, 18, 1061–1065. [Google Scholar] [CrossRef]
  30. Sabatino, D.; Proulx, C.; Pohankova, P.; Ong, H.; Lubell, W.D. Structure-activity relationships of GHRP-6 azapeptide ligands of the CD36 scavenger receptor by solid-phase submonomer azapeptide synthesis. J. Am. Chem. Soc. 2011, 133, 12493–12506. [Google Scholar] [CrossRef]
  31. Altiti, A.S.; He, M.; VanPatten, S.; Cheng, K.F.; Ahmed, U.; Chiu, P.Y.; Mughrabi, I.T.; Jabari, B.A.; Burch, R.M.; Manogue, K.R.; et al. Thiocarbazate building blocks enable the construction of azapeptides for rapid development of therapeutic candidates. Nat. Commun. 2022, 13, 7127. [Google Scholar] [CrossRef]
  32. Karplus, P.A. Experimentally observed conformation-dependent geometry and hidden strain in proteins. Protein Sci. 1996, 5, 1406–1420. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  33. Melton, S.D.; Smith, M.S.; Chenoweth, D.M. Incorporation of Aza-Glycine into Collagen Peptides. J. Org. Chem. 2020, 85, 1706–1711. [Google Scholar] [CrossRef]
  34. Etzkorn, F.A.; Ware, R.I.; Pester, A.M.; Troya, D. Conformational Analysis of n→π* Interactions in Collagen Triple Helix Models. J. Phys. Chem. B 2019, 123, 496–503. [Google Scholar] [CrossRef] [PubMed]
  35. Melton, S.D.; Chenoweth, D.M. Variation in the Yaa position of collagen peptides containing azaGlycine. Chem. Commun. 2018, 54, 11937–11940. [Google Scholar] [CrossRef] [PubMed]
  36. Zhang, Y.; Malamakal, R.M.; Chenoweth, D.M. Aza-Glycine Induces Collagen Hyperstability. J. Am. Chem. Soc. 2015, 137, 12422–12425. [Google Scholar] [CrossRef]
  37. Harris, T.; Chenoweth, D.M. Sterics and Stereoelectronics in Aza-Glycine: Impact of Aza-Glycine Preorganization in Triple Helical Collagen. J. Am. Chem. Soc. 2019, 141, 18021–18029. [Google Scholar] [CrossRef]
  38. Kasznel, A.J.; Harris, T.; Porter, N.J.; Zhang, Y.; Chenoweth, D.M. Aza-proline effectively mimics l-proline stereochemistry in triple helical collagen. Chem. Sci. 2019, 10, 6979–6983. [Google Scholar] [CrossRef] [Green Version]
  39. Kasznel, A.J.; Zhang, Y.; Hai, Y.; Chenoweth, D.M. Structural Basis for Aza-Glycine Stabilization of Collagen. J. Am. Chem. Soc. 2017, 139, 9427–9430. [Google Scholar] [CrossRef]
  40. Ro, S.; Lee, H.J.; Ahn, I.; Shin, D.; Lee, K.; Yoon, C.; Choi, Y. Torsion angle based design of peptidomimetics: A dipeptidic template adopting beta-I turn (Ac-Aib-AzGly-NH2). Bioorg. Med. Chem. 2001, 9, 1837–1841. [Google Scholar] [CrossRef]
  41. McMechen, M.A.; Willis, P.C.; Proulx, G.C. Aza-amino acids disrupt b-sheet secondary structures. Molecules 2019, 24, 1919. [Google Scholar] [CrossRef] [Green Version]
  42. Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  43. Marenich, A.V.; Cramer, C.J.; Truhlar, D.G. Universal Solvation Model Based on Solute Electron Density and on a Continuum Model of the Solvent Defined by the Bulk Dielectric Constant and Atomic Surface Tensions. J. Phys. Chem. B 2009, 113, 6378–6396. [Google Scholar] [CrossRef]
  44. Brenner, V.; Gloaguen, E.; Mons, M. Rationalizing the diversity of amide–amide H-bonding in peptides using the natural bond orbital method. Phys. Chem. Chem. Phys. 2019, 21, 24601–24619. [Google Scholar] [CrossRef] [PubMed]
  45. Alemán, C.; Puiggalí, J. Conformational Preferences of the Asparagine Residue. Gas-Phase, Aqueous Solution, and Chloroform Solution Calculations on the Model Dipeptide. J. Phys. Chem. B 1997, 101, 3441–3446. [Google Scholar] [CrossRef]
  46. André, F.; Vicherat, A.; Boussard, G.; Aubry, A.; Marraud, M. Aza-peptides. III. Experimental structural analysis of aza-alanine and aza-asparagine-containing peptides. J. Pept. Res. 1997, 50, 372–381. [Google Scholar] [CrossRef] [PubMed]
  47. André, F.; Boussard, G.; Bayeul, D.; Didierjean, C.; Aubry, A.; Marraud, M. Aza-peptides. II. X-ray structures of aza-alanine and aza-asparagine-containing peptides. J. Pept. Res. 1997, 49, 556–562. [Google Scholar] [CrossRef] [PubMed]
  48. Baruah, K.; Sahariah, B.; Sakpal, S.S.; Deka, J.K.R.; Bar, A.K.; Bagchi, S.; Sarma, B.K. Stabilization of Azapeptides by N(amide)···H-N(amide) Hydrogen Bonds. Org. Lett. 2021, 23, 4949–4954. [Google Scholar] [CrossRef]
  49. Abbadi, A.; McHarfi, M.; Aubry, A.; Premilat, S.; Boussard, G.; Marraud, M. Involvement of side functions in peptide structures: The Asx turn. Occurrence and conformational aspects. J. Am. Chem. Soc. 1991, 113, 2729–2735. [Google Scholar] [CrossRef]
  50. Duddy, W.J.; Nissink, J.W.; Allen, F.H.; Milner-White, E.J. Mimicry by asx- and ST-turns of the four main types of β-turn in proteins. Protein Sci. 2004, 13, 3051–3055. [Google Scholar] [CrossRef] [Green Version]
  51. D’Mello, V.C.; Goldsztejn, G.; Rao Mundlapati, V.; Brenner, V.; Gloaguen, E.; Charnay-Pouget, F.; Aitken, D.J.; Mons, M. Characterization of Asx Turn Types and Their Connate Relationship with β-Turns. Chemistry 2022, 28, e202104328. [Google Scholar] [CrossRef] [PubMed]
  52. Froimowitz, M. HyperChem: A software package for computational chemistry and molecular modeling. Biotechniques 1993, 14, 1010–1013. [Google Scholar] [PubMed]
  53. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Software: Gaussian 16, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2019. [Google Scholar]
  54. Lu, T.; Chen, F. Multiwfn: A multifunctional wavefunction analyzer. J. Comput. Chem. 2012, 33, 580–592. [Google Scholar] [CrossRef] [PubMed]
Molecules 28 05454 g001 550
Figure 1. Azapeptide models studied in this work (A) Definition of torsional angle parameters of azaamino acid residue-containing tripeptide models, Ac-azaXaa-Pro-NHMe [Xaa = Asn (4), Asp (5), Ala (6)]; (B) The backbone (ϕ, ψ) distribution of azaXaa and Pro residues, respectively, calculated at the B3LYP/6-311++G(d, p) level of theory in the gas phase. The nomenclature proposed by Karplus et al. [32] is used: αR: right-handed α-helix; αL: a mirror image of α-helix; βS: region primarily involved in β-sheet formation; βP: regions associated with extended polyproline-like helix or β-sheet; γ and γ′: γ and inverse-γ turns; δR: the bridge region; δL: a mirror image of δR region; ε: the extensive region with ϕ > 0, ψ = ±180°; ε′ and ε′′: mirror images of the two parts of the ε region; ζ: a region associated with residues preceding Pro [19].
Figure 1. Azapeptide models studied in this work (A) Definition of torsional angle parameters of azaamino acid residue-containing tripeptide models, Ac-azaXaa-Pro-NHMe [Xaa = Asn (4), Asp (5), Ala (6)]; (B) The backbone (ϕ, ψ) distribution of azaXaa and Pro residues, respectively, calculated at the B3LYP/6-311++G(d, p) level of theory in the gas phase. The nomenclature proposed by Karplus et al. [32] is used: αR: right-handed α-helix; αL: a mirror image of α-helix; βS: region primarily involved in β-sheet formation; βP: regions associated with extended polyproline-like helix or β-sheet; γ and γ′: γ and inverse-γ turns; δR: the bridge region; δL: a mirror image of δR region; ε: the extensive region with ϕ > 0, ψ = ±180°; ε′ and ε′′: mirror images of the two parts of the ε region; ζ: a region associated with residues preceding Pro [19].
Molecules 28 05454 g001
Molecules 28 05454 g002 550
Figure 2. Cyclic motifs formed by hydrogen bonds in Ac-azaXaa-Pro-NHMe [Xaa = Asn (4), Asp (5), Ala (6)].
Figure 2. Cyclic motifs formed by hydrogen bonds in Ac-azaXaa-Pro-NHMe [Xaa = Asn (4), Asp (5), Ala (6)].
Molecules 28 05454 g002
Molecules 28 05454 g003 550
Figure 3. NBO overlaps of tttANP-1 and tctANP-2 in the gas phase. ‘t’ and ‘c’ represents ‘trans’ and ‘cis’ amide bond, respectively. The E(2) value is shown in blue color for B3LYP and red color for B3LYP-D3 functional.
Figure 3. NBO overlaps of tttANP-1 and tctANP-2 in the gas phase. ‘t’ and ‘c’ represents ‘trans’ and ‘cis’ amide bond, respectively. The E(2) value is shown in blue color for B3LYP and red color for B3LYP-D3 functional.
Molecules 28 05454 g003
Molecules 28 05454 g004 550
Figure 4. NBO overlaps of the lowest energy conformer tttADP-1 (@B3LYP) and tctADP-3 (@B3LYP-D3) in the gas phase. The E(2) value is shown (See Supporting Information Tables S14 and S17).
Figure 4. NBO overlaps of the lowest energy conformer tttADP-1 (@B3LYP) and tctADP-3 (@B3LYP-D3) in the gas phase. The E(2) value is shown (See Supporting Information Tables S14 and S17).
Molecules 28 05454 g004
Molecules 28 05454 g005 550
Figure 5. NBO overlaps of tttAAP-1 in the gas phase. The E(2) value is shown in blue color for B3LYP and red color for B3LYP-D3 functional.
Figure 5. NBO overlaps of tttAAP-1 in the gas phase. The E(2) value is shown in blue color for B3LYP and red color for B3LYP-D3 functional.
Molecules 28 05454 g005
Molecules 28 05454 g006 550
Figure 6. Definition of structure and torsion angles for β-turn (A) and Asx-turn (B). The representative β-turn and Asx-turn for Ac-azaAsn-Pro-NHMe (4, ANP) and Ac-azaAsp-Pro-NHMe (5, ADP) optimized at the B3LYP/6-311++G(d, p) level of theory in the isolated form. HB bond lengths (Å) found in turns are in red color.
Figure 6. Definition of structure and torsion angles for β-turn (A) and Asx-turn (B). The representative β-turn and Asx-turn for Ac-azaAsn-Pro-NHMe (4, ANP) and Ac-azaAsp-Pro-NHMe (5, ADP) optimized at the B3LYP/6-311++G(d, p) level of theory in the isolated form. HB bond lengths (Å) found in turns are in red color.
Molecules 28 05454 g006
Table
Table 1. Relative energies in (kcal/mol) of model compounds (4), (5), and (6) at B3LYP (M1) and B3LYP-D3 (M2) functionals with the 6-311++G (d, p) basis set in the isolated form.
Table 1. Relative energies in (kcal/mol) of model compounds (4), (5), and (6) at B3LYP (M1) and B3LYP-D3 (M2) functionals with the 6-311++G (d, p) basis set in the isolated form.
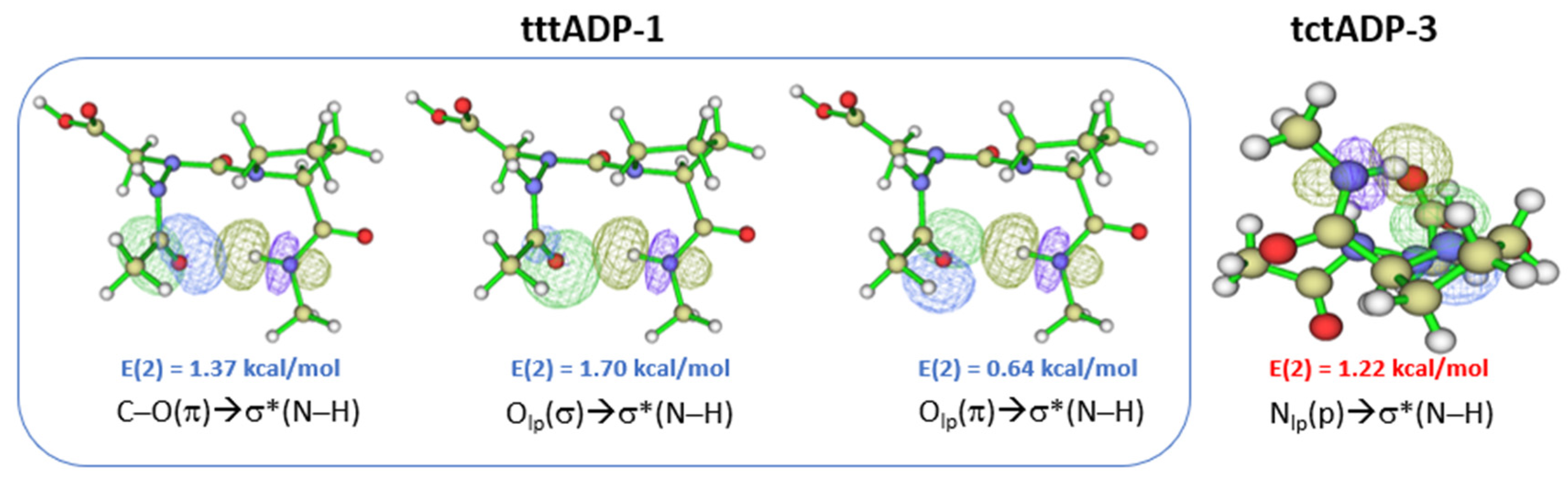
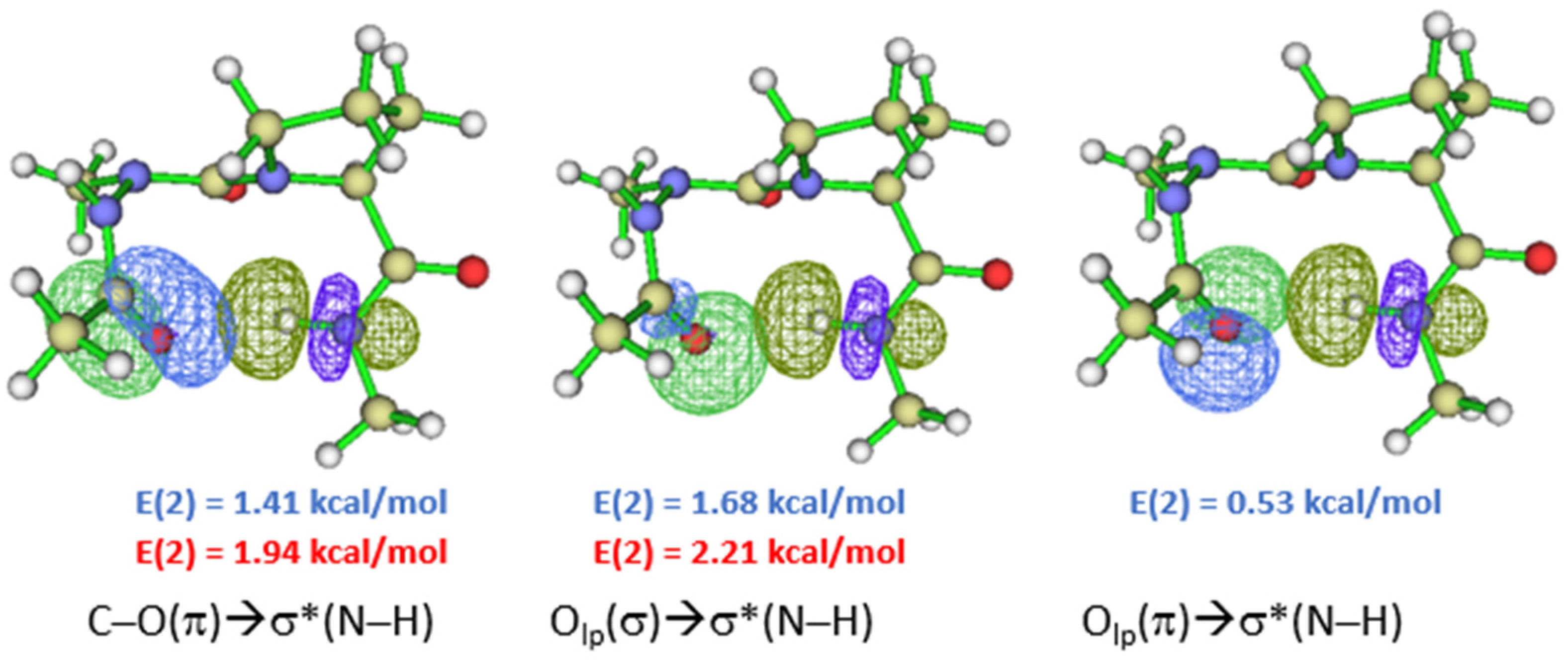
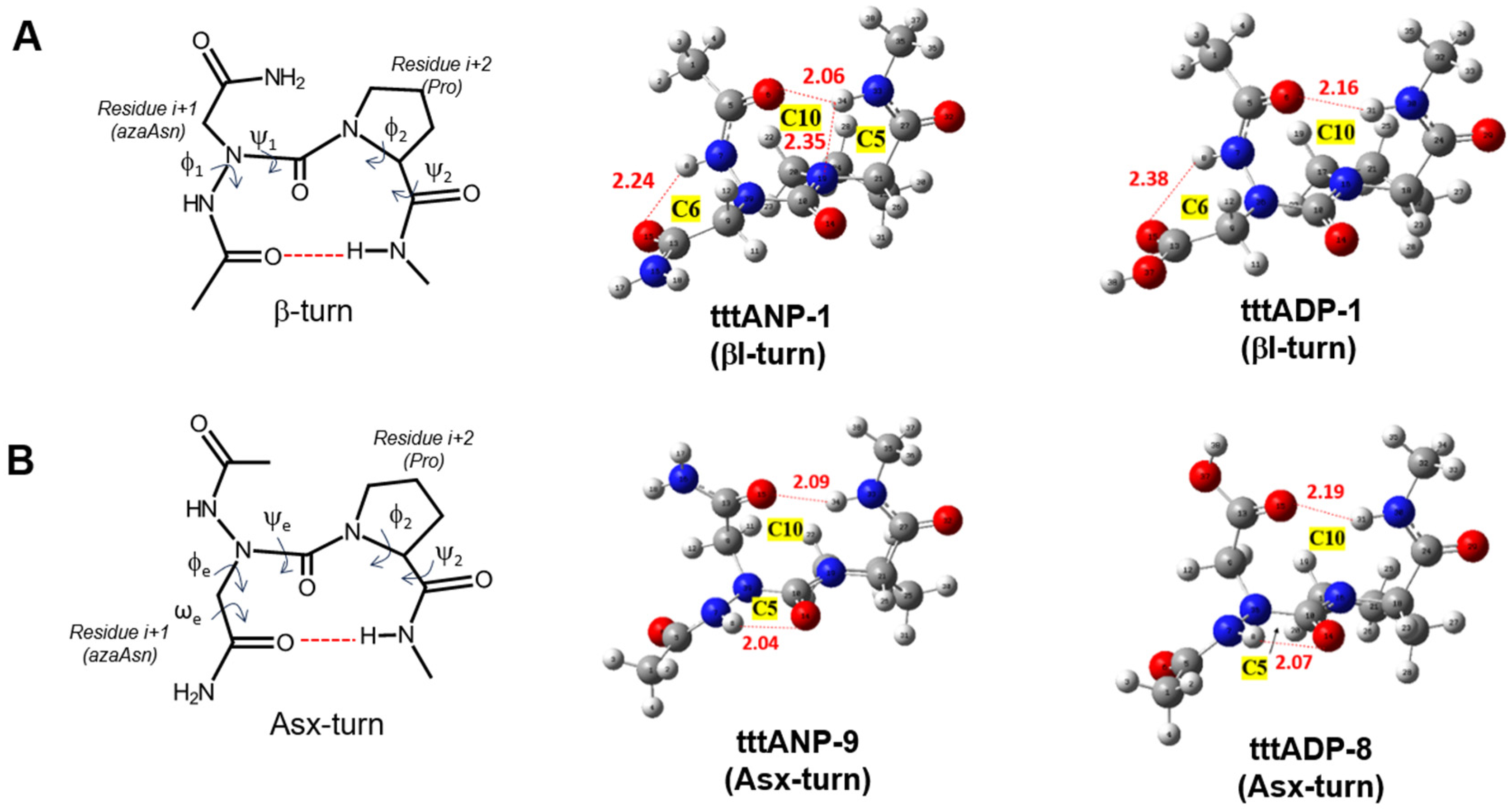
Ac-azaAsn-Pro-NHMeAc-azaAsp-Pro-NHMeAc-azaAla-Pro-NHMe
ΔE(M1)ΔE(M2) ΔE(M1)ΔE(M2) ΔE(M1)ΔE(M2)
tttANP-1a 0.000.94tttADP-1c 0.000.69tttAAP-1e 0.00f 0.00
tctANP-20.94b 0.00tttADP-21.002.70tttAAP-20.751.13
tttANP-31.322.64tctADP-31.07d 0.00tttAAP-31.002.51
tttANP-41.381.95tctADP-41.440.38tttAAP-41.263.70
tttANP-51.962.89cttADP-52.072.57tctAAP-51.441.82
cttANP-62.453.07tttADP-62.953.33cttAAP-61.512.26
tctANP-72.642.01tttADP-73.334.39cttAAP-71.632.20
tttANP-82.643.20tttADP-83.705.46tctAAP-82.261.26
tttANP-92.894.52tttADP-94.525.33tttAAP-92.704.89
cttANP-103.073.26cttADP-104.524.58tctAAP-102.762.20
cttANP-113.262.95cttADP-114.584.83cttAAP-113.513.01
tttANP-124.084.89cctADP-124.833.39tctAAP-123.705.27
tctANP-134.273.20tctADP-135.084.89cctAAP-133.772.01
tctANP-144.463.33tttADP-145.657.53tttAAP-143.894.64
tttANP-154.465.65cctADP-156.024.89cctAAP-154.332.95
cctANP-164.522.32tctADP-166.343.83cttAAP-164.966.46
cctANP-174.643.20tctADP-176.787.22cctAAP-175.525.02
cttANP-184.834.96cctADP-186.974.96ttcAAP-186.285.58
tctANP-195.023.26cctADP-197.405.40cctAAP-196.345.46
cttANP-205.405.71tctADP-207.666.21cccAAP-208.476.15
cctANP-215.464.46tttADP-217.729.04tccAAP-2110.107.66
cctANP-225.583.26tctADP-228.036.97cccAAP-2211.929.73
cttANP-235.965.77cttADP-238.037.97
tctANP-246.717.22ttcADP-249.298.84
tctANP-256.905.52tccADP-2510.107.47
cttANP-267.597.53cttADP-2610.8611.11
tttANP-277.599.66cttADP-2711.3010.73
ttcANP-287.977.59cccADP-2813.439.22
tccANP-299.166.21cctADP-2913.4312.61
tttANP-309.7911.36cccADP-3016.1312.74
cctANP-319.989.48
cccANP-3210.356.34
cccANP-3314.7511.80
a E = −1005.6114 a.u; b E = −1005.6582 a.u; c E = −1025.4846 a.u; d E = −1025.5303 a.u; e E = −836.8502 a.u; f E = −836.8898 a.u.
Table
Table 2. Relative energies in (kcal/mol) of model compounds 4, 5, and 6 at the SMD/B3LYP/6-311++G (d, p) (M3) and the SMD/B3LYP-D3/6-311++G(d, p) (M4) levels of theory in water.
Table 2. Relative energies in (kcal/mol) of model compounds 4, 5, and 6 at the SMD/B3LYP/6-311++G (d, p) (M3) and the SMD/B3LYP-D3/6-311++G(d, p) (M4) levels of theory in water.
Ac-azaAsn-Pro-NHMeAc-azaAsp-Pro-NHMeAc-azaAla-Pro-NHMe
ΔE(M3)ΔE(M4) ΔE(M3)ΔE(M4) ΔE(M3)ΔE(M4)
tttANP-11.131.51tttADP-13.142.70tttAAP-1e 0.00f 0.00
tctANP-21.38b 0.00tttADP-25.215.71tttAAP-22.013.07
tttANP-33.074.52tctADP-33.641.38tttAAP-32.764.27
tttANP-43.013.07tctADP-45.153.58tttAAP-42.706.46
tttANP-52.763.45cttADP-56.846.28tctAAP-53.263.95
cttANP-64.644.96tttADP-64.273.45cttAAP-64.965.46
tctANP-74.273.70tttADP-72.822.45cttAAP-75.155.71
tttANP-81.511.57tttADP-85.405.77tctAAP-82.010.88
tttANP-92.954.20tttADP-9c 0.00d 0.00tttAAP-92.644.64
cttANP-106.786.59cttADP-105.655.27tctAAP-101.822.26
cttANP-117.156.34cttADP-117.666.90cttAAP-112.822.32
tttANP-12a 0.000.82cctADP-126.023.45tctAAP-125.026.34
tctANP-133.334.89tctADP-135.844.33cctAAP-134.463.07
tctANP-142.952.07tttADP-146.096.90tttAAP-141.696.34
tttANP-154.645.84cctADP-159.297.78cctAAP-154.583.01
cctANP-163.642.38tctADP-166.025.08cttAAP-163.774.96
cctANP-173.772.07tctADP-174.714.64cctAAP-175.525.02
cttANP-183.514.02cctADP-187.154.58ttcAAP-184.337.22
tctANP-193.072.13cctADP-196.024.14cctAAP-194.463.95
cttANP-204.334.58tctADP-207.405.15cccAAP-207.346.21
cctANP-216.785.96tttADP-215.086.84tccAAP-217.915.58
cctANP-226.024.08tctADP-225.153.45cccAAP-2211.049.29
cttANP-235.584.02cttADP-235.904.83
tctANP-242.703.51ttcADP-245.1510.42
tctANP-254.144.20tccADP-2510.176.46
cttANP-263.893.45cttADP-268.037.53
tttANP-274.085.84cttADP-277.596.21
ttcANP-2810.179.79cccADP-2810.736.40
tccANP-299.356.15cctADP-299.918.22
tttANP-303.075.71cccADP-3012.807.72
cctANP-316.345.52
cccANP-328.224.64
cccANP-3312.5610.10
a E = −1005.6567 a.u; b E = −1005.7028 a.u; c E = −1025.5295 a.u; d E = −1025.5733 a.u; e E = −836.8844 a.u; f E = −836.9238 a.u.
Table
Table 3. Backbone dihedral angles of residues at the i + 1 and the i + 2 positions for the major types of β turns.
Table 3. Backbone dihedral angles of residues at the i + 1 and the i + 2 positions for the major types of β turns.
Turnϕi+1 (°) Asx ϕeψi+1 (°) Asx ψeϕi+2 (°)ψi+2 (°)
βI−60−30−900
βI′6030900
βII−60120800
βII′60−120−800
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

El Khabchi, M.; Mcharfi, M.; Benzakour, M.; Fitri, A.; Benjelloun, A.T.; Song, J.-W.; Lee, K.-B.; Lee, H.-J. Computational Investigation of Conformational Properties of Short Azapeptides: Insights from DFT Study and NBO Analysis. Molecules 2023, 28, 5454. https://doi.org/10.3390/molecules28145454

AMA Style

El Khabchi M, Mcharfi M, Benzakour M, Fitri A, Benjelloun AT, Song J-W, Lee K-B, Lee H-J. Computational Investigation of Conformational Properties of Short Azapeptides: Insights from DFT Study and NBO Analysis. Molecules. 2023; 28(14):5454. https://doi.org/10.3390/molecules28145454

Chicago/Turabian Style

El Khabchi, Mouna, Mohammed Mcharfi, Mohammed Benzakour, Asmae Fitri, Adil Touimi Benjelloun, Jong-Won Song, Kang-Bong Lee, and Ho-Jin Lee. 2023. "Computational Investigation of Conformational Properties of Short Azapeptides: Insights from DFT Study and NBO Analysis" Molecules 28, no. 14: 5454. https://doi.org/10.3390/molecules28145454

APA Style

El Khabchi, M., Mcharfi, M., Benzakour, M., Fitri, A., Benjelloun, A. T., Song, J. -W., Lee, K. -B., & Lee, H. -J. (2023). Computational Investigation of Conformational Properties of Short Azapeptides: Insights from DFT Study and NBO Analysis. Molecules, 28(14), 5454. https://doi.org/10.3390/molecules28145454

Article Metrics

Back to TopTop