Molecular Simulation Study on the Wettability of a Surface Texturized with Hierarchical Pillars
Abstract
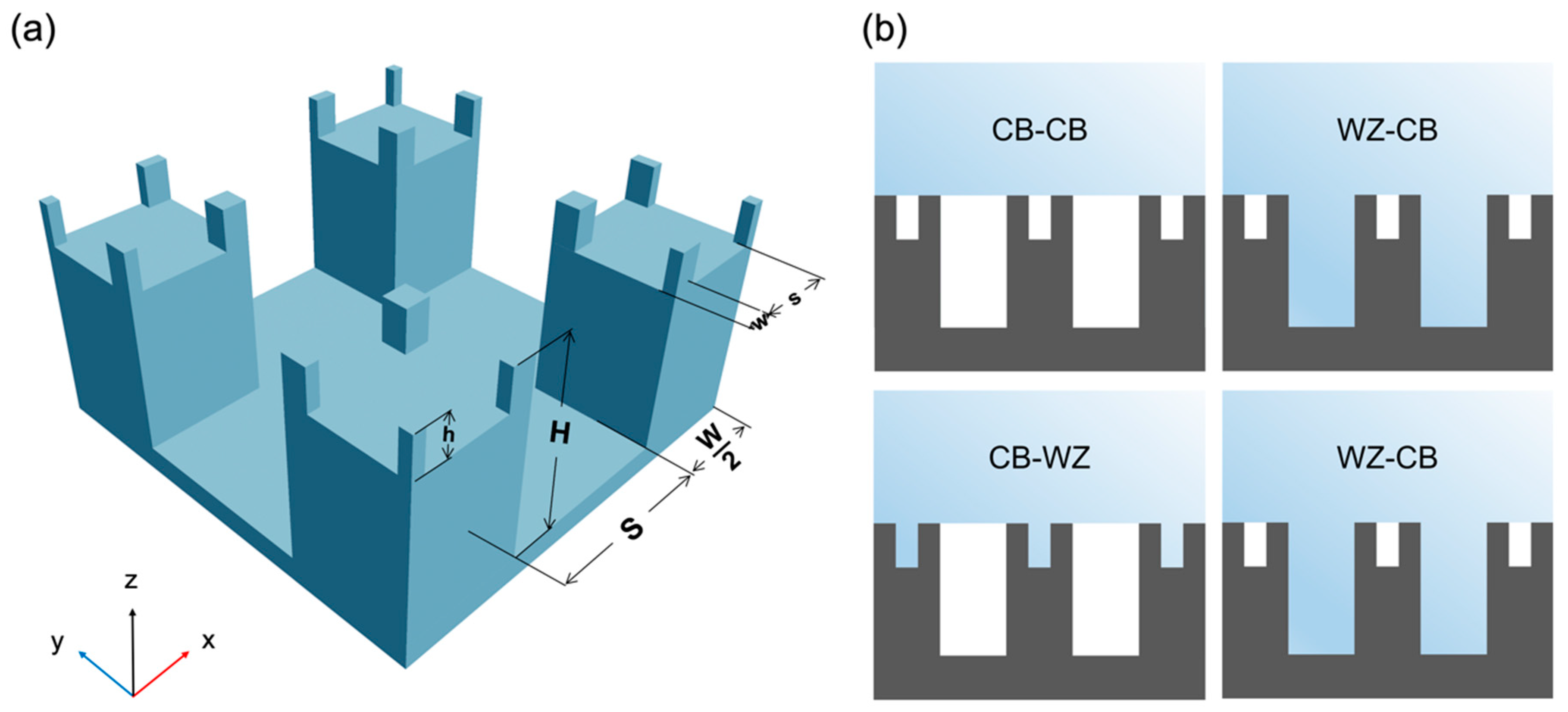
1. Introduction
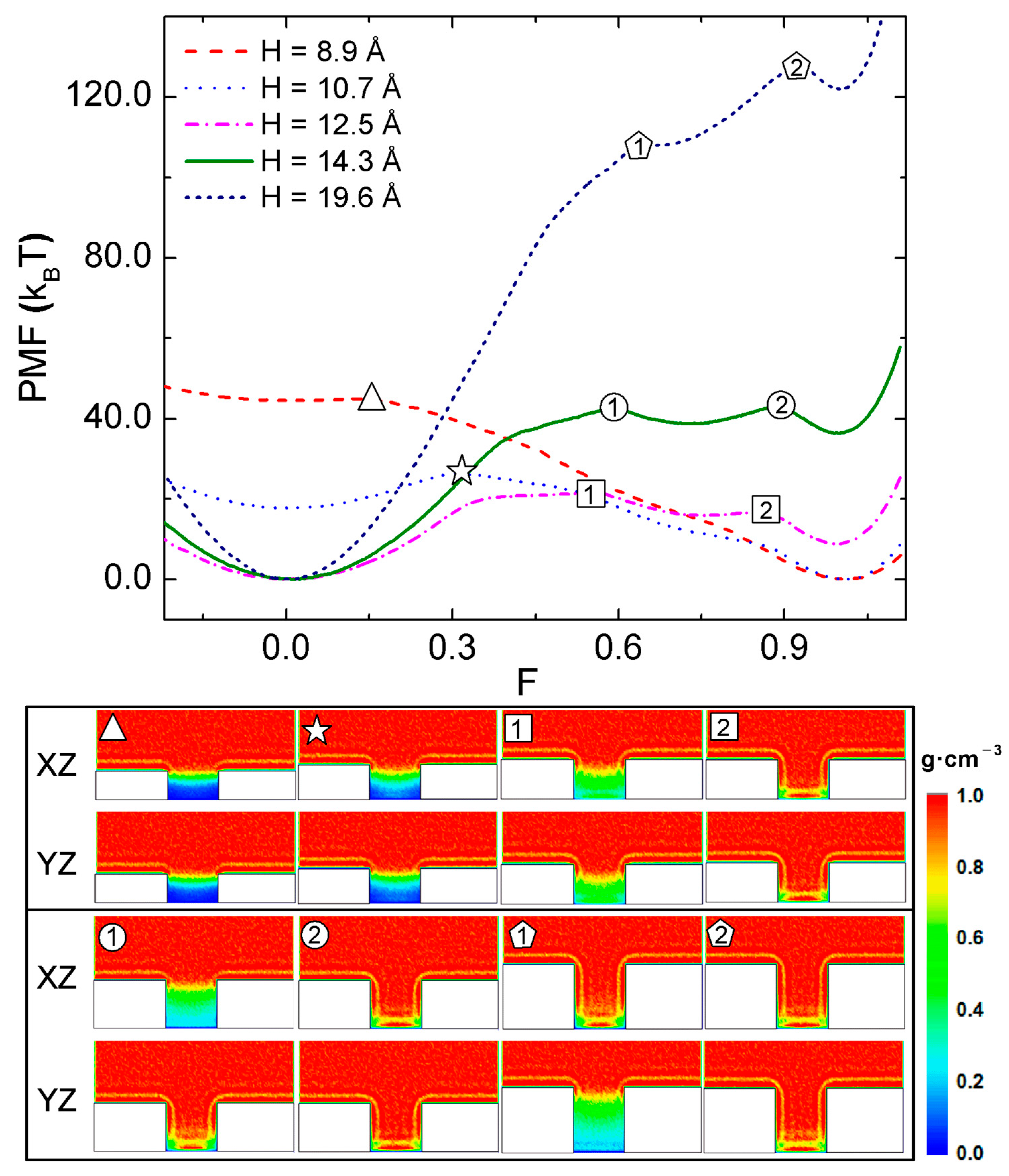
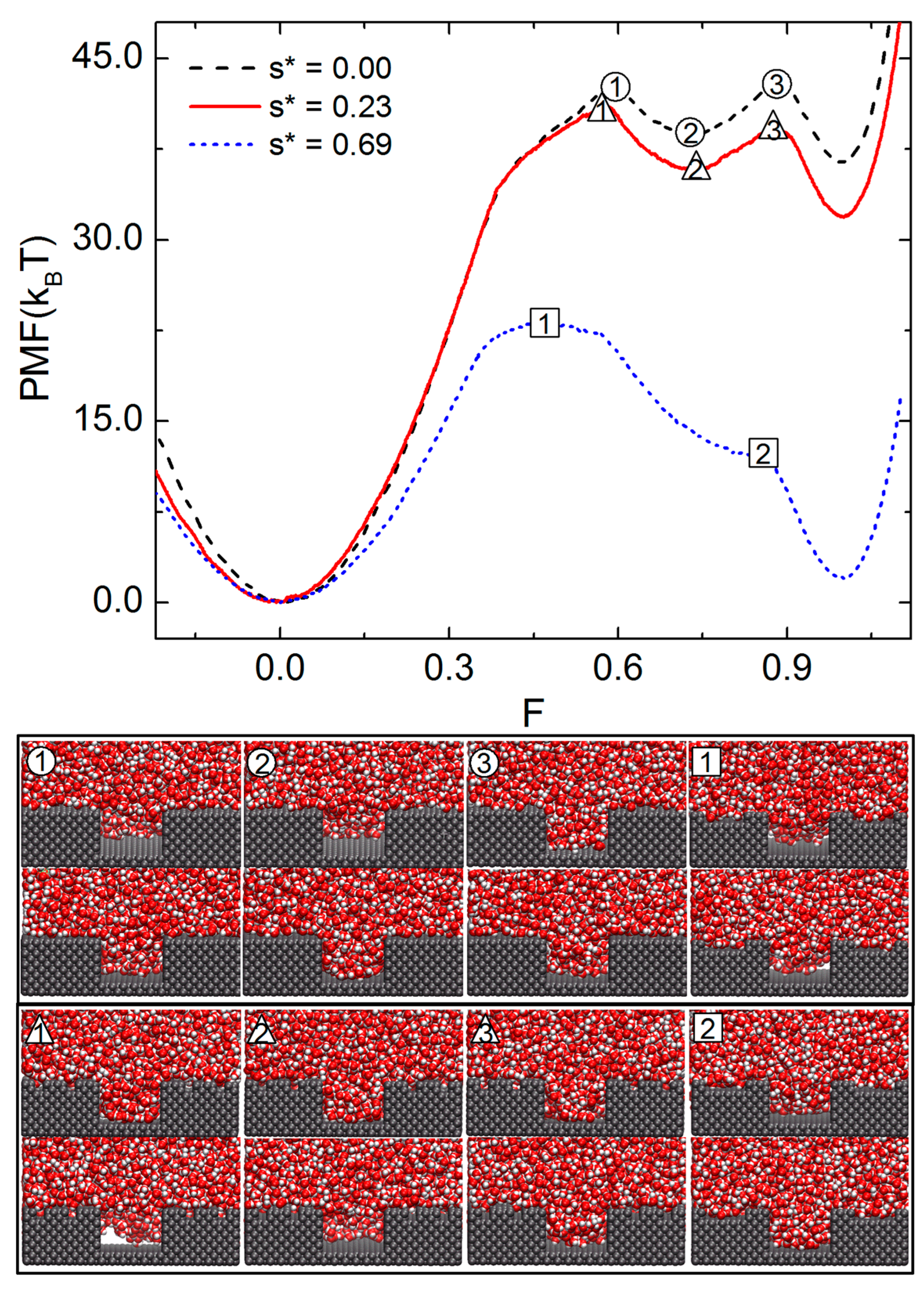
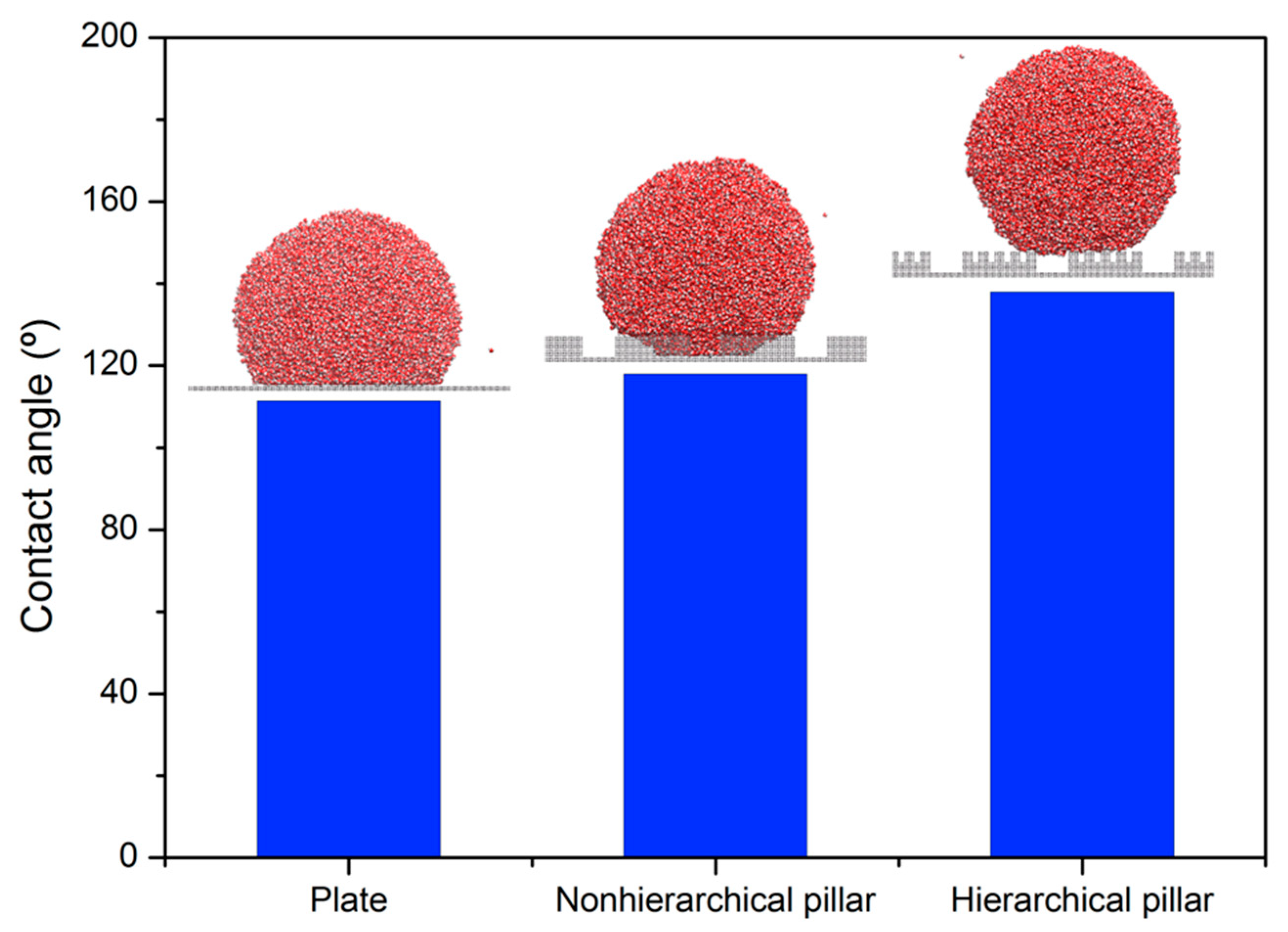
2. Results and Discussion
3. Simulation Methods
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Li, W.; Zhan, Y.; Yu, S. Applications of superhydrophobic coatings in anti-icing: Theory, mechanisms, impact factors, challenges and perspectives. Prog. Org. Coat. 2021, 152, 106117. [Google Scholar] [CrossRef]
- Shen, Y.; Jin, M.; Wu, X.; Tao, J.; Luo, X.; Chen, H.; Lu, Y.; Xie, Y. Understanding the frosting and defrosting mechanism on the superhydrophobic surfaces with hierarchical structures for enhancing anti-frosting performance. Appl. Therm. Eng. 2019, 156, 111–118. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, T.; Wu, M.; Wei, W. Durable superhydrophobic surface with hierarchical microstructures for efficient water collection. Surf. Coat. Technol. 2021, 419, 127279. [Google Scholar] [CrossRef]
- Rasouli, S.; Rezaei, N.; Hamedi, H.; Zendehboudi, S.; Duan, X. Superhydrophobic and superoleophilic membranes for oil-water separation application: A comprehensive review. Mater. Des. 2021, 204, 109599. [Google Scholar] [CrossRef]
- Sun, P.; Feng, X.; Tian, G.; Zhang, X.; Chu, J. Ultrafast Self-Healing Superhydrophobic Surface for Underwater Drag Reduction. Langmuir 2022, 38, 10875–10885. [Google Scholar] [CrossRef] [PubMed]
- Mollicone, J.-P.; Battista, F.; Gualtieri, P.; Casciola, C.M. Superhydrophobic surfaces to reduce form drag in turbulent separated flows. AIP Adv. 2022, 12, 075003. [Google Scholar] [CrossRef]
- Dalawai, S.P.; Saad Aly, M.A.; Latthe, S.S.; Xing, R.; Sutar, R.S.; Nagappan, S.; Ha, C.-S.; Kumar Sadasivuni, K.; Liu, S. Recent Advances in durability of superhydrophobic self-cleaning technology: A critical review. Prog. Org. Coat. 2020, 138, 105381. [Google Scholar] [CrossRef]
- Vazirinasab, E.; Jafari, R.; Momen, G. Application of superhydrophobic coatings as a corrosion barrier: A review. Surf. Coat. Technol. 2018, 341, 40–56. [Google Scholar] [CrossRef]
- Yang, C.; Cui, S.; Weng, Y.; Wu, Z.; Liu, L.; Ma, Z.; Tian, X.; Fu, R.K.Y.; Chu, P.K.; Wu, Z. Scalable superhydrophobic T-shape micro/nano structured inorganic alumina coatings. Chem. Eng. J. 2021, 409, 128142. [Google Scholar] [CrossRef]
- Cherupurakal, N.; Mozumder, M.S.; Mourad, A.-H.I.; Lalwani, S. Recent advances in superhydrophobic polymers for antireflective self-cleaning solar panels. Renew. Sustain. Energy Rev. 2021, 151, 111538. [Google Scholar] [CrossRef]
- Kim, D.H.; Park, J.-H.; Lee, T.I.; Myoung, J.-M. Superhydrophobic Al-doped ZnO nanorods-based electrically conductive and self-cleanable antireflecting window layer for thin film solar cell. Sol. Energy Mater. Sol. Cells 2016, 150, 65–70. [Google Scholar] [CrossRef]
- Vüllers, F.; Gomard, G.; Preinfalk, J.B.; Klampaftis, E.; Worgull, M.; Richards, B.; Hölscher, H.; Kavalenka, M.N. Bioinspired Superhydrophobic Highly Transmissive Films for Optical Applications. Small 2016, 12, 6144–6152. [Google Scholar] [CrossRef]
- Wang, S.; Wang, H.; Zhang, D.; Dou, Y.; Li, W.; Cao, F.; Yin, L.; Wang, L.; Zhang, Z.-J.; Zhang, J.; et al. Perovskite nanocrystals-polymer composites with a micro/nano structured superhydrophobic surface for stable and efficient white light-emitting diodes. Chem. Eng. J. 2022, 437, 135303. [Google Scholar] [CrossRef]
- Kwon, T.W.; Jang, J.; Ambrosia, M.S.; Ha, M.Y. Molecular dynamics study on the hydrophobicity of a surface patterned with hierarchical nanotextures. Colloids Surf. A Physicochem. Eng. Asp. 2018, 559, 209–217. [Google Scholar] [CrossRef]
- Li, H.; Yan, T. Importance of moderate size of pillars and dual-scale structures for stable superhydrophobic surfaces: A molecular dynamics simulation study. Comput. Mater. Sci. 2020, 175, 109613. [Google Scholar] [CrossRef]
- Gao, Y.; Liu, Y.; Jiang, J.; Zhu, C.; Zuhlke, C.; Alexander, D.; Francisco, J.S.; Zeng, X.C. Multiple Wetting-Dewetting States of a Water Droplet on Dual-Scale Hierarchical Structured Surfaces. JACS Au 2021, 1, 955–966. [Google Scholar] [CrossRef]
- Kwon, T.W.; Jang, J.; Sim, G.H.; Park, S.H.; Ha, M.Y. Wetting Behavior of a Surface with Dual-Scale Structures. Langmuir 2021, 37, 7995–8006. [Google Scholar] [CrossRef]
- Lin, H.-P.; Chen, L.-J. Direct observation of wetting behavior of water drops on single micro-scale roughness surfaces of rose petal effect. J. Colloid Interface Sci. 2021, 603, 539–549. [Google Scholar] [CrossRef]
- Kim, H.; Saha, J.K.; Jang, J. Drying Transition of Water Confined between Hydrophobic Pillars. J. Phys. Chem. C 2012, 116, 19233–19239. [Google Scholar] [CrossRef]
- Giacomello, A.; Meloni, S.; Chinappi, M.; Casciola, C.M. Cassie-Baxter and Wenzel states on a nanostructured surface: Phase diagram, metastabilities, and transition mechanism by atomistic free energy calculations. Langmuir 2012, 28, 10764–10772. [Google Scholar] [CrossRef]
- Cansoy, C.E.; Erbil, H.Y.; Akar, O.; Akin, T. Effect of pattern size and geometry on the use of Cassie–Baxter equation for superhydrophobic surfaces. Colloids Surf. A Physicochem. Eng. Asp. 2011, 386, 116–124. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Bhushan, B. Patterned Nonadhesive Surfaces: Superhydrophobicity and Wetting Regime Transitions. Langmuir 2008, 24, 1525–1533. [Google Scholar] [CrossRef]
- Wenzel, R.N. Resistance of solid surfaces to wetting by water. Ind. Eng. Chem. 1936, 28, 988–994. [Google Scholar] [CrossRef]
- Cassie, A.B.D.; Baxter, S. Wettability of porous surfaces. Trans. Faraday Soc. 1944, 40, 546–551. [Google Scholar] [CrossRef]
- Yang, Z.; Zhu, C.; Zheng, N.; Le, D.; Zhou, J. Superhydrophobic Surface Preparation and Wettability Transition of Titanium Alloy with Micro/Nano Hierarchical Texture. Materials 2018, 11, 2210. [Google Scholar] [CrossRef]
- Nikosokhan, R.; Norouzbeigi, R.; Velayi, E. Fabrication of cobalt-based superhydrophobic coating with micro/nano hierarchical structure without additional hydrophobization treatment. Ceram. Int. 2021, 47, 30711–30721. [Google Scholar] [CrossRef]
- Kwon, T.W.; Lee, K.H.; Seo, Y.M.; Jang, J.; Ha, M.Y. Dynamic Wetting Behaviors of Water Droplets on Surfaces with Dual Structures at the Nanoscale. Int. J. Multiph. Flow 2020, 129, 103352. [Google Scholar] [CrossRef]
- Zhang, Z.; Ha, M.Y.; Jang, J. Contrasting water adhesion strengths of hydrophobic surfaces engraved with hierarchical grooves: Lotus leaf and rose petal effects. Nanoscale 2017, 9, 16200–16204. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Kim, H.; Ha, M.Y.; Jang, J. Molecular dynamics study on the wettability of a hydrophobic surface textured with nanoscale pillars. Phys. Chem. Chem. Phys. 2014, 16, 5613–5621. [Google Scholar] [CrossRef] [PubMed]
- Bai, L.; Kim, K.; Ha, M.Y.; Ahn, Y.; Jang, J. Molecular Insights on the Wetting Behavior of a Surface Corrugated with Nanoscale Domed Pillars. Langmuir 2021, 37, 9336–9345. [Google Scholar] [CrossRef]
- Abdoul-Carime, H.; Berthias, F.; Feketeová, L.; Marciante, M.; Calvo, F.; Forquet, V.; Chermette, H.; Farizon, B.; Farizon, M.; Märk, T.D. Velocity of a Molecule Evaporated from a Water Nanodroplet: Maxwell–Boltzmann Statistics versus Non-Ergodic Events. Angew. Chem. Int. Ed. 2015, 54, 14685–14689. [Google Scholar] [CrossRef] [PubMed]
- VandeVondele, J.; Mohamed, F.; Krack, M.; Hutter, J.; Sprik, M.; Parrinello, M. The influence of temperature and density functional models in ab initio molecular dynamics simulation of liquid water. J. Chem. Phys. 2004, 122, 014515. [Google Scholar] [CrossRef]
- Hung, S.W.; Hsiao, P.Y.; Chen, C.P.; Chieng, C.C. Wettability of Graphene-Coated Surface: Free Energy Investigations Using Molecular Dynamics Simulation. J. Phys. Chem. C 2015, 119, 8103–8111. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; Grigera, J.R.; Straatsma, T.P. The Missing Term in Effective Pair Potentials. J. Phys. Chem. 1987, 91, 6269–6271. [Google Scholar] [CrossRef]
- Crozier, P.S.; Rowley, R.L.; Henderson, D. Molecular-dynamics simulations of ion size effects on the fluid structure of aqueous electrolyte systems between charged model electrodes. J. Chem. Phys. 2001, 114, 7513–7517. [Google Scholar] [CrossRef]
- Tildesley, D.; Allen, M. Computer Simulation of Liquids; Clarendon: Oxford, UK, 1987. [Google Scholar]
- Ryckaert, J.-P.; Ciccotti, G.; Berendsen, H.J.C. Numerical integration of the cartesian equations of motion of a system with constraints: Molecular dynamics of n-alkanes. J. Comput. Phys. 1977, 23, 327–341. [Google Scholar] [CrossRef]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A Gen. Phys. 1985, 31, 1695–1697. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Torrie, G.M.; Valleau, J.P. Nonphysical sampling distributions in Monte Carlo free-energy estimation: Umbrella sampling. J. Comput. Phys. 1977, 23, 187–199. [Google Scholar] [CrossRef]
- Tribello, G.A.; Bonomi, M.; Branduardi, D.; Camilloni, C.; Bussi, G. PLUMED 2: New feathers for an old bird. Comput. Phys. Commun. 2014, 185, 604–613. [Google Scholar] [CrossRef]
- Khalkhali, M.; Kazemi, N.; Zhang, H.; Liu, Q. Wetting at the nanoscale: A molecular dynamics study. J. Chem. Phys. 2017, 146, 114704. [Google Scholar] [CrossRef]
- Wang, F.-C.; Zhao, Y.-P. Contact angle hysteresis at the nanoscale: A molecular dynamics simulation study. Colloid Polym. Sci. 2013, 291, 307–315. [Google Scholar] [CrossRef]
- Walther, J.H.; Jaffe, R.; Halicioglu, T.; Koumoutsakos, P. Carbon Nanotubes in Water: Structural Characteristics and Energetics. J. Phys. Chem. B 2001, 105, 9980–9987. [Google Scholar] [CrossRef]
- Heinz, H.; Lin, T.-J.; Kishore Mishra, R.; Emami, F.S. Thermodynamically Consistent Force Fields for the Assembly of Inorganic, Organic, and Biological Nanostructures: The INTERFACE Force Field. Langmuir 2013, 29, 1754–1765. [Google Scholar] [CrossRef] [PubMed]
- Pramanik, C.; Gissinger, J.R.; Kumar, S.; Heinz, H. Carbon Nanotube Dispersion in Solvents and Polymer Solutions: Mechanisms, Assembly, and Preferences. ACS Nano 2017, 11, 12805–12816. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, K.; Choi, S.; Zhang, Z.; Jang, J. Molecular Simulation Study on the Wettability of a Surface Texturized with Hierarchical Pillars. Molecules 2023, 28, 4513. https://doi.org/10.3390/molecules28114513
Kim K, Choi S, Zhang Z, Jang J. Molecular Simulation Study on the Wettability of a Surface Texturized with Hierarchical Pillars. Molecules. 2023; 28(11):4513. https://doi.org/10.3390/molecules28114513
Chicago/Turabian StyleKim, Kiduk, Seyong Choi, Zhengqing Zhang, and Joonkyung Jang. 2023. "Molecular Simulation Study on the Wettability of a Surface Texturized with Hierarchical Pillars" Molecules 28, no. 11: 4513. https://doi.org/10.3390/molecules28114513
APA StyleKim, K., Choi, S., Zhang, Z., & Jang, J. (2023). Molecular Simulation Study on the Wettability of a Surface Texturized with Hierarchical Pillars. Molecules, 28(11), 4513. https://doi.org/10.3390/molecules28114513






