Opening a Band Gap in Biphenylene Monolayer via Strain: A First-Principles Study
Abstract
1. Introduction
2. Results and Discussion
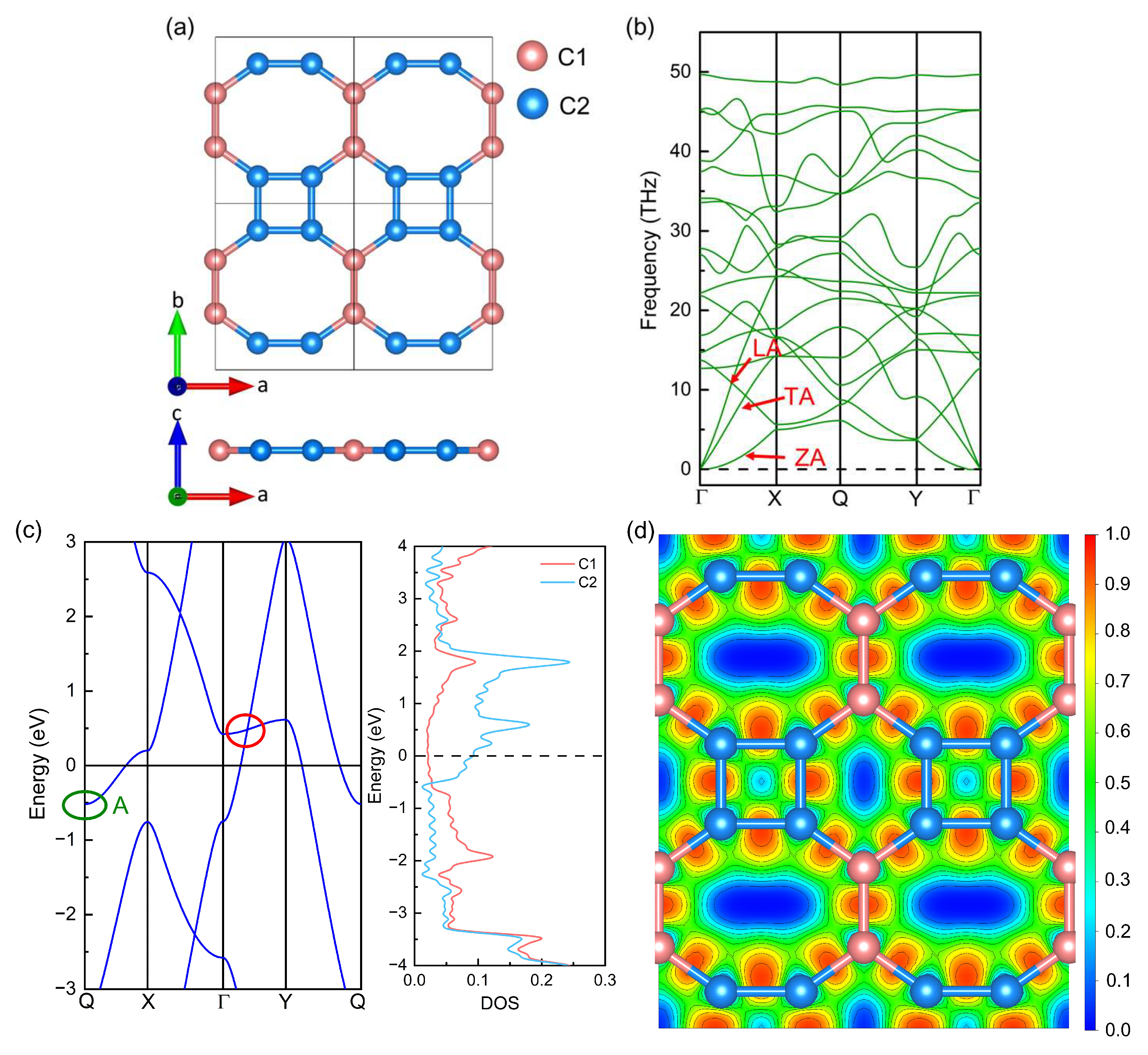
2.1. Geometrical Structure and Electronic Properties of Original Biphenylene
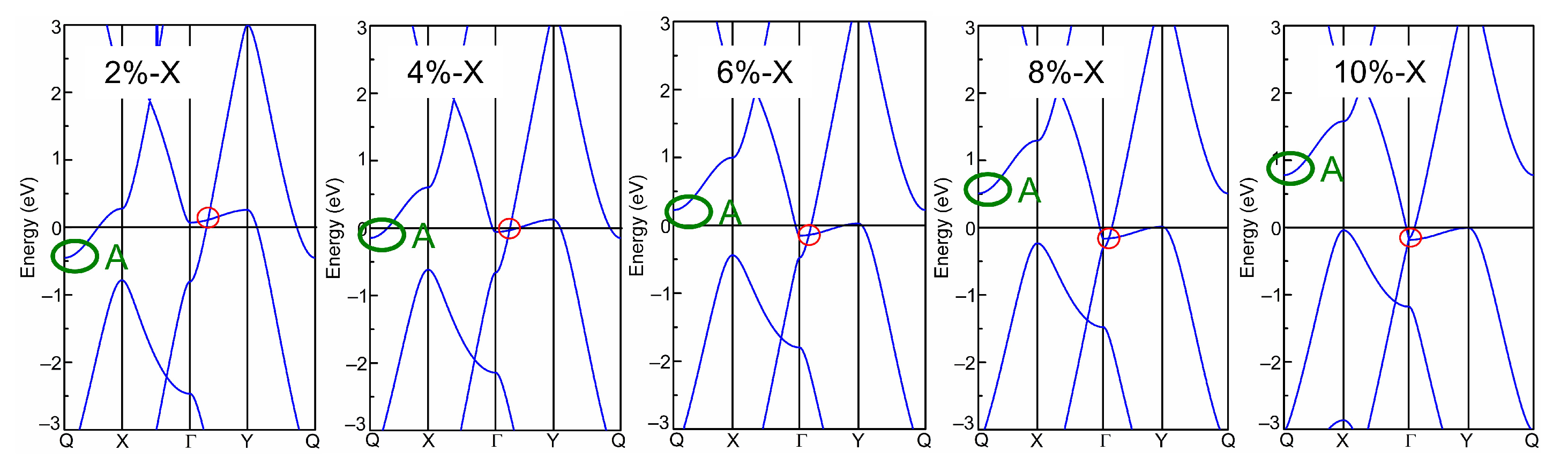
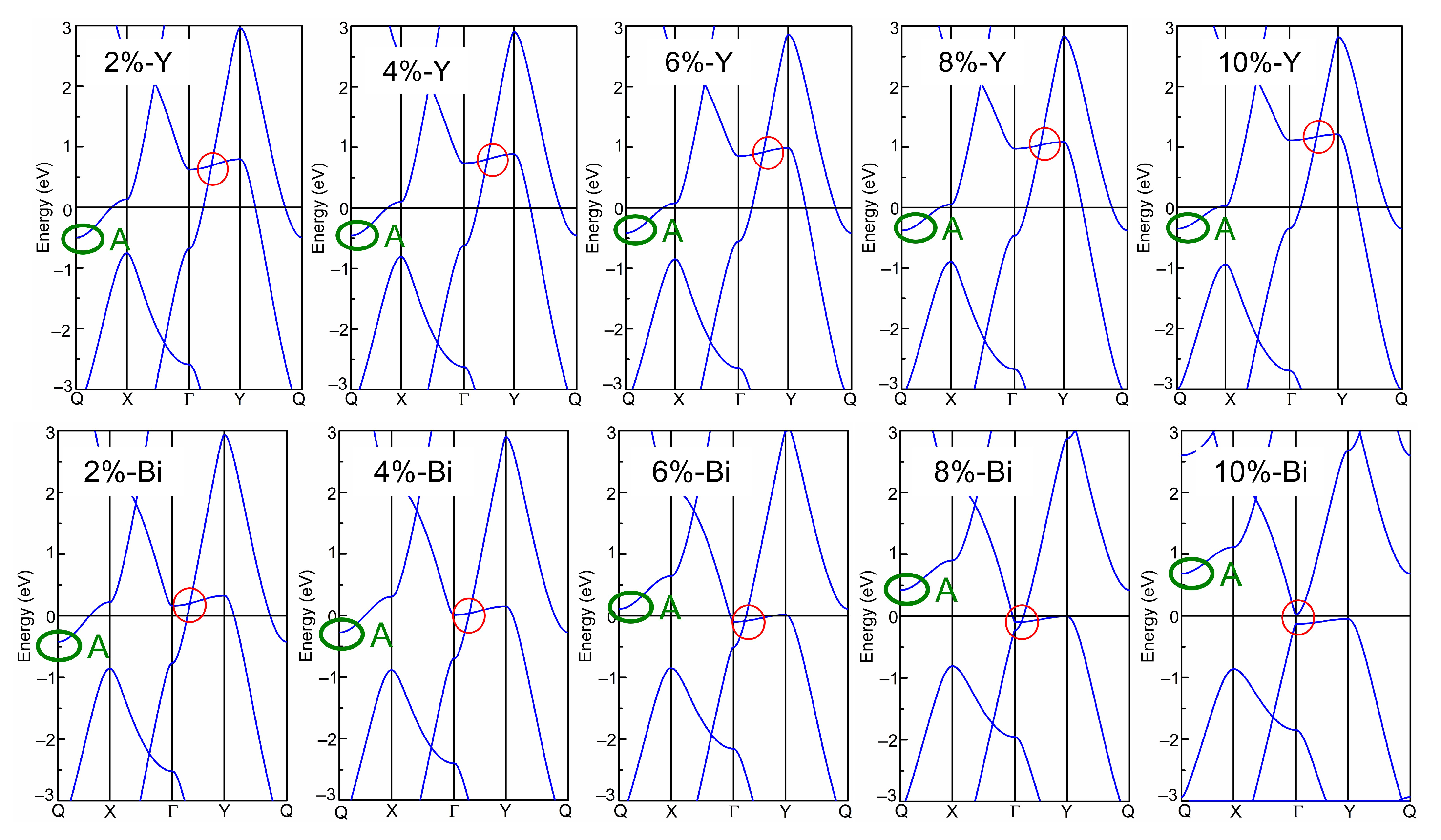
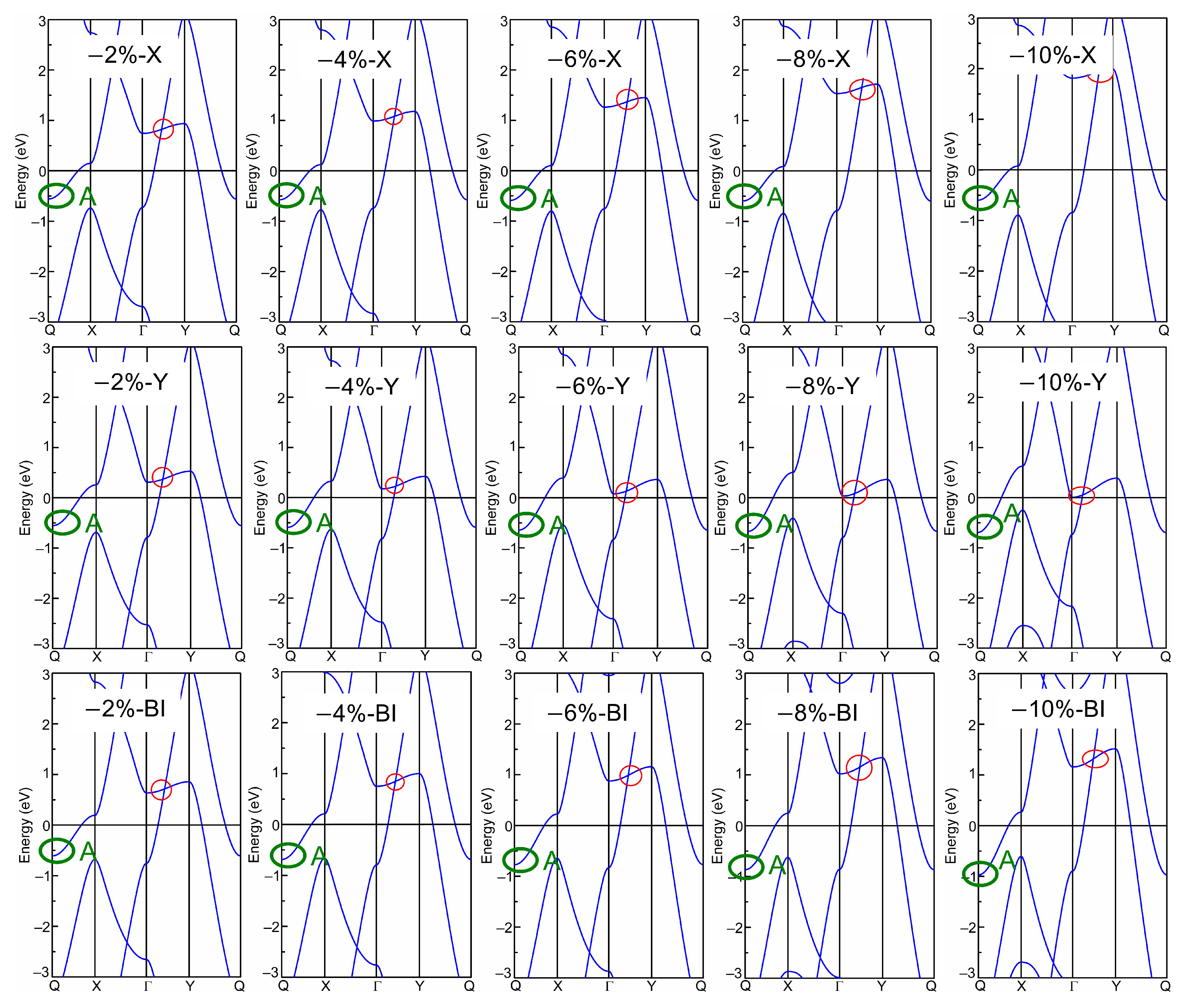
2.2. Effect of Strain on Electronic Properties and Geometrical Structure
2.3. Effect of Strain on Phonic Properties
3. Computational Details
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Fortuna, L.; Buscarino, A. Smart Materials. Materials 2022, 15, 6307. [Google Scholar] [CrossRef] [PubMed]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef] [PubMed]
- Bolotin, K.I.; Sikes, K.J.; Jiang, Z.; Klima, M.; Fudenberg, G.; Hone, J.; Kim, P.; Stormer, H.L. Ultrahigh electron mobility in suspended graphene. Solid State Commun. 2008, 146, 351–355. [Google Scholar] [CrossRef]
- Chen, S.; Moore, A.L.; Cai, W.; Suk, J.W.; An, J.; Mishra, C.; Amos, C.; Magnuson, C.W.; Kang, J.; Shi, L.; et al. Raman Measurements of Thermal Transport in Suspended Monolayer Graphene of Variable Sizes in Vacuum and Gaseous Environments. ACS Nano 2011, 5, 321–328. [Google Scholar] [CrossRef]
- Balandin, A.A. Thermal properties of graphene and nanostructured carbon materials. Nat. Mater. 2011, 10, 569–581. [Google Scholar] [CrossRef]
- Lee, C.; Wei, X.; Kysar, J.W.; Hone, J. Measurement of the Elastic Properties and Intrinsic Strength of Monolayer Graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef]
- Lherbier, A.; Blase, X.; Niquet, Y.-M.; Triozon, F.; Roche, S. Charge transport in chemically doped 2D graphene. Phys. Rev. Lett. 2008, 101, 036808. [Google Scholar] [CrossRef]
- Cui, Z.; Yang, K.; Shen, Y.; Yuan, Z.; Dong, Y.; Yuan, P.; Li, E. Toxic gas molecules adsorbed on intrinsic and defective WS2: Gas sensing and detection. Appl. Surf. Sci. 2023, 613, 155978. [Google Scholar] [CrossRef]
- Biel, B.; Blase, X.; Triozon, F.; Roche, S. Anomalous Doping Effects on Charge Transport in Graphene Nanoribbons. Phys. Rev. Lett. 2009, 102, 096803. [Google Scholar] [CrossRef]
- Yin, W.-J.; Xie, Y.-E.; Liu, L.-M.; Wang, R.-Z.; Wei, X.-L.; Lau, L.; Zhong, J.-X.; Chen, Y.-P. R-graphyne: A new two-dimensional carbon allotrope with versatile Dirac-like point in nanoribbons. J. Mater. Chem. A 2013, 1, 5341–5346. [Google Scholar] [CrossRef]
- Su, S.; Barlas, Y.; Li, J.; Shi, J.; Lake, R. Effect of intervalley interaction on band topology of commensurate graphene/EuO heterostructures. Phys. Rev. B 2017, 95, 075418. [Google Scholar] [CrossRef]
- Li, X.; Wang, Q.; Jena, P. ψ-Graphene: A New Metallic Allotrope of Planar Carbon with Potential Applications as Anode Materials for Lithium-Ion Batteries. J. Phys. Chem. Lett. 2017, 8, 3234–3241. [Google Scholar] [CrossRef]
- Yin, H.; Shi, X.; He, C.; Martinez-Canales, M.; Li, J.; Pickard, C.J.; Tang, C.; Ouyang, T.; Zhang, C.; Zhong, J. Stone-Wales graphene: A two-dimensional carbon semimetal with magic stability. Phys. Rev. B 2019, 99, 041405. [Google Scholar] [CrossRef]
- Wang, Z.; Zhou, X.-F.; Zhang, X.; Zhu, Q.; Dong, H.; Zhao, M.; Oganov, A.R. Phagraphene: A Low-Energy Graphene Allotrope Composed of 5–6–7 Carbon Rings with Distorted Dirac Cones. Nano Lett. 2015, 15, 6182–6186. [Google Scholar] [CrossRef]
- Zhang, S.; Zhou, J.; Wang, Q.; Chen, X.; Kawazoe, Y.; Jena, P. Penta-graphene: A new carbon allotrope. Proc. Natl. Acad. Sci. USA 2015, 112, 2372–2377. [Google Scholar] [CrossRef]
- Jiang, J.-W.; Leng, J.; Li, J.; Guo, Z.; Chang, T.; Guo, X.; Zhang, T. Twin graphene: A novel two-dimensional semiconducting carbon allotrope. Carbon 2017, 118, 370–375. [Google Scholar] [CrossRef]
- Fan, Q.; Yan, L.; Tripp, M.W.; Krejčí, O.; Dimosthenous, S.; Kachel, S.R.; Chen, M.; Foster, A.S.; Koert, U.; Liljeroth, P.; et al. Biphenylene network: A nonbenzenoid carbon allotrope. Science 2021, 372, 852–856. [Google Scholar] [CrossRef]
- Hudspeth, M.A.; Whitman, B.W.; Barone, V.; Peralta, J.E. Electronic Properties of the Biphenylene Sheet and Its One-Dimensional Derivatives. ACS Nano 2010, 4, 4565–4570. [Google Scholar] [CrossRef]
- Son, Y.-W.; Jin, H.; Kim, S. Magnetic Ordering, Anomalous Lifshitz Transition, and Topological Grain Boundaries in Two-Dimensional Biphenylene Network. Nano Lett. 2022, 22, 3112–3117. [Google Scholar] [CrossRef]
- Rublev, P.; Tkachenko, N.V.; Boldyrev, A.I. Overlapping electron density and the global delocalization of π-aromatic fragments as the reason of conductivity of the biphenylene network. J. Comput. Chem. 2022, 44, 168–178. [Google Scholar] [CrossRef]
- Pereira, M.L.; da Cunha, W.F.; de Sousa, R.T.; Amvame Nze, G.D.; Galvão, D.S.; Ribeiro, L.A. On the mechanical properties and fracture patterns of the nonbenzenoid carbon allotrope (biphenylene network): A reactive molecular dynamics study. Nanoscale 2022, 14, 3200–3211. [Google Scholar] [CrossRef] [PubMed]
- Mortazavi, B.; Shapeev, A.V. Anisotropic mechanical response, high negative thermal expansion, and outstanding dynamical stability of biphenylene monolayer revealed by machine-learning interatomic potentials. FlatChem 2022, 32, 100347. [Google Scholar] [CrossRef]
- Luo, Y.; Ren, C.; Xu, Y.; Yu, J.; Wang, S.; Sun, M. A first principles investigation on the structural, mechanical, electronic, and catalytic properties of biphenylene. Sci. Rep. 2021, 11, 19008. [Google Scholar] [CrossRef] [PubMed]
- Ying, P.; Liang, T.; Du, Y.; Zhang, J.; Zeng, X.; Zhong, Z. Thermal transport in planar sp2-hybridized carbon allotropes: A comparative study of biphenylene network, pentaheptite and graphene. Int. J. Heat Mass Transfer 2022, 183, 122060. [Google Scholar] [CrossRef]
- Veeravenkata, H.P.; Jain, A. Density functional theory driven phononic thermal conductivity prediction of biphenylene: A comparison with graphene. Carbon 2021, 183, 893–898. [Google Scholar] [CrossRef]
- Liu, P.-F.; Li, J.; Zhang, C.; Tu, X.-H.; Zhang, J.; Zhang, P.; Wang, B.-T.; Singh, D.J. Type-II Dirac cones and electron-phonon interaction in monolayer biphenylene from first-principles calculations. Phys. Rev. B 2021, 104, 235422. [Google Scholar] [CrossRef]
- Zhang, P.; Ouyang, T.; Tang, C.; He, C.; Li, J.; Zhang, C.; Hu, M.; Zhong, J. The intrinsic thermal transport properties of the biphenylene network and the influence of hydrogenation: A first-principles study. J. Mater. Chem. C 2021, 9, 16945–16951. [Google Scholar] [CrossRef]
- Ren, X.; Wang, K.; Yu, Y.; Zhang, D.; Zhang, G.; Cheng, Y. Tuning the mechanical anisotropy of biphenylene by boron and nitrogen doping. Comp. Mater. Sci. 2023, 222, 112119. [Google Scholar] [CrossRef]
- Ren, K.; Shu, H.; Huo, W.; Cui, Z.; Xu, Y. Tuning electronic, magnetic and catalytic behaviors of biphenylene network by atomic doping. Nanotechnology 2022, 33, 345701. [Google Scholar] [CrossRef]
- Baryshnikov, G.V.; Karaush, N.N.; Minaev, B.F. The Electronic Structure of Heteroannelated Cyclooctatetraenes and their UV-Vis Absorption Spectra. Chem. Heterocycl. Compd. 2014, 50, 349–363. [Google Scholar] [CrossRef]
- Chandra Jha, P.; Minaev, B.; Ågren, H. One-and two-photon Absorptions in asymmetrically substituted free-base porphyrins: A density functional theory study. J. Chem. Phys. 2008, 128, 074302. [Google Scholar] [CrossRef]
- Minaev, B.F.; Zakharov, I.I.; Zakharova, O.I.; Tselishtev, A.B.; Filonchook, A.V.; Shevchenko, A.V. Photochemical Water Decomposition in the Troposphere: DFT Study with a Symmetrized Kohn–Sham Formalism. Chem. Phys. Chem. 2010, 11, 4028–4034. [Google Scholar] [CrossRef]
- Ren, K.; Tang, W.; Sun, M.; Cai, Y.; Cheng, Y.; Zhang, G. A direct Z-scheme PtS2/arsenene van der Waals heterostructure with high photocatalytic water splitting efficiency. Nanoscale 2020, 12, 17281–17289. [Google Scholar] [CrossRef]
- Choi, S.-M.; Jhi, S.-H.; Son, Y.-W. Effects of strain on electronic properties of graphene. Phys. Rev. B 2010, 81, 081407. [Google Scholar] [CrossRef]
- Wang, K.; Hu, T.; Jia, F.; Zhao, G.; Liu, Y.; Solovyev, I.V.; Pyatakov, A.P.; Zvezdin, A.K.; Ren, W. Magnetic and electronic properties of Cr2Ge2Te6 monolayer by strain and electric-field engineering. Appl. Phys. Lett. 2019, 114, 092405. [Google Scholar] [CrossRef]
- Zhang, R.; Yu, G.; Gao, Y.; Huang, X.; Chen, W. Applying surface strain and coupling with pure or N/B-doped graphene to successfully achieve high HER catalytic activity in 2D layered SnP3-based nanomaterials: A first-principles investigation. Inorg. Chem. Front. 2020, 7, 647–658. [Google Scholar] [CrossRef]
- Lin, L.; Sherrell, P.; Liu, Y.; Lei, W.; Zhang, S.; Zhang, H.; Wallace, G.G.; Chen, J. Engineered 2D Transition Metal Dichalcogenides-A Vision of Viable Hydrogen Evolution Reaction Catalysis. Adv. Energy Mater. 2020, 10, 1903870. [Google Scholar] [CrossRef]
- You, B.; Tang, M.T.; Tsai, C.; Abild-Pedersen, F.; Zheng, X.; Li, H. Enhancing Electrocatalytic Water Splitting by Strain Engineering. Adv. Mater. 2019, 31, 1807001. [Google Scholar] [CrossRef]
- Zhang, C.; Yu, G.; Ku, R.; Huang, X.; Chen, W. Theoretical investigation on the high HER catalytic activity of 2D layered GeP3 nanomaterials and its further enhancement by applying the surface strain or coupling with graphene. Appl. Surf. Sci. 2019, 481, 272–280. [Google Scholar] [CrossRef]
- Yin, Y.; Li, D.; Hu, Y.; Ding, G.; Zhou, H.; Zhang, G. Phonon stability and phonon transport of graphene-like borophene. Nanotechnology 2020, 31, 315709. [Google Scholar] [CrossRef]
- McQuade, G.A.; Plaut, A.S.; Usher, A.; Martin, J. The thermal expansion coefficient of monolayer, bilayer, and trilayer graphene derived from the strain induced by cooling to cryogenic temperatures. Appl. Phys. Lett. 2021, 118, 203101. [Google Scholar] [CrossRef]
- Cenker, J.; Sivakumar, S.; Xie, K.; Miller, A.; Thijssen, P.; Liu, Z.; Dismukes, A.; Fonseca, J.; Anderson, E.; Zhu, X.; et al. Reversible strain-induced magnetic phase transition in a van der Waals magnet. Nat. Nanotechnol. 2022, 17, 256–261. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.; Yu, J.; Yuan, S. Strain-tunable magnetic and electronic properties of monolayer CrI3. Phys. Chem. Chem. Phys. 2019, 21, 7750–7755. [Google Scholar] [CrossRef] [PubMed]
- Gui, G.; Li, J.; Zhong, J. Band structure engineering of graphene by strain: First-principles calculations. Phys. Rev. B 2008, 78, 075435. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, C.; Liang, S.-J.; Ma, Z.; Xu, K.; Liu, X.; Zhang, L.; Admasu, A.S.; Cheong, S.-W.; Wang, L.; et al. Strain-Sensitive Magnetization Reversal of a van der Waals Magnet. Adv. Mater. 2020, 32, 2004533. [Google Scholar] [CrossRef]
- Metzger, C.; Rémi, S.; Liu, M.; Kusminskiy, S.V.; Castro Neto, A.H.; Swan, A.K.; Goldberg, B.B. Biaxial Strain in Graphene Adhered to Shallow Depressions. Nano Lett. 2010, 10, 6–10. [Google Scholar] [CrossRef]
- Chu, Y.; Sang, Y.; Liu, Y.; Liu, Y.; Xu, Z.; Chen, J.; Liu, F.; Li, S.; Sun, B.; Wang, X. Reduced thermal conductivity of epitaxial GaAsSb on InP due to lattice mismatch induced biaxial strain. J. Appl. Phys. 2021, 130, 015106. [Google Scholar] [CrossRef]
- Feng, Z.; Fang, M.; Li, R.; Ma, B.; Wang, H.; Ding, H.; Su, G.; Tao, K.; Tang, Y.; Dai, X. Tuning lattice strain in biphenylene for enhanced electrocatalytic oxygen reduction reaction in proton exchange membrane fuel cells. Int. J. Hydrog. Energy 2022, 47, 36294–36305. [Google Scholar] [CrossRef]
- Yang, G.; Hu, Y.; Qiu, Z.; Li, B.-L.; Zhou, P.; Li, D.; Zhang, G. Abnormal strain-dependent thermal conductivity in biphenylene monolayer using machine learning interatomic potential. Appl. Phys. Lett. 2023, 122, 082202. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comp. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio simulation of the metal/nonmetal transition in expanded fluid mercury. Phys. Rev. B 1997, 55, 7539. [Google Scholar] [CrossRef]
- Gonze, X. Adiabatic density-functional perturbation theory. Phys. Rev. A 1995, 52, 1096. [Google Scholar] [CrossRef]
- Tan, Z.W.; Wang, J.-S.; Gan, C.K. First-principles study of heat transport properties of graphene nanoribbons. Nano Lett. 2011, 11, 214–219. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, W.; Cheng, Y.; Zhang, M.; Wang, H.; Zhang, G. Magnetic order-dependent phonon properties in 2D magnet CrI3. Nanoscale 2021, 13, 10882–10890. [Google Scholar] [CrossRef]
- Bafekry, A.; Faraji, M.; Fadlallah, M.M.; Jappor, H.R.; Karbasizadeh, S.; Ghergherehchi, M.; Gogova, D. Biphenylene monolayer as a two-dimensional nonbenzenoid carbon allotrope: A first-principles study. J. Phys. Condens. Matter 2022, 34, 015001. [Google Scholar] [CrossRef]
- Silvi, B.; Savin, A. Classification of chemical bonds based on topological analysis of electron localization functions. Nature 1994, 371, 683–686. [Google Scholar] [CrossRef]
- Wang, V.; Xu, N.; Liu, J.-C.; Tang, G.; Geng, W.-T. VASPKIT: A user-friendly interface facilitating high-throughput computing and analysis using VASP code. Comput. Phys. Commun. 2021, 267, 108033. [Google Scholar] [CrossRef]
- Cai, Y.; Zhang, G.; Zhang, Y.-W. Layer-dependent Band Alignment and Work Function of Few-Layer Phosphorene. Sci. Rep. 2014, 4, 6677. [Google Scholar] [CrossRef]
- Qiao, J.; Kong, X.; Hu, Z.-X.; Yang, F.; Ji, W. High-mobility transport anisotropy and linear dichroism in few-layer black phosphorus. Nat. Commun. 2014, 5, 4475. [Google Scholar] [CrossRef] [PubMed]
- Yu, S.; Xiong, H.D.; Eshun, K.; Yuan, H.; Li, Q. Phase transition, effective mass and carrier mobility of MoS2 monolayer under tensile strain. Appl. Surf. Sci. 2015, 325, 27–32. [Google Scholar] [CrossRef]
- Cai, Y.; Zhang, A.; Feng, Y.P.; Zhang, C.; Teoh, H.F.; Ho, G.W. Strain effects on work functions of pristine and potassium-decorated carbon nanotubes. J. Chem. Phys. 2009, 131, 224701. [Google Scholar] [CrossRef] [PubMed]
- Robinson, J.A.; LaBella, M.; Zhu, M.; Hollander, M.; Kasarda, R.; Hughes, Z.; Trumbull, K.; Cavalero, R.; Snyder, D. Contacting graphene. Appl. Phys. Lett. 2011, 98, 053103. [Google Scholar] [CrossRef]
- Lee, E.J.H.; Balasubramanian, K.; Weitz, R.T.; Burghard, M.; Kern, K. Contact and edge effects in graphene devices. Nat. Nanotechnol. 2008, 3, 486–490. [Google Scholar] [CrossRef]
- Wang, K.; Ren, K.; Zhang, D.; Cheng, Y.; Zhang, G. Phonon properties of biphenylene monolayer by first-principles calculations. Appl. Phys. Lett. 2022, 121, 042203. [Google Scholar] [CrossRef]
- Cai, Y.; Lan, J.; Zhang, G.; Zhang, Y.-W. Lattice vibrational modes and phonon thermal conductivity of monolayer MoS2. Phys. Rev. B 2014, 89, 035438. [Google Scholar] [CrossRef]
- Cai, Y.; Ke, Q.; Zhang, G.; Feng, Y.P.; Shenoy, V.B.; Zhang, Y.-W. Giant Phononic Anisotropy and Unusual Anharmonicity of Phosphorene: Interlayer Coupling and Strain Engineering. Adv. Funct. Mater. 2015, 25, 2230–2236. [Google Scholar] [CrossRef]
- O’Connell, A.D.; Hofheinz, M.; Ansmann, M.; Bialczak, R.C.; Lenander, M.; Lucero, E.; Neeley, M.; Sank, D.; Wang, H.; Weides, M.; et al. Quantum ground state and single-phonon control of a mechanical resonator. Nature 2010, 464, 697–703. [Google Scholar] [CrossRef]
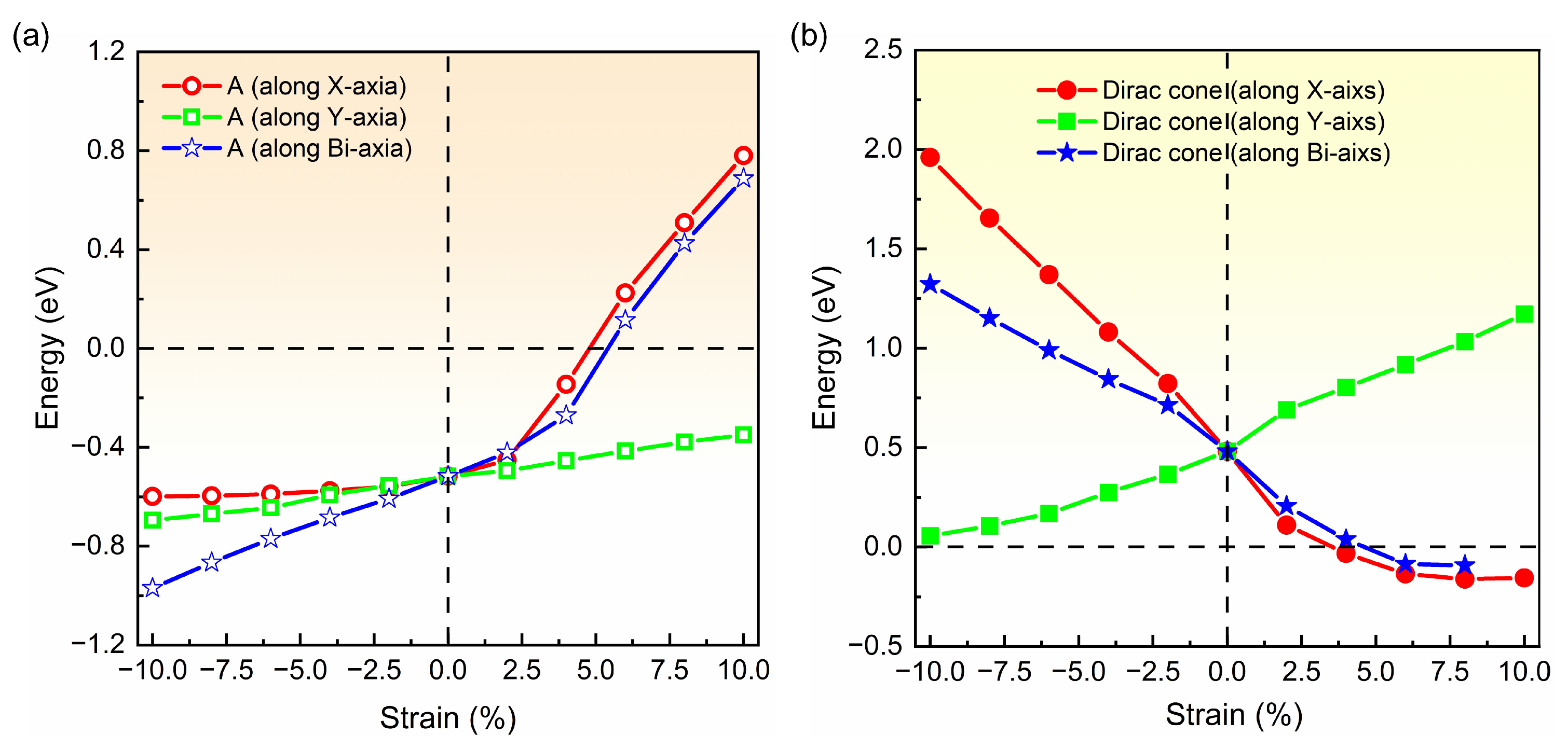
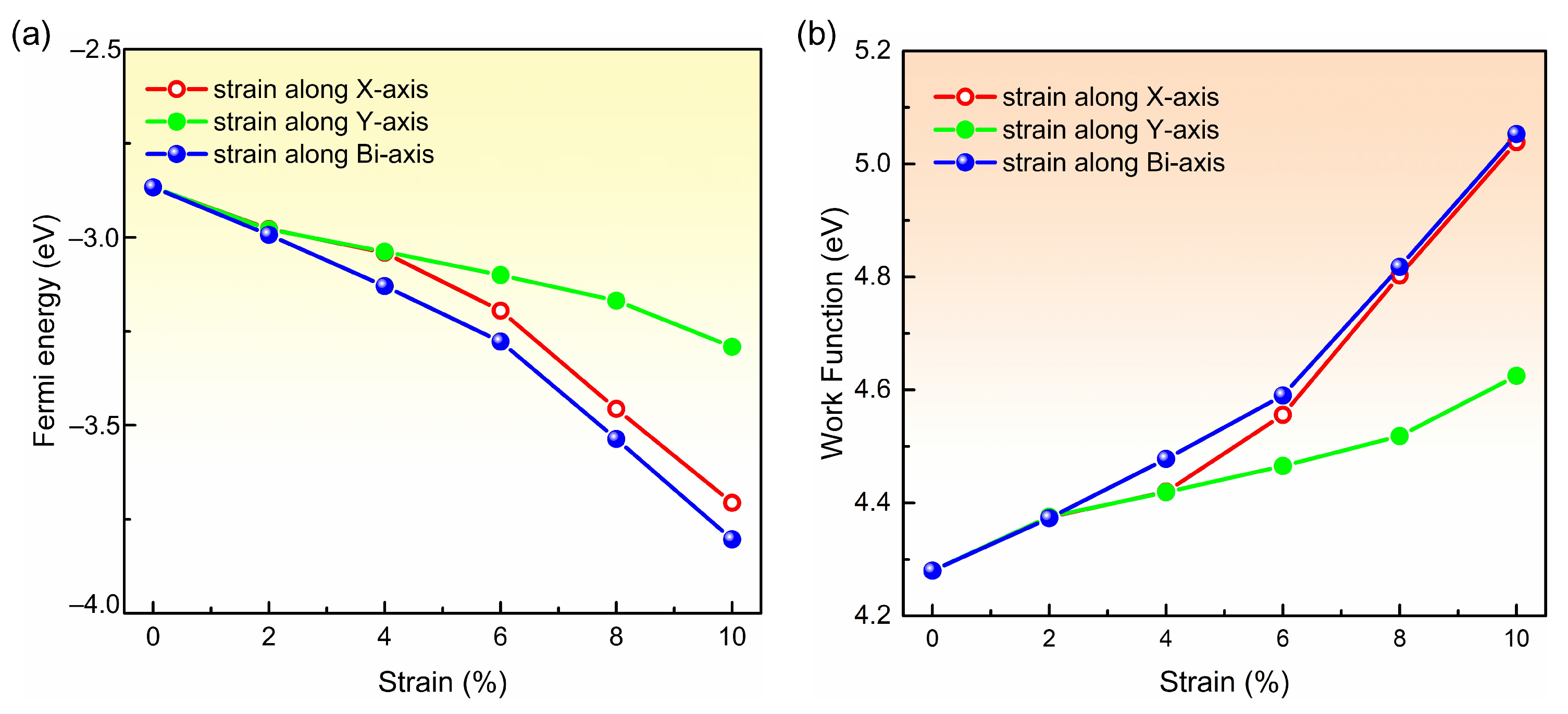

| Strain (%) | C1-C1 Bond Length (Å) | C1-C2 Bond Length (Å) | C2-C2 Bond Length (Å) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| x | y | bi | x | y | bi | x | y | bi | |
| −10 | 1.372 | 1.344 | 1.272 | 1.334 | 1.347 | 1.266 | 1.340 | 1.424 | 1.303 |
| −8 | 1.388 | 1.361 | 1.305 | 1.348 | 1.359 | 1.295 | 1.360 | 1.433 | 1.333 |
| −6 | 1.403 | 1.380 | 1.338 | 1.362 | 1.371 | 1.323 | 1.382 | 1.441 | 1.363 |
| −4 | 1.419 | 1.400 | 1.373 | 1.377 | 1.383 | 1.352 | 1.405 | 1.448 | 1.393 |
| −2 | 1.433 | 1.423 | 1.408 | 1.433 | 1.395 | 1.380 | 1.428 | 1.452 | 1.422 |
| 0 | 1.448 | 1.448 | 1.448 | 1.407 | 1.407 | 1.407 | 1.456 | 1.456 | 1.456 |
| 2 | 1.462 | 1.473 | 1.491 | 1.422 | 1.420 | 1.433 | 1.491 | 1.456 | 1.496 |
| 4 | 1.475 | 1.503 | 1.537 | 1.436 | 1.432 | 1.459 | 1.530 | 1.460 | 1.534 |
| 6 | 1.489 | 1.536 | 1.593 | 1.447 | 1.443 | 1.480 | 1.576 | 1.464 | 1.588 |
| 8 | 1.498 | 1.575 | 1.664 | 1.462 | 1.453 | 1.497 | 1.612 | 1.466 | 1.630 |
| 10 | 1.504 | 1.620 | 1.751 | 1.476 | 1.461 | 1.510 | 1.649 | 1.466 | 1.673 |
| Strain (%) | C1-C1-C2 Bond Angle (°) | C2-C2-C1 Bond Angle (°) | C2-C2-C2 Bond Angle (°) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| x | y | bi | x | y | bi | x | y | bi | |
| −10 | 129.94 | 119.81 | 124.69 | 140.06 | 150.19 | 145.31 | 90 | 90 | 90 |
| −8 | 128.82 | 121.03 | 124.75 | 141.18 | 148.97 | 145.26 | 90 | 90 | 90 |
| −6 | 127.75 | 122.17 | 124.82 | 142.24 | 147.83 | 145.18 | 90 | 90 | 90 |
| −4 | 126.76 | 123.22 | 124.85 | 143.24 | 146.78 | 145.15 | 90 | 90 | 90 |
| −2 | 125.80 | 124.14 | 124.87 | 144.20 | 145.86 | 145.13 | 90 | 90 | 90 |
| 0 | 125.03 | 125.03 | 125.03 | 144.97 | 144.97 | 144.97 | 90 | 90 | 90 |
| 2 | 124.42 | 125.74 | 125.27 | 145.58 | 144.26 | 144.73 | 90 | 90 | 90 |
| 4 | 123.97 | 126.52 | 125.41 | 146.03 | 143.48 | 144.59 | 90 | 90 | 90 |
| 6 | 123.61 | 127.22 | 125.84 | 146.39 | 142.78 | 144.16 | 90 | 90 | 90 |
| 8 | 123.05 | 127.83 | 125.70 | 146.95 | 142.17 | 144.30 | 90 | 90 | 90 |
| 10 | 122.56 | 128.27 | 125.33 | 147.44 | 141.73 | 144.67 | 90 | 90 | 90 |
| Phonon Mode | Phonon Frequency f (THz) | ||||||
|---|---|---|---|---|---|---|---|
| −10%-x | −10%-y | −10%-bi | Unstrained | 10%-x | 10%-y | 10%-bi | |
| 8.55 | 8.52 | −2.75 | 12.70 | 13.19 | 13.51 | 14.02 | |
| 10.68 | 9.01 | −12.09 | 13.73 | 14.16 | 15.81 | 14.57 | |
| 11.27 | 11.08 | −3.66 | 14.69 | 15.76 | 16.11 | 16.38 | |
| 16.82 | 15.79 | 14.64 | 16.68 | 17.28 | 16.80 | 17.25 | |
| 18.71 | 20.74 | 14.55 | 21.86 | 22.25 | 21.61 | 17.70 | |
| 20.43 | 20.91 | 17.44 | 22.20 | 22.88 | 22.39 | 19.65 | |
| 28.75 | 28.59 | 29.18 | 27.03 | 25.42 | 23.90 | 21.95 | |
| 35.18 | 35.54 | 41.40 | 27.80 | 25.91 | 26.51 | 22.32 | |
| 37.37 | 39.21 | 42.07 | 33.58 | 27.89 | 28.79 | 24.40 | |
| 38.72 | 40.32 | 48.28 | 34.11 | 28.48 | 30.19 | 26.10 | |
| 48.42 | 46.37 | 58.39 | 37.46 | 32.40 | 30.60 | 26.93 | |
| 48.71 | 48.56 | 60.51 | 38.84 | 33.28 | 31.61 | 28.70 | |
| 53.06 | 51.11 | 64.10 | 45.18 | 37.02 | 39.02 | 31.79 | |
| 59.40 | 53.26 | 67.81 | 45.24 | 38.15 | 41.41 | 34.52 | |
| 62.69 | 58.46 | 74.44 | 49.67 | 41.62 | 42.39 | 37.00 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, Y.; Ren, K.; Wei, Y.; Yang, D.; Cui, Z.; Wang, K. Opening a Band Gap in Biphenylene Monolayer via Strain: A First-Principles Study. Molecules 2023, 28, 4178. https://doi.org/10.3390/molecules28104178
Hou Y, Ren K, Wei Y, Yang D, Cui Z, Wang K. Opening a Band Gap in Biphenylene Monolayer via Strain: A First-Principles Study. Molecules. 2023; 28(10):4178. https://doi.org/10.3390/molecules28104178
Chicago/Turabian StyleHou, Yinlong, Kai Ren, Yu Wei, Dan Yang, Zhen Cui, and Ke Wang. 2023. "Opening a Band Gap in Biphenylene Monolayer via Strain: A First-Principles Study" Molecules 28, no. 10: 4178. https://doi.org/10.3390/molecules28104178
APA StyleHou, Y., Ren, K., Wei, Y., Yang, D., Cui, Z., & Wang, K. (2023). Opening a Band Gap in Biphenylene Monolayer via Strain: A First-Principles Study. Molecules, 28(10), 4178. https://doi.org/10.3390/molecules28104178








