Mechanical Constraint Effect on DNA Persistence Length
Abstract
1. Introduction
2. Model and Methods
2.1. Relationship between DNA Persistence Length and Critical Buckling Length
2.2. Molecular Dynamics Simulation
3. Results and Discussion
3.1. Identify Effective Mechanical Constraints in Different Experiments
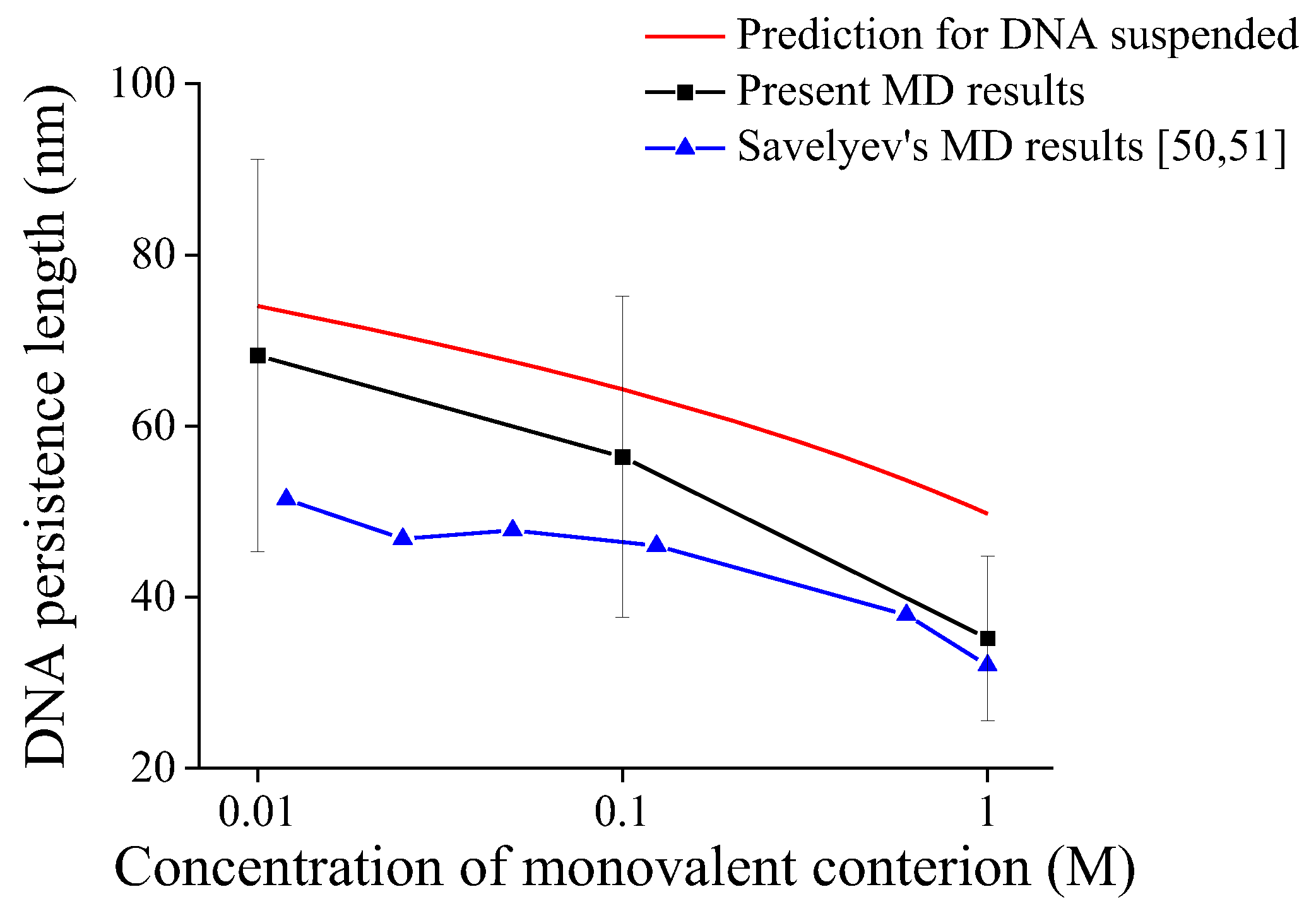
3.2. End-Constraint Effect on Ionic Dependence of DNA Persistence Length
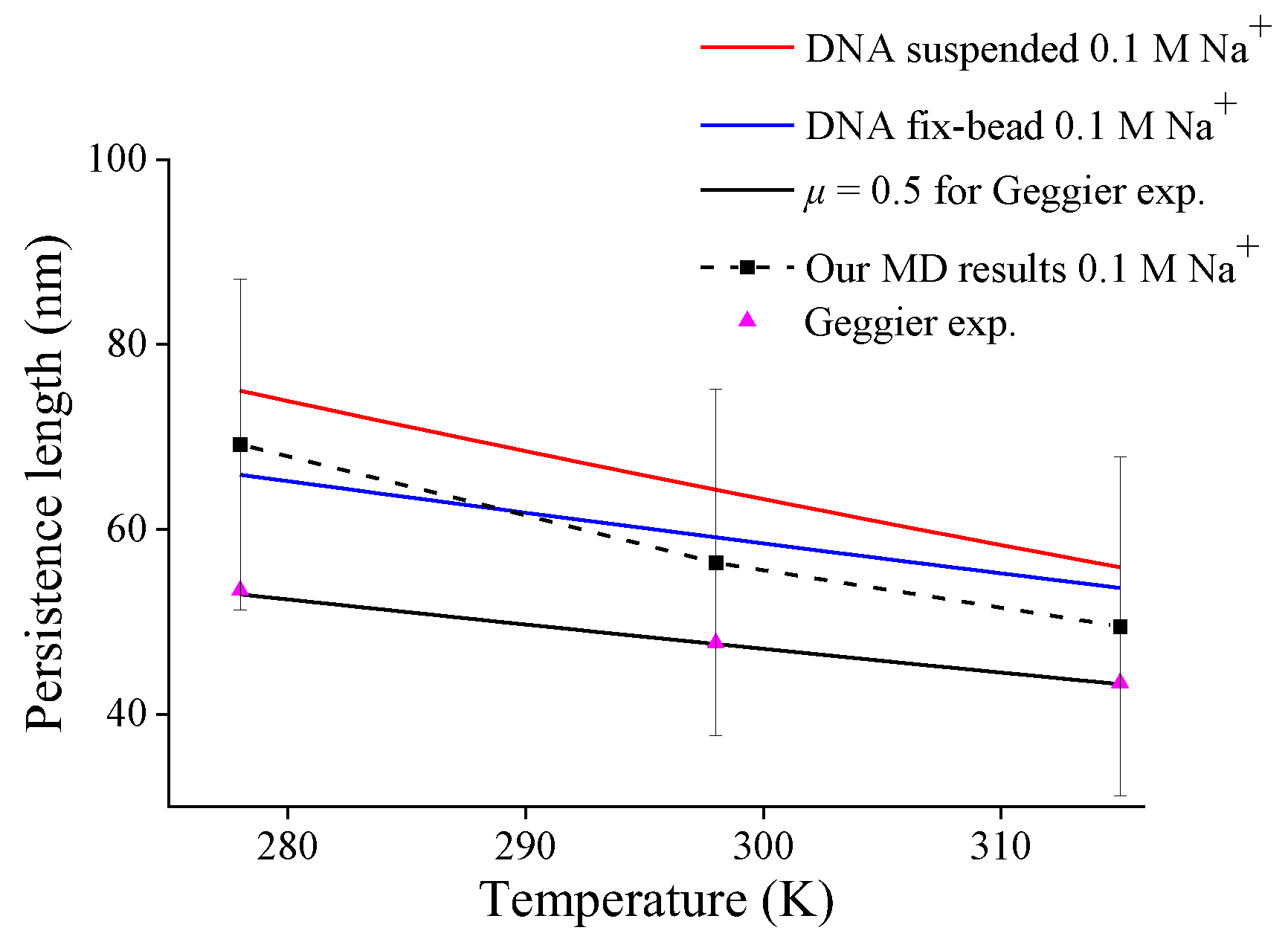
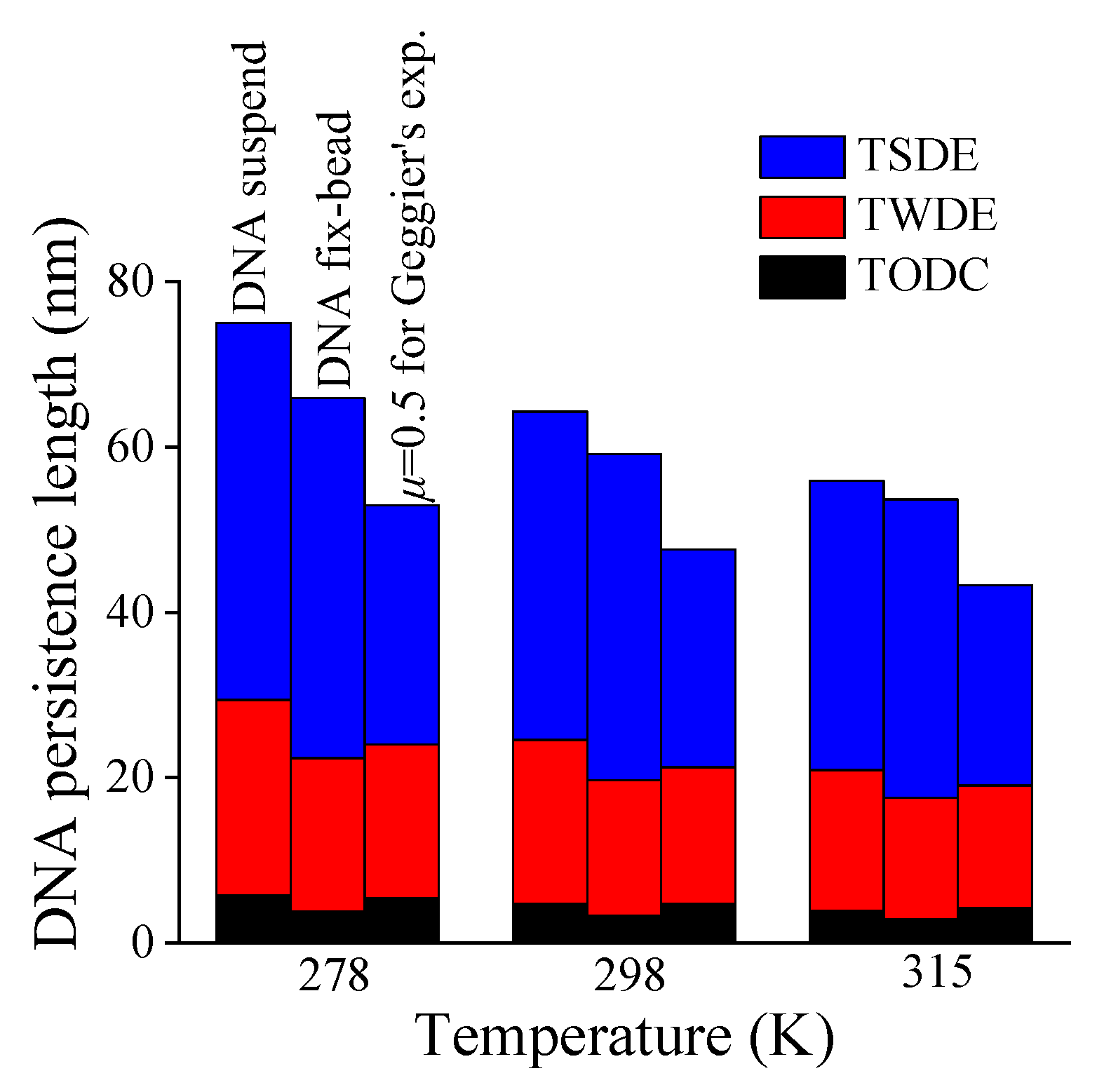
3.3. End-Constraint Effect on Temperature Dependence of DNA Persistence Length
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Evilevitch, A. The mobility of packaged phage genome controls ejection dynamics. eLife 2018, 7, 21. [Google Scholar] [CrossRef] [PubMed]
- Hagerman, P.J. Flexibility of DNA. Annu. Rev. Biophys. Biophys. Chem. 1988, 17, 265–286. [Google Scholar] [CrossRef]
- Peters, J.P.; Maher, L.J. DNA curvature and flexibility in vitro and in vivo. Q. Rev. Biophys. 2010, 43, 23–63. [Google Scholar] [CrossRef]
- Zhang, C.Y.; Zhang, N.H. Influence of microscopic interactions on the flexible mechanical properties of viral DNA. Biophys. J. 2018, 115, 763–772. [Google Scholar] [CrossRef] [PubMed]
- Rothemund, P.W.K. Folding DNA to create nanoscale shapes and patterns. Nature 2006, 440, 297–302. [Google Scholar] [CrossRef]
- Bloomfield, V.A.; Crothers, D.M.; Ignacio Tinoco, J. Nucleic Acids: Structures, Properties and Functions; University Science Books: Sausalito, CA, USA, 2000. [Google Scholar]
- Nelson, P. Biological Physics: Energy, Information, Life; W.H. Freeman and Co.: New York, NY, USA, 2004. [Google Scholar]
- Peterlin, A. Light scattering by very stiff chain molecules. Nature 1953, 171, 259–260. [Google Scholar] [CrossRef]
- Brunet, A.; Tardin, C.; Salomé, L.; Rousseau, P.; Destainville, N.; Manghi, M. Dependence of DNA persistence length on ionic strength of solutions with monovalent and divalent salts: A joint theory-experiment study. Macromolecules 2015, 48, 3641–3652. [Google Scholar] [CrossRef]
- Baumann, C.G.; Smith, S.B.; Bloomfield, V.A.; Bustamante, C. Ionic effects on the elasticity of single DNA molecules. Proc. Natl. Acad. Sci. USA 1997, 94, 6185–6190. [Google Scholar] [CrossRef]
- Geggier, S.; Kotlyar, A.; Vologodskii, A. Temperature dependence of DNA persistence length. Nucleic Acids Res. 2011, 39, 1419–1426. [Google Scholar] [CrossRef]
- Bazoni, R.F.; Lima, C.H.M.; Ramos, E.B.; Rocha, M.S. Force-dependent persistence length of DNA–intercalator complexes measured in single molecule stretching experiments. Soft Matter 2015, 11, 4306–4314. [Google Scholar] [CrossRef]
- Maaloum, M.; Muller, P.; Harlepp, S. DNA-intercalator interactions: Structural and physical analysis using atomic force microscopy in solution. Soft Matter 2013, 9, 11233–11240. [Google Scholar] [CrossRef]
- Mantelli, S.; Muller, P.; Harlepp, S.; Maaloum, M. Conformational analysis and estimation of the persistence length of DNA using atomic force microscopy in solution. Soft Matter 2011, 7, 3412–3416. [Google Scholar] [CrossRef]
- Borochov, N.; Eisenberg, H.; Kam, Z. Dependence of DNA conformation on the concentration of salt. Biopolymers 1981, 20, 231–235. [Google Scholar] [CrossRef] [PubMed]
- Sobel, E.; Harpst, J. Effects of Na+ on the persistence length and excluded volume of T7 bacteriophage DNA. Biopolymers 1991, 31, 1559–1564. [Google Scholar] [CrossRef] [PubMed]
- Cairney, K.L.; Harrington, R.E. Flow birefringence of T7 phage DNA: Dependence on salt concentration. Biopolymers 1982, 21, 923–934. [Google Scholar] [CrossRef] [PubMed]
- Ranjith, P.; Yan, J.; Marko, J.F. Nucleosome hopping and sliding kinetics determined from dynamics of single chromatin fibers in Xenopus egg extracts. Proc. Natl. Acad. Sci. USA 2007, 104, 13649–13654. [Google Scholar] [CrossRef]
- Fabian, R.; Gaire, S.; Tyson, C.; Adhikari, R.; Pegg, I.; Sarkar, A. A horizontal magnetic tweezers for studying single DNA molecules and DNA-binding proteins. Molecules 2021, 26, 4781. [Google Scholar] [CrossRef]
- Bustamante, C.; Smith, S.B.; Liphardt, J.; Smith, D. Single-molecule studies of DNA mechanics. Curr. Opin. Struct. Biol. 2000, 10, 279–285. [Google Scholar] [CrossRef]
- Seol, Y.; Li, J.; Nelson, P.C.; Perkins, T.T.; Betterton, M. Elasticity of short DNA molecules: Theory and experiment for contour lengths of 0.6–7 μm. Biophys. J. 2007, 93, 4360–4373. [Google Scholar] [CrossRef]
- Nir, G.; Chetrit, E.; Vivante, A.; Garini, Y.; Berkovich, R. The role of near-wall drag effects in the dynamics of tethered DNA under shear flow. Soft Matter 2018, 14, 2219–2226. [Google Scholar] [CrossRef]
- Segall, D.E.; Nelson, P.C.; Phillips, R. Volume-exclusion effects in tethered-particle experiments: Bead size matters. Phys. Rev. Lett. 2006, 96, 088306. [Google Scholar] [CrossRef]
- Lipiec, E.; Sofińska, K.; Seweryn, S.; Wilkosz, N.; Szymonski, M. Revealing DNA structure at liquid/solid interfaces by AFM-based high-resolution imaging and molecular spectroscopy. Molecules 2021, 26, 6476. [Google Scholar] [CrossRef] [PubMed]
- Wiggins, P.A.; Van Der Heijden, T.; Moreno-Herrero, F.; Spakowitz, A.; Phillips, R.; Widom, J.; Dekker, C.; Nelson, P.C. High flexibility of DNA on short length scales probed by atomic force microscopy. Nat. Nanotechnol. 2006, 1, 137–141. [Google Scholar] [CrossRef] [PubMed]
- Bednar, J.; Furrer, P.; Katritch, V.; Stasiak, A.; Dubochet, J.; Stasiak, A. Determination of DNA persistence length by cryo-electron microscopy. Separation of the static and dynamic contributions to the apparent persistence length of DNA. J. Mol. Biol. 1995, 254, 579–594. [Google Scholar] [CrossRef] [PubMed]
- Faas, F.G.A.; Rieger, B.; van Vliet, L.J.; Cherny, D.I. DNA deformations near charged surfaces: Electron and atomic force microscopy views. Biophys. J. 2009, 97, 1148–1157. [Google Scholar] [CrossRef] [PubMed]
- Ariel, G.; Andelman, D. Persistence length of a strongly charged rodlike polyelectrolyte in the presence of salt. Phys. Rev. E 2003, 67, 11. [Google Scholar] [CrossRef]
- Golestanian, R.; Kardar, M.; Liverpool, T.B. Collapse of stiff polyelectrolytes due to counterion fluctuations. Phys. Rev. Lett. 1999, 82, 4456–4459. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Rouzina, I.; Shklovskii, B.I. Negative electrostatic contribution to the bending rigidity of charged membranes and polyelectrolytes screened by multivalent counterions. Phys. Rev. E 1999, 60, 7032–7039. [Google Scholar] [CrossRef]
- Odijk, T. Polyelectrolytes near the rod limit. J. Polym. Sci. 1977, 15, 477–483. [Google Scholar] [CrossRef]
- Skolnick, J.; Fixman, M. Electrostatic persistence length of a wormlike polyelectrolyte. Macromolecules 1977, 10, 944–948. [Google Scholar] [CrossRef]
- Podgornik, R.; Hansen, P.L.; Parsegian, V.A. Elastic moduli renormalization in self-interacting stretchable polyelectrolytes. J. Chem. Phys. 2000, 113, 9343–9350. [Google Scholar] [CrossRef]
- Manning, G.S. The persistence length of DNA is reached from the persistence length of its null isomer through an internal electrostatic stretching force. Biophys. J. 2006, 91, 3607–3616. [Google Scholar] [CrossRef] [PubMed]
- Kurzthaler, C. Elastic behavior of a semiflexible polymer in 3D subject to compression and stretching forces. Soft Matter 2018, 14, 7634–7644. [Google Scholar] [CrossRef] [PubMed]
- Thompson, D.W.; Thompson, D.A.W. On Growth and Form; Cambridge University Press: Cambridge, UK, 1942. [Google Scholar]
- Zandi, R.; Dragnea, B.; Travesset, A.; Podgornik, R. On virus growth and form. Phys. Rep. 2020, 847, 1–102. [Google Scholar] [CrossRef]
- Si, D.-Q.; Liu, X.-Y.; Wu, J.-B.; Hu, G.-H. Modulation of DNA conformation in electrolytic nanodroplets. Phys. Chem. Chem. Phys. 2022, 24, 6002–6010. [Google Scholar] [CrossRef]
- Manning, G.S. Counterion condensation theory constructed from different models. Phys. A 1996, 231, 236–253. [Google Scholar] [CrossRef]
- Manning, G.S. Electrostatic free energy of the DNA double helix in counterion condensation theory. Biophys. Chem. 2002, 101, 461–473. [Google Scholar] [CrossRef]
- Russel, W.B.; Saville, D.A.; Schowalter, W.R. Colloidal Dispersions; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Tadmor, R.; Hernández-Zapata, E.; Chen, N.; Pincus, P.; Israelachvili, J.N. Debye length and double-layer forces in polyelectrolyte solutions. Macromolecules 2002, 35, 2380–2388. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Gere, J.M. Theory of Elastic Stability, 2nd ed.; Dover Publications, Inc.: New York, NY, USA, 1989. [Google Scholar]
- Wu, Y.-Y.; Bao, L.; Zhang, X.; Tan, Z.-J. Flexibility of short DNA helices with finite-length effect: From base pairs to tens of base pairs. J. Chem. Phys. 2015, 142, 125103. [Google Scholar] [CrossRef]
- Lindorff-Larsen, K.; Piana, S.; Palmo, K.; Maragakis, P.; Klepeis, J.L.; Dror, R.O.; Shaw, D.E. Improved side-chain torsion potentials for the Amber ff99SB protein force field. Proteins 2010, 78, 1950–1958. [Google Scholar] [CrossRef]
- Van Der Spoel, D.; Lindahl, E.; Hess, B.; Groenhof, G.; Mark, A.E.; Berendsen, H.J. GROMACS: Fast, flexible, and free. J. Comput. Chem. 2005, 26, 1701–1718. [Google Scholar] [CrossRef] [PubMed]
- Lavery, R.; Moakher, M.; Maddocks, J.H.; Petkeviciute, D.; Zakrzewska, K. Conformational analysis of nucleic acids revisited: Curves+. Nucleic Acids Res. 2009, 37, 5917–5929. [Google Scholar] [CrossRef] [PubMed]
- Frontali, C.; Dore, E.; Ferrauto, A.; Gratton, E.; Bettini, A.; Pozzan, M.R.; Valdevit, E. An absolute method for the determination of the persistence length of native DNA from electron micrographs. Biopolymers 1979, 18, 1353–1373. [Google Scholar] [CrossRef] [PubMed]
- Rizzo, V.; Schellman, J. Flow dichroism of T7 DNA as a function of salt concentration. Biopolymers 1981, 20, 2143–2163. [Google Scholar] [CrossRef]
- Savelyev, A. Do monovalent mobile ions affect DNA’s flexibility at high salt content? Phys. Chem. Chem. Phys. 2012, 14, 2250–2254. [Google Scholar] [CrossRef]
- Savelyev, A.; Papoian, G.A. Chemically accurate coarse graining of double-stranded DNA. Proc. Natl. Acad. Sci. USA 2010, 107, 20340–20345. [Google Scholar] [CrossRef]
- Malmberg, C.; Maryott, A. Dielectric constant of water from 0° to 100 °C. J. Res. Natl. Bur. Stand. 1956, 56, 1–8. [Google Scholar] [CrossRef]
- Schroeder, D.V. An Introduction to Thermal Physics; Addison Wesley Longman: Reading, UK, 1999. [Google Scholar]
- Marmur, J.; Doty, P. Determination of the base composition of deoxyribonucleic acid from its thermal denaturation temperature. J. Mol. Biol. 1962, 5, 109–118. [Google Scholar] [CrossRef]
- Kahn, J.D. DNA, flexibly flexible. Biophys. J. 2014, 107, 282–284. [Google Scholar] [CrossRef][Green Version]
- Yuan, C.; Chen, H.; Lou, X.W.; Archer, L.A. DNA bending stiffness on small length scales. Phys. Rev. Lett. 2008, 100, 018102. [Google Scholar] [CrossRef]
- Fields, A.P.; Meyer, E.A.; Cohen, A.E. Euler buckling and nonlinear kinking of double-stranded DNA. Nucleic Acids Res. 2013, 41, 9881–9890. [Google Scholar] [CrossRef] [PubMed]
- Wilber, J.P. Buckling of graphene layers supported by rigid substrates. J. Comput. Theor. Nanosci. 2010, 7, 2338–2348. [Google Scholar] [CrossRef]
- Abu-Salih, S.; Elata, D. Analytic postbuckling solution of a pre-stressed infinite beam bonded to a linear elastic foundation. Int. J. Solids Struct. 2005, 42, 6048–6058. [Google Scholar] [CrossRef]
- Wang, J.-Z.; Li, R.-H. Stretching strongly confined semiflexible polymer chain. Appl. Math. Mech. Engl. 2014, 35, 1233–1238. [Google Scholar] [CrossRef]
- Vafabakhsh, R.; Ha, T. Extreme bendability of DNA less than 100 base pairs long revealed by single-molecule cyclization. Science 2012, 337, 1097–1101. [Google Scholar] [CrossRef] [PubMed]
- Bažant, Z.P.; Cedolin, L. Stability of Structures: Elastic, Inelastic, Fracture and Damage Theories; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2010. [Google Scholar]




| Experiment Method | DNA Constrained States | Temperature K | Kind of Salt | Concentrations of Salt mol/L | DNA Contour Length bp | DNA Persistence Length nm | Fitted Effective Length Factor μ | Fitted Critical Buckling Length lcb nm | Goodness of Fit |
|---|---|---|---|---|---|---|---|---|---|
| Rayleigh light scattering [16] | Suspended in solution | 293 | NaH2PO4 | 0.005–3 | 39,000 | 38–78 | 0.668 | 9.16 | 0.893 |
| Linear dichroism [49] | Suspended in solution | 298 | NaCl | 0.002–1 | 39,000 | 40.2–66.9 | 1.256 | 4.18 | 0.652 |
| Flow birefringence [17] | Suspended in solution | 298 | NaCl | 0.002–1 | 39,000 | 39.9–72.3 | 1.065 | 5.14 | 0.815 |
| Dynamic light scattering [15] | Suspended in solution | 298 | NaCl | 0.005–4 | 6594 | 32.0–91.4 | 1.095 | 5.31 | 0.761 |
| Optical tweezers [10] | Fix-bead | 298 | NaCl | 0.00186–0.586 | 48,500 | 45.1–96.3 | 0.635 | 9.64 | 0.511 |
| Tethered-particle method [9] | Fix-bead | 298 | NaCl | 0.015–3.33 | 2060 | 34.9–54.7 | 0.835 | 6.52 | 0.824 |
| Tethered-particle method [9] | Fix-bead | 298 | NaCl | 0.015–3.33 | 1201 | 31.3–52.2 | 0.686 | 7.88 | 0.911 |
| Tethered-particle method [9] | Fix-bead | 298 | MgCl2 | 0.003–0.104 | 2060 | 37.2–50.1 | 0.736 | 8.95 | 0.614 |
| Tethered-particle method [9] | Fix-bead | 298 | MgCl2 | 0.003–0.104 | 1201 | 36.7–46.1 | 0.700 | 9.18 | 0.624 |
| Electron microscopy [48] | Adsorbed on substrate | 298 | NaCl | 0.03–0.5 | 4800 | 54.5–145.6 | 0.489 | 15.83 | 0.404 |
| DNA Constrained States | Effective Length Factors μ ± Standard Deviation | Critical Buckling Length lcb ± Standard Deviation |
|---|---|---|
| Suspended in solution | 1.021 ± 0.250 | 5.95 ± 2.20 |
| Fix-bead constraint | 0.718 ± 0.075 | 8.43 ± 1.25 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.-Y.; Zhang, N.-H. Mechanical Constraint Effect on DNA Persistence Length. Molecules 2022, 27, 7769. https://doi.org/10.3390/molecules27227769
Zhang C-Y, Zhang N-H. Mechanical Constraint Effect on DNA Persistence Length. Molecules. 2022; 27(22):7769. https://doi.org/10.3390/molecules27227769
Chicago/Turabian StyleZhang, Cheng-Yin, and Neng-Hui Zhang. 2022. "Mechanical Constraint Effect on DNA Persistence Length" Molecules 27, no. 22: 7769. https://doi.org/10.3390/molecules27227769
APA StyleZhang, C.-Y., & Zhang, N.-H. (2022). Mechanical Constraint Effect on DNA Persistence Length. Molecules, 27(22), 7769. https://doi.org/10.3390/molecules27227769







