Modeling the Electronic Absorption Spectra of the Indocarbocyanine Cy3
Abstract
:1. Introduction
2. Computational Methods
3. Results and Discussion
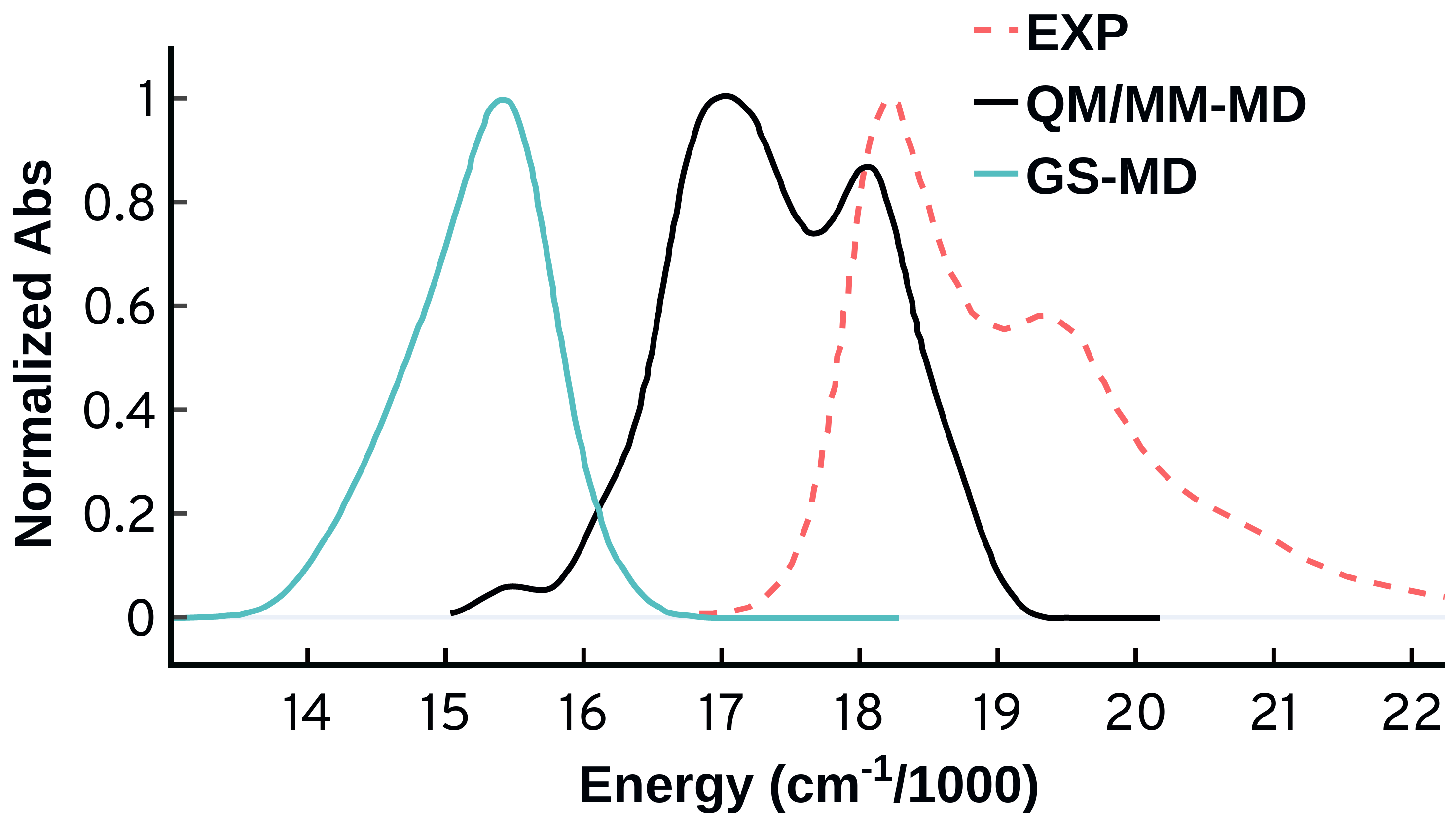
3.1. Classical Ensemble: QM/MM-MD
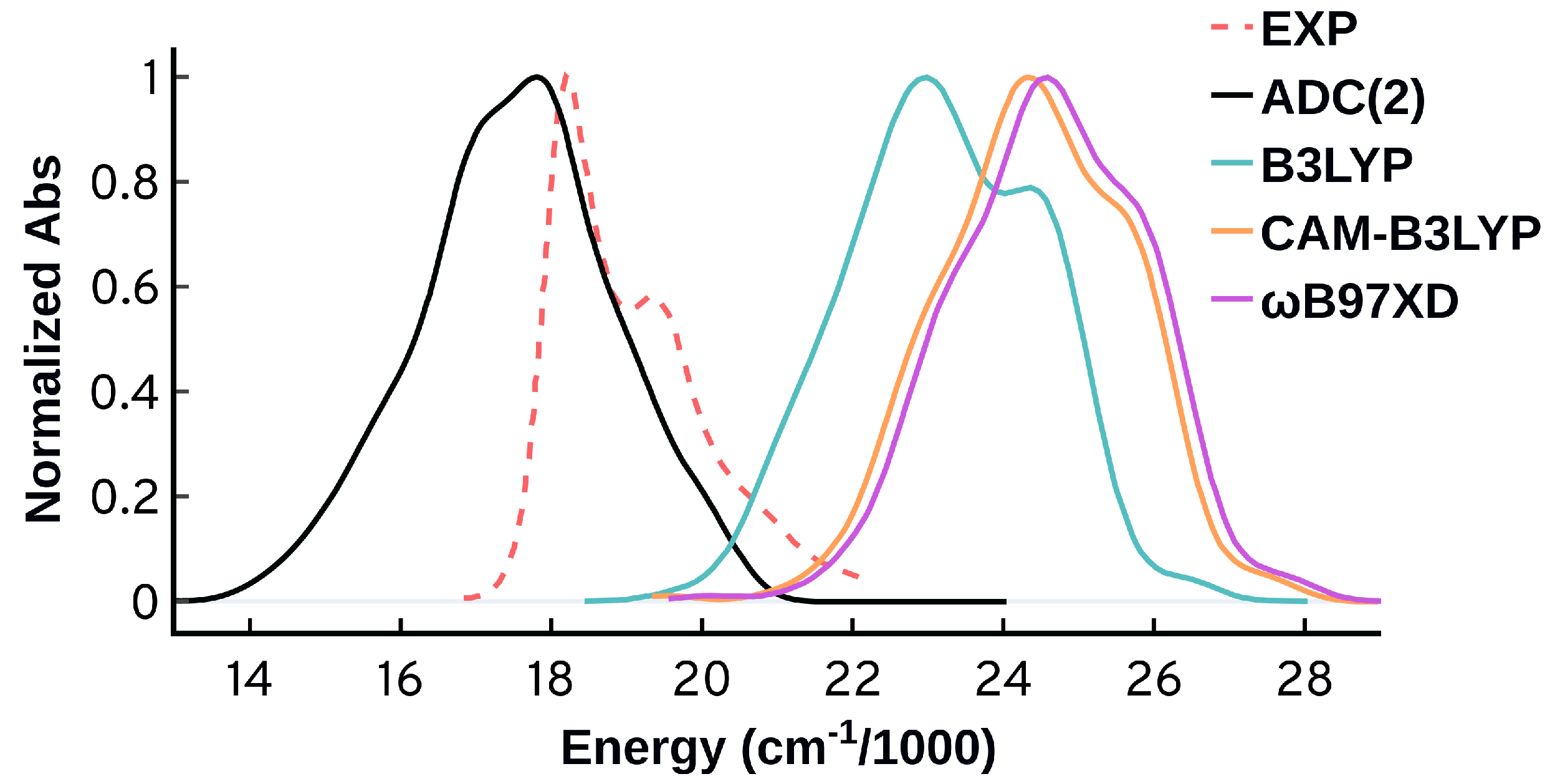
3.1.1. Electronic Structure Effect
3.1.2. Sampling Effect
3.1.3. Force Field Effect
3.2. Quantum Ensemble: Wigner-Distribution
3.3. Franck-Condon Approach
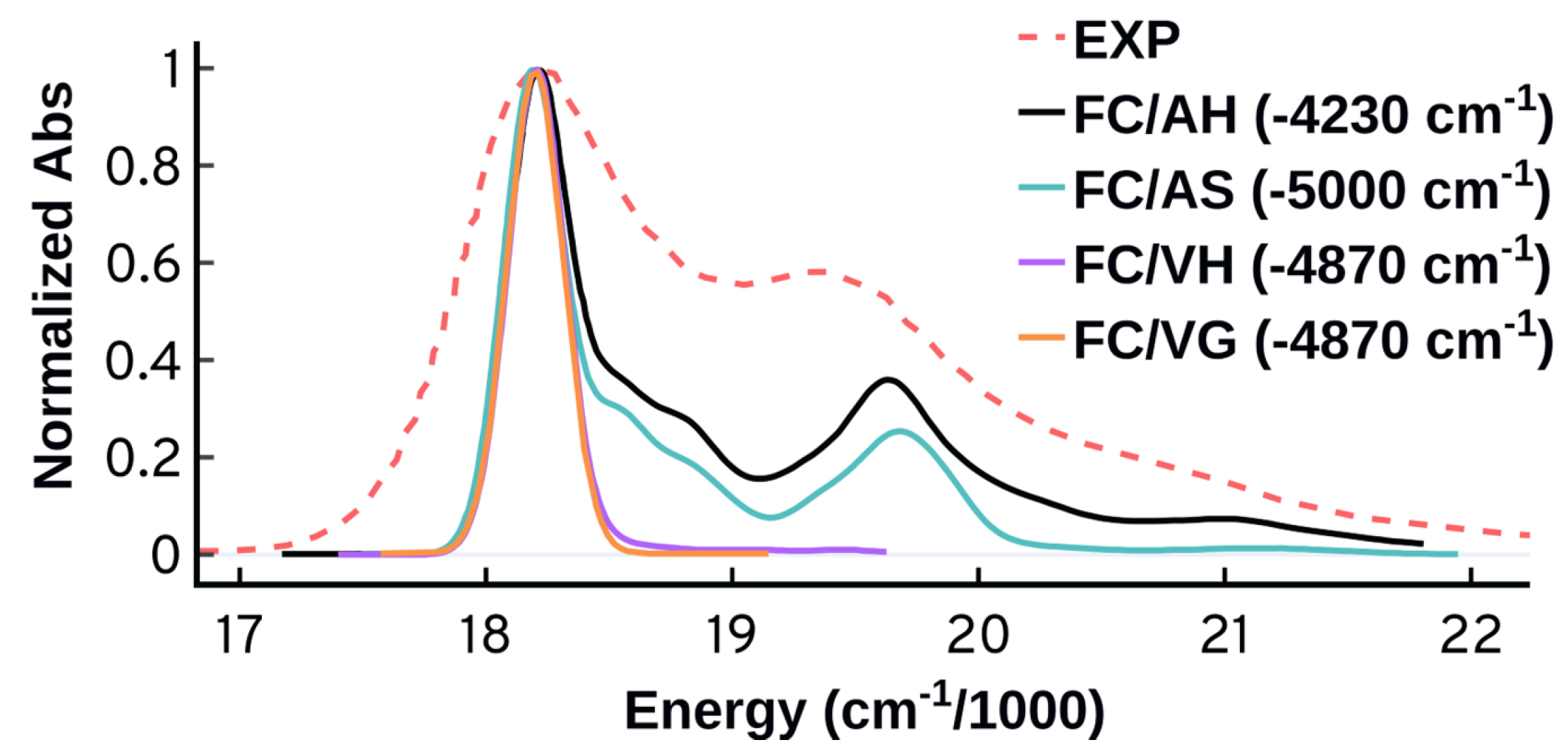
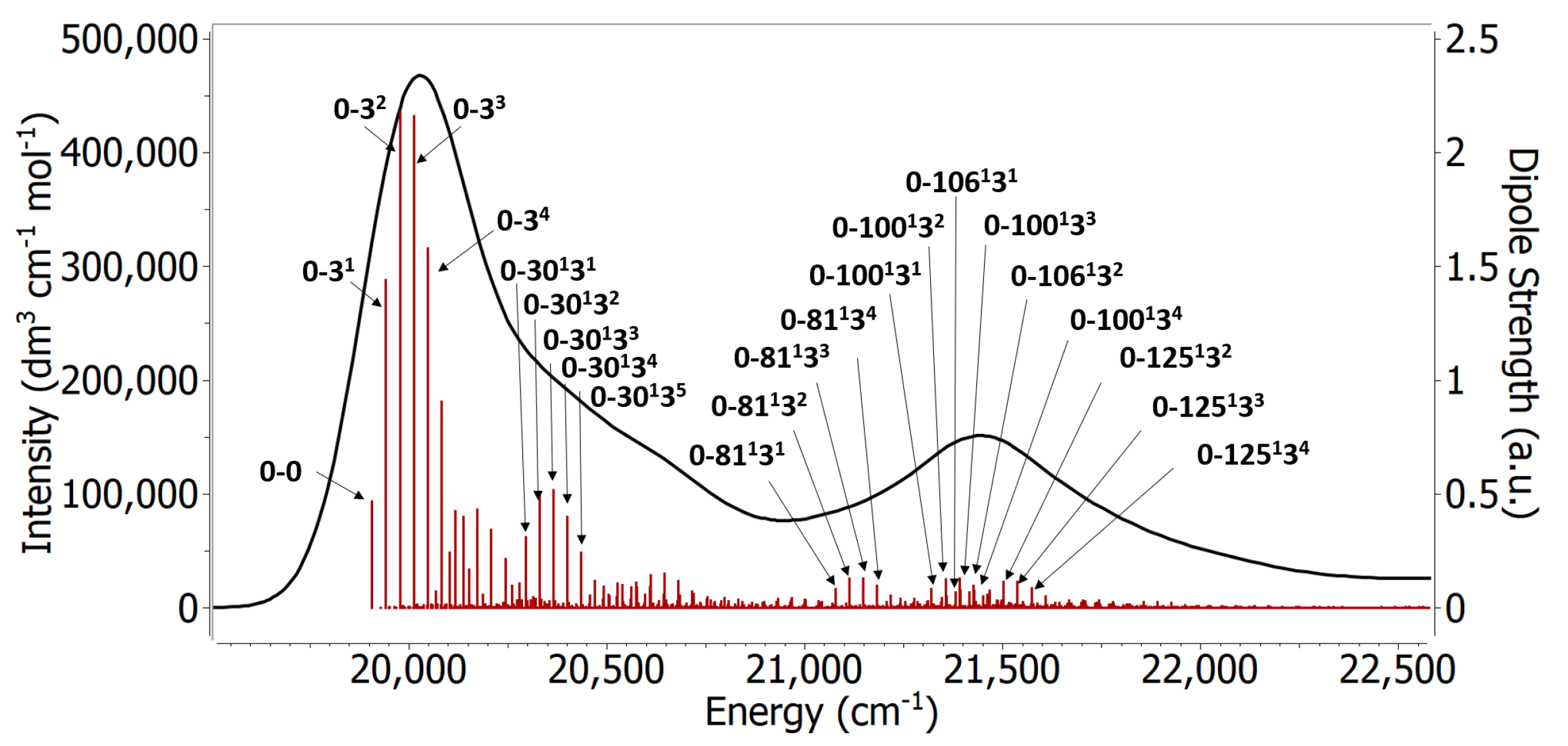
3.3.1. Spectra at 0 K
3.3.2. Origin of the Vibronic Structure
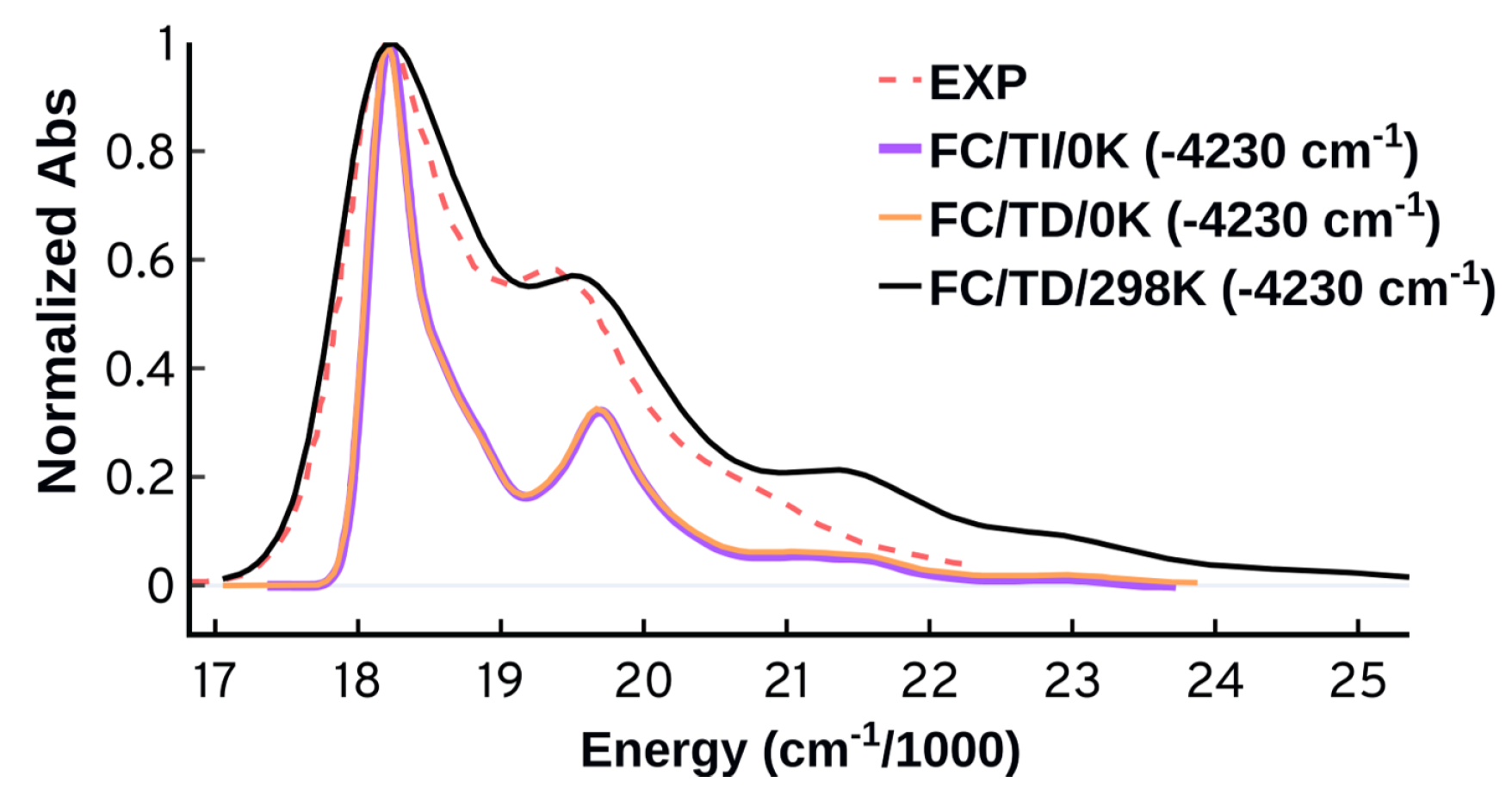
3.3.3. Temperature Effect
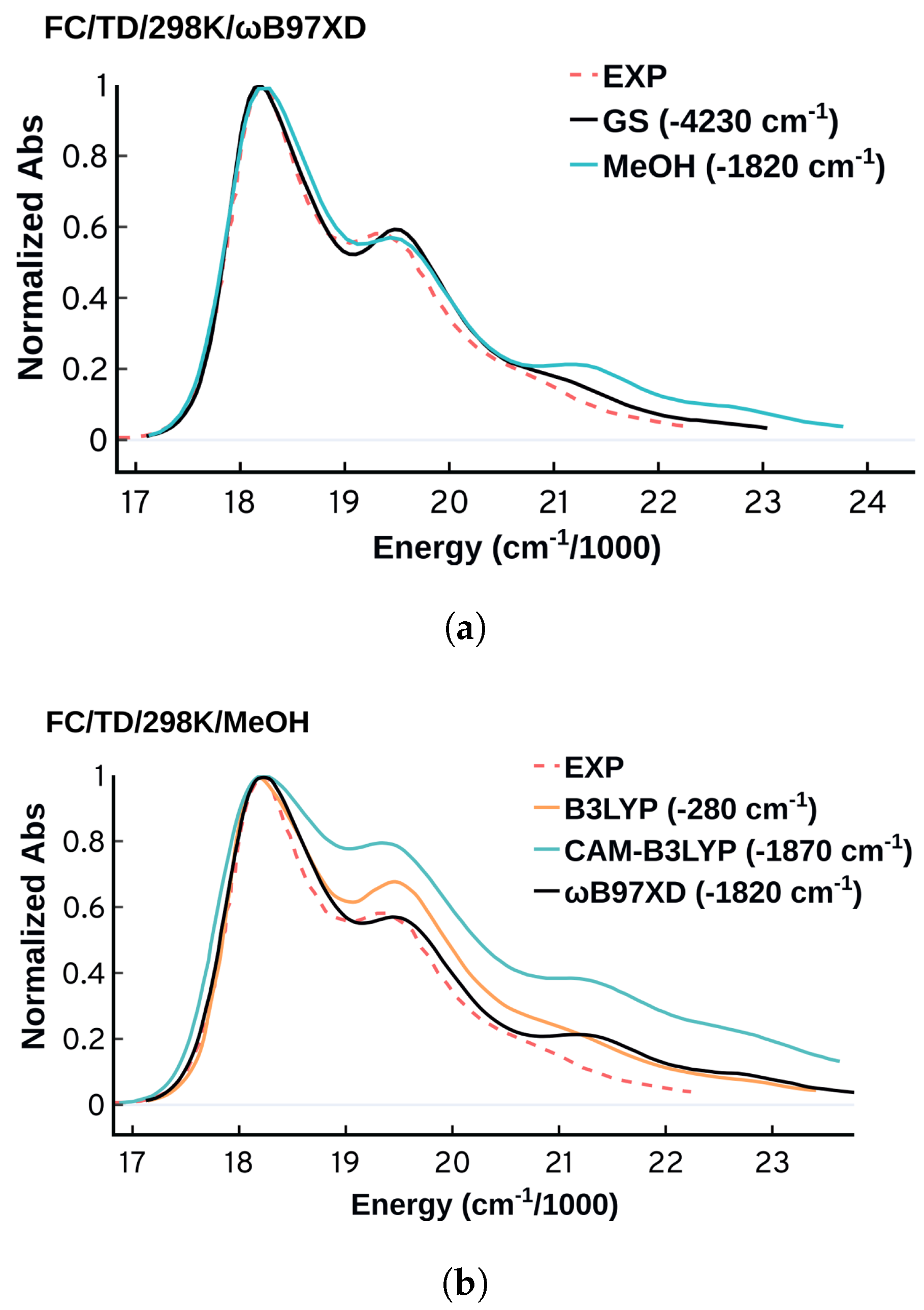
3.3.4. Environment and Functional Effects
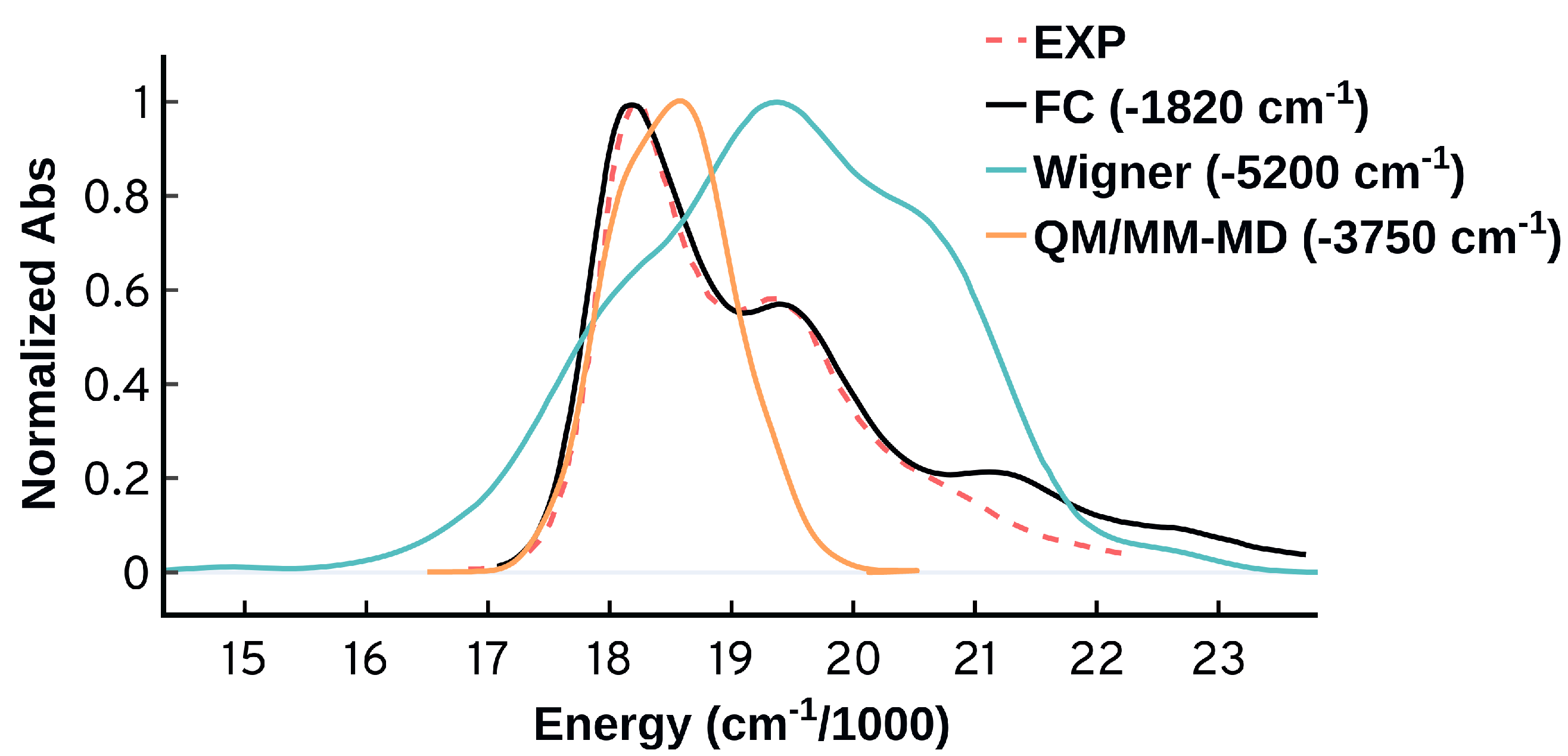
3.4. Comparing the Ensemble and Franck–Condon Approaches
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Levitus, M.; Ranjit, S. Cyanine dyes in biophysical research: The photophysics of polymethine fluorescent dyes in biomolecular environments. Q. Rev. Biophys. 2011, 44, 123. [Google Scholar] [CrossRef] [PubMed]
- Mishra, A.; Behera, R.K.; Behera, P.K.; Mishra, B.K.; Behera, G.B. Cyanines during the 1990s: A review. J. Chem. Rev. 2000, 100, 1973–2012. [Google Scholar] [CrossRef] [PubMed]
- Lee, W.; von Hippel, P.H.; Marcus, A.H. Internally labeled Cy3/Cy5 DNA constructs show greatly enhanced photo-stability in single-molecule FRET experiments. Nucleic Acids Res. 2014, 42, 5967–5977. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lavis, L.D.; Raines, R.T. Bright ideas for chemical biology. ACS Chem. Biol. 2008, 3, 142–155. [Google Scholar] [CrossRef] [Green Version]
- Heussman, D.; Kittell, J.; Kringle, L.; Tamimi, A.; von Hippel, P.H.; Marcus, A.H. Measuring Local Conformations and Conformational Disorder of (Cy3)2 Dimer Labeled DNA Fork Junctions Using Absorbance, Circular Dichroism and Two-Dimensional Fluorescence Spectroscopy. Faraday Discuss. 2019, 216, 211–235. [Google Scholar] [CrossRef]
- Kringle, L.; Sawaya, N.P.D.; Widom, J.; Adams, C.; Raymer, M.G.; Aspuru-Guzik, A.; Marcus, A.H. Temperature-Dependent Conformations of Exciton-Coupled Cy3 Dimers in Double-Stranded DNA. J. Chem. Phys. 2018, 148, 085101. [Google Scholar] [CrossRef]
- Hart, S.M.; Banal, J.L.; Bathe, M.; Schlau-Cohen, G.S. Identification of nonradiative decay pathways in Cy3. J. Phys. Chem. Lett. 2020, 11, 5000–5007. [Google Scholar] [CrossRef]
- Mustroph, H.; Reiner, K.; Mistol, J.; Ernst, S.; Keil, D.; Hennig, L. Relationship between the molecular structure of cyanine dyes and the vibrational fine structure of their electronic absorption spectra. ChemPhysChem 2009, 10, 835–840. [Google Scholar] [CrossRef]
- Mustroph, H.; Towns, A. Fine Structure in Electronic Spectra of Cyanine Dyes: Are Sub-Bands Largely Determined by a Dominant Vibration or a Collection of Singly Excited Vibrations. ChemPhysChem 2018, 19, 1016. [Google Scholar] [CrossRef] [Green Version]
- Guillaume, M.; Liégeois, V.; Champagne, B.; Zutterman, F. Time-dependent density functional theory investigation of the absorption and emission spectra of a cyanine dye. Chem. Phys. Lett. 2007, 446, 165–169. [Google Scholar] [CrossRef]
- Alia, J.D.; Flack, J.A. Unspecified verticality of Franck–Condon transitions, absorption and emission spectra of cyanine dyes, and a classically inspired approximation. RSC Adv. 2020, 10, 43153–43167. [Google Scholar] [CrossRef]
- Zakharova, G.; Avakyan, V.; Markelov, V.; Svyatoslavskii, N.; Svyatoslavskaya, T.; Chibisov, A. Effect of cucurbituril on the primary photoprocesses in indocarbocyanine dyes in water. High Energy Chem. 2015, 49, 407–414. [Google Scholar] [CrossRef]
- Heussman, D.; Kittell, J.; von Hippel, P.H.; Marcus, A.H. Temperature-dependent local conformations and conformational distributions of cyanine dimer labeled single-stranded–double-stranded DNA junctions by 2D fluorescence spectroscopy. J. Chem. Phys. 2022, 156, 045101. [Google Scholar] [CrossRef] [PubMed]
- Nicoli, F.; Roos, M.K.; Hemmig, E.A.; Di Antonio, M.; de Vivie-Riedle, R.; Liedl, T. Proximity-Induced H-Aggregation of Cyanine Dyes on DNA-Duplexes. J. Phys. Chem. A 2016, 120, 9941–9947. [Google Scholar] [CrossRef]
- Nogueira, J.J.; González, L. Computational Photophysics in the Presence of an Environment. Annu. Rev. Phys. Chem. 2018, 69, 473–497. [Google Scholar] [CrossRef]
- Zuehlsdorff, T.J.; Shedge, S.V.; Lu, S.Y.; Hong, H.; Aguirre, V.P.; Shi, L.; Isborn, C.M. Vibronic and environmental effects in simulations of optical spectroscopy. Annu. Rev. Phys. Chem. 2021, 72, 165–188. [Google Scholar] [CrossRef]
- Zuehlsdorff, T.J.; Isborn, C.M. Modeling absorption spectra of molecules in solution. Int. J. Quantum Chem. 2019, 119, e25719. [Google Scholar] [CrossRef] [Green Version]
- Zuehlsdorff, T.J.; Isborn, C.M. Combining the ensemble and Franck-Condon approaches for calculating spectral shapes of molecules in solution. J. Chem. Phys. 2018, 148, 024110. [Google Scholar] [CrossRef]
- Zuehlsdorff, T.J.; Montoya-Castillo, A.; Napoli, J.A.; Markland, T.E.; Isborn, C.M. Optical spectra in the condensed phase: Capturing anharmonic and vibronic features using dynamic and static approaches. J. Chem. Phys. 2019, 151, 074111. [Google Scholar] [CrossRef]
- Barbatti, M.; Sen, K. Effects of different initial condition samplings on photodynamics and spectrum of pyrrole. Int. J. Quantum Chem. 2016, 116, 762–771. [Google Scholar] [CrossRef] [Green Version]
- De Vetta, M.; Baig, O.; Steen, D.; Nogueira, J.J.; González, L. Assessing configurational sampling in the quantum mechanics/molecular mechanics calculation of Temoporfin absorption spectrum and triplet density of states. Molecules 2018, 23, 2932. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Marx, D.; Parrinello, M. Ab initio path integral molecular dynamics: Basic ideas. J. Chem. Phys. 1996, 104, 4077–4082. [Google Scholar] [CrossRef]
- Roche, M. On the polyatomic Franck-Condon factors. Chem. Phys. Lett 1990, 168, 556–558. [Google Scholar] [CrossRef]
- Faulkner, T.R.; Richardson, F. On the calculation of polyatomic Franck–Condon factors: Application to the 1 A 1 g → 1 B 2 u absorption band of benzene. J. Chem. Phys. 1979, 70, 1201–1213. [Google Scholar] [CrossRef]
- Toniolo, A.; Persico, M. Efficient calculation of Franck–Condon factors and vibronic couplings in polyatomics. J. Comput. Chem. 2001, 22, 968–975. [Google Scholar] [CrossRef]
- Hazra, A.; Nooijen, M. Derivation and efficient implementation of a recursion formula to calculate harmonic Franck–Condon factors for polyatomic molecules. Int. J. Quantum Chem. 2003, 95, 643–657. [Google Scholar] [CrossRef]
- Jankowiak, H.C.; Stuber, J.; Berger, R. Vibronic transitions in large molecular systems: Rigorous prescreening conditions for Franck-Condon factors. J. Chem. Phys. 2007, 127, 234101. [Google Scholar] [CrossRef]
- Li, S.L.; Truhlar, D.G. Franck–Condon models for simulating the band shape of electronic absorption spectra. J. Chem. Theory Comput. 2017, 13, 2823–2830. [Google Scholar] [CrossRef]
- Hazra, A.; Chang, H.H.; Nooijen, M. First principles simulation of the UV absorption spectrum of ethylene using the vertical Franck-Condon approach. J. Chem. Phys. 2004, 121, 2125–2136. [Google Scholar] [CrossRef]
- Law, Y.; Hassanali, A. The importance of nuclear quantum effects in spectral line broadening of optical spectra and electrostatic properties in aromatic chromophores. J. Chem. Phys. 2018, 148, 102331. [Google Scholar] [CrossRef]
- Santoro, F.; Improta, R.; Lami, A.; Bloino, J.; Barone, V. Effective method to compute Franck-Condon integrals for optical spectra of large molecules in solution. J. Chem. Phys. 2007, 126, 084509. [Google Scholar] [CrossRef] [PubMed]
- Baiardi, A.; Bloino, J.; Barone, V. General time dependent approach to vibronic spectroscopy including Franck–Condon, Herzberg–Teller, and Duschinsky effects. J. Chem. Theory Comput. 2013, 9, 4097–4115. [Google Scholar] [CrossRef] [PubMed]
- Petrenko, T.; Neese, F. Analysis and prediction of absorption band shapes, fluorescence band shapes, resonance Raman intensities, and excitation profiles using the time-dependent theory of electronic spectroscopy. J. Chem. Phys. 2007, 127, 164319. [Google Scholar] [CrossRef]
- Orlandi, G.; Siebrand, W. Theory of vibronic intensity borrowing. Comparison of Herzberg-Teller and Born-Oppenheimer coupling. J. Chem. Phys. 1973, 58, 4513–4523. [Google Scholar] [CrossRef]
- Biczysko, M.; Bloino, J.; Santoro, F.; Barone, V. Time-Independent Approaches to Simulate Electronic Spectra Lineshapes. From Small Molecules to Macrosystems in Computational Strategies for Spectroscopy: From Small Molecules to Nanosystems; Barone, V., Ed.; Wiley: Chichester, UK, 2011; pp. 361–443. [Google Scholar]
- Ferrer, F.J.A.; Santoro, F. Comparison of vertical and adiabatic harmonic approaches for the calculation of the vibrational structure of electronic spectra. Phys. Chem. Chem. Phys. 2012, 14, 13549–13563. [Google Scholar] [CrossRef] [PubMed]
- Begušić, T.; Cordova, M.; Vaníček, J. Single-hessian thawed gaussian approximation. J. Chem. Phys. 2019, 150, 154117. [Google Scholar] [CrossRef] [Green Version]
- Tang, J.; Lee, M.T.; Lin, S.H. Effects of the Duschinsky mode-mixing mechanism on temperature dependence of electron transfer processes. J. Chem. Phys. 2003, 119, 7188–7196. [Google Scholar] [CrossRef]
- Baiardi, A.; Bloino, J.; Barone, V. General formulation of vibronic spectroscopy in internal coordinates. J. Chem. Phys. 2016, 144, 084114. [Google Scholar] [CrossRef] [Green Version]
- Santoro, F.; Jacquemin, D. Going beyond the vertical approximation with time-dependent density functional theory. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2016, 6, 460–486. [Google Scholar] [CrossRef]
- Götze, J.P.; Karasulu, B.; Thiel, W. Computing UV/vis spectra from the adiabatic and vertical Franck-Condon schemes with the use of Cartesian and internal coordinates. J. Chem. Phys. 2013, 139, 234108. [Google Scholar] [CrossRef]
- Santoro, F.; Lami, A.; Improta, R.; Bloino, J.; Barone, V. Effective method for the computation of optical spectra of large molecules at finite temperature including the Duschinsky and Herzberg–Teller effect: The Q x band of porphyrin as a case study. J. Chem. Phys. 2008, 128, 224311. [Google Scholar] [CrossRef] [PubMed]
- Luis, J.M.; Torrent-Sucarrat, M.; Solà, M.; Bishop, D.M.; Kirtman, B. Calculation of Franck–Condon factors including anharmonicity: Simulation of the C2H4 + 2B3u ← C2H41Ag band in the photoelectron spectrum of ethylene. J. Chem. Phys. 2005, 122, 184104. [Google Scholar] [PubMed] [Green Version]
- Pettersen, E.F.; Goddard, T.D.; Huang, C.C.; Couch, G.S.; Greenblatt, D.M.; Meng, E.C.; Ferrin, T.E. UCSF Chimera—A visualization system for exploratory research and analysis. J. Comput. Chem. 2004, 25, 1605–1612. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Van Der Spoel, D.; Lindahl, E.; Hess, B.; Groenhof, G.; Mark, A.E.; Berendsen, H.J. GROMACS: Fast, flexible, and free. J. Comput. Chem. 2005, 26, 1701–1718. [Google Scholar] [CrossRef]
- Graen, T.; Hoefling, M.; Grubmüller, H. AMBER-DYES: Characterization of charge fluctuations and force field parameterization of fluorescent dyes for molecular dynamics simulations. J. Chem. Theory Comput. 2014, 10, 5505–5512. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Wolf, R.M.; Caldwell, J.W.; Kollman, P.A.; Case, D.A. Development and testing of a general amber force field. J. Comput. Chem. 2004, 25, 1157–1174. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian˜16 Revision C.01; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Bayly, C.I.; Cieplak, P.; Cornell, W.; Kollman, P.A. A well-behaved electrostatic potential based method using charge restraints for deriving atomic charges: The RESP model. J. Phys. Chem. 1993, 97, 10269–10280. [Google Scholar] [CrossRef]
- Wang, J.; Wang, W.; Kollman, P.A.; Case, D.A. Antechamber: An accessory software package for molecular mechanical calculations. J. Am. Chem. Soc. 2001, 222, U403. [Google Scholar]
- Lindorff-Larsen, K.; Piana, S.; Palmo, K.; Maragakis, P.; Klepeis, J.L.; Dror, R.O.; Shaw, D.E. Improved side-chain torsion potentials for the Amber ff99SB protein force field. Proteins Struct. Funct. Bioinform. 2010, 78, 1950–1958. [Google Scholar] [CrossRef] [Green Version]
- Shen, J.W.; Li, J.; Zhao, Z.; Zhang, L.; Peng, G.; Liang, L. Molecular dynamics study on the mechanism of polynucleotide encapsulation by chitosan. Sci. Rep. 2017, 7, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Jorgensen, W.L. Quantum and statistical mechanical studies of liquids. 10. Transferable intermolecular potential functions for water, alcohols, and ethers. Application to liquid water. J. Am. Chem. Soc. 1981, 103, 335–340. [Google Scholar] [CrossRef]
- Berendsen, H.J.; Postma, J.v.; van Gunsteren, W.F.; DiNola, A.; Haak, J.R. Molecular dynamics with coupling to an external bath. J. Chem. Phys. 1984, 81, 3684–3690. [Google Scholar] [CrossRef] [Green Version]
- Bussi, G.; Donadio, D.; Parrinello, M. Canonical sampling through velocity rescaling. J. Chem. Phys. 2007, 126, 014101. [Google Scholar] [CrossRef] [Green Version]
- Parrinello, M.; Rahman, A. Polymorphic transitions in single crystals: A new molecular dynamics method. J. Appl. Phys. 1981, 52, 7182–7190. [Google Scholar] [CrossRef]
- Nosé, S.; Klein, M. Constant pressure molecular dynamics for molecular systems. Mol. Phys. 1983, 50, 1055–1076. [Google Scholar] [CrossRef]
- Cuendet, M.A.; van Gunsteren, W.F. On the calculation of velocity-dependent properties in molecular dynamics simulations using the leapfrog integration algorithm. J. Chem. Phys. 2007, 127, 184102. [Google Scholar] [CrossRef] [PubMed]
- Darden, T.; York, D.; Pedersen, L. Particle mesh Ewald: An N·log (N) method for Ewald sums in large systems. J. Chem. Phys. 1993, 98, 10089–10092. [Google Scholar] [CrossRef] [Green Version]
- ChemShell, a Computational Chemistry Shell. Available online: www.chemshell.org (accessed on 23 June 2022).
- Sherwood, P.; de Vries, A.H.; Guest, M.F.; Schreckenbach, G.; Catlow, C.R.A.; French, S.A.; Sokol, A.A.; Bromley, S.T.; Thiel, W.; Turner, A.J.; et al. QUASI: A general purpose implementation of the QM/MM approach and its application to problems in catalysis. J. Mol. Struct. THEOCHEM 2003, 632, 1–28. [Google Scholar] [CrossRef]
- Pople, J.; Seeger, R.; Krishnan, R. Variational configuration interaction methods and comparison with perturbation theory. Int. J. Quantum Chem. 1977, 12, 149–163. [Google Scholar] [CrossRef]
- Oumi, M.; Maurice, D.; Lee, T.J.; Head-Gordon, M. A diagnostic for the applicability of the CIS and CIS (D) excitation energy methods. Chem. Phys. Lett. 1997, 279, 151–157. [Google Scholar] [CrossRef]
- Christiansen, O.; Koch, H.; Jørgensen, P. The second-order approximate coupled cluster singles and doubles model CC2. Chem. Phys. Lett. 1995, 243, 409–418. [Google Scholar] [CrossRef]
- Dreuw, A.; Wormit, M. The algebraic diagrammatic construction scheme for the polarization propagator for the calculation of excited states. WIREs Comput. Mol. Sci. 2015, 5, 82–95. [Google Scholar] [CrossRef]
- Abu-Awwad, F.; Politzer, P. Variation of parameters in Becke-3 hybrid exchange-correlation functional. J. Comput. Chem. 2000, 21, 227–238. [Google Scholar] [CrossRef]
- Yanai, T.; Tew, D.P.; Handy, N.C. A new hybrid exchange–correlation functional using the Coulomb-attenuating method (CAM-B3LYP). Chem. Phys. Lett. 2004, 393, 51–57. [Google Scholar] [CrossRef] [Green Version]
- Rohrdanz, M.A.; Martins, K.M.; Herbert, J.M. A long-range-corrected density functional that performs well for both ground-state properties and time-dependent density functional theory excitation energies, including charge-transfer excited states. J. Chem. Phys. 2009, 130, 054112. [Google Scholar] [CrossRef] [Green Version]
- Chai, J.D.; Head-Gordon, M. Long-range corrected hybrid density functionals with damped atom–atom dispersion corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615–6620. [Google Scholar] [CrossRef] [Green Version]
- Peverati, R.; Truhlar, D.G. M11-L: A local density functional that provides improved accuracy for electronic structure calculations in chemistry and physics. J. Phys. Chem. Lett. 2012, 3, 117–124. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. Density functional for spectroscopy: No long-range self-interaction error, good performance for Rydberg and charge-transfer states, and better performance on average than B3LYP for ground states. J. Phys. Chem. A 2006, 110, 13126–13130. [Google Scholar] [CrossRef]
- Local Version of TURBOMOLE V6.5 2013, a Development of University of Karlsruhe and Forschungszentrum Karlsruhe GmbH, 1989–2007. TURBOMOLE GmbH Since 2007. Available online: https://www.turbomole.org/ (accessed on 23 June 2022).
- Frisch, M.; Trucks, G.; Schlegel, H.; Scuseria, G.; Robb, M.; Cheeseman, J.; Scalmani, G.; Barone, V.; Petersson, G.; Nakatsuji, H.; et al. Gaussian 16 Revision a. 03. 2016; Gaussian Inc.: Wallingford, CT, USA, 2016; Volume 2. [Google Scholar]
- Barbatti, M.; Granucci, G.; Ruckenbauer, M.; Plasser, F.; Crespo-Otero, R.; Pittner, J.; Persico, M.; Lischka, H. NEWTON-X: A Package for Newtonian Dynamics Close to the Crossing Seam (v. 2.2). 2018. Available online: https://newtonx.org/ (accessed on 23 June 2022).
- Sorour, M.I.; Kistler, K.A.; Marcus, A.H.; Matsika, S. Accurate Modeling of Excitonic Coupling in Cyanine Dye Cy3. J. Phys. Chem. A 2021, 125, 7852–7866. [Google Scholar] [CrossRef]
- Frisch, M.; Trucks, G.; Schlegel, H.; Scuseria, G.; Robb, M.; Cheeseman, J.; Scalmani, G.; Barone, V.; Petersson, G.; Nakatsuji, H.; et al. Gaussian 09, Revision C.01. 2010; Gaussian Inc.: Wallingford, CT, USA, 2010. [Google Scholar]
- Lin, S.H.; Eyring, H. Study of the Franck-Condon and Herzberg-Teller Approximations. Proc. Natl. Acad. Sci. USA 1974, 71, 3802–3804. [Google Scholar] [CrossRef] [Green Version]
- Mennucci, B.; Tomasi, J. Continuum solvation models: A new approach to the problem of solute’s charge distribution and cavity boundaries. J. Chem. Phys. 1997, 106, 5151–5158. [Google Scholar] [CrossRef]
- Cossi, M.; Rega, N.; Scalmani, G.; Barone, V. Energies, structures, and electronic properties of molecules in solution with the C-PCM solvation model. J. Comput. Chem. 2003, 24, 669–681. [Google Scholar] [CrossRef] [PubMed]
- Send, R.; Valsson, O.; Filippi, C. Electronic excitations of simple cyanine dyes: Reconciling density functional and wave function methods. J. Chem. Theory Comput. 2011, 7, 444–455. [Google Scholar] [CrossRef]
- Zhekova, H.; Krykunov, M.; Autschbach, J.; Ziegler, T. Applications of time dependent and time independent density functional theory to the first π to π* transition in cyanine dyes. J. Chem. Theory Comput. 2014, 10, 3299–3307. [Google Scholar] [CrossRef] [PubMed]
- Momeni, M.R.; Brown, A. Why do TD-DFT excitation energies of BODIPY/aza-BODIPY families largely deviate from experiment? Answers from electron correlated and multireference methods. J. Chem. Theory Comput. 2015, 11, 2619–2632. [Google Scholar] [CrossRef]
- Le Guennic, B.; Jacquemin, D. Taking up the cyanine challenge with quantum tools. Acc. Chem. Res. 2015, 48, 530–537. [Google Scholar] [CrossRef]
- Head-Gordon, M.; Rico, R.J.; Oumi, M.; Lee, T.J. A doubles correction to electronic excited states from configuration interaction in the space of single substitutions. Chem. Phys. Lett 1994, 219, 21–29. [Google Scholar] [CrossRef] [Green Version]
- Sarkar, R.; Boggio-Pasqua, M.; Loos, P.F.; Jacquemin, D. Benchmarking TD-DFT and wave function methods for oscillator strengths and excited-state dipole moments. J. Chem. Theory Comput. 2021, 17, 1117–1132. [Google Scholar] [CrossRef]
- Shaw, R.A.; Johnston-Wood, T.; Ambrose, B.; Craggs, T.D.; Hill, J.G. CHARMM-DYES: Parameterization of fluorescent dyes for use with the CHARMM force field. J. Chem. Theory Comput. 2020, 16, 7817–7824. [Google Scholar] [CrossRef]
- Wang, R.; Ozhgibesov, M.; Hirao, H. Partial hessian fitting for determining force constant parameters in molecular mechanics. J. Comput. Chem. 2016, 37, 2349–2359. [Google Scholar] [CrossRef]
- Feyereisen, M.; Fitzgerald, G.; Komornicki, A. Use of approximate integrals in ab initio theory. An application in MP2 energy calculations. Chem. Phys. Lett. 1993, 208, 359–363. [Google Scholar] [CrossRef]
- Charaf-Eddin, A.; Planchat, A.; Mennucci, B.; Adamo, C.; Jacquemin, D. Choosing a functional for computing absorption and fluorescence band shapes with TD-DFT. J. Chem. Theory Comput. 2013, 9, 2749–2760. [Google Scholar] [CrossRef] [PubMed]
- Charaf-Eddin, A.; Cauchy, T.; Felpin, F.X.; Jacquemin, D. Vibronic spectra of organic electronic chromophores. RSC Adv. 2014, 4, 55466–55472. [Google Scholar] [CrossRef] [Green Version]
- Benkyi, I.; Tapavicza, E.; Fliegl, H.; Sundholm, D. Calculation of vibrationally resolved absorption spectra of acenes and pyrene. Phys. Chem. Chem. Phys. 2019, 21, 21094–21103. [Google Scholar] [CrossRef] [Green Version]
- Tapavicza, E.; Furche, F.; Sundholm, D. Importance of vibronic effects in the UV–Vis spectrum of the 7, 7, 8, 8-tetracyanoquinodimethane anion. J. Chem. Theory Comput. 2016, 12, 5058–5066. [Google Scholar] [CrossRef]
- Fothergill, J.W.; Hernandez, A.C.; Knowlton, W.B.; Yurke, B.; Li, L. Ab initio studies of exciton interactions of Cy5 dyes. J. Phys. Chem. A 2018, 122, 8989–8997. [Google Scholar] [CrossRef]
- Barone, V.; Bloino, J.; Biczysko, M. Vibrationally-resolved electronic spectra in GAUSSIAN 09. Revision A 2009, 2, 1–20. [Google Scholar]
- Fortino, M.; Bloino, J.; Collini, E.; Bolzonello, L.; Trapani, M.; Faglioni, F.; Pedone, A. On the simulation of vibrationally resolved electronic spectra of medium-size molecules: The case of styryl substituted BODIPYs. Phys. Chem. Chem. Phys. 2019, 21, 3512–3526. [Google Scholar] [CrossRef]
- Schepers, B.; Gohlke, H. AMBER-DYES in AMBER: Implementation of fluorophore and linker parameters into AmberTools. J. Chem. Phys. 2020, 152, 221103. [Google Scholar] [CrossRef]
- Abou-Hatab, S.; Carnevale, V.; Matsika, S. Modeling solvation effects on absorption and fluorescence spectra of indole in aqueous solution. J. Chem. Phys. 2021, 154, 064104. [Google Scholar] [CrossRef]
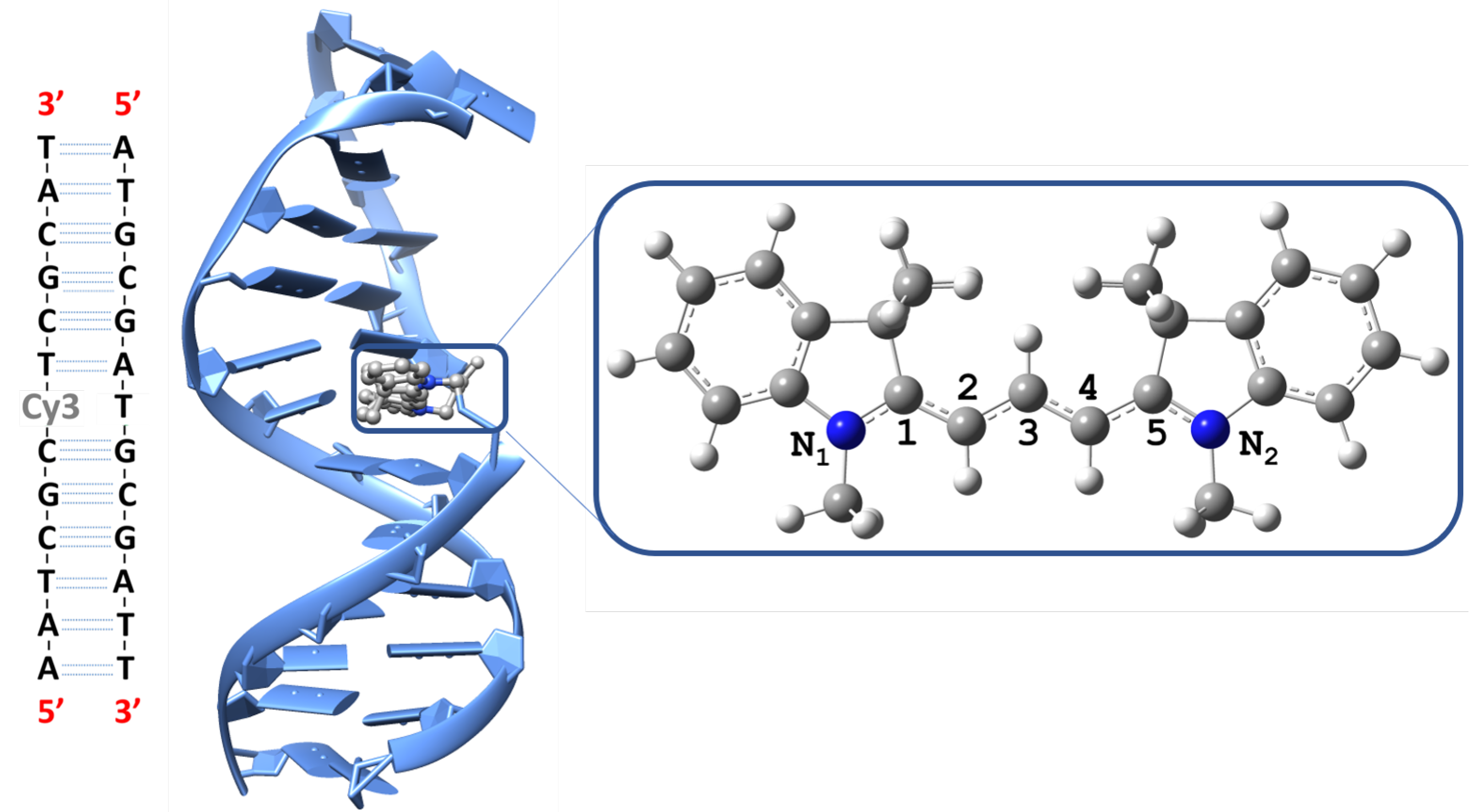



| /B97XD() | /B97XD() | /FF [46] | k/FF [46] | |
|---|---|---|---|---|
| R(N,1) | 1.3435 | 1.3712 | 1.391 | 343760 |
| R(1,2) | 1.3966 | 1.3960 | 1.4510 | 326770 |
| R(2,3) | 1.3938 | 1.4071 | 1.3379 | 470620 |
| R(3,4) | 1.3938 | 1.4071 | 1.4511 | 326770 |
| R(4,5) | 1.3966 | 1.3960 | 1.3390 | 469030 |
| R(5,N) | 1.3435 | 1.3712 | 1.355 | 387100 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sorour, M.I.; Marcus, A.H.; Matsika, S. Modeling the Electronic Absorption Spectra of the Indocarbocyanine Cy3. Molecules 2022, 27, 4062. https://doi.org/10.3390/molecules27134062
Sorour MI, Marcus AH, Matsika S. Modeling the Electronic Absorption Spectra of the Indocarbocyanine Cy3. Molecules. 2022; 27(13):4062. https://doi.org/10.3390/molecules27134062
Chicago/Turabian StyleSorour, Mohammed I., Andrew H. Marcus, and Spiridoula Matsika. 2022. "Modeling the Electronic Absorption Spectra of the Indocarbocyanine Cy3" Molecules 27, no. 13: 4062. https://doi.org/10.3390/molecules27134062
APA StyleSorour, M. I., Marcus, A. H., & Matsika, S. (2022). Modeling the Electronic Absorption Spectra of the Indocarbocyanine Cy3. Molecules, 27(13), 4062. https://doi.org/10.3390/molecules27134062







