Limitations of Linear Dichroism Spectroscopy for Elucidating Structural Issues of Light-Harvesting Aggregates in Chlorosomes
Abstract
1. Introduction
2. Theoretical Background: Linear Dichroism for Tubular Aggregates
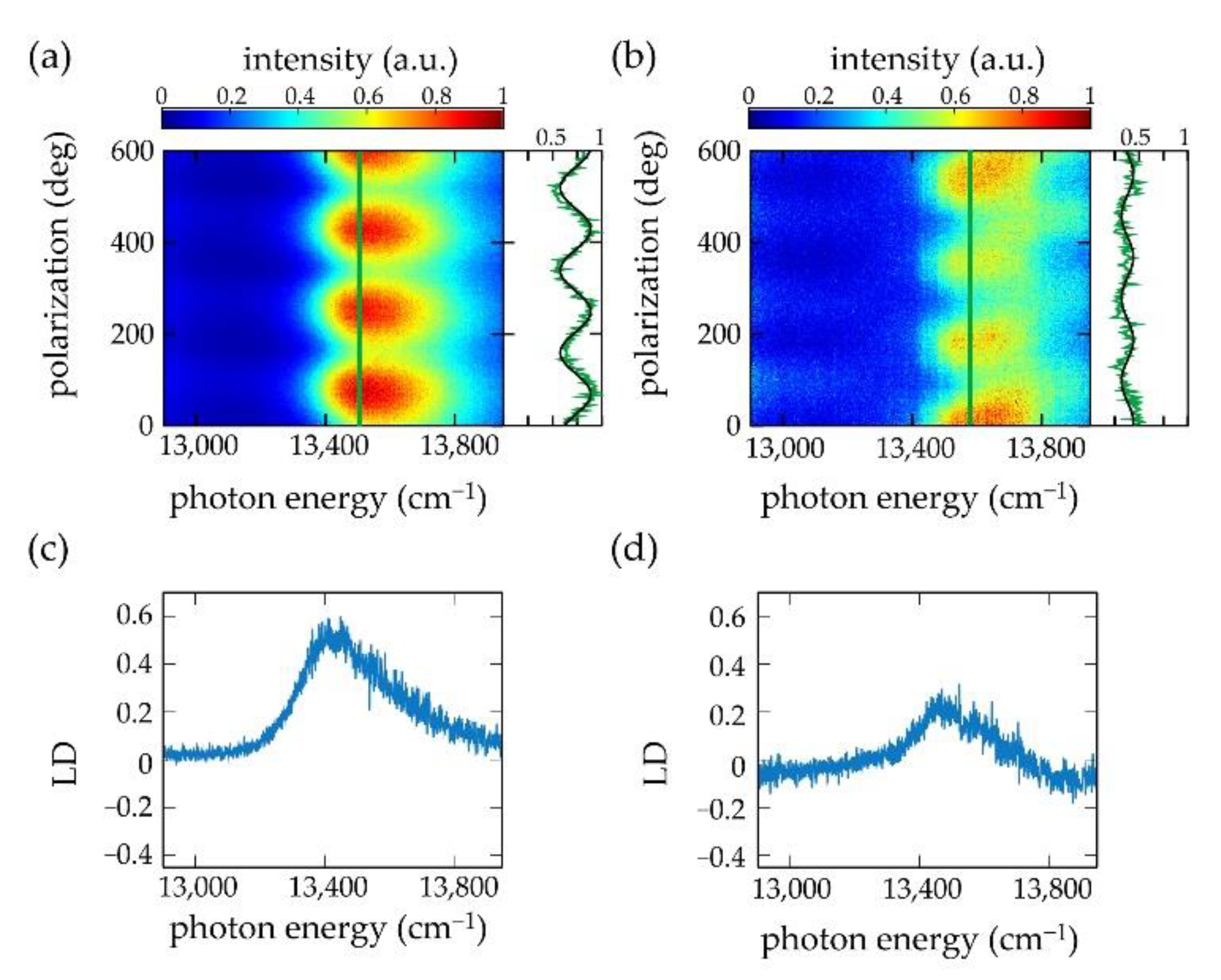
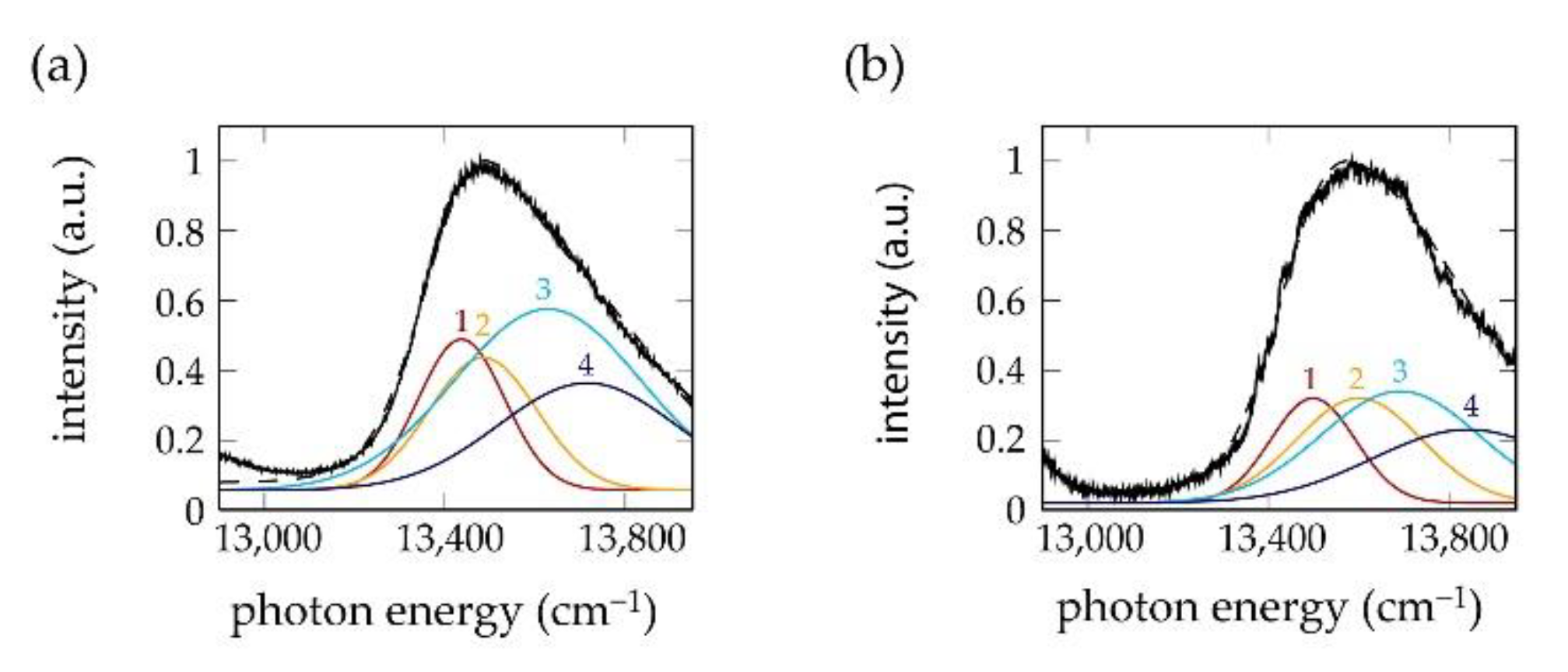
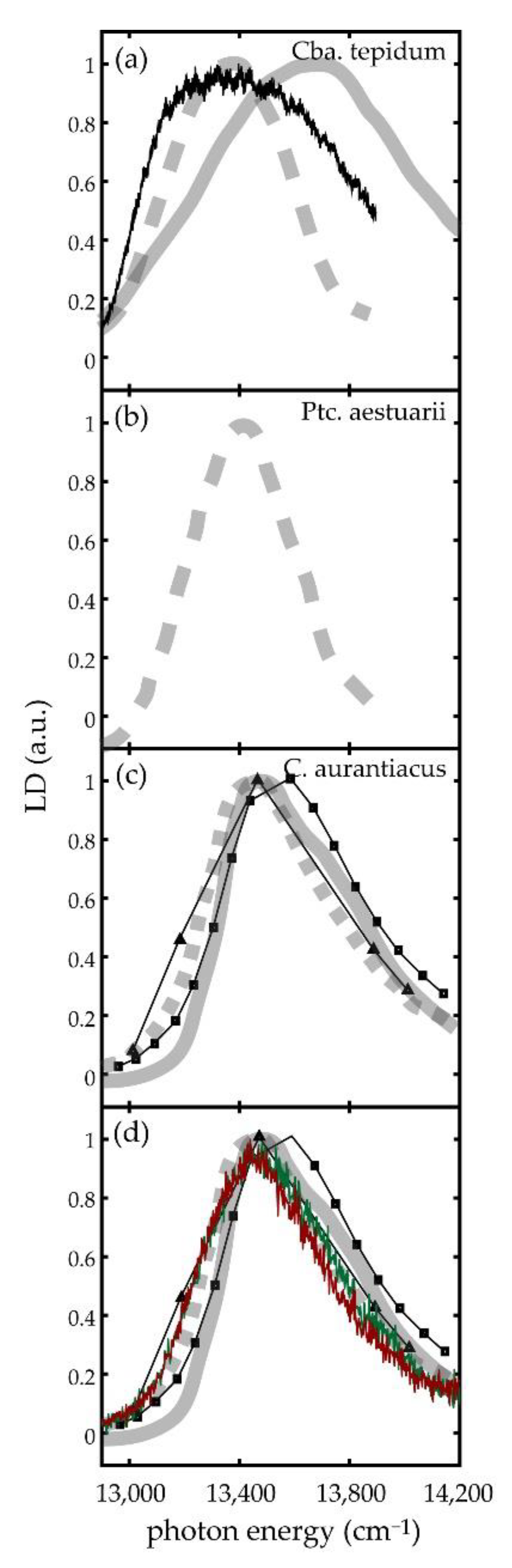
3. Results: Linear Dichroism of Chlorosomes
4. Discussion
5. Materials and Methods
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Saer, R.G.; Blankenship, R.E. Light harvesting in phototrophic bacteria: Structure and function. Biochem. J. 2017, 474, 2107–2131. [Google Scholar] [CrossRef]
- Bryant, D.A.; Canniffe, D.P. How nature designs light-harvesting antenna systems: Design principles and functional realization in chlorophototrophic prokaryotes. J. Phys. B At. Mol. Opt. 2018, 51, 33001. [Google Scholar] [CrossRef]
- Beatty, J.T.; Overmann, J.; Lince, M.T.; Manske, A.K.; Lang, A.S.; Blankenship, R.E.; van Dover, C.L.; Martinson, T.A.; Plumley, F.G. An obligately photosynthetic bacterial anaerobe from a deep-sea hydrothermal vent. Proc. Natl. Acad. Sci. USA 2005, 102, 9306–9310. [Google Scholar] [CrossRef] [PubMed]
- Oostergetel, G.T.; van Amerongen, H.; Boekema, E.J. The chlorosome: A prototype for efficient light harvesting in photosynthesis. Photosynth. Res. 2010, 104, 245–255. [Google Scholar] [CrossRef]
- Overmann, J.; Cypionka, H.; Pfennig, N. An extremely low-light adapted phototrophic sulfur bacterium from the Black Sea. Limnol. Oceangr. 1992, 37, 150–155. [Google Scholar] [CrossRef]
- Alster, J.; Kabeláč, M.; Tuma, R.; Pšenčík, J.; Burda, J.V. Computational study of short-range interactions in bacteriochlorophyll aggregates. Comput. Theor. Chem. 2012, 998, 87–97. [Google Scholar] [CrossRef]
- Oostergetel, G.T.; Reus, M.; Gomez Maqueo Chew, A.; Bryant, D.A.; Boekema, E.J.; Holzwarth, A.R. Long-range organization of bacteriochlorophyll in chlorosomes of Chlorobium tepidum investigated by cryo-electron microscopy. FEBS Lett. 2007, 581, 5435–5439. [Google Scholar] [CrossRef]
- Chew, A.G.M.; Bryant, D.A. Chlorophyll biosynthesis in bacteria: The origins of structural and functional diversity. Annu. Rev. Microbiol. 2007, 61, 113–129. [Google Scholar] [CrossRef]
- Liu, Z.; Bryant, D.A. Bacteriochlorophyll biosynthesis and assembly in green chlorophototrophic bacteria: Theme and variations. In Handbook of Porphyrin Science: With Applications to Chemistry, Physics, Materials Science, Engineering, Biology and Medicine; Kadish, K.M., Smith, K.M., Guilard, R., Eds.; World Scientific Publishing: Singapore, 2012; pp. 107–140. [Google Scholar]
- Blankenship, R.E.; Olson, J.M.; Miller, M. Antenna complexes from green photosynthetic bacteria. In Anoxygenic Photosynthetic Bacteria; Blankenship, R.E., Madigan, M.T., Bauer, C.E., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1995; pp. 399–435. ISBN 978-0-306-47954-0. [Google Scholar]
- Tamiaki, H.; Tateishi, S.; Nakabayashi, S.; Shibata, Y.; Itoh, S. Linearly polarized light absorption spectra of chlorosomes, light-harvesting antennas of photosynthetic green sulfur bacteria. Chem. Phys. Lett. 2010, 484, 333–337. [Google Scholar] [CrossRef]
- Furumaki, S.; Vacha, F.; Habuchi, S.; Tsukatani, Y.; Bryant, D.A.; Vacha, M. Absorption linear dichroism measured directly on a single light-harvesting system: The role of disorder in chlorosomes of green photosynthetic bacteria. J. Am. Chem. Soc. 2011, 133, 6703–6710. [Google Scholar] [CrossRef]
- Ganapathy, S.; Oostergetel, G.T.; Reus, M.; Tsukatani, Y.; Gomez Maqueo Chew, A.; Buda, F.; Bryant, D.A.; Holzwarth, A.R.; de Groot, H.J.M. Structural variability in wild-type and bchQ bchR mutant chlorosomes of the green sulfur bacterium chlorobaculum tepidum. Biochemistry-US 2012, 51, 4488–4498. [Google Scholar] [CrossRef]
- Ganapathy, S.; Oostergetel, G.T.; Wawrzyniak, P.K.; Reus, M.; Gomez Maqueo Chew, A.; Buda, F.; Boekema, E.J.; Bryant, D.A.; Holzwarth, A.R.; de Groot, H.J.M. Alternating syn-anti bacteriochlorophylls form concentric helical nanotubes in chlorosomes. Proc. Natl. Acad. Sci. USA 2009, 106, 8525–8530. [Google Scholar] [CrossRef]
- Pšenčík, J.; Butcher, S.J.; Tuma, R. Chlorosomes: Structure, Function and Assembly. Struct. Basis Biol. Energy Gener. 2014, 39, 77–109. [Google Scholar] [CrossRef]
- Fetisova, Z.G.; Kharchenko, S.G.; Abdourakhmanov, I.A. Strong orientational ordering of the near-infrared transition moment vectors of light-harvesting antenna bacterioviridin in chromatophores of the green photosynthetic bacterium Chlorobium limicola. FEBS Lett. 1986, 199, 234–236. [Google Scholar] [CrossRef]
- Van Dorssen, R.J.; Vasmel, H.; Amesz, J. Pigment organization and energy transfer in the green photosynthetic bacterium Chloroflexus aurantiacus: II. The chlorosome. Photosynth. Res. 1986, 9, 33–45. [Google Scholar] [CrossRef] [PubMed]
- Van Amerongen, H.; Vasmel, H.; van Grondelle, R. Linear Dichroism of Chlorosomes from Chloroflexus Aurantiacus in Compressed Gels and Electric Fields. Biophys. J. 1988, 54, 65–76. [Google Scholar] [CrossRef]
- Griebenow, K.; Holzwarth, A.R.; van Mourik, F.; van Grondelle, R. Pigment organization and energy transfer in green bacteria. 2. Circular and linear dichroism spectra of protein-containing and protein-free chlorosomes isolated from Chloroflexus aurantiacus strain Ok-70-fl. Biochim. Biophy. Acta Bioenerg. 1991, 1058, 194–202. [Google Scholar] [CrossRef]
- Frese, R.; Oberheide, U.; van Stokkum, I.; van Grondelle, R.; Foidl, M.; Oelze, J.; van Amerongen, H. The organization of bayteriochlorophyll c in clorosomes from Chloroflexus aurantiacus and the structural role of carotenoids and protein. Photosynthesis Res. 1997, 54, 115–126. [Google Scholar] [CrossRef]
- Matsuura, K.; Hirota, M.; Shimada, K.; Mimuro, M. Spectral forms and orientation of bacteriochlorophylls c and α in chlorosomes of the green photosynthetic bacterium Chloroflexus aurantiacus. Photochem. Photobiol. 1993, 57, 92–97. [Google Scholar] [CrossRef]
- Betti, J.A.; Blankenship, R.E.; Natarajan, L.V.; Dickinson, L.C.; Fuller, R.C. Antenna organization and evidence for the function of a new antenna pigment species in the green photosynthetic bacterium Chloroflexus aurantiacus. Biochim. Biophy. Acta Bioenerg. 1982, 680, 194–201. [Google Scholar] [CrossRef]
- Tian, Y.; Camacho, R.; Thomsson, D.; Reus, M.; Holzwarth, A.R.; Scheblykin, I.G. Organization of Bacteriochlorophylls in Individual Chlorosomes from Chlorobaculum tepidum Studied by 2-Dimensional Polarization Fluorescence Microscopy. J. Am. Chem. Soc. 2011, 133, 17192–17199. [Google Scholar] [CrossRef]
- Furumaki, S.; Yabiku, Y.; Habuchi, S.; Tsukatani, Y.; Bryant, D.A.; Vacha, M. Circular Dichroism Measured on Single Chlorosomal Light-Harvesting Complexes of Green Photosynthetic Bacteria. J. Phys. Chem. Lett. 2012, 3, 3545–3549. [Google Scholar] [CrossRef] [PubMed]
- Saga, Y.; Wazawa, T.; Mizoguchi, T.; Ishii, Y.; Yanagida, T.; Tamiaki, H. Spectral heterogeneity in single light-harvesting chlorosomes from green sulfur photosynthetic bacterium Chlorobium tepidum. Photochem. Photobiol. 2002, 75, 433. [Google Scholar] [CrossRef]
- Saga, Y.; Tamiaki, H.; Shibata, Y.; Itoh, S. Excitation energy transfer in individual light-harvesting chlorosome from green photosynthetic bacterium Chloroflexus aurantiacus at cryogenic temperature. Chem. Phys. Lett. 2005, 409, 34–37. [Google Scholar] [CrossRef]
- Shibata, Y.; Saga, Y.; Tamiaki, H.; Itoh, S. Low-temperature fluorescence from single chlorosomes, photosynthetic antenna complexes of green filamentous and sulfur bacteria. Biophys. J. 2006, 91, 3787–3796. [Google Scholar] [CrossRef] [PubMed]
- Jendrny, M.; Aartsma, T.J.; Köhler, J. Fluorescence excitation spectra from individual chlorosomes of the green sulfur bacterium Chlorobaculum tepidum. J. Phys. Chem. Lett. 2012, 3, 3745–3750. [Google Scholar] [CrossRef]
- Jendrny, M.; Aartsma, T.J.; Köhler, J. Insights into the excitonic states of individual chlorosomes from Chlorobaculum tepidum. Biophys. J. 2014, 106, 1921–1927. [Google Scholar] [CrossRef]
- Ikonen, T.P.; Li, H.; Pšenčík, J.; Laurinmaki, P.A.; Butcher, S.J.; Frigaard, N.-U.; Serimaa, R.E.; Bryant, D.A.; Tuma, R. X-ray scattering and electron cryomicroscopy study on the effect of carotenoid biosynthesis to the structure of Chlorobium tepidum chlorosomes. Biophys. J. 2007, 93, 620–628. [Google Scholar] [CrossRef][Green Version]
- Pšenčík, J.; Ikonen, T.P.; Laurinmaki, P.; Merckel, M.C.; Butcher, S.J.; Serimaa, R.E.; Tuma, R. Lamellar organization of pigments in chlorosomes, the light harvesting complexes of green photosynthetic bacteria. Biophys. J. 2004, 87, 1165–1172. [Google Scholar] [CrossRef]
- Li, X.; Buda, F.; de Groot, H.J.M.; Sevink, G.J.A. Contrasting Modes of self-assembly and hydrogen-bonding heterogeneity in chlorosomes of Chlorobaculum tepidum. J. Phys. Chem. C Nanomater. Interfaces 2018, 122, 14877–14888. [Google Scholar] [CrossRef]
- Li, X.; Buda, F.; de Groot, H.J.M.; Sevink, G.J.A. Dynamic disorder drives exciton transfer in tubular chlorosomal assemblies. J. Phys. Chem. B 2020, 125, 4026–4035. [Google Scholar] [CrossRef]
- Linnanto, J.M.; Korppi-Tommola, J.E.I. Investigation on chlorosomal antenna geometries: Tube, lamella and spiral-type self-aggregates. Photosynth. Res. 2008, 96, 227–245. [Google Scholar] [CrossRef] [PubMed]
- Kasha, M.; Rawls, H.R.; El-Bayoumi, A.M. The exciton model in molecular spectroscopy. Pure Appl. Chem. 1965, 11. [Google Scholar] [CrossRef]
- Hestand, N.J.; Tempelaar, R.; Knoester, J.; Jansen, T.L.C.; Spano, F.C. Exciton mobility control through sub−Å packing modifications in molecular crystals. Phys. Rev. B 2015, 91. [Google Scholar] [CrossRef]
- Hestand, N.J.; Spano, F.C. Molecular Aggregate photophysics beyond the Kasha Model: Novel design principles for organic materials. Acc. Chem. Res. 2017, 50, 341–350. [Google Scholar] [CrossRef] [PubMed]
- Brixner, T.; Hildner, R.; Köhler, J.; Lambert, C.; Würthner, F. Exciton transport in molecular aggregates—From natural antennas to synthetic chromophore systems. Adv. Energy Mater. 2017, 7, 1700236. [Google Scholar] [CrossRef]
- Davydov, A.S. Theory of Molecular Excitons; Plenum Press: New York, NY, USA, 1971. [Google Scholar]
- Knox, R.S. Theory of Excitons. In Theory of Excitons; Ehrenreich, H., Seitz, F., Turnbull, D., Eds.; Academic Press: Cambridge, MA, USA, 1963. [Google Scholar]
- Garab, G. Linear and Circular Dichroism. In Biophysical Techniques in Photosynthesis. Advances in Photosynthesis and Respiration; Amesz, J., Hoff, A.J., Eds.; Springer: Dordrecht, The Netherland, 1996; pp. 11–40. [Google Scholar] [CrossRef]
- Günther, L.M.; Jendrny, M.; Bloemsma, E.A.; Tank, M.; Oostergetel, G.T.; Bryant, D.A.; Knoester, J.; Köhler, J. Structure of light-harvesting aggregates in individual chlorosomes. J. Phys. Chem. B 2016, 120, 5367–5376. [Google Scholar] [CrossRef]
- Günther, L.M.; Löhner, A.; Reiher, C.; Kunsel, T.; Jansen, T.L.C.; Tank, M.; Bryant, D.A.; Knoester, J.; Köhler, J. Structural variations in chlorosomes from wild-type and a bchQR mutant of Chlorobaculum tepidum revealed by single-molecule spectroscopy. J. Phys. Chem. B 2018, 122, 6712–6723. [Google Scholar] [CrossRef]
- Li, X.; Buda, F.; de Groot, H.J.M.; Sevink, G.J.A. Molecular insight in the optical response of tubular chlorosomal assemblies. J. Phys. Chem. C 2019, 124, 16462–16478. [Google Scholar] [CrossRef]
- Balaban, S.; Tamiaki, H.; Holzwarth, A.R. Chlorins programmed for self-assembly. In Supermolecular Dye Chemistry; Würthner, F., Ed.; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–38. ISBN 978-3-540-27758-3. [Google Scholar]
- Sengupta, S.; Ebeling, D.; Patwardhan, S.; Zhang, X.; von Berlepsch, H.; Bottcher, C.; Stepanenko, V.; Uemura, S.; Hentschel, C.; Fuchs, H.; et al. Biosupramolecular nanowires from chlorophyll dyes with exceptional charge-transport properties. Angew. Chem. Int. Edit. 2012, 51, 6378–6382. [Google Scholar] [CrossRef]
- Eisele, D.M.; Arias, D.H.; Fu, X.; Bloemsma, E.A.; Steiner, C.P.; Jensen, R.A.; Rebentrost, P.; Eisele, H.; Tokmakoff, A.; Lloyd, S.; et al. Robust excitons inhabit soft supramolecular nanotubes. Proc. Natl. Acad. Sci. USA 2014, 111, E3367–E3375. [Google Scholar] [CrossRef]
- Löhner, A.; Kunsel, T.; Röhr, M.I.S.; Jansen, T.L.C.; Sengupta, S.; Würthner, F.; Knoester, J.; Köhler, J. Spectral and structural variations of biomimetic light-harvesting nanotubes. J. Phys. Chem. Lett. 2019, 10, 2715–2724. [Google Scholar] [CrossRef] [PubMed]
- Didraga, C.; Pugžlys, A.; Hania, P.R.; von Berlepsch, H.; Duppen, K.; Knoester, J. Structure, spectroscopy, and microscopic model of tubular carbocyanine dye aggregates. J. Phys. Chem. B 2004, 108, 14976–14985. [Google Scholar] [CrossRef]
- Eisele, D.M.; Cone, C.W.; Bloemsma, E.A.; Vlaming, S.M.; van der Kwaak, C.G.F.; Silbey, R.J.; Bawendi, M.G.; Knoester, J.; Rabe, J.P.; Vanden Bout, D.A. Utilizing redox-chemistry to elucidate the nature of exciton transitions in supramolecular dye nanotubes. Nat. Chem. 2012, 4, 655–662. [Google Scholar] [CrossRef] [PubMed]
- Stradomska, A.; Knoester, J. Shape of the Q band in the absorption spectra of porphyrin nanotubes: Vibronic coupling or exciton effects? J. Chem. Phys. 2010, 133, 94701. [Google Scholar] [CrossRef]
- Spitz, C.; Knoester, J.; Ouart, A.; Daehne, S. Polarized absorption and anomalous temperature dependence of fluorescence depolarization in cylindrical J-aggregates. Chem. Phys. 2002, 275, 271–284. [Google Scholar] [CrossRef]
- Didraga, C.; Knoester, J. Excitons in tubular molecular aggregates. J. Lumin. 2004, 110, 239–245. [Google Scholar] [CrossRef]
- Didraga, C.; Klugkist, J.A.; Knoester, J. Optical properties of helical cylindrical molecular aggregates: The homogeneous limit. J. Phys. Chem. B 2002, 106, 11474–11486. [Google Scholar] [CrossRef]
- Frigaard, N.-U.; Chew, A.G.M.; Li, H.; Maresca, J.A.; Bryant, D.A. Chlorobium tepidum: Insights into the structure, physiology, and metabolism of a green sulfur bacterium derived from the complete genome sequence. Photosynth. Res. 2003, 78, 93–117. [Google Scholar] [CrossRef]
- Vlaming, S.M.; Bloemsma, E.A.; Nietiadi, M.L.; Knoester, J. Disorder-induced exciton localization and violation of optical selection rules in supramolecular nanotubes. J. Chem. Phys. 2011, 134, 114507. [Google Scholar] [CrossRef]
- de Ruijter, W.P.F.; Magis, J.G.; Miller, M.; Aartsma, T.J. Photodynamics of Light-Harvesting Systems—Structural Variations in Chlorosomes Revealed by Single Particle Spectroscopy and AFM; Universiteit Leiden: Leiden, The Netherlands, 2005. [Google Scholar]
- Chew, A.G.M.; Frigaard, N.-U.; Bryant, D.A. Bacteriochlorophyllide c C-82 and C-121 methyltransferases are essential for adaptation to low light in Chlorobaculum tepidum. J. Bacteriol. 2007, 189, 6176–6184. [Google Scholar] [CrossRef]
- Maresca, J.A.; Chew, A.G.M.; Ros Ponsati, M.; Frigaard, N.-U.; Ormerod, J.G.; Bryant, D.A. The bchU gene of Chlorobium tepidum encodes the C-20 methyltransferase in bacteriochlorophyll c biosynthesis. J. Bacteriol. 2004, 186, 2558–2566. [Google Scholar] [CrossRef]
- Abetz, V. Isoporous block copolymer membranes. Macromol. Rapid Commun. 2015, 36, 10–22. [Google Scholar] [CrossRef] [PubMed]
- Vassilieva, E.V.; Frigaard, N.-U.; Bryant, D.A. Chlorosomes: The light-harvesting complexes of the green bacteria. Spectrum (Cent. Photochem. Sci.) 2000, 13, 7–13. [Google Scholar]



| A | B | C | D | |
|---|---|---|---|---|
| Good Agreement | Good Agreement (But Sign Flip) | Reasonable Agreement | No Agreement | |
| WT-group1 | 42% (22) | 32% (17) | 11% (6) | 15% (8) |
| 85% (45) | ||||
| bchR | 62% (41) | 27% (18) | 6% (4) | 5% (3) |
| 95% (63) | ||||
| bchQR | 35% (10) | 41% (12) | 10% (3) | 14% (4) |
| 86% (25) |
| Type 1: No Node | Type 2: One Node | Type 3: Two Nodes | Relative Abundance T1:T2:T3 | ||||
|---|---|---|---|---|---|---|---|
| LD Type | + | − | + − | − + | + − + | − + − | |
| WT | |||||||
| 68 | 4 | 0 | 94%: 6%: 0% | ||||
| method 1 | 67 | 1 | 1 | 3 | 0 | 0 | |
| WT-group1 Sign flips 32% (17) | |||||||
| 49 | 4 | 0 | 92%:8%:0% | ||||
| method 1 | 48 | 1 | 1 | 3 | 0 | 0 | |
| method 2 | 27 | 15 | 7 | 3 | 1 | 0 | |
| 42 | 10 | 1 | 79%: 19%:2% | ||||
| WT-group2 | |||||||
| 19 | 0 | 0 | 100%:0%:0% | ||||
| method 1 | 19 | 0 | 0 | 0 | 0 | 0 | |
| bchR mutant sign flips 27% (18) | |||||||
| 52 | 14 | 0 | 79%:21%:0% | ||||
| method 1 | 52 | 0 | 4 | 10 | 0 | 0 | |
| method 2 | 40 | 11 | 12 | 3 | 0 | 0 | |
| 51 | 15 | 0 | 77%:23%:0% | ||||
| bchQR mutant sign flips 41% (12) | |||||||
| 21 | 6 | 2 | 72%:21%:7% | ||||
| method 1 | 21 | 0 | 2 | 4 | 2 | 0 | |
| method 2 | 10 | 7 | 5 | 4 | 2 | 1 | |
| 17 | 9 | 3 | 59%:31%:10% | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Günther, L.M.; Knoester, J.; Köhler, J. Limitations of Linear Dichroism Spectroscopy for Elucidating Structural Issues of Light-Harvesting Aggregates in Chlorosomes. Molecules 2021, 26, 899. https://doi.org/10.3390/molecules26040899
Günther LM, Knoester J, Köhler J. Limitations of Linear Dichroism Spectroscopy for Elucidating Structural Issues of Light-Harvesting Aggregates in Chlorosomes. Molecules. 2021; 26(4):899. https://doi.org/10.3390/molecules26040899
Chicago/Turabian StyleGünther, Lisa M., Jasper Knoester, and Jürgen Köhler. 2021. "Limitations of Linear Dichroism Spectroscopy for Elucidating Structural Issues of Light-Harvesting Aggregates in Chlorosomes" Molecules 26, no. 4: 899. https://doi.org/10.3390/molecules26040899
APA StyleGünther, L. M., Knoester, J., & Köhler, J. (2021). Limitations of Linear Dichroism Spectroscopy for Elucidating Structural Issues of Light-Harvesting Aggregates in Chlorosomes. Molecules, 26(4), 899. https://doi.org/10.3390/molecules26040899






