Lorentz Violation by the Preferred Frame Effects and Cosmic and Gamma Ray Propagation
Abstract
:1. Introduction
2. Special Relativity
2.1. Kinematics
2.2. Free Particle Dynamics
3. Electromagnetic Field Dynamics
4. Electromagnetic Waves
5. Gamma-Ray Propagation
5.1. Attenuation due to the Pair-Production Process
5.2. Attenuation due to Other Processes
5.2.1. Attenuation Processes
5.2.2. The Preferred Frame Effects
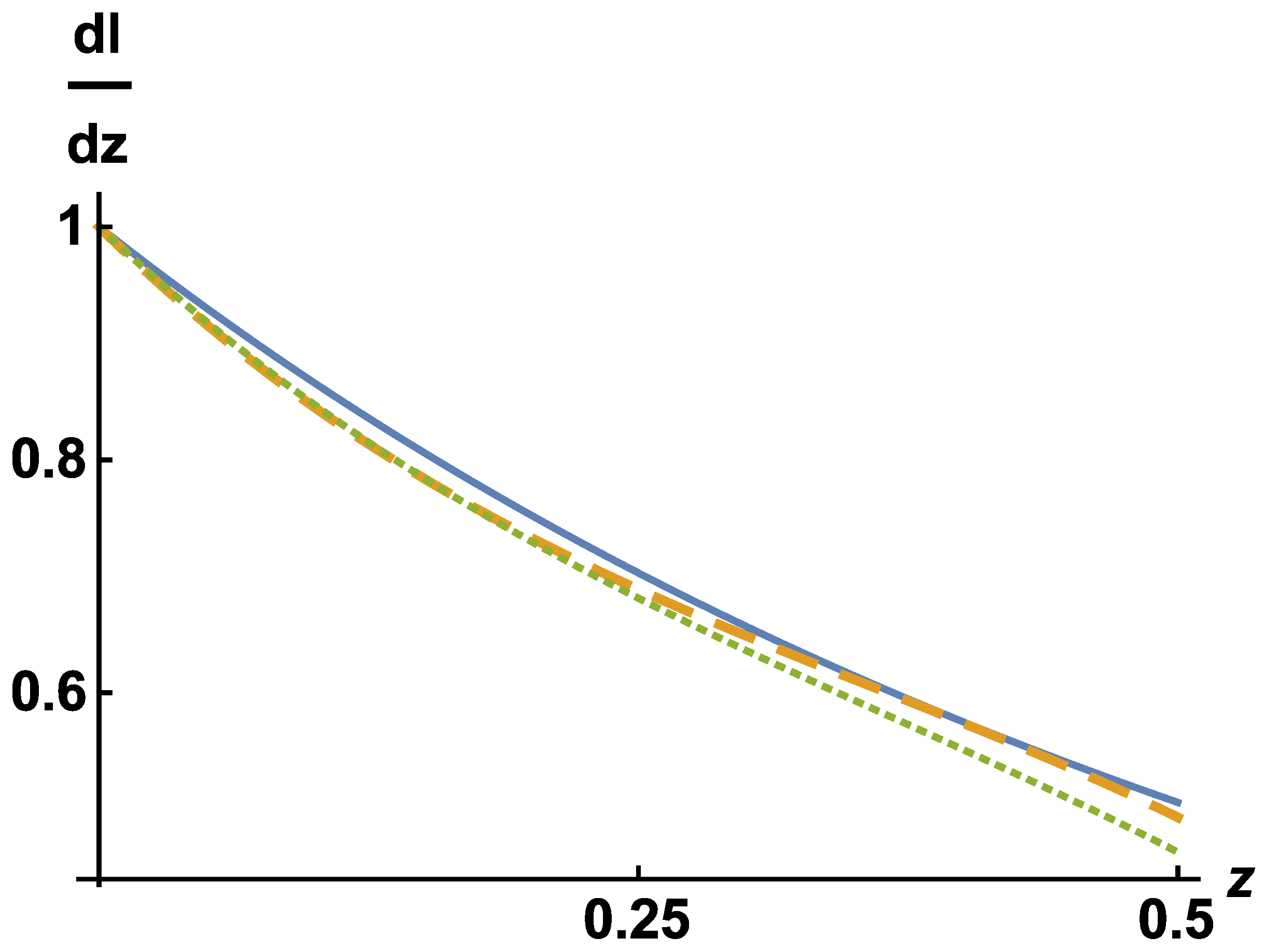
5.3. Astrophysical Tests for Vacuum Dispersion and Vacuum Birefringence
6. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | For a discussion of the one-way vs. two-way speed of light issue and the related issues of conventionality of simultaneity and clock synchronization, see, e.g., [30,31,32,33,34,35,36,37]; a discussion of those issues in the context of the ‘relativity with a preferred frame’ can be found in [27,38]. Here, it is only worth noting that the transformations derived in the present framework differ conceptually from other transformations incorporating the anisotropy of the one-way speed of light that are repeatedly derived in the literature (e.g., [30,31,32,33]). In the latter, the anisotropy is a feature that emerges due to changing the synchronization procedure, which is equivalent to a change of coordinates, while, in the present framework, the anisotropy is governed entirely by a physical law. |
| 2 | |
| 3 | It should be noted in this connection that, in the cosmological context, the rest frame is defined by the large-scale structure; in particular, when the terms ’rest frame’ or ’CMB frame’ are used in cosmological applications, the frame more or less coinciding with our galaxy is meant. Thus, using the terms ’rest frame’ or ’CMB frame’ in that context inevitably implies the space (and time) averaging over the scales on which the assumptions of homogeneity and isotropy accepted in the cosmological models are valid. The same is implied for the theories treating propagation of astroparticles on cosmological scales, moreover that those theories should include effects of the cosmological expansion which are calculated on the basis of homogeneous and isotropic cosmological models [47]. Therefore, the experiments on the solar system scale intended to detect phenomena related to motion of the Earth, as, for example, asymmetry of lifetimes of particles parallel and antiparallel to the direction of motion of the Earth with respect to the CMB frame [44], are irrelevant to the frameworks (such as the present one) designed to describe phenomena on cosmological scales. |
| 4 |
References
- Kostelecky, V.A.; Samuel, S. Spontaneous breaking of Lorentz symmetry in string theory. Phys. Rev. D 1989, 39, 683. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kostelecky, V.A.; Samuel, S. Gravitational phenomenology in higher–dimensional theories and strings. Phys. Rev. D 1989, 40, 1886. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kostelecky, V.A. (Ed.) Proceedings of the Second Meeting on CPT and Lorentz Symmetry, Bloomington, IN, USA, 15–18 August 2001; World Scientific: Singapore, 2002. [Google Scholar]
- Oriti, D. Approaches to Quantum Gravity: Toward a New Understanding of Space, Time and Matter; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Colladay, D.; Kostelecky, V.A. CPT violation and the standard model. Phys. Rev. D 1997, 55, 6760. [Google Scholar] [CrossRef] [Green Version]
- Colladay, D.; Kostelecky, V.A. Lorentz-violating extension of the standard model. Phys. Rev. D 1998, 58, 116002. [Google Scholar] [CrossRef] [Green Version]
- Kostelecky, V.A. Gravity, Lorentz violation, and the standard model. Phys. Rev. D 2004, 69, 105009. [Google Scholar] [CrossRef] [Green Version]
- Jacobson, T.; Mattingly, D. Gravity with a dynamical preferred frame. Phys. Rev. D 2001, 64, 024028. [Google Scholar] [CrossRef] [Green Version]
- Jacobson, T.; Mattingly, D. Einstein-ether waves. Phys. Rev. D 2004, 70, 024003. [Google Scholar] [CrossRef] [Green Version]
- Jacobson, T. Einstein-ather gravity: A status report. In Proceedings of the Conference ’From Quantum to Emergent Gravity: Theory and Phenomenology’, Trieste, Italy, 11–15 June 2007; SISSA: Trieste, Italy, 2007. v.2: PoS QG-Ph:020,2007; Sissa Medialab srl Partita IVA. [Google Scholar]
- Oost, J.; Mukohyama, S.; Wang, A. Constraints on Einstein-aether theory after GW170817. Phys. Rev. D 2018, 97, 124023. [Google Scholar] [CrossRef] [Green Version]
- Jacobson, T.; Liberati, S.; Mattingly, D. Threshold effects and Planck scale Lorentz violation: Combined constraints from high energy astrophysics. Phys. Rev. D 2003, 67, 124011. [Google Scholar] [CrossRef] [Green Version]
- Coleman, S.R.; Glashow, S.L. High-Energy Tests of Lorentz Invariance. Phys. Rev. D 1999, 59, 116008. [Google Scholar] [CrossRef] [Green Version]
- Liberati, S. Tests of Lorentz invariance: A 2013 update. Class. Quantum Gravity 2013, 30, 133001. [Google Scholar] [CrossRef]
- Aloisio, R.; Blasi, P.; Ghia, P.L.; Grillo, A.F. Probing the structure of space-time with cosmic rays. Phys. Rev. D 2000, 62, 053010. [Google Scholar] [CrossRef] [Green Version]
- Mattingly, D. Modern tests of lorentz invariance. Living Rev. Relativ. 2005, 8, 5. [Google Scholar] [CrossRef] [Green Version]
- Scully, S.T.; Stecker, F.W. Lorentz invariance violation and the observed spectrum of ultrahigh energy cosmic rays. Astropart. Phys. 2009, 31, 220. [Google Scholar] [CrossRef] [Green Version]
- Bi, X.J.; Cao, Z.; Li, Y.; Yuan, Q. Testing Lorentz invariance with ultra high energy cosmic ray spectrum. Phys. Rev. D 2009, 79, 083015. [Google Scholar] [CrossRef] [Green Version]
- Maccione, L.; Taylor, A.M.; Mattingly, D.M. Planck-scale Lorentz violation constrained by Ultra-High-Energy Cosmic Rays. J. Cosmol. Astropart. Phys. 2009, 2009, 022. [Google Scholar] [CrossRef]
- Scully, S.T.; Stecker, F.W. Testing Lorentz invariance with neutrinos from ultrahigh energy cosmic ray interactions. Astropart. Phys. 2011, 34, 575. [Google Scholar] [CrossRef] [Green Version]
- Saveliev, A.; Maccione, L.; Sigl, G. Lorentz invariance violation and chemical composition of ultrahigh-energy cosmic rays. J. Cosmol. Astropart. Phys. 2011, 2011, 046. [Google Scholar] [CrossRef] [Green Version]
- Stecker, F.W.; Scully, S.; Liberati, S.; Mattingly, D. Searching for traces of Planck-scale physics with high energy neutrinos. Phys. Rev. D 2015, 91, 045009. [Google Scholar] [CrossRef] [Green Version]
- Boncioli, D.; di Matteo, A.; Salamida, F.; Aloisio, R.; Blasi, P.; Ghia, P.L.; Grillo, A.F.; Petrera, S.; Pierog, T. Future prospects of testing Lorentz invariance with UHECRs. In Proceedings of the 34th International Cosmic Ray Conference (ICRC2015), Hague, The Netherlands, 30 July–6 August 2015. [Google Scholar]
- Stecker, F.W. Testing Lorentz symmetry using high energy astrophysics observations. Symmetry 2017, 9, 201. [Google Scholar] [CrossRef]
- Boncioli, D.; Pierre Auger Collaboration. Probing Lorentz symmetry with the Pierre Auger Observatory. In Proceedings of the 35th International Cosmic Ray Conference (ICRC2017), Bexco, Busan, Korea, 12–20 July 2017. [Google Scholar]
- Lang, R.G.; Filipčič, A.; Kukec Mezek, G.; Stanič, S.; Trini, M.; Vorobiov, S.; Yang, L.; Zavrtanik, D.; Zavrtanik, M.; Zehrer, L. Testing Lorentz Invariance Violation at the Pierre Auger Observatory. In Proceedings of the 36th International Cosmic Ray Conference, Madison, WI, USA, 24 July–1 August 2019. [Google Scholar]
- Burde, G.I. Special Relativity with a Preferred Frame and the Relativity Principle. J. Mod. Phys. 2018, 9, 1591. [Google Scholar] [CrossRef] [Green Version]
- Burde, G.I. Cosmological models based on relativity with a privileged frame. Int. J. Mod. Phys. D 2020, 29, 2050038. [Google Scholar] [CrossRef]
- Burde, G.I. Particle dynamics and GZK limit in relativity with a preferred frame. Astropart. Phys. 2021, 126, 102526. [Google Scholar] [CrossRef]
- Edwards, W.F. Special relativity in anisotropic space. Am. J. Phys. 1963, 31, 482. [Google Scholar] [CrossRef]
- Winnie, J.A. Special relativity without one-way velocity assumptions: Part II. Phil. Sci. 1970, 37, 223. [Google Scholar] [CrossRef]
- Tangherlini, F.R. The Velocity of Light in Uniformly Moving Frame. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 1958. Reproduced in: Abraham Zelmanov J. 2009, 2, 44. [Google Scholar]
- Ungar, A.A. The Lorentz transformation group of the special theory of relativity without Einstein’s isotropy convention. Phil. Sci. 1986, 53, 395. [Google Scholar] [CrossRef]
- Ungar, A.A. Formalism to deal with Reichenbach’s special theory of relativity. Found. Phys. 1991, 21, 691. [Google Scholar] [CrossRef]
- Anderson, R.; Vetharaniam, I.; Stedman, G.E. Conventionality of synchronisation, gauge dependence and test theories of relativity. Phys. Rep. 1998, 295, 93. [Google Scholar] [CrossRef]
- Minguzzi, E. On the conventionality of simultaneity. Found. Phys. Lett. 2002, 15, 153. [Google Scholar] [CrossRef] [Green Version]
- Rizzi, G.; Ruggiero, M.L.; Serafini, A. Synchronization gauges and the principles of special relativity. Found. Phys. 2004, 34, 1835. [Google Scholar] [CrossRef] [Green Version]
- Burde, G.I. Special relativity kinematics with anisotropic propagation of light and correspondence principle. Found. Phys. 2016, 46, 1573. [Google Scholar] [CrossRef]
- Robertson, H.P. Postulate versus observation in the special theory of relativity. Rev. Mod. Phys. 1949, 21, 378. [Google Scholar] [CrossRef] [Green Version]
- Mansouri, R.; Sexl, S.U. A test theory of special relativily: I. Simultaneity and slow clock synchronization. Gen. Rel. Grav. 1977, 8, 497. [Google Scholar] [CrossRef]
- Mansouri, R.; Sexl, S.U. A test theory of special relativily: II. First order tests. Gen. Rel. Grav. 1977, 8, 515. [Google Scholar] [CrossRef]
- Mansouri, R.; Sexl, S.U. A test theory of special relativily: III. Second order tests. Gen. Rel. Grav. 1977, 8, 809. [Google Scholar] [CrossRef]
- Lammerzahl, C. Test theories for Lorentz invariance. Lect. Notes Phys. 2006, 702, 349. [Google Scholar]
- de Angelis, A.; Maria, M.d.; Antonelli, M.; Dreucci, M. A search for directional violations of the Lorentz invariance through the study of a possible anisotropy of particle lifetimes. IL Nuovo Cimento 2011, 34 C, 323. [Google Scholar]
- Alhulaimi, B.; Coley, A.; Sandin, P. Anisotropic Einstein–aether cosmological models. J. Math. Phys. 2013, 54, 042503. [Google Scholar] [CrossRef] [Green Version]
- Kanno, S.; Soda, J. Lorentz violating inflation. Phys. Rev. D 2006, 74, 063505. [Google Scholar] [CrossRef] [Green Version]
- De Angelis, A.; Galanti, G.; Roncadelli, M. Transparency of the Universe to gamma rays. Mon. Not. Roy. Astron. Soc. 2013, 432, 3245. [Google Scholar] [CrossRef] [Green Version]
- Weinberg, S. Cosmology; Oxford University Press: Oxford, UK, 2008. [Google Scholar]
- Aloisio, R. Acceleration and propagation of ultra-high energy cosmic rays. Prog. Theor. Exp. Phys. 2017, 12, 12A102. [Google Scholar] [CrossRef] [Green Version]
- Aloisio, R.; Blasi, P.; Mitri, I.D.; Petrera, S. Selected Topics in Cosmic Ray Physics. In Multiple Messengers and Challenges in Astroparticle Physics; Aloisio, R., Coccia, E., Vissani, F., Eds.; Springer International Publishing AG: Cham, Switzerland, 2018; pp. 1–96. [Google Scholar]
- Anchordoqui, L.A. Ultra-High-Energy Cosmic Rays. Phys. Rept. 2019, 801, 1. [Google Scholar] [CrossRef] [Green Version]
- Greisen, K. End to the cosmic-ray spectrum? Phys. Rev. Lett. 1966, 16, 748. [Google Scholar] [CrossRef]
- Zatsepin, G.T.; Kuzmin, V.A. Upper limit of the spectrum of cosmic rays. Pisma Zh. Ekps. Teor. Fiz. 1966, 4, 114, English translation: JETP Lett. 1966, 4, 78. [Google Scholar]
- Aab, A.; Abreu, P.; Aglietta, M.A.R.C.O.; Ahn, E.J.; Al Samarai, I.; Albuquerque, I.F.M.; Allekotte, I.; Allen, J.; Allison, P.; Almela, A.; et al. Depth of maximum of air-shower profiles at the Pierre Auger Observatory II: Composition implications. Phys. Rev. D 2014, 90, 122006. [Google Scholar] [CrossRef] [Green Version]
- Aab, A.; Abreu, P.; Aglietta, M.A.R.C.O.; Ahn, E.J.; Al Samarai, I.; Albuquerque, I.F.M.; Allekotte, I.; Allison, P.; Almela, A.; Alvarez Castillo, J.; et al. Evidence for a mixed mass composition at the ‘ankle’ in the cosmic-ray spectrum. Phys. Lett. B 2016, 762, 288. [Google Scholar] [CrossRef] [Green Version]
- Bluman, G.W.; Kumei, S. Symmetries and Differential Equations, Applied Mathematical Sciences; Springer: New York, NY, USA, 1989; Volume 81. [Google Scholar]
- Olver, P.J. Applications of Lie Groups to Differential Equations (Graduate Texts in Mathematics: Volume 107); Springer: New York, NY, USA, 1993. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. The Classical Theory of Fields; Pergamon Press: Oxford, UK, 1971. [Google Scholar]
- Carroll, S.M.; Field, G.B.; Jackiw, R. Limits on a Lorentz- and parity-violating modification of electrodynamics. Phys. Rev. D 1990, 41, 1231. [Google Scholar] [CrossRef]
- Kostelecky’, V.A.; Mewes, M. Signals for Lorentz violation in electrodynamics. Phys. Rev. D 2002, 66, 056005. [Google Scholar] [CrossRef] [Green Version]
- Kostelecky, V.A.; Mewes, M. Electrodynamics with Lorentz-violating operators of arbitrary dimension. Phys. Rev. D 2009, 80, 015020. [Google Scholar] [CrossRef] [Green Version]
- Lang, R.G.; Martnez-Huerta, H.; de Souza, V. Limits on the Lorentz Invariance Violation from UHECR astrophysics. Astrophys. J. 2018, 853, 23. [Google Scholar] [CrossRef]
- Lang, R.G.; Martnez-Huerta, H.; de Souza, V. Improved limits on Lorentz invariance violation from astrophysical gamma-ray sources. Phys. Rev. D 2019, 99, 043015. [Google Scholar] [CrossRef] [Green Version]
- Martnez-Huerta, H.; Lang, R.G.; de Souza, V. Lorentz Invariance Violation Tests in Astroparticle Physics. Symmetry 2020, 12, 1232. [Google Scholar] [CrossRef]
- Cheng, H.; Wu, T.T. Cross Sections for Two-Pair Production at Infinite Energy. Phys. Rev. D 1970, 2, 2103–2104. [Google Scholar] [CrossRef]
- Brown, R.W.; Hunt, W.F.; Mikaelian, K.O.; Muzinich, I.J. Role of γ + γ⟶ e+ + e− + e+ + e− in Photoproduction, Colliding Beams, and Cosmic Photon Absorption. Phys. Rev. D 1973, 8, 3083–3102. [Google Scholar] [CrossRef]
- Demidov, S.V.; Kalashev, O.E. Double pair production by ultra–high–Energy Cosmic Ray Photons. J. Exp. Theor. Phys. 2009, 108, 764. [Google Scholar] [CrossRef] [Green Version]
- Ruffini, R.; Vereshchagin, G.; Xue, S.-S. Electron-positron pairs in physics and astrophysics: From heavy nuclei to black holes. Phys. Rep. 2010, 487, 1. [Google Scholar] [CrossRef] [Green Version]
- Ruffini, R.; Vereshchagin, G.V.; Xue, S.S. Cosmic absorption of ultra high energy particles. Astrophys. Space Sci. 2016, 361, 82. [Google Scholar] [CrossRef] [Green Version]
- Jelley, J.V. High-energy γ-ray absorption in Space by a 3.5°K microwave field. Phys. Rev. Lett. 1966, 16, 479–481. [Google Scholar] [CrossRef]
- Gould, R.J.; Schreder, D. Opacity of the Universe to High-Energy Photons. Phys. Rev. Lett. 1966, 16, 252. [Google Scholar] [CrossRef]
- Berezinsky, V.; Kalashev, O. High-energy electromagnetic cascades in extragalactic space: Physics and features. Phys. Rev. D 2016, 94, 023007. [Google Scholar] [CrossRef] [Green Version]
- Alves Batista, R.; Saveliev, A. The Gamma-ray Window to Intergalactic Magnetism. Universe 2021, 7, 223. [Google Scholar] [CrossRef]
- Bonometto, S.A.; Marcolungo, P. Metagalactic opacity to photons of energy larger than 1017 eV. Lett. Nuovo C. 1972, 5, 595–603. [Google Scholar] [CrossRef]
- Bonometto, S.A.; Lucchin, F.; Marcolungo, P. Induced Pair Production and Opacity Due to Black-body Radiation. Astron. Astrophys. 1974, 31, 41. [Google Scholar]
- Dermer, C.D.; Schlickeiser, R. Effects of triplet pair production on ultrarelativistic electrons in a soft photon field. Astron. Astrophys. 1991, 252, 414. [Google Scholar]
- Mastichiadis, A.; Protheroe, R.J.; Szabo, A.P. The Effect of Triplet Production on Pair/Compton Cascades in Thermal Radiation. Mon. Not. R. Astron. Soc. 1994, 266, 910. [Google Scholar] [CrossRef] [Green Version]
- De Angelis, A.; Mansutti, O.; Persic, M.; Roncadelli, M. Photon propagation and the very high energy γ–ray spectra of blazars: How transparent is the Universe? Mon. Not. R. Astron. Soc. Lett. 2009, 394, L21–L25. [Google Scholar] [CrossRef] [Green Version]
- Costamante, L. Gamma-Rays from Blazars and the Extragalactic Background Light. Int. J. Mod. Phys. D 2013, 22, 1330025. [Google Scholar] [CrossRef] [Green Version]
- Horns, D.; Jacholkowska, A. Gamma rays as probes of the Universe. Comptes Rendus Phys. 2016, 17, 632648. [Google Scholar] [CrossRef]
- Abdalla1, H.; Bottcher, M. EBL Inhomogeneity and Hard-Spectrum Gamma-Ray Sources. Astrophys. J. 2017, 835, 237. [Google Scholar] [CrossRef] [Green Version]
- Dzhatdoev, T.A.; Khalikov, E.V.; Kircheva, A.P.; Lyukshin, A.A. Electromagnetic cascade masquerade: A way to mimic γ–axion–like particle mixing effects in blazar spectra. Astron. Astrophys. 2017, 603, A59. [Google Scholar] [CrossRef] [Green Version]
- Franceschini, A. Photon–photon interactions and the opacity of the universe in gamma rays. Universe 2021, 7, 146. [Google Scholar] [CrossRef]
- Albert, J.; Aliu, E.; Anderhub, H.; Antonelli, L.A.; Antoranz, P.; Backes, M.; Baixeras, C.; Barrio, J.A.; Bartko, H.; Bastieri, D.; et al. Very-high-energy gamma rays from a distant quasar: How transparent is the universe? Science 2008, 320, 1752–1754. [Google Scholar] [CrossRef] [Green Version]
- Horns, D.; Meyer, M. Indications for a pair-production anomaly from the propagation of VHE gamma-rays. J. Cosmol. Astropart. Phys. 2012, 2, 33. [Google Scholar] [CrossRef] [Green Version]
- Meyer, M.; Horns, D.; Raue, M. First lower limits on the photon-axion-like particle coupling from very high energy gamma-ray observations. Phys. Rev. D 2013, 87, 035027. [Google Scholar] [CrossRef] [Green Version]
- Archambault, S.; Aune, T.; Behera, B.; Beilicke, M.; Benbow, W.; Berger, K.; Bird, R.; Biteau, J.; Bugaev, V.; Byrum, K.; et al. Deep broadband observations of the distant gamma–ray Blazar PKS 1424+240. Astrophys. J. Lett. 2014, 785, L16. [Google Scholar] [CrossRef] [Green Version]
- Finke, J.D.; Razzaque, S.; Dermer, C.D. Modeling the extragalactic background Llight from stars and dust. Astrophys. J. 2010, 712, 238. [Google Scholar] [CrossRef]
- Furniss, A.; Williams, D.A.; Danforth, C.; Fumagalli, M.; Prochaska, J.X.; Primack, J.; Urry, C.M.; Stocke, J.; Filippenko, A.V.; Neely, W. The firm redshift lower limit of the most distant TeV-detected blazar PKS 1424+240. Astrophys. J. Lett. 2013, 768, L31. [Google Scholar] [CrossRef]
- Horns, D.; Maccione, L.; Meyer, M.; Mirizzi, A.; Montanino, D.; Roncadelli, M. Hardening of TeV gamma spectrum of AGNs in galaxy clusters by conversions of photons into axionlike particles. Phys. Rev. D 2012, 86, 075024. [Google Scholar] [CrossRef] [Green Version]
- Troitsky, S. Towards discrimination between galactic and intergalactic axion-photon mixing. Phys. Rev. D 2016, 93, 045014. [Google Scholar] [CrossRef] [Green Version]
- Galanti, G.; Tavecchio, F.; Roncadelli, M.; Evoli, C. Blazar VHE spectral alterations induced by photon–ALP oscillations. Mon. Not. R. Astron. Soc. 2019, 487, 123132. [Google Scholar] [CrossRef] [Green Version]
- Kostelecky, V.A.; Mewes, M. Cosmological constraints on Lorentz violation in electrodynamics. Phys. Rev. Lett. 2001, 87, 251304. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kostelecky, V.A.; Mewes, M. Sensitive polarimetric search for relativity violations in gamma-ray bursts. Phys. Rev. Lett. 2006, 97, 140401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kostelecky, V.A.; Mewes, M. Lorentz-violating electrodynamics and the cosmic microwave background. Phys. Rev. Lett. 2007, 99, 011601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kostelecky, V.A.; Mewes, M. Astrophysical tests of Lorentz and CPT violation with photons. Astrophys. J. 2008, 689, L1. [Google Scholar] [CrossRef] [Green Version]
- Alam, S.; Ata, M.; Bailey, S.; Beutler, F.; Bizyaev, D.; Blazek, J.A.; Bolton, A.S.; Brownstein, J.R.; Burden, A.; Chuang, C.-H.; et al. The clustering of galaxies in the completed SDSS-III Baryon Oscillation Spectroscopic Survey: Cosmological analysis of the DR12 galaxy sample. Mon. Not. R. Astron. Soc. 2017, 470, 2617. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burde, G.I. Lorentz Violation by the Preferred Frame Effects and Cosmic and Gamma Ray Propagation. Galaxies 2021, 9, 119. https://doi.org/10.3390/galaxies9040119
Burde GI. Lorentz Violation by the Preferred Frame Effects and Cosmic and Gamma Ray Propagation. Galaxies. 2021; 9(4):119. https://doi.org/10.3390/galaxies9040119
Chicago/Turabian StyleBurde, Georgy I. 2021. "Lorentz Violation by the Preferred Frame Effects and Cosmic and Gamma Ray Propagation" Galaxies 9, no. 4: 119. https://doi.org/10.3390/galaxies9040119
APA StyleBurde, G. I. (2021). Lorentz Violation by the Preferred Frame Effects and Cosmic and Gamma Ray Propagation. Galaxies, 9(4), 119. https://doi.org/10.3390/galaxies9040119





