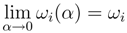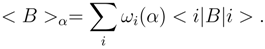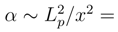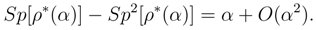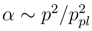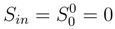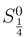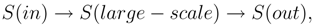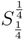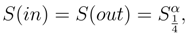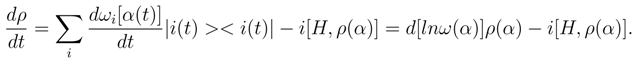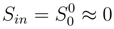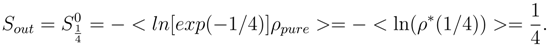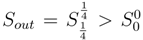Deformed Density Matrix and Quantum Entropy of the Black Hole
Abstract
:1 Introduction. Deformed Density Matrix in QMFL

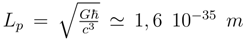 and α′ > 0 is a constant. In [9] it was shown that this constant may be chosen equal to 1. However, here we will use α′ as an arbitrary constant without giving it any definite value. Equation (1) is identified as the Generalized Uncertainty Relations in Quantum Mechanics.
and α′ > 0 is a constant. In [9] it was shown that this constant may be chosen equal to 1. However, here we will use α′ as an arbitrary constant without giving it any definite value. Equation (1) is identified as the Generalized Uncertainty Relations in Quantum Mechanics.

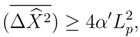
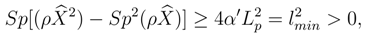
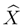 is the coordinate operator. Expression (5) gives the measuring rule used in well-known quantum mechanics QM. As distinct from QM, however, in the are considered here the right-hand side of (5) can not be brought arbitrary close to zero as it is limited by
is the coordinate operator. Expression (5) gives the measuring rule used in well-known quantum mechanics QM. As distinct from QM, however, in the are considered here the right-hand side of (5) can not be brought arbitrary close to zero as it is limited by 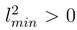 , where because of GUR lmin ∼ Lp.
, where because of GUR lmin ∼ Lp.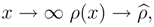 where
where  is the density matrix in QM. Since from [2],[5], [7] it follows that at Planck’s scale Sp[ρ] < 1, then for such scales ρ = ρ(x), where x is the scale, is not a density matrix as it is generally defined in QM. On Planck’s scale ρ(x) is referred to as ”density pro-matrix”. As follows from the above, the density matrix
is the density matrix in QM. Since from [2],[5], [7] it follows that at Planck’s scale Sp[ρ] < 1, then for such scales ρ = ρ(x), where x is the scale, is not a density matrix as it is generally defined in QM. On Planck’s scale ρ(x) is referred to as ”density pro-matrix”. As follows from the above, the density matrix  appears in the limit [1],[2]:
appears in the limit [1],[2]:
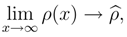
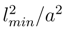 , whose specific weight decreases as the scale a expressed in units of lmin is going up. In the notation system used for
, whose specific weight decreases as the scale a expressed in units of lmin is going up. In the notation system used for  , where x is the scale for the fundamental deformation parameter.
, where x is the scale for the fundamental deformation parameter.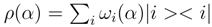 , where
, where
- 0 < α ≤ 1/4.
- The vectors |i > form a full orthonormal system.
- ωi(α) ≥ 0, and for all i the finite limit
exists.
- Sp[ρ(α)] = ∑i ωi(α) < 1, ∑i ωi = 1.
- For every operator B and any α there is a mean operator B depending on α:

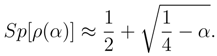
 is inferred from the relation
is inferred from the relation

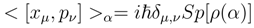
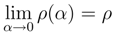 as the density matrix. Evidently, in the limit α → 0 we return to QM.
as the density matrix. Evidently, in the limit α → 0 we return to QM.- The above limit covers both Quantum and Classical Mechanics. Indeed, since
Għ/c3x2, we obtain:
- (ħ ≠ 0, x → ∞) ⇒ (α → 0) for QM;
- (ħ → 0, x → ∞) ⇒ (α → 0) for Classical Mechanics;
- As a matter of fact, the deformation parameter α should assume the value 0 < α ≤ 1. As seen from (8), however, Sp[ρ(α)] is well defined only for 0 < α ≤ 1/4. That is if x = ilmin and i ≥ 2, then there is no any problem. At the point of x = lmin there is a singularity related to the complex values following from Sp[ρ(α)] , i.e. to the impossibility of obtaining a diagonalized density pro-matrix at this point over the field of real numbers. For this reason definition 1 has no sense at the point x = lmin.
- We consider possible solutions for (7). For instance, one of the solutions of (7), at least to the first order in α, iswhere all αi > 0 are independent of α and their sum is equal to 1. In this way Sp[ρ∗(α)] = exp(−α). We can easily verify thatNote that in the momentum representation
, where ppl is the Planck momentum. When present in the matrix elements, exp(−α) can damp the contribution of great momenta in a perturbation theory.
2 Entropy Density Matrix and Information Loss Problem
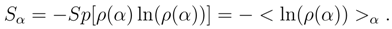
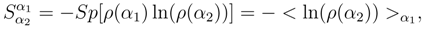
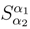 has a clear physical meaning: the entropy density is computed on the scale associated with the deformation parameter α2 by the observer who is at a scale corresponding to the deformation parameter α1. Note that with this approach the diagonal element Sα = ,of the described matrix
has a clear physical meaning: the entropy density is computed on the scale associated with the deformation parameter α2 by the observer who is at a scale corresponding to the deformation parameter α1. Note that with this approach the diagonal element Sα = ,of the described matrix 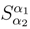 is the density of entropy measured by the observer who is at the same scale as the measured object associated with the deformation parameter α. In [2] Section 6 such a construction was used implicitly in derivation of the semiclassical Bekenstein-Hawking formula for the Black Hole entropy:
is the density of entropy measured by the observer who is at the same scale as the measured object associated with the deformation parameter α. In [2] Section 6 such a construction was used implicitly in derivation of the semiclassical Bekenstein-Hawking formula for the Black Hole entropy:
- a)
- For the initial (approximately pure) state
- b)
- Using the exponential ansatz(9),we obtain:
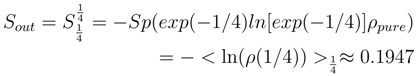
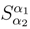 (10) is reduced only to one element
(10) is reduced only to one element 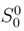 and so we can not test anything. Moreover, in previous works relating the quantum mechanics of black holes and information paradox [19],[35,36] the initial and final states when a particle hits the hole are treated proceeding from different theories(QM and QMFL respectively):
and so we can not test anything. Moreover, in previous works relating the quantum mechanics of black holes and information paradox [19],[35,36] the initial and final states when a particle hits the hole are treated proceeding from different theories(QM and QMFL respectively):
- 1)
- For the observer in the large-scale limit (producing measurements in the semiclassical approximation) α1 = 0S(in) =
(Origin singularity)
S(out) =(Singularity in Black Hole)
So S(in) = S(out) =. Consequently, the initial and final densities of entropy are equal and there is no any information loss.
- 2)
- For the observer moving together with the information flow in the general situation we have the chain:where S(large − scale) =
= S. Here S is the ordinary entropy at quantum mechanics(QM), but S(in) = S(out) =
,value considered in QMFL. So in this case the initial and final densities of entropy are equal without any loss of information.
- 3)
- This case is a special case of 2), when we do not come out of the early Universe considering the processes with the participation of black mini-holes only. In this case the originally specified chain becomes shorter by one Section:and member S(large − scale) =(Early Universe, origin singularity, QMFL, density pro-matrix)→ (Black Mini-Hole, singularity, QMFL, density pro-matrix),
= S disappears at the corresponding chain of the entropy density associated with the large-scale consideration:
It is, however, obvious that in case S(in) = S(out) =the density of entropy is preserved. Actually this event was mentioned in [2],where from the basic principles it has been found that black mini-holes do not radiate, just in agreement with the results of other authors [21,22,23,24]. As a result, it’s possible to write briefly
where α - any value in the interval 0 < α ≤ 1/4.
3 Entropy Bounds, Entropy Density and Holographic Principle
- An approach proposed in [34],[4] and in the present paper gives a deeper insight into the cause of high entropy for Planck’s black hole remnants, namely: high entropy density that by this approach at Planck scales takes place for every fixed observer including that on a customary scale, i.e. on α ≈ 0. In [4] using the exponential ansatz (Section 3) it has been demonstrated how this density can increase in the vicinity of the singularities withup towhen the initial state measured by the observer is pure.As demonstrated in [34],[4], increase in the entropy density will be realized also for the observer moving together with the information flow:
, though to a lesser extent than in the first case. Obviously, provided the existing solutions for (7) are different from the exponential ansatz, the entropy density for them
will be increasing as compared to
with a tendency of α2 to 1/4.
- In works of J.Bekenstein, [26] in particular, a ”universal entropy bound” has been used [27]:where M is the total gravitational mass of the matter and R is the radius of the smallest sphere that barely fits around a system. This bound is, however, valid for a weakly gravitating matter system only. In case of black hole remnants under study it is impossible to assume that on Planck scales we are concerned with a weakly gravitating matter system, as in this case the transition to the Planck’s energies is realized where quantum-gravitational effects are appreciable, and within the proposed paradigm parameter α ≈ 0 is changed by the parameter α > 0 or equally QM is changed by QMFL.
- This necessitates mentioning of the recent findings of R.Bousso [28],[29], who has derived the Bekenstein’s ”universal entropy bound” for a weakly gravitating matter system, and among other things in flat space, from the covariant entropy bound [30] associated with the holographic principle of Hooft-Susskind [31],[32],[33].
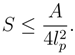
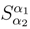 by its definition [34],[4] falls into 2D objects, being associated with
by its definition [34],[4] falls into 2D objects, being associated with 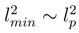 [2] and hence implicitly pointing to the holographic principle.
[2] and hence implicitly pointing to the holographic principle.4 Quantum corrections to black hole entropy. Heuristic approach
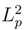 (e.g.[37],[38]). Also it is essential to derive this result from the basic principles given in this paper.
(e.g.[37],[38]). Also it is essential to derive this result from the basic principles given in this paper.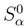 point b), in Section 2 may be written in the form of a series
point b), in Section 2 may be written in the form of a series
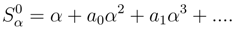
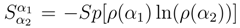 may be considered as a series of two variables α1 and α2. Fixing one of them, e.g. α1, it is possible to expand the series in terms of α2 parameter and to obtain the quantum corrections to the main result as more and more higher-order terms of this series. In the process, (13) is a partial case of the approach to α1 = 0 and α2 close to 1/4.
may be considered as a series of two variables α1 and α2. Fixing one of them, e.g. α1, it is possible to expand the series in terms of α2 parameter and to obtain the quantum corrections to the main result as more and more higher-order terms of this series. In the process, (13) is a partial case of the approach to α1 = 0 and α2 close to 1/4.5 Conclusion
References
- Shalyt-Margolin, A.E.; Suarez, J.G. Quantum Mechanics of the Early Universe and its Limiting Transition.
- Shalyt-Margolin, A.E.; Suarez, J.G. Quantum Mechanics at Planck’s scale and Density Matrix. Intern.Journ.of Mod.Phys. 2003, D.12, 1265. [Google Scholar]
- Shalyt-Margolin, A.E.; Ya, A. Tregubovich,Deformed Density Matrix and Generalized Uncertainty Relation in Thermodynamics. Mod. Phys. Lett. 2004, A19, 71. [Google Scholar]
- Shalyt-Margolin, A.E. Non-Unitary and Unitary Transitions in Generalized Quantum Mechanics, New Small Parameter and Information Problem Solving. Mod. Phys. Lett. 2004, A19, 391. [Google Scholar]
- Shalyt-Margolin, A.E. Pure States, Mixed States and Hawking Problem in Generalized Quantum Mechanics. Mod. Phys. Lett. 2004, A19, 2037. [Google Scholar] [CrossRef]
- Shalyt-Margolin, A.E. The Universe as a Nonuniform Lattice in Finite-Volume Hypercube.I.Fundamental Definitions and Particular Features. Intern.Journ.of Mod.Phys. 2004, D.13, 853. [Google Scholar]
- Shalyt-Margolin, A.E. The Density Matrix Deformation in Physics of the Early Universe and Some of its Implications. In Quantum Cosmology Research Trends. Horizons in World Physics; Volume 246, pp. 49–91. Nova Science Publishers, Inc.: Hauppauge, NY, 2005. [Google Scholar]
- Veneziano, G. A stringly nature needs just two constant. Europhys.Lett. 1986, 2, 199. [Google Scholar] Amati, D.; Ciafaloni, M.; Veneziano, G. Can spacetime be probed below the string size? Phys.Lett. 1989, B216, 41. [Google Scholar] Witten, E. Reflections on the Fate of Spacetime. Phys.Today 1996, 49, 24. [Google Scholar]
- Adler, R.J.; Santiago, D.I. On Gravity and the Uncertainty Principle. Mod.Phys.Lett. 1999, A14, 1371. [Google Scholar]
- Ahluwalia, D.V. Wave-Particle duality at the Planck scale: Freezing of neutrino oscillations. Phys.Lett. 2000, A275, 31. [Google Scholar] Interface of Gravitational and Quantum Realms. Mod.Phys.Lett. 2002, A17, 1135. Maggiore, M. A Generalized Uncertainty Principle in Quantum Gravity. Phys.Lett. 1993, B304, 65. [Google Scholar]
- Maggiore, M. Quantum Groups,Gravity and Generalized Uncertainty Principle. Phys.Rev. 1994, D49, 5182. [Google Scholar] Capozziello, S.; Lambiase, G.; Scarpetta, G. The Generalized Uncertainty Principle from Quantum Geometry. Int.J.Theor.Phys. 2000, 39, 15. [Google Scholar]
- Maggiore, M. The algebraic structure of the generalized uncertainty principle. Phys.Lett. 1993, B319, 83. [Google Scholar]
- Garay, L. Quantum Gravity and Minimum Length. Int.J.Mod.Phys.A. 1995, 10, 145. [Google Scholar]
- Ng, Y.J.; van Dam, H. Measuring the Foaminess of Space-Time with Gravity-Wave Interferometers. Found.Phys. 2000, 30, 795. [Google Scholar]
- Ng, Y.J.; van Dam, H. On Wigner’s clock and the detectability space-time foam with gravitational-wave interferometers. Phys.Lett. 2000, B477, 429. [Google Scholar]
- Baez, J.C.; Olson, S.J. Uncertainty in Measurment of Distance. Class.Quant.Grav. 2002, 19, L121. [Google Scholar]
- Guth, A.H. Inflation and EternaL Inflation. Phys.Rept. 2000, 333, 555. [Google Scholar]
- Kempf, A.; Mangano, G.; Mann, R.B. Hilbert Space Representation of the Minimal Length Uncertainty Relation. Phys.Rev. 1995, D52, 1108. [Google Scholar]
- Hawking, S. Phys.Rev. 1976, D14, 2460.
- Heisenberg, W. Zeitsch.fur Phys 1927, 43, 172.
- Adler, R.; Chen, P.; Santiago, D. Gen.Rel.Grav. 2001, 33, 2101.
- Chen, P.; Adler, R. Nucl.Phys.Proc.Suppl. 2003, 124, 103.
- Helfer, A.D. Rept.Prog.Phys. 2003, 66, 943.
- Custodio, P.S.; Horvath, J.E. Class.Quant.Grav. 2003, 20, L197.
- Bekenstein, J.D. Black holes and information theory. Contemp.Phys. 2003, 45, 31. [Google Scholar]
- Bekenstein, J.D. Entropy bounds and black hole remnants. Phys.Rev. 1994, D49, 1912. [Google Scholar]
- Bekenstein, J.D. A universal upper bound on the entropy to energy ratio for bounded systems. Phys.Rev. 1981, D23, 287. [Google Scholar] [CrossRef]
- Bousso, R. Light-sheets and Bekenstein’s bound. Phys.Rev.Lett. 2003, 90, 1213. [Google Scholar]
- Bousso, R. Flat space physics from holography. JHEP 2004, 0405, 050. [Google Scholar]
- Bousso, R. A Covariant Entropy Conjecture. JHEP 1999, 9907, 004. [Google Scholar]
- Hooft, G.’t. Dimension reduction in quantum gravity. The Holographic Principle. (Opening Lecture).
- Susskind, L. The world as a hologram. Journ.Math.Phys. 1995, 36, 6377. [Google Scholar]
- Bousso, R. The holographic principle. Rev.Mod.Phys. 2002, 74, 825. [Google Scholar]
- Shalyt-Margolin, A.E. Deformed density matrix, Density of entropy and Information problem.
- Strominger, A. preprint. hep-th/9501071.
- Giddings, S. preprint. hep-th/9508151.
- Khriplovich, I.B.; Korkin, R.V. How Is the Maximum Entropy of a Quantized Surface Related to Its Area?
- Khriplovich, I.B. Quantization and entropy of black holes. Khriplovich, I.B. Entropy and Area of Black Holes in Loop Quantum Gravity. Phys.Lett. 2002, B537, 125. [Google Scholar]
- Majumdar, P. Black hole entropy: classical and quantum aspects. In Expanded version of lectures given at the YATI Conference on Black Hole Astrophysics, Kolkata, India, April 2001. Das, S.; Majumdar, P.; Bhaduri, R.K. Class.Quant.Grav. 2002, 19, 2355. Vagenas, E.C. Phys.Lett. 2002, B533, 302.
- Majumdar, J. Black Holes in String Theory. Black Holes and D-branes. Nucl.Phys.Proc.Suppl. 1998, 61A. Nucl.Phys.Proc.Suppl. 1998, 62, 428.
- Maldacena, J.; Strominger, A. Statistical Entropy for Four-Dimensional Extremal Black Holes. Phys.Rev.Lett. 1996, 77, 428. [Google Scholar]
- Johnson, C.V.; Khuri, R.R.; Myers, R.C. Entropy of 4D Extremal Black Holes. Phys.Lett. 1996, B378, 78. [Google Scholar]
- Horowitz, G.T. Quantum States of Black Holes. gr-qc/9704072.
©2006 by MDPI (http://www.mdpi.org). Reproduction for noncommercial purposes permitted.
Share and Cite
Shalyt-Margolin, A.E. Deformed Density Matrix and Quantum Entropy of the Black Hole. Entropy 2006, 8, 31-43. https://doi.org/10.3390/e8010031
Shalyt-Margolin AE. Deformed Density Matrix and Quantum Entropy of the Black Hole. Entropy. 2006; 8(1):31-43. https://doi.org/10.3390/e8010031
Chicago/Turabian StyleShalyt-Margolin, A. E. 2006. "Deformed Density Matrix and Quantum Entropy of the Black Hole" Entropy 8, no. 1: 31-43. https://doi.org/10.3390/e8010031
APA StyleShalyt-Margolin, A. E. (2006). Deformed Density Matrix and Quantum Entropy of the Black Hole. Entropy, 8(1), 31-43. https://doi.org/10.3390/e8010031