Thermal Fisher Information for a Rotating BTZ Black Hole
Abstract
1. Introduction
2. General Formalism
2.1. Spacetime: The Rotating BTZ Black Hole
2.1.1. Co-Rotating Detector Trajectory
2.1.2. QFT in Curved Spacetime
2.1.3. Temperature of the Quantum Field
2.2. Unruh–DeWitt Particle Detectors
2.3. Fisher Information
3. Derivations
3.1. Metrology with Unruh–DeWitt Detectors
3.2. Response Rate for a Co-Rotating Detector
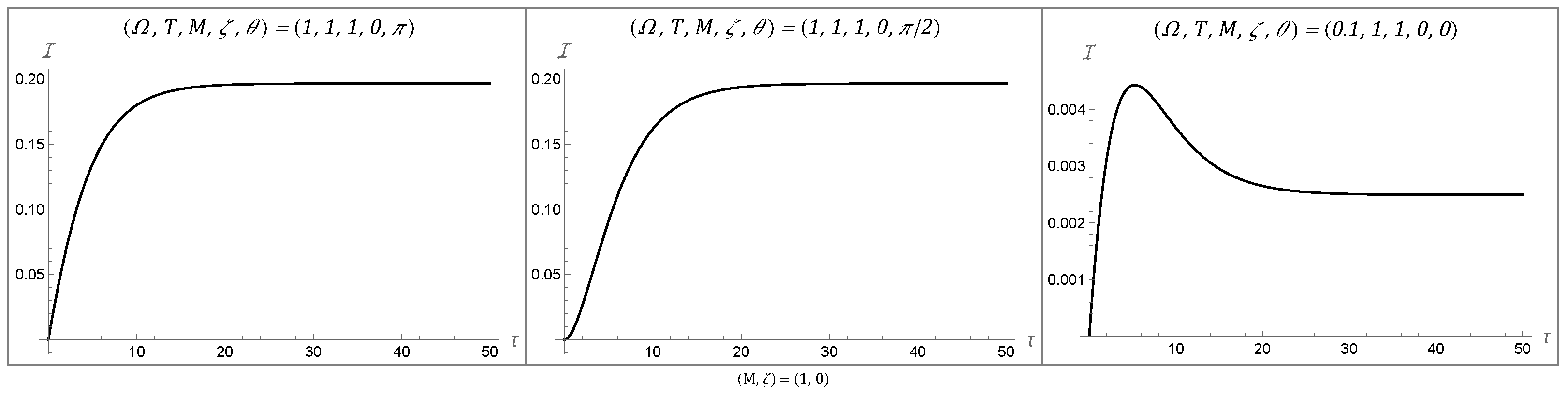
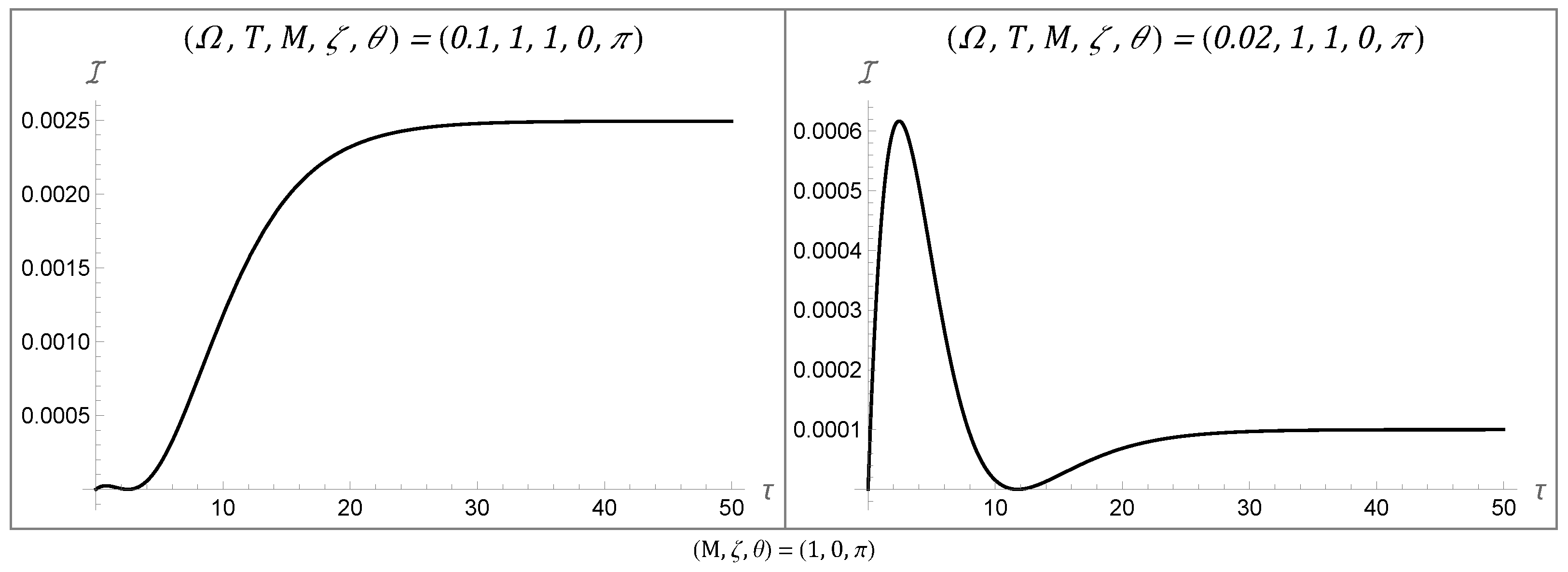
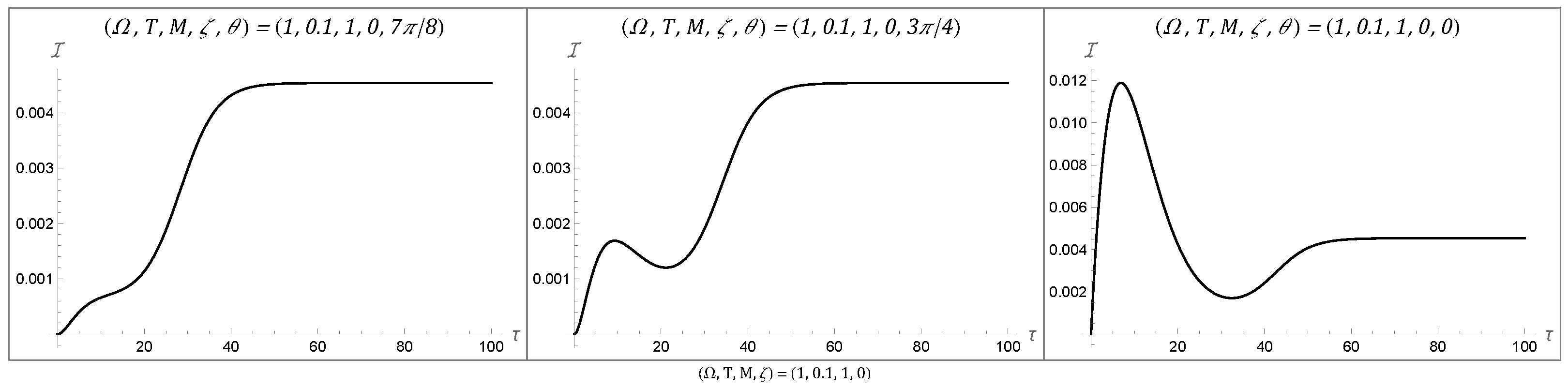
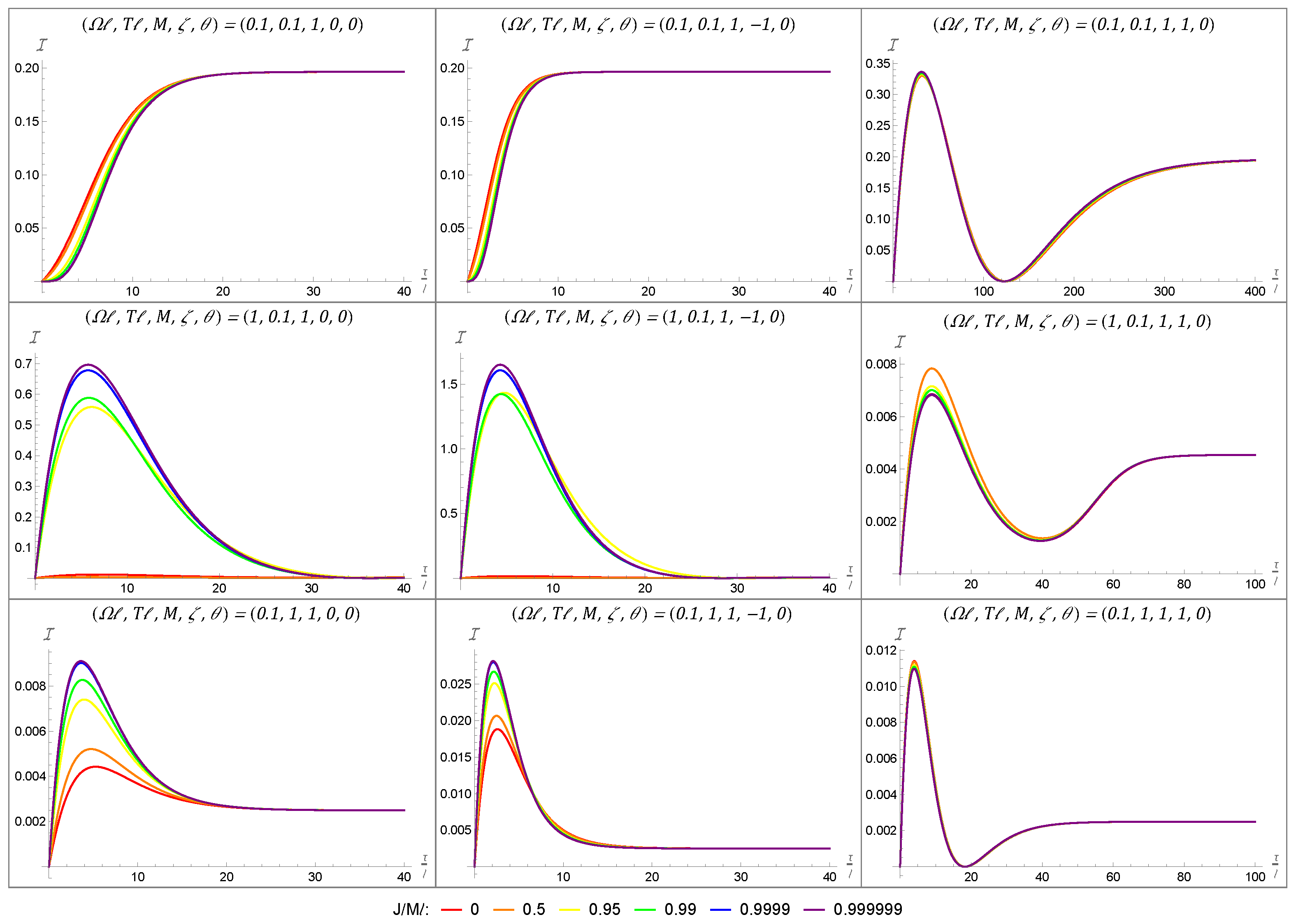
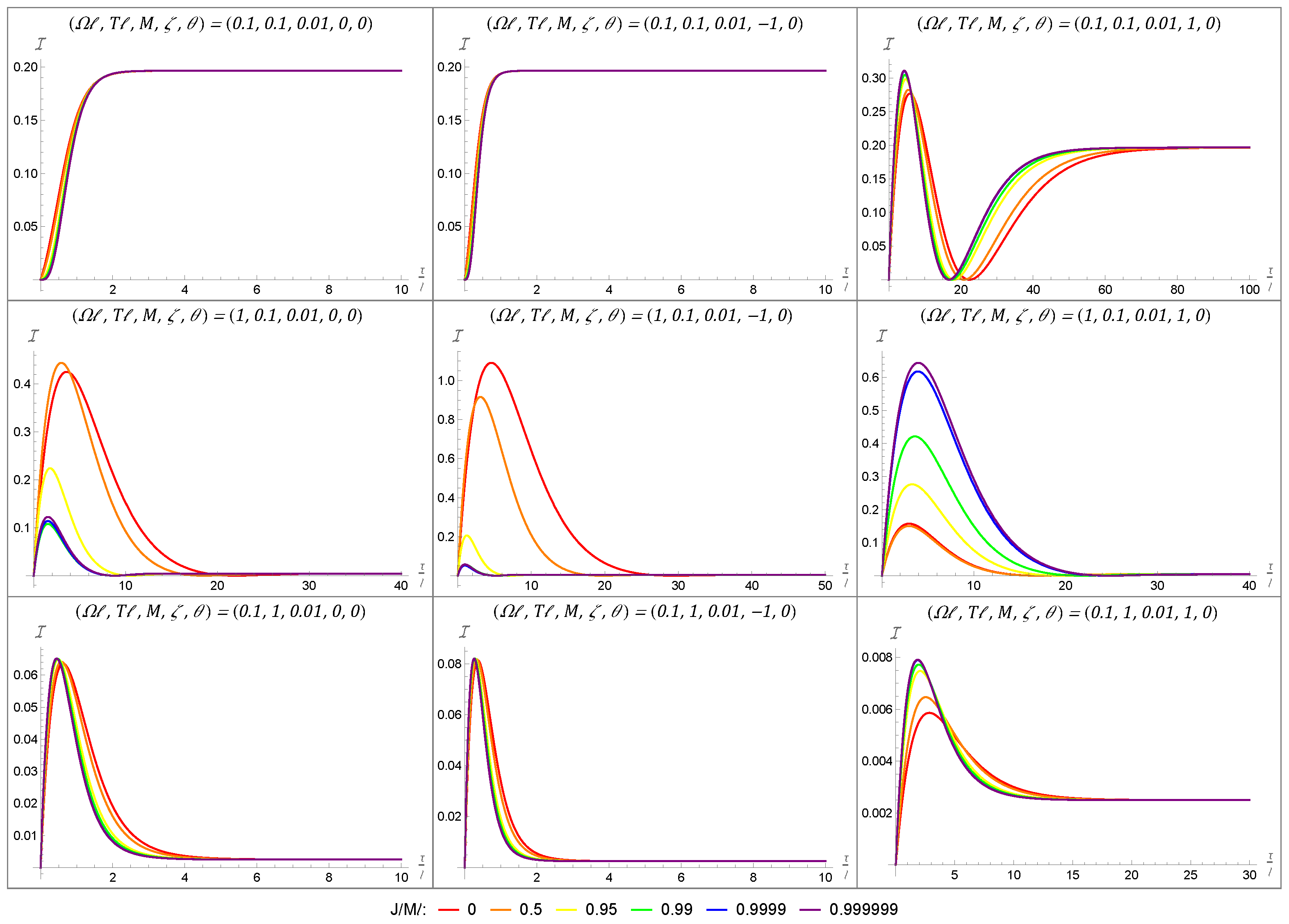
4. Results
4.1. Temporal Behaviors of the Fisher Information
4.2. Fisher Information Under Varying Angular Momentum
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- A century of correct predictions. Nat. Phys. 2019, 15, 415. [CrossRef]
- Fan, X.; Myers, T.; Sukra, B.; Gabrielse, G. Measurement of the Electron Magnetic Moment. Phys. Rev. Lett. 2023, 130, 071801. [Google Scholar] [CrossRef]
- Birrell, N.D.; Davies, P. Quantum Fields in Curved Space; Cambridge University Press: Cambridge, UK, 1984. [Google Scholar]
- Unruh, W.G. Notes on black hole evaporation. Phys. Rev. D 1976, 14, 870. [Google Scholar] [CrossRef]
- Hawking, S.W. Particle Creation by Black Holes. Commun. Math. Phys. 1975, 43, 199–220, Erratum in Commun. Math. Phys. 1976, 46, 206. [Google Scholar] [CrossRef]
- DeWitt, B.S. Quantum gravity: The new synthesis. In General relativity: An Einstein Centenary Survey; Hawking, S., Israel, W., Eds.; Cambridge University Press: Cambridge, UK, 1979; pp. 680–745. [Google Scholar]
- Mann, R.B.; Ralph, T.C. Relativistic quantum information. Class. Quantum Gravity 2012, 29, 220301. [Google Scholar] [CrossRef]
- Dowker, F. Useless Qubits in “Relativistic Quantum Information”. arXiv 2011, arXiv:1111.2308. [Google Scholar] [CrossRef]
- Sorkin, R. Impossible Measurements on Quantum Fields. In Directions in General Relativity: Proceedings of the 1993 International Symposium, Maryland: Papers in Honor of Dieter Brill; Hu, B.L., Jacobson, T.A., Eds.; Cambridge University Press: Cambridge, UK, 1993; pp. 293–305. [Google Scholar]
- Hotta, M. Quantum measurement information as a key to energy extraction from local vacuums. Phys. Rev. D 2008, 78, 045006. [Google Scholar] [CrossRef]
- Pozas-Kerstjens, A.; Martín-Martínez, E. Harvesting correlations from the quantum vacuum. Phys. Rev. D 2015, 92, 064042. [Google Scholar] [CrossRef]
- Pozas-Kerstjens, A.; Martín-Martínez, E. Entanglement harvesting from the electromagnetic vacuum with hydrogenlike atoms. Phys. Rev. D 2016, 94, 064074. [Google Scholar] [CrossRef]
- Cliche, M.; Kempf, A. Relativistic quantum channel of communication through field quanta. Phys. Rev. A 2010, 81, 012330. [Google Scholar] [CrossRef]
- Landulfo, A.G. Nonperturbative approach to relativistic quantum communication channels. Phys. Rev. D 2016, 93, 104019. [Google Scholar] [CrossRef]
- Kasprzak, M.; Tjoa, E. Transmission of quantum information through quantum fields in curved spacetimes. J. Phys. A Math. Theor. 2025, 58, 095301. [Google Scholar] [CrossRef]
- Gray, F.; Kubizňák, D.; May, T.; Timmerman, S.; Tjoa, E. Quantum imprints of gravitational shockwaves. J. High Energy Phys. 2021, 2021, 54. [Google Scholar] [CrossRef]
- Perche, T.R.; Martín-Martínez, E. Geometry of spacetime from quantum measurements. Phys. Rev. D 2022, 105, 066011. [Google Scholar] [CrossRef]
- Ahmadi, M.; Bruschi, D.E.; Sabín, C.; Adesso, G.; Fuentes, I. Relativistic Quantum Metrology: Exploiting relativity to improve quantum measurement technologies. Sci. Rep. 2014, 4, 4996. [Google Scholar] [CrossRef]
- Ahmadi, M.; Bruschi, D.E.; Fuentes, I. Quantum metrology for relativistic quantum fields. Phys. Rev. D 2014, 89, 065028. [Google Scholar] [CrossRef]
- Braunstein, S.L.; Caves, C.M. Statistical distance and the geometry of quantum states. Phys. Rev. Lett. 1994, 72, 3439–3443. [Google Scholar] [CrossRef]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum Metrology. Phys. Rev. Lett. 2006, 96, 010401. [Google Scholar] [CrossRef]
- Paris, M.G. Quantum estimation for quantum technology. Int. J. Quantum Inf. 2009, 7, 125–137. [Google Scholar] [CrossRef]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Advances in quantum metrology. Nat. Photon. 2011, 5, 222–229. [Google Scholar] [CrossRef]
- Degen, C.L.; Reinhard, F.; Cappellaro, P. Quantum sensing. Rev. Mod. Phys. 2017, 89, 035002. [Google Scholar] [CrossRef]
- DeMille, D.; Hutzler, N.R.; Rey, A.M.; Zelevinsky, T. Quantum sensing and metrology for fundamental physics with molecules. Nat. Phys. 2024, 20, 741–749. [Google Scholar] [CrossRef]
- Collaboration, T.L.S. A gravitational wave observatory operating beyond the quantum shot-noise limit. Nat. Phys. 2011, 7, 962–965. [Google Scholar] [CrossRef]
- Akutsu, T.; Ando, M.; Arai, K.; Arai, Y.; Araki, S.; Araya, A.; Aritomi, N.; Aso, Y.; Bae, S.; Bae, Y.; et al. Overview of KAGRA: Detector design and construction history. PTEP 2021, 2021, 05A101. [Google Scholar] [CrossRef]
- Cepollaro, C.; Giacomini, F.; Paris, M.G.A. Gravitational time dilation as a resource in quantum sensing. Quantum 2023, 7, 946. [Google Scholar] [CrossRef]
- Zhao, X.; Yang, Y.; Chiribella, G. Quantum Metrology with Indefinite Causal Order. Phys. Rev. Lett. 2020, 124, 190503. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Feng, J.; Zhang, Y.Z.; Fan, H. Quantum estimation in an expanding spacetime. Ann. Phys. 2018, 397, 336–350. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, J.; Wang, M.; Jing, J.; Tian, Z. Parameter estimation in cosmic string spacetime by using the inertial and accelerated detectors. Class. Quant. Grav. 2020, 37, 065017. [Google Scholar] [CrossRef]
- Yang, Y.; Jing, J.; Tian, Z. Probing cosmic string spacetime through parameter estimation. Eur. Phys. J. C 2022, 82, 688. [Google Scholar] [CrossRef]
- Aspachs, M.; Adesso, G.; Fuentes, I. Optimal Quantum Estimation of the Unruh-Hawking Effect. Phys. Rev. Lett. 2010, 105, 151301. [Google Scholar] [CrossRef]
- Feng, J.; Zhang, J.J. Quantum Fisher information as a probe for Unruh thermality. Phys. Lett. B 2022, 827, 136992. [Google Scholar] [CrossRef]
- Tian, Z.; Wang, J.; Jing, J.; Dragan, A. Entanglement Enhanced Thermometry in the Detection of the Unruh Effect. Ann. Phys. 2017, 377, 1–9. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, Y.; Jia, C.; Jing, J. Quantum thermometry in electromagnetic field of cosmic string spacetime. Quant. Inf. Proc. 2023, 22, 18. [Google Scholar] [CrossRef]
- Du, H.; Mann, R.B. Fisher information as a probe of spacetime structure: Relativistic quantum metrology in (A)dS. J. High Energy Phys. 2021, 2021, 1–22. [Google Scholar] [CrossRef]
- Patterson, E.; Mann, R.B. Fisher information of a black hole spacetime. J. High Energy Phys. 2023, 2023, 214. [Google Scholar] [CrossRef]
- Robbins, M.P.G.; Henderson, L.J.; Mann, R.B. Entanglement amplification from rotating black holes. Class. Quant. Grav. 2022, 39, 02LT01. [Google Scholar] [CrossRef]
- Robbins, M.P.G.; Mann, R.B. Anti-Hawking phenomena around a rotating BTZ black hole. Phys. Rev. D 2022, 106, 045018. [Google Scholar] [CrossRef]
- Bañados, M.; Teitelboim, C.; Zanelli, J. Black hole in three-dimensional spacetime. Phys. Rev. Lett. 1992, 69, 1849–1851. [Google Scholar] [CrossRef]
- Carlip, S. The (2 + 1)-dimensional black hole. Class. Quantum Gravity 1995, 12, 2853. [Google Scholar] [CrossRef]
- Lifschytz, G.; Ortiz, M. Scalar field quantization on the (2 + 1)-dimensional black hole background. Phys. Rev. D 1994, 49, 1929–1943. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and entropy. Phys. Rev. D 1973, 7, 2333–2346. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Carter, B.; Hawking, S.W. The Four laws of black hole mechanics. Commun. Math. Phys. 1973, 31, 161–170. [Google Scholar] [CrossRef]
- Hawking, S.W. Breakdown of Predictability in Gravitational Collapse. Phys. Rev. D 1976, 14, 2460–2473. [Google Scholar] [CrossRef]
- Kubo, R. Statistical-Mechanical Theory of Irreversible Processes. I. General Theory and Simple Applications to Magnetic and Conduction Problems. J. Phys. Soc. Jpn. 1957, 12, 570. [Google Scholar] [CrossRef]
- Martin, P.C.; Schwinger, J. Theory of Many-Particle Systems. I. Phys. Rev. 1959, 115, 1342–1373. [Google Scholar] [CrossRef]
- Lopp, R.; Martín-Martínez, E. Quantum delocalization, gauge, and quantum optics: Light-matter interaction in relativistic quantum information. Phys. Rev. A 2021, 103, 013703. [Google Scholar] [CrossRef]
- Rao, C.R. Information and the accuracy attainable in the estimation of statistical parameters. In Breakthroughs in Statistics; Springer: Berlin/Heidelberg, Germany, 1992; pp. 235–247. [Google Scholar]
- Cramér, H. Mathematical Methods of Statistics; Princeton University Press: Princeton, NJ, USA, 1999; Volume 43. [Google Scholar]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2007. [Google Scholar] [CrossRef]
- Chen, L.; Feng, J. Quantum Fisher information of a cosmic qubit undergoing non-Markovian de Sitter evolution. arXiv 2024, arXiv:2411.11490. [Google Scholar] [CrossRef]
- Han, S.W.; Chen, W.; Chen, L.; Ouyang, Z.; Feng, J. The reliable quantum master equation of the Unruh-DeWitt detector. arXiv 2025, arXiv:2502.06411. [Google Scholar]
- Kaplanek, G.; Tjoa, E. Effective master equations for two accelerated qubits. Phys. Rev. A 2023, 107, 012208. [Google Scholar] [CrossRef]
- Benatti, F.; Floreanini, R. Entanglement generation in uniformly accelerating atoms: Reexamination of the Unruh effect. Phys. Rev. A 2004, 70, 012112. [Google Scholar] [CrossRef]
- Manzano, D. A short introduction to the Lindblad master equation. AIP Adv. 2020, 10, 025106. [Google Scholar] [CrossRef]
- Henderson, L.J.; Hennigar, R.A.; Mann, R.B.; Smith, A.R.; Zhang, J. Anti-Hawking phenomena. Phys. Lett. B 2020, 809, 135732. [Google Scholar] [CrossRef]
- Hodgkinson, L.; Louko, J. Static, stationary, and inertial Unruh-DeWitt detectors on the BTZ black hole. Phys. Rev. D 2012, 86, 064031. [Google Scholar] [CrossRef]
- Suryaatmadja, C.; Arabaci, C.S.; Robbins, M.P.G.; Foo, J.; Zych, M.; Mann, R.B. Signatures of rotating black holes in quantum superposition. Phys. Rev. D 2024, 110, 066018. [Google Scholar] [CrossRef]
- Preciado-Rivas, M.R.; Naeem, M.; Mann, R.B.; Louko, J. More excitement across the horizon. Phys. Rev. D 2024, 110, 025002. [Google Scholar] [CrossRef]
- Wang, S.; Preciado-Rivas, M.R.; Spadafora, M.; Mann, R.B. Singular excitement beyond the horizon of a rotating black hole. Phys. Rev. D 2024, 110, 065013. [Google Scholar] [CrossRef]
- Biermann, S.; Erne, S.; Gooding, C.; Louko, J.; Schmiedmayer, J.; Unruh, W.G.; Weinfurtner, S. Unruh and analogue Unruh temperatures for circular motion in 3 + 1 and 2 + 1 dimensions. Phys. Rev. D 2020, 102, 085006. [Google Scholar] [CrossRef]
- Bunney, C.R.D.; Barroso, V.S.; Biermann, S.; Geelmuyden, A.; Gooding, C.; Ithier, G.; Rojas, X.; Louko, J.; Weinfurtner, S. Third sound detectors in accelerated motion. New J. Phys. 2024, 26, 065001. [Google Scholar] [CrossRef]
- Weinfurtner, S.; Tedford, E.W.; Penrice, M.C.J.; Unruh, W.G.; Lawrence, G.A. Measurement of stimulated Hawking emission in an analogue system. Phys. Rev. Lett. 2011, 106, 021302. [Google Scholar] [CrossRef]
- Steinhauer, J. Observation of quantum Hawking radiation and its entanglement in an analogue black hole. Nat. Phys. 2016, 12, 959. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patterson, E.A.; Mann, R.B. Thermal Fisher Information for a Rotating BTZ Black Hole. Entropy 2025, 27, 478. https://doi.org/10.3390/e27050478
Patterson EA, Mann RB. Thermal Fisher Information for a Rotating BTZ Black Hole. Entropy. 2025; 27(5):478. https://doi.org/10.3390/e27050478
Chicago/Turabian StylePatterson, Everett A., and Robert B. Mann. 2025. "Thermal Fisher Information for a Rotating BTZ Black Hole" Entropy 27, no. 5: 478. https://doi.org/10.3390/e27050478
APA StylePatterson, E. A., & Mann, R. B. (2025). Thermal Fisher Information for a Rotating BTZ Black Hole. Entropy, 27(5), 478. https://doi.org/10.3390/e27050478




