Quantum Models of Consciousness from a Quantum Information Science Perspective
Abstract
1. Introduction
2. Quantum Consciousness Emerging from the Microtubules Within Neurons
Superradiant Excitonic States in Microtubules
3. Quantum Consciousness Emerging from the EM Field Surrounding Neurons
Synchronized Firing Through the Correlations Between Neurons
4. Quantum Consciousness Emerging from the Molecular Interactions Among Neurons
- Possess a long nuclear-spin coherence time to function as a qubit.
- Have a method for transporting this qubit throughout the brain and into neurons.
- Include a molecular scale quantum memory for storing the qubits.
- Contain a mechanism for quantum entangling multiple qubits.
- Initiate a chemical reaction that triggers quantum measurements, which in turn determine subsequent neuron firing rates, among other things.
5. Study of the Entanglement Preservation
6. Theoretical Explanation
6.1. Hamiltonian Transformation
- for ,
- for ,
- for ,
- for ,
6.2. Calculation for
6.3. Interpretation of the Results
6.4. Numerical Verification
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- McCulloch, W.S.; Pitts, W. A logical calculus of the ideas immanent in nervous activity. Bull. Math. Biophys. 1943, 5, 115–133. [Google Scholar] [CrossRef]
- Hodgkin, A.L.; Huxley, A.F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 1952, 117, 500–544. [Google Scholar] [CrossRef]
- Jackson, P.T. Quantum Mind and Social Science: Unifying Physical and Social Ontology; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar] [CrossRef]
- Globus, G. Quantum brain theory and the appearing of world. J. Integr. Neurosci. 2017, 16, S37–S42. [Google Scholar] [CrossRef]
- Litt, A.; Eliasmith, C.; Kroon, F.W.; Weinstein, S.; Thagard, P. Is the brain a quantum computer? Cogn. Sci. 2006, 30, 593–603. [Google Scholar] [CrossRef]
- Perlovsky, L.I. Physics of the mind. Front. Syst. Neurosci. 2016, 10, 84. [Google Scholar] [CrossRef]
- Schoeller, F.; Perlovsky, L.; Arseniev, D. Physics of mind: Experimental confirmations of theoretical predictions. Phys. Life Rev. 2018, 25, 45–68. [Google Scholar] [CrossRef]
- Galadí, J.A. The mind-body problem: An overview of proposed solutions. In The Theory of Mind Under Scrutiny: Psychopathology, Neuroscience, Philosophy of Mind and Artificial Intelligence; Springer: Cham, Switzerland, 2024; Volume 34, pp. 435–467. [Google Scholar]
- Suojanen, M. Conscious Experience and Quantum Consciousness Theory: Theories, Causation, and Identity. Logos Electron. J. Philos. 2019, 26, 14–34. [Google Scholar] [CrossRef]
- McGowan, B. Consciousness and Physicalism; CUNY Academic Works: New York, NY, USA, 2024. [Google Scholar]
- Bayne, T.; Seth, A.K.; Massimini, M.; Shepherd, J.; Cleeremans, A.; Fleming, S.M.; Malach, R.; Mattingley, J.B.; Menon, D.K.; Owen, A.M.; et al. Tests for consciousness in humans and beyond. Trends Cogn. Sci. 2024, 28, 454–466. [Google Scholar] [CrossRef]
- Hiley, B.J.; Pylkkänen, P. Can Quantum Mechanics Solve the Hard Problem of Consciousness? In Consciousness and Quantum Mechanics; Gao, S., Ed.; Oxford University Press: Oxford, UK, 2022. [Google Scholar]
- Adams, B.; Petruccione, F. Quantum effects in the brain: A review. AVS Quantum Sci. 2020, 2, 5170. [Google Scholar] [CrossRef]
- Yago Malo, J.; Cicchini, G.M.; Morrone, M.C.; Chiofalo, M.L. Quantum spin models for numerosity perception. PLoS ONE 2023, 18, e0284610. [Google Scholar] [CrossRef] [PubMed]
- Vitiello, G. Dissipation and memory capacity in the quantum brain model. Int. J. Mod. Phys. 1995, 9, 973–989. [Google Scholar] [CrossRef]
- Pribram, K.H. Brain and Perception: Holonomy and Structure in Figural Processing; Psychology Press: Hove, UK, 2013. [Google Scholar]
- Nishiyama, A.; Tanaka, S.; Tuszynski, J.A.; Tsenkova, R. Holographic Brain Theory: Super-Radiance, Memory Capacity and Control Theory. Int. J. Mol. Sci. 2024, 25, 2399. [Google Scholar] [CrossRef]
- Penrose, R.; Hameroff, S.R. What ‘Gaps’? Reply to Grush and Churchland. J. Conscious. Stud. 1995, 2, 98–111. [Google Scholar]
- Hameroff, S.; Penrose, R. Orchestrated reduction of quantum coherence in brain microtubules: A model for consciousness. Math. Comput. Simul. 1996, 40, 453–480. [Google Scholar] [CrossRef]
- Hameroff, S.R.; Penrose, R. Conscious events as orchestrated space-time selections. J. Conscious. Stud. 1996, 3, 36–53. [Google Scholar] [CrossRef]
- Hameroff, S.R. Quantum computation in brain microtubules? The Penrose–Hameroff ‘Orch OR’ model of consciousness. Philos. Trans. R. Soc. London Ser. Math. Phys. Eng. Sci. 1998, 356, 1869–1896. [Google Scholar] [CrossRef]
- Hameroff, S.; Penrose, R. Consciousness in the universe: A review of the Orch ORtheory. Phys. Life Rev. 2014, 11, 39–78. [Google Scholar] [CrossRef]
- McFadden, J. Quantum Evolution—The New Science of Life, 1st ed.; HarperCollins: London, UK, 2000. [Google Scholar]
- McFadden, J. Synchronous firing and its influence on the brain’s electromagnetic field. J. Conscious. Stud. 2002, 9, 23–50. [Google Scholar]
- McFadden, J. The conscious electromagnetic information (Cemi) field theory. J. Conscious. Stud. 2002, 9, 45–60. [Google Scholar]
- McFadden, J. The CEMI field theory: Closing the loop. J. Conscious. Stud. 2013, 20, 153–168. [Google Scholar]
- McFadden, J. The CEMI field theory: Gestalt information and the meaning of meaning. J. Conscious. Stud. 2013, 20, 152–182. [Google Scholar]
- Fisher, M.P. Quantum cognition: The possibility of processing with nuclear spins in the brain. Ann. Phys. 2015, 362, 593–602. [Google Scholar] [CrossRef]
- Gassab, L.; Pusuluk, O.; Müstecaplıoğlu, Ö.E. Geometrical optimization of spin clusters for the preservation of quantum coherence. Phys. Rev. 2024, 109, 012424. [Google Scholar] [CrossRef]
- Hameroff, S.R. The brain is both neurocomputer and quantum computer. Cogn. Sci. 2007, 31, 1035–1045. [Google Scholar] [CrossRef]
- Penrose, R. The Emperor’s New Mind: Concerning Computers, Minds, and the Laws of Physics; Oxford University Press, Inc.: Oxford, UK, 1989. [Google Scholar]
- Penrose, R. Shadows of the Mind: A Search for the Missing Science of Consciousness, 1st ed.; Oxford University Press, Inc.: Oxford, UK, 1996. [Google Scholar]
- Donadi, S.; Piscicchia, K.; Curceanu, C.; Diósi, L.; Laubenstein, M.; Bassi, A. Underground test of gravity-related wave function collapse. Nat. Phys. 2021, 17, 74–78. [Google Scholar] [CrossRef]
- Penrose, R. On Gravity’s role in Quantum State Reduction. Gen. Relativ. Gravit. 1996, 28, 581–600. [Google Scholar] [CrossRef]
- Penrose, R. Wavefunction collapse as a real gravitational effect. In Mathematical Physics 2000; Imperial College Press: London, UK, 2000; pp. 266–282. [Google Scholar] [CrossRef]
- Penrose, R. Black holes, quantum theory and cosmology. J. Phys. Conf. Ser. 2009, 174, 012001. [Google Scholar] [CrossRef]
- Hameroff, S.R.; Watt, R.C. Information processing in microtubules. J. Theor. Biol. 1982, 98, 549–561. [Google Scholar] [CrossRef] [PubMed]
- Hameroff, S.R. Ultimate Computing: Biomolecular Consciouness and Nano Technology; Elsevier Science Inc.: Amsterdam, The Netherlands, 1987. [Google Scholar]
- Jibu, M.; Hagan, S.; Hameroff, S.R.; Pribram, K.H.; Yasue, K. Quantum optical coherence in cytoskeletal microtubules: Implications for brain function. Biosystems 1994, 32, 195–209. [Google Scholar] [CrossRef] [PubMed]
- Hameroff, S.R. Quantum Coherence in Microtubules: A Neural Basis for Emergent Consciousness? J. Conscious. Stud. 1994, 1, 91–118. [Google Scholar]
- Hameroff, S.; Nip, A.; Porter, M.; Tuszynski, J. Conduction pathways in microtubules, biological quantum computation, and consciousness. Biosystems 2002, 64, 149–168. [Google Scholar] [CrossRef] [PubMed]
- Craddock, T.J.; Tuszynski, J.A. On the role of the microtubules in cognitive brain functions. NeuroQuantology 2007, 5, 32–57. [Google Scholar] [CrossRef]
- Mavromatos, N.E. Quantum coherence in (brain) microtubules and efficient energy and information transport. Proc. J. Phys. Conf. Ser. IOP Publ. 2011, 329, 012026. [Google Scholar] [CrossRef]
- Craddock, T.J.A.; Friesen, D.; Mane, J.; Hameroff, S.; Tuszynski, J.A. The feasibility of coherent energy transfer in microtubules. J. R. Soc. Interface 2014, 11, 20140677. [Google Scholar] [CrossRef] [PubMed]
- Craddock, J.A.T.; Hameroff, R.S.; Ayoub, T.A.; Klobukowski, M.; Tuszynski, A.J. Anesthetics act in quantum channels in brain microtubules to prevent consciousness. Curr. Top. Med. Chem. 2015, 15, 523–533. [Google Scholar] [CrossRef]
- Tegmark, M. Importance of quantum decoherence in brain processes. Phys. Rev. 2000, 61, 4194. [Google Scholar] [CrossRef] [PubMed]
- Hagan, S.; Hameroff, S.R.; Tuszyński, J.A. Quantum computation in brain microtubules: Decoherence and biological feasibility. Phys. Rev. 2002, 65, 061901. [Google Scholar] [CrossRef] [PubMed]
- Babcock, N.; Montes-Cabrera, G.; Oberhofer, K.; Chergui, M.; Celardo, G.; Kurian, P. Ultraviolet superradiance from mega-networks of tryptophan in biological architectures. J. Phys. Chem. 2024, 128, 4035–4046. [Google Scholar] [CrossRef]
- Kalra, A.P.; Benny, A.; Travis, S.M.; Zizzi, E.A.; Morales-Sanchez, A.; Oblinsky, D.G.; Craddock, T.J.; Hameroff, S.R.; MacIver, M.B.; Tuszynski, J.A.; et al. Electronic energy migration in microtubules. ACS Cent. Sci. 2023, 9, 352–361. [Google Scholar] [CrossRef] [PubMed]
- Patwa, H.; Babcock, N.S.; Kurian, P. Quantum-enhanced photoprotection in neuroprotein architectures emerges from collective light-matter interactions. Front. Phys. 2024, 12, 1387271. [Google Scholar] [CrossRef]
- Scholes, G.D.; Fleming, G.R.; Chen, L.X.; Aspuru-Guzik, A.; Buchleitner, A.; Coker, D.F. Using coherence to enhance function in chemical and biophysical systems. Nature 2017, 543, 647–656. [Google Scholar] [CrossRef] [PubMed]
- McFadden, J.; Al-Khalili, J. The origins of quantum biology. Proc. R. Soc. 2018, 474, 20180674. [Google Scholar] [CrossRef] [PubMed]
- Spano, F.C.; Mukamel, S. Superradiance in molecular aggregates. J. Chem. Phys. 1989, 91, 683–700. [Google Scholar] [CrossRef]
- Grad, J.; Hernandez, G.; Mukamel, S. Radiative decay and energy transfer in molecular aggregates: The role of intermolecular dephasing. Phys. Rev. 1988, 37, 3835–3846. [Google Scholar] [CrossRef] [PubMed]
- Akkermans, E.; Gero, A.; Kaiser, R. Photon Localization and Dicke Superradiance in Atomic Gases. Phys. Rev. Lett. 2008, 101, 103602. [Google Scholar] [CrossRef]
- Bienaimé, T.; Bachelard, R.; Piovella, N.; Kaiser, R. Cooperativity in light scattering by cold atoms. Fortschritte Phys. 2013, 61, 377–392. [Google Scholar] [CrossRef]
- Mostafazadeh, A. Pseudo-Hermiticity versus PT symmetry: The necessary condition for the reality of the spectrum of a non-Hermitian Hamiltonian. J. Math. Phys. 2002, 43, 205–214. [Google Scholar] [CrossRef]
- Mostafazadeh, A. Pseudo-Hermiticity versus PT-symmetry. II. A complete characterization of non-Hermitian Hamiltonians with a real spectrum. J. Math. Phys. 2002, 43, 2814–2816. [Google Scholar] [CrossRef]
- Mostafazadeh, A. Pseudo-Hermiticity versus PT-symmetry III: Equivalence of pseudo-Hermiticity and the presence of antilinear symmetries. J. Math. Phys. 2002, 43, 3944–3951. [Google Scholar] [CrossRef]
- Brody, D.C. Biorthogonal quantum mechanics. J. Phys. Math. Theor. 2013, 47, 035305. [Google Scholar] [CrossRef]
- Celardo, G.; Angeli, M.; Craddock, T.; Kurian, P. On the existence of superradiant excitonic states in microtubules. New J. Phys. 2019, 21, 023005. [Google Scholar] [CrossRef]
- Baumgratz, T.; Cramer, M.; Plenio, M.B. Quantifying coherence. Phys. Rev. Lett. 2014, 113, 140401. [Google Scholar] [CrossRef] [PubMed]
- Pusuluk, O.; Farrow, T.; Deliduman, C.; Vedral, V. Emergence of correlated proton tunnelling in water ice. Proc. R. Soc. 2019, 475, 20180867. [Google Scholar] [CrossRef]
- Theurer, T.; Killoran, N.; Egloff, D.; Plenio, M.B. Resource Theory of Superposition. Phys. Rev. Lett. 2017, 119, 230401. [Google Scholar] [CrossRef] [PubMed]
- Pusuluk, O. Biorthogonal resource theory of genuine quantum superposition. arXiv 2024, arXiv:2210.02398. [Google Scholar] [CrossRef]
- Yeşiller, M.H.; Pusuluk, O. Electron delocalization in aromaticity as a superposition phenomenon. arXiv 2024, arXiv:2307.00672. [Google Scholar] [CrossRef]
- Dubin, F.; Melet, R.; Barisien, T.; Grousson, R.; Legrand, L.; Schott, M.; Voliotis, V. Macroscopic coherence of a single exciton state in an organic quantum wire. Nat. Phys. 2006, 2, 32–35. [Google Scholar] [CrossRef]
- Popper, K.R.; Lindahl, B.I.B.; Århem, P. A discussion of the mind-brain problem. Theor. Med. 1993, 14, 167–180. [Google Scholar] [CrossRef] [PubMed]
- Lindahl, B.; Århem, P. Mind as a force field: Comments on a new interactionistic hypothesis. J. Theor. Biol. 1994, 171, 111–122. [Google Scholar] [CrossRef] [PubMed]
- Libet, B.W. A testable theory of mind-brain interaction. J. Conscious. Stud. 1994, 1, 119–126. [Google Scholar]
- Libet, B. Conscious mind as a field. J. Theor. Biol. 1996, 178, 223–224. [Google Scholar] [CrossRef][Green Version]
- Pockett, S. The Nature of Consciousness: A Hypothesis, 1st ed.; iUniverse: Bloomington, IA, USA, 2000. [Google Scholar]
- John, E. A Field Theory of Consciousness. Conscious. Cogn. 2001, 10, 184–213. [Google Scholar] [CrossRef] [PubMed]
- Pockett, S. The electromagnetic field theory of consciousness: A testable hypothesis about the characteristics of conscious as opposed to non-conscious fields. J. Conscious. Stud. 2012, 19, 191–223. [Google Scholar]
- Varela, F.; Lachaux, J.P.; Rodriguez, E.; Martinerie, J. The brainweb: Phase synchronization and large-scale integration. Nat. Rev. Neurosci. 2001, 2, 229–239. [Google Scholar] [CrossRef] [PubMed]
- Bertagna, F.; Lewis, R.; Silva, S.R.P.; McFadden, J.; Jeevaratnam, K. Effects of electromagnetic fields on neuronal ion channels: A systematic review. Ann. N. Y. Acad. Sci. 2021, 1499, 82–103. [Google Scholar] [CrossRef] [PubMed]
- McFadden, J. The electromagnetic will. NeuroSci 2021, 2, 291–304. [Google Scholar] [CrossRef]
- McFadden, J. Integrating information in the brain’s EM field: The cemi field theory of consciousness. Neurosci. Conscious. 2020, 2020, niaa016. [Google Scholar] [CrossRef]
- McFadden, J. Consciousness: Matter or EMF? Front. Hum. Neurosci. 2023, 16, 1024934. [Google Scholar] [CrossRef]
- McFadden, J. Carving nature at its joints: A Comparison of CEMI field theory with integrated information theory and global workspace theory. Entropy 2023, 25, 1635. [Google Scholar] [CrossRef] [PubMed]
- Jibu, M.; Yasue, K. 15. Quantum Brain Dynamics and Quantum Field Theory. In Brain and Being; Globus, G.G., Pribram, K.H., Vitiello, G., Eds.; John Benjamins Publishing Company: Amsterdam, The Netherlands, 2004; pp. 269–292. [Google Scholar] [CrossRef]
- Jibu, M.; Pribram, K.H.; Yasue, K. From Conscious Experience to Memory Storage and Retrieval: The Role of Quantum Brain Dynamics and Boson Condensation of Evanescent Photons. Int. J. Mod. Phys. 1996, 10, 1735–1754. [Google Scholar] [CrossRef]
- Jibu, M.; Yasue, K. Quantum Measurement by Quantum Brain. In Stochasticity and Quantum Chaos, Proceedings of the 3rd Max Born Symposium, Sobótka Castle, Poland, 15–17 September 1993; Haba, Z., Cegła, W., Jakóbczyk, L., Eds.; Springer: Dordrecht, The Netherlands, 1995; pp. 185–194. [Google Scholar] [CrossRef]
- Yao, Y.; Xiao, X.; Ge, L.; Sun, C.P. Quantum coherence in multipartite systems. Phys. Rev. 2015, 92, 022112. [Google Scholar] [CrossRef]
- Tan, K.C.; Kwon, H.; Park, C.Y.; Jeong, H. Unified view of quantum correlations and quantum coherence. Phys. Rev. 2016, 94, 022329. [Google Scholar] [CrossRef]
- Ma, J.; Yadin, B.; Girolami, D.; Vedral, V.; Gu, M. Converting Coherence to Quantum Correlations. Phys. Rev. Lett. 2016, 116, 160407. [Google Scholar] [CrossRef]
- Marletto, C.; Vedral, V. Gravitationally induced entanglement between two massive particles is sufficient evidence of quantum effects in gravity. Phys. Rev. Lett. 2017, 119, 240402. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Morley, G.W.; Ulbricht, H.; Toroš, M.; Paternostro, M.; Geraci, A.A.; Barker, P.F.; Kim, M.S.; Milburn, G. Spin entanglement witness for quantum gravity. Phys. Rev. Lett. 2017, 119, 240401. [Google Scholar] [CrossRef] [PubMed]
- Kumar, A.; Lim, Y.K.; Arumugam, P.; Złośnik, T.; Paterek, T. Probing modified gravity with entanglement of microspheres. Phys. Rev. 2024, 109, L101501. [Google Scholar] [CrossRef]
- Izadyari, M.; Pusuluk, O.; Sinha, K.; Müstecaplıoğlu, O. Steady-state entanglement generation via Casimir-Polder interactions. arXiv 2024, arXiv:2406.02270. [Google Scholar]
- Weingarten, C.P.; Doraiswamy, P.M.; Fisher, M. A new spin on neural processing: Quantum cognition. Front. Hum. Neurosci. 2016, 10, 218250. [Google Scholar] [CrossRef]
- Straub, J.S.; Nowotarski, M.S.; Lu, J.; Sheth, T.; Jiao, S.; Fisher, M.P.; Shell, M.S.; Helgeson, M.E.; Jerschow, A.; Han, S. Phosphates form spectroscopically dark state assemblies in common aqueous solutions. Proc. Natl. Acad. Sci. USA 2023, 120, e2206765120. [Google Scholar] [CrossRef] [PubMed]
- Straub, J.S. The Quantum Brain: Explorations and Adventures With Posner Molecules; University of California: Santa Barbara, CA, USA, 2023. [Google Scholar]
- Habraken, W.J.; Tao, J.; Brylka, L.J.; Friedrich, H.; Bertinetti, L.; Schenk, A.S.; Verch, A.; Dmitrovic, V.; Bomans, P.H.; Frederik, P.M.; et al. Ion-association complexes unite classical and non-classical theories for the biomimetic nucleation of calcium phosphate. Nat. Commun. 2013, 4, 1507. [Google Scholar] [CrossRef] [PubMed]
- Deline, M.L.; Straub, J.; Patel, M.; Subba, P.; Grashei, M.; van Heijster, F.H.; Pirkwieser, P.; Somoza, V.; Livingstone, J.D.; Beazely, M.; et al. Lithium isotopes differentially modify mitochondrial amorphous calcium phosphate cluster size distribution and calcium capacity. Front. Physiol. 2023, 14, 1200119. [Google Scholar] [CrossRef]
- Deline, M.L.; Grashei, M.; van Heijster, F.H.; Schilling, F.; Straub, J.; Fromme, T. Adenylate kinase derived ATP shapes respiration and calcium storage of isolated mitochondria. Biochim. Biophys. Acta-(Bba)-Bioenerg. 2021, 1862, 148409. [Google Scholar] [CrossRef] [PubMed]
- Player, T.C.; Hore, P. Posner qubits: Spin dynamics of entangled Ca9(PO4)6 molecules and their role in neural processing. J. R. Soc. Interface 2018, 15, 20180494. [Google Scholar] [CrossRef]
- Adams, B.; Sinayskiy, I.; Agarwal, S.; Petruccione, F. Entanglement and coherence in pure and doped Posner molecules. arXiv 2023, arXiv:2310.13484. [Google Scholar]
- Agarwal, S.; Kattnig, D.R.; Aiello, C.D.; Banerjee, A.S. The Biological Qubit: Calcium Phosphate Dimers, Not Trimers. J. Phys. Chem. Lett. 2023, 14, 2518–2525. [Google Scholar] [CrossRef]
- Halpern, N.Y.; Crosson, E. Quantum information in the Posner model of quantum cognition. Ann. Phys. 2019, 407, 92–147. [Google Scholar] [CrossRef]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press on Demand: Oxford, UK, 2002. [Google Scholar]
- Cattaneo, M.; Giorgi, G.L.; Maniscalco, S.; Zambrini, R. Local versus global master equation with common and separate baths: Superiority of the global approach in partial secular approximation. New J. Phys. 2019, 21, 113045. [Google Scholar] [CrossRef]
- Hofer, P.P.; Perarnau-Llobet, M.; Miranda, L.D.M.; Haack, G.; Silva, R.; Brask, J.B.; Brunner, N. Markovian master equations for quantum thermal machines: Local versus global approach. New J. Phys. 2017, 19, 123037. [Google Scholar] [CrossRef]
- Trushechkin, A.S.; Volovich, I.V. Perturbative treatment of inter-site couplings in the local description of open quantum networks. Europhys. Lett. 2016, 113, 30005. [Google Scholar] [CrossRef]
- Benabdallah, F.; Zad, H.A.; Daoud, M.; Ananikian, N. Dynamics of quantum correlations in a qubit-qutrit spin system under random telegraph noise. Phys. Scr. 2021, 96, 125116. [Google Scholar] [CrossRef]
- Mazzola, L.; Maniscalco, S.; Piilo, J.; Suominen, K.A.; Garraway, B.M. Pseudomodes as an effective description of memory: Non-Markovian dynamics of two-state systems in structured reservoirs. Phys. Rev. A At. Mol. Opt. Phys. 2009, 80, 012104. [Google Scholar] [CrossRef]
- Garraway, B.M. Decay of an atom coupled strongly to a reservoir. Phys. Rev. 1997, 55, 4636. [Google Scholar] [CrossRef]
- Garraway, B. Nonperturbative decay of an atomic system in a cavity. Phys. Rev. 1997, 55, 2290. [Google Scholar] [CrossRef]
- Huang, P.; Zheng, H. Quantum dynamics of a qubit coupled with a structured bath. J. Phys. Condens. Matter 2008, 20, 395233. [Google Scholar] [CrossRef][Green Version]
- Deçordi, G.; Vidiella-Barranco, A. Two coupled qubits interacting with a thermal bath: A comparative study of different models. Opt. Commun. 2017, 387, 366–376. [Google Scholar] [CrossRef]
- Rebentrost, P.; Serban, I.; Schulte-Herbrüggen, T.; Wilhelm, F. Optimal control of a qubit coupled to a non-Markovian environment. Phys. Rev. Lett. 2009, 102, 090401. [Google Scholar] [CrossRef] [PubMed]
- Kofman, A.; Kurizki, G.; Sherman, B. Spontaneous and induced atomic decay in photonic band structures. J. Mod. Opt. 1994, 41, 353–384. [Google Scholar] [CrossRef]
- Naseem, M.T.; Müstecaplıoğlu, Ö.E. Ground-state cooling of mechanical resonators by quantum reservoir engineering. Commun. Phys. 2021, 4, 95. [Google Scholar] [CrossRef]
- Xue, S.; James, M.R.; Shabani, A.; Ugrinovskii, V.; Petersen, I.R. Quantum filter for a non-Markovian single qubit system. In Proceedings of the 2015 IEEE Conference on Control Applications (CCA), Sydney, NSW, Australia, 21–23 September 2015; pp. 19–23. [Google Scholar]
- Naseem, M.T.; Misra, A.; Müstecaplioğlu, Ö.E.; Kurizki, G. Minimal quantum heat manager boosted by bath spectral filtering. Phys. Rev. Res. 2020, 2, 033285. [Google Scholar] [CrossRef]
- El Allati, A.; El Anouz, K.; Chakour, M.B.A.; Al-Kuwari, S. Non-Markovian effects on the performance of a quantum Otto refrigerator. Phys. Lett. 2024, 496, 129316. [Google Scholar] [CrossRef]
- Wißmann, S.; Karlsson, A.; Laine, E.M.; Piilo, J.; Breuer, H.P. Optimal state pairs for non-Markovian quantum dynamics. Phys. Rev. A At. Mol. Opt. Phys. 2012, 86, 062108. [Google Scholar] [CrossRef]
- Swenson, R. A grand unified theory for the unification of physics, life, information and cognition (mind). Philos. Trans. R. Soc. 2023, 381, 20220277. [Google Scholar] [CrossRef] [PubMed]
- Görnitz, T. Quantum theory and the nature of consciousness. Found. Sci. 2018, 23, 475–510. [Google Scholar] [CrossRef]
- Zheltikov, A.M. The critique of quantum mind: Measurement, consciousness, delayed choice, and lost coherence. Phys. Uspekhi 2018, 61, 1016. [Google Scholar] [CrossRef]
- Josephson, B.D. The physics of mind and thought. Act. Nerv. Super. 2019, 61, 86–90. [Google Scholar] [CrossRef]
- Samarawickrama, M. Mathematical Modeling of Consciousness for Unifying Causation, Relativity and Quantum Mechanics. Proc. J. Phys. Conf. Ser. IOP Publ. 2024, 2701, 012051. [Google Scholar] [CrossRef]


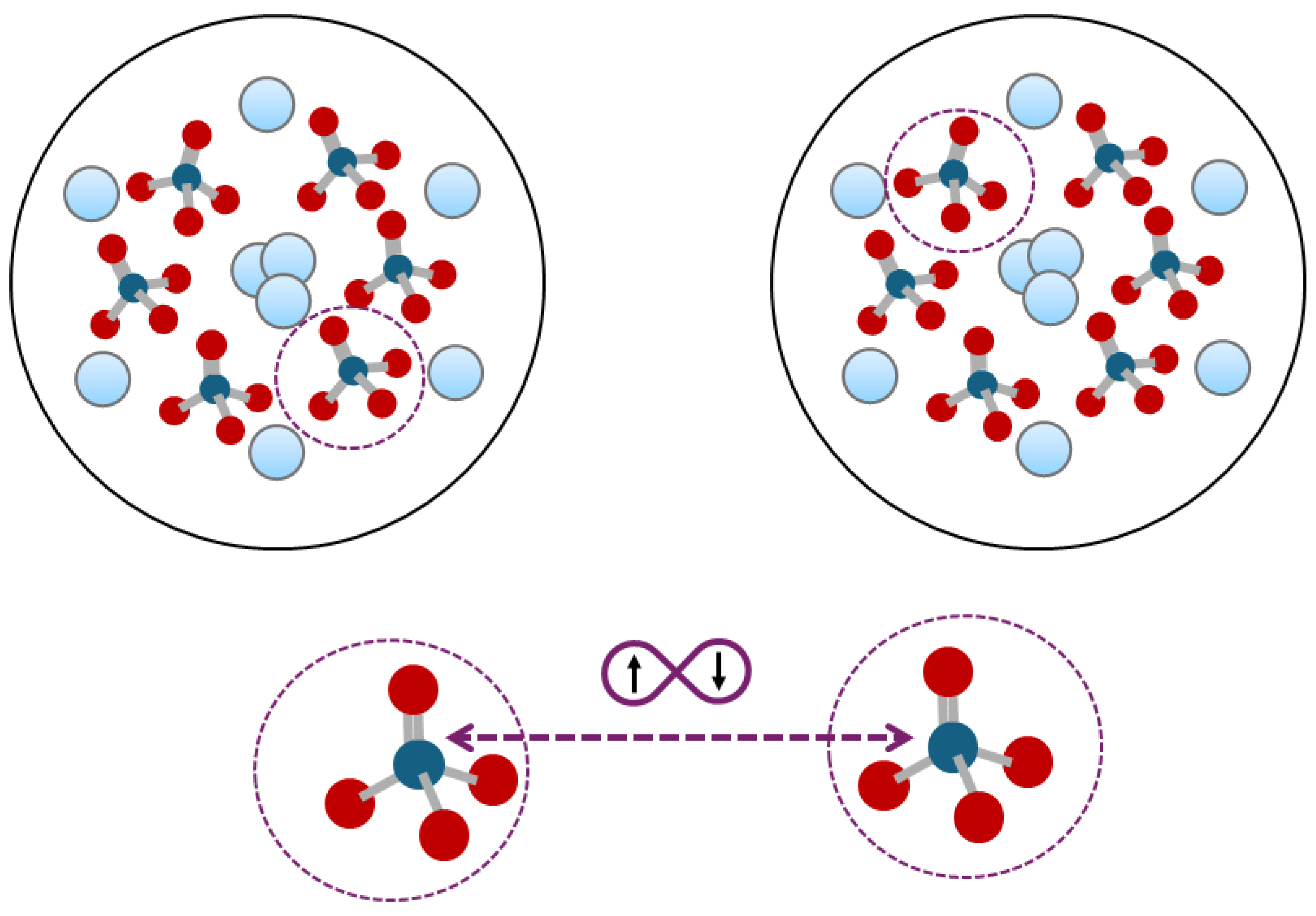
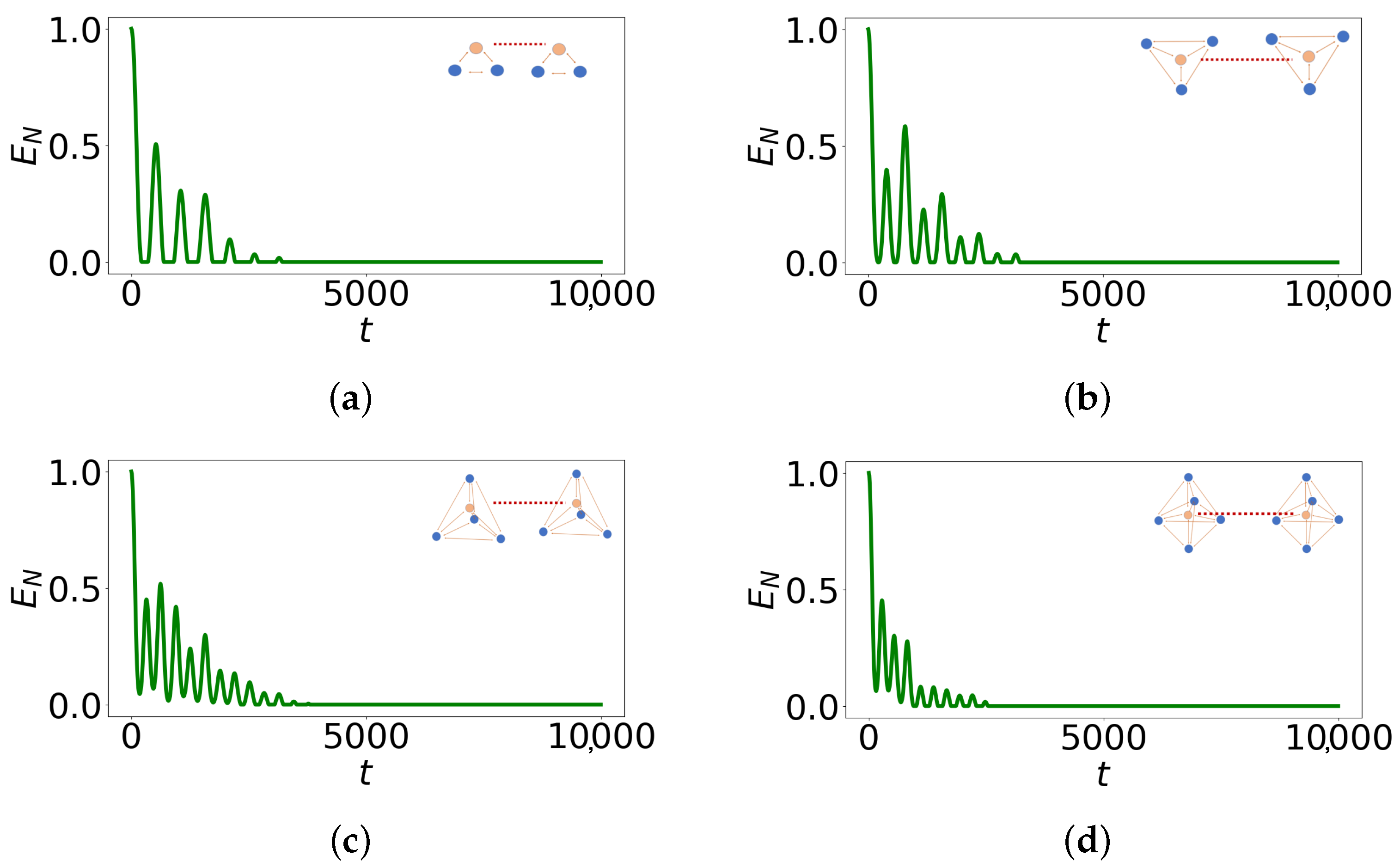

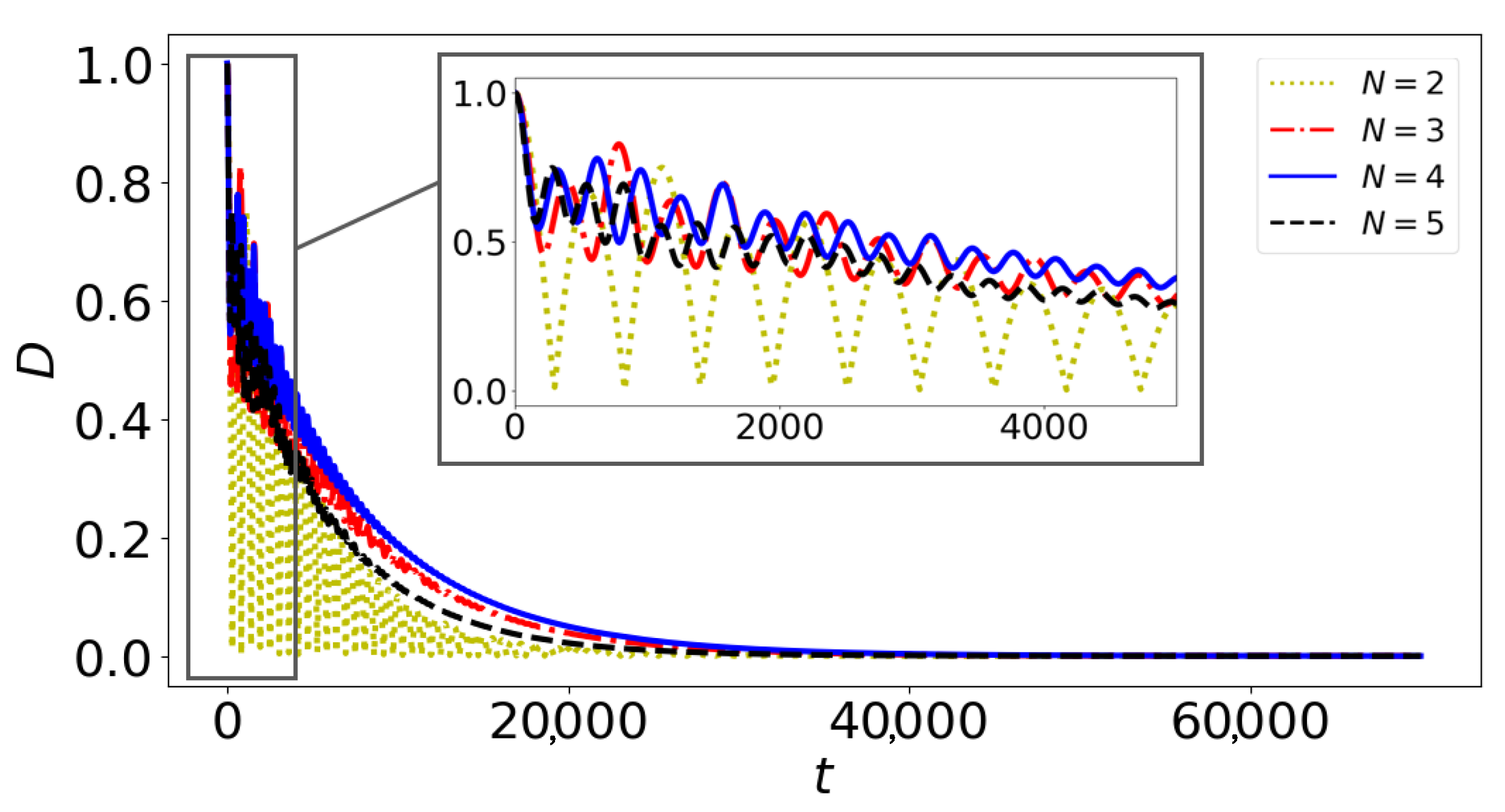
| 3 | 4 | 5 | 6 | |||||
|---|---|---|---|---|---|---|---|---|
| 2250 | 3210 | 2330 | 3230 | 1260 | 3800 | 1140 | 2540 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gassab, L.; Pusuluk, O.; Cattaneo, M.; Müstecaplıoğlu, Ö.E. Quantum Models of Consciousness from a Quantum Information Science Perspective. Entropy 2025, 27, 243. https://doi.org/10.3390/e27030243
Gassab L, Pusuluk O, Cattaneo M, Müstecaplıoğlu ÖE. Quantum Models of Consciousness from a Quantum Information Science Perspective. Entropy. 2025; 27(3):243. https://doi.org/10.3390/e27030243
Chicago/Turabian StyleGassab, Lea, Onur Pusuluk, Marco Cattaneo, and Özgür E. Müstecaplıoğlu. 2025. "Quantum Models of Consciousness from a Quantum Information Science Perspective" Entropy 27, no. 3: 243. https://doi.org/10.3390/e27030243
APA StyleGassab, L., Pusuluk, O., Cattaneo, M., & Müstecaplıoğlu, Ö. E. (2025). Quantum Models of Consciousness from a Quantum Information Science Perspective. Entropy, 27(3), 243. https://doi.org/10.3390/e27030243








