Thermodynamic Properties of Hydrogen Adsorbed on Graphite Surfaces at Temperatures Above 100 K: A Molecular Dynamics and Classical Density Functional Theory Study
Abstract
1. Introduction
2. Theory
2.1. Classical Density Functional Theory
2.2. Solid–Fluid Interaction
2.3. Adsorption
3. Methods
3.1. Molecular Dynamics Simulations
3.2. Locating the Dividing Surface
3.3. Adsorption Parameters
4. Results and Discussion
4.1. Location of the Dividing Surface
4.2. Adsorption
4.3. Thermodynamic Properties of Adsorbed Hydrogen
5. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Dillon, A.C.; Heben, M.J. Hydrogen storage using carbon adsorbents: Past, present and future. Appl. Phys. A Mater. Sci. Process. 2001, 72, 133–142. [Google Scholar] [CrossRef]
- Patchkovskii, S.; Tse, J.S.; Yurchenko, S.N.; Zhechkov, L.; Heine, T. Graphene nanostructures as tunable storage media for molecular hydrogen. Proc. Natl. Acad. Sci. USA 2005, 102, 10439–10444. [Google Scholar] [CrossRef] [PubMed]
- Schlapbach, L.; Züttel, A. Hydrogen-storage materials for mobile applications. Nature 2001, 414, 353–358. [Google Scholar] [CrossRef]
- Züttel, A. Hydrogen storage methods. Naturwissenschaften 2004, 91, 157–172. [Google Scholar] [CrossRef] [PubMed]
- Steele, B.C.H.; Heinzel, A. Materials for fuel-cell technologies. Nature 2001, 414, 345–352. [Google Scholar] [CrossRef]
- Balog, R.; Jørgensen, B.; Nilsson, L.; Andersen, M.; Rienks, E.; Bianchi, M.; Fanetti, M.; Lægsgaard, E.; Baraldi, A.; Lizzit, S.; et al. Bandgap opening in graphene induced by patterned hydrogen adsorption. Nat. Mater. 2010, 9, 315–319. [Google Scholar] [CrossRef]
- Popov, V. Carbon nanotubes: Properties and application. Mater. Sci. Eng. R Rep. 2004, 43, 61–102. [Google Scholar] [CrossRef]
- Kanashenko, S.; Gorodetsky, A.; Chernikov, V.; Markin, A.; Zakharov, A.; Doyle, B.; Wampler, W. Hydrogen adsorption on and solubility in graphites. J. Nucl. Mater. 1996, 233-237, 1207–1212. [Google Scholar] [CrossRef]
- Mattera, L.; Rosatelli, F.; Salvo, C.; Tommasini, F.; Valbusa, U.; Vidali, G. Selective adsorption of 1H2 and 2H2 on the (0001) graphite surface. Surf. Sci. 1980, 93, 515–525. [Google Scholar] [CrossRef]
- Gao, Y.; Li, Y.; Zhang, L.; Huang, H.; Hu, J.; Shah, S.M.; Su, X. Adsorption and removal of tetracycline antibiotics from aqueous solution by graphene oxide. J. Colloid Interface Sci. 2012, 368, 540–546. [Google Scholar] [CrossRef]
- Mills, G.; Jónsson, H.; Schenter, G.K. Reversible work transition state theory: Application to dissociative adsorption of hydrogen. Surf. Sci. 1995, 324, 305–337. [Google Scholar] [CrossRef]
- Yu, J.G.; Yu, L.Y.; Yang, H.; Liu, Q.; Chen, X.H.; Jiang, X.Y.; Chen, X.Q.; Jiao, F.P. Graphene nanosheets as novel adsorbents in adsorption, preconcentration and removal of gases, organic compounds and metal ions. Sci. Total Environ. 2015, 502, 70–79. [Google Scholar] [CrossRef]
- Ye, Z.; Chen, D.; Pan, Z.; Zhang, G.; Xia, Y.; Ding, X. An improved Langmuir model for evaluating methane adsorption capacity in shale under various pressures and temperatures. J. Nat. Gas Sci. Eng. 2016, 31, 658–680. [Google Scholar] [CrossRef]
- Kapoor, A.; Ritter, J.; Yang, R.T. An extended Langmuir model for adsorption of gas mixtures on heterogeneous surfaces. Langmuir 1990, 6, 660–664. [Google Scholar] [CrossRef]
- Kurniawan, A.; Sutiono, H.; Indraswati, N.; Ismadji, S. Removal of basic dyes in binary system by adsorption using rarasaponin–bentonite: Revisited of extended Langmuir model. Chem. Eng. J. 2012, 189-190, 264–274. [Google Scholar] [CrossRef]
- Zhang, P.; Wang, L. Extended Langmuir equation for correlating multilayer adsorption equilibrium data. Sep. Purif. Technol. 2010, 70, 367–371. [Google Scholar] [CrossRef]
- Ayawei, N.; Ebelegi, A.N.; Wankasi, D. Modelling and Interpretation of Adsorption Isotherms. J. Chem. 2017, 2017, 3039817. [Google Scholar] [CrossRef]
- Yao, C. Extended and improved Langmuir equation for correlating adsorption equilibrium data. Sep. Purif. Technol. 2000, 19, 237–242. [Google Scholar] [CrossRef]
- Langmuir, I. The adsorption of gases on plane surfaces of glass, mica and platinum. J. Am. Chem. Soc. 1918, 40, 1361–1403. [Google Scholar] [CrossRef]
- Simon, J.M.; Haas, O.E.; Kjelstrup, S. Adsorption and Desorption of H2 on Graphite by Molecular Dynamics Simulations. J. Phys. Chem. C 2010, 114, 10212–10220. [Google Scholar] [CrossRef]
- Trinh, T.; Bedeaux, D.; Simon, J.M.; Kjelstrup, S. Thermodynamic characterization of two layers of CO2 on a graphite surface. Chem. Phys. Lett. 2014, 612, 214–218. [Google Scholar] [CrossRef]
- Trinh, T.; Bedeaux, D.; Simon, J.M.; Kjelstrup, S. Calculation of the chemical potential and the activity coefficient of two layers of CO2 adsorbed on a graphite surface. Phys. Chem. Chem. Phys. 2015, 17, 1226–1233. [Google Scholar] [CrossRef] [PubMed]
- Kojima, Y. Hydrogen storage materials for hydrogen and energy carriers. Int. J. Hydrogen Energy 2019, 44, 18179–18192. [Google Scholar] [CrossRef]
- Gibbs, J.W. On the Equilibrium of Heterogeneous Substances; The Academy New Haven: New Haven, CT, USA, 1874. [Google Scholar]
- Bråten, V.; Zhang, D.T.; Hammer, M.; Aasen, A.; Schnell, S.K.; Wilhelmsen, Ø. Equation of state for confined fluids. J. Chem. Phys. 2022, 156, 244504. [Google Scholar] [CrossRef] [PubMed]
- Sauer, E.; Gross, J. Classical Density Functional Theory for Liquid–Fluid Interfaces and Confined Systems: A Functional for the Perturbed-Chain Polar Statistical Associating Fluid Theory Equation of State. Ind. Eng. Chem. Res. 2017, 56, 4119–4135. [Google Scholar] [CrossRef]
- Rehner, P. Interfacial Properties Using Classical Density Functional Theory: Curved Interfaces and Surfactants. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2021. [Google Scholar]
- Stierle, R.; Sauer, E.; Eller, J.; Theiss, M.; Rehner, P.; Ackermann, P.; Gross, J. Guide to efficient solution of PC-SAFT classical Density Functional Theory in various Coordinate Systems using fast Fourier and similar Transforms. Fluid Phase Equilibria 2020, 502, 112306. [Google Scholar] [CrossRef]
- Evans, R.; Oettel, M.; Roth, R.; Kahl, G. New developments in classical density functional theory. J. Phys. Condens. Matter 2016, 28, 240401. [Google Scholar] [CrossRef] [PubMed]
- Tarazona, P. Free-energy density functional for hard spheres. Phys. Rev. A 1985, 31, 2672–2679. [Google Scholar] [CrossRef]
- Aasen, A.; Jervell, V.G.; Hammer, M.; Strøm, B.A.; Skarsvåg, H.L.; Wilhelmsen, Ø. Bulk and interfacial thermodynamics of ammonia, water and their mixtures. Fluid Phase Equilibria 2024, 584, 114125. [Google Scholar] [CrossRef]
- Gross, J. A density functional theory for vapor-liquid interfaces using the PCP-SAFT equation of state. J. Chem. Phys. 2009, 131, 204705. [Google Scholar] [CrossRef]
- Rehner, P.; Gross, J. Multiobjective Optimization of PCP-SAFT Parameters for Water and Alcohols Using Surface Tension Data. J. Chem. Eng. Data 2020, 65, 5698–5707. [Google Scholar] [CrossRef]
- Sauer, E.; Gross, J. Prediction of Adsorption Isotherms and Selectivities: Comparison between Classical Density Functional Theory Based on the Perturbed-Chain Statistical Associating Fluid Theory Equation of State and Ideal Adsorbed Solution Theory. Langmuir 2019, 35, 11690–11701. [Google Scholar] [CrossRef]
- Aasen, A.; Blokhuis, E.M.; Wilhelmsen, Ø. Tolman lengths and rigidity constants of multicomponent fluids: Fundamental theory and numerical examples. J. Chem. Phys. 2018, 148, 204702. [Google Scholar] [CrossRef] [PubMed]
- Wilhelmsen, Ø.; Trinh, T.T.; Kjelstrup, S.; van Erp, T.S.; Bedeaux, D. Heat and mass transfer across interfaces in complex nanogeometries. Phys. Rev. Lett. 2015, 114, 065901. [Google Scholar] [CrossRef] [PubMed]
- Rehner, P.; Aasen, A.; Wilhelmsen, Ø. Tolman lengths and rigidity constants from free-energy functionals—General expressions and comparison of theories. J. Chem. Phys. 2019, 151, 244710. [Google Scholar] [CrossRef] [PubMed]
- Lafitte, T.; Apostolakou, A.; Avendaño, C.; Galindo, A.; Adjiman, C.S.; Müller, E.A.; Jackson, G. Accurate statistical associating fluid theory for chain molecules formed from Mie segments. J. Chem. Phys. 2013, 139, 154504. [Google Scholar] [CrossRef]
- Hammer, M.; Aasen, A.; Ervik, Å.; Wilhelmsen, Ø. Choice of reference, the influence of non-additivity and challenges in thermodynamic perturbation theory for mixtures. J. Chem. Phys. 2020, 152, 134106. [Google Scholar] [CrossRef]
- Roth, R. Introduction to Density Functional Theory of Classical Systems: Theory and Applications; Lecture Notes. 2006. Available online: https://bytebucket.org/knepley/cdft-git/wiki/papers/Lecture_Notes_on_DFT__Roland_Roth.pdf (accessed on 6 February 2025).
- Hammer, M.; Bauer, G.; Stierle, R.; Gross, J.; Wilhelmsen, Ø. Classical density functional theory for interfacial properties of hydrogen, helium, deuterium, neon, and their mixtures. J. Chem. Phys. 2023, 158, 104107. [Google Scholar] [CrossRef]
- NTNU Department of Chemistry. ThermoTools: SurfPack Open Source Surface- and Interfacial Properties. 2024. Available online: https://github.com/thermotools/SurfPack (accessed on 6 February 2025).
- Steele, W.A. The physical interaction of gases with crystalline solids: I. Gas-solid energies and properties of isolated adsorbed atoms. Surf. Sci. 1973, 36, 317–352. [Google Scholar] [CrossRef]
- Albesa, A.G.; Llanos, J.L.; Vicente, J. Comparative Study of Methane Adsorption on Graphite. Langmuir 2008, 24, 3836–3840. [Google Scholar] [CrossRef]
- Chang, C.K.; Tun, H.; Chen, C.C. An activity-based formulation for Langmuir adsorption isotherm. Adsorption 2020, 26, 375–386. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695–1697. [Google Scholar] [CrossRef]
- Trinh, T.T.; Vlugt, T.J.; Hägg, M.B.; Bedeaux, D.; Kjelstrup, S. Simulation of Pore Width and Pore Charge Effects on Selectivities of CO2 vs. H2 from a Syngas-like Mixture in Carbon Mesopores. Energy Procedia 2015, 64, 150–159. [Google Scholar] [CrossRef][Green Version]
- Mayo, S.L.; Olafson, B.D.; Goddard, W.A. DREIDING: A generic force field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar] [CrossRef]
- Aasen, A.; Hammer, M.; Ervik, Å.; Müller, E.A.; Wilhelmsen, Ø. Equation of state and force fields for Feynman–Hibbs-corrected Mie fluids. I. Application to pure helium, neon, hydrogen, and deuterium. J. Chem. Phys. 2019, 151, 064508. [Google Scholar] [CrossRef]
- Morgan, W. Thermal expansion coefficients of graphite crystals. Carbon 1972, 10, 73–79. [Google Scholar] [CrossRef]
- Aasen, A.; Hammer, M.; Müller, E.A.; Wilhelmsen, Ø. Equation of state and force fields for Feynman–Hibbs-corrected Mie fluids. II. Application to mixtures of helium, neon, hydrogen, and deuterium. J. Chem. Phys. 2020, 152, 074507. [Google Scholar] [CrossRef]
- Aasen, A.; Hammer, M.; Lasala, S.; Jaubert, J.N.; Wilhelmsen, Ø. Accurate quantum-corrected cubic equations of state for helium, neon, hydrogen, deuterium and their mixtures. Fluid Phase Equilibria 2020, 524, 112790. [Google Scholar] [CrossRef]
- van Westen, T.; Bauer, G.; Gross, J. Corresponding-states framework for classical and quantum fluids—Beyond Feynman–Hibbs. J. Chem. Phys. 2025, 162, 031101. [Google Scholar] [CrossRef]
- Wilhelmsen, Ø.; Aasen, A.; Skaugen, G.; Aursand, P.; Austegard, A.; Aursand, E.; Gjennestad, M.A.; Lund, H.; Linga, G.; Hammer, M. Thermodynamic Modeling with Equations of State: Present Challenges with Established Methods. Ind. Eng. Chem. Res. 2017, 56, 3503–3515. [Google Scholar] [CrossRef]
- SINTEF Energy Research/NTNU Department of Chemistry. ThermoTools: ThermoPack Open Source Thermodynamics Library. 2024. Available online: https://github.com/thermotools/thermopack (accessed on 6 February 2025).
- Benard, P.; Chahine, R. Determination of the adsorption isotherms of hydrogen on activated carbons above the critical temperature of the adsorbate over wide temperature and pressure ranges. Langmuir 2001, 17, 1950–1955. [Google Scholar] [CrossRef]
- Hirscher, M.; Panella, B. Nanostructures with high surface area for hydrogen storage. J. Alloys Compd. 2005, 404, 399–401. [Google Scholar] [CrossRef]
- Panella, B.; Hirscher, M.; Roth, S. Hydrogen adsorption in different carbon nanostructures. Carbon 2005, 43, 2209–2214. [Google Scholar] [CrossRef]
- Zhou, L.; Zhou, Y.; Sun, Y. Studies on the mechanism and capacity of hydrogen uptake by physisorption-based materials. Int. J. Hydrogen Energy 2006, 31, 259–264. [Google Scholar] [CrossRef]
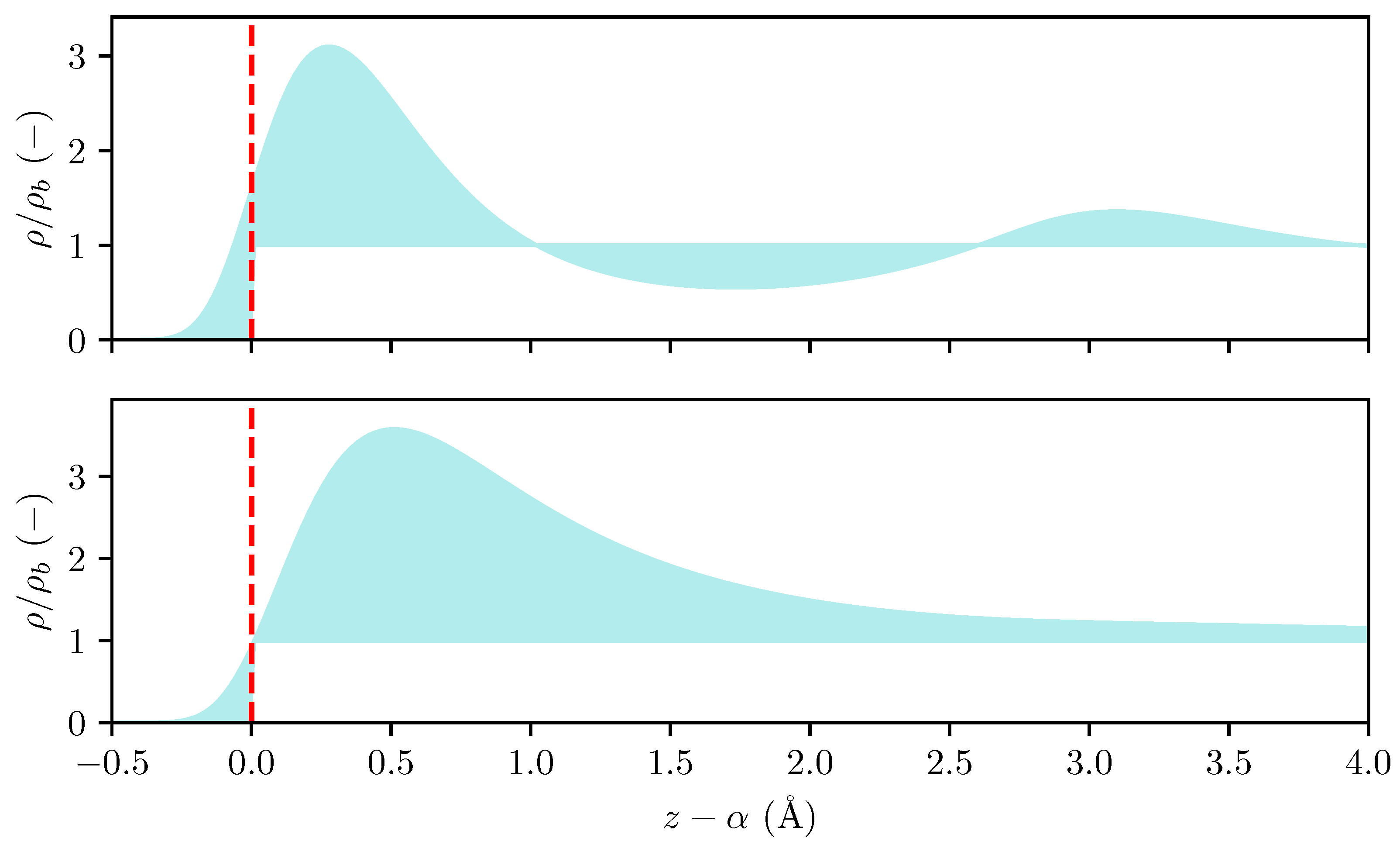

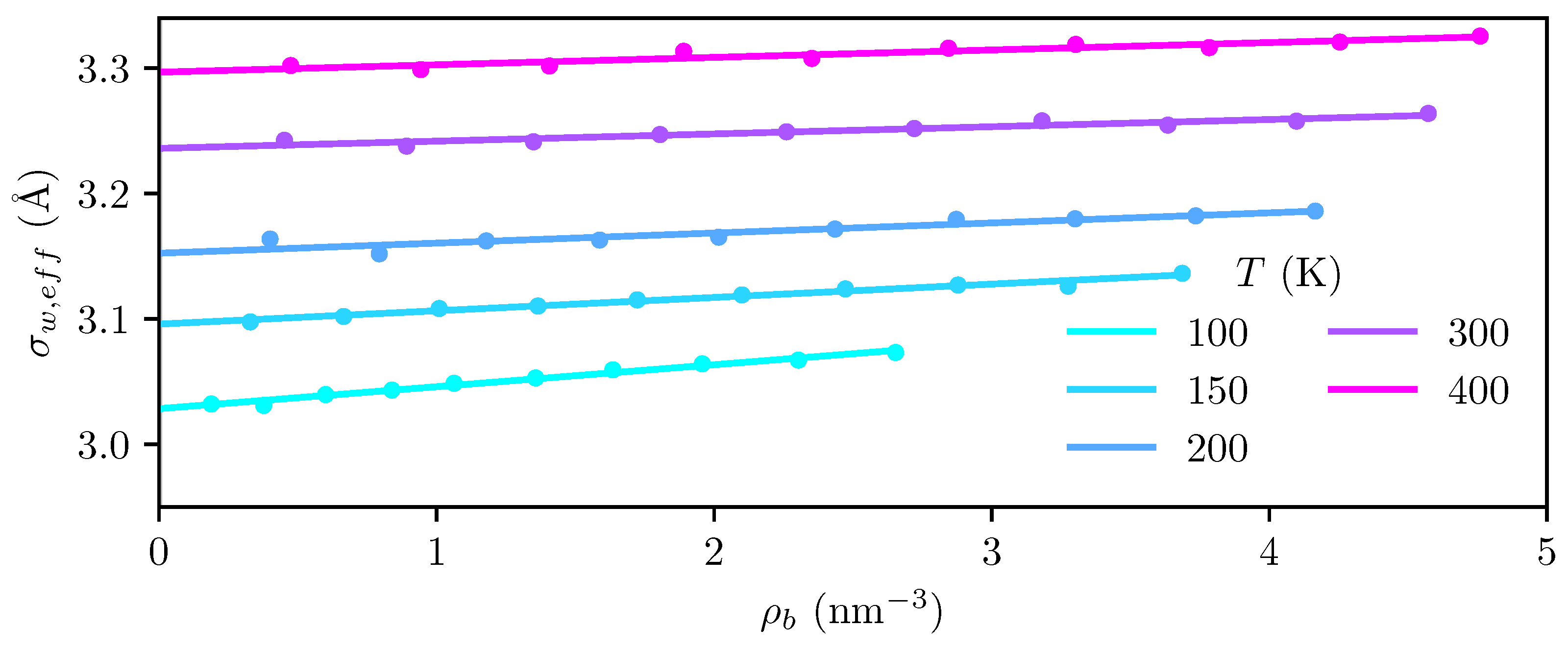

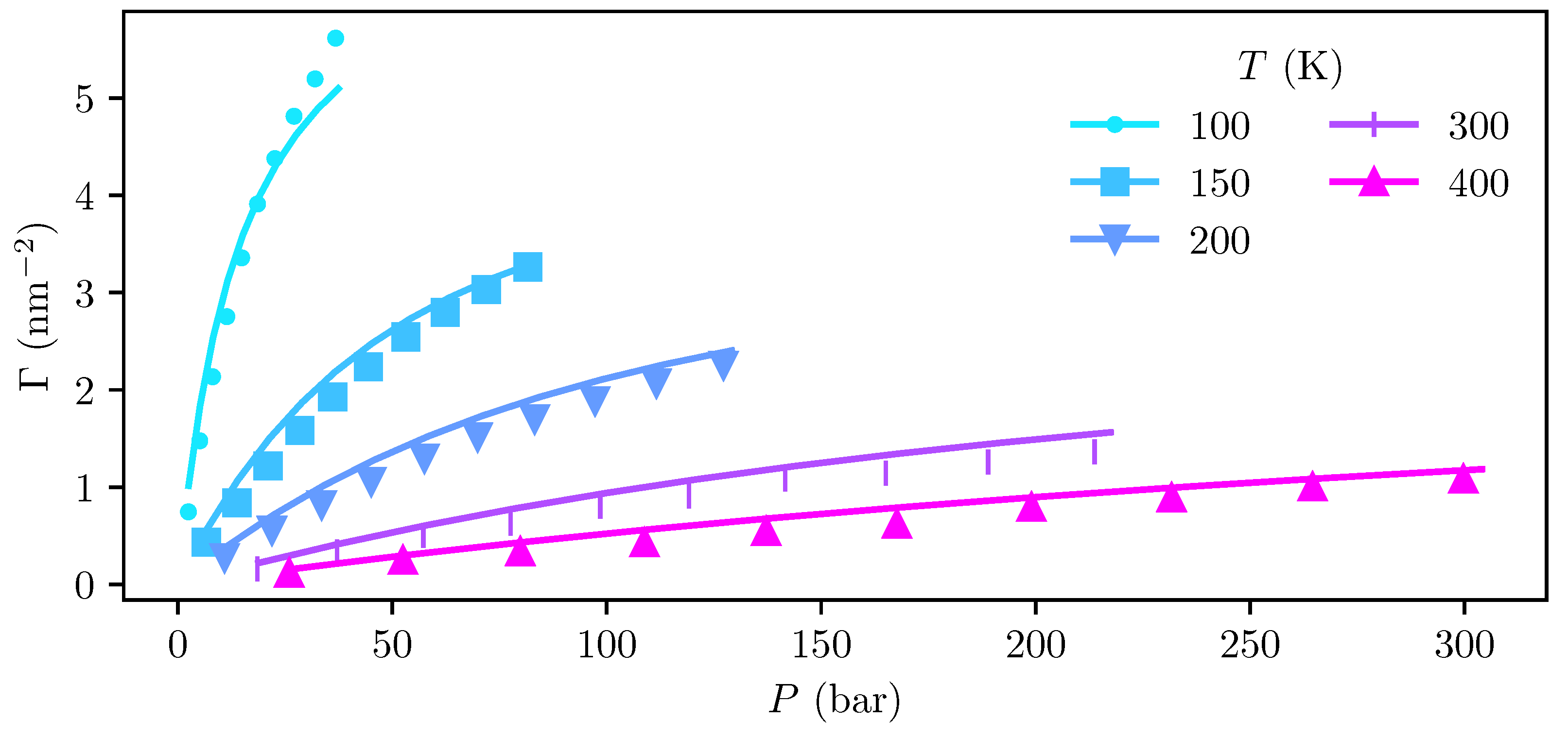
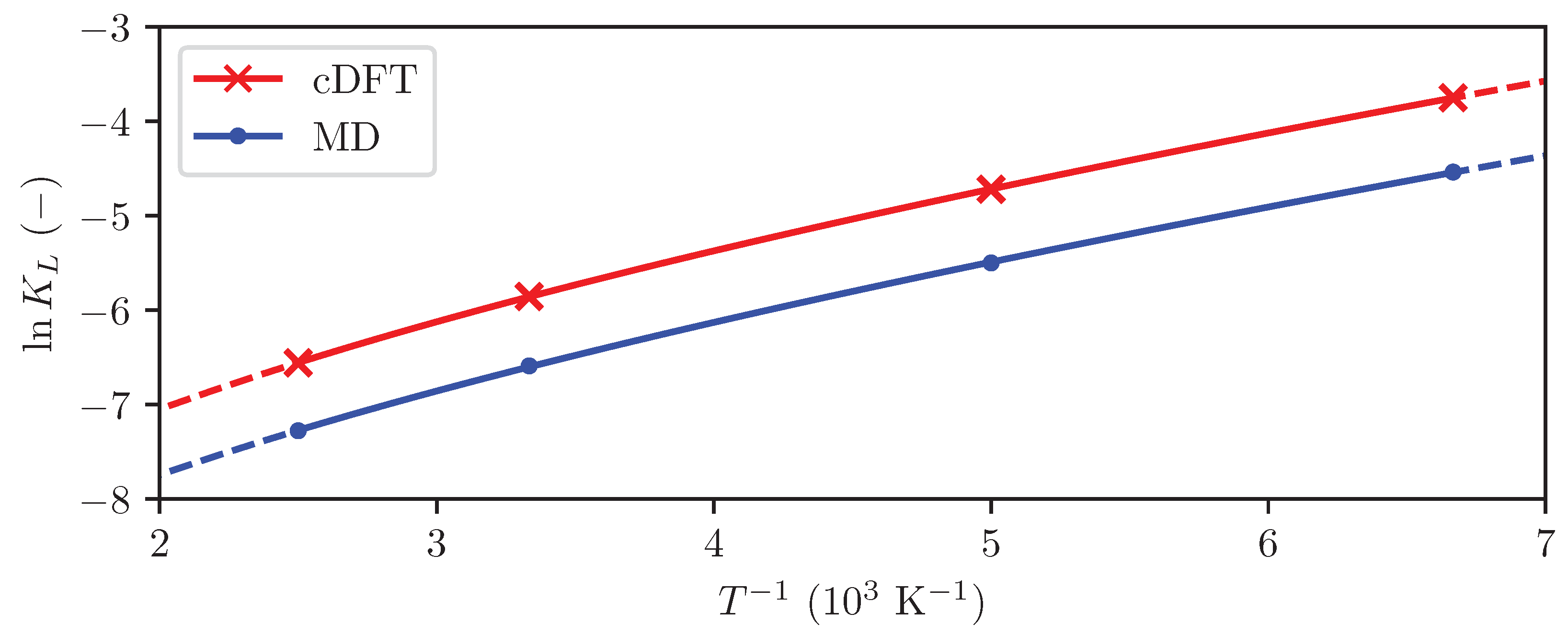
| Species | (Å) | () | ||
|---|---|---|---|---|
| H2 | 3.26 | 17.93 | 8 | 6 |
| Carbon | 3.33 | 26.0 | 12 | 6 |
| Graphite | Force constant | Equilibrium | ||
| () | position | |||
| Bond stretching | Å−2 | |||
| Angle bending | ||||
| Torsion | − | |||
| Isotherm | 150 K–400 K | 100 K | 150 K | 200 K | 300 K | 400 K | |
|---|---|---|---|---|---|---|---|
| cDFT | 7.10 | 12.04 | 9.99 | 8.32 | 6.36 | 5.36 | |
| (μmol m−2) | MD | 10.91 | 18.10 | 13.40 | 13.48 | 10.80 | 7.97 |
| cDFT | − | − | 4.54 | 5.50 | 6.59 | 7.28 | |
| MD | − | − | 3.75 | 4.72 | 5.86 | 6.56 |
| Method | (298 K) (kJ mol−1) | (298 K) (J mol−1 K−1) | (J mol−1 K−1) |
|---|---|---|---|
| cDFT | |||
| MD |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jervell, V.G.; Hammer, M.; Wilhelmsen, Ø.; Trinh, T.T. Thermodynamic Properties of Hydrogen Adsorbed on Graphite Surfaces at Temperatures Above 100 K: A Molecular Dynamics and Classical Density Functional Theory Study. Entropy 2025, 27, 184. https://doi.org/10.3390/e27020184
Jervell VG, Hammer M, Wilhelmsen Ø, Trinh TT. Thermodynamic Properties of Hydrogen Adsorbed on Graphite Surfaces at Temperatures Above 100 K: A Molecular Dynamics and Classical Density Functional Theory Study. Entropy. 2025; 27(2):184. https://doi.org/10.3390/e27020184
Chicago/Turabian StyleJervell, Vegard G., Morten Hammer, Øivind Wilhelmsen, and Thuat T. Trinh. 2025. "Thermodynamic Properties of Hydrogen Adsorbed on Graphite Surfaces at Temperatures Above 100 K: A Molecular Dynamics and Classical Density Functional Theory Study" Entropy 27, no. 2: 184. https://doi.org/10.3390/e27020184
APA StyleJervell, V. G., Hammer, M., Wilhelmsen, Ø., & Trinh, T. T. (2025). Thermodynamic Properties of Hydrogen Adsorbed on Graphite Surfaces at Temperatures Above 100 K: A Molecular Dynamics and Classical Density Functional Theory Study. Entropy, 27(2), 184. https://doi.org/10.3390/e27020184







