Finite-Size Scaling in the Ageing Dynamics of the 1D Glauber–Ising Model †
Abstract
1. Introduction
- Slow dynamics (relaxations are slower than might be described by simple exponentials);
- Absence of time-translation invariance;
- Dynamical scaling.
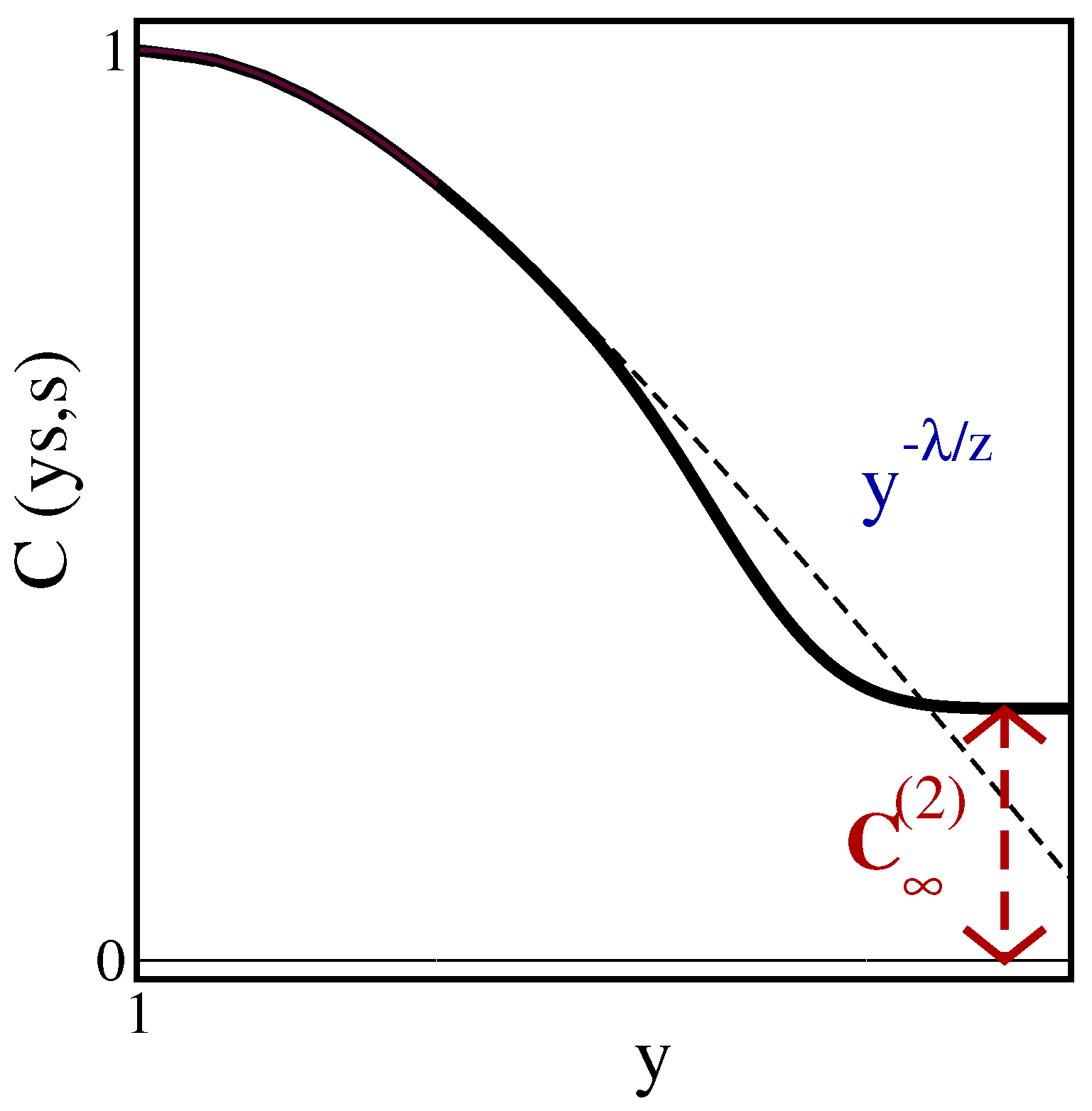
2. Critical Relaxations in Infinite-Size Systems
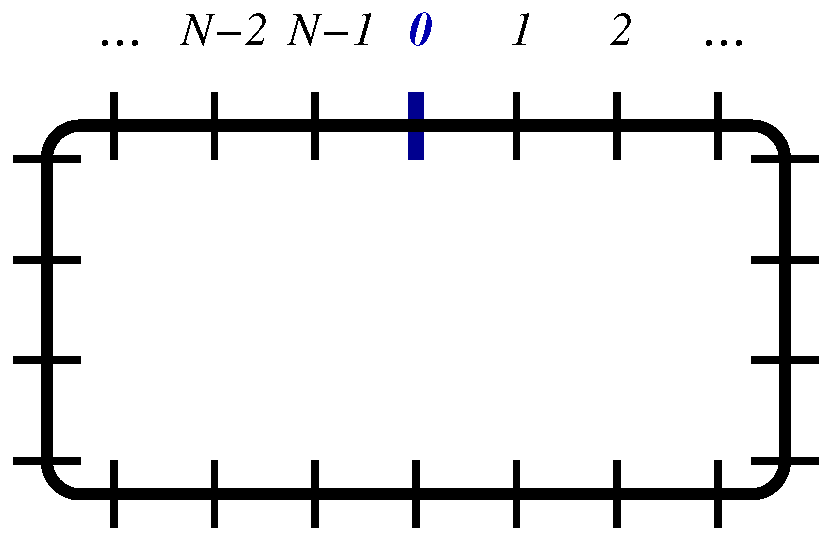
2.1. The Glauber–Ising Model
2.2. The Discrete Case
2.3. The Continuum Limit
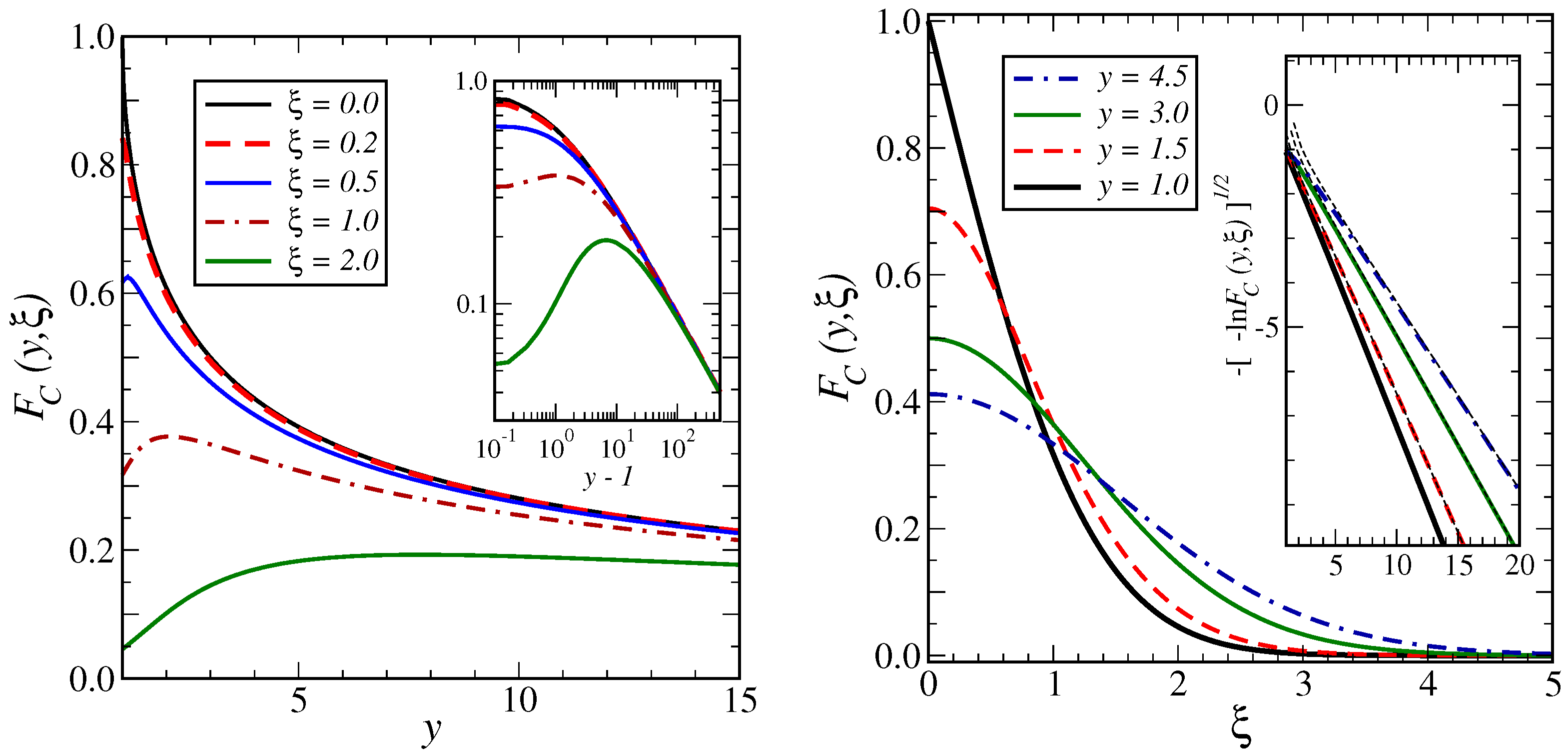
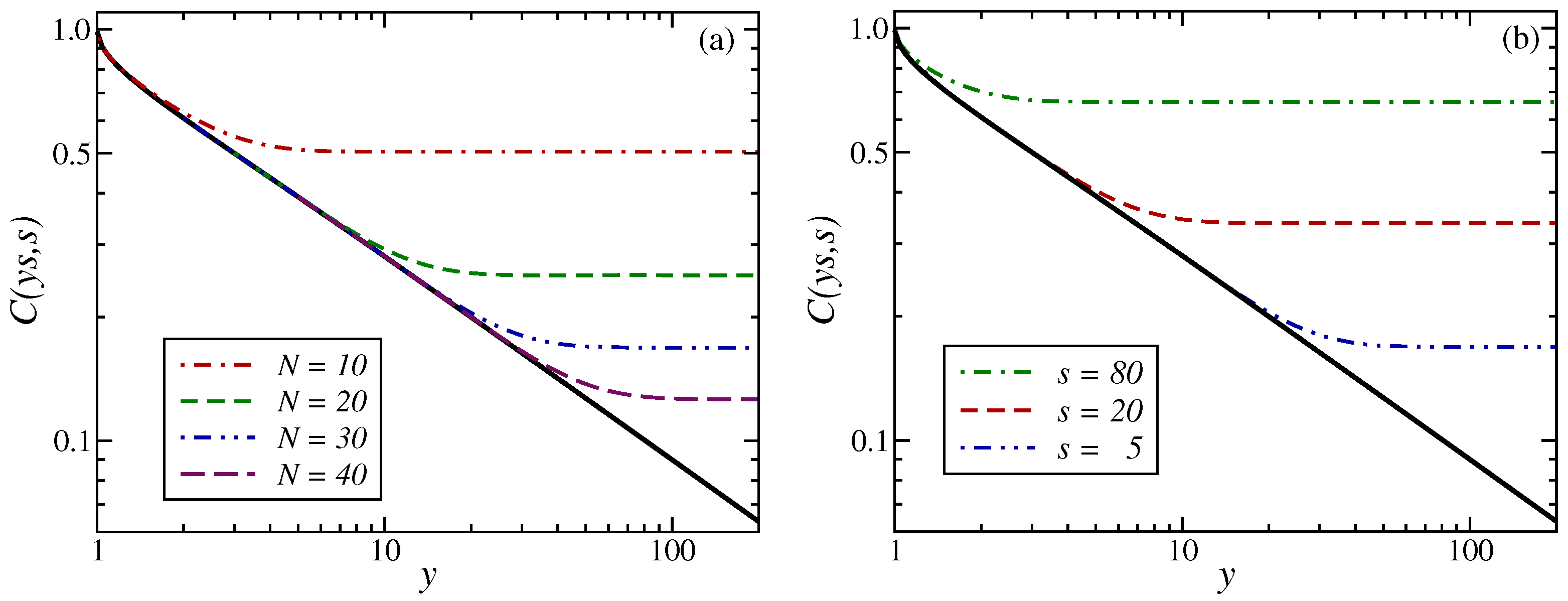
3. Critical Relaxations in Finite-Size Systems
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Analytical Derivations: Discrete Case
Appendix B. Analytical Derivations: Continuum Limit
Appendix C. Finite-Size Single-Time Correlator
Appendix D. Finite-Size Two-Time Correlator
Appendix E. On Humbert Function Identities
- Heuristic argument. We start from the integral representation (A32b) [77]. It turns out that the contributions of interest to us come from the upper limit of the integration, whereas the lower limit merely contributes terms of algebraic size, which can be discarded. Therefore, we may split the relevant integral , where is some constant to be fixed later. We concentrate on the second term, which reads
References
- Arceri, F.; Landes, F.P.; Berthier, L.; Biroli, G. A statistical mechanics perspective on glasses and ageing. In Encyclopedia of Complexity and Systems Science; Meyers, R.A., Ed.; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar] [CrossRef]
- Vincent, E. Encyclopedia of Condensed Matter Physics; Chakraborty, T., Ed.; Oxford University Press: Oxford, UK, 2024; Volume 2, pp. 371–387. [Google Scholar] [CrossRef]
- Struik, L.C.E. Physical Ageing in Amorphous Polymers and Other Materials; Elsevier: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Bray, A.J. Theory of Phase Ordering Kinetics. Adv. Phys. 1994, 43, 357. [Google Scholar] [CrossRef]
- Bray, A.J.; Rutenberg, A.D. Growth Laws for Phase Ordering. Phys. Rev. 1994, E49, R27. [Google Scholar] [CrossRef] [PubMed]
- Bray, A.J.; Rutenberg, A.D. The Energy-Scaling Approach to Phase-Ordering Growth Laws. Phys. Rev. 1994, E51, 5499. [Google Scholar] [CrossRef]
- Christiansen, H.; Majumder, S.; Janke, W. Phase-ordering kinetics of the long-range Ising model. Phys. Rev. 2019, E99, 011301. [Google Scholar] [CrossRef] [PubMed]
- Christiansen, H.; Majumder, S.; Henkel, M.; Janke, W. Ageing in the long-range Ising model. Phys. Rev. Lett. 2020, 125, 180601. [Google Scholar] [CrossRef]
- Corberi, F.; Lippiello, E.; Politi, P. One dimensional phase-ordering in the Ising model with space decaying interactions. J. Stat. Phys. 2019, 176, 510. [Google Scholar] [CrossRef]
- Corberi, F.; Lippiello, E.; Politi, P. Universality in the time correlations of the long-range 1d Ising model. J. Stat. Mech. 2019, 2019, 074002. [Google Scholar] [CrossRef]
- Corberi, F.; dello Russo, S.; Smaldone, L. Ordering kinetics with long-range interactions: Interpolating between voter and Ising models. J. Stat. Mech. 2024, 2024, 093206. [Google Scholar] [CrossRef]
- Müller, F.; Christiansen, H.; Janke, W. Non-universality in ageing during phase-separation of the two-dimensional long-range Ising model. Phys. Rev. Lett. 2024, 133, 237102. [Google Scholar] [CrossRef]
- Cugliandolo, L.F. Slow Relaxations and Non-Equilibrium Dynamics in Condensed Matter; Barrat, J.-L., Feiglman, M., Kurchan, J., Dalibard, J., Eds.; Les Houches LXXVII; Springer: Berlin/Heidelberg, Germany, 2003; pp. 367–521. [Google Scholar] [CrossRef]
- Henkel, M.; Pleimling, M. Non-Equilibrium Phase Transitions Vol. 2: Ageing and Dynamical Scaling Far from Equilibrium; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Puri, S.; Wadhawan, V. (Eds.) Kinetics of Phase Transitions; Taylor and Francis: London, UK, 2009. [Google Scholar]
- Täuber, U.C. Critical Dynamics: A Field-Theory Approach to Equilibrium and Non-Equilibrium Scaling Behaviour; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar] [CrossRef]
- Mason, N.; Pargellis, A.N.; Yurke, B. Scaling behavior of two-time correlations in a twisted nematic liquid crystal. Phys. Rev. Lett. 1993, 70, 190. [Google Scholar] [CrossRef]
- Almeida, R.; Takeuchi, K. Phase-ordering kinetics in the Allen-Cahn (Model A) class: Universal aspects elucidated by electrically-induced transition in liquid crystals. Phys. Rev. 2021, E104, 054103. [Google Scholar] [CrossRef]
- Henkel, M. Non-equilibrium relaxations: Ageing and finite-size effects. Condens. Matt. Phys. 2023, 26, 13501. [Google Scholar] [CrossRef]
- Joh, Y.G.; Orbach, R.; Wood, G.G.; Hammann, J.; Vincent, E. Finite-size effects on spin-glass dynamics. J. Phys. Soc. Jpn. 2004, 69 (Suppl. A), 215. [Google Scholar] [CrossRef]
- Kenning, G.G.; Tennant, D.M.; Rost, C.M.; Garrote da Silva, F.; Walters, B.J.; Zhai, Q.; Harrison, D.C.; Dahlberg, E.D.; Orbach, R.L. End of ageing as a probe of finite-size effects near the spin-glass transition temperature. Phys. Rev. 2018, B98. [Google Scholar] [CrossRef]
- Barber, M.N. Finite-size scaling. In Phase Transitions and Critical Phenomena; Domb, C., Lebowitz, J.L., Eds.; Academic Press: London, UK, 1983; Volume 8, p. 146. [Google Scholar]
- Brankov, J.G.; Danchev, D.M.; Tonchev, N.S. Theory of Critical Phenomena in Finite-Size Systems; World Scientific: Singapore, 2000. [Google Scholar]
- Brézin, É. An investigation of finite size scaling. J. Phys. 1982, 43, 15–22. [Google Scholar] [CrossRef]
- Fisher, M.E. Critical Phenomena, Proceedings of the 51st International School of Physics “Enrico Fermi”; Green, M.S., Ed.; Academic Press: London, UK, 1971; p. 1. [Google Scholar]
- Suzuki, M. Static and Dynamic Finite-Size Scaling Theory Based on the Renormalization Group Approach. Prog. Theor. Phys. 1977, 58, 1142. [Google Scholar] [CrossRef]
- Alcaraz, F.C.; Droz, M.; Henkel, M.; Rittenberg, V. Reaction-diffusion processes, critical dynamics and quantum chains. Ann. Phys. 1994, 230, 250. [Google Scholar] [CrossRef]
- Krebs, K.; Pfannmüller, M.P.; Wehefritz, B.; Hinrichsen, H. Finite-Size Scaling Studies of One-Dimensional Reaction-Diffusion Systems. Part I. Analytical Results. J. Stat. Phys. 1994, 78, 1429. [Google Scholar] [CrossRef]
- Krebs, K.; Pfannmüller, M.P.; Simon, H.; Wehefritz, B. Finite-Size Scaling Studies of One-Dimensional Reaction-Diffusion Systems. Part II. Numerical Methods. J. Stat. Phys. 1994, 78, 1471. [Google Scholar] [CrossRef]
- Barbier, D.; de Freitas Pimenta, P.H.; Cugliandolo, L.F.; Stariolo, D.A. Finite size effects and loss of self-averageness in the relaxational dynamics of the spherical Sherrington-Kirkpatrick model. J. Stat. Mech. 2021, arXiv:2103.12654. [Google Scholar] [CrossRef]
- Fernandez, L.A.; Marinari, E.; Martin-Mayor, V.; Paga, I.; Ruiz-Lorenzo, J.J. Dimensional cross-over in the ageing dynamics of spin glasses in a film geometry. Phys. Rev. 2019, B100, 184412. [Google Scholar] [CrossRef]
- Zamponi, N.; Zamponi, E.; Cannas, S.A.; Chialvo, D.R. Universal dynamics of mitochondrial networks: A finite-size scaling analysis. Sci. Rep. 2022, 12, 17074. [Google Scholar] [CrossRef] [PubMed]
- Zhai, Q.; Harrison, D.C.; Tennant, D.; Dahlberg, E.D.; Kenning, G.G.; Orbach, R.L. Glassy dynamics in CuMn thin-film multilayers. Phys. Rev. 2017, B95, 054304. [Google Scholar] [CrossRef]
- Zhai, Q.; Martin-Mayor, V.; Schlagel, D.L.; Kenning, G.G.; Orbach, R.L. On the Slowing Down of Spin Glass Correlation Length Growth: Simulations meet experiments. Phys. Rev. 2019, B100, 094202. [Google Scholar] [CrossRef]
- Christiansen, H.; Majumder, S.; Janke, W.; Henkel, M. Finite-size effects in ageing can be interpreted as sub-ageing. Phys. Rev. Lett. 2025. [Google Scholar] [CrossRef]
- Ionita, F.; Meyer-Ortmanns, H. Physical ageing of classical oscillators. Phys. Rev. Lett. 2014, 112, 094101. [Google Scholar] [CrossRef]
- Warkotsch, D.; Institute for Theoretical Physics, University of Leipzig, Leipzig, Germany; Henkel, M.; Groupe de Physique Statistique, Institut Jean Lamour, Universite de Lorraine Nancy, B.P. 70239, F-54506 Vandoeuvre-les-Nancy Cedex, France; Janke, W.; Institute for Theoretical Physics, University of Leipzig, Leipzig, Germany. Unpublished work. 2025. [Google Scholar]
- Henkel, M. Generalised time-translation-invariance in simple ageing. In Springer Proceedings in Mathematics and Statistics; Dobrev, V., Ed.; Springer: Berlin/Heidelberg, Germany, 2025; Volume 473. [Google Scholar]
- Berche, B.; Henkel, M.; Kenna, R. Critical phenomena: 150 years since Cagniard de la Tour. J. Phys. Stud. 2009, 13, 3201. [Google Scholar] [CrossRef]
- Henkel, M.; Schütz, G.M. On the universality of the fluctuation-dissipation ratio in non-equilibrium critical dynamics. J. Phys. 2004, A37, 591. [Google Scholar] [CrossRef]
- Glauber, R. Time-dependent statistics of the Ising model. J. Math. Phys. 1963, 4, 294. [Google Scholar] [CrossRef]
- Godrèche, C.; Luck, J.-M. Response of non-equilibrium systems at criticality: Exact results for the Glauber-Ising chain. J. Phys. 2000, A33, 1151. [Google Scholar] [CrossRef]
- Lippiello, E.; Zannetti, M. Fluctuation dissipation ratio in the one-dimensional kinetic Ising model. Phys. Rev. 2000, E61, 3369. [Google Scholar] [CrossRef]
- Mayer, P.; Sollich, P. General solution for multispin two-time correlation and response functions in the Glauber-Ising chain. J. Phys. 2004, A37, 9. [Google Scholar] [CrossRef]
- Bray, A.J. Nonequilibrium Statistical Mechanics in One Dimension; Privman, V., Ed.; Cambridge University Press: Cambridge, UK, 1997; pp. 143–165. [Google Scholar]
- Aliev, M.A. Generating function of spin correlations functions for kinetic Glauber-Ising model with time-dependent transition rates. J. Math. Phys. 2009, 50, 083302. [Google Scholar] [CrossRef]
- Droz, M.; Rácz, Z.; Schmidt, J. One-dimensional kinetic Ising model with competing dynamics: Steady-state correlations and relaxation times. Phys. Rev. 1989, A39, 2141. [Google Scholar] [CrossRef] [PubMed]
- Felderhof, B.U. Spin relaxation in the Ising chain. Rep. Math. Phys. 1971, 1, 215, Erratum in Rep. Math. Phys. 1971, 2, 151. [Google Scholar] [CrossRef]
- Godrèche, C.; Luck, J.-M. The Glauber-Ising chain under low-temperature protocols. J. Phys. 2022, A55, 495001. [Google Scholar] [CrossRef]
- Krapivsky, P.L.; Redner, S.; Ben-Naim, E. A Kinetic View of Statistical Physics; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Mayer, P.; Berthier, L.; Garrahan, J.P.; Sollich, P. Fluctuation-dissipation relations in the non-equilibrium critical dynamics of Ising models. Phys. Rev. 2003, E68, 016116. [Google Scholar] [CrossRef]
- Mayer, P.; Sollich, P.; Berthier, L.; Garrahan, J.P. Dynamic heterogeneity in the Glauber-Ising chain. J. Stat. Mech. 2005, 2005, P05002. [Google Scholar] [CrossRef]
- Verley, G.; Chétrite, R.; Lacoste, D. Modified fluctuation-dissipation theorem near non-equilibrium states and applications to the Glauber-Ising chain. J. Stat. Mech. 2011. [Google Scholar] [CrossRef]
- Durang, X.; Fortin, J.-Y.; del Biondo, D.; Henkel, M.; Richert, J. Exact correlations in the one-dimensional coagulation-diffusion process by the empty-interval method. J. Stat. Mech. 2010, 2010, P04002. [Google Scholar] [CrossRef]
- Durang, X.; Fortin, J.-Y.; Henkel, M. Exact two-time correlation and response functions in the one-dimensional coagulation-diffusion process by the empty-interval-particle method. J. Stat. Mech. 2011, 2011, P02030. [Google Scholar] [CrossRef]
- Fortin, J.-Y. Crossover properties of a one-dimensional reaction-diffusion process with a transport current. J. Stat. Mech. 2010. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; Dover: New York, NY, USA, 1965. [Google Scholar]
- Prudnikov, A.P.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series, Vol. 3: More Special Functions; Gordon and Breach: New York, NY, USA, 1990. [Google Scholar]
- Srivastava, H.M.; Karlsson, P.W. Multiple Gaussian Hypergeometric Series; Ellis Horwood: Chichester, UK, 1985. [Google Scholar]
- Christiansen, H.; Majumder, S.; Janke, W. Coarsening and Aging of Lattice Polymers: Influence of Bond Fluctuations. J. Chem. Phys. 2017, 147, 094902. [Google Scholar] [CrossRef]
- Agrawal, R.; Corberi, F.; Lippiello, E.; Politi, P.; Puri, S. Kinetics of the Two-dimensional Long-range Ising Model at Low Temperatures. Phys. Rev. 2021, E103, 012108. [Google Scholar] [CrossRef]
- Agrawal, R.; Corberi, F.; Insalata, F.; Puri, S. Asymptotic States of Ising Ferromagnets with Long-range Interactions. Phys. Rev. 2022, E105, 034131. [Google Scholar] [CrossRef] [PubMed]
- Agrawal, R.; Corberi, F.; Lippiello, E.; Puri, S. Ordering dynamics of the random-field long-range Ising model in one dimension. Phys. Rev. 2023, E108, 044131. [Google Scholar] [CrossRef]
- Christiansen, H.; Majumder, S.; Janke, W. Zero-temperature coarsening in the two-dimensional long-range Ising model. Phys. Rev. 2021, E103, 052122. [Google Scholar] [CrossRef]
- Corberi, F.; Lippiello, E.; Zannetti, M. Scaling and universality in the aging kinetics of the two-dimensional clock model. Phys. Rev. 2006, E74, 04106. [Google Scholar] [CrossRef] [PubMed]
- Corberi, F.; Iannone, A.; Kumar, M.; Lippiello, E.; Politi, P. Coexistence of coarsening and mean-field relaxation in the long-range Ising chain. Sci. Post Phys. 2021, 10, 109. [Google Scholar] [CrossRef]
- Gessert, D.; Christiansen, H.; Janke, W. Ageing following a zero-temperature quench in the d=3 Ising model. Phys. Rev. 2024, E109, 044148. [Google Scholar] [CrossRef]
- Janke, W.; Christiansen, H.; Majumder, S. The role of magnetisation in phase-ordering kinetics of the short-range and long-range Ising model. Eur. Phys. J. ST 2023, 232, 1693. [Google Scholar] [CrossRef]
- Majumder, S.; Janke, W. Evidence of aging and dynamic scaling in the collapse of a polymer. Phys. Rev. 2016, E93, 032506. [Google Scholar] [CrossRef]
- Majumder, S.; Christiansen, H.; Janke, W. Understanding nonequilibrium scaling laws governing collapse of a polymer. Eur. Phys. J. 2020, 93, 142. [Google Scholar] [CrossRef]
- Prudnikov, A.P.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series, Vol. 2: Special Functions; Gordon and Breach: New York, NY, USA, 1986. [Google Scholar]
- Prudnikov, A.P.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series, Vol. 1: Elementary Functions; Gordon and Breach: New York, NY, USA, 1986. [Google Scholar]
- Itzykson, C.; Drouffe, J.-M. Théorie Statistique des Champs; CNRS: Paris, France, 1989; Volume 2. [Google Scholar]
- Hang, P.-C.; Luo, M.-J. Note on the Humbert function Ψ1. arXiv 2024, arXiv:2410.21985. [Google Scholar] [CrossRef]
- Hang, P.-C.; Luo, M.-J. Asymptotics of the Humbert Function Ψ1 for Two Large Arguments. SIGMA 2024, 20, 074. [Google Scholar] [CrossRef]
- Hang, P.-C.; Luo, M.-J. Asymptotics of Saran’s hypergeometric function FK. J. Math. Anal. Appl. 2025, 541, 128707. [Google Scholar] [CrossRef]
- Wald, S.; Henkel, M. Lindblad dynamics of the quantum spherical model. Int. Transf. Spec. Funct. 2018, 29, 95. [Google Scholar] [CrossRef]
- Hang, P.-C.; Henkel, M.; Luo, M.-J. Asymptotics of the Humbert functions Ψ1 and Ψ2. J. Approx. Theory 2025, arXiv:2501.07281. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Henkel, M. Finite-Size Scaling in the Ageing Dynamics of the 1D Glauber–Ising Model. Entropy 2025, 27, 139. https://doi.org/10.3390/e27020139
Henkel M. Finite-Size Scaling in the Ageing Dynamics of the 1D Glauber–Ising Model. Entropy. 2025; 27(2):139. https://doi.org/10.3390/e27020139
Chicago/Turabian StyleHenkel, Malte. 2025. "Finite-Size Scaling in the Ageing Dynamics of the 1D Glauber–Ising Model" Entropy 27, no. 2: 139. https://doi.org/10.3390/e27020139
APA StyleHenkel, M. (2025). Finite-Size Scaling in the Ageing Dynamics of the 1D Glauber–Ising Model. Entropy, 27(2), 139. https://doi.org/10.3390/e27020139





